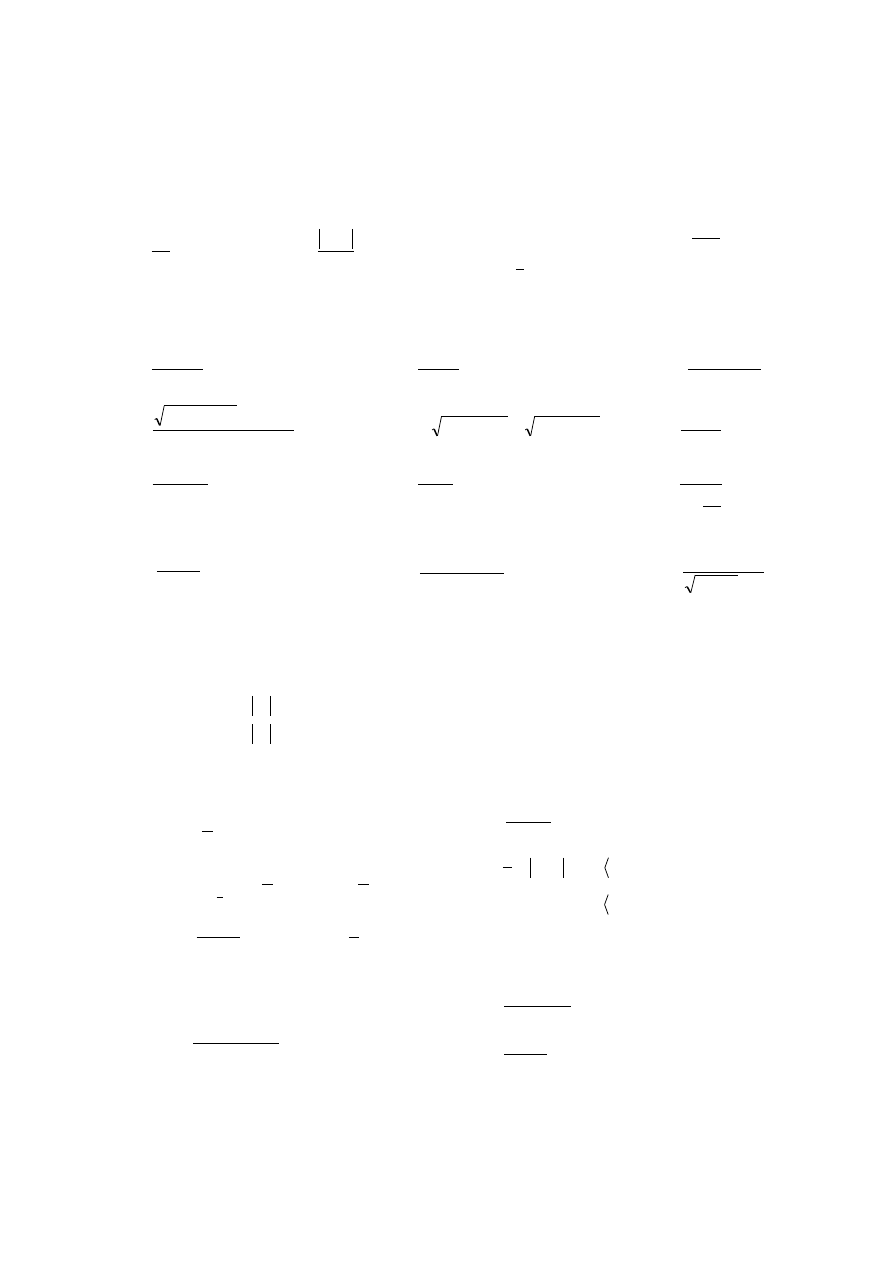
GRANICE FUNKCJI

Zad.1. Czy istnieją następujące granice funkcji?

a)

5

0

1

lim

x

x



b)























x

x

x

x

1

1

lim

1

c)

x

x

tg

lim

2





d)

16

1

4

2

3

lim





x

x

Zad.2. Obliczyć granice funkcji:

a)

5

25

lim

2

5







x

x

x

b)

2

6

1

1

1

lim

x

x

x







c)

1

2

1

2

lim

5

3

1













x

x

x

x

x

d)





x

x

x

x

x











1

2

1

lim

2

0

e)



























2

2

1

1

lim

x

x

x

x

x

f)

x

x

x

8

sin

5

sin

lim

0



g)

2

0

cos

1

lim

x

x

x





h)

x

x

x

7

2

tg

lim

0



i)

8

sin

8

lim

8

x

x

x







j)

2

2

1

lim

2

2

x

x

x

x





















k)

15

8

6

5

lim

2

2

3













x

x

x

x

x

l)

1

3

1

5

lim

0









x

x

tg

x

Zad.3. Zbadać ciągłość funkcji, określić rodzaj ewentualnych punktów nieciągłości:

a)

























2

4

2

4

2

2

)

(

2

x

x

x

x

x

f

b)









2

2

2

5

0

( )

2

0

4

log

12

4

x

x

f x

x

x

x

x

 









 











c)

1

2

5

2

1

1

2

0

2

( )

1

3

log

0

2

2

2

3

2

3

2

x

x

x

f x

x

x

x

x

 





 





  

 

  



 







 













 









d)























































,

2

2

,

1

1

3

1

1

,

2

2

)

(

2

2

2

x

x

x

x

x

x

x

x

w

e)

 

2

3

8

20

2

8

3

5

2

x

x

x

f x

x

x

x

 



 









 

 



f)

 











































3

9

ln

3

6

8

3

3

9

3

sin

2

2

x

x

x

x

x

x

x

x

f