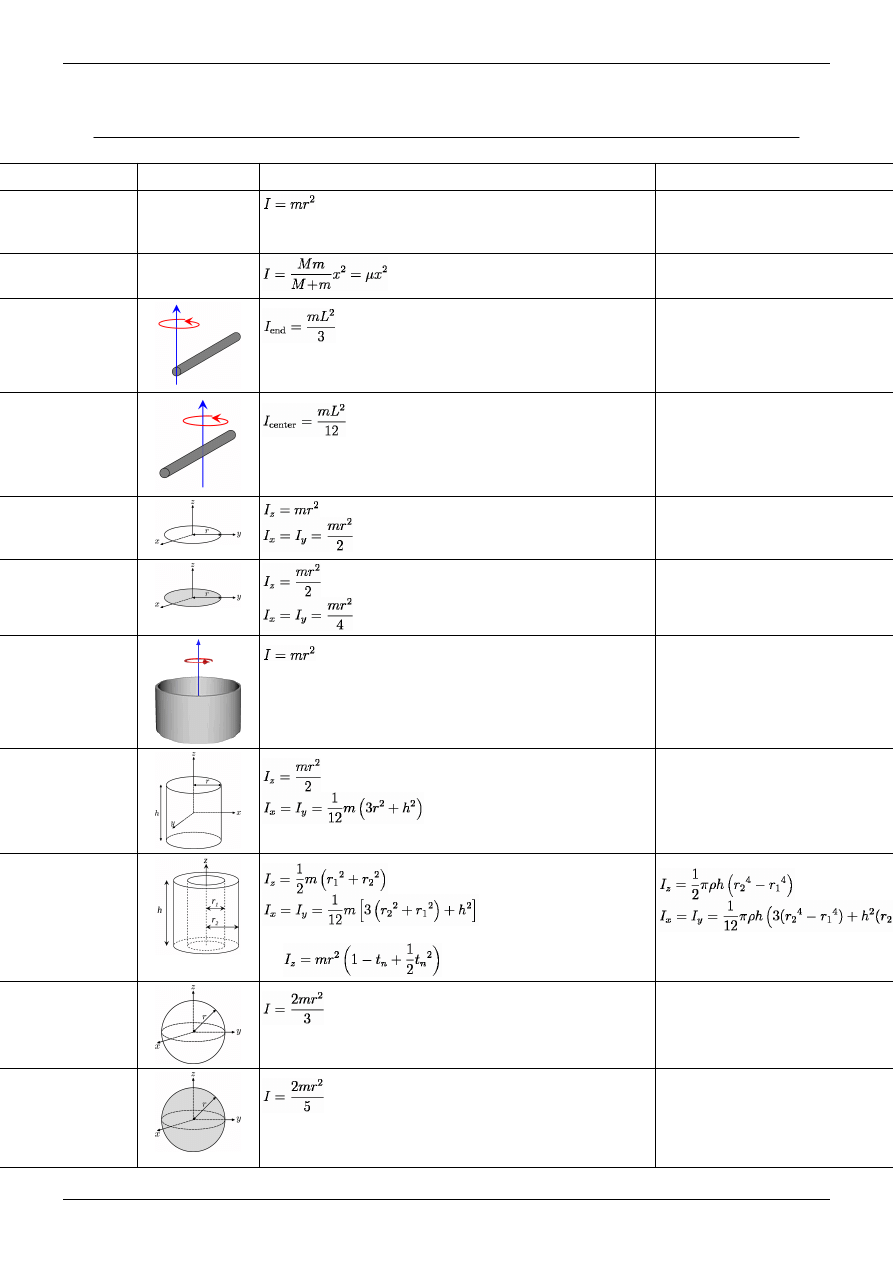
List of moments of inertia

1

List of moments of inertia

Description

Figure

Moment(s) of inertia

Comment

Point mass m at a distance r from the axis

of rotation.

A point mass does not have a moment of inertia around its own

axis, but by using the parallel axis theorem a moment of inertia

around a distant axis of rotation is achieved.

Two point masses, M and m, with reduced

mass

and separated by a distance, x.

—

Rod of length L and mass m

(Axis of rotation at the end of the rod)

  [1]

This expression assumes that the rod is an infinitely thin (but

rigid) wire. This is also a special case of the thin rectangular plate

with axis of rotation at the end of the plate, with h = L and w = 0.

Rod of length L and mass m

  [1]

This expression assumes that the rod is an infinitely thin (but

rigid) wire. This is a special case of the thin rectangular plate with

axis of rotation at the center of the plate, with w = L and h = 0.

Thin circular hoop of radius r and mass m

This is a special case of a torus for b=0. (See below.), as well as of

a thick-walled cylindrical tube with open ends, with r

1

=r

2

and

h=0.

Thin, solid disk of radius r and mass m

This is a special case of the solid cylinder, with h=0.

Thin cylindrical shell with open ends, of

radius r and mass m

  [1]

This expression assumes the shell thickness is negligible. It is a

special case of the thick-walled cylindrical tube for r

1

=r

2

. Also, a

point mass (m) at the end of a rod of length r has this same

moment of inertia and the value r is called the radius of gyration.

Solid cylinder of radius r, height h and

mass m

  [1]

This is a special case of the thick-walled cylindrical tube, with

r

1

=0. (Note: X-Y axis should be swapped for a standard right

handed frame)

Thick-walled cylindrical tube with open

ends, of inner radius r

1

, outer radius r

2

,

length h and mass m

  [1][2]

or when defining the normalized thickness t

n

 = t/r and letting r = r

2

,

then

With a density of ρ and the same geometry

Sphere (hollow) of radius r and mass m

  [1]

A hollow sphere can be taken to be made up of two stacks of

infinitesimally thin, circular hoops, where the radius differs from

0 to r (or a single stack, , where the radius differs from -r to r).

Ball (solid) of radius r and mass m

  [1]

A sphere can be taken to be made up of two stacks of

infinitesimally thin, solid discs, where the radius differs from 0 to

r (or a single stack, where the radius differs from -r to r). Also, it

can be taken to be made up of infinitesimally thin, hollow spheres,

where the radius differs from 0 to r.

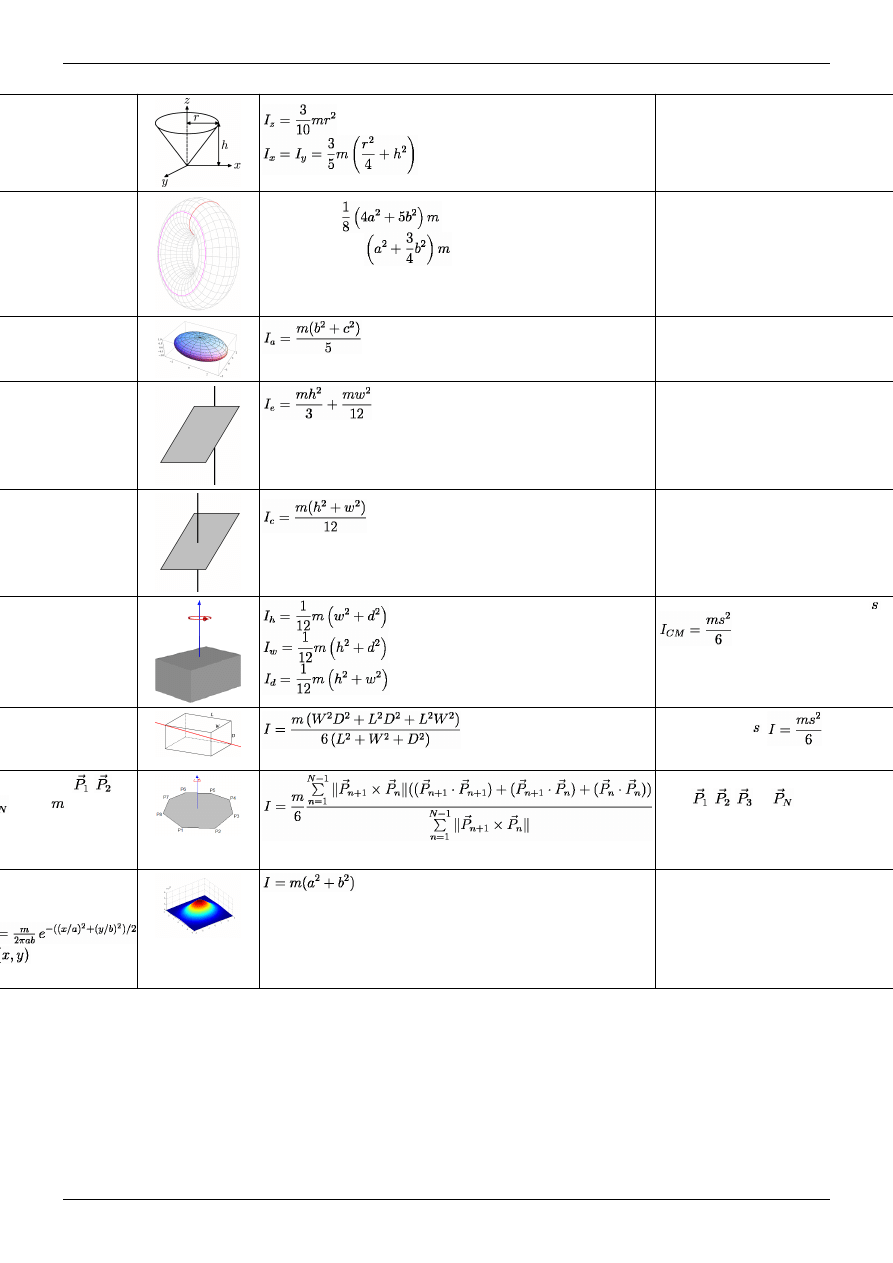
List of moments of inertia

2

Right circular cone with radius r, height h

and mass m

  [3]

  [3]

—

Torus of tube radius a, cross-sectional

radius b and mass m.

About a diameter:

  [4]

About the vertical axis:

  [4]

—

Ellipsoid (solid) of semiaxes a, b, and c

with axis of rotation a and mass m

—

Thin rectangular plate of height h and of

width w and mass m

(Axis of rotation at the end of the plate)

—

Thin rectangular plate of height h and of

width w and mass m

  [1]

—

Solid cuboid of height h, width w, and

depth d, and mass m

For a similarly oriented cube with sides of length

,

.

Solid cuboid of height D, width W, and

length L, and mass m with the longest

diagonal as the axis.

For a cube with sides

,

.

Plane polygon with vertices

,

,

, ...,

and mass

uniformly

distributed on its interior, rotating about an

axis perpendicular to the plane and passing

through the origin.

This expression assumes that the polygon is star-shaped. The

vectors

,

,

, ...,

are position vectors of the

vertices.

Infinite disk with mass normally

distributed on two axes around the axis of

rotation (i.e.

Where :

is the mass-density as a

function of x and y).

—

List of moments of inertia

3

References

[1] Raymond A. Serway (1986). Physics for Scientists and Engineers, second ed.. Saunders College Publishing. p. 202. ISBN 0-03-004534-7.

[2] Classical Mechanics - Moment of inertia of a uniform hollow cylinder (http:/

/

www.

livephysics.

com/

problems-and-answers/

classical-mechanics/

find-moment-of-inertia-of-a-uniform-hollow-cylinder.

html). LivePhysics.com. Retrieved on 2008-01-31.

[3] Ferdinand P. Beer and E. Russell Johnston, Jr (1984). Vector Mechanics for Engineers, fourth ed.. McGraw-Hill. p. 911.

ISBN 0-07-004389-2.

[4] Eric W. Weisstein. "Moment of Inertia — Ring" (http:/

/

scienceworld.

wolfram.

com/

physics/

MomentofInertiaRing.

html). Wolfram

Research. . Retrieved 2010-03-25.

Article Sources and Contributors

4

Article Sources and Contributors

List of moments of inertia  Source: http://en.wikipedia.org/w/index.php?oldid=521803613  Contributors: 11kravitzn, A. di M., A19grey, Annom, AquaDTRS, ArnoldReinhold, Ave matthew,
Baccyak4H, Bearasauras, Biscuittin, Bochum, Centrx, CiaPan, Copsi, CosineKitty, Ctrl build, Da Joe, Dexter411, Dger, Dinglebarf, Dudecon, Email4mobile, Ems57fcva, Fresheneesz, Gerweck,
Giftlite, Glome83, Grendelkhan, Hammerite, Hanavi, Headbomb, Hemmingsen, Herbee, Icairns, Icemaja, InverseHypercube, J-Wiki, JForget, Jeffcanfly, Joerger, Johnmc67, Just James,
KasugaHuang, Kevin B12, Kolkje, Krishnavedala, LPFR, Laurascudder, Masrudin, Mikiemike, Minesweeper, NOrbeck, Nick Number, Obiliz, Paste, Patrick, Pearlsawmeismyname, Prince Max
(scientist), Pt, Qef, Ram einstein, RedWolf, Scientific29, Segv11, Sonett72, Starwiz, Stpasha, Tagishsimon, TimBentley, Tresiden, Truthnlove, Zigger, 169 anonymous edits

Image Sources, Licenses and Contributors

Image:moment of inertia rod end.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_rod_end.svg  License: Creative Commons Zero  Contributors:
User:Krishnavedala

Image:moment of inertia rod center.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_rod_center.svg  License: Creative Commons Zero  Contributors:
User:Krishnavedala

Image:moment of inertia hoop.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_hoop.svg  License: GNU Free Documentation License  Contributors: Original
MetaPost program by en:User:Grendelkhan Program tweaked adjust axes labels to match bitmap version and to avoid negative coordinates in PostScript output and rendered as SVG by Qef

Image:moment of inertia disc.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_disc.svg  License: GNU Free Documentation License  Contributors: Original
MetaPost program by en:User:Grendelkhan Program tweaked adjust axes labels and fill in the grey disc to match bitmap version and to avoid negative coordinates in PostScript output and
rendered as SVG by Qef

Image:moment of inertia thin cylinder.png  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_thin_cylinder.png  License: Public Domain  Contributors:
http://www.vectorsite.net/gfxzmain.html

Image:moment of inertia solid cylinder.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_solid_cylinder.svg  License: GNU Free Documentation License
 Contributors: Original MetaPost program by en:User:Grendelkhan Program tweaked to avoid negative coordinates in PostScript output and rendered as SVG by Qef

Image:moment of inertia thick cylinder h.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_thick_cylinder_h.svg  License: Creative Commons Zero
 Contributors: User:Krishnavedala

Image:moment of inertia hollow sphere.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_hollow_sphere.svg  License: GNU Free Documentation License
 Contributors: Original MetaPost program by en:User:Grendelkhan Program tweaked adjust axes labels to match bitmap version and to avoid negative coordinates in PostScript output and
rendered as SVG by Qef

Image:moment of inertia solid sphere.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_solid_sphere.svg  License: GNU Free Documentation License
 Contributors: Original MetaPost program by en:User:Grendelkhan Program tweaked adjust axes labels and fill in the grey circle to match bitmap version and to avoid negative coordinates in
PostScript output and rendered as SVG by Qef

Image:moment of inertia cone.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_cone.svg  License: GNU Free Documentation License  Contributors: Original
MetaPost program by en:User:Grendelkhan Program tweaked to avoid negative coordinates in PostScript output and rendered as SVG by Qef Manually edited in inkscape to fixing intersection by
Wiso

Image:torus cycles.png  Source: http://en.wikipedia.org/w/index.php?title=File:Torus_cycles.png  License: GNU Free Documentation License  Contributors: User:Fropuff

Image:Ellipsoid 321.png  Source: http://en.wikipedia.org/w/index.php?title=File:Ellipsoid_321.png  License: Public domain  Contributors: Thrawn562 at en.wikipedia

Image:Recplaneoff.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Recplaneoff.svg  License: Public Domain  Contributors: User:Reubot

Image:Recplane.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Recplane.svg  License: Public Domain  Contributors: User:Reubot

Image:moment of inertia solid rectangular prism.png  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_inertia_solid_rectangular_prism.png  License: Public Domain
 Contributors: http://www.vectorsite.net/gfxzmain.html

Image:Moment of Inertia Cuboid.svg  Source: http://en.wikipedia.org/w/index.php?title=File:Moment_of_Inertia_Cuboid.svg  License: Creative Commons Zero  Contributors:
User:Krishnavedala

Image:Polygon moment of inertia.png  Source: http://en.wikipedia.org/w/index.php?title=File:Polygon_moment_of_inertia.png  License: Public Domain  Contributors: Glome83

File:Gaussian 2D.png  Source: http://en.wikipedia.org/w/index.php?title=File:Gaussian_2D.png  License: Public Domain  Contributors: User:Michael Hardy

License

Creative Commons Attribution-Share Alike 3.0 Unported
//creativecommons.org/licenses/by-sa/3.0/