April,2007 ME114

Descriptive Geometry Lecture
s

1

Introduction to Descriptive

Geometry

ME114

COMPUTER AIDED

ENGINEERING DRAWING II

April,2007 ME114

Descriptive Geometry Lecture
s

2

•Gaspard Monge(1746-1818), the
father of descriptive geometry,
developed a graphical protocol which
creates three-dimensional virtual
space on a two-dimensional plane.

•Monge became a scientific and
mathematical aide to Napoleon
during his reign as general and
emperor of France.

Descriptive Geometry

April,2007 ME114

Descriptive Geometry Lecture
s

3

Definitions(1)

Projective Geometry [1]
• The branch of

geometry

dealing with

the properties and invariants of
geometric figures under

projection

. In

older literature, projective geometry
is sometimes called "higher
geometry," "geometry of position," or
"descriptive geometry" (Cremona
1960, pp. v-vi).

April,2007 ME114

Descriptive Geometry Lecture
s

4

Definitions(2)

• Descriptive Geometry builds on the

practice, evolved over centuries, of
displaying two images of a single
object simultaneously; one image is
seen from one direction while a
second image is seen from a direction
90° rotated (e.g., a "front" and a "side"
view)[2].

April,2007 ME114

Descriptive Geometry Lecture
s

5

Definitions(3)

• Descriptive Geometry is a

graphical communication system,
concerned with describing space in a
mathematical way, so that the
geometrical objects and their
interaction can be imagined and
drawn[3].

April,2007 ME114

Descriptive Geometry Lecture
s

6

Definitions(4)

• Descriptive Geometry can be defined as

the projection of three-dimensional figures

onto a two-dimensional plane of paper in

such a manner as to allow geometric

manipulations to determine

• lengths,
• angles,
• shapes
• and other descriptive information
concerning the figures [8].

April,2007 ME114

Descriptive Geometry Lecture
s

7

Projection

• When representing a 3-D object on the 2-D

sheet of paper, the number of dimensions

is reduced from 3 to 2.

• The general process of reducing the

number of dimensions of a given object is
called

projection

[6]. Mainly, there are

two different ways of doing this. According

to the position of observer;

• Perpective and
• Parallel Projections.

April,2007 ME114

Descriptive Geometry Lecture
s

8

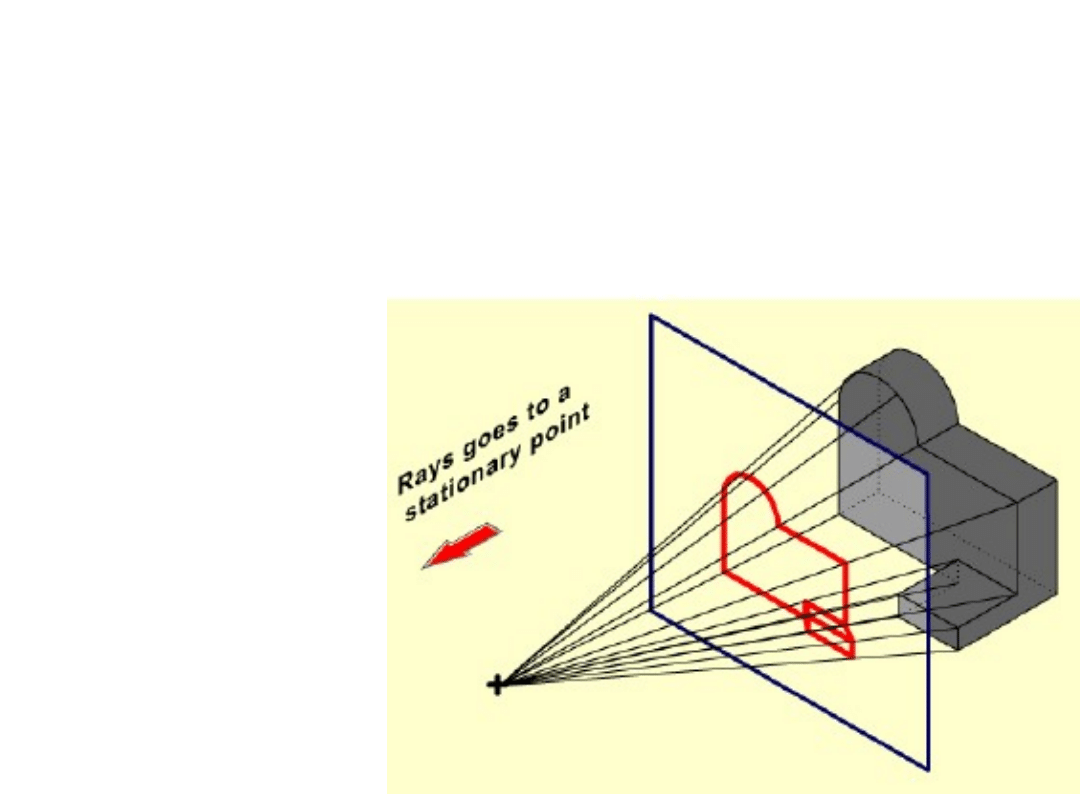
Perspective Projection

Perspective(Central) projection is a type of

drawing

, or

rendering, that graphically approximates on a planar (two-
dimensional) surface (e.g.

paper

or

painting canvas

) the images of

three-dimensional objects so as to approximate actual visual
perception [4].

The projection or
drawing upon the
plane is produced by
the points where
projectors pierce the
plane of projection
(piercing points). In
this case, where the
observer is relatively
close to the object,
the projectors form a
“cone” of projectors,
resulting projection is
known as a
perspective
projection[5].

April,2007 ME114

Descriptive Geometry Lecture
s

9

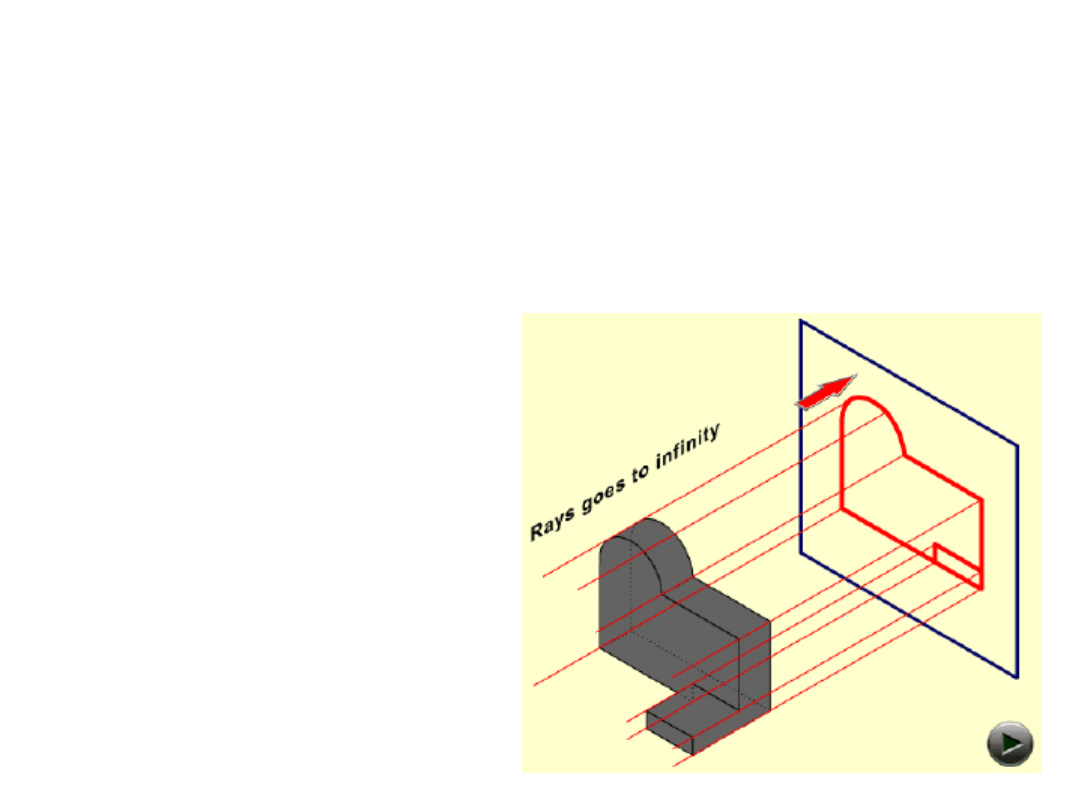
• Descriptive geometry uses the

image-creating technique of
imaginary, parallel projectors
emanating from an imaginary object
and intersecting an imaginary plane
of projection at right angles. The
cumulative points of intersections
create the desired image.

Parallel Projection

April,2007 ME114

Descriptive Geometry Lecture
s

10

•Descriptive Geometry is based on Parallel
Projection, in most cases parallel, orthogonal
projection [6].

Parallel Projection

In particular, an
orthogonal projection
of a three-dimensional
object onto a plane is
obtained by
intersections of lines
drawn through all
points of the object
orthogonally to the
plane of projection [7].

April,2007 ME114

Descriptive Geometry Lecture
s

11

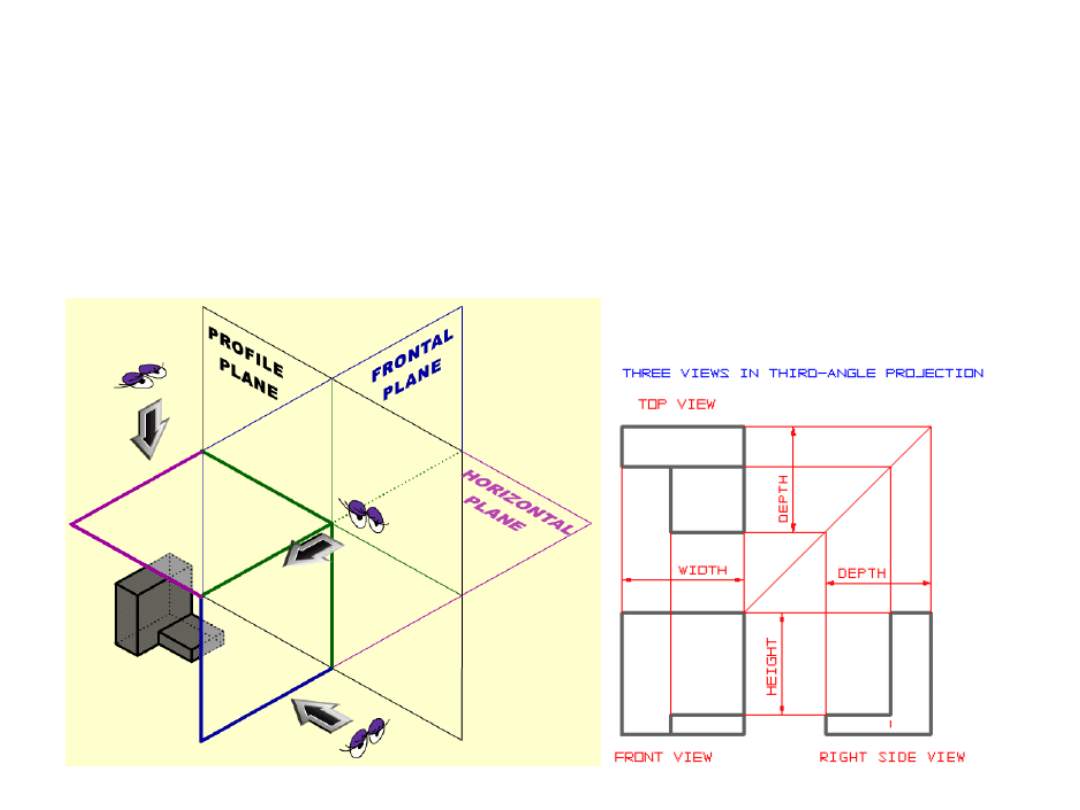
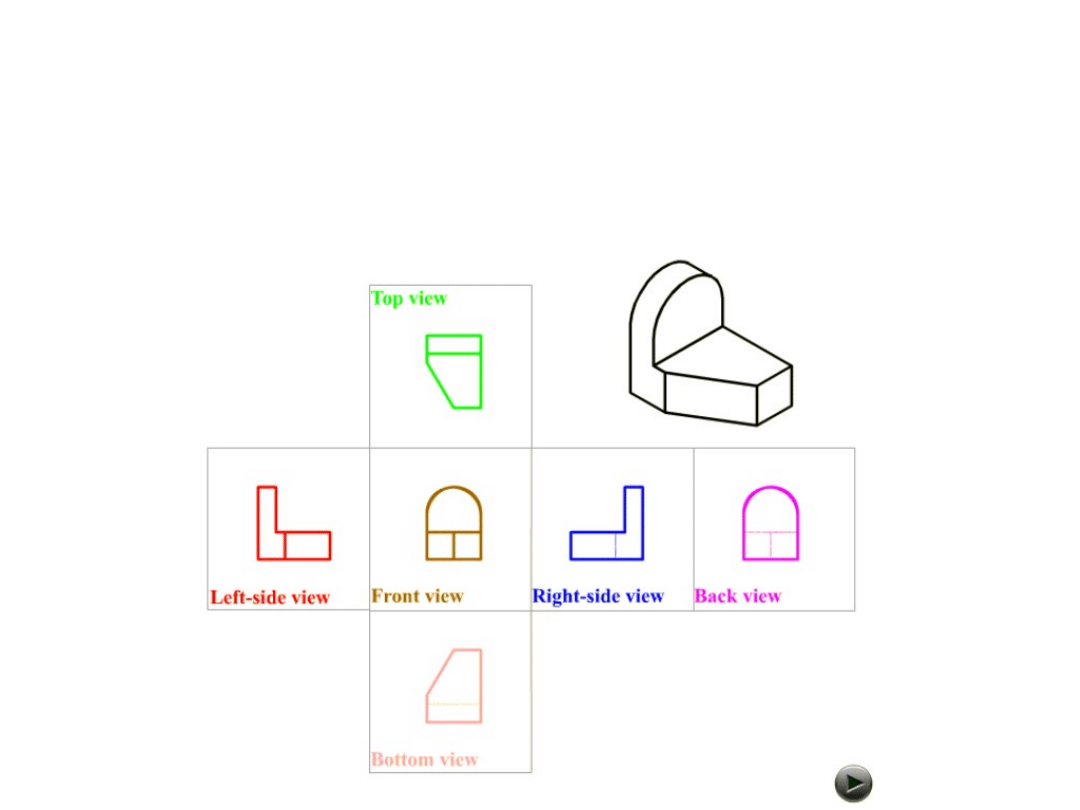
Orthographic projection

• Orthographic projection is a means of representing a three-

dimensional

(3D) object in two dimensions (2D). It uses multiple

views of the object, from points of view rotated about the object's
center through increments of 90°.

April,2007 ME114

Descriptive Geometry Lecture
s

12

Orthographic projection

• Equivalently, the views may be considered to be obtained by

rotating the object about its center through increments of 90°.

April,2007 ME114

Descriptive Geometry Lecture
s

13

Protocols(1)

• Project two images of an object into mutually

perpendicular, arbitrary directions. Each
image view accommodates three dimensions
of space, two dimensions displayed as full-
scale, mutually-perpendicular axes and one
as an invisible (point view) axis
receding(going back) into the image space
(depth). Each of the two adjacent image
views shares a full-scale view of one of the
three dimensions of space.

April,2007 ME114

Descriptive Geometry Lecture
s

14

Protocols(2)

• Either of these images may serve as

the beginning point for a third
projected view. The third view may
begin a fourth projection, and on ad
infinitum (continue forever). These
sequential projections each represent
a circuitous, 90° turn in space in
order to view the object from a
different direction.

April,2007 ME114

Descriptive Geometry Lecture
s

15

Protocols(3)

• Each new projection utilizes a

dimension in full scale that appears as
point-view dimension in the previous
view. To achieve the full-scale view of
this dimension and accommodate it
within the new view requires one to
ignore the previous view and proceed
to the second previous view where
this dimension appears in full-scale.

April,2007 ME114

Descriptive Geometry Lecture
s

16

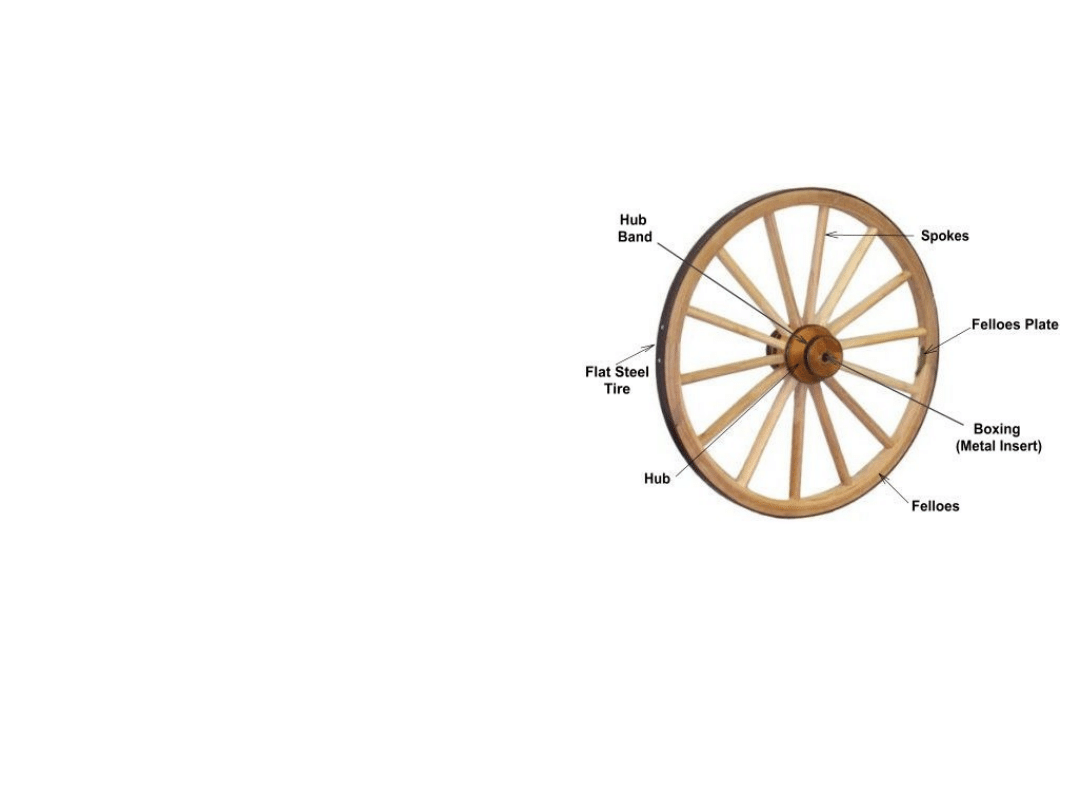
Protocols(4)

• Each new view may be

created by projecting into

any of an infinite number

of directions, perpendicular

to the previous direction of

projection. (Envision the

many directions of the

spokes of a wagon wheel

each perpendicular to the

direction of the axle.)

•The result is one of stepping circuitously about an

object in 90° turns and viewing the object from each

step. Each new view is added as an additional view to

an orthographic projection layout display and appears in

an "unfolding of the glass box model".

April,2007 ME114

Descriptive Geometry Lecture
s

17

Protocols(5)

• Aside from the Orthographic, six standard

principal views (Front; Right Side; Left

Side; Top; Bottom; Rear), descriptive

geometry strives to yield three basic

solution views:

• the true length of a line (i.e., full size, not

foreshortened),

• the point view (end view) of a line,
• and the true shape of a plane (i.e., full

size to scale, or not foreshortened).

April,2007 ME114

Descriptive Geometry Lecture
s

18

Protocols(5 cont’d)

• These often serve to determine the direction

of projection for the subsequent view. By

the 90° circuitous stepping process,

• projecting in any direction from the point

view of a line yields its true length view;

• projecting in a direction parallel to a true

length line view yields its point view,

• projecting the point view of any line on a

plane yields the plane's edge view;

• projecting in a direction perpendicular to the

edge view of a plane will yield the true

shape (to scale) view.

April,2007 ME114

Descriptive Geometry Lecture
s

19

Protocols(cont’d)

• These various views may be

called upon to help solve
engineering problems posed
by solid-geometry principles.

• It promotes visualization and spatial

analytical abilities, as well as the
intuitive ability to recognize the
direction of viewing for best presenting
a geometric problem for solution.

April,2007 ME114

Descriptive Geometry Lecture
s

20

Representative Examples(1)

• The best direction to view:

• Two

skew lines

(pipes, perhaps) in general

positions in order to determine the location of

their shortest connector (common

perpendicular)

• Two skew lines (pipes) in general positions

such that their shortest connector is seen in

full scale

• Two skew lines in general positions such the shortest

connector parallel to a given plane is seen in full scale

(say, to determine the position and the dimension of the

shortest connector at a constant distance from a
radiating surface)

April,2007 ME114

Descriptive Geometry Lecture
s

21

Representative Examples(2)

• A plane surface such that a hole drilled

perpendicular is seen in full scale, as if looking

through the hole (say, to test for clearances with

other drilled holes)

• A plane equidistant from two skew lines in

general positions (say, to confirm safe radiation

distance?)

• The shortest distance from a point to a plane

(say, to locate the most economical position for

bracing)

• The line of intersection between two surfaces,

including curved surfaces (say, for the most

economical sizing of sections?)

• The true size of the angle between two planes

April,2007 ME114

Descriptive Geometry Lecture
s

22

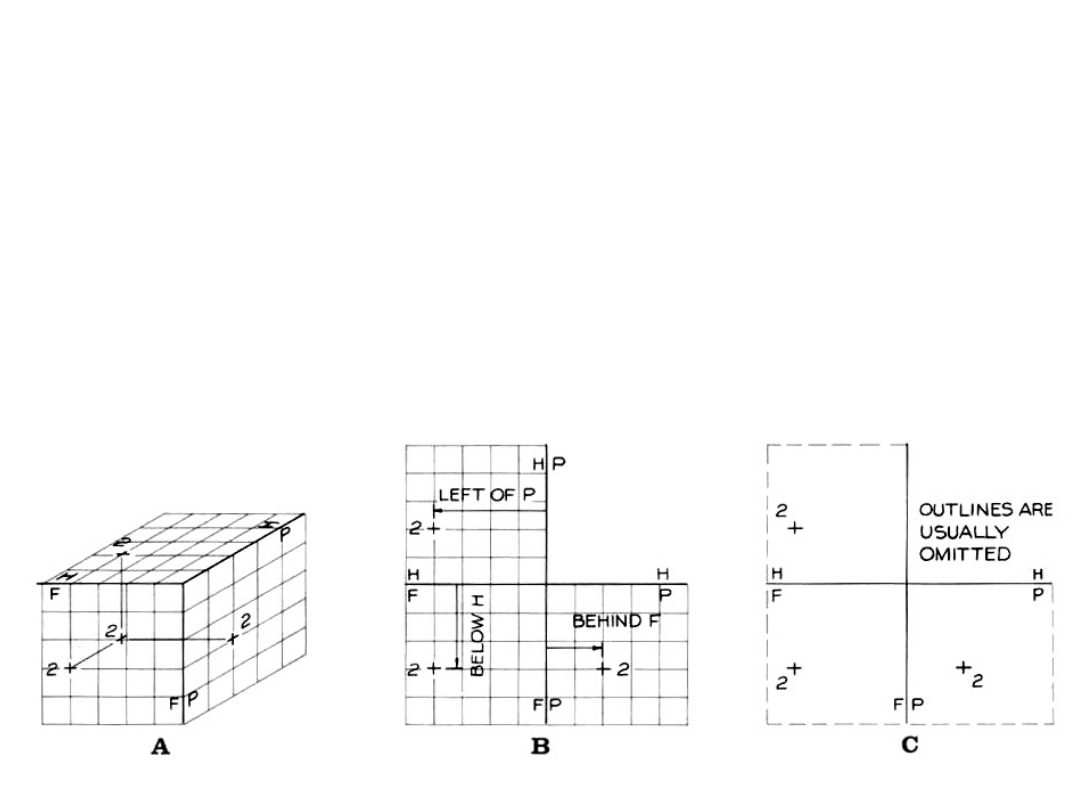
Some Axioms

• A few axioms of projective geometry are:

• 1. If A and B are distinct points on a plane, there is

at least one line containing both A and B.

• 2. If A and B are distinct points on a plane, there is

not more than one line containing both A and B.

• 3. Any two lines in a plane have at least one point

of the plane (which may be the point at infinity) in

common.

(Veblen and Young 1938, Kasner and Newman 1989).

April,2007 ME114

Descriptive Geometry Lecture
s

23

Geometric Elements: Point

• A point is a theoretical location in space and it is

without dimensions.

• A point must be projected perpendicularly onto at

least two principal planes to establish its true
position.

*

April,2007 ME114

Descriptive Geometry Lecture
s

24

Geometric Elements: Line

• Imagine a point in space:
• Imagine that this point starts moving slowly.

April,2007 ME114

Descriptive Geometry Lecture
s

25

Geometric Elements: Line

• Imagine now this point to move very fast in

one direction only.

• As you see it move in your mind, it will
leave a trace: this trace will be a straight
line.

April,2007 ME114

Descriptive Geometry Lecture
s

26

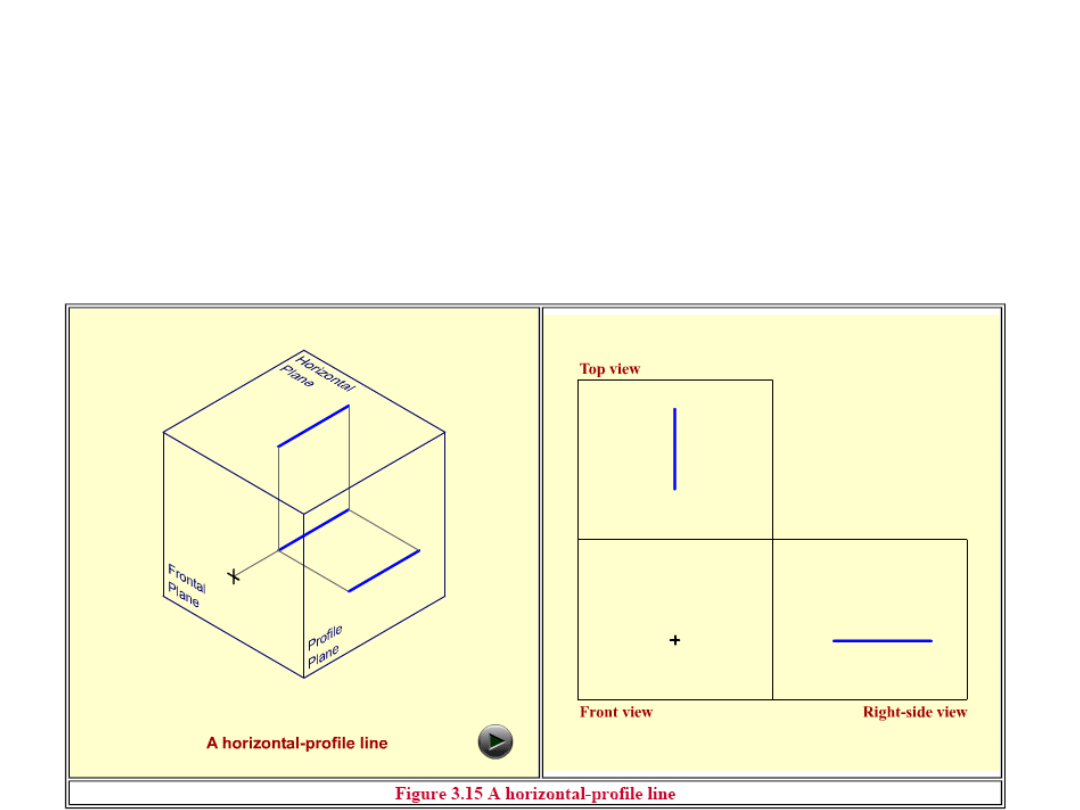
Geometric Elements: Line

• A line is considered to be infinite in length,

the portion between any two points on it
simply specifies a segment.

• Line Positions: A line may lie in two, one

or none of the principal planes of projection.

April,2007 ME114

Descriptive Geometry Lecture
s

27

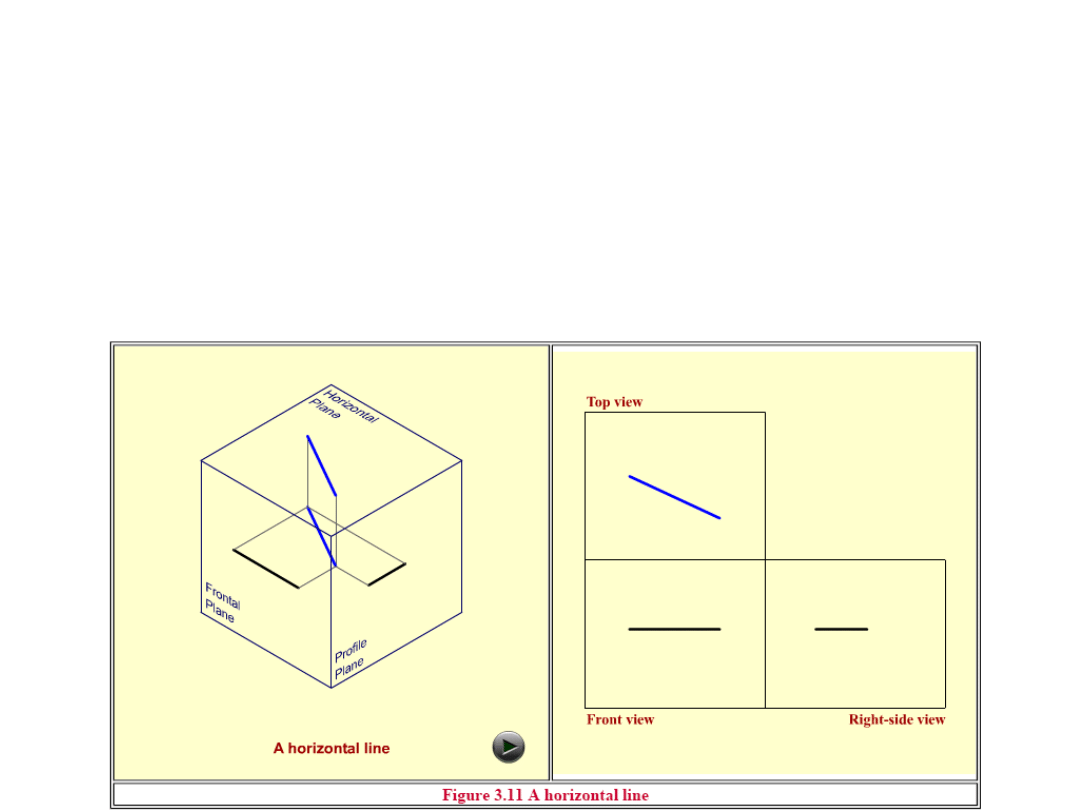
Geometric Elements: Line

• 1. If it lies in two principal planes of projection, it will be

seen normal (seen with the true length) in the two of front,
top or right side views; and it is named for the planes it lies
in. That line appear normal in two principal planes will be
seen as a point in the third view.

April,2007 ME114

Descriptive Geometry Lecture
s

28

Geometric Elements: Line

• 2. If it lies in one principal plane of projection, it will be seen

normal (seen with its true length) in only one of the
principal planes and it is named for the plane it lies in. That
line lying in one principal plane of projection may be
inclined to the other two principal planes. An auxiliary view
is required to show the end view of that line.

April,2007 ME114

Descriptive Geometry Lecture
s

29

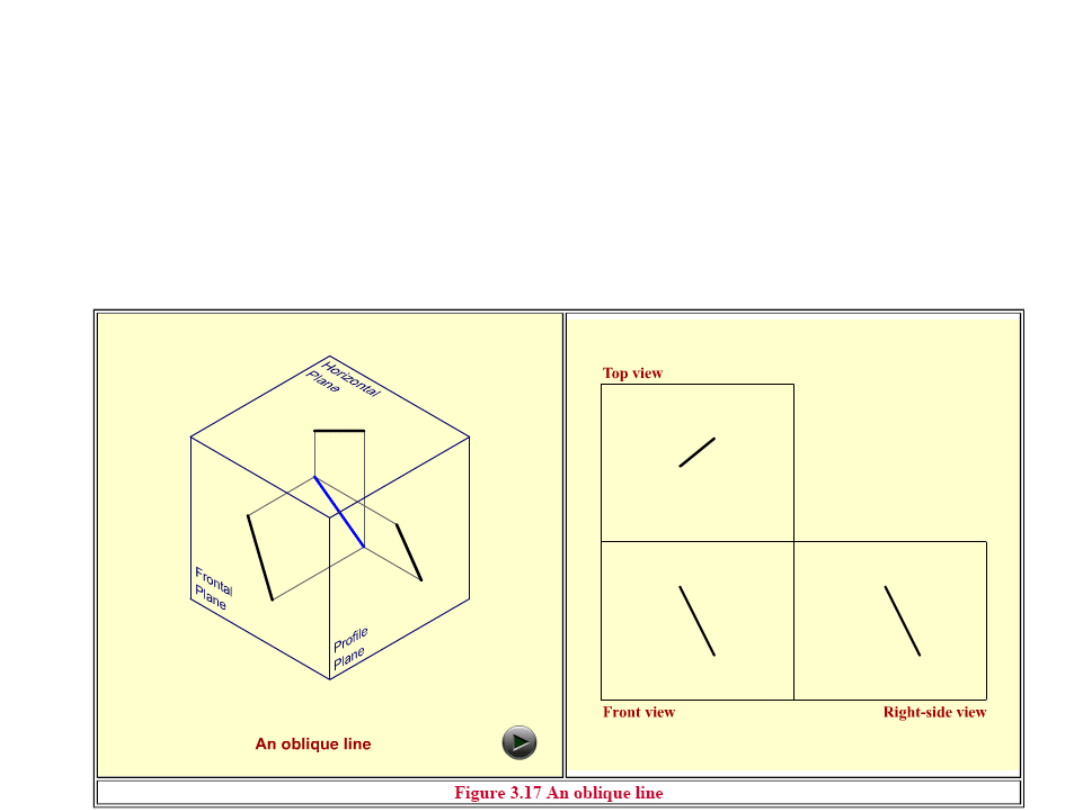
Geometric Elements: Line

3. If it lies in none of the principal planes of projection, it is

called an “oblique line”, will not appear normal in any of
the principal views. Two auxiliary views are required to
show the normal and edge views of that line.

April,2007 ME114

Descriptive Geometry Lecture
s

30

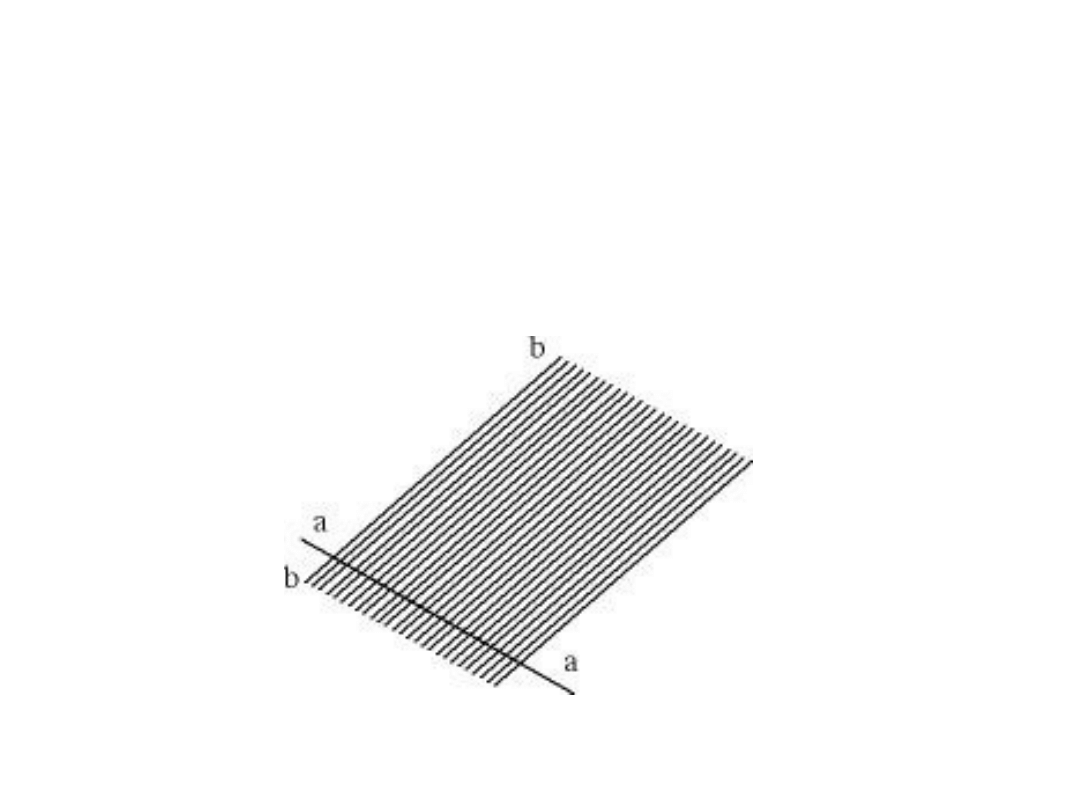
Geometric Elements: Plane

• Now imagine that straight line is stationary for a moment. You

would like it to move like the point moved, but you need a
direction. Another straight line will cross over this line, which
we will call a. The line which gives direction will be called b.

This movement again leaves a trace. In this case the trace is a
plane.

April,2007 ME114

Descriptive Geometry Lecture
s

31

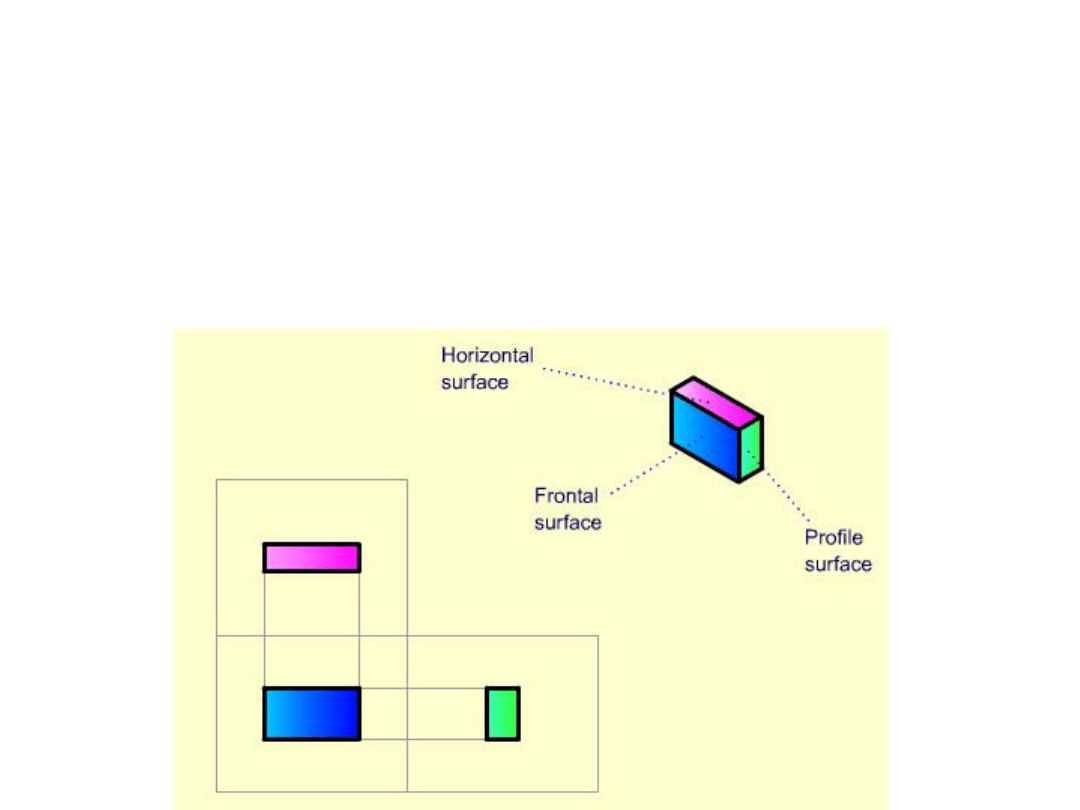
Geometric Elements: Plane

• A plane can be defined by three points, one

point and one line, two parallel lines, or two
intersecting lines. Planes are often to be
infinite in size. The definition of a plane
simply sets its orientation in 3D space.

• Plane Positions: A plane may be parallel

to one of the principal planes of projections,
inclined to two principal planes of
projection, or inclined to all three principal
planes.

April,2007 ME114

Descriptive Geometry Lecture
s

32

Geometric Elements: Plane

• 1. If the plane is parallel to one of the principal planes it

receives its name from that principal plane and it will be seen
normal in that principal plane.

April,2007 ME114

Descriptive Geometry Lecture
s

33

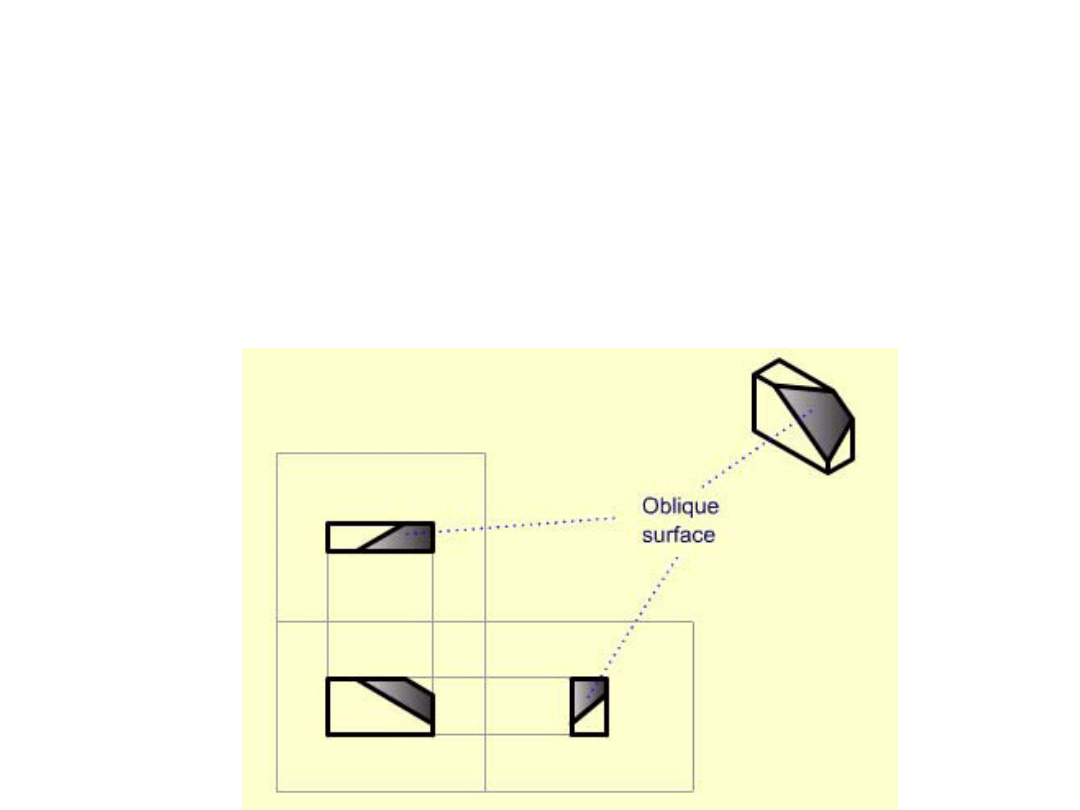
Geometric Elements: Plane

• 2. If the plane is inclined to one of the principal planes it is also

inclined to another principal plane. It can not be seen normal in any of
the orthographic views. It will appear as a line (edge view) in one view.
An auxiliary view is required to show the normal view of the plane.

April,2007 ME114

Descriptive Geometry Lecture
s

34

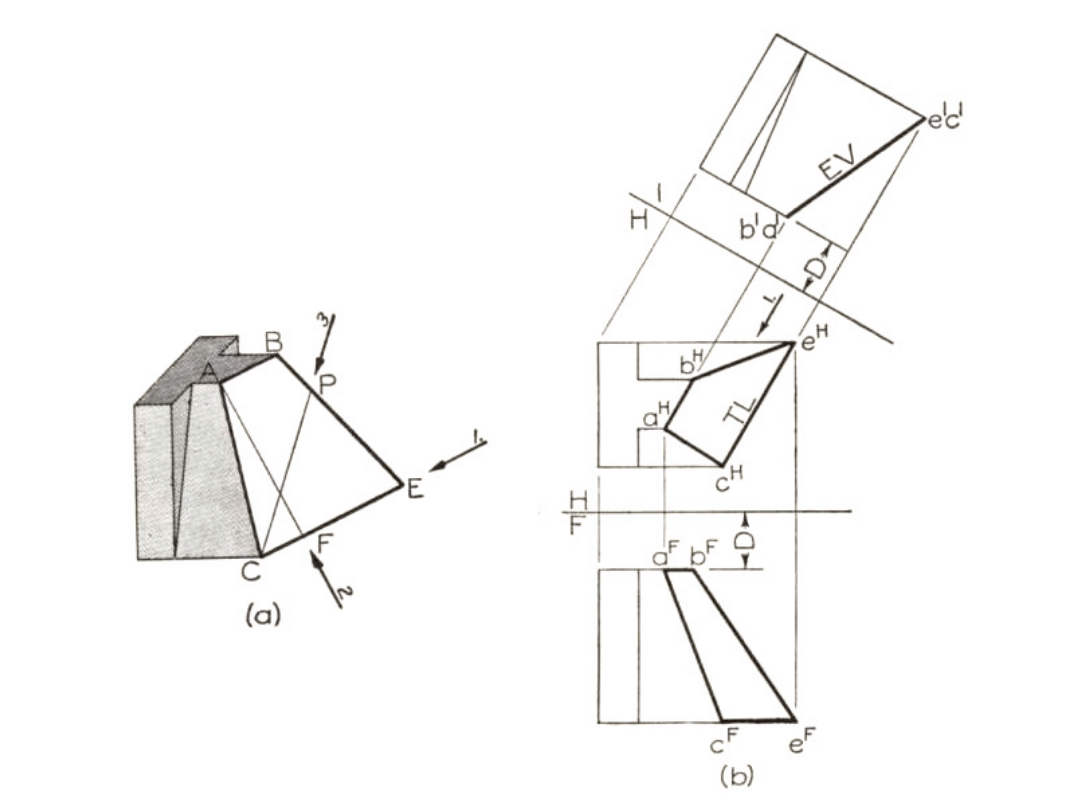
Geometric Elements: Plane

• 3. If the plane is inclined to all of the three principal planes, it

is called as oblique plane. It will not appear as a normal or as
an edge view in any of the principal views. Two auxiliary views
are required to show the edge and normal views of the plane.

April,2007 ME114

Descriptive Geometry Lecture
s

35

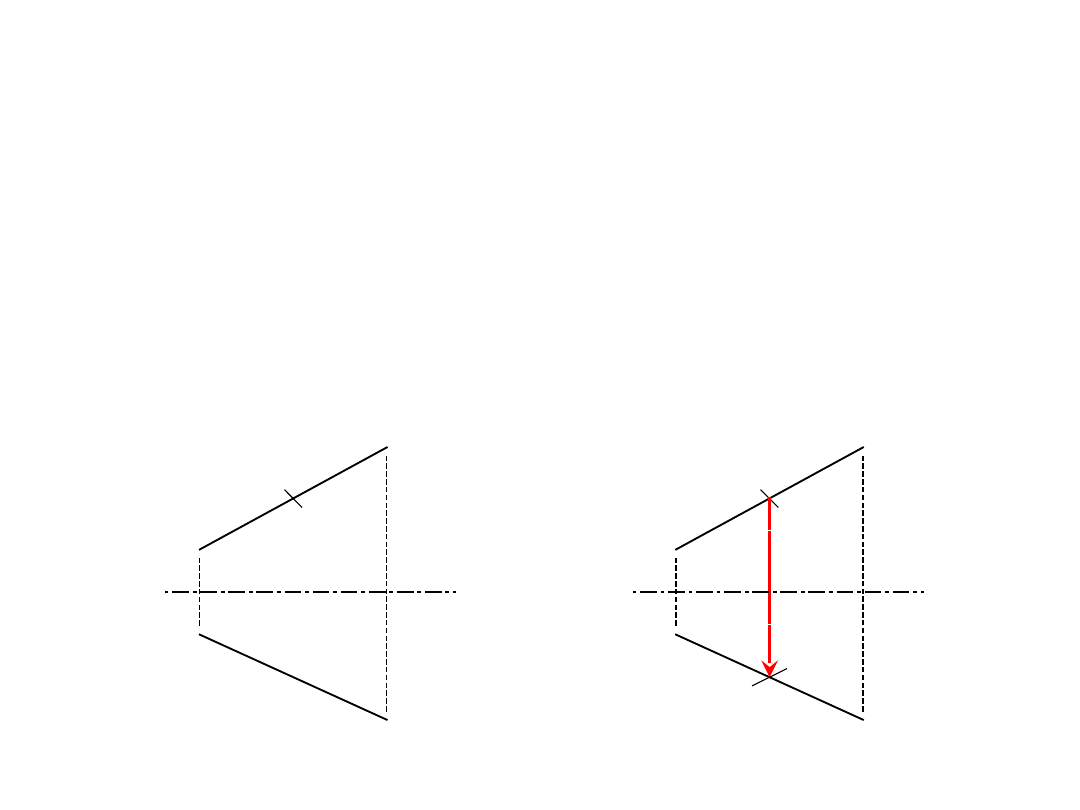
Geometric
Elements: Plane

April,2007 ME114

Descriptive Geometry Lecture
s

36

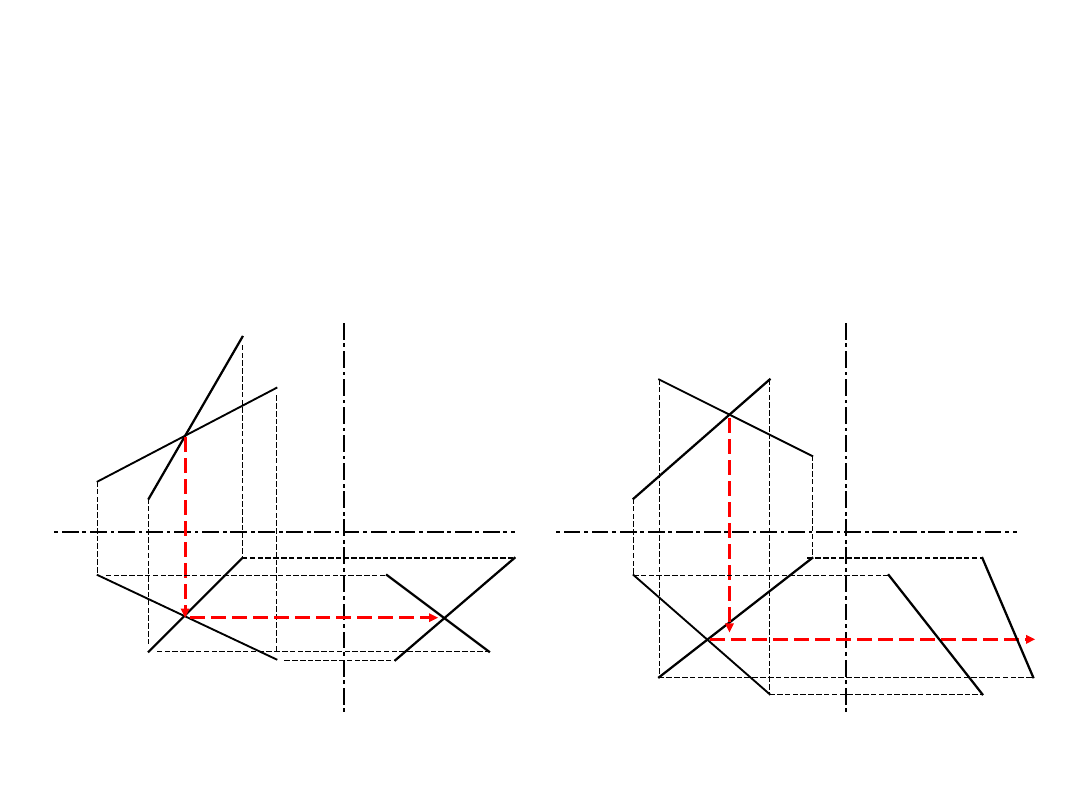
Location of a Point on a Line

• The top and front views of line 1-2 are shown in the figure.

Point O is located on the line in the top view and it is required
that the front view of the point be found.

• A point on a line that is shown orthographically can be found

on the front view by projection. The direction of the projection
is perpendicular to the reference line between the two views.

H
F

1

1

2

2

O

GIVEN

H
F

1

1

2

2

O

SOLUTION

O

April,2007 ME114

Descriptive Geometry Lecture
s

37

Intersecting and Non-

Intersecting Lines

• Lines that intersect have a point of intersection that lies on both lines

and is common to both. Point O is a point of intersection since it
projects to a common crossing point in the three views given in (a).

(b)

H
F

1

3

2

4

O

4

1

2

3

4

1

P

3

F

?

?

2

H
F

5

5

6

6

O

7

6

8

5

8

7

O

O

P

(a)

7

8

April,2007 ME114

Descriptive Geometry Lecture
s

38

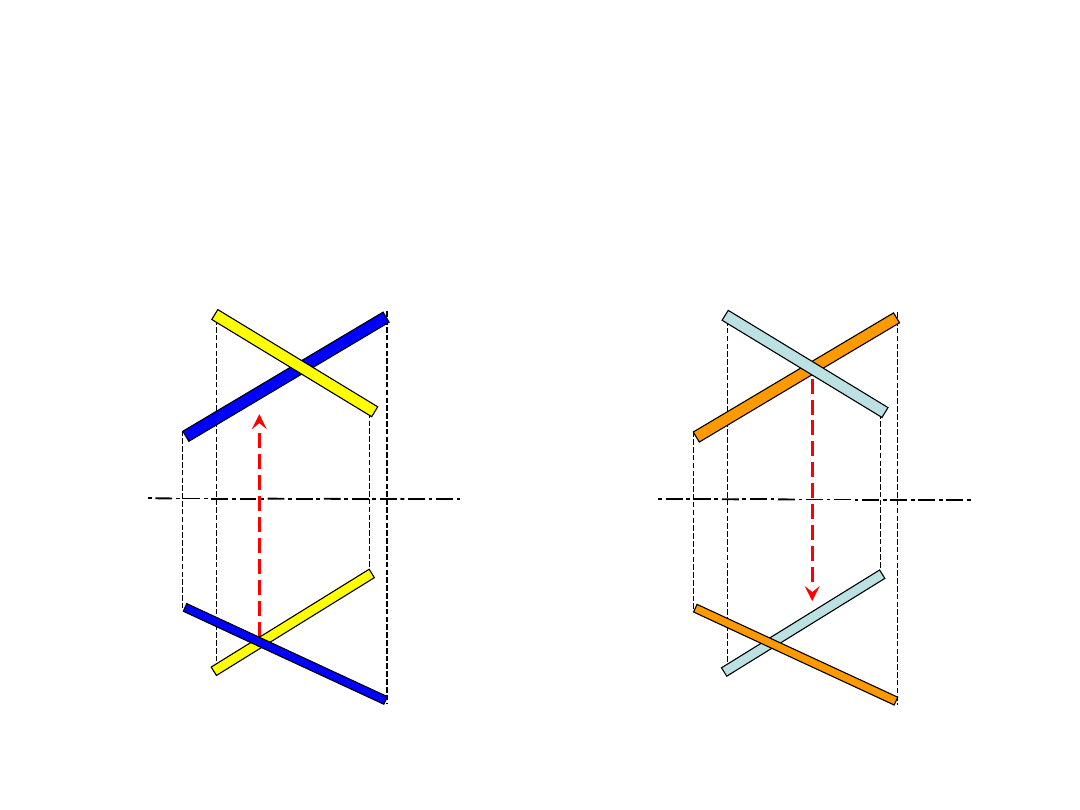
Visibility of Crossing Lines

• Lines AB and CD do not intersect, however, it is necessary to

determine the visibility of the lines by analysis.

(a)

(b)

B

H
F

A

A

C

B

D

C

D

[CD] is HIGHER

[AB] is IN

FRONT

H
F

A

A

C

B

B

D

C

D

April,2007 ME114

Descriptive Geometry Lecture
s

39

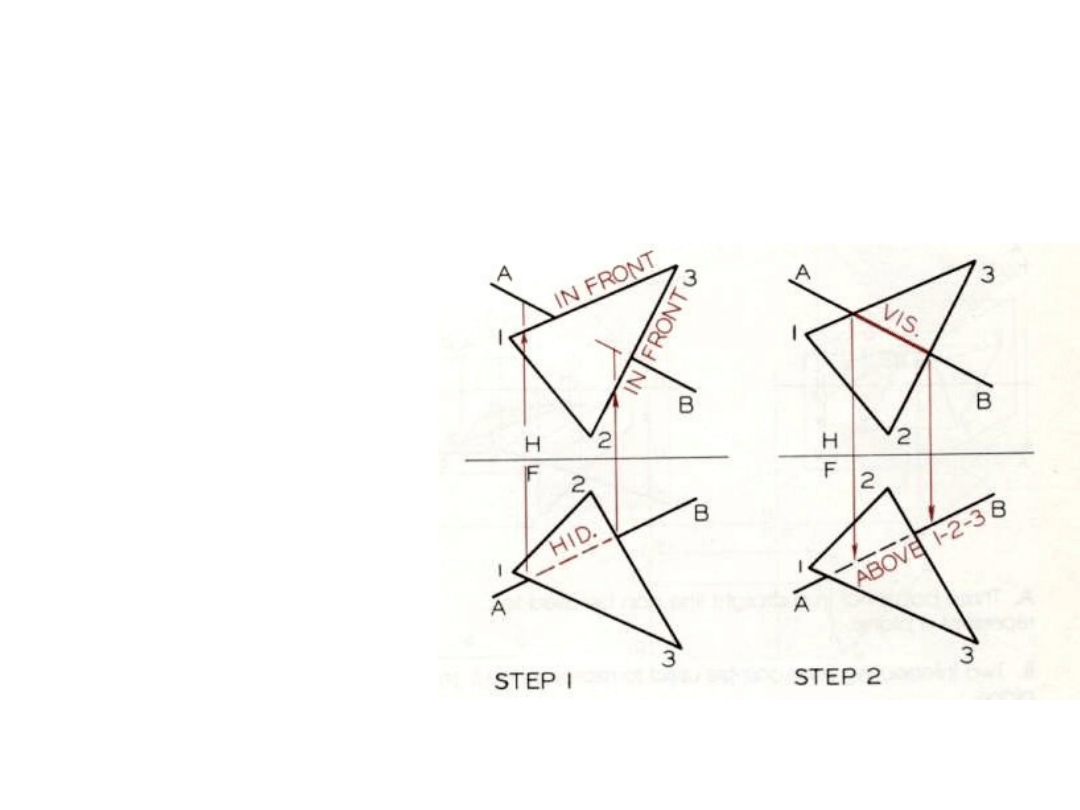
Visibility of a Line and a Plane

Step 1.

Project the points where AB
crosses the plane in the
front view to the top view.
These projectors encounter
lines 1-3 and 2-3 of the
plane first; therefore, the
plane is in front of the line,
making the line invisible in
the front view.

Step 2.

Project the points where AB
crosses the plane in the top
view to the front view. These
projectors encounter line AB
first; the line is higher than
the plane, and the line is
visible in the top view.

REQUIRED:

Find the visibility of the plane and the line in both views.

April,2007 ME114

Descriptive Geometry Lecture
s

40

A Line on a Plane

• A line, say AB, is given on the front view of the plane

and it is required to find the top view of that line.

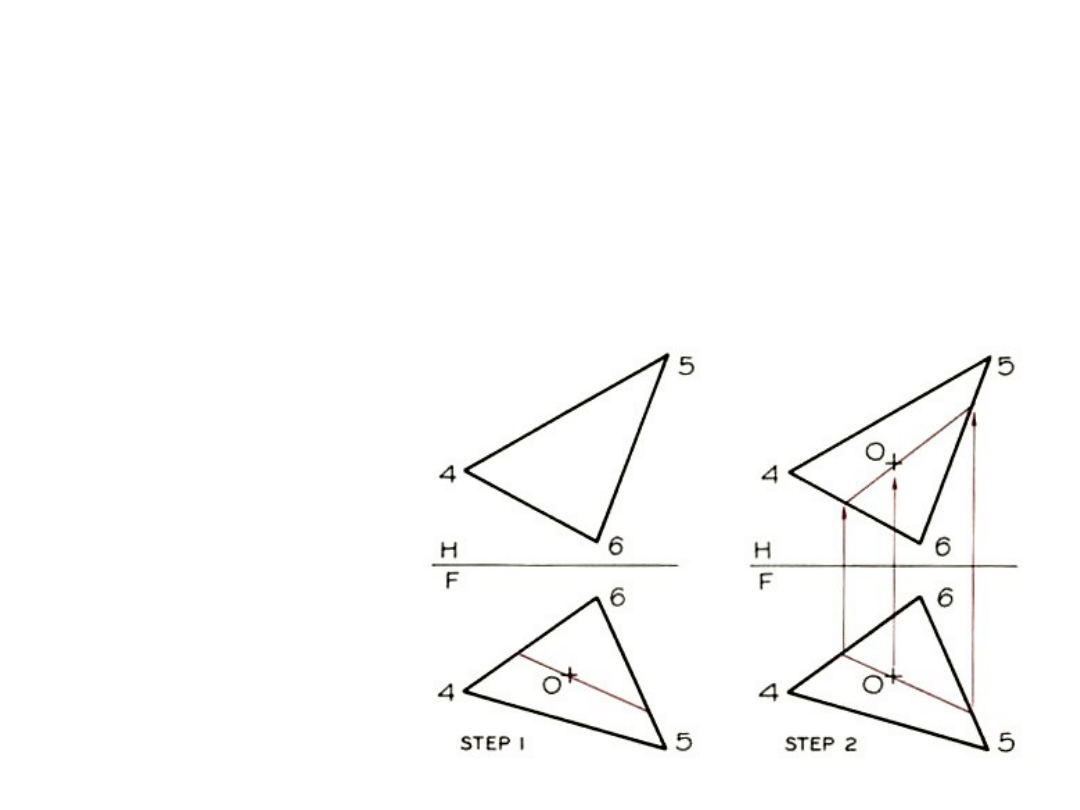
April,2007 ME114

Descriptive Geometry Lecture
s

41

A Point on a Plane

• A point, say O, is given on the front view of plane 4-5-6 and it

is required to locate the point on the plane in the top view.

Step 2.
Project the ends of the
line to the top view
and the draw the line.
Point O is projected to
the line.

Step 1.
Draw a line through
the given view of
point O in any
convenient direction
except vertical.

April,2007 ME114

Descriptive Geometry Lecture
s

42

Piercing Points

• Unless a line is in or parallel to a plane, it must

intersect the plane. This intersection point, called “a
piercing point”, may be within the limits of the line
segment or plane as given, or it may be necessary
to extend one or both, in which case the piercing
point can be considered imaginary.

There are two methods to find the piercing points:

- Auxiliary-View Method
- Two-View Method

April,2007 ME114

Descriptive Geometry Lecture
s

43

Piercing Points

Auxiliary-View Method: An edge view of a plane contains all points in
the plane. Therefore, in a view which shows the given plane in edge
view, the point at which the given line intersects the edge view of the
plane is the point common to both-the piercing point.

April,2007 ME114

Descriptive Geometry Lecture
s

44

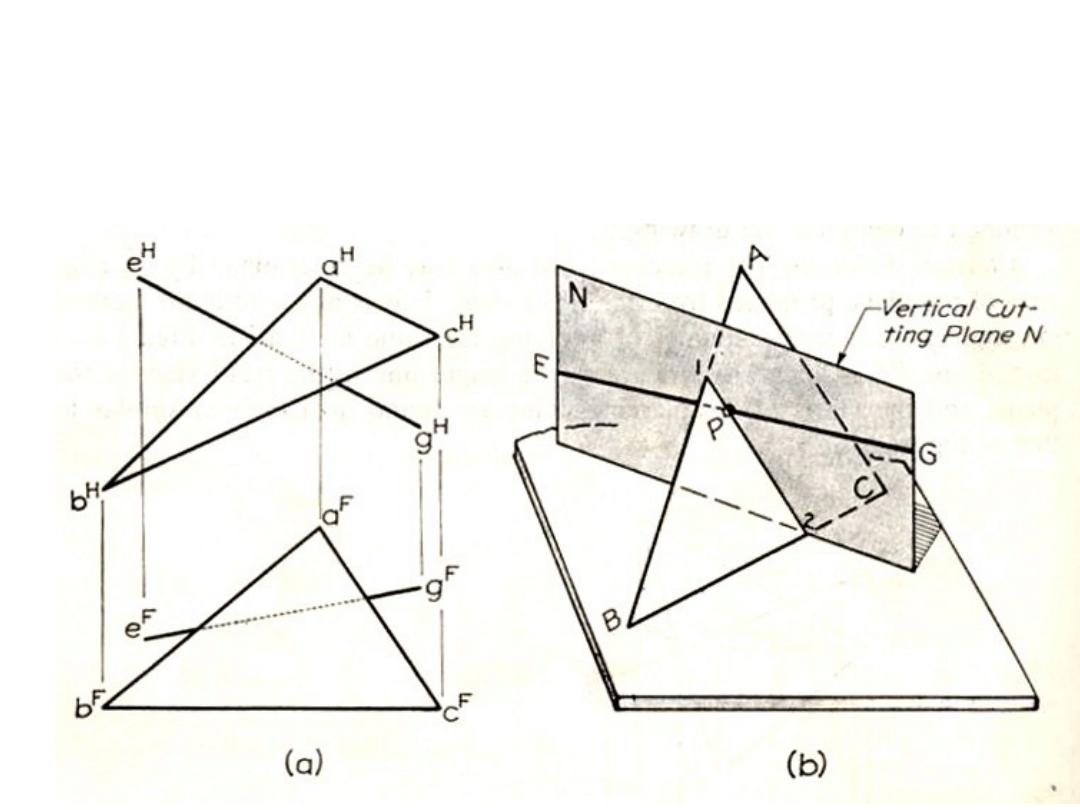
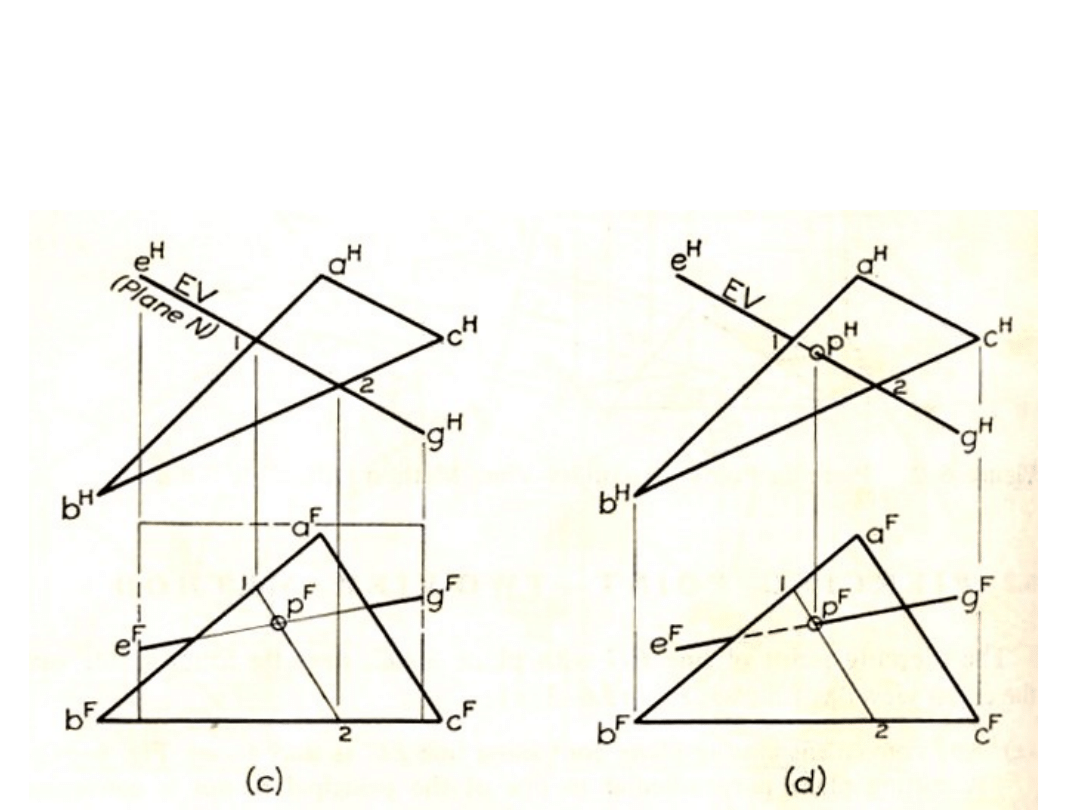
Piercing Points

Two-View Method: The piercing point of line EG with plane

ABC, may be found using only the given views as follows;

a.

Any convenient cutting plane containing line EG is
introduced. A cutting plane perpendicular to one of the
principal planes is convenient because it appears in edge
view in a principal view. This simplifies the following step.

b.

The line of intersection 1,2 between this cutting plane
and plane ABC is determined.

c.

Since lines EG and 1,2 both lie in the cutting plane, they
intersect, locating point P.

d.

Since line 1,2 also lies in plane ABC, point P is the
required piercing point of line EG with the plane ABC.

April,2007 ME114

Descriptive Geometry Lecture
s

45

Piercing Points

Two-View Method

April,2007 ME114

Descriptive Geometry Lecture
s

46

Piercing Points

Two-View Method

April,2007 ME114

Descriptive Geometry Lecture
s

47

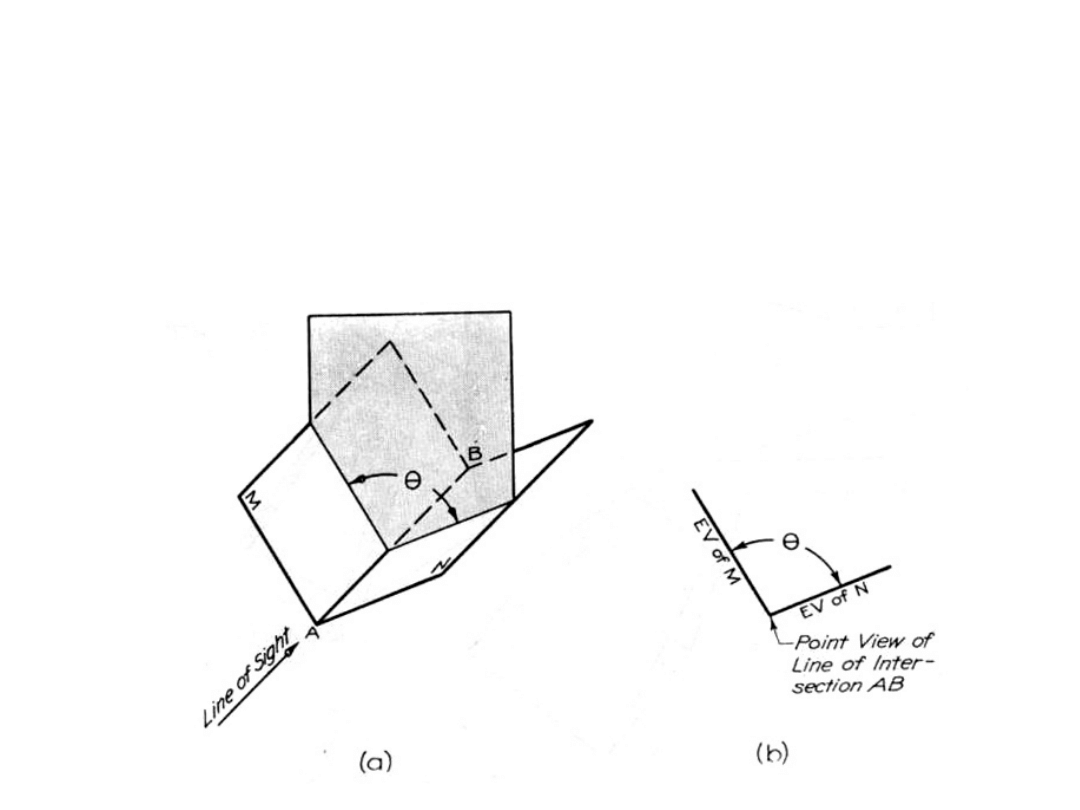
Angle Between Planes

Dihedral Angle: The angle formed by two intersecting planes is
called a dihedral angle. A view in which each of the given planes
appears in edge view shows the true size of the dihedral angle.

April,2007 ME114

Descriptive Geometry Lecture
s

48

Angle Between Planes

Dihedral Angle - Line of Intersection Given

April,2007 ME114

Descriptive Geometry Lecture
s

49

Parallelism

If two lines are oblique lines and they appear parallel in two adjacent views, they are truly
parallel.

April,2007 ME114

Descriptive Geometry Lecture
s

50

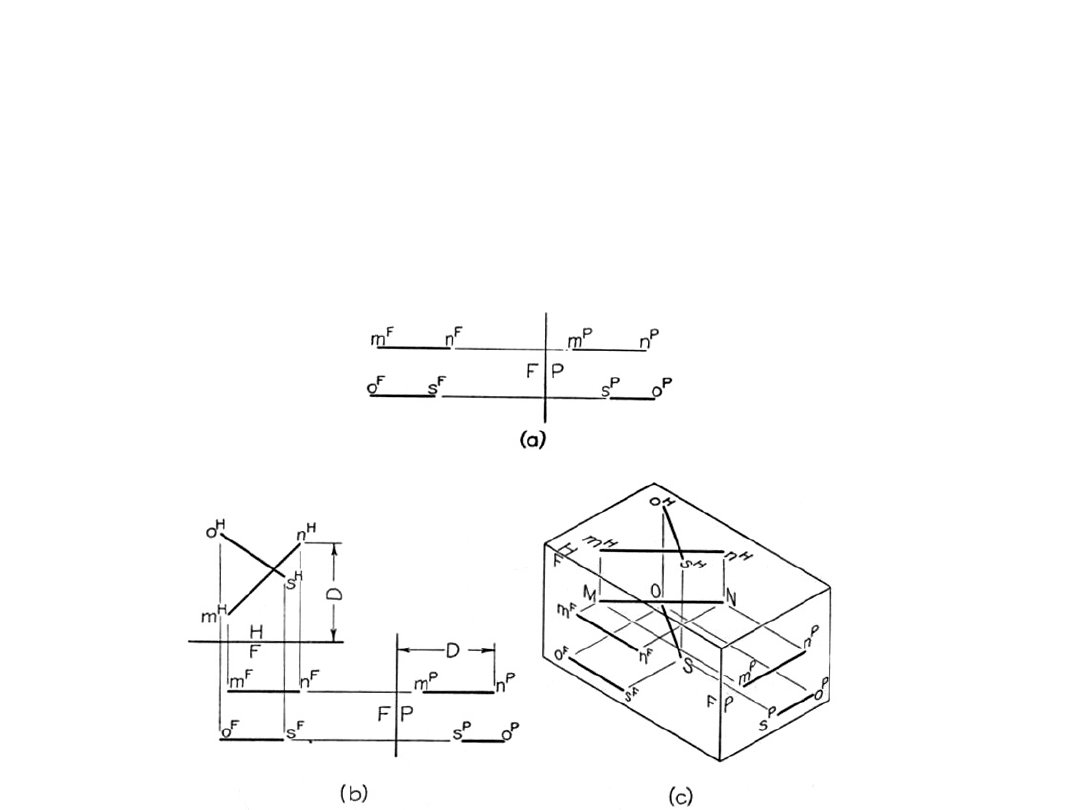
Parallelism

Check of Parallelism of Principal Lines: However, lines which appear normal, such as horizontal lines MN and OS in the figure,
may appear parallel in the F and P views but not really be parallel. Therefore, normal lines may need to be checked in all three
principal views.

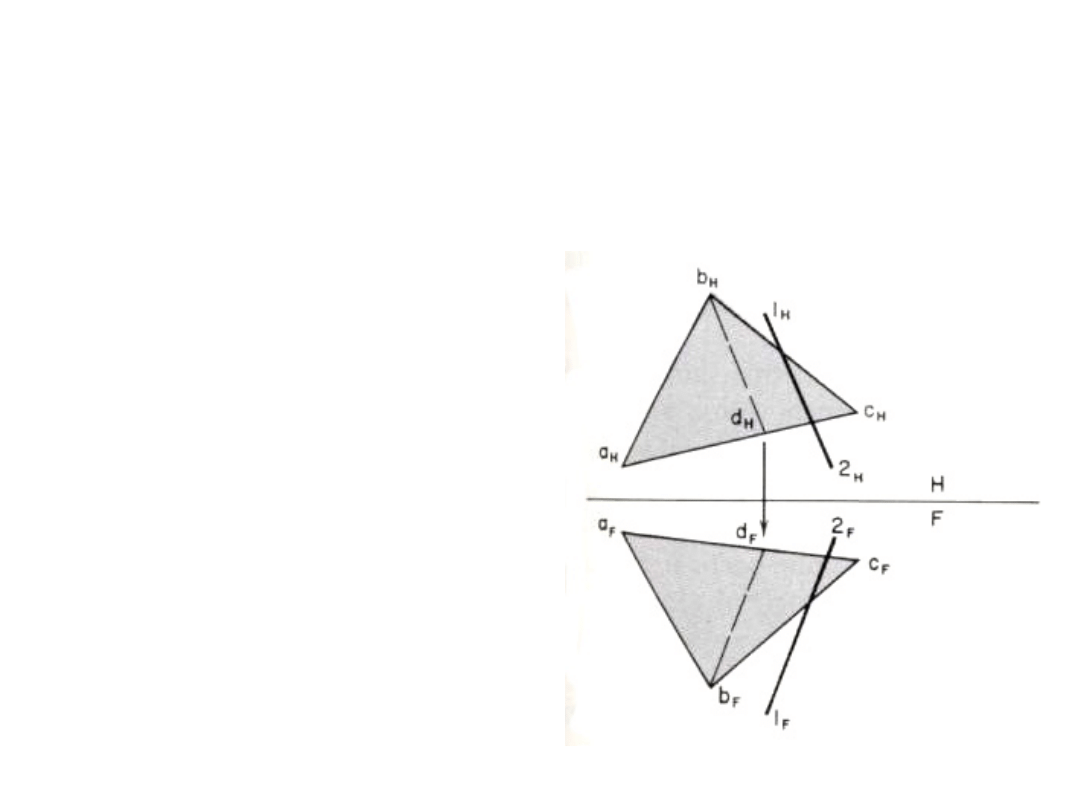
April,2007 ME114

Descriptive Geometry Lecture
s

51

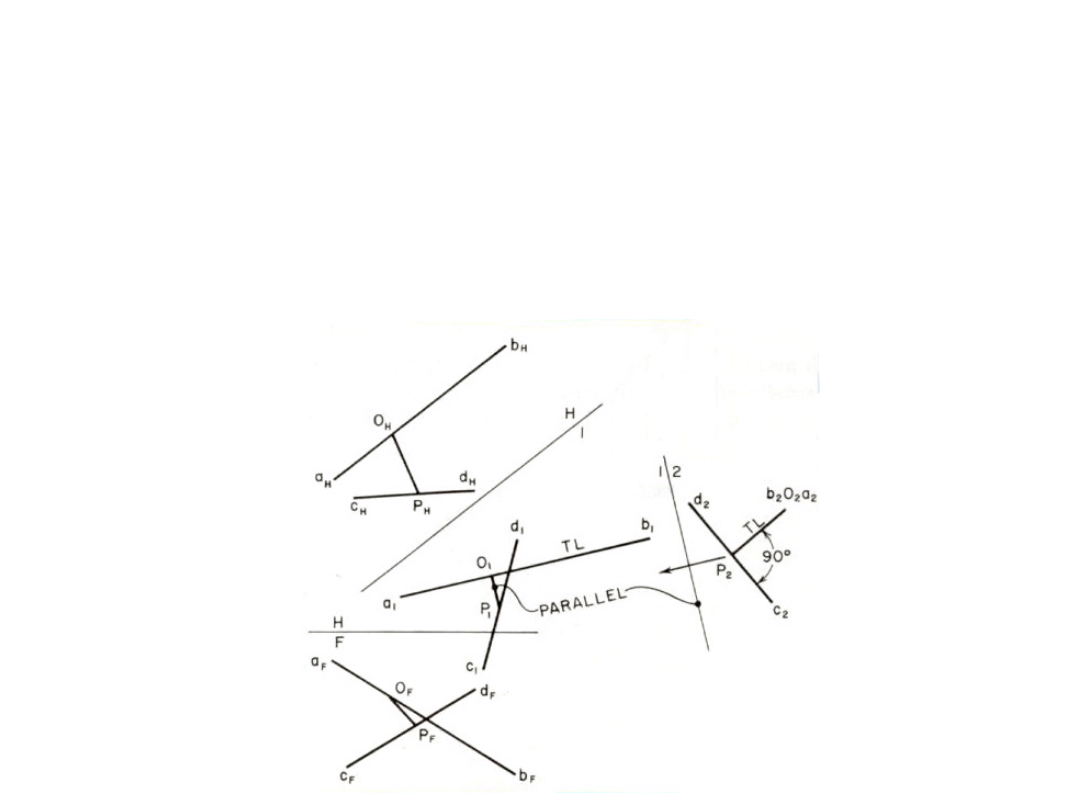
A Line Parallel to a Plane

A line is parallel to a plane if the line remains parallel
to a corresponding line of the plane in all views.

First, a line BD parallel to
line 1-2 is added to the
plane in the H view. Line
BD is projected into the
plane in the F view.

Second, line 1-2 is forced
to be parallel to BD in the
F view. Line 1-2 is now
parallel to the plane

April,2007 ME114

Descriptive Geometry Lecture
s

52

Perpendicularity

“If a line is perpendicular to a plane, it is
perpendicular to every line in the plane.”

In orthographic
projection:

If two lines are
perpendicular,
they appear in
any view
showing at least
one of the lines
in true length.

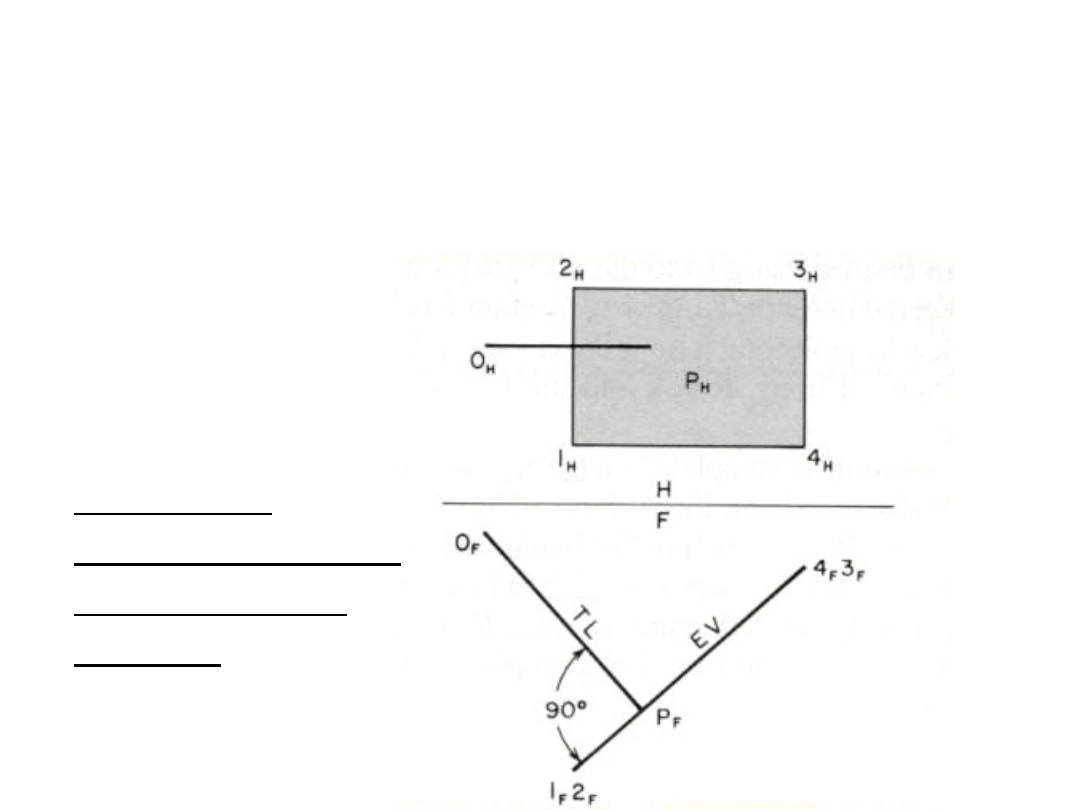
April,2007 ME114

Descriptive Geometry Lecture
s

53

A Line Perpendicular to a

Plane

A line is perpendicular to a plane when the line is true
length at a 90

0

angle to an edge view of the plane.

A line
perpendicular to
a plane is the
shortest
distance from
a point to a
plane.

April,2007 ME114

Descriptive Geometry Lecture
s

54

Shortest Distance from

a Point to a Line

The use of spatial analysis has practical applications. If, for
example, we want to hook up a waterline from a point in a
house to the water main, how can we find the shortest
distance? The shortest distance saves material costs, all other
things being equal.

The shortest distance from a point to a line is a perpendicular
from the point to the line. The shortest distance shows true
length when the original line is a point view.

April,2007 ME114

Descriptive Geometry Lecture
s

55

Shortest Distance

from a Line to a Line

In design work we may need the shortest connector between two
lines which are not parallel and do not intersect. Such lines are
called skew lines. We note that the shortest connector between
two skew lines is the connector perpendicular to each line.

April,2007 ME114

Descriptive Geometry Lecture
s

56

Intersection of Two Planes

The intersection of two planes is a straight line common
to the plane, and its position is therefore determined by
any two points common to the planes. Points common to
two planes may be found by any one of the three general
methods:

1.

The auxiliary-view method

2.

The two-view(piercing-point) method

3.

The cutting-plane method.

Since the piercing-point of a line in a plane may be determined
by using only the two views, the line of intersection of two
planes may be located by applying this piercing-point method
twice, or more if necessary for accuracy.

April,2007 ME114

Descriptive Geometry Lecture
s

57

Intersection of Planes with

Solids

Problems involving the intersections of two surfaces in
general may be solved by one of two methods:

1.Lines in one surface are selected and their piercing points
with the other surface are found. For practical reasons, the
selected lines should be of a type convenient to handle,
such as straight lines or circles.
2.Additional cutting surfaces are introduced, cutting pairs of
lines from the given surfaces. The point of intersection of
the two lines of one pair is a point common to the given
surfaces and is therefore on their line of intersection. The
additional cutting surfaces are usually planes but may be
spheres for certain problems.
The methods are employed in finding the intersections of
planes with the surfaces of solids.

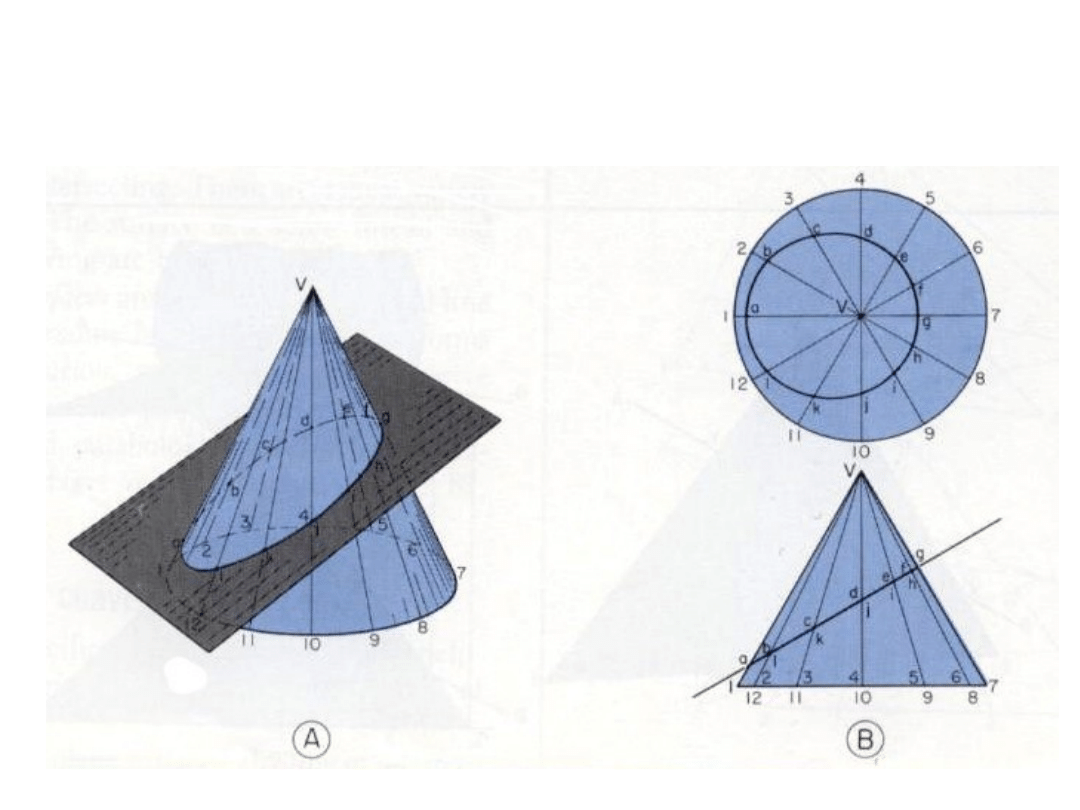
April,2007 ME114

Descriptive Geometry Lecture
s

58

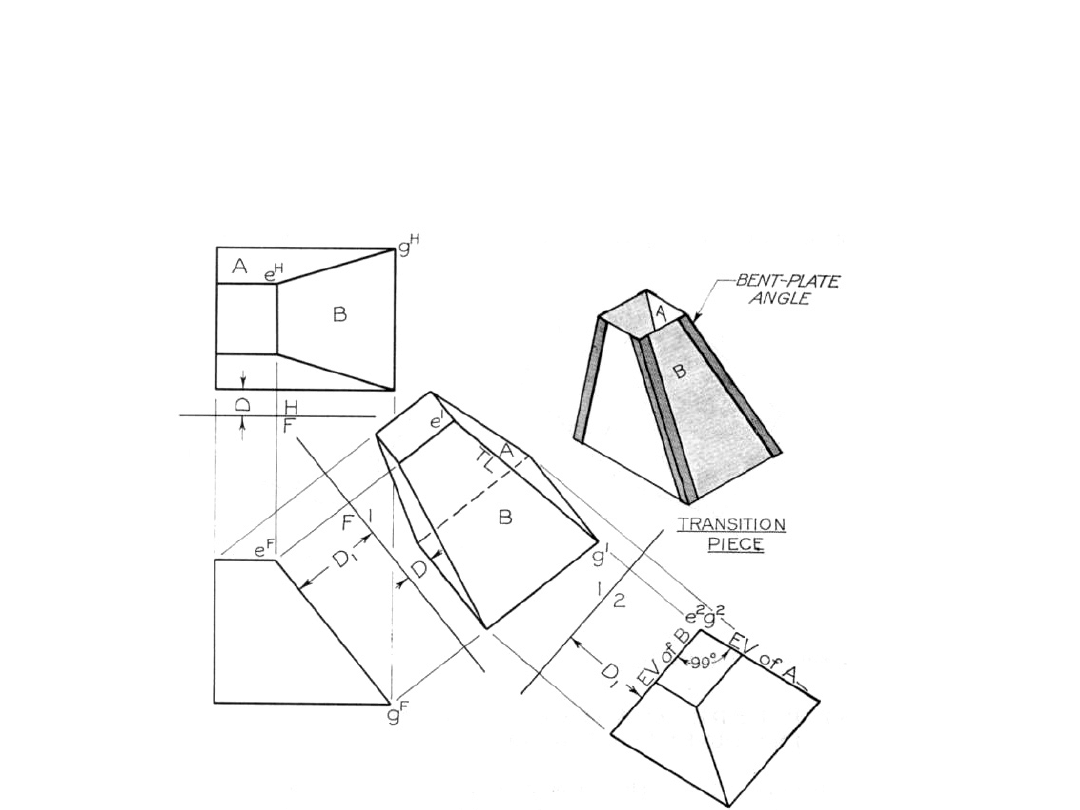
Intersection of Planes with

Solids

Example

April,2007 ME114

Descriptive Geometry Lecture
s

59

References

[1] http://mathworld.wolfram.com/ProjectiveGeometry.html

[2]

http://en.wikipedia.org/wiki/Descriptive_geometry

[3]

http://mathsisgoodforyou.com/topicsPages/geometry/descri
ptive.htm

[4]

http://en.wikipedia.org/wiki/Perspective_projection

[5] ME113 Lecture Notes, Chapter 3, METU
[6] An Analytical Introduction to Descriptive Geometry, Adrian

B. Biran

[7] http://en.wikipedia.org/wiki/Orthogonal_projection
[8] Engineering Design Graphics, James H. Earle, 4th Edition
[9] Descriptive Geometry, E.G.Pare, R.O. Loving, I.L.Hill, 3rd

Edition