Summary

Predicting explosive response to jet attack is dif®cult due to the

complex nature of the projectile. The shock duration term in the cri-

tical energy (Ec) criterion developed by Walker and Wasley for plate

impact is modi®ed for application to round-nosed rods which appear to

simulate jet attack. Experimental data on explosive initiation threshold

in the cases of plate or rod impacts, LS DYNA2D simulations and data

obtained with the new criterion are in good agreement.

1. Introduction

The critical energy (Ec) criterion developed by Walker

and Wasley

(1)

for plane shock initiation of bare hetero-

geneous explosives is widely used to determine the response

of explosive submitted to ¯yer plate impacts. This criterion

has been carefully checked by De Longueville, Fauquignon

and Moulard

(2)

. However, using the criterion in its original

form for rod impacts, Moulard

(3)

found that the experi-

mental value of Ec for a given explosive was considerably

higher than with plate impacts. As a result, James

(4,5)

developed a model based on a maximum shock volume and

showed that a simple modi®cation of the de®nition of the

time term within the criterion allows to apply it to ¯at-nosed

rod impacts. The purpose of this paper is to show that an

analytical modi®cation of the original criterion allows

similar values of Ec to be obtained with shaped charge jet

impacts.

As this modi®ed criterion provides data in good agree-

ment with experimental results, we discuss some of its

implications.

2. Modi®cation of the Criterion

2.1. Introduction

According to Walker and Wasley, the critical energy

represents the minimum energy per unit area transferred

into the explosive upon impact of a ¯yer plate and leading

to initiation. The critical energy is related to the shock

parameters in the explosive by the relationship:

Ec ˆ P ut

…1†

where P is the shock pressure, u the particle velocity, and t

the shock duration.

As no account is taken of chemical energy release in the

explosive, Ec can be considered as a trigger energy only.

In the case of ¯at-nosed rod impacts, James

(4)

evaluated

the time t as the maximum duration of 1D shock in the

explosive. The two de®nitions are the same for plate impact

but differ for other projectile geometries. Table 1 gives the

expression of t with respect to various projectile geome-

tries. (U is the shock velocity, R

0

the jet radius, e the plate

thickness, c

1

the sound speed in the shocked material).

An Analytical Extension of the Critical Energy Criterion Used to

Predict Bare Explosive Response to Jet Attack

F. Peugeot

Etablissement Technique de Bourges, Route de Guerry, F-18015 Bourges cedex (France)

M. Quidot

SocieÂte Nationale des Poudres et Explosifs, Centre de Recherches de Bouchet, F-91710 Vert le Petit (France)

H. N. Presles

Laboratoire de Combustion et de DeÂtonique, ENSMA, F-86034 Poitiers (France)

Eine analytische Erweiterung der Kriterien fuÈr die kritische

Energie zur Voraussage der Reaktion freiliegender Explosivstoffe

bei Einwirkung von Hohlladungsstrahlen

Die Voraussage der Reaktion von Explosivstoffen bei Einwirkung

von Hohlladungsstrahlen ist schwierig aufgrund der komplexen Natur

der Projektile. Der Term der Stoûdauer im Kriterium der kritischen

Energie Ec, von Walker und Wasley entwickelt fuÈr den Plattenauf-

schlag, wurde modi®ert fuÈr die Anwendung von abgerundeten StaÈben,

die den Jet-Einschlag simulieren. Die experimentellen Daten des

Grenzwertes fuÈr die Explosivstof®nitiierung im Fall von Platten- oder

StabaufschlaÈgen, die LS DYNA2D-Simulationen und die mit den

neuen Kriterien erhaltenen Daten stimmen gut uÈberein.

Une extension analytique au criteÁre de l'eÂnergie critique pour

preÂdire la reÂponse d'un explosif soumis aÁ une attaque par jet

PreÂdire la reÂponse d'un explosif soumis aÁ une attaque par jet de

charge creuse s'aveÁre dif®cile compte tenu de la nature complexe de

l'agresseur. A®n de pouvoir appliquer le criteÁre de l'eÂnergie critique

(Ec) deÂveloppe par Walker et Wasley pour l'impact de plaque, aux

projectiles cylindriques aÁ extreÂmite heÂmispheÂrique tels que les jets de

charge creuse, le temps d'application du stimulus est modi®e analy-

tiquement. Il s'aveÁre que les seuils d'initation ainsi calculeÂs offrent de

bonnes comparaisons avec les reÂsultats epeÂrimentaux collecteÂs ou les

simulations numeÂriques reÂaliseÂes.

# WILEY-VCH Verlag GmbH, D-69451 Weinheim, 1998

0721-3115/98/0306±0117 $17.50‡:50=0

Propellants, Explosives, Pyrotechnics 23, 117±122 (1998)

117

2.2 Jet Model

In the case of jet impact, it is necessary to have a jet

model in order to develop an analytical relationship giving

the time corresponding to the maximum 1D shock duration

in the explosive. Despite the possible complexities of the jet

such as velocity gradient, irregular changes in jet diameter

or density variation Pirotais

(6)

and Mader

(7)

, modeled the jet

by an in®nite cylindrical projectile with ¯at end. Moulard

(3)

and Bahl

(8)

performed experiments with ¯at-nosed rods.

Nevertheless, James

(9)

showed that most of published data

on jet impacts into bare explosives support the assumption

that experimental results for jets are comparable to results

from round-nosed rods rather than ¯at-nosed cylinders. As

the penetration of the barrier used to attenuate the jet tends

to round the nose of the projectile and as the jet tip exhibits

a mushroom shape while ¯ying, we support his conclusion

and have modeled the jet by an in®nite round-nosed rod.

2.3 t Modi®cation

Using the assumption that jets behave like round-nosed

rods, we have developed an analytical relation to use the

critical energy concept with this kind of projectiles. Look-

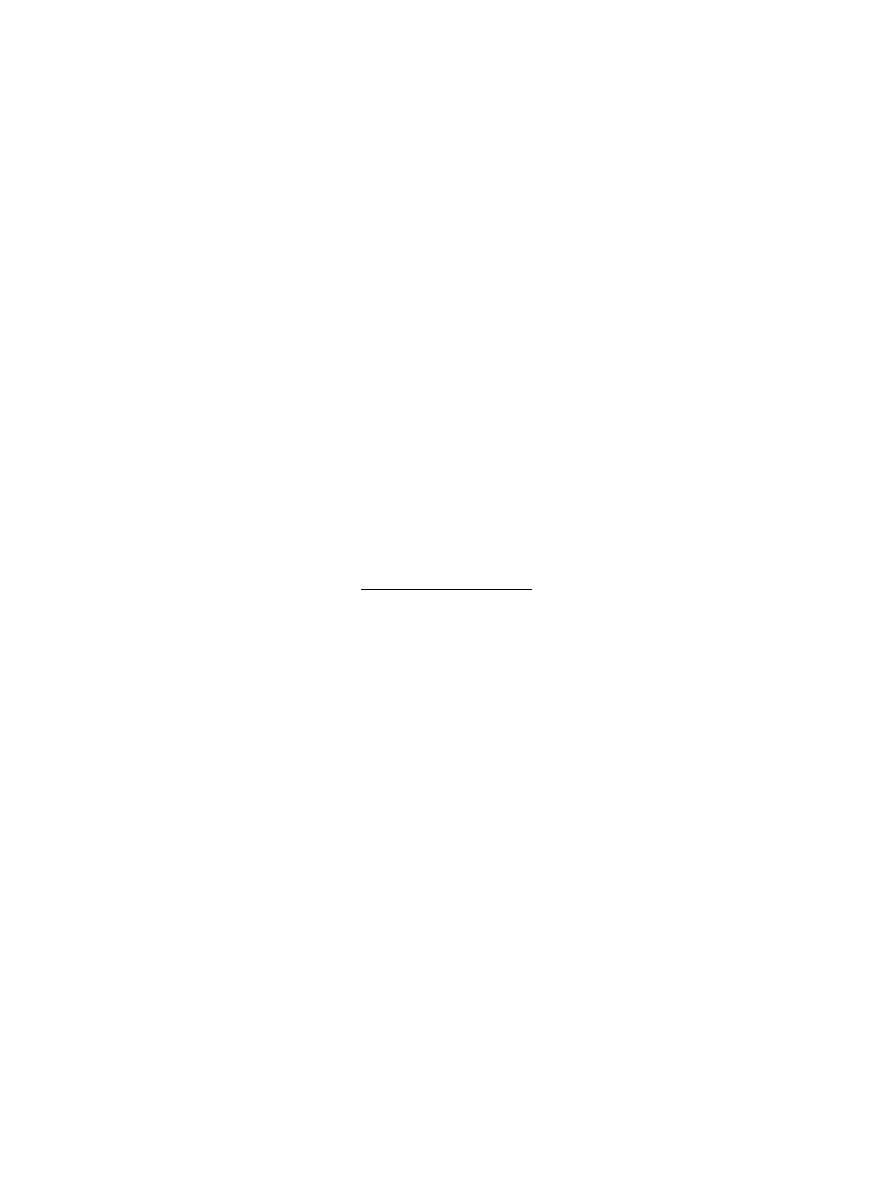
ing at the interaction of the tip of the projectile and the

explosive (Figure 1), at ®rst, the contact point moves

radially with a very high velocity compared to the shock

velocity in the explosive.

V

radial

ˆ V

j

cos b

…2†

where V

j

is the jet velocity, b measures the azimuth and

cos b ˆ 1 ÿ

u

R

0

t

…3†

So, for the target, the projectile acts nearly like a ¯at-faced

rod.

However, the contact point speed drops quickly and

becomes smaller than the shock speed. At the moment t

1

,

when the contact point speed equals the shock speed,

release waves begin to proceed towards the axis (Figure 2)

and the target begins to know that the front of the rod is

rounded. From simple geometrical considerations the azi-

muth b* is given by:

b ˆ arctan

u

c

1

…4†

So, the time t

1

corresponding to the maximum 1D shock

volume is given by:

t

1

ˆ

R

0

u

1 ÿ cos arctan

u

c

1

…5†

where R

0

is the initial radius of the jet.

Introducing the relation (5) in the critical energy criterion

(1), we obtain an analytical criterion:

E

c

ˆ PR

0

1 ÿ cos arctan

u

c

1

…6a†

which can also be written:

E

c

ˆ PR

0

1 ÿ

1


1 ‡

u

c

1

2

s

0
B

B

B

B

@

1
C

C

C

C

A

…6b†

3. Hydrocode Simulation of Round-Nosed Rod Impacts

3.1 Introduction

LS DYNA2D, a hydrodynamic code

(10)

is used to cal-

culate the shock initiation threshold of bare PBX-9404

impacted by copper jets. This code, which integrates an

erosion model, is an explicit two dimensional lagrangian

®nite element code for analyzing the large deformation

dynamic response of inelastics solids and structures. We

Figure 1. Snapshot of shock structure shortly after impact of a round-

nosed rod on a target.

Figure 2. Snapshot of waves propagation.

Table 1. Expression of t with Respect to Various Impact Geometries

Geometry

t

Comments

plate

2e

U

simpli®ed relation

¯at-nosed rod

R

0

3c

1

analytical relation

round-nosed rod and sphere

R

0

9c

1

empirical relation

118 F. Peugeot, M. Quidot, and H. N. Presles

Propellants, Explosives, Pyrotechnics 23, 117±122 (1998)

used the Lee-Tarver (LT) phenomenological shock initia-

tion models [(LT) two terms

(11)

and (LT) three terms

(12)

].

The JWL equation of state is used to simulate the pres-

sure-volume-energy relationship for the material in both the

unreacted and the reacted states.

3.2 Computations

The jet is modeled as a round-nosed rod whose radius and

velocity can be changed. Due to the axisymmetric nature of

the problem, only half of the domain was simulated. The

areas submitted to large and intense deformation are ®nely

gridded to minimize the overlapping of the projectile and

target elements during the penetration process. Elsewhere,

for the present problem, the penetration is controlled by the

failure at strength parameter (fs). In order to eliminate any

penetration effects, we also compute Arbitrary Lagrangian

Eulerian cases (ALE).

The different initiation models [(LT) two terms or three

terms], and the different methods (lagrangian or ALE) give

the same results.

3.3 Results

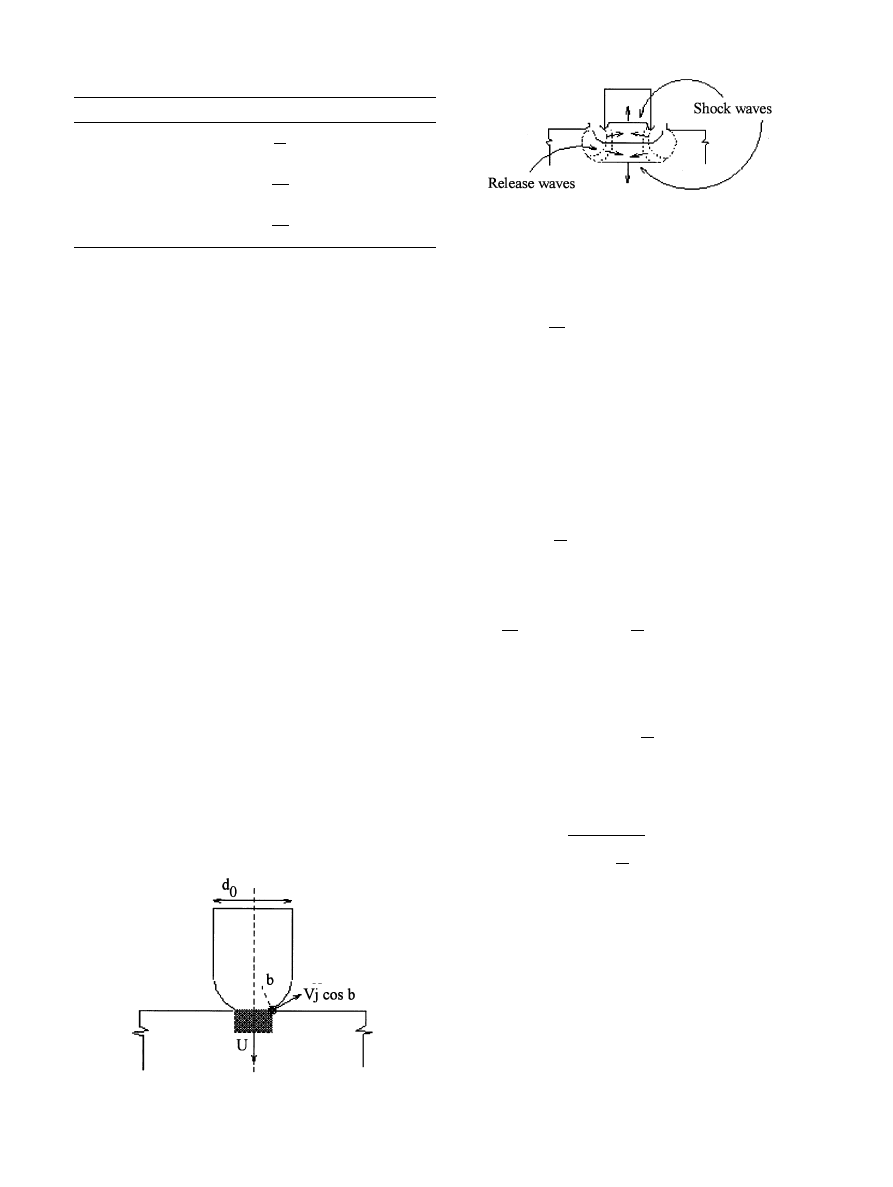
The behaviour of PBX-9404 submitted to copper jet

impact has been calculated with respect to diameter and jet

velocity (Table 2).

In order to analyse the results, three cases are discussed:

First case: V

j

ˆ 1.9 mm=ms, d

j

ˆ 2.6 mm (Fig. 3)

The initial shock compression gives rise to a beginning of

reaction. The chemical reaction rate increases while going

closer to the interface explosive=projectile. Later, the shock

pressure decreases and the reaction is nowhere complete.

There will be no detonation.

Second case: Vj ˆ 1.9 mm=ms, d

j

ˆ 5 mm (Fig. 4)

At the beginning, there is no difference with the ®rst case.

Nevertheless, later, a detonation well characterized by a ®ne

front propagates (reaction rate ˆ 1). The copper jet initiates

a detonation which propagates only if it is sustained by the

jet long enough to become a stable curved detonation front.

This is a detonation case.

Third case: V

j

ˆ 3 mm=ms, d

j

ˆ 2 mm

The reaction is complete at the interface target=projectile

but no evidence of self-sustained propagation is visible. The

detonation will not propagate. The no-propagation case is a

particular no-detonation case.

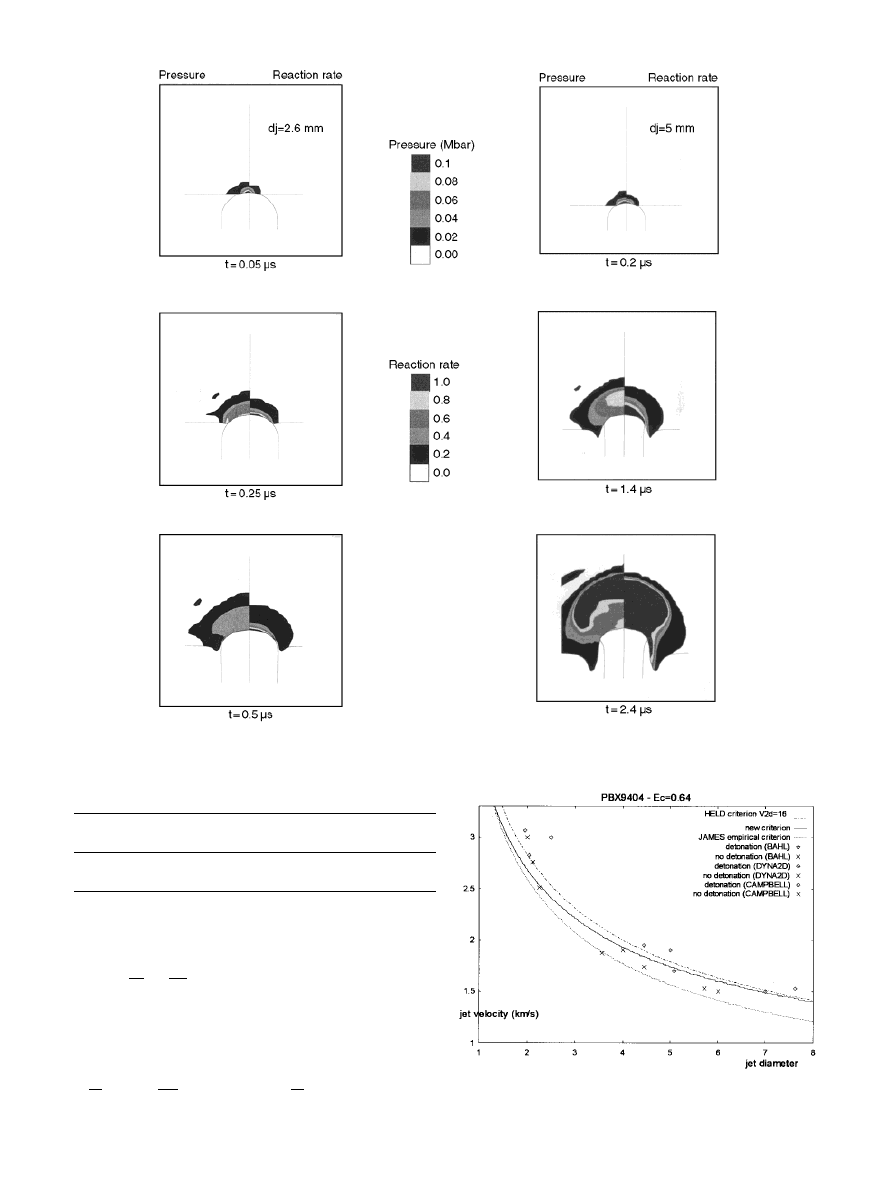
4. Validation of the New Criterion

Now, we compare the different criteria used to evaluate

the response of two explosive compositions, PBX-9404 and

CompB, submitted to round-nosed cylinders or shaped

charge jet impacts. Table 3 gives Hugoniot data, the value

of Ec obtained from 1D impacts and value of V

2

d constant

obtained from jet impact experiments for each composition.

Our new criterion, James' empirical one (Table 1) and the

empirical criterion obtained by Held (V

2

d) were used to

draw curves of constant energy per unit area (Figures 5

and 6).

Except in the small range of jet diameter, our criterion

can be seen to lie within a very short distance from (V

2

d)

criterion which gives a good ®t to the experimental and

numerical data we collected

(2,6,7)

. James' criterion seems to

be less relevant.

5. Discussion and Conclusion

5.1 Relationship between the Diameter of Round-Nosed

and Flat-Nosed Projectiles

This relationship is obtained equating the time t for ¯at-

nosed rod (Table 1) with that for round-nosed rod projectile

given by Eq. (5):

d

flat

ˆ d

round

3c

1

u

1 ÿ cos arctan

u

c

1

…7a†

ˆ 3d

round

u=c

1

1 ‡

u

c

1

2

‡



1 ‡ …u=c

1

p

†

2

0
B

B

B

@

1
C

C

C

A

…7b†

We can approximate this expression:

d

flat



3
2

d

round

u

c

1

…7c†

The ratio d

¯at

=d

round

depends on shock wave characteristics

(Figure 7) unlike the ratio d

flat

=d

round

ˆ 1=3 provided by

James' relationship (Table 1).

5.2 Critical Energy and Critical Diameter

Quidot

(13)

has proposed a relation between the Chapman

Jouguet pressure P

CJ

, the polytropic coef®cient g

CJ

, the

Table 2. Results of Simulations of Round-Nosed Impacts on PBX-

9404

V

j

d

j

Result

mm =ms

mm

1.5

7

detonation

6

no detonation

1.9

5

detonation

4

no detonation

3

2.5

detonation

2

no propagation

Propellants, Explosives, Pyrotechnics 23, 117±122 (1998)

An Analytical Extension of the Critical Energy Criterion 119

critical diameter of detonation d

c

and the critical energy in

the critical condition of the detonation propagation E

cd

:

E

cd

ˆ

1

12

P

CJ

d

c

g

CJ

…9†

Equating (6) and (9) leads to a relationship between the

explosive characteristics (P

CJ

, g

CJ

, d

c

, c

0

, s) and the jet

characteristics (V

j

, d

j

).

d

c

d

j

ˆ 12g

CJ

P

P

CJ

1 ÿ cos arctan

u

c

1

…10†

Figure 4. Impact of a copper jet on PBX-9404. [(LT) two terms-

lagrangian-no detonation].

Table 3. Hugoniot Data (c

0

, s) and Sensitivity Constants (Ec, V

2

d)

for PBX-9404 and CompB

Material

density

g=cm

3

c

0

(8)

mm=ms

s

(8)

Ec

(12)

MJ=mn

2

V

2

d

(4)

mm

3

m s

ÿ2

PBX-9404

1.84

2.43

2.56

0.64±0.70

16 2

CompB

1.712

2.71

1.86

1.5±2.1

27 2

Figure 3. Impact of a copper jet on PBX-9404. [(LT) two terms-

lagrangian-no detonation].

Figure 5. Comparison of three criteria for PBX-9404 impacted by jets

or round-nosed cylinders.

120 F. Peugeot, M. Quidot, and H. N. Presles

Propellants, Explosives, Pyrotechnics 23, 117±122 (1998)

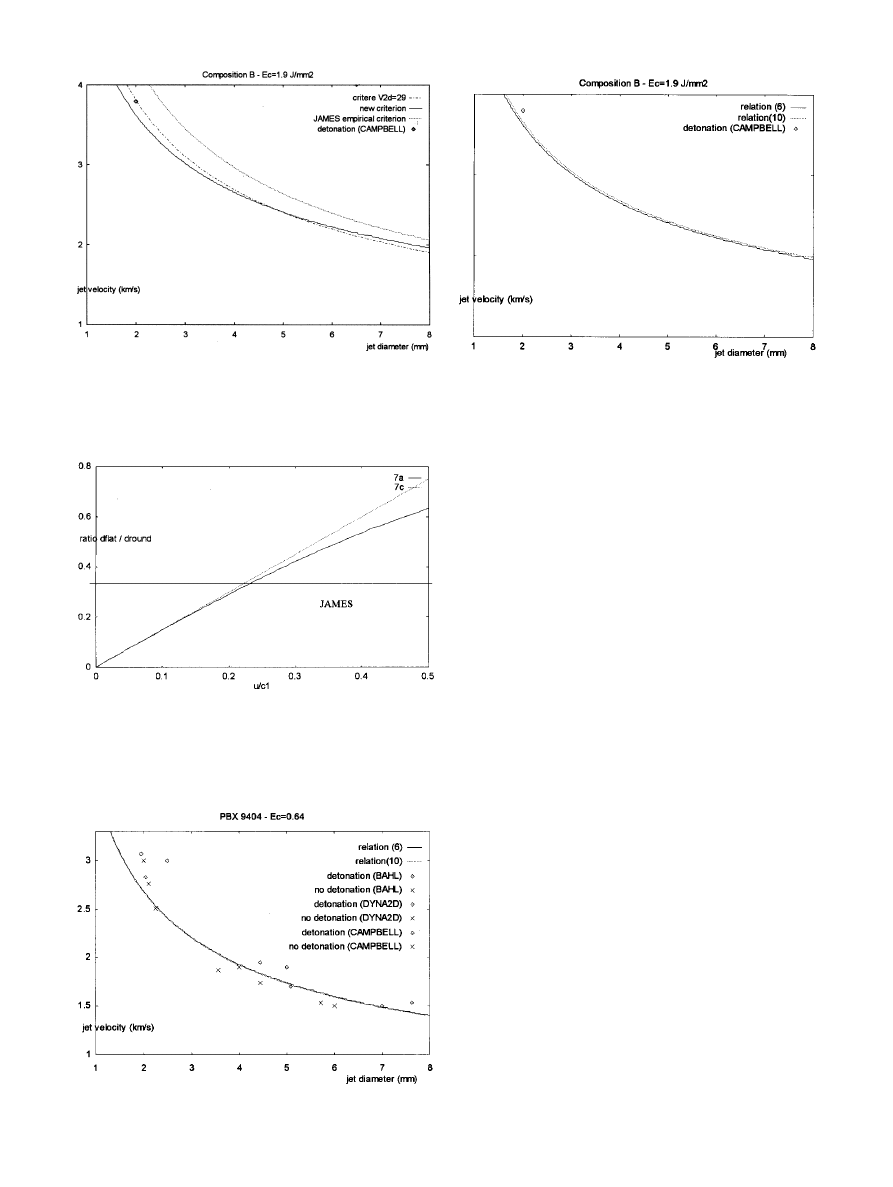
As it can be seen on Figures 8 and 9, curves obtained with

relations (10) and (6) are very close.

5.3 Conclusions

Finally, our new criterion has led to an analytical rela-

tionship for the initiation threshold of explosives submitted

to jet impact. It has been validated by comparison with

experimental data and computations for two explosive

compositions, PBX-9404 and Comp B.

As the agreement is less good with small diameter jets,

our criterion can only be applied to the low and inter-

mediate impact velocity range. The upper velocity region

will be the subject of further studies.

6. References

(1) F. E. Walker and R. J. Wasley, Explosivstoffe 17, 9 (1969).

(2) Y. De Longueville, C. Fauquignon, and H. Moulard, ``Initiation

of Several Condensed Explosives by a Given Duration Shock

Wave'', Sixth Symposium (International) on Detonation (1976).

(3) H. Moulard, ``Critical Condition for Shock Initiation of Deton-

ation by Small Projectile Impact'', Seventh Symposium (Inter-

national) on Detonation (1981).

(4) H. R. James, ``Critical Energy Criterion for the Shock Initiation

of Explosives by Projectile Impact'', Propellants, Explosives,

Pyrotechnics 13, 35 (1988).

(5) H. R. James and D. B. Hewitt, ``Critical Energy Criterion for the

Initiation of Explosives by Spherical Projectiles'', Propellants,

Explosives, Pyrotechnics 14, 123 (1989).

(6) D. Pirotais, J. P. Plottard, and J. C. Braconnier, ``Numerical

Simulation of Jet Penetration of HMX and TATB Explosives'',

Eight Symposium (International) on Detonation (1985).

(7) C. L. Mader and G. H. Pimbley, ``Jet Initiation of Explosives'',

LASL Report LA-8647 (1981).

(8) K. L. Bahl, H. C. Vantine, and R. C. Weingart, ``The Shock Initiation

of Bare and Covered Explosives by Projectile Impact'', Proc.

Seventh Symposium (International) on Detonation, (1981), p. 858.

(9) H. R. James, ``Predicting the Response of Explosives to Attack

by High Density Shaped Charge Jets'', J. Energ. Mater.7(4±5),

243±265 (1989).

(10) J. O. Hallquist,, ``User's Manual for DYNA2D'', LLNL 18756

(1984).

Figure 8. Comparison of relation (6a) and (10) for PBX-9404.

Figure 9. Comparison of relation (6) and (10) for Comp B.

Figure 6. Comparison of three criteria for CompB impacted by jets.

Figure 7. Comparison ¯at-nosed and round-nosed projectile.

Propellants, Explosives, Pyrotechnics 23, 117±122 (1998)

An Analytical Extension of the Critical Energy Criterion 121

(11) E. L. Lee and C. M. Tarver, ``Phenomenological Model of Shock

Initiation in Heterogeneous Explosives'', Phys. Fluids 23 (12),

(1980).

(12) C. M. Tarver, J. O. Hallquist, and L. M. Erickson ``Modeling

Short Pulse Duration Shock Initiation of Solid Explosives'', Proc.

Eight Symposium (International) on Detonation, (1985), p. 951.

(13) M. Quidot, ``Un modeÁle simple de cineÂtique de reÂaction. Appli-

cation aux explosifs insensibles'', Revue Scienti®que et Techni-

que de la DeÂfense, 1995±4 (1995).

Acknowledgement

The authors would like to acknowledge the support and

encouragements of Mr J. P. Romain of the ENSMA Deto-

nation and Combustion Laboratory.

(Received September 24, 1996; Ms 65=96)

122 F. Peugeot, M. Quidot, and H. N. Presles

Propellants, Explosives, Pyrotechnics 23, 117±122 (1998)