Shock Waves (2000) 10: 95–101

Pitot pressures of correctly-expanded and underexpanded

free jets from axisymmetric supersonic nozzles

H. Katanoda, Y. Miyazato, M. Masuda, K. Matsuo

Graduate School of Engineering Sciences, Kyushu University, Kasuga, Fukuoka 816-8580, Japan

Received 4 February 1998 / Accepted 27 August 1998

Abstract. The structures of the axisymmetric free jets from supersonic nozzles with the exit Mach numbers

of 1.5 and 2.0 are studied with special attention to the decay of the Pitot pressures downstream of the

Mach disk. The Pitot pressure probe and schlieren method are used in the experiments to diagnose the

flowfield. A TVD numerical method is also applied to the Euler equations, and the computed jet structures

are compared with experiments. In the underexpanded jet, the experimentally obtained Pitot pressure

near the jet centerline is found to substantially recover downstream of the Mach disk. By comparing the

numerical computation, this phenomenon is thought to be caused by the turbulent momentum transfer to

the central region from the region outside the slip line where the stagnation pressure loss is small.

Key words: Compressible flow, Supersonic jet, Axisymmetric flow, Pitot pressure

1 Introduction

Supersonic free jets are widely used in engineering applica-

tions such as sootblowers ( Moskal et al. 1993), jet burners

( Shimada et al. 1994) and rocket engines ( Neilson et al.

1969). The sootblower is used to remove the fireside de-

posit in kraft recovery boilers, where high pressure steam

accelerated to supersonic speed by a nozzle washes off the

deposits on the heat transfer surfaces of the boiler. The

cleaning power of the jet is thought to be enhanced by the

increase in the local total pressure, which is related to the

Pitot pressure.

Much work has been done experimentally ( Ashkenas

and Sherman 1966, Driftmyer 1972), analytically ( Tam

1972) and numerically ( Dash and Thorpe 1981,Kobayashi

et al. 1984, Saito et al. 1986, Viswanathan and Sankar

1995, Thies and Tam 1996) on the structure of axisym-

metric supersonic free jets. However, most of these studies

have been restricted to the correctly-expanded jets from

supersonic nozzles or the underexpanded jets from sonic

nozzles, and little information is available on the under-

expanded free jets from supersonic nozzles ( Love et al.

1959,Fox 1974). Although the decay of the Pitot pressure

in underexpanded supersonic free jets is very important

for the design of the sootblower nozzles, its characteristics

downstream of the Mach disk are not well understood.

The present paper describes the distributions of the

Pitot pressures both in the correctly-expanded and the

underexpanded supersonic free jets issuing from axisym-

Correspondence to: H. Katanoda

(e-mail: [e-mail])

metric supersonic nozzles, with special emphasis on its

decay downstream of the potential core.

2 Experimental setup and method

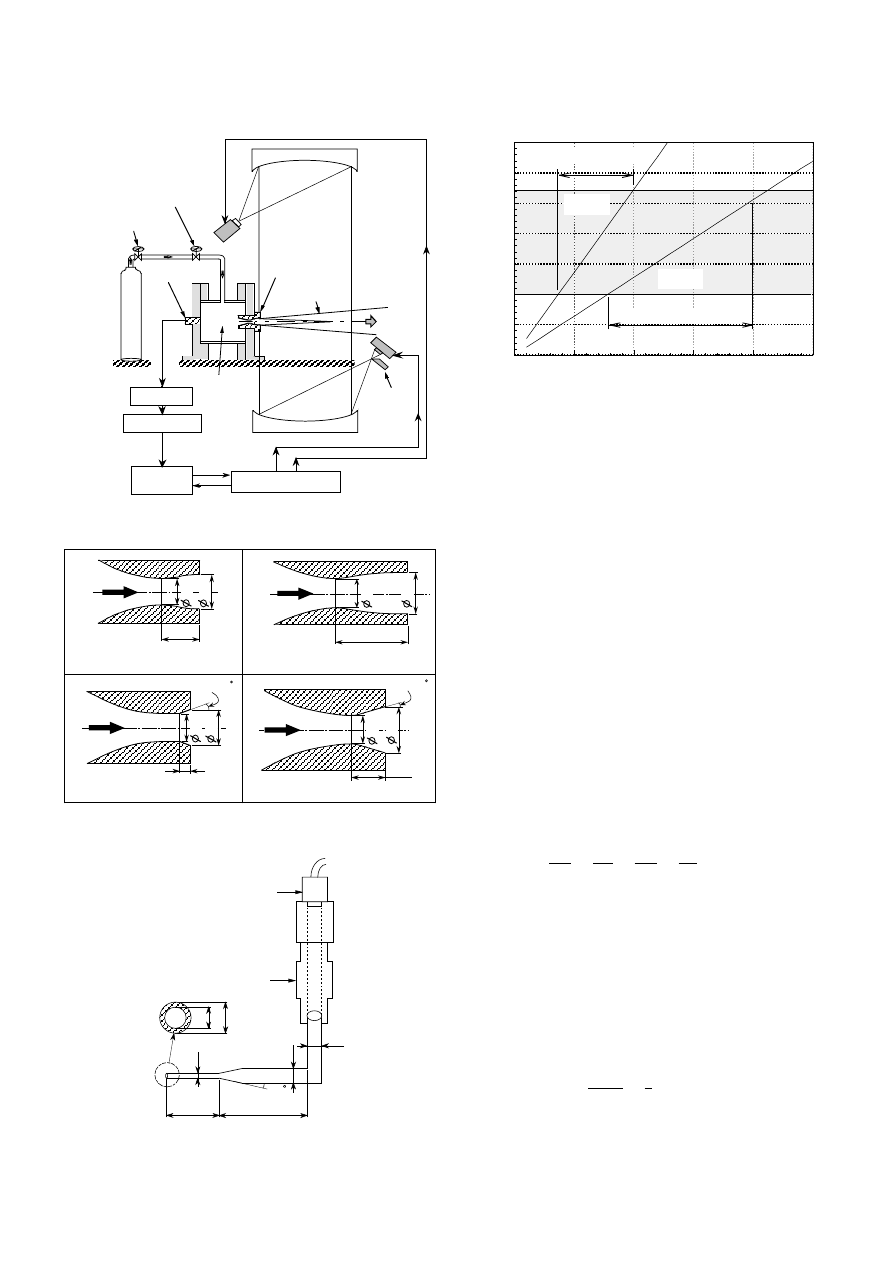
A schematic diagram of the experimental setup is shown

in Fig. 1. The working gas was nitrogen, which, in a high

pressure reservoir, was supplied to the plenum chamber

through the pressure control valve. After the gas was stag-

nated in the plenum chamber, it was accelerated by the

test nozzle and discharged into the atmosphere. The pres-

sure in the plenum chamber was carefully adjusted by the

pressure control valve so that the pressure ratio across the

nozzle was kept constant at a desired value.

Four axisymmetric nozzles used in the present experi-

ments are shown in Fig. 2. The number 1.5 or 2.0 in the

names indicates the exit Mach number calculated by the

one-dimensional isentropic theory. The nozzles labeled as

L had a supersonic part contoured by an approximate the-

ory ( Foelsch 1949), and those labeled as C had a conical

supersonic part with the divergence half angle of 7.5

◦

. The

conical nozzles are often used in sootblowers because of

their manufacturing simplicity. In the design of the con-

toured nozzle, the displacement effect of the boundary

layer was not considered. When operating these nozzles

under a correct-expansion pressure ratio, the stagnation

pressure was adjusted by monitoring the Pitot pressure at

the nozzle exit plane in order to match the static pressure

at the nozzle exit with the ambient pressure.

The jet flowfield was measured by the standard Pitot

pressure probe shown in Fig. 3. The tip of this probe had a

1.0-mm outer diameter, a 0.9-mm inner diameter and was

96

H. Katanoda et al.: Pitot pressures of correctly-expanded and underexpanded free jets

Valve

Nozzle

Supersonic jet

Light source

Camera

Knife edge

Mirror

Mirror

Amplifier

A/D converter

Signal controller

N gas reservoir

2

Pressure
control valve

Personal
computer

Plenum
chamber

transducer

Pressure

Fig. 1. Experimental setup

Nozzle 2.0C

6.9

7.8

6.0

Flow

(d)

=7.5

θ

(a)

(b)

(c)

Nozzle 1.5L

6.0

6.5

Flow

8.6

Nozzle 1.5C

14.8

7.8

6.0

Flow

Nozzle 2.0L

6.0

6.5

2.1

Flow

=7.5

θ

Fig. 2. Nozzle geometry

1.0

5.0

30

50

5.0

Pressure

transducer

Joint

0.9

1.0

30

Fig. 3. Pitot pressure probe

0.5

1.0

1.5

2.0

2.5

3.0

3.5

0

5

10

15

20

25

Range of experiments

Range of experiments

M

d

=2.0

M

d

=1.5

p

e

/ p

b

p

o1

/ p

b

Fig. 4. Experimental conditions

30 mm in length. The Pitot pressure probe was mounted

on the traversing device to scan the flowfield. As shown

in Fig. 1, the spark schlieren method was used to visual-

ize the flowfield. The spark light source had a duration of

20 ns, which is shorter than the typical turbulence charac-

teristic time in the jet. This means that the present system

visualized the instantaneous pattern of the flowfield.

The experimental conditions are summarized in Fig. 4.

The nozzle with the design exit Mach number M

d

= 1.5

was tested with the ratio of the stagnation pressure p

◦1

to the back pressure p

b

from 4.0 to 10, and these pressure

ratios corresponded to the ratio of the nozzle exit pressure

p

e

to p

b

from 1.0 to 2.7. For the nozzle with M

d

= 2.0, the

range of the tests was p

◦1

/p

b

= 8.0 to 20 (p

e

/p

b

= 1.0 to

2.5).

3 Numerical method

The structure of the supersonic free jets was also obtained

numerically under the same conditions as for the present

experiments. The basic equations are the Euler equations

in cylindrical coordinates, and are written as:

∂U

∂t

+

∂F

∂x

+

∂G

∂r

+

W

r

= 0 ,

(1)

U =






ρ

ρu

ρv

e




 , F =






ρu

ρu



+ p

ρuv

(e + p) u




 ,

G =






ρv

ρuv

ρv



+ p

(e + p) v




 , W =






ρv

ρuv

ρv



(e + p) v




 ,

e =

p

γ − 1

+

ρ
2

(u

2

+ v

2

) ,

where x is the axial distance from the nozzle exit plane,

r the radial distance from the jet centerline, t the time,

H. Katanoda et al.: Pitot pressures of correctly-expanded and underexpanded free jets

97

p the pressure, ρ the density, u the axial velocity, v the

radial velocity and e the sum of the internal energy and the

kinetic energy per unit volume, respectively. The specific

heat ratio γ was taken as 1.4. Equation (1) was solved with

the equation of state for an ideal gas. To capture shock

waves in underexpanded free jets, numerical fluxes were

evaluated using the Chakravarthy–Osher-type TVD finite-

difference scheme ( Yee 1987). Numerical solutions were

advanced in time by using an explicit three-step Runge–

Kutta method.

The computational domain consists of both the inside

and the outside of the nozzle. The following boundary con-

ditions are assumed: perfect slip on the nozzle wall, zero

normal gradient on the outermost surface of the domain

and axial symmetry on the flow centerline. At the nozzle

throat, physical quantities are calculated by the approx-

imate theory of the transonic flow with a circular throat

( Shapiro 1954).

4 Results and discussion

4.1 Experimental and numerical flow visualization

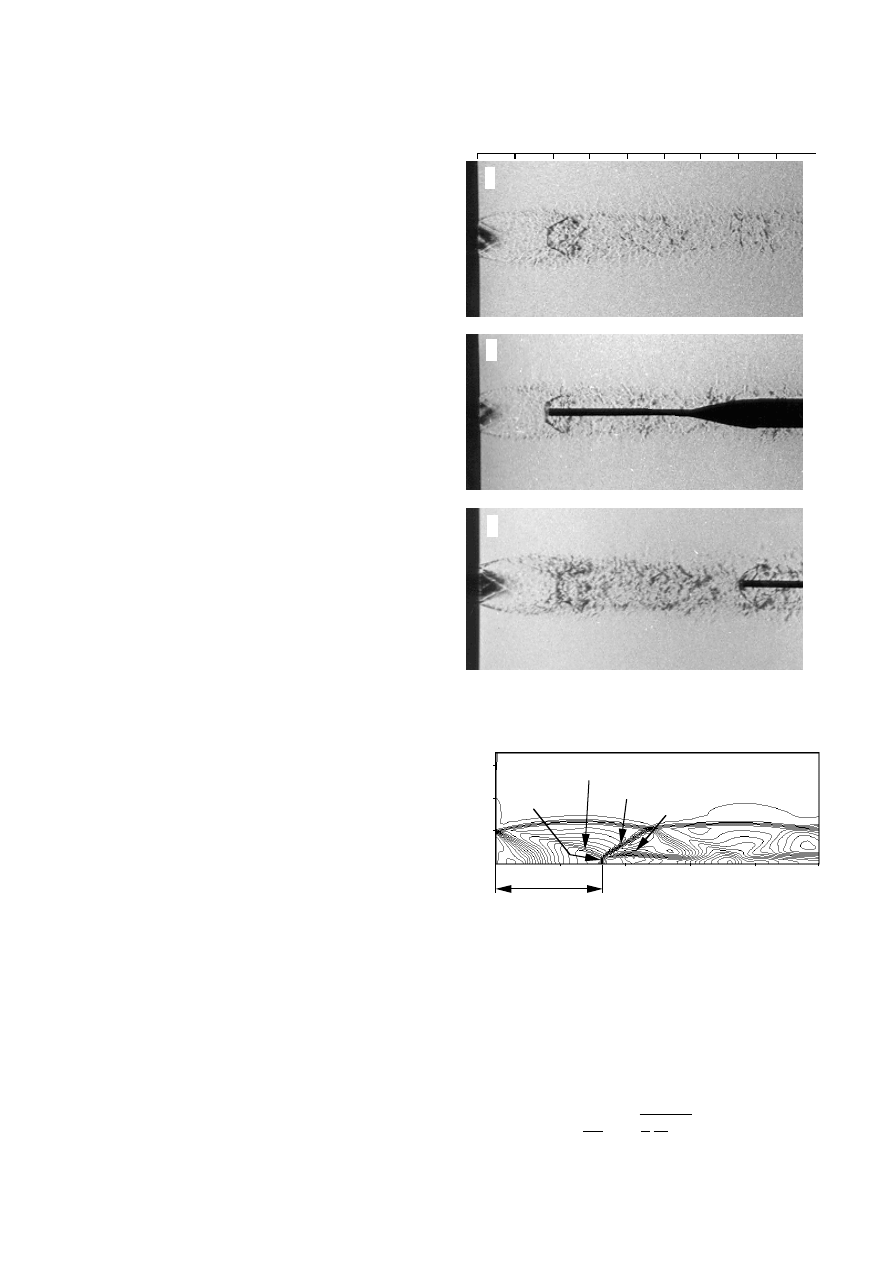
Figure 5 shows three schlieren photographs of the under-

expanded free jets from the nozzle 1.5L. They were taken

for the same nozzle pressure ratio p

◦1

/p

b

= 8.0, and the

corresponding exit pressure ratio p

e

/p

b

was 2.18. The scale

shown in the upper part of the figure is the axial distance

x from the nozzle exit normalized by the nozzle exit di-

ameter D. In Fig. 5a, the Pitot pressure probe is not in-

serted and the Mach disk is observed at x/D = 1.78. In

Fig. 5b and c, the Pitot pressure probe is inserted on the

jet axis with its tip at x/D = 1.80 and 6.67, respectively.

By comparing Fig. 5a and b, the Mach disk location and

diameter do not seem to be affected by the probe. As

shown in Fig. 5c, the bow shock wave is observed in front

of the probe, indicating the flow at this point as being

supersonic. The bow shock wave near the flow centerline,

however, is almost normal to the axis, so that the Pitot

pressure probe measures the stagnation pressure behind

the normal shock wave.

Figure 6 shows the density contours obtained by the

present numerical simulation for the nozzle 1.5L with the

pressure ratio p

◦1

/p

b

= 8.0 (p

e

/p

b

= 2.18). The numeri-

cally obtained flow parameters on the jet axis fluctuated at

each calculation step. The calculated density was, there-

fore, averaged over 3000 successive calculation steps. The

Mach disk, barrel shock wave, reflected shock wave and

slip line are clearly seen in this figure. The downstream

distance from the nozzle exit to the Mach disk, x

m

/D,

equals 1.65 and is close to the value 1.78 obtained exper-

imentally (Fig. 5a).

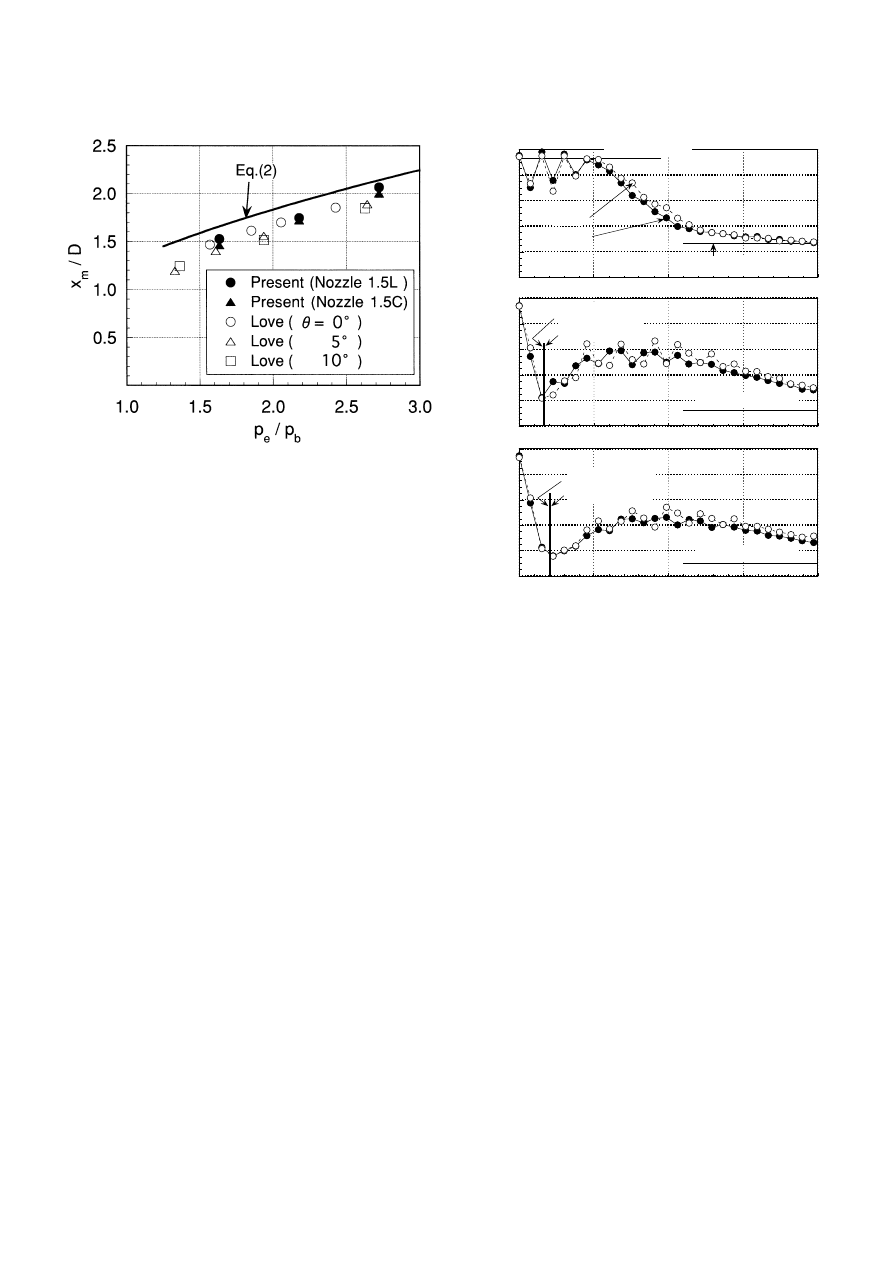
4.2 Mach disk

The location of the Mach disk was measured from schlieren

photographs for various experimental conditions, and the

normalized distance x

m

/D is plotted against the nozzle

x/D

1

2

3

4

5

6

7

8

0

a

b

c

Fig. 5a–c. Schlieren photographs of supersonic free jets; nozzle

1.5L, p

◦1

/p

b

= 8.0 (p

e

/p

b

= 2.18). a Without Pitot tube; b

Pitot tube at x/D = 1.80; c Pitot tube at x/D = 6.67

0

x/D

Mach disk

Barrel shock wave

Reflected shock wave

Slip line

1

2

3

4

5

x /D

m

0.5

1.0

1.5

r/D

Fig. 6. Computed density contours; nozzle 1.5L, p

◦1

/p

b

= 8.0

(p

e

/p

b

= 2.18)

exit pressure ratio p

e

/p

b

in Fig. 7 for the nozzles 1.5L and

1.5C. This figure also includes the previous experimental

data ( Love et al. 1959) for supersonic air jets from three

nozzles with the exit Mach number 1.5 and a divergence

half angle θ at the exit of 0

◦

, 5

◦

and 10

◦

. Driftmyer (1972)

summarized experimental results and proposed the follow-

ing empirical formula for the Mach disk location:

x

m

D

=

r

γ

2

p

e

p

b

M

2

d

.

(2)

98

H. Katanoda et al.: Pitot pressures of correctly-expanded and underexpanded free jets

Fig. 7. Measured Mach disk locations; nozzles 1.5L and 1.5C

The solid line in Fig. 7 is the result calculated using the

above formula (2). The present experimental data agree

well with the previous experiments for the nozzle with θ

= 0

◦

. It is also shown that x

m

/D becomes smaller with

the increase in θ for the same exit pressure ratio. In the

previous data, x

m

/D decreases by 5–8% with the increase

in θ from 0

◦

to 5

◦

. On the other hand, the present x

m

/D

decreases by 2–4% with the increase in θ from 0

◦

to 7.5

◦

.

Equation (2) also predicts slightly larger values of x

m

/D

than the experimental data, even for the nozzle with θ =

0

◦

, which gives the largest x

m

/D.

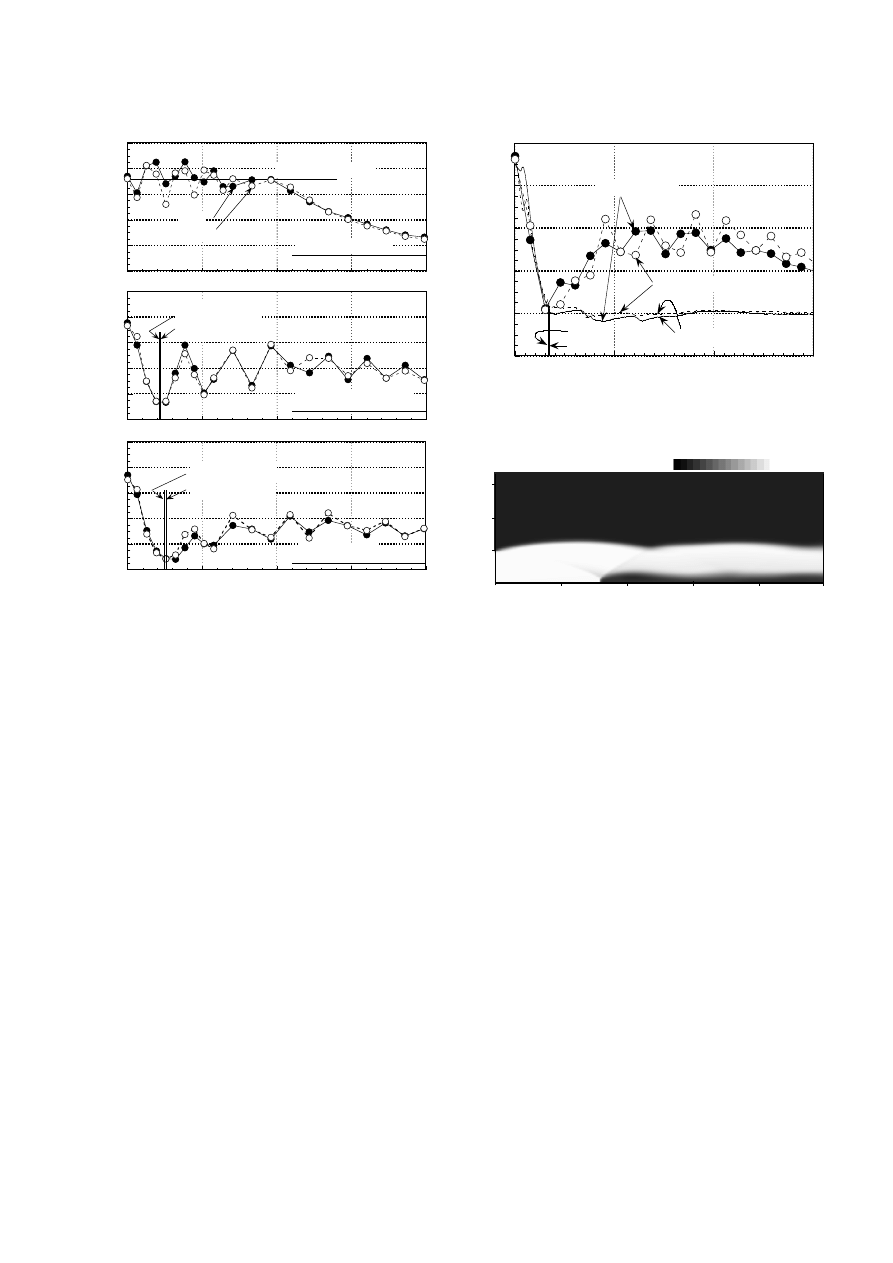
4.3 Pitot pressure

The Pitot pressure distributions were measured along the

centerline of nozzles 1.5L and 1.5C, and the results are

plotted in Fig. 8 as a function of x/D. In this figure, p

i

is

the Pitot pressure, p

◦1

the plenum chamber pressure, and

p

b

the back pressure, respectively. Figure 8a corresponds

to the flowfield of the correctly-expanded jet. The p

ie

in

this figure is the Pitot pressure at the nozzle exit calcu-

lated by the one-dimensional theory, and is shown to agree

well with the experiments for both nozzles. Although the

pressure ratio across the nozzle is correct, the jet immedi-

ately downstream of the nozzle exit indicates the existence

of compression and expansion waves. As mentioned pre-

viously, nozzle 1.5L has a wall contour computed by an

approximate theory. The Pitot pressure distribution for

this nozzle in the range 0 < x/D <∼ 5, however, suggests

that the weak compression and expansion waves still exist

in the flowfield. Nozzle 1.5L was designed to be used as a

sootblower nozzle so that the nozzle length is limited. Con-

sequently, it is probably not possible to cancel expansion

and compression waves generated in the nozzle completely.

In addition to this, Fourguette et al. (1991) observed a

similar phenomenon through their experiments with the

contoured axisymmetric nozzle for the exit Mach number

0

0.2

0.4

0.6

0.8

1.0

x

m

/ D for 1.5C

x

m

/ D for 1.5L

p

b

/ p

o 1

=0.13

p

i

/ p

o1

0

0.2

0.4

0.6

0.8

1.0

1.5L

1.5C

p

ie

/ p

o 1

=0.93

p

b

/ p

o 1

=0.27

p

i

/ p

o1

0.2

0.4

0.6

0.8

1.0

0

5

10

15

20

p

i

/ p

o1

x/D

x

m

/ D for 1.5C

x

m

/ D for 1.5L

p

b

/ p

o 1

=0.10

a

b

c

Fig. 8a–c. Pitot pressure distributions along the centerline;

nozzles 1.5L and 1.5C. a p

◦1

/p

b

= 3.7; b p

◦1

/p

b

= 8.0; c

p

◦1

/p

b

= 10

of 1.5. They attributed this to the weak shock waves gen-

erated in the nozzle. The shock waves in the nozzles were

discussed for the conical ( Migdal and Kosson 1965) and

contoured ( Legge and Dettleff 1986) nozzles, respectively.

Their results indicated that a weak shock wave was gen-

erated immediately downstream of the nozzle throat and

this could not be eliminated when the nozzle shape was ax-

isymmetric. The expansion and compression wave pattern

shown in Fig. 8a is probably attributed to this effect. Due

to this effect, the Pitot pressures fluctuate from the nozzle

exit to x/D ∼ 5, where the potential core is terminated.

Downstream of x/D ∼ 5, the Pitot pressures of nozzles

1.5L and 1.5C decrease gradually with x/D and approach

the back pressure. In this region, the effect of turbulence

generated in the jet boundary extends to the centerline

and decreases the Pitot pressure. Although the schlieren

photographs do not reveal the flow structure near the cen-

terline, the effect of turbulence can be seen qualitatively

in Fig. 5.

Figure 8b,c shows the jet for the underexpanded pres-

sure ratios. The x

m

/D in this figure is the Mach disk loca-

tion obtained from the schlieren measurements. As shown

in this figure, the Pitot pressure decreases rapidly imme-

diately downstream of the nozzle exit, and takes the min-

imum value at x/D = 1.5 ∼ 2. This x/D value corre-

sponds to the Mach disk location as observed from the

schlieren photographs. The Pitot pressure distributions

H. Katanoda et al.: Pitot pressures of correctly-expanded and underexpanded free jets

99

0

0.2

0.4

0.6

0.8

1.0

p

i

/ p

o1

x

m

/ D for 2.0C

x

m

/ D for 2.0L

p

b

/ p

o 1

=0.06

0

0.2

0.4

0.6

0.8

1.0

p

i

/ p

o1

2.0L

2.0C

p

ie

/ p

o 1

=0.72

p

b

/ p

o 1

=0.14

0.2

0.4

0.6

0.8

1.0

0

5

10

15

20

p

i

/ p

o1

x/D

p

b

/ p

o 1

=0.05

x

m

/ D for 2.0C

x

m

/ D for 2.0L

a

b

c

Fig. 9a–c. Pitot pressure distributions along the centerline;

nozzles 2.0L and 2.0C. a p

◦1

/p

b

= 7.4; b p

◦1

/p

b

= 16; c

p

◦1

/p

b

= 20

downstream of the Mach disk indicate that the compli-

cated compression and expansion wave pattern exists in

this region. In particular, the Pitot pressure increases af-

ter the Mach disk under the underexpansion condition.

This can not be explained by the inviscid flow theory.

A similar phenomenon with the Pitot pressure recovering

downstream of the Mach disk was also reported by Don-

aldson et al. (1971), who investigated the underexpanded

free air jet obtained from the convergent nozzle. We shall

discuss this below.

The Pitot pressure distributions were also measured

for nozzles 2.0L and 2.0C, and the results are plotted in

Fig. 9. As shown in this figure, the behavior of the Pitot

pressure does not differ greatly from Fig. 8, except that

the compression and expansion wave pattern downstream

of the Mach disk seems more distinct than in Fig. 8. The

Pitot pressure downstream of the Mach disk is also seen

to increase with x/D.

To investigate the recovery of the Pitot pressure down-

stream of the Mach disk, the jet flowfield was computed

with the method described in the previous section. The

numerical Pitot pressure was calculated by assuming the

normal shock wave standing in front of the tube when the

local flow speed was supersonic; when the local flow was

subsonic, the numerical Pitot pressure was simply taken

as the local stagnation pressure. As described before, the

0.2

0.4

0.6

0.8

1.0

0

5

10

15

p

i

/ p

o1

x/D

Nozzle 1.5L

Nozzle 1.5C

Computation

x

m

/ D for 1.5C

x

m

/ D for 1.5L

Fig. 10. Measured and computed Pitot pressure distributions;

nozzles 1.5L and 1.5C, p

◦1

/p

b

= 8.0 (p

e

/p

b

= 2.18)

x/D

0.5

1.0

1.5

r/D

0

1

2

3

4

5

0.125

1.0

p /p

0

01

Fig. 11. Computed total pressure spatial distribution; nozzle

1.5L, p

◦1

/p

b

= 8.0 (p

e

/p

b

= 2.18)

solutions of 3000 successive time steps were averaged to

get the numerical Pitot pressure. The Pitot pressure dis-

tributions along the jet centerline are shown in Fig. 10

for the nozzle 1.5L and 1.5C. In this figure, both com-

puted and experimental results are shown for the pres-

sure ratio p

◦1

/p

b

= 8.0 (p

e

/p

b

= 2.18). The experimen-

tal Pitot pressure decreases rapidly from the nozzle exit

to the Mach disk, and agrees reasonably well with the

computation. The computed results in this region, how-

ever, show a slight increase in the Pitot pressure at about

x/D ∼ 0.5. This increase is observed even in the flow from

the contoured nozzle 1.5L, and is probably attributed to

the shock wave generated in the nozzle. Downstream of

the Mach disk, a large discrepancy is observed between the

experimental and computed results; the computed Pitot

pressure does not increase there.

The total pressure distributions are also computed for

the nozzle 1.5L with the pressure ratio p

◦1

/p

b

= 8.0 (p

e

/p

b

= 2.18), and the results are shown in Figs. 11 and 12. Fig-

ure 11 presents the total pressure field, and shows that the

total pressure decreases abruptly downstream of the Mach

disk. This low total pressure region, however, is restricted

to the vicinity of the centerline, and the decrease in total

pressure is small in the region outside the slip line. This

tendency is quantitatively shown in Fig. 12, where the to-

tal pressures are plotted as a function of x/D for three

different radial positions. In Fig. 12, r is the radial dis-

tance measured from the centerline and r

m

the radius of

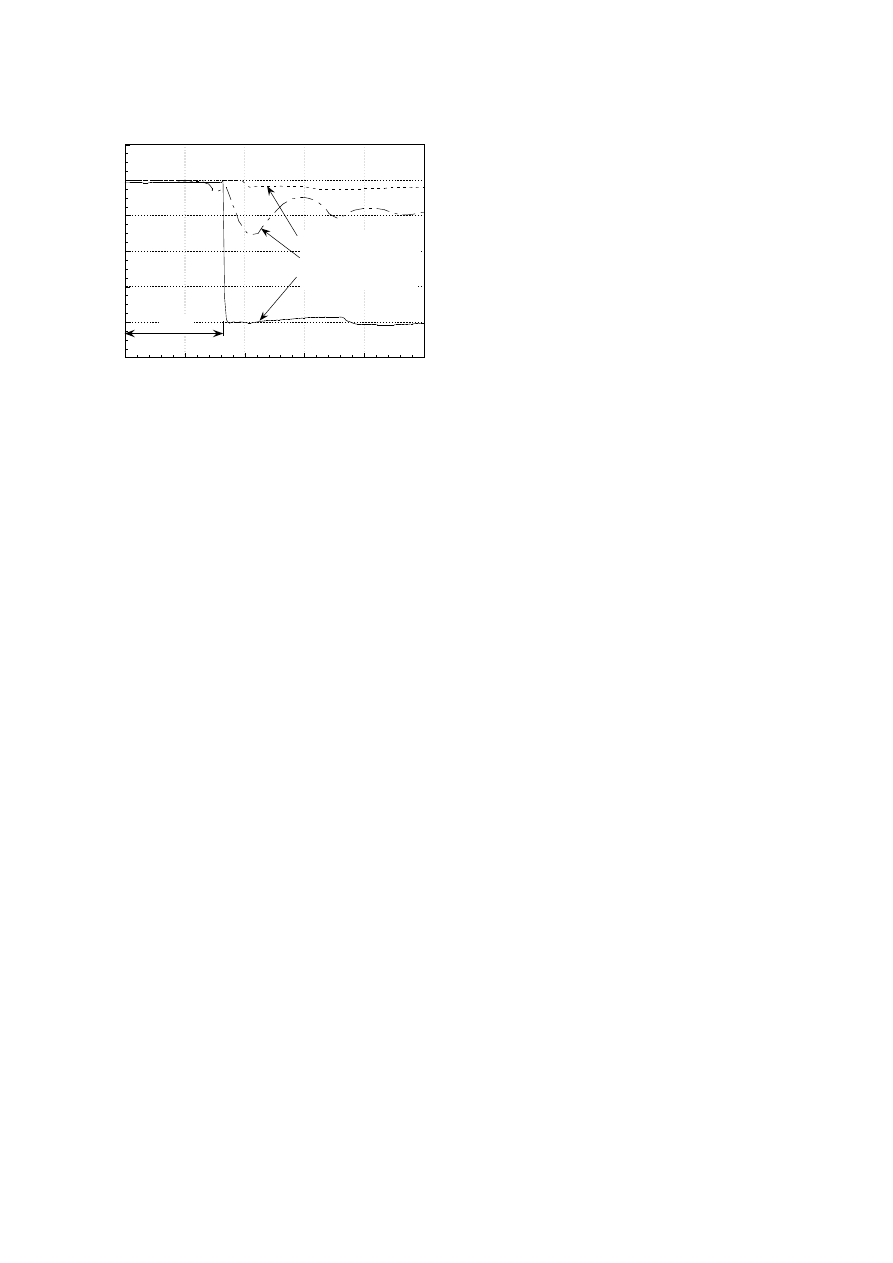
100

H. Katanoda et al.: Pitot pressures of correctly-expanded and underexpanded free jets

0.2

0.4

0.6

0.8

1.0

1.2

0

1

2

3

4

5

p

o

/ p

o1

x /D

r /D=0.35 ( > r

m

/D )

r /D=0.20 ( > r

m

/D )

x

m

/ D

r /D=0 ( < r

m

/D )

Fig. 12. Computed total pressure in the jet; nozzle 1.5L

p

◦1

/p

b

= 8.0 (p

e

/p

b

= 2.18)

the Mach disk. According to the density contours shown

in Fig. 6, the Mach disk is formed at x

m

/D = 1.65 with

its radius r

m

/D = 0.1. The total pressure on the center-

line decreases from 1.0 to 0.2 across the Mach disk, and

this decrease agrees well with the calculation by the one-

dimensional normal shock relations. The total pressure at

r/D = 0.2, however, does not decrease appreciably after

the Mach disk, and it decreases and increases periodically

due probably to the complicated expansion and compres-

sion waves generated in the jet. At r/D = 0.35, the total

pressure decreases at the position of the reflected shock

wave x/D ∼ 2, but the amount of the decrease is not large.

These numerical results for the inviscid jet suggest that

the Pitot pressure recovery observed in the experiments

downstream of the Mach disk is caused by the transfer

of momentum from the outer high total pressure region

to the central part of the jet. This momentum transfer is

probably caused by the difference in velocity across the

slip line. Previous experiments on the axisymmetric sonic

jet of air (Yip et al. 1989) indicated that the large-scale

vortical structure existed along the slip line. The helical or

flapping instability wave of the jet generated by acoustic

disturbances near the nozzle exit (Tam et al. 1994) may

also affect the momentum transfer.

5 Concluding remarks

The Pitot pressure distributions have been studied both

experimentally and numerically for the axisymmetric free

jets obtained from supersonic nozzles. The tested nozzles

had an exit Mach number of 1.5 and 2.0 with contoured

or 7.5

◦

half–angle conical supersonic part. Results of the

present study are summarized as follows.
(1) With the correct expansion pressure ratio, the jet from

both contoured and conical nozzles show a similar wave

pattern; the compression and expansion waves are gen-

erated in the jet resulting in the cell structure in the

potential core region, and the Pitot pressure decreases

with the increase in axial distance downstream of the

potential core. The flow pattern of the contoured noz-

zle does not differ greatly from that of the conical noz-

zle. This is probably attributed to the length of the

contoured nozzle being too short to cancel the com-

pression and expansion waves generated in the nozzle

and also to the axisymmetric geometry of the nozzle.

(2) When the pressure ratio across the nozzle exceeds the

correct expansion ratio, the Pitot pressure is found to

recover significantly downstream of the potential core.

To interpret this, the Pitot and total pressure distri-

butions were computed with the Euler equations. The

results indicate that, although the Pitot pressure in

the vicinity of the jet centerline does not increase after

the first Mach disk, the total pressure outside the slip

line is almost equal to its inviscid value. This suggests

that the experimentally observed increase in the Pitot

pressure downstream of the Mach disk is attributed to

the transfer of momentum from the region outside the

slip line to the central part of the jet.

Acknowledgements. The authors would like to express their

thanks to Mr. J. Tanaka and Mr. H. Saito for their assistance

in the experimental works.

References

Ashkenas H, Sherman FS (1966) Experimental methods in rar-

efied gas dynamics. Rarefied Gas Dynamics, 2, Academic

Press, New York, pp 84–105

Dash SM, Thorpe RD (1981) Shock-capturing method for one-

and two-phase supersonic exhaust flow. AIAA J 19:842–851

Donaldson CD, Snedeker RS (1971) A study of free jet im-

pingement. Part I. Mean properties of free and impinging

jets. J Fluid Mech 45:281–324

Driftmyer RT (1972) A correlation of freejet data. AIAA J

10:1093–1095

Foelsch K (1949) The analytical design of an axially symmetric

Laval nozzle for a parallel and uniform jet. J Aeronaut Sci

16:161–188

Fourguette DC, Mungal MG, Dibble RW (1991) Time evolu-

tion of the shear layer of a supersonic axisymmetric jet.

AIAA J 29:1123–1130

Fox JH (1974) On the structure of jet plumes. AIAA J 12:105–

107

Kobayashi H, Nakagawa T, Nishida M (1984) Density mea-

surements in freejets by laser interferometry. Proc 14th

Int Symp on Rarefied Gas Dynamics, University of Tokyo

Press, pp 501–508

Legge H, Dettleff G (1986) Pitot pressure and heat-transfer

measurements in hydrazine thruster plumes. J Spacecraft

and Rockets 23:357–362

Love ES, Grigsby CE, Lee LP, Woodling MJ (1959) Exper-

imental and theoretical studies of axisymmetric free jet.

NASA TR R-6

Migdal D, Kosson R (1965) Shock prediction in conical nozzle.

AIAA J 3:1554–1556

Moskal TE, Bunton MA, Jordan CA (1993) Results of labora-

tory testing and field trials of improved sootblower nozzles.

TAPPI 1993 Engineering Conference Proceedings, TAPPI

Press, Atlanta, pp 963–967

H. Katanoda et al.: Pitot pressures of correctly-expanded and underexpanded free jets

101

Neilson JH, Gilchrist A, Lee CK (1969) Control forces in rocker

nozzles produced by a secondary gas stream inclined at

various angles to the nozzle axis. J Mech Eng Sci 11:175–

180

Saito T, Nakatsuji H, Teshima K (1986) Numerical simula-

tion and visualization of freejet flow-fields. Trans Japan

Soc Aero Space Sci 34:161–165

Shapiro AH (1954) The dynamics and thermodynamics of com-

pressible fluid flow. The Ronald Press, New York, vol 2,

pp 826–833

Shimada S, Koyama T, Sakusabe K (1994) Development

of drying-disintegration system by high-temperature jet.

Shigen-to-Sozai 110:1179–1183

Tam CKW (1972) On the noise of a nearly ideally expanded

supersonic jet. J Fluid Mech 51:69–96

Tam CKW, Ahuja KK, Jones III RR (1994) Screech tones from

free and ducted supersonic jets. AIAA J 32:917–922

Thies AT, Tam CKW (1996) Computation of turbulent ax-

isymmetric and nonaxisymmetric jet flows using the k-ε

model. AIAA J 34:309–316

Viswanathan K, Sankar LN (1995) Toward the direct calcu-

lation of noise: fluid/acoustic coupled simulation. AIAA J

33:2271–2279

Yee HC (1987) Upwind and symmetric shock-capturing

schemes. NASA TM-89464

Yip B, Lyons K, Long M, Mungal MG, Barlow R, Dibble R

(1989) Visualization of supersonic underexpanded jet by

planar Rayleigh scattering. Phys Fluids A 1:1449–1449