- 1 -

LSD2000: International Workshop on Limit State Design in Geotechnical Engineering

Melbourne, Australia, 18 November 2000

Stability analysis for shallow foundations -

Eurocode 7 and the new generation of DIN codes

B. Schuppener
Federal Waterways Engineering and Research Institute, Karlsruhe, Germany

U. Smoltczyk
Böblingen, Germany

ABSTRACT: The relevant features of Eurocodes 0 and 7 – the concept of limit states and the partial
factor method – are described. In particular it is shown how the factors of safety are to be introduced
in the three approaches proposed by the new version of Eurocode 7 for the verification of ultimate
limit states. The approach adopted in the new generation of geotechnical DIN codes and the basic
principles of the new DIN 1054 are then presented. The main features are that the partial factors on the
actions of the ground and of the structure have the same value and only one single calculation is re-
quired to verify a limit state. Moreover Germany favours the approach, in which the partial factors are
neither applied to

ϕ

´ or c´ nor directly to the actions but to the action effects and the characteristic

values of the resistances in the last step of the verification of the ultimate limit states of geotechnical
structures. Based on a long tradition three design situations are introduced to account for different
probabilities of failure and the need for different safety levels. The procedures and results of the three
approaches specified in Eurocode 7 are compared taking the dimensioning of the width of the founda-
tion of a cantilever stem wall as an example.

1

INTRODUCTION

In future, verification of ultimate limit states by calculation will be performed in accordance with the
partial factor concept throughout the entire construction sector in Europe. To put it simply, the concept
states that it must be verified that the design value R

d

of the resistance

is greater than the design value

E

d

of the actions or the action effects:

R

d

≥

E

d

However, it turned out that the member states were unable to reach a consensus of opinion on the

implementation of this limit state equation in geotechnical design in the draft of Part 1 of Eurocode 7
(ENV 1997-1, 1994). The principal criticism expressed not only by Germany but also by other Euro-
pean countries concerned the intended procedure for verifying the stability of foundations by calcula-
tion. The procedure involves the use of two different stability analyses – the investigation of cases B
and C. Firstly, this attracted criticism as it would have doubled the amount of effort required to verify
the stability of foundations by calculation after implementation of EC 7. Secondly, the safety philoso-
phy on which the procedure was based was strongly criticised in Germany and other member states
(Gudehus and Weissenbach, 1996, Schuppener et al., 1998, Stocker, 1997, Weissenbach et al. 1999).

After lengthy discussions, a compromise was reached by which the new version of EC 7 would in

future not specify a single procedure only but would give member states a choice of three different
approaches to verifying the stability of foundations by calculation. Each state would then have to
specify, in a National Application Document (NAD), which of the three approaches was to be applied.
In a NAD the suggested partial safety values of EC7 will either have to be confirmed or altered if nec-
essary according to national experience.

- 2 -

2

LIMIT STATES AND PARTIAL FACTOR METHOD OF EUROCODES 0 AND 7

The revised EC 7 and the new versions of the German geotechnical codes are based on Eurocode 0
(prEN 1990, draft July 2000) which contains provisions that are applicable to all areas of building and
civil engineering and thus do not have to be specified again separately in each Eurocode. In particular,
this includes the definition of the limit states for which verification is required in building and civil
engineering and how the partial factors are to be applied in stability analyses and introduced into limit
state equations. There are two possible approaches when determining the design values of resistances
and actions of the ground:

In the Material Factor Approach the partial factors

γ

m

are applied to the characteristic values of the

material properties of the structure or the ground to determine the design value of the resistance R

d

of

the structure or the ground or the design value of the action of the ground E

d

. Thus the following

equations are derived for resistances and actions of the ground:

R

d

= R {(tan

ϕ

k

)

/

γ

m

, c

k

/

γ

m

}

E

d

= E {(tan

ϕ

k

)

/

γ

m

, c

k

/

γ

m

}

where:
R is a function describing the resistance of the ground - e.g. passive earth pressure, bearing capacity

or sliding resistance of a footing - determined with factored values of the characteristic shear pa-
rameters

ϕ

k

and c

k

,

E is a function describing the action of the ground - e.g. active earth pressure - determined with fac-

tored values of the characteristic shear parameters

ϕ

k

and c

k

,

γ

m

is the partial factor for the shear parameters of the ground taking account of the possibility of unfa-
vourable deviations of the shear parameters from their characteristic values and uncertainties in
modelling the resistance and/or actions.

In the Resistance and Action Factor Approach the design values of the resistances R

d

and actions E

d

are determined by applying the partial factors

γ

R

and

γ

E

to the characteristic values of the resistance R

k

and of the actions or action effects E

k

of the structure or the ground:

R

d

= R

k

/

γ

R

E

d

= E

k

⋅

γ

E

where

γ

R

is the partial factor for the resistance of the ground, taking account of the possibility of unfavour-
able deviations of the shear parameters from their characteristic values and uncertainties in model-
ling the resistance,

γ

E

is the partial factor for the actions or action effects taking account of the possibility of unfavourable
deviations of the shear parameters from their characteristic values and uncertainties in modelling
the resistance and/or actions.

The Material Factor Approach was the only approach specified in the previous version of EC 7

(ENV 1997-1 (1994)). As the Resistance and Action Factor Approach has now been introduced in
EC 0 (prEN 1990, draft July 2000) for building and civil engineering as a whole, there are no longer
any obstacles to applying it in geotechnical engineering and including it in the new version of EC7.
This now enables two other verification approaches to be included as alternatives to the methods used
hitherto in Case B and Case C (see table 1).

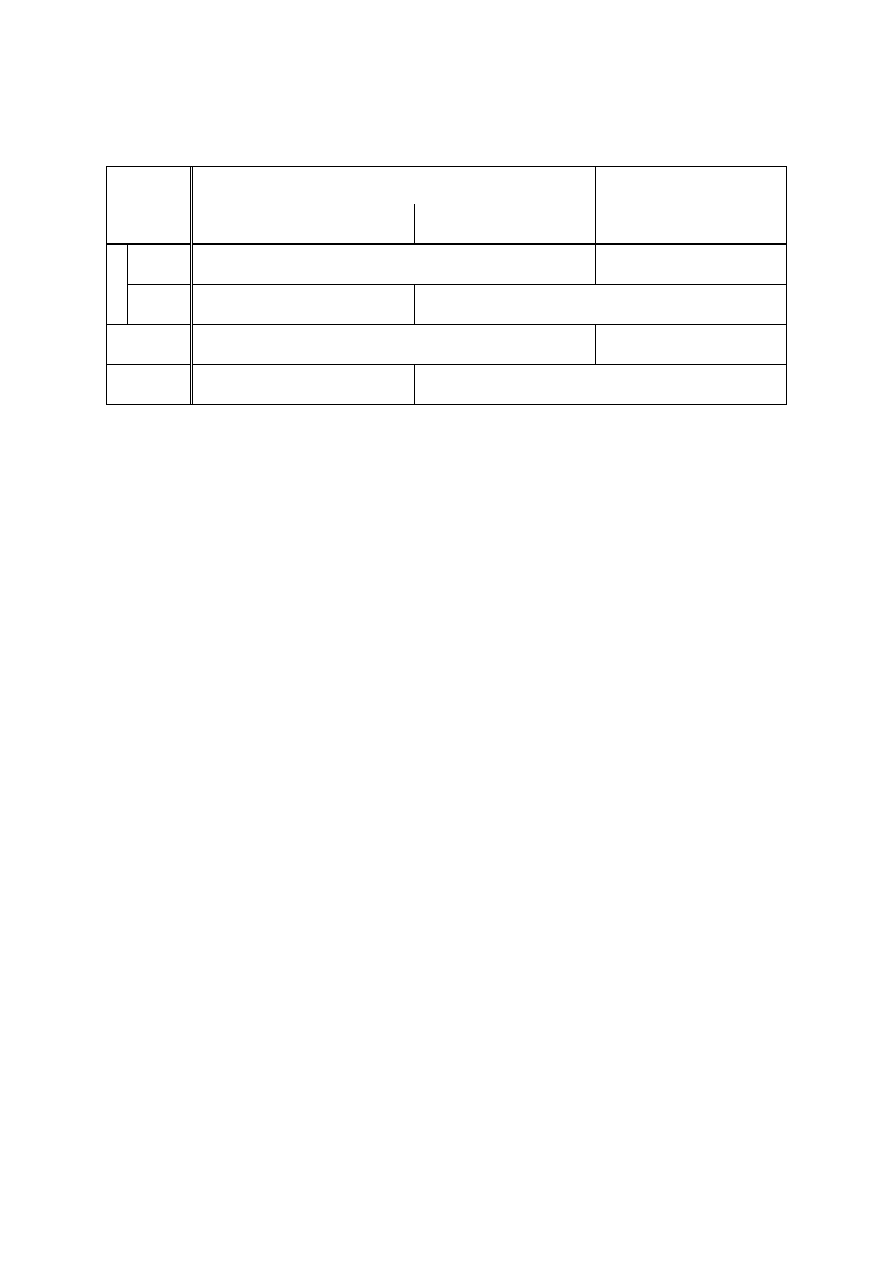
- 3 -

Table 1: Sets of partial factors for the approaches 1 to 3 to verify ultimate limit states of foundations
and retaining structures according to EC0 and EC7 –1

Action or action effects

Approach

of the structure

of the ground

Resistance of the ground

Case B

γ

G

= 1.35,

γ

G,fav

= 1.00,

γ

Q

= 1.50

γ

ϕ

= 1.00,

γ

c

= 1.00

1

Case C

γ

G

= 1.00,

γ

Q

= 1.30

γ

ϕ

= 1.25,

γ

c

= 1.25

2

γ

G

= 1.35,

γ

G,fav

= 1.00,

γ

Q

= 1,50

γ

Ep

=

γ

Gb

=1.40,

γ

Sl

= 1.10

3

γ

G

=1.35,

γ

G,fav

=1.00,

γ

Q

=1.50

γ

ϕ

=1.25,

γ

c

=1.25

Approach 1 corresponds to the approach originally specified in EC 7 according to which two

analyses - referred to as “Case B” and “Case C” – were required. Case B of Approach 1 is primarily
intended to cover the uncertainties in the actions. Partial factors are therefore applied to all actions –
both of the structure and of the ground – with a distinction being made between unfavourable perma-
nent (

γ

G

), favourable permanent (

γ

G,fav

) and variable loads (

γ

Q

). It aims to provide a safe geotechnical

design in the event of unfavourable deviations of the actions from their characteristic values, while the

characteristic values of the angle of friction

ϕ

´

k

and cohesion c´

k

are taken as soil parameters (

γ

ϕ

=

γ

c

= 1.00)

.

In Case C of Approach 1, it is principally the uncertainties in the material characteristics that are

investigated. The partial factors on the soil parameters

γ

ϕ

and

γ

c

are therefore greater than 1. In con-

trast, it is assumed that the permanent actions correspond to the characteristic values while the variable
actions are slightly higher than the characteristic values, providing a conservative design.

Approach 2 corresponds to the joint proposal put forward by Germany and France in which a single

analysis is deemed sufficient.

The same partial factors are applied to the actions and action effects of

the structure and the soil in this approach,

γ

G

being taken as 1.35 for permanent loads and

γ

Q

as 1.50

for variable loads. The partial factors for soil resistances vary between

γ

Ep

=

γ

Gb

= 1.40 for passive

earth resistance and ground bearing capacity and

γ

Sl

= 1.10 for sliding. The values chosen ensure that

the level of safety is equivalent to that provided by the former global safety concept. In approach 2
geotechnical design thus takes account of the unfavourable deviations of the resistance of the soil and
the actions of both the soil and of the structure from their characteristic values by applying partial
factors greater than 1 to both the actions and the resistances in the inequation for geotechnical ultimate
limit states. This approach thus corresponds in content and form to the partial safety concept specified
in EC 0 for the verification of stability by calculation in all areas of structural design in building and
civil engineering.

In Approach 3, both the actions and the resistances of the ground are determined using the design

shear parameters, i.e. partial factors are applied to the characteristic shear parameters. The actions due
to the structure are dealt with in the same way as in Approach 2.

3

BASIC PRINCIPLES OF THE NEW GERMAN GEOTECHNICAL CODE DIN 1054

Apart from the basic concepts specified in EC0 and EC7, priority has been given in German geotech-
nical coding to the principle that the concept applied in the verification of geotechnical limit states
should be as similar as possible to that applied in the verification of structural limit states. In most
cases the same engineer will perform the geotechnical as well as the structural verifications for foun-

- 4 -

dations and retaining walls, so switching from one concept to another must be avoided. This meant
that

-

the values of the partial factors on the actions of the ground and those of the structure should be the
same (see table 2) and

-

only a single calculation based on the characteristic values of the actions and the resistances should
suffice to verify a limit state – instead of the two Cases B and C proposed in the draft of EC7 pub-
lished in 1994.

Moreover, Germany favoured the Resistance and Action Factor Approach in which the safety fac-

tors are neither applied to

ϕ

´ or c´ nor directly to the actions but to the characteristic action effects

(internal forces, bending moments, etc.) and the characteristic values of the resistances in the last step
of the verification of the ultimate limit state.

Design Situations to account for different probabilities of failure and the need for different safety

levels constitute the fourth important feature of German geotechnical coding (also see prEN 1990) in
accordance with a long tradition of design situations in geotechnical DIN codes and other geotechnical
recommendations. There are Design Situation 1 (DS1) for permanent situations, Design Situation 2
(DS2) for the stage of construction or transient structures and Design Situation 3 (DS3) for accidental
situations concerning both actions and resistances (see table 2 and 3).

Table 2: Proposed partial safety factors on action effects E

k

Actions

Symbol

DS1

DS2

DS3

Permanent actions including water, active earth pressure

γ

G

1.35

1.20

1.00

Unfavourable variable actions

γ

Q

1.50

1.30

1.00

Table 3: Proposed partial safety factors on resistances R

k

of the ground

Resistances

Symbol

DS1

DS2

DS3

Passive earth pressure and ground bearing resistance

γ

Ep

,

γ

Gb

1.40

1.30

1.20

Sliding

γ

Sl

1.10

1.10

1.10

Pile resistance in compression (from pile tests)

γ

Pc

1.20

1.20

1.20

Pile resistance in tension (from pile tests)

γ

Pt

1.30

1.30

1.30

Pull-out resistance of grouted anchors

γ

A

1.20

1.15

1.10

Shear parameter: tan

ϕ

´ and c´ (only for slope stability)

γ

ϕ

,

γ

c

1.30

1.20

1.10

Experience in Germany has shown that the former global safety concept has hitherto ensured that

foundations could be designed economically and with an adequate degree of safety. It is for this reason
that the safety level used hitherto in the global safety concept has been selected as a base quantity and
the partial factors of the new partial safety concept calibrated against it. This was done by “splitting”
up the global factor

η

in two partial factors –

γ

R

for the resistance and

γ

G,Q

a mean value for permanent

and variable actions and action effects:

η

=

γ

R

⋅

γ

G,Q

(1)

The partial factors for the resistance of the ground

γ

R

were then determined by means of equation

(1), inserting the value

η

of the old global safety concept and the prescribed partial safety factors

γ

G,Q

for permanent and variable actions specified in Eurocode 0 (ENV 1990, draft October 1999):

γ

R

=

η

/

γ

G,Q

The steps of the design procedure proposed by the German geotechnical DIN codes are very similar to
those put forward by structural engineers:
1. Estimated sizing and assessment of the static design system of the geotechnical structure (footing,

retaining wall, strutted sheet pile wall, piles etc).

2. Determination of the characteristic actions of the structure and of the soil, i.e. the most realistic and

probable actions.

- 5 -

3. Determination of the characteristic action effects E

ki

, e.g. strut-, anchor- or supporting-forces, the

resultant characteristic forces in the base level of a footing or in the earth pressure support of a wall
etc.

4. Determination of the characteristic resistances R

ki

e.g.:

- for structural elements: the characteristic bending moment or the characteristic compressive

strength according to the standards for the considered material,

- for soil: the characteristic bearing capacity of shallow foundations, the characteristic passive

earth pressure or the characteristic bearing capacity of piles, anchors and nails determined by
calculations, tests or comparable experience.

5. Verification of the ultimate limit state in every relevant cross section of the structure and in the

soil:

−

The design effects of the actions E

di

are obtained by multiplying the characteristic effects E

ki

of

the actions by partial safety factors e.g. for permanent structures with

γ

G

= 1.35 for permanent

actions and

γ

Q

= 1.50 for variable actions (see table 2)

−

The design resistances R

di

are obtained by dividing the characteristic values R

ki

by their corre-

sponding safety factors for the structure (e.g. for steel see Eurocode 2 (EN 1992 (1991)), for
concrete see Eurocode 3 (EN 1992 (1992)) and for soil (see table 3).

The basic equation:

Σ

R

di

≥

Σ

E

di

is verified in the final step of the ultimate limit state analyses. If it is not fulfilled the sizing shall be
improved.

The merits of this concept for the geotechnical and structural verifications of foundations and re-

taining walls are:
1. As this calculation works with characteristic values of actions, which are also used for the verifica-

tion of the serviceability limit state, no separate calculation is necessary for the input of the deter-
mination of the displacements.

2. The concept is open for all analytical methods of verification. Steps 3 and 4 allow for the classical

methods, the theory of elasticity, ultimate load method, spring models, the finite element method
and cinematic element method.

3. The procedure corresponds to the concept of the Eurocodes for structural engineering (EN 1992

Eurocode 2 (1991), EN 1993 Eurocode 3 (1992). Thus geotechnical engineering does not need a
separate concept as proposed in the 1994 version of Eurocode 7. The procedure can therefore easily
be understood and adopted by students and practising engineers, which makes it very user-friendly.

4

GEOTECHNICAL DESIGN OF A CANTILEVER STEM WALL – A COMPARISION OF
THE THREE APPROACHES

4.1

Geometry and loads

The procedures and results of the three design approaches specified in EC 7 (EN 1997-1, 2000) will be
compared taking as an example the design of a cantilever stem wall (see figure 1) which has already
been used by Simpson & Driscoll (1998) for comparative calculations. The width B of the foundation
slab of the cantilever stem wall is to be determined. In geotechnical design, this is done by demon-
strating that the limit state equations with the required partial factors are satisfied for both bearing
resistance failure and for sliding for the width B selected in advance.

The earth pressure is determined in accordance with DIN 4085-100 (1996). In the stability analysis,

the active earth pressure acting on a fictitious vertical wall is applied at the end of the foundation slab
of the cantilever stem wall. The bearing capacity of the ground is calculated using the formulae given
in DIN 4017-100 (1996). The partially mobilised passive earth pressure in front of the wall, E

phmob,d

=

E

ph

/

γ

Ep

, is taken to be an favourable action when verifying bearing resistance failure in all three ap-

proaches.

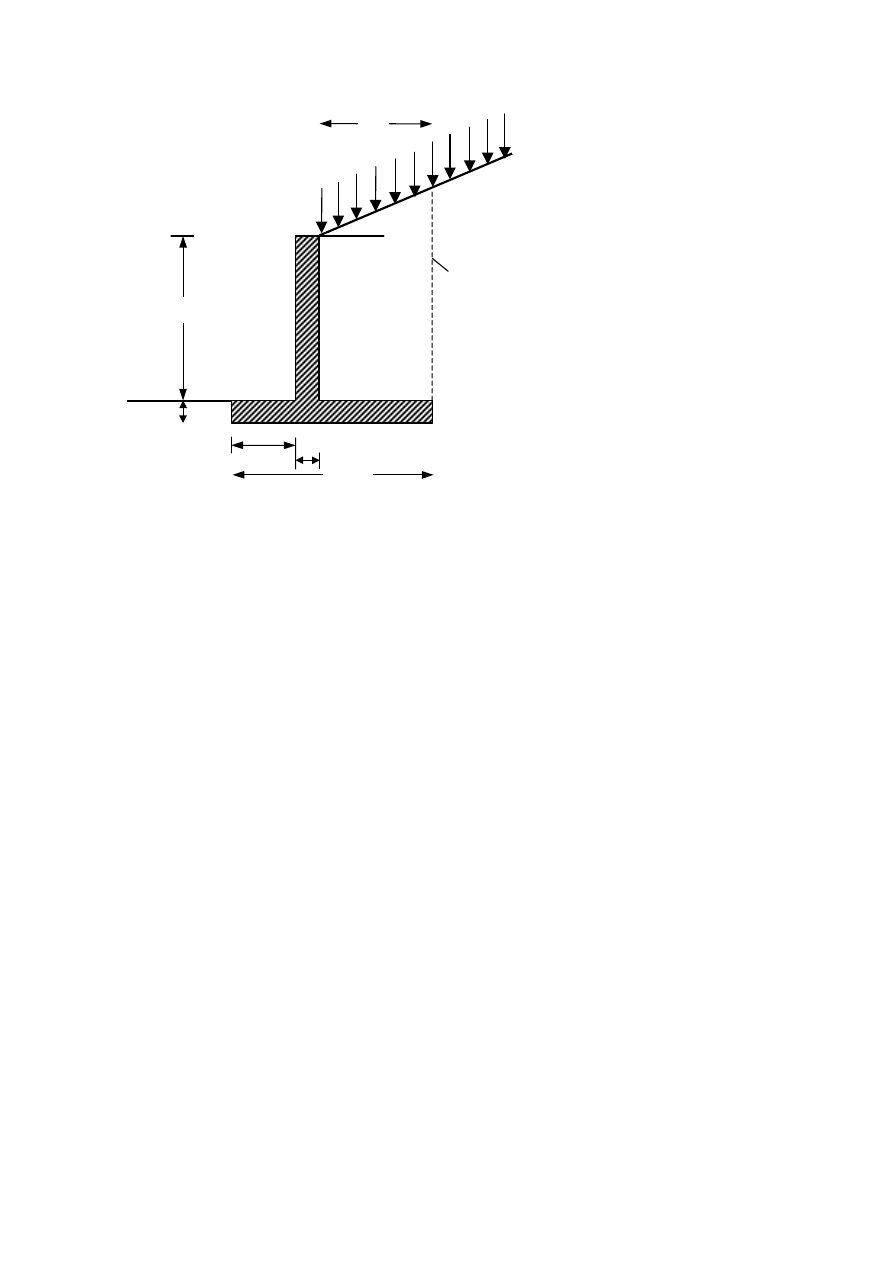
- 6 -

Figure 1: Cantilever stem wall, dimensions and loads

4.2

Design according to Approach 1

Each of the calculations – Case B and Case C - is performed with design values. Owing to the stabi-
lising moment, the action due to the self-weight of the soil acting on the foundation slab is assumed to
be favourable (

γ

G,fav

= 1.00) in Case B - while the action due to the self-weight of the retaining wall is

unfavourable (

γ

G

= 1.35). Determination of the design ground bearing resistance is based on the verti-

cal and horizontal components and the eccentricity of the design value of the resultant action effect in
the base level of the foundation. The results of both analyses are shown in table 4. The calculation
demonstrates that Case C is relevant for the design of the foundation width B in approach 1. Owing to
the higher design values of the shear parameters, the design bearing resistance R

Gb,d

of Case B is

nearly three times higher than in Case C while in both cases the vertical components V

d

of design

value of the resultant action effects differ only to a small extent.

4.3

Design according to Approach 2

In Approach 2 the calculations to determine the resultant action effect at the base level of the founda-
tion are performed with characteristic values. The determination of the characteristic ground bearing
resistance is then based on the characteristic values of the vertical and horizontal components and the
eccentricity of the resultant action effect at the base level of the foundation. The partial factors are not
introduced until the final step of the calculation when the limit state equations for bearing resistance
failure and sliding are verified. No distinction is made between favourable and unfavourable perma-
nent actions, in accordance with DIN 1054, a single partial factor

γ

G

= 1.35 being applied to all perma-

nent action effects instead. If a distinction between favourable and unfavourable permanent actions is
to be made in accordance with EC 7 the determination of the bearing resistance must be based on the
design value of the resultant action effect in the base level of the foundation. The results of both analy-
ses are given in table 4.

4.4

Design according to Approach 3

In Approach 3, all calculations are performed with design values as in approach 1. The action due to
the self-weight of the soil acting on the foundation slab is taken to be favourable (

γ

G,fav

= 1.00) owing

to the resultant stabilising moment while the action due to the self-weight of the retaining wall is unfa-
vourable (

γ

G

= 1.35). Determination of the design ground bearing resistance is based on the vertical

and horizontal components and the eccentricity of the design value of the resultant action effect in the
base level of the foundation. The results are shown in table 4.

h = 6,0 m

1)

β

= 20°

p

k

= 5 kN/m²

Fictitious wall to

determine the

action due to

active earth

pressure

0,95 m 0,7 m

B = ?

1)

This part of the variable action

must only be considered in the
structural design of the wall

- 7 -

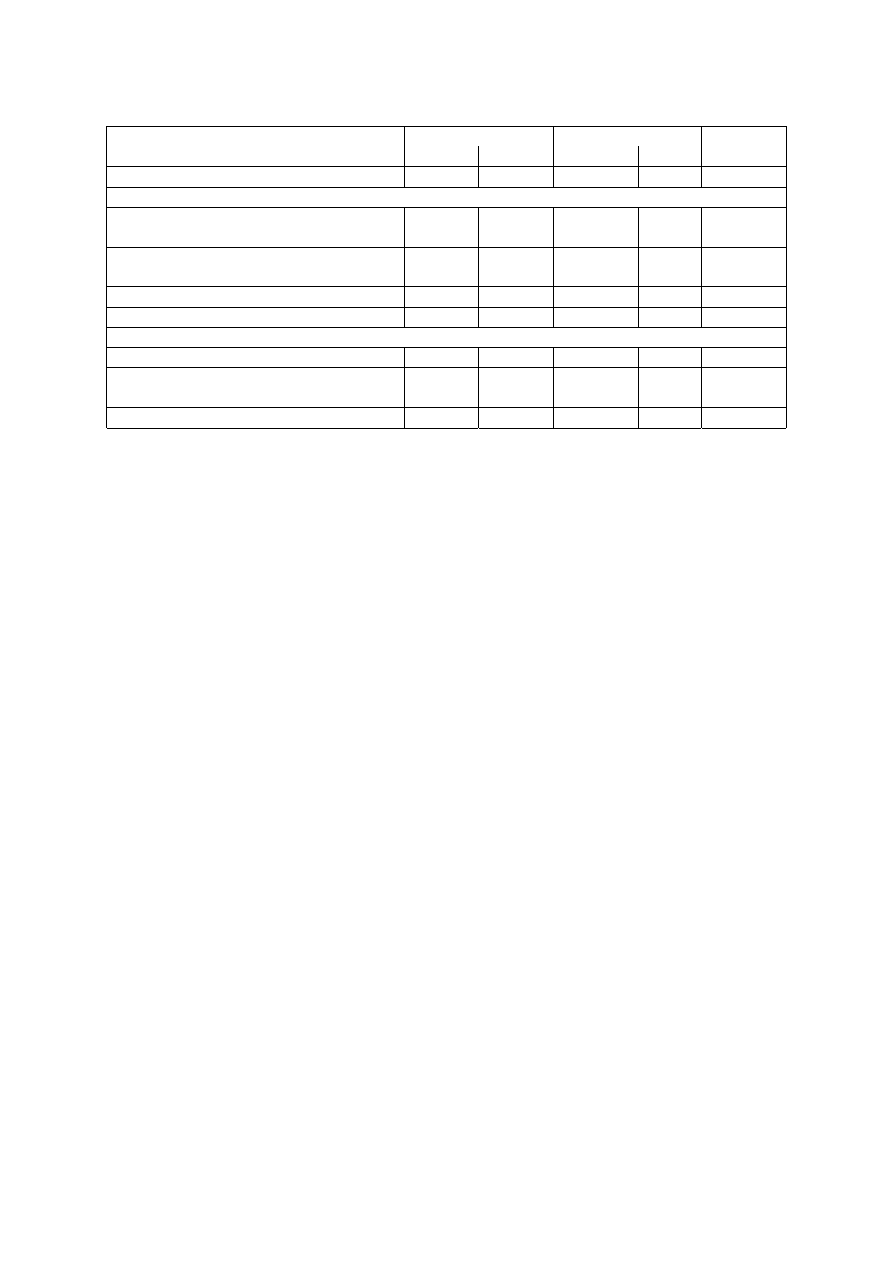
Table 4: Results of the comparative stablility calculations

Approach 1

Approach 2

Case B

Case C

DIN 1054

EC7

Approach

3

Width of foundation B [m]

5,00

5,00

3,40

3,80

4,90

Verification of safety against bearing resistance failure

Vertical component V

d

of the resultant

action effect in the base level [kN/m]

806

717

605

601

773

Inclination of the resultant action effect

tan

δ

=H

d

/V

d

(H

k

/V

k

)

0.34

0.40

(0.36)

0.39

0.37

Bearing resistance R

Gb,d

[kN/m]

2177

734

616

627

767

Degree of mobilisation f

Gb

= V

d

/ R

Gb,d

0.37

0.98

0.98

0.96

1.01

Verification of safety against sliding

Sliding resistance R

Sl,d

[kN/m]

559

393

291

327

422

Design value of the horizontal actions

Σ

H

d

[kN/m]

301

305

260

270

306

Degree of mobilisation f

Gl

=

Σ

H

d

/R

Sl,d

0.55

0.78

0.89

0.83

0.73

4.5

Results and conclusions

In all three approaches, safety against ground bearing resistance failure is relevant for the design of the
width B of the foundation.

The smallest foundation dimension B resulting from the application of Approach 2 is 3.40 m if

DIN 1054 is followed and each permanent action – favourable and unfavourable - is multiplied by the
same partial factor

γ

G

= 1.35. If the proposal given in EC 7 is followed and a factor of only

γ

G,fav

= 1.00

is applied to the self-weight of the soil acting on the foundation, the angle of the resultant action effect
tan

δ

increases and the bearing resistance therefore decreases. This is not compensated for by the re-

duction of the vertical component V

d

of the design value of the resultant action effect in the base level

of the foundation, resulting in a wider foundation with a width B = 3.80 m being required.

The main reason for the much lower foundation width that results when applying Approach 2 is the

much higher design ground bearing resistance R

Gb,d

that results for the same loads and dimensions

when applying the two other approaches. In the approach laid down in DIN 1054, the design ground
bearing resistance R

Gb,d

is determined by first calculating the characteristic ground bearing resistance

R

Gb,k

using the characteristic shear parameters

ϕ

’

k

and c’

k

. The design bearing resistance R

Gb,d

= R

Gb,k

/

γ

Gb

is then obtained by dividing the the characteristic ground bearing resistance by the partial factor for

the bearing resistance failure,

γ

Gb

= 1.40. In contrast, R

Gb,d

is determined using the design values of the

shear parameters

ϕ

’

d

and c’

d

in approaches 1 and 3. In the case we are dealing with here, a reduction in

the angle of friction

ϕ

’

k

= 32.5° to

ϕ

’

d

= 27.0° lowers the ground bearing resistance to around half of

that determined when a characteristic angle of friction

ϕ

’

k

= 32.5° is applied. The greater foundation

widths obtained using approaches 1 and 3 are thus due on the one hand to the additional safety in-
cluded when dealing with the favourable permanent actions and on the other hand to the greater level
of safety in respect of the bearing resistance resulting from the proposed partial factors for the shear
parameters.

The difference between approach 1 (Case C) and approach 3 when establishing the required foun-

dation width B is insignificant in the example we are dealing with here. Approach 3 results in a some-
what smaller width B despite the higher vertical component V

d

of the action effect in the base level of

the foundation as the angle of the resultant (tan

δ

) decreases and thus the bearing resistance increases,

which influences the results in the way shown here.

To sum up, it can be said that a far more economical shallow foundation design is obtained when

following Approach 2 with the partial factors specified in DIN 1054. The partial factors for the actions
due to the structure and the ground as well as those for the ground resistances have been specified such
that the level of safety provided by the global safety concept used hitherto is maintained. The safety
level of this concept has been tried and tested in practice for decades. Thus, applying the partial fac-
tors specified in DIN 1054 to the design of geotechnical structures not only ensures an adequate de-

- 8 -

gree of safety, it is also considerably more economical, as the comparison with the other approaches
has illustrated.

The detailed numerical calculations according to the three approaches can be ordered from the

author by email:

[e-mail]

.

5

REFERENCES

DIN 4017-100 (1996) Berechnung des Grundbruchwiderstandes von Flachgründungen Teil 100:

Berechnung nach dem Konzept mit Teilsicherheitsbeiwerten, Beuth, Berlin

DIN 4085-100 (1996) Berechnung des Erddrucks Teil 100: : Berechnung nach dem Konzept mit

Teilsicherheitsbeiwerten, Beuth, Berlin

DIN 1054 (1999) Standsicherheitsnachweise im Erd- und Grundbau, Draft February 2000, Beuth,

Berlin

ENV 1997-1 Eurocode 7 (1994): Geotechnical design, Part 1: General rules. European Committee for

Standardisation (CEN) Brussels

EN 1997-1 Eurocode 7 (2000): Geotechnical design, Part 1: General rules. European Committee for

Standardisation (CEN) Brussels, draft April 2000

prEN 1990 Eurocode 0 - Basis of design (1999), European Committee for Standardisation (CEN)

Brussels, draft July

2000

EN 1992 Eurocode 2 (1991) Design of concrete structures, European Committee for Standardisation

(CEN) Brussels

EN 1993 Eurocode 3 (1992) Design of steel structures, European Committee for Standardisation

(CEN) Brussels

Gudehus, G. & Weißenbach, A. (1996) Limit state design of structural parts at and in the ground,

Ground Engineering

Schuppener, B., Walz, B., Weißenbach, A., Hock-Berghaus, K. (1998), EC7 – A critical review and a

proposal for an improvement: a German perspective, Ground Engineering,

Simpson, B. & Driscoll, R. (1998) Eurocode 7 – a commentary. Construction Research Communica-

tions Ltd., London

Stocker, M. (1997) Eurocode 7 – all problems solved? European Foundations, a Ground Engineering

Publication

Weißenbach, A., Gudehus, G. and Schuppener, B. (1999)

Proposals for the application of the partial

safety factor concept in geotechnical engineering, geotechnik special issue: German contributions
to European standardization