Physics homework #04

1. A boy exerts a force of 11 N at 29

◦

above the horizontal on a 6.4 kg sledge. Find the work done

by the boy and the final speed of the sledge after it moves 2 m, assuming the sledge starts with an
initial speed of 0.5 m/s and slides horizontally without friction.

2. A particle of mass m is attached to a string of length L and whirled in a vertical circle about a fixed

point O to which the other end of the string is attached. (a) Find an expression for the tension T
in the string at the instant that the particle’s speed is v and the string makes an angle θ with the
vertical. Graph T (θ) for m = 0.1 kg, g = 10 m/s

2

, L = 1 m and v = 5 m/s. (b) Find the value of

velocity if we know that T = 0 at the highest point of the circle (assume L = 1 m, m = 0.2 kg).
What happens if the velocity is smaller than this value?

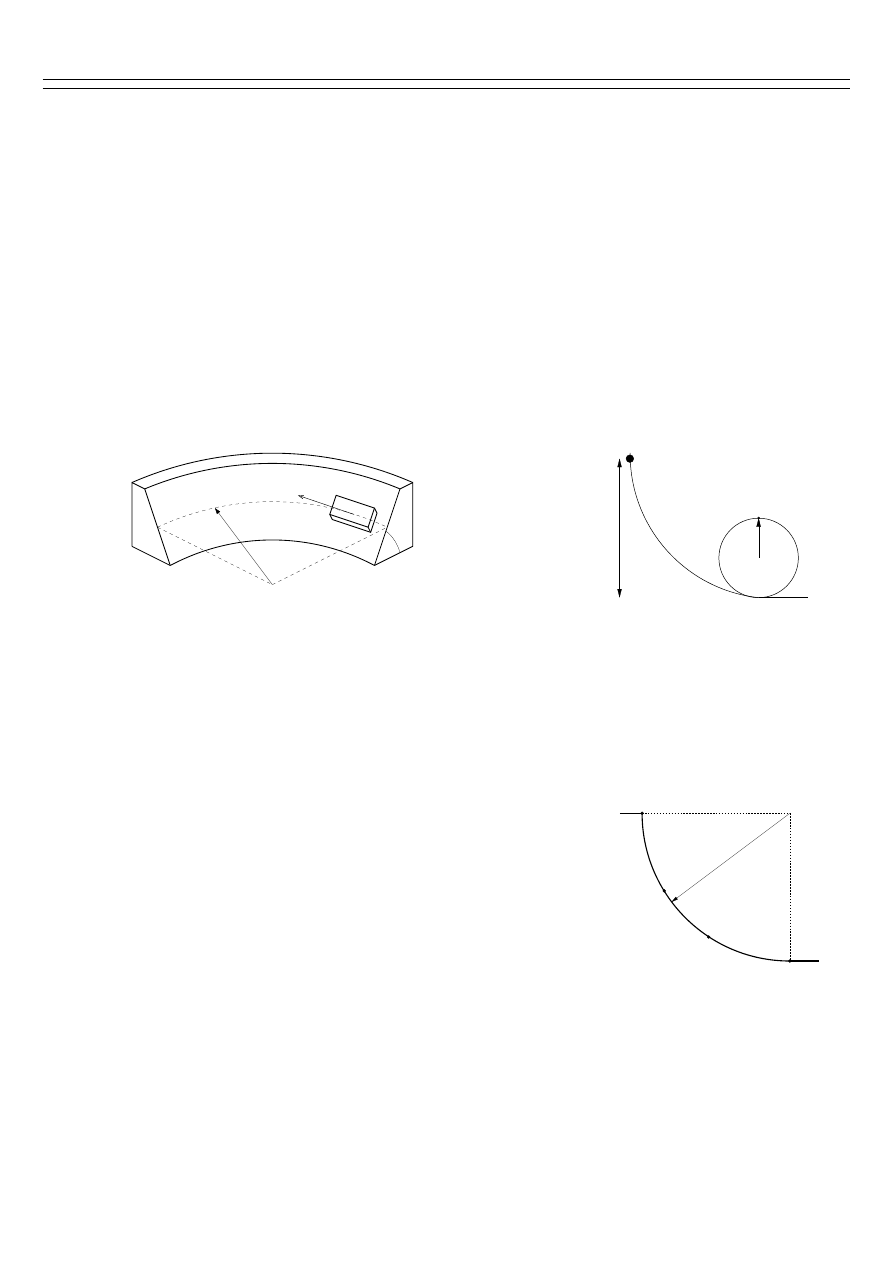
3. A section of a cloverleaf highway exit has the radius R = 25 m (Fig.1). (a) What is the banking

angle θ of the roadbed if we know that cars travelling at 40 km/h need no frictional force from the
tires to negotiate the turn? (b) The static and kinetic coefficients of friction between the tires and
the road are µ

s

= 0.9 and µ

k

= 0.8. Show that the maximum speed at which a car can enter the

curve without sliding toward the top edge of the banked turn is approx. 25 m/s.

Fig.1

θ

R

v

Fig.2

h

R

A

4. A 0.5-g bead slides without friction around a circular loop-the-loop (Fig.2). The bead is released

from a height h = 3.5R. What is its speed at point A? How large is the normal force on it (at A)?

5. A boy skateboards down a curved playground ramp. If we treat the boy and his skateboard as a

particle of total mass m = 25.0 kg, he moves through a quarter-circle with radius R = 3.0 m. He
starts from rest at point A and there is no friction. (a) Find his speed at the bottom of the ramp (at
point D – see Fig.3). (b) Draw free-body diagrams for points A, B, C and D. (c) Find the normal
force that acts on him at the bottom of the curve (at D). (d) Find work done by the normal force.

6. Suppose that the ramp from the previous prob-

lem is not frictionless, and that the speed at point
D is only 6.0 m/s. (a) Draw free-body diagrams
for points A, B, C and D. (b) What work was
done by the friction force?

Fig.3

R

C

B

A

D

7. We want to load a 12-kg crate into a truck by sliding it up a ramp 2.5 m long, inclined at 30

◦

. A

worker, giving no thought to friction, calculates that he can get the crate up the ramp by giving it
an initial speed of 5.0 m/s at the bottom and letting it go. But friction is not negligible; the crate
slides 1.6 m up the ramp, stops, and slides back down. (a) Assuming that the friction force acting
on the crate is constant, find its magnitude. (b) How fast is the crate moving when it reaches the
bottom of the ramp?

8. A glider with mass m = 0.2 kg sits on a frictionless horizontal air track, connected to a spring with

force constant k = 5.0 N/m. You pull on the glider, stretching the spring 0.1 m, and then release
it with no initial velocity. The glider begins to move back toward its equilibrium position (x = 0).
What is its x-velocity when x = 0.08 m?

9. Suppose that the glider from the previous problem is initially at rest at x = 0, with the spring

unstretched. You then apply a constant force

−

→

F in the +x-direction with magnitude 0.61 N to the

glider. What is the glider’s velocity when it has moved to x = 0.10 m?

10. In a “worst-case” design scenario, a 2000-kg lift with broken cables is falling at 4.0 m/s when it

first contacts a cushioning spring at the bottom of the shaft. The spring is supposed to stop the
lift, compressing 2.0 m as it does so. During the motion a safety clamp applies a constant 17,000-N
frictional force to the lift. As a design consultant, you are asked to determine what the force constant
of the spring should be.

11. You are rearranging your furniture and wish to move a 40.0-kg desk 2.5 m across the room. However,

the straight-line path is blocked by a heavy coffee table that you don’t want to move. Instead, you
slide the desk in a dogleg path over the floor; the doglegs are 2.0 m and 1.5 m long. Compared to
the straight-line path, how much more work must you do to push the desk in the dogleg path? The
coefficient of kinetic friction is 0.2.

Maciej Wo loszyn

WFiIS AGH

http://fatcat.ftj.agh.edu.pl/~woloszyn/phys/