
SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #257
2
4
2
3
3
1
3
3
4
2
4
2
3
1
3
1
3
3
1
2
4
3
2
3
2
Bridges #258
1
3
4
2
3
5
2
2
1
3
3
2
3
3
6
2
2
2
1
2
3
1
2
2
3
3
Bridges #259
2
1
2
2
2
3
1
1
3
5
2
3
2
3
1
3
3
2
2
5
2
2
2
2
4
Bridges #260
2
3
2
2
3
2
1
1
3
2
3
4
2
4
2
2
1
2
3
3
3
3
3
2
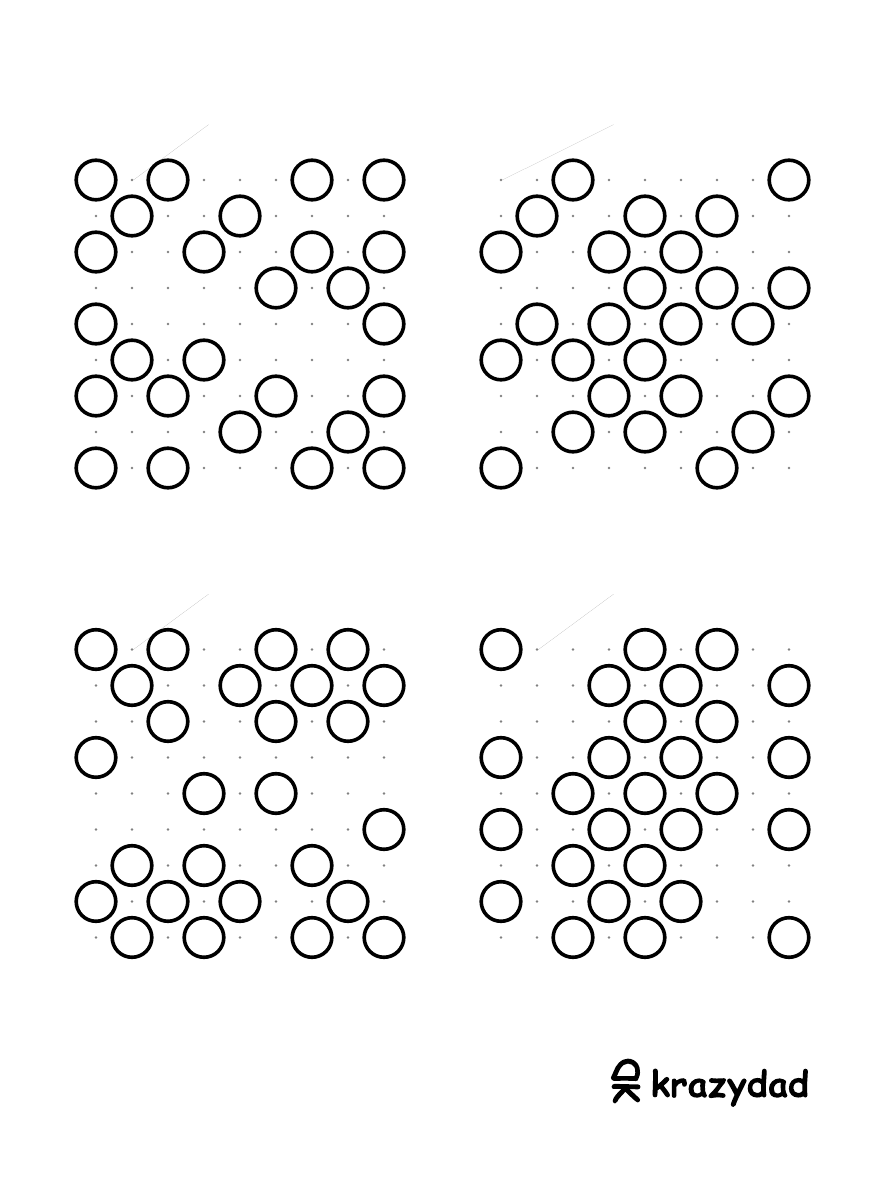
SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #261
2
3
4
2
3
1
2
3
3
3
3
3
3
3
4
3
4
3
3
1
2
4
2
4
4
2
Bridges #262
2
4
1
3
3
2
3
2
2
3
4
2
5
5
2
4
2
1
2
3
2
2
2
1
3
1
Bridges #263
3
3
2
1
2
1
2
2
2
3
3
3
2
2
3
3
4
1
2
1
2
4
1
4
3
3
Bridges #264
3
3
3
3
2
2
1
2
5
5
1
3
1
4
2
3
2
2
3
4
3
1
2
3
3
3
3

SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #265
2
3
3
1
1
3
2
2
3
3
4
4
1
1
1
4
5
4
2
1
1
3
2
2
4
2
2
Bridges #266
3
2
2
4
1
2
4
2
4
5
1
2
2
2
2
3
3
2
4
2
2
5
2
1
2
Bridges #267
1
4
3
2
1
3
2
3
3
1
3
2
4
4
3
4
2
2
2
1
3
2
3
2
4
2
Bridges #268
3
5
2
2
4
2
3
3
4
2
3
1
2
2
5
3
3
1
2
2
3
2
2
5
2
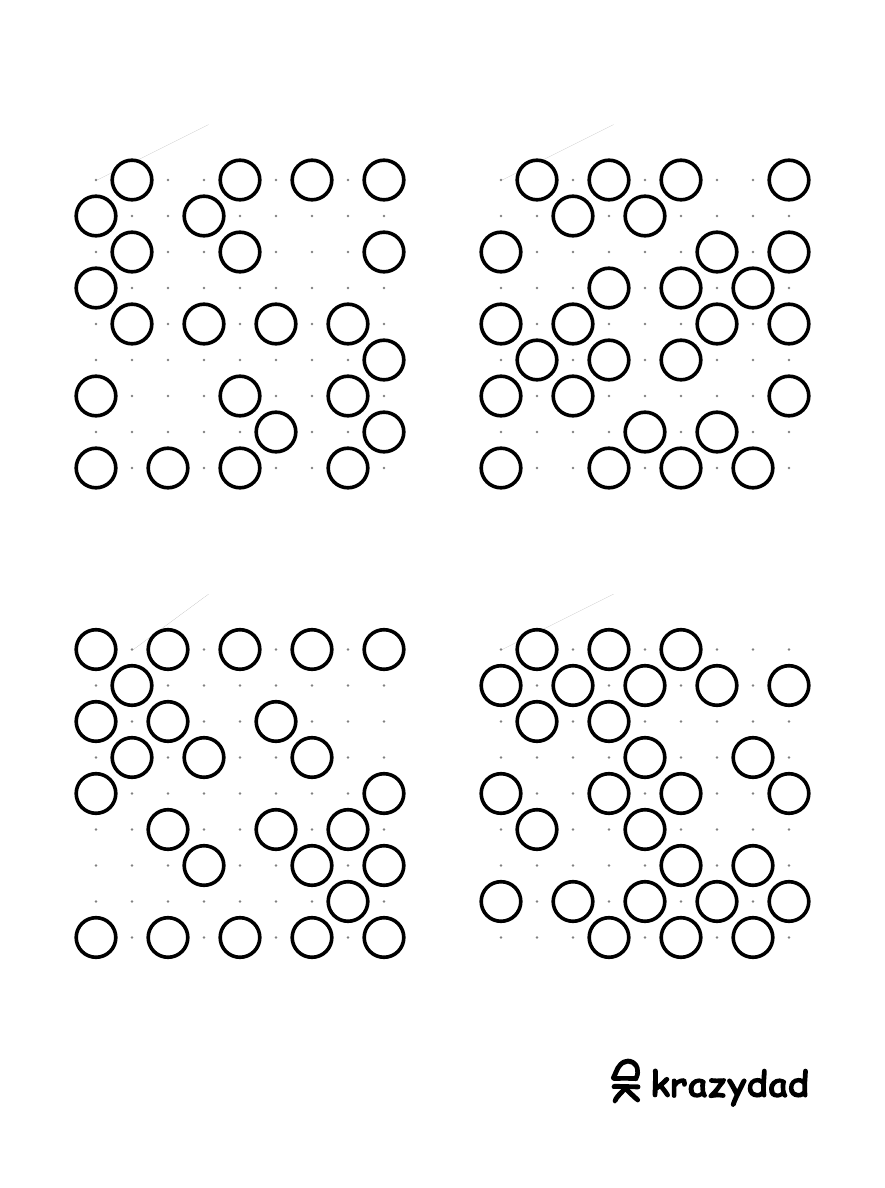
SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #269
2
4
1
2
3
2
2
5
4
3
3
2
2
4
3
4
4
3
2
3
2
2
4
2
Bridges #270
4
4
4
3
3
1
2
2
5
2
3
1
4
4
3
4
2
2
1
4
3
2
1
3
2
4
4
3
Bridges #271
2
4
3
4
2
2
3
3
2
4
3
2
4
2
2
3
3
1
2
3
1
3
3
2
3
2
Bridges #272
1
4
2
3
1
3
3
2
1
3
3
2
4
2
1
3
2
4
2
3
3
3
3
1
2
1
3
3
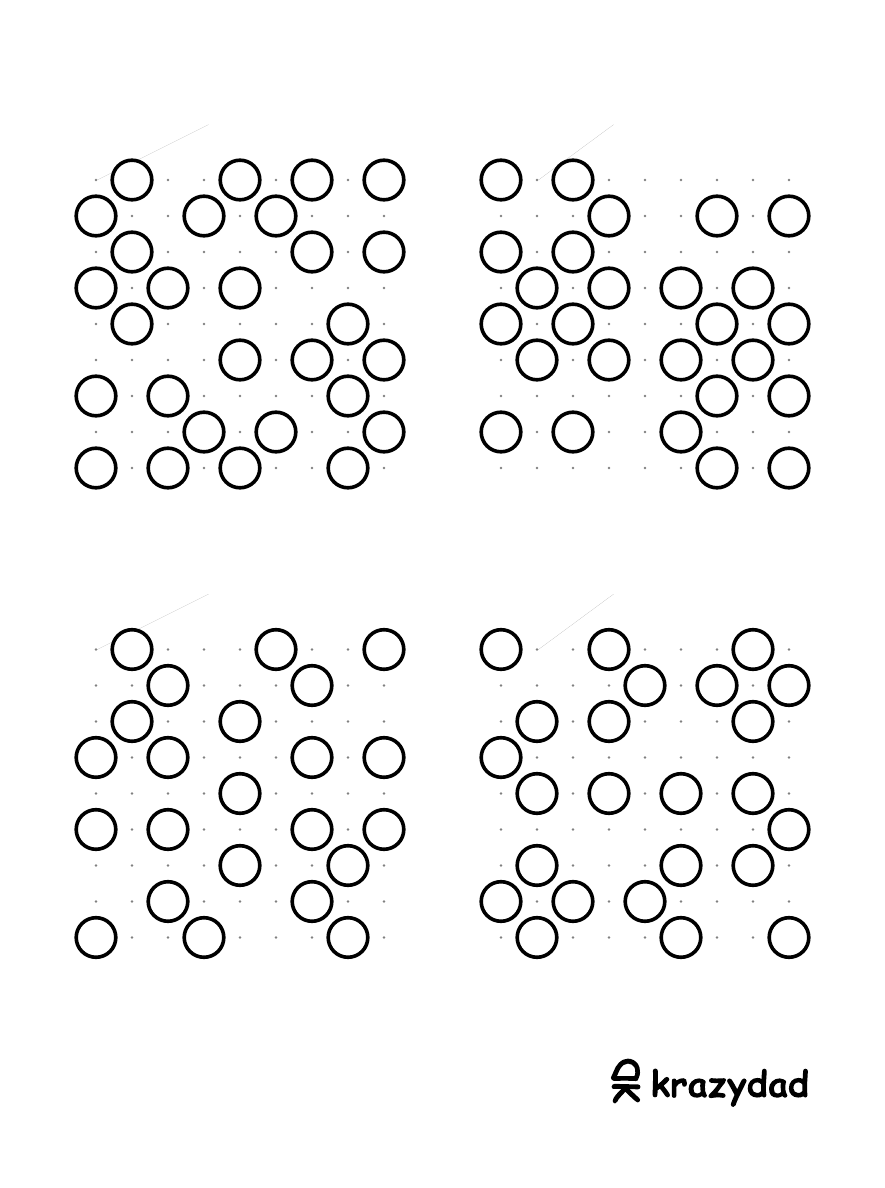
SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #273
1
3
4
2
4
3
1
3
6
4
3
3
3
1
1
3
3
4
3
4
2
2
4
4
2
3
3
1
Bridges #274
1
2
2
3
2
3
4
2
3
2
2
3
2
1
2
4
4
5
4
3
5
3
3
2
2
3
Bridges #275
2
3
3
2
4
2
3
1
2
4
3
2
2
5
3
2
2
3
3
1
3
3
2
Bridges #276
3
4
2
2
3
3
2
3
1
3
3
2
1
2
2
1
4
3
3
3
2
1
3
2
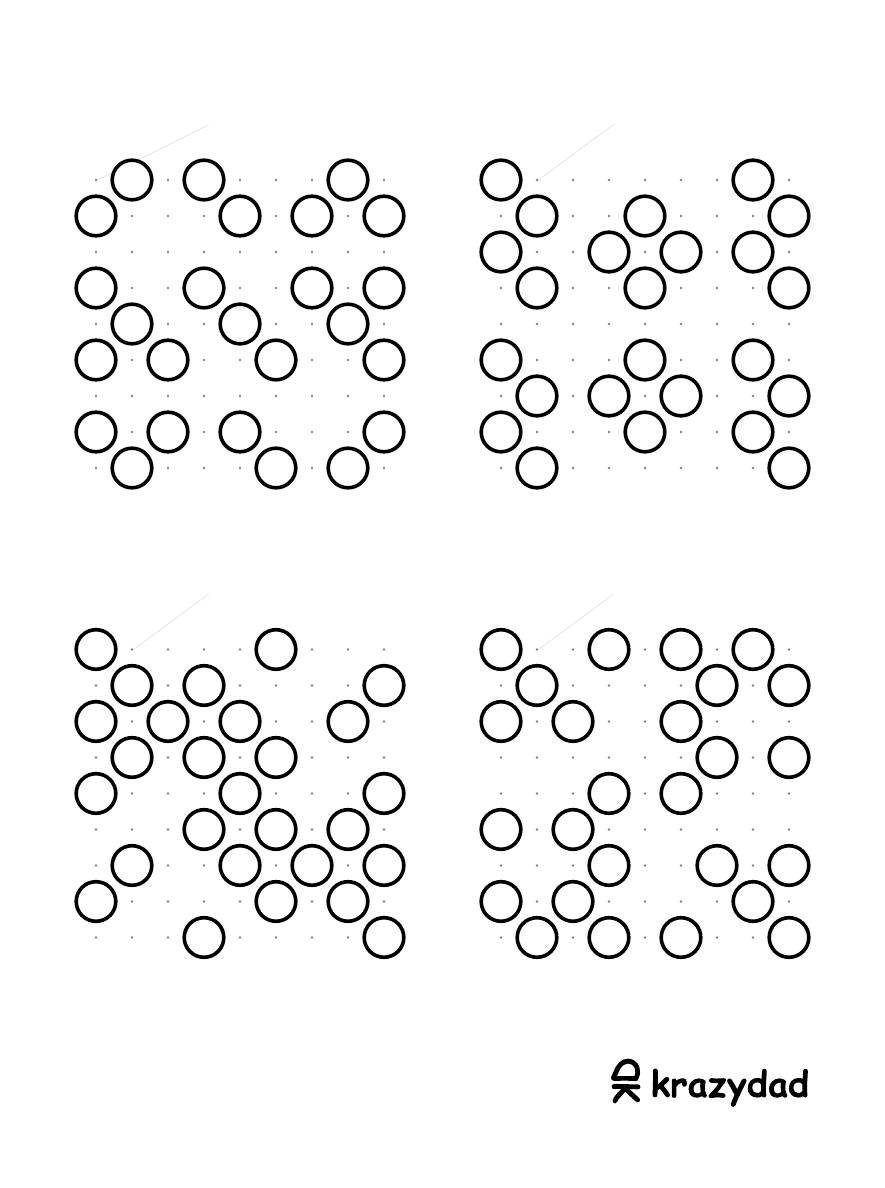
SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #277
1
3
3
1
2
3
1
3
4
3
2
2
4
4
2
2
2
4
1
3
2
2
2
3
1
Bridges #278
3
1
2
4
3
4
2
3
2
1
3
3
3
4
4
3
4
2
2
1
2
4
2
2
Bridges #279
4
2
1
2
2
4
2
3
3
2
4
2
2
1
2
2
3
3
2
3
2
3
2
3
2
2
3
Bridges #280
2
3
4
2
1
3
2
2
4
2
3
3
4
3
3
4
4
3
3
4
4
1
2
4
4
4
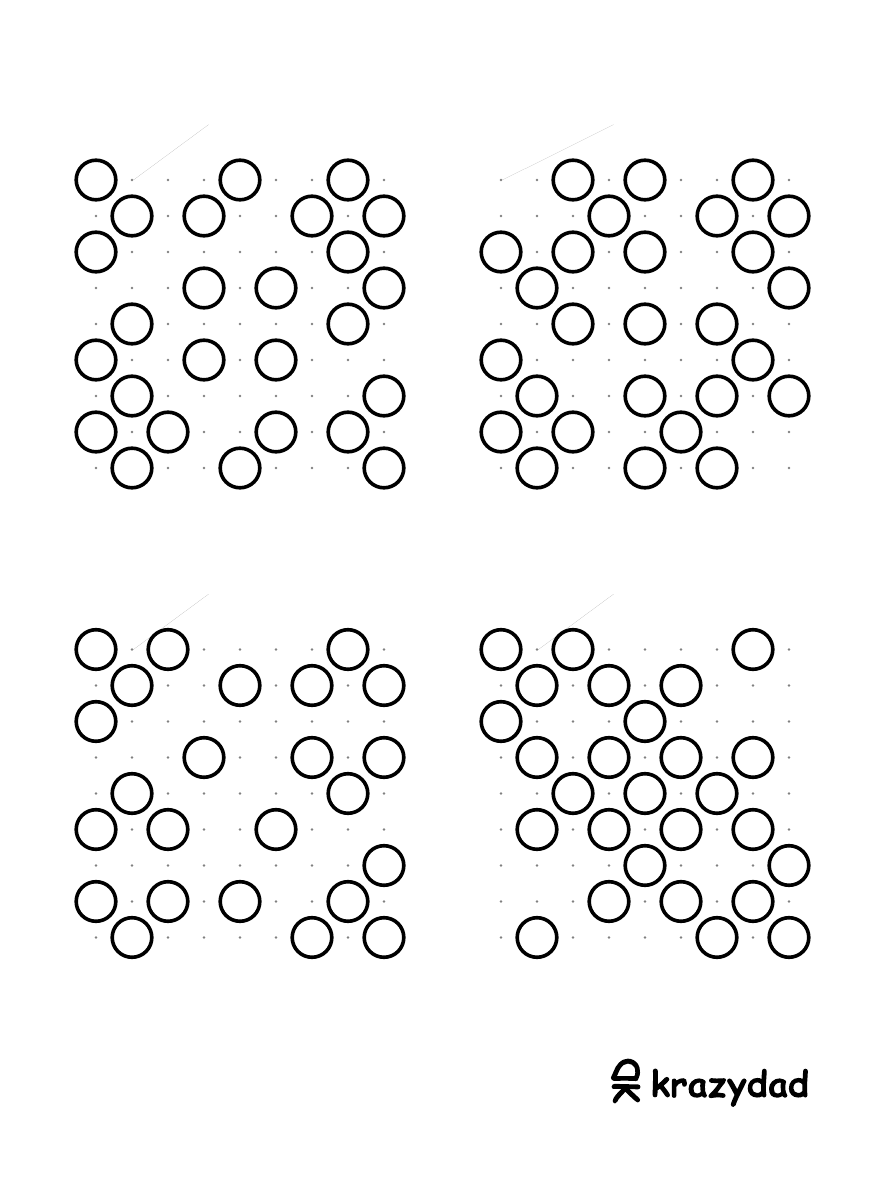
SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #281
4
4
2
2
4
3
3
4
2
3
2
2
3
3
4
1
3
2
3
3
2
3
2
1
3
4
Bridges #282
3
3
2
2
4
2
3
5
2
3
2
3
2
4
4
3
2
4
3
4
3
3
2
1
1
2
2
Bridges #283
3
4
2
2
2
3
2
3
2
3
3
3
3
4
3
1
4
2
3
2
2
1
3
4
Bridges #284
3
3
2
2
2
2
1
1
3
1
2
3
1
4
1
3
3
1
2
2
2
4
3
1
3
4
3
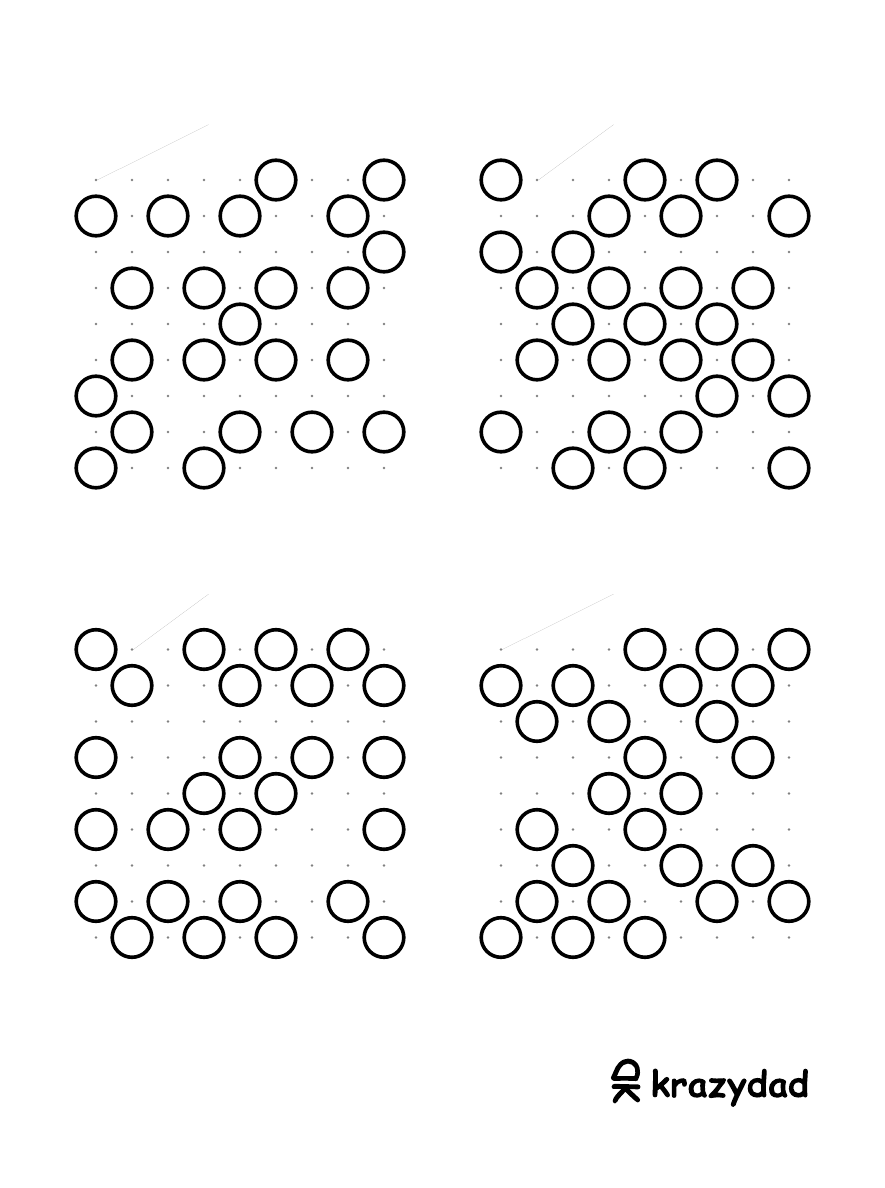
SMALL BRIDGES BY KRAZYDAD, BOOK 9
Connect these islands with bridges until each island can be reached from any other island, and each island has as many
outgoing bridges as its number. You may only connect islands vertically or horizontally and bridges may not cross.
There may be one or two bridges connecting pairs of islands, but no more than two. Each puzzle has a unique solution
that can be found without making guesses.
All puzzles © 2010 KrazyDad.com
Bridges #285
2
3
3
2
3
3
3
2
2
3
2
1
3
1
2
1
3
2
3
2
3
3
2
Bridges #286
2
2
1
1
3
4
5
3
1
2
3
3
2
2
1
3
4
3
3
2
5
3
3
2
2
3
2
Bridges #287
3
3
3
1
1
2
3
3
4
3
1
2
1
2
3
2
3
3
2
2
4
2
2
3
3
3
Bridges #288
1
2
3
3
4
3
2
2
4
2
2
4
2
2
3
1
1
3
2
2
2
2
3
2
2
1
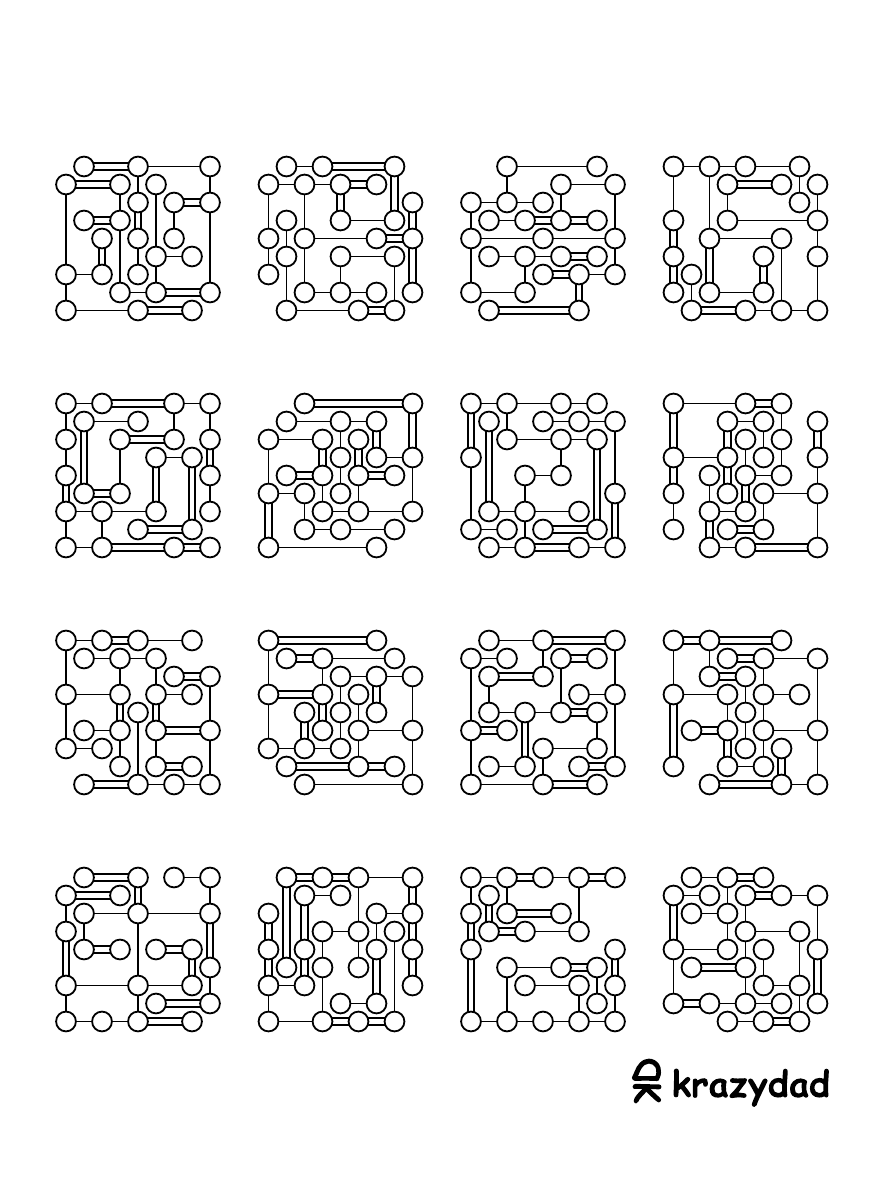
Answers #257 - #272
All puzzles © 2010 KrazyDad.com
Bridges #257
2
4
2
3
3
1
3
3
4
2
4
2
3
1
3
1
3
3
1
2
4
3
2
3
2
Bridges #258
1
3
4
2
3
5
2
2
1
3
3
2
3
3
6
2
2
2
1
2
3
1
2
2
3
3
Bridges #259
2
1
2
2
2
3
1
1
3
5
2
3
2
3
1
3
3
2
2
5
2
2
2
2
4
Bridges #260
2
3
2
2
3
2
1
1
3
2
3
4
2
4
2
2
1
2
3
3
3
3
3
2
Bridges #261
2
3
4
2
3
1
2
3
3
3
3
3
3
3
4
3
4
3
3
1
2
4
2
4
4
2
Bridges #262
2
4
1
3
3
2
3
2
2
3
4
2
5
5
2
4
2
1
2
3
2
2
2
1
3
1
Bridges #263
3
3
2
1
2
1
2
2
2
3
3
3
2
2
3
3
4
1
2
1
2
4
1
4
3
3
Bridges #264
3
3
3
3
2
2
1
2
5
5
1
3
1
4
2
3
2
2
3
4
3
1
2
3
3
3
3
Bridges #265
2
3
3
1
1
3
2
2
3
3
4
4
1
1
1
4
5
4
2
1
1
3
2
2
4
2
2
Bridges #266
3
2
2
4
1
2
4
2
4
5
1
2
2
2
2
3
3
2
4
2
2
5
2
1
2
Bridges #267
1
4
3
2
1
3
2
3
3
1
3
2
4
4
3
4
2
2
2
1
3
2
3
2
4
2
Bridges #268
3
5
2
2
4
2
3
3
4
2
3
1
2
2
5
3
3
1
2
2
3
2
2
5
2
Bridges #269
2
4
1
2
3
2
2
5
4
3
3
2
2
4
3
4
4
3
2
3
2
2
4
2
Bridges #270
4
4
4
3
3
1
2
2
5
2
3
1
4
4
3
4
2
2
1
4
3
2
1
3
2
4
4
3
Bridges #271
2
4
3
4
2
2
3
3
2
4
3
2
4
2
2
3
3
1
2
3
1
3
3
2
3
2
Bridges #272
1
4
2
3
1
3
3
2
1
3
3
2
4
2
1
3
2
4
2
3
3
3
3
1
2
1
3
3
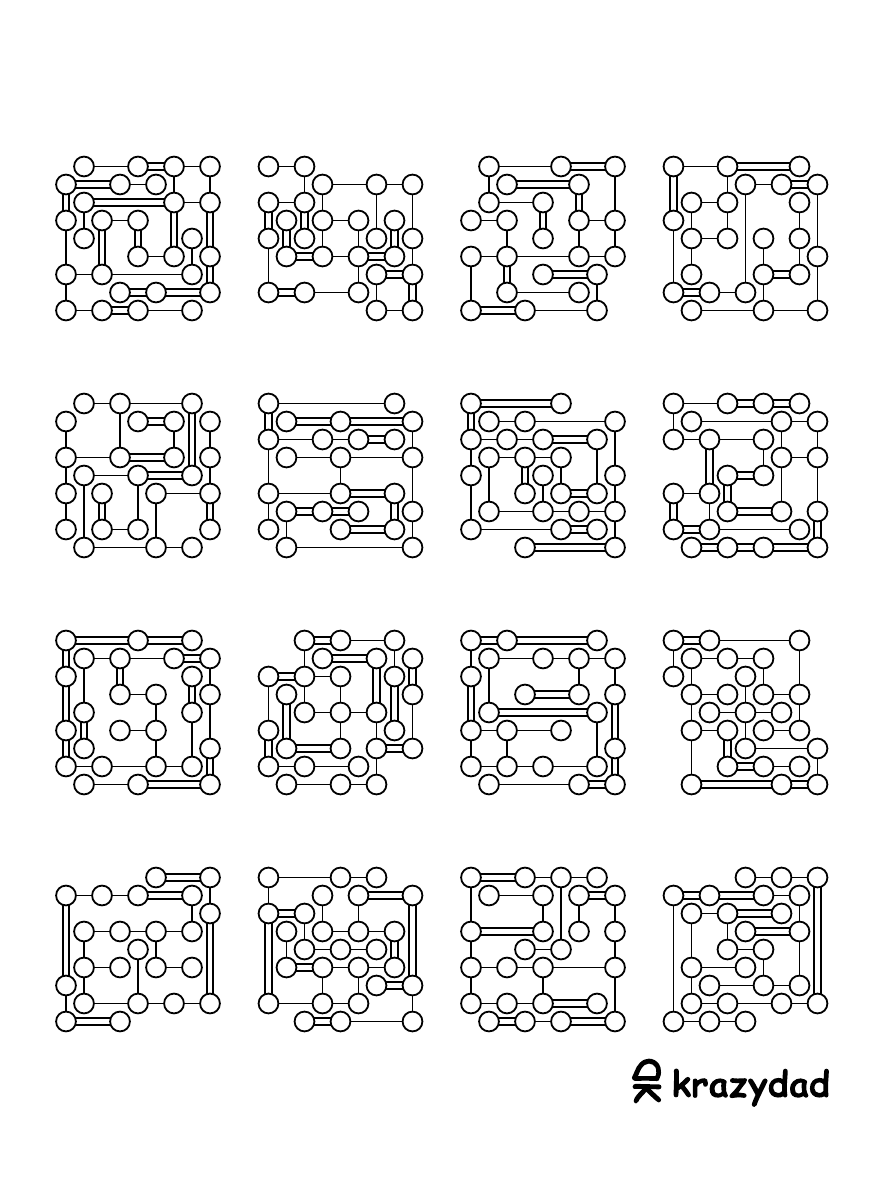
Answers #273 - #288
All puzzles © 2010 KrazyDad.com
Bridges #273
1
3
4
2
4
3
1
3
6
4
3
3
3
1
1
3
3
4
3
4
2
2
4
4
2
3
3
1
Bridges #274
1
2
2
3
2
3
4
2
3
2
2
3
2
1
2
4
4
5
4
3
5
3
3
2
2
3
Bridges #275
2
3
3
2
4
2
3
1
2
4
3
2
2
5
3
2
2
3
3
1
3
3
2
Bridges #276
3
4
2
2
3
3
2
3
1
3
3
2
1
2
2
1
4
3
3
3
2
1
3
2
Bridges #277
1
3
3
1
2
3
1
3
4
3
2
2
4
4
2
2
2
4
1
3
2
2
2
3
1
Bridges #278
3
1
2
4
3
4
2
3
2
1
3
3
3
4
4
3
4
2
2
1
2
4
2
2
Bridges #279
4
2
1
2
2
4
2
3
3
2
4
2
2
1
2
2
3
3
2
3
2
3
2
3
2
2
3
Bridges #280
2
3
4
2
1
3
2
2
4
2
3
3
4
3
3
4
4
3
3
4
4
1
2
4
4
4
Bridges #281
4
4
2
2
4
3
3
4
2
3
2
2
3
3
4
1
3
2
3
3
2
3
2
1
3
4
Bridges #282
3
3
2
2
4
2
3
5
2
3
2
3
2
4
4
3
2
4
3
4
3
3
2
1
1
2
2
Bridges #283
3
4
2
2
2
3
2
3
2
3
3
3
3
4
3
1
4
2
3
2
2
1
3
4
Bridges #284
3
3
2
2
2
2
1
1
3
1
2
3
1
4
1
3
3
1
2
2
2
4
3
1
3
4
3
Bridges #285
2
3
3
2
3
3
3
2
2
3
2
1
3
1
2
1
3
2
3
2
3
3
2
Bridges #286
2
2
1
1
3
4
5
3
1
2
3
3
2
2
1
3
4
3
3
2
5
3
3
2
2
3
2
Bridges #287
3
3
3
1
1
2
3
3
4
3
1
2
1
2
3
2
3
3
2
2
4
2
2
3
3
3
Bridges #288
1
2
3
3
4
3
2
2
4
2
2
4
2
2
3
1
1
3
2
2
2
2
3
2
2
1
Wyszukiwarka
Podobne podstrony:
BR SM b2
BR MD b9
BR SM b3
BR SM b5
BR SM b10
BR LG b9
BR SM b1
BR SM b6
BR SM b7
BR SM b8
BR SM b4
Sm BR SQ on Grid
Program studiów SM IE
2015 06 podst SM
br chemiczne zagroz id 92610 Nieznany (2)
EMP7700 ASM E B SM
więcej podobnych podstron