Chapter 7

POLY-OPTIMISATION OF MINING VENTILATION
IN UNDERGROUND MINES

A. Strumiński	B. Madeja-Strumińska
Wrocław University of Technology	Wrocław University of Technology

ABSTRACT

A concept of the optimal control of the air flow in underground mines is presented in the paper. The method described applies both passive and active networks, in which generated natural sequences have constant values. During the optimisation process the criterion function, which determines the stabilisation of the air currents, the cost of the energy used for ventilation and takes into consideration the operational conditions in the mine, is minimised.

KEYWORDS

Designing, air flow optimisation, safety, cost of energy, operational conditions

introduction

The basic problems of the theory of mine ventilation network are the issues of determination of free and forced flow in the mine (Bystroń 1982, Madeja Strumińska, et. al. 2000).

The determination of the free flow of the air in a mine ventilation network consists in describing directions and streams of air volume (volume expense) in the network distributing the air and fan operation parameters, if we know, a priori the mine ventilation diagrams, air routes resistance and fan characteristics. Moreover for active ventilation networks it is necessary to know the distribution mine air temperatures.

In order to ensure effective methane or climate hazard control and the necessary, intensity ventilation of underground mine workings, according to mining regulations, it is necessary to consider the forced air flows in the mine ventilation networks. This flow is connected with having such installations in the underground mine as separating or choke stoppings, ventilation bridges, supplementary fans etc. The main purpose of this equipment is to direct the appropriate amounts of air to the underground crew's working sites.

The determination of the forced flow in general, is based on establishing the main and supplementary fan stations' ram effect, the dissipation of useful energy in the choke stoppings and the resistance of those stoppings. However it is necessary to know the air volume and the flow directions in the network of air routes, the aerodynamic resistance of those branches and, in the case of an active ventilation network, the temperature of the mine's air.

When designing the air flow, using one of the known methods, (Strumiński 1985) it turns out that the particulars (required) air flow can usually be obtained at the different placings of such elements such as choke stoppings or supplementary fans.

Every variant of the ventilation element lay-out in the mine have their specific properties. They can be both favourable or unfavourable because of the transport of materials, ventilation efficiency, air current stability, costs of energy used for airflow control etc. In specific cases we aim to site the regulation elements underground in the mine, in order to achieve an optimum solution from the point of view of operation, energy consumption or the ventilation network safety. In such way, into the plan of the forced airflow in the mine ventilation network, besides its main purpose i.e. the assurance of the required mine work ventilation intensity, we can also introduce some additional aims.

In this paper - the poly-optimization of the forced air flow in a mine ventilation network, it is understood as such a flow, which beside the basic target is optimal because of the stability of the airflows , costs of energy used for the air flow control and the operational conditions in the mine.

OPTIMisation of the air flow TO ATTAIN air current stability, costs of ventilation energy and the operating conditions in the mine

The safety of the ventilation network means a state of this network enabling the mine crew to work in conditions which do not threaten health and life (Strumiński, et. al. 1994).

As the experiments proved (Bystroń 1975) the hazard caused by a particular air current depends on the useful power dissipation in that current. The lower the dissipation the greater the hazard. Evaluating the air current in underground mines may be achieved using the existing classifications (Bystroń, et. al. 1975, Strumiński, et al. 1994). Especially according to (Bystroń, et. al. 1975) we can distinguish the following air currents: very strong current (
), strong current (1200 W  _f   W), medium current (240 W  _f   W), weak current (50 W  _f   W), very week current (0 W  _f   W). According to earlier knowledge (Bystroń, et al. 1975, Strumiński, et. al. 1994) very weak currents should not be tolerated in mine ventilation networks, because such currents can be a source of very serious hazards for the mine crew during a fire.

Determination of the optimal air flow from the point of ventilation network safety can be reduced to such designing of the forced air flow in the mine, to ensure the supply of required air streams to the mine crew working sites with a simultaneous assurance of the required useful power dissipation in the particular air currents. To solve this problem we usually use the mine works programme and the air amounts determined for particular workings. The useful power dissipation
of the particular air currents is calculated using the following formula

(1)

where:

- useful energy dissipation in the air split
of the ventilation network, J/m³,

- air volume stream (volume expense) in this split, m³/s,

- aerodynamic resistance of this split, kg/m⁷.

The useful power dissipations calculated using the formula (1) are analysed according to the above criteria and those currents are chosen which do not meet the criteria. For those currents there are a priori established such useful power dissipations which meet those criteria For example if the air currents are weak or very weak, then depending on the particular rock-mass conditions power dissipations are established at the level of medium, strong or very strong currents.

Next, already knowing the useful power dissipation the streams of the air volume in the strips are calculated, depending on:

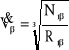
(2)

After that, using the principle for the ventilation network node (I Kirchhoff's law) new air flows for the network is calculated and than again once more the useful power dissipation in each air split of the network and those dissipations meet the assumed a priori criteria.. Therefore, for that airflow, the specific stability of all airflows in the mine ventilation network will be ensured.

Obtaining the required airflow with maintaining the required useful power dissipations generally requires the use of airflow controllers in the mine ventilation network. Type of controllers can be different as well as their spacing within the ventilation network. However we still seek one optimal method of the airflow control from the point of view of air current safety (useful power dissipation).

In order to solve this problem we can use, among other things, the aerodynamic potential properties (Bystroń 1982) which says that the sum of its drop along the closed circuit of each internal mesh is equal zero, which can be presented using the following matrix equations:

(3)

where:

- matrix of the mark factors defined by the formula

, (4)

and
has the value of +1, if the air flow direction in the air split
of the internal mesh
is concordant with assumed direction of movement on the mesh circuit, value of -1, if those directions are not concordant and zero if the air split
is not a part of the chosen internal mesh
;

- vector of aerodynamic potential drops

; (5)

0 - zero vector of m-rows,

m - number of all concerned independent, internal mesh of the network;

b - number of all independent air splits of the network coming into composition of independent internal meshes.

The decrease of the aerodynamic potential in the air split
of the active network satisfies the relationship

(6)

where
and
mean respectively useful energy dissipation in the active split of the network and natural, local sequence generated in that split.

Taking into account the relationship (6) in the matrix equation (1) we get:

(7)

where:

(8)

(9)

In the mine ventilation networks, due to the assumed air flow and because of the need to ensure the specific stability of the air currents (useful power dissipation), the law for the networks mesh is usually not satisfied (II Kirchhoff's law). Therefore the equation (7) is not satisfied either (7).

With the assumption that the natural sequences generated in the active air splits of the ventilation networks are constant and do not depend on the mine working resistance and assuming that useful energy dissipation satisfy the relationship:

(10)

and

(11)

where

and
mean specific resistance of
air split and the air volume stream, respectively, we can deliver the following matrix formula

(12)

In the equation (12) the following designations are introduced

(13)

(14)

The vector elements (14) are determined according to the relationship

(15)

where

(16)

and

(17)

Corrections
in the equations (10) and (12) are the searched unknowns,
is a matrix of unknown coefficients, and
is a vector of free terms.

Further we make an assumption that the corrections of the useful energy
, should be selected to be lowest possible, and the equation (10) has to be satisfied.

According to the above assumptions the corrections should satisfy the condition

(18)

where

j - unit vector of b elements,

L⁽²⁾ - vector of squares of useful energy dissipation correction

(19)

Effective solution of the issue of criterion function minimisation (18) with limits of (12) can be obtained using the method of Lagrange's multipliers (Seidler, et al. 1980). According to this method simultaneous fulfilment of the condition (18) and the matrix equation (12) occurs when

(20)

reaches the minimum, and ^T means the transposed vector of Lagrange's vector of multiplies 

Relationship (20) reaches the local minimum when:

(21)

When differentiating the relationship (20) in respect of variables l_f and equating the first partial derivatives to zero we can obtain the following formula of the corrections of useful energy:

(22)

where

I - unit matrix of b rank,

^T - transposed matrix of  matrix.

Substituting in the equation (12) the L vector, by the right side of the relationship (22) we obtain the equation:

(23)

enabling the unique establishment of vector 

(24)

when
mean the matrix which is inverse to the
matrix.

Knowing the multipliers vector  we can calculate L vector of useful energy dissipation corrections, using the relationship (22).

If any
element of vector L (13) is bigger than zero i.e. when.
, then in the particular air network split it is necessary to install a choke stopping, w where the useful power dissipation should be
. In the case when
, in the particular air current no choke stopping is placed. When, however, vector elements (13) are smaller than zero, i.e. when l_f < 0 inequality is satisfied, then the specific air splits should be equipped with supplementary fans with ram effect of
.

Knowing the useful energy dissipation in the choke stoppings it is possible to calculate aerodynamic resistance of those stoppings and possible cross-section fields of control windows in the choke stoppings (Strumiński 1985).

Then with a knowledge where the choke stoppings and supplementary fans should be located and what are their parameters like, for example energy dissipation in the choke stoppings and supplementary fans ram effect, it is possible to determine the ram effect (depressions) of the main fan station installed in the downcast shafts. (Strumiński 1985).

If in any  air split of the ventilation network it is necessary to place the choke (control) stopping or supplementary fan, than the resulting useful power dissipation of that split determined on the basis of (1) and (10) formulas, can be presented in the form of the relationship:

(25)

Because streams of the air volume
are determined a priori in order to ensure the proper intensity of mine operations ventilation and required stability of the air currents, and energy dissipation corrections l_f are the minimal determined during the optimisation procedure, therefore also final useful energy dissipations (25) are also minimal which can occur in the air splits of the planned ventilation network

Electric power N at the motor terminals of the main fan, which should be paid, taking into consideration the  efficiency of ventilation device including motor and fan, is

(26)

where N_fu (W) is a sum of useful dissipations which took place in the specific air splits of the ventilation network determined from the formula (25).

It is evident that K_p is the cost of energy used during one year by the fan station of N power, is

(27)

and k_j is a price of 1kWh of the electric power.

Therefore the minimal cost of energy used for main ventilation of the mine is connected with using the airflow lay-out where the sum of the power dissipation is as small as possible. This can be obtained by optimising the airflow in the mine ventilation network using the method described in the paper.

In many cases, due to the operating conditions (for example much haulage) it is not possible to install air flow regulators in every air split of the ventilation network. Therefore in such cases we can a priori determine those splits where the choke stoppings or supplementary van can be located. Because the regulators are located only in the strictly determined splits of the network, it is necessary to calculate the corrections of the useful energy dissipation not for the all air splits but only for the determined ones. Therefore in the matrix equation (12) corrections of useful energy dissipation for the splits without ventilation equipment equal zero. Thus using the presented method it is possible to obtain the air flow which is not only optimal due to the stability of the air currents and cost of energy used for the main ventilation but also due to the operating condition. It should be noticed that the planned ventilation network can be in some cases adapted to the free air flow. It means that the mine ventilation network should be designed in such way that it would not be necessary to use choke stoppings or supplementary fans.

Airflows resulting from this method should be maintained a) To ensure stability of flow and b) be sufficient to meet the hazard protection regulations under defined stages of mining operations.

Aerodynamic resistance of the planned mine workings are usually determined on the basis of assumed workings geometry and the type of their support. Changing the geometry of the working, for example its lateral cross-section, it is possible to change the aerodynamic resistance of this working. Therefore it is possible to introduce some changes in the useful energy dissipation amount in the separate air splits of the ventilation network by changing their lateral cross-sections using for example the following relationship (Strumiński 1985)

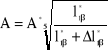
(28)

The formula (28) is correct with the assumption that numbers of specific resistances _f do not depend on the lateral cross-section area of the mine workings but on the type of their support and
, l_f, A^*, A mean respectively: useful energy dissipation determined using the data from the ventilation project (
), correction of that dissipation resulting from the optimisation procedure (l_f), lateral cross-section area of the mine working determined in the project (A^*) and the lateral cross-section area of the workings which assures the free air flow in the ventilation network (A). Some workings, however, should have their cross-section area determined in the project without the possibility of their change due to the necessity of the certain mine machines movement or ensuring the regulatory air velocity. In such cases in the matrix equation (12), l_f correction will be equal zero.

When the calculations show the necessity of enlarging or reducing the cross-section areas of some mine workings, and the operational considerations allow for such change, then the working should be made in bigger or smaller dimensions. Otherwise it will be necessary to use the underground supplementary fans or choke stoppings.

Conclusion

The method concept presented in the paper concerning the optimisation of the mine air flow can be used practically in underground mines. It especially enables design of a ventilation network such that the ventilation will be optimal taking into account safety, costs of electric power used for air distribution and the operating conditions like transport, mine machines movement etc.

Using this method requires knowledge about the mine ventilation lay-out, aerodynamic resistance of the mine workings, planned amounts of air necessary for the proper work places ventilation, criterion values of the useful power dissipation in the air splits of the ventilation network and in the case of active networks, additionally, generated in those air splits, natural thrusts (natural depressions).

references

Bystroń H., 1975, „Stability of air currents of the mine ventilation network”, Mine Safety Conference, Washington

Bystroń H., Baranowski J., Madeja B., Strachacki A., 1975, „Design methods of the forced air flows in the passive ventilation networks with depending currents”, Prace GIG, Announcement no 634, Katowice

Bystroń H., 1982, „Basic notions and relations describing quasi-stationary flow of the wet air in the working of the underground mine”, Przegląd Górniczy, nr 11-12

Madeja-Strumińska B., Rosiek F., Sikora M., Strumiński A., Urbański J., Turkiewicz W., Wach J., 2000, „Problems of safety, economic effectiveness and the ventilation optimisation of the mine panes in copper mines”. Oficyna Wyd. Politechniki Wrocławskiej, Wrocław

Seidler J., Badach A., Molisz W., 1980, Methods of solving the optimisation problems. Wyd. Naukowo-Techniczne, Warszawa

Strumiński A., 1985, Optimisation of air flows in the planned ventilation networks. Zakład Narodowy im. Ossolińskich, Wrocław

Strumiński A., Madeja-Strumińska B., 1994, „Forming the power dissipation in the aspect of the ventilation network stability”, XX Dni Techniki ROW - XI Seminarium, Wodzisław Śl.

2

I SZKOŁA AEROLOGII GÓRNICZEJ 1999

5

44

PROCEEDINGS OF THE 7^TH INTERNATIONAL MINE VENTILATION CONGRESS

45

POLY-OPTIMISATION OF MINING VENTILATION

---