Opracowanie: Małgorzata Sztokfisz
WYKŁAD 5
KONSTRUKCJA CAŁKI WZGLĘDEM MIARY
(Ω, U, μ) - przestrzeń z miarą
ƒ: Ω→R
I ETAP KONSTRUKCJI CAŁKI WZGLĘDEM MIARY
DEFINICJA 5.1 (FUNKCJA PROSTA)
ƒ - funkcja prosta :⇔ jeżeli funkcja przyjmuje skończoną ilość wartości {α1, ..., αn}
DEFINICJA 5.2 (FUNKCJA CHARAKTERYSTYCZNA)
E∈U
χE : Ω→R ∧ 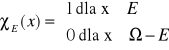
WNIOSEK 5.1 (POSTAĆ NORMALNA FUNKCJI PROSTEJ)
ƒ - funkcja prosta (definicja 5.1.)
![]()
Objaśnienie:
Gdy: x∈Ek , to: L=ƒ(x)= αk ![]()
⇒ L=P
Każda funkcja prosta jest kombinacją liniową funkcji charakterystycznych.
Istnieje nieskończenie wiele postaci normalnych funkcji prostej.
TWIERDZENIE 5.1 (WŁASNOŚCI FUNKCJI PROSTYCH)
ε - zbiór wszystkich funkcji prostych określonych na Ω
∀ƒ,g∈ε ∀α,β∈R (αƒ+βg)∈ ε
∀ƒ,g∈ε sup.{ƒ,g }∈ ∧ inf{ƒ,g}∈ ε
∀ x∈Ω (sup.{ƒ,g })(x)=max{ƒ(x),g(x)}
∀ x∈Ω (inf.{ƒ,g })(x)=min{ƒ(x),g(x)}
∀ƒ∈ε ƒ∈ ε
∀ƒ∈ε ∀E∈U ƒχE∈ ε
∀ƒ,g∈ε (ƒg)∈ ε
Dowód:
Ad. 1) Niech:
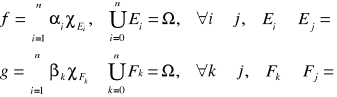
x={ Ei∩Fj: i={0, ..., n}∧ j={0, ..., m}}
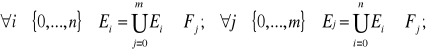
Uwaga:
A∩B=∅ to χA∪B = χA+χB
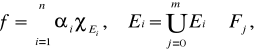
gdzie Ei∩Fj są parami rozłączne,
tzn. (Ei∩Fj)∩( Ei∩Fk)=∅ dla ![]()
![]()
![]()
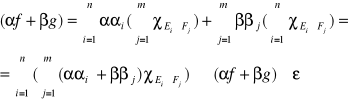
Ad. 2.)
korzystając z ad. 1) można zapisać:
![]()
![]()
analogicznie:
![]()
Ad. 3.)
![]()
![]()
Ad. 4.)
![]()
DEFINICJA 5.3 (FUNKCJA PROSTA CAŁKOWALNA)
![]()
ƒ - funkcja prosta całkowalna :⇔
DEFINICJA 5.4 (CAŁKA Z FUNKCJI PROSTEJ)
ƒ - funkcja prosta całkowalna ![]()
![]()
TWIERDZENIE 5.2
Wartość całki nie zależy od postaci normalnej funkcji prostej.
Z. ƒ - funkcja prosta całkowalna
![]()
![]()
Ei pokrywają Ω i są parami rozłączne
Fj pokrywają Ω i są parami rozłączne
D.
Analogicznie jak w dowodzie ad. 1.) (twierdzenie 5.1.)
![]()
![]()
![]()
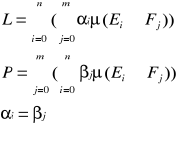
⇒ L=P
TWIERDZENIE 5.3 (WŁASNOŚCI CAŁKI Z FUNKCJI PROSTEJ)
funkcji prostych całkowalnych
![]()
całkowalna
![]()
E∈U ∧ μ(E)<∞ to:
![]()
bo: αi=1
ƒ - funkcja prosta całkowalna ∧ ƒ≥0 ⇒
Dowód:
na podstawie dowodu ad. 1.) (twierdzenie 5.1.)
Ad. 1.)
![]()
![]()
![]()
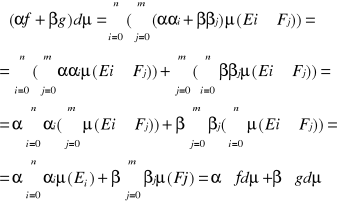
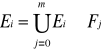
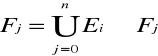
![]()
![]()
(zbiory ![]()
są parami rozłączne)
Ad. 2.)
![]()
![]()
![]()
Ad. 3.)
ƒ>0 ∧ ![]()
⇒ ![]()
,
bo: ![]()
II ETAP KONSTRUKCJI CAŁKI WZGLĘDEM MIARY
ε+ - zbiór całkowalnych funkcji prostych nieujemnych
TWIERDZENIE 5.4
Z. (un)n∈N⊂ε+, un
(vn)n∈N⊂ε+, vn
D.
ε1+={ƒ: Ω→R+, ∃(un) ⊂ε+, un ∧ ![]()
}
{ ![]()
:⇔ ∀x∈R ![]()
}
DEFINICJA 5.5 (CAŁKA Z FUNKCJI NIEUJEMNEJ)
Niech: ƒ∈ε1+ ∧ ![]()
, (un) ⊂ε+ ∧ un
![]()
:= ![]()
WNIOSEK 5.2
z twierdzenia 5.4. wynika, że całka z funkcji nieujemnej nie zależy od wyboru ciągu funkcji prostych
(un)∈ε+ ∧ un ∧ ![]()
∀ƒ,g∈ε1+ ∀α,β∈R+
(αƒ+βg)∈ε1+ ∧ ![]()
∀ƒ,g∈ε1+, (ƒg)∈ε1+ ∧ sup{ƒ,g}∈ε1+ ∧ inf{ƒ,g}∈ε1+
ƒ≤g ⇒
(własności 2), 3), 4) wynikają z odpowiednich własności całki z funkcji prostych i własności granic)
Dowód:
Ad. 2)
![]()
, un∈ε+ ∧ un
![]()
, vn∈ε+ ∧ vn
α,β∈R+ (αƒ+βg)=![]()
α,β∈R+ ∧ un ∧ vn ⇒ ![]()
∧ ![]()
∈ε+
⇒ ![]()
∈ε1+ ∧
![]()
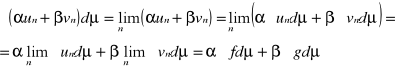
III ETAP KONSTRUKCJI CAŁKI WZGLĘDEM MIARY
DEFINICJA 5.6 (FUNKCJA CAŁKOWALNA)
Niech:
ƒ: Ω→R
ƒ+: Ω ∋ →ƒ+(x) := max {ƒ (x), 0}
ƒ-: Ω∋ →ƒ_(x) := max {-ƒ (x), 0}
ƒ = ƒ+ - ƒ-
ƒ - μ- całkowalna :⇔ ƒ+, ƒ- są μ- całkowalne
tzn. ∃ ((un)⊂ε+, un ∧ (vn)⊂ε+, vn )
![]()
![]()
∧ ![]()
![]()
:= ![]()
Uwaga:
L1(μ) - zbiór funkcji μ- całkowalnych
ε+ ⊂ ε1+ ⊂ L1(μ)
KONSTRUKCJA CAŁKI WZGLĘDEM MIARY
- PODSUMOWANIE -
Całka z funkcji prostej
![]()
to ![]()
Całka z funkcji nieujemnej
![]()
, un⊂ε+ ∧ un
to: ![]()
Całka z dowolnej funkcji μ- całkowalnej
ƒ∈L1(μ) ∧ ƒ = ƒ+-ƒ- ∧ ƒ+, ƒ-∈ ε1+
to: ![]()
DEFINICJA 5.7 (CAŁKA PO ZBIORZE)
E∈U E - zbiór mierzalny
![]()
TWIERDZENIE 5.5 (WŁASNOŚCI CAŁKI)
∀ƒ,g∈L1 (μ) ∀α,β∈R
(αƒ+βg)∈ L1(μ) ∧ ![]()
∀ƒ∈L1(μ) ƒ∈L1(μ)

∀ƒ,g∈L1(μ) ƒ≤g ⇒
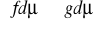
(En)n∈N⊂U ∧ ∀i≠j Ei∩Ej=∅
to: 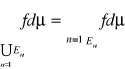
Wszystkie te własności wynikają z odpowiednich właściwości całki z funkcji nieujemnej.
Ważne: (wniosek)
μ(E)=0 ⇒
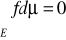

⇒
Dowód:
Ad.1)

,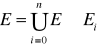
μ(E)=0 ⇒ ∀i∈{0, ..., n} μ(E∩Ei)=0 ![]()
![]()
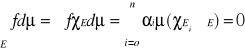
ƒ∈ε1+,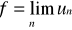
∧ un
![]()
,
bo ![]()
(I ETAP)
ƒ = ƒ+ - ƒ-=0, bo ƒ+=0, ƒ-=0
![]()
,
bo na podstawie 2): ![]()
1
α1
α2
α3
E1
E0
E2
ƒ- funkcja prosta :⇔
:⇔ ∃(α1, ..., αn∈R ∧ E1, ..., En∈U
∧ Ei ∩Ej =∅ dla i≠j) 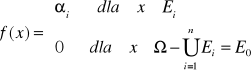
1
E
y=ƒ(x)
y=g(x)
E3
E1
E4
E2
F1
F2
F3
α1
α2
α3
α4
1
B
A
![]()
E
(*)
*
*
y=(inf.{ƒ,g })(x)
y=(sup.{ƒ,g })(x)
Wyszukiwarka