2

Mysteries and Secrets

...the whole procedure was an act of despair because a theoretical interpre-
tation had to be found at any price, no matter how high that might be...
(Planck on the statistical mechanics basis of his radiation law)

Sommerfeld’s very exhaustive discussion of Couette flow led to the conclu-
sion that this type of flow remains stable for all viscosities. For a time, after
this negative result had been obtained, it was thought that the method of
small oscillations was unsuitable for the theoretical solution of the prob-
lem of transition to turbulence. It transpired later that this view was not
justified, because Couette flow is a very special and restricted example.
(Schlichting in Boundary Layer Theory, p 465, McGraw-Hill 1979)

2.1 Mysteries

We shall also demonstrate the usefulness of computational turbulence in basic
science by resolving the following unsolved mysteries, which have haunted
scientists over centuries:

• d’Alembert’s Mystery: Zero drag of inviscid flow,

• Loschmidt’s Mystery: Violation of the 2nd law of thermodynamics,

• Sommerfeld’s Mystery: Stability of Couette flow.

All these mysteries reflect paradoxes, where phenomena predicted by math-
ematics are not at all observed in reality. Since science is supposed to be
rational and based on mathematics, paradoxes are catastrophic for the credi-
bility of science, and thus have to be resolved (or covered up), in one way or
the other, at any price.

In d’Alembert’s Mystery formulated in 1752 [29], mathematics predicts

that a body may move through a fluid with zero (very small) viscosity, like air
and water, with zero (very small) resistance or drag. But everybody knows that
this is impossible; the drag increases roughly quadratically with the velocity
and becomes very substantial for higher velocities.

30

2 Mysteries and Secrets

In Loschmidt’s Mystery formulated in 1876 [84], mathematics of systems

with zero viscosity predicts that time reversal and a perpetuum mobile is pos-
sible. But everybody knows that time is always moving forward and that a
perpetuum mobile is impossible, as expressed by the 2nd law of thermody-
namics.

In Sommerfeld’s Mystery from 1908 [104], mathematics predicts that the

simplest of all flows, Couette flow with a stationary linear velocity profile, is
stable and thus should exist. But nobody has ever observed this flow in a fluid
with small viscosity.

The cover up of d’Alembert’s Mystery is to blame the assumption of zero

viscosity for the erroneous prediction: In reality there is always some possibly
very very small viscosity (of some nature), which changes everything. We will
below argue that such explanations are not scientifically satisfactory and we
shall instead present a new resolution based on computational turbulence in
the inviscid Euler equations.

The cover up of Loschmidt’s Mystery is to introduce statistical mechan-

ics based on microscopic games of roulette. We will below argue that such
an explanation is cumbersome scientifically, and we shall instead present a
new resolution demonstrated through computational turbulence in the Euler
equations.

The cover up of Sommerfeld’s Mystery is to say that a linear velocity

profile is too simple for the mathematical theory to apply, which evidently
is not scientifically satisfactory either. We will below computationally study
Couette flow and we will find that it is not stable, just as observed. After this
experience we will be able to theoretically understand, using mathematics and
avoiding the pitfall of Sommerfeld, why Couette flow is not stable.

2.2 Secrets

As applications of computational turbulence we shall uncover (some of the)
secrets of the following activities based on turbulent incompressible flow of air
and water:

• ball sports,

• flying,

• sailing,

• racing.

In all ball sports including football (soccer), baseball, tennis, golf and

table-tennis, the player that can control the spin of the ball to give it a de-
sired curved path, has an important advantage. The fluid mechanics giving
the spinning ball a curved path, depending on the direction of the spin, is re-
ferred to as the Magnus effect which creates a lift force perpendicular to the
direction of motion and spin. Below we will study aerodynamics of ball sports

2.2 Secrets

31

computationally including the dependence on the spin, speed and the rough-
ness of the surface of the ball. Of course, the flow of air around a spinning
ball is turbulent (Fig. 2.1).

Fig. 2.1. Turbulent flow around a spinning ball.

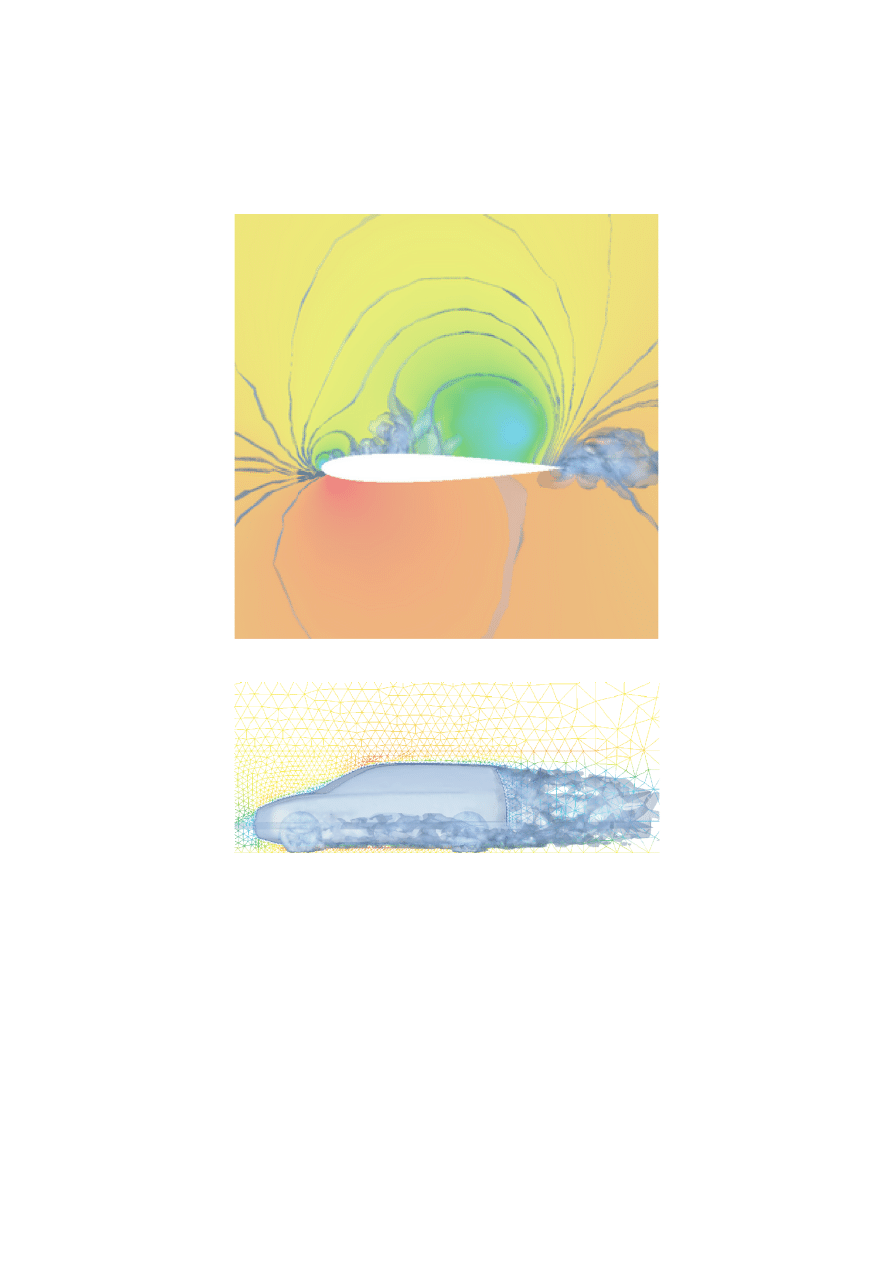
To understand why flying is possible, we will below simulate the turbulent

flow around a wing (Fig. 2.2), and this way uncover how the necessary lift and
unavoidable drag is generated. We shall also see that without computational
turbulence it is impossible to mathematically predict lift and drag, in partic-
ular at take-off and landing, where the angle of attack of the wing against
the flow is large and the flow is very turbulent. We shall thus compute the
turbulent flow around a wing at different angles of attack and discover that
the flow features are very different for the low angle of attack at cruising speed
and the high angle of attack at take-off and landing. We shall see that clas-
sical analytical mathematical methods may give fairly reasonable predictions
for (very) long wings at small angles of attack, but not so for normal wings
and/or large angles of attack. To cruise at 30 000 feet is one thing, and to
take off and land a completely different game.

Sailing is similar to flying from a fluid mechanics point of view, with the

sail when going against the wind acting like a wing giving a lift force pulling
the sail and boat against the wind but also tilting the boat. Needless to say,
the flow of air around a sail is turbulent, and thus computational turbulence
certainly opens new insights into the art of sailing and how to win Americas
Cup. Also the keel of a sailing boat acts like a wing and gives a pull partly
balancing the side force from the sail.

Modern cars are designed to have small drag, since fuel consumption di-

rectly couples to drag, and for racing cars also the lift is of concern since a
flying racing car is hard to control. Computational turbulence offers new pos-
sibilities of car design, since traditional experimental testing of prototypes in
wind tunnels is very slow and costly (Fig. 2.3).

32

2 Mysteries and Secrets

Fig. 2.2. Turbulent flow around a wing.

Fig. 2.3. Turbulent flow around a car (geometry courtesy of Volvo Car Corpora-
tion).