The sound field

and how it is measured

Jakob Christensen-Dalsgaard,

Jakob Christensen-Dalsgaard,

CSC

CSC

Contents:

1.

1.

Introduction and definition of the sound

Introduction and definition of the sound

field

field

2.

2.

Parameters of sound

Parameters of sound

3.

3.

Sound emitters – acoustic monopoles and

Sound emitters – acoustic monopoles and

dipoles

dipoles

4.

4.

Manipulations of the sound field

Manipulations of the sound field

5.

5.

Measuring the sound field

Measuring the sound field

a) by the animals

a) by the animals

b) by microphones

b) by microphones

The sound field –
introduction 1



Strict definition : the sound field is

Strict definition : the sound field is

the

the

pressure gradient

pressure gradient

, i.e. the

, i.e. the

particle acceleration radiating

particle acceleration radiating

from the sound source.

from the sound source.

- an analogue to the electrical field

- an analogue to the electrical field

(the potential gradient or force

(the potential gradient or force

acting on a unit charge)

acting on a unit charge)

’Colloquial’ uses of the term
’sound field’:



Near field (1)

Near field (1)

, the region near the sound

, the region near the sound

emitter where medium motion is

emitter where medium motion is

dominated by local hydrodynamic flow –

dominated by local hydrodynamic flow –

also called the

also called the

hydrodynamic near field

hydrodynamic near field



Near field (2)

Near field (2)

, the region near the sound

, the region near the sound

emitter where sound radiation is complex

emitter where sound radiation is complex

due to interferences between sound

due to interferences between sound

radiated from different regions – also

radiated from different regions – also

called the

called the

geometric near field

geometric near field



Far field

Far field

, the region far from the sound

, the region far from the sound

emitter where medium motion is

emitter where medium motion is

dominated by the propagating sound wave

dominated by the propagating sound wave

Colloquial uses of ’sound
field’ 2



Free sound field

Free sound field

, i.e. a sound field without

, i.e. a sound field without

reflected components far away from emitter

reflected components far away from emitter



Diffuse sound field,

Diffuse sound field,

a sound field with

a sound field with

reflected component and ultimately zero

reflected component and ultimately zero

radiated sound energy

radiated sound energy



The term

The term

’closed-field sound’

’closed-field sound’

is used for

is used for

sound in small enclosures (earphone

sound in small enclosures (earphone

couplers) that are essentially pressure

couplers) that are essentially pressure

chambers.

chambers.

Pressure and motion
parameters of sound



The sound wave propagates in an elastic

The sound wave propagates in an elastic

medium and generates alternating

medium and generates alternating

condensations and rarefactions of the

condensations and rarefactions of the

medium particles

medium particles



The particles are displaced and oscillate

The particles are displaced and oscillate

in

in

the propagation direction

the propagation direction

around their

around their

rest position (no net movements although

rest position (no net movements although

the sound wave propagates)

the sound wave propagates)

(an acoustic particle is a ’tiny bulk’ of medium,

(an acoustic particle is a ’tiny bulk’ of medium,

so small that it can be regarded as a unit and

so small that it can be regarded as a unit and

so big that it retains fluid properties)

so big that it retains fluid properties)

Motion parameters of sound

Three related parameters are used:

Three related parameters are used:

1.

1.

Displacement

Displacement

, x(t)

, x(t)

2.

2.

Velocity

Velocity

3.

3.

Acceleration

Acceleration

NB. Particle velocity should not be confused with

NB. Particle velocity should not be confused with

sound velocity. Particle velocity is proportional

sound velocity. Particle velocity is proportional

to source level, whereas sound velocity is a

to source level, whereas sound velocity is a

constant only depending on properties of the

constant only depending on properties of the

medium.

medium.

t

x

t

v











)

(

2

2

)

(

t

x

t

v

t

a



















Motion parameters of
sound 2



The medium motion parameters are

The medium motion parameters are

vectors

vectors

parallel to the propagation direction of the

parallel to the propagation direction of the

sound wave and thus

sound wave and thus

directional

directional



Sound pressure

Sound pressure

, in contrast, is non-

, in contrast, is non-

directional

directional



However, the

However, the

pressure gradient

pressure gradient

is directional

is directional



Note that the motion parameters are

Note that the motion parameters are

ambiguous

ambiguous

- the particles oscillate both

- the particles oscillate both

parallel

parallel

and

and

antiparallel

antiparallel

to the sound

to the sound

propagation direction.

propagation direction.

1

2

3

Propagation of the sound
wave.



The figure shows the time course of displacement experienced

The figure shows the time course of displacement experienced

by each of the acoustic particles as the sound propagates

by each of the acoustic particles as the sound propagates

(direction shown by left arrow) (note that the particles are

(direction shown by left arrow) (note that the particles are

displaced along the axis (black line) only).

displaced along the axis (black line) only).



Particle 1 leads and at the instant when particle 2 has its peak

Particle 1 leads and at the instant when particle 2 has its peak

velocity - at rest position – particle 1 and 3 move against it,

velocity - at rest position – particle 1 and 3 move against it,

creating a peak pressure.

creating a peak pressure.

Therefore, the particle velocity is

Therefore, the particle velocity is

in phase with the pressure in the propagating sound wave.

in phase with the pressure in the propagating sound wave.

Particle velocity



Close to the sound source there is no simple relation

Close to the sound source there is no simple relation

between pressure and particle velocity. Velocity must be

between pressure and particle velocity. Velocity must be

measured independently

measured independently



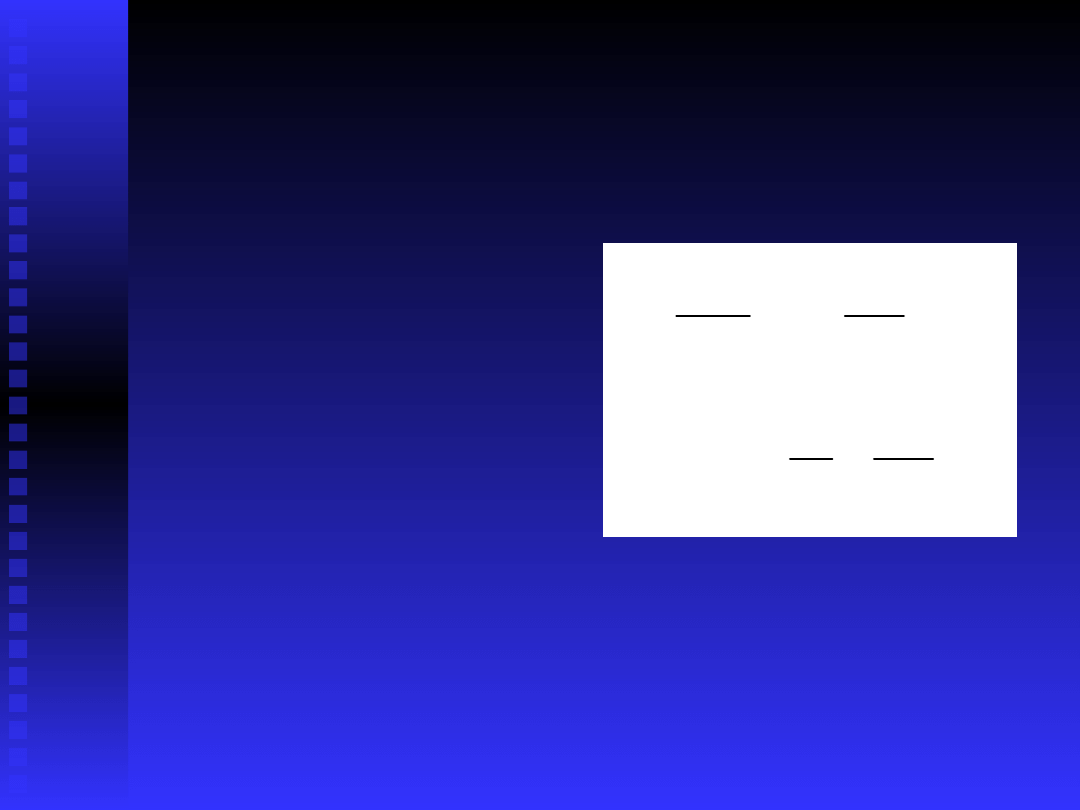
From Newtons 2. Law,

From Newtons 2. Law,



Thus, velocity is proportional to the integral of the

Thus, velocity is proportional to the integral of the

pressure gradient

pressure gradient



Note that particle velocities are much smaller in water

Note that particle velocities are much smaller in water

than in air (by a factor 3570 for identical sound pressures)

than in air (by a factor 3570 for identical sound pressures)

dt

r

p

v

r

p

t

v

r

r

































1

Particle velocity 2

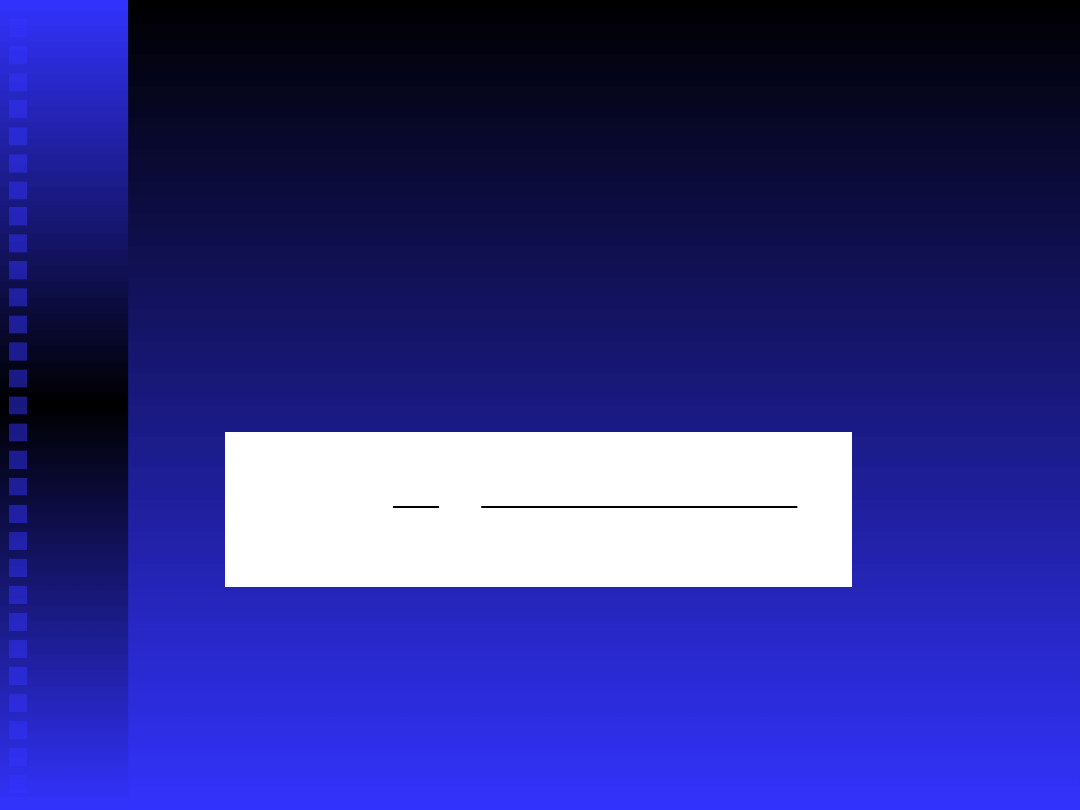
Particle velocity can be measured by estimating the

Particle velocity can be measured by estimating the

pressure gradient.

pressure gradient.

This is done by measuring the pressure difference

This is done by measuring the pressure difference

on two closely spaced hydrophones or

on two closely spaced hydrophones or

microphones, integrating and scaling,

microphones, integrating and scaling,

i.e.

i.e.

Note that this is the velocity component on the axis

Note that this is the velocity component on the axis

of the two transducers. There are two additional

of the two transducers. There are two additional

orthogonal components of particle velocity.

orthogonal components of particle velocity.





dt

r

t

p

t

p

t

v

r









)

(

)

(

1

)

(

2

1



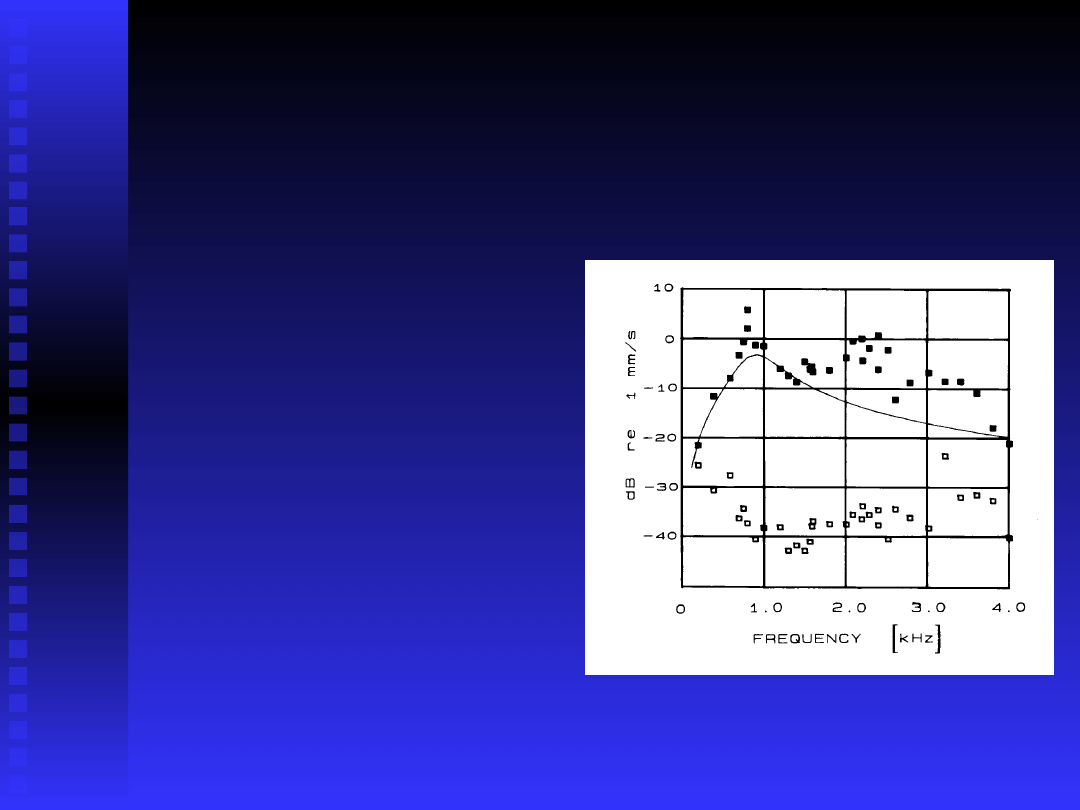
Particle velocity
measurements-
an example



The figure shows laser

The figure shows laser

measurements of

measurements of

clawed frog tympanic

clawed frog tympanic

disk vibrations (filled

disk vibrations (filled

squares) and particle

squares) and particle

velocities measured

velocities measured

using the pressure

using the pressure

gradient method (two

gradient method (two

closely spaced

closely spaced

hydrophones)

hydrophones)

(from Christensen-

(from Christensen-

Dalsgaard et al. 1990)

Dalsgaard et al. 1990)

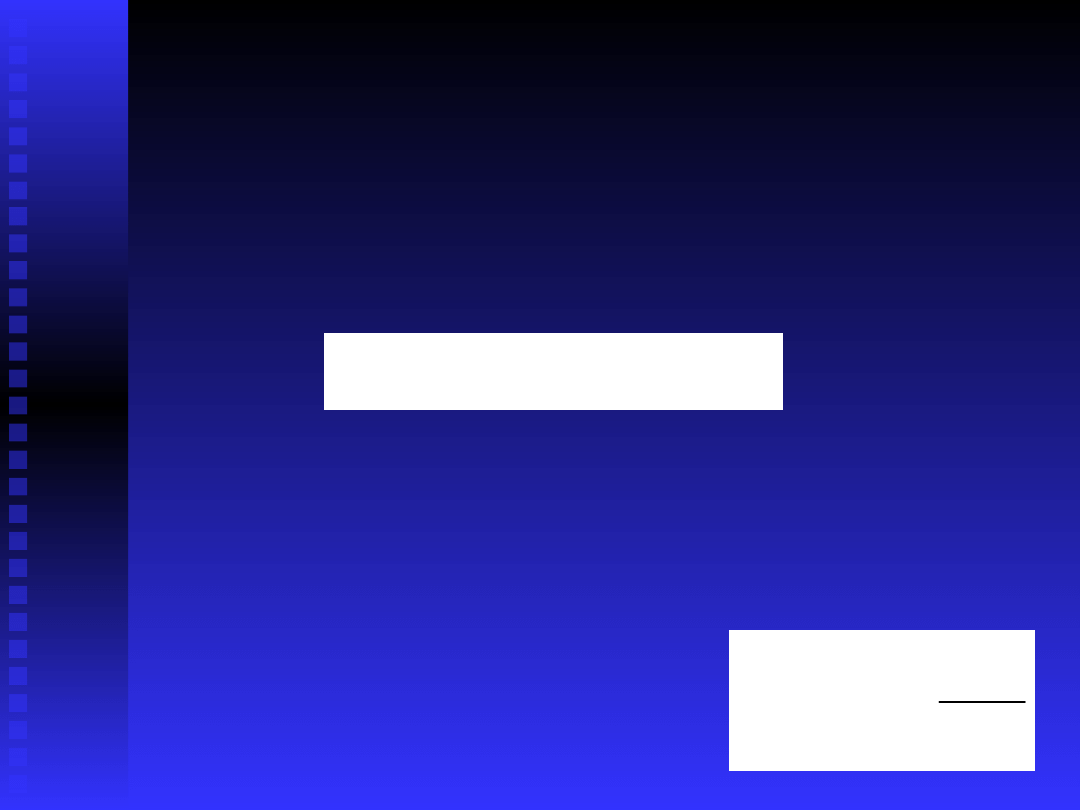
Sound intensity 1



Far away from the sound source

Far away from the sound source

(local flow is negligible) sound

(local flow is negligible) sound

pressure and particle velocity are

pressure and particle velocity are

related by Ohms acoustical law

related by Ohms acoustical law



where Z is the characteristic

where Z is the characteristic

impedance of the medium,

impedance of the medium,





the

the

density and c the speed of sound

density and c the speed of sound



Here sound intensity (energy flow

Here sound intensity (energy flow

per unit area) can be calculated as:

per unit area) can be calculated as:

c

Z

Z

v

p











,

c

p

v

p

I











2

Sound intensity 2



Sound intensity is calculated from the particle

Sound intensity is calculated from the particle

velocity as the time average of pressure and

velocity as the time average of pressure and

particle velocity:

particle velocity:



Note that velocity components 90 deg out of

Note that velocity components 90 deg out of

phase with pressure cancel. These components

phase with pressure cancel. These components

belong to the reactive, non-propagating sound

belong to the reactive, non-propagating sound

field. Examples are standing waves, local flow

field. Examples are standing waves, local flow

near the sound source, but also in diffuse

near the sound source, but also in diffuse

sound fields the intensity vector will vanish.

sound fields the intensity vector will vanish.

r

r

v

p

I







The acoustic monopole

Two kinds of disturbances generated

Two kinds of disturbances generated

by the monopole:

by the monopole:

1.

1.

Local flow-medium displaced

Local flow-medium displaced

radially by pulsations of sphere

radially by pulsations of sphere

2.

2.

Propagating sound wave radiating

Propagating sound wave radiating

out from sphere

out from sphere

In the monopole, local flow vectors are

In the monopole, local flow vectors are

aligned with sound propagation

aligned with sound propagation

direction

direction

Acoustic monopole-
animation

http://www.kettering.edu/~drussell/demos.html

The acoustic monopole 2

The two terms mentioned above show up in the equation for

The two terms mentioned above show up in the equation for

radial particle velocity (r distance, U

radial particle velocity (r distance, U

0

0

source velocity, k

source velocity, k

wave number)

wave number)

(sound-wave term)

(sound-wave term)

(local flow term)

(local flow term)

Pressure is given by the equation:

Pressure is given by the equation:

Thus, in the sound wave

Thus, in the sound wave

term, pressure and velocity

term, pressure and velocity

are in phase. Pressure and local flow velocity are 90 deg.

are in phase. Pressure and local flow velocity are 90 deg.

out of phase.

out of phase.









kr

t

U

r

a

kr

t

U

r

ka

v















cos

)

sin

0

2

2

0

2





kr

t

U

r

cka

p











sin

0

2

The acoustic dipole
(translating sphere)

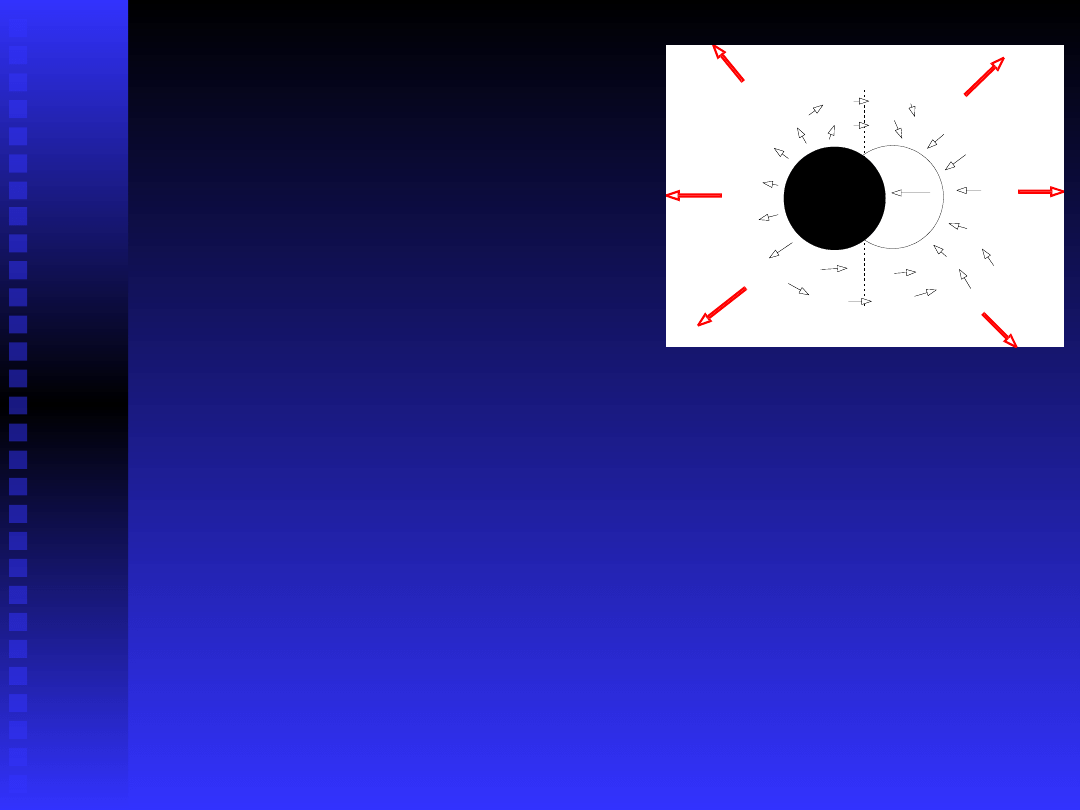
The acoustic dipole is equi-

The acoustic dipole is equi-

valent to two monopoles 180

valent to two monopoles 180

deg out of phase.Therefore, at

deg out of phase.Therefore, at

equal distances from the centers of the monopoles,

equal distances from the centers of the monopoles,

sound pressures cancel (stippled line), i.e. sound

sound pressures cancel (stippled line), i.e. sound

radiates in a 'figure-eight'-pattern (red arrows).

radiates in a 'figure-eight'-pattern (red arrows).

Local flow field is shown by arrows. If wavelength is

Local flow field is shown by arrows. If wavelength is

large compared to sphere, sound emission is

large compared to sphere, sound emission is

'short-circuited' by local flow. Note that, unlike

'short-circuited' by local flow. Note that, unlike

the monopole the dipole local flow field is not

the monopole the dipole local flow field is not

aligned with the sound field.

aligned with the sound field.

Acoustic dipole -
animation

http://www.kettering.edu/~drussell/demos.html

The acoustic quadrupole



A quadrupole is two connected

A quadrupole is two connected

dipoles. The sound emission is

dipoles. The sound emission is

more complicated, and only an

more complicated, and only an

animation will be shown here:

animation will be shown here:

http://www.kettering.edu/~drussell/demos.html

Local flow vs. near/far
field

Traditionally, the local flow has been called a near-

Traditionally, the local flow has been called a near-

field effect. Near/far fields are not very precise

field effect. Near/far fields are not very precise

terms, however, (for one thing, ’near field’ is

terms, however, (for one thing, ’near field’ is

used for two different effects) and should be

used for two different effects) and should be

avoided for the following reasons:

avoided for the following reasons:



1) Animals have receptors for medium motion

1) Animals have receptors for medium motion

or sound pressure. Hence, any motion or sound

or sound pressure. Hence, any motion or sound

pressure whether originating from local flow or

pressure whether originating from local flow or

sound wave can stimulate the relevant

sound wave can stimulate the relevant

receptors - i.e. there are no specialized near-

receptors - i.e. there are no specialized near-

field/far field receptors.

field/far field receptors.

Local flow vs. Near/far
field 2



2) The rules of thumb for ’extension’ of the near

2) The rules of thumb for ’extension’ of the near

field (e.g. 1/6th wavelength) only hold for

field (e.g. 1/6th wavelength) only hold for

monopole sound emitters. For dipoles and

monopole sound emitters. For dipoles and

quadrupoles, the local flow continues to

quadrupoles, the local flow continues to

dominate at infinite distances at some

dominate at infinite distances at some

directions.

directions.

It is recommended to distinguish between the

It is recommended to distinguish between the

local hydrodynamic flow and the sound wave. It

local hydrodynamic flow and the sound wave. It

is also recommended to

is also recommended to

measure the medium

measure the medium

motion

motion

when working within a wavelength of

when working within a wavelength of

the sound emitter.

the sound emitter.

Manipulations of the
sound field

1. Local flow/sound considerations:

1. Local flow/sound considerations:

Most important for

Most important for



low frequencies

low frequencies



Underwater sound.

Underwater sound.

There is no way to avoid local flow generation by a

There is no way to avoid local flow generation by a

sound emitter.

sound emitter.

Move away from sound emitter (at least a wavelength)

Move away from sound emitter (at least a wavelength)

If you are interested in particle motion sensitivity

If you are interested in particle motion sensitivity

minimize sound emission of stimulator (use small

minimize sound emission of stimulator (use small

vibrating spheres or air puffs) and

vibrating spheres or air puffs) and

Calibrate the motion component directly

Calibrate the motion component directly

Standing wave tubes



In a standing wave, sound pressure

In a standing wave, sound pressure

and particle velocity are 90 deg out of

and particle velocity are 90 deg out of

phase, so distinct pressure and

phase, so distinct pressure and

velocity nodes form in a standing

velocity nodes form in a standing

wave tube. Such devices have

wave tube. Such devices have

traditionally been used to investigate

traditionally been used to investigate

whether ears responded to the

whether ears responded to the

pressure or velocity component of

pressure or velocity component of

sound

sound

Diffuse/free sound fields



For investigations of directional hearing it is

For investigations of directional hearing it is

desirable to avoid reflected components in the

desirable to avoid reflected components in the

sound field, i.e. to work in a free sound field.

sound field, i.e. to work in a free sound field.



The most obvious solution is an anechoic room

The most obvious solution is an anechoic room

with structures that absorb reflections.

with structures that absorb reflections.



Anechoic rooms are nearly always too small

Anechoic rooms are nearly always too small

(making it difficult to avoid reflections at low

(making it difficult to avoid reflections at low

frequencies)

frequencies)



Audiometric cabins (such as the IAC) are

Audiometric cabins (such as the IAC) are

sound- proof, but not really anechoic, at least

sound- proof, but not really anechoic, at least

not below 1000 Hz.

not below 1000 Hz.

Free sound fields



Reflections can be removed digitally:

Reflections can be removed digitally:



If the reflections do not overlap the

If the reflections do not overlap the

investigated structures’ impulse

investigated structures’ impulse

response, short transients can be

response, short transients can be

used to excite the structure A time

used to excite the structure A time

window is chosen that just contains

window is chosen that just contains

the impulse response and eliminates

the impulse response and eliminates

the echoes.

the echoes.

Loudspeakers:directivity,
radiation, baffles



Loudspeakers vary tremendously in

Loudspeakers vary tremendously in

the sound field they generate. It is up

the sound field they generate. It is up

to the experimenter to select/build

to the experimenter to select/build

omnidirectional speakers or very

omnidirectional speakers or very

directional ones depending on the

directional ones depending on the

question asked.

question asked.



The low-frequency radiation of

The low-frequency radiation of

speakers can be improved dramatically

speakers can be improved dramatically

by baffles.

by baffles.

Measuring the sound field



1) by animals:

1) by animals:



The two parameters of sound: Sound pressure is

The two parameters of sound: Sound pressure is

non-directional. Typical receivers are closed with

non-directional. Typical receivers are closed with

sound access from one side only (these receivers

sound access from one side only (these receivers

actually respond to the pressure difference across

actually respond to the pressure difference across

the membrane.

the membrane.



Medium motion is directional (albeit with 180 deg.

Medium motion is directional (albeit with 180 deg.

ambiguity. Simplest receivers are the diverse types

ambiguity. Simplest receivers are the diverse types

of sensory hairs with some kind of intrinsic

of sensory hairs with some kind of intrinsic

directionality. Note that combining a measure of

directionality. Note that combining a measure of

medium motion with pressure can resolve the 180

medium motion with pressure can resolve the 180

deg ambiguity, in far-field sound, at least.

deg ambiguity, in far-field sound, at least.

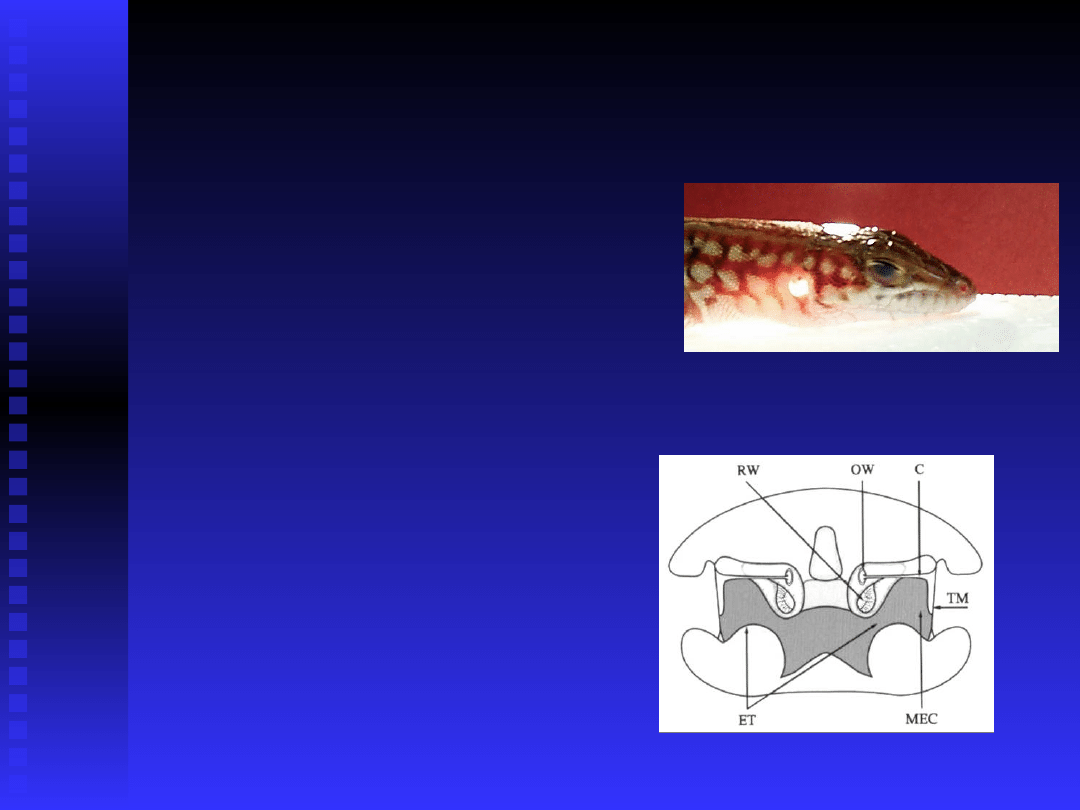
Measuring the sound field
2



Third type of receivers are

Third type of receivers are

the pressure-difference (or

the pressure-difference (or

–gradient) receivers. Here

–gradient) receivers. Here

sound can enter both sides

sound can enter both sides

of a membrane producing

of a membrane producing

cancellation when sound

cancellation when sound

pressures at the two sides

pressures at the two sides

have identical amplitudes

have identical amplitudes

and phases. These

and phases. These

receivers are only

receivers are only

directional in a narrow

directional in a narrow

frequency range.

frequency range.

Measuring the sound field
3



With instruments:

With instruments:



Sound pressure is measured with

Sound pressure is measured with

microphones that respond to the

microphones that respond to the

pressure gradient across a

pressure gradient across a

membrane. Pressure gradient

membrane. Pressure gradient

microphones can be constructed to

microphones can be constructed to

allow sound to enter both sides om

allow sound to enter both sides om

membrane.

membrane.

Measuring the sound field
4



Sound intensity measurements use two

Sound intensity measurements use two

(phase-matched) microphones or

(phase-matched) microphones or

hydrophones to estimate the pressure

hydrophones to estimate the pressure

gradient (and hence the particle velocity)

gradient (and hence the particle velocity)

and calculate the time average of p*v. This

and calculate the time average of p*v. This

measurement gives the active, radiating

measurement gives the active, radiating

sound emitted from the source.

sound emitted from the source.



Direct measurements of particle velocity is

Direct measurements of particle velocity is

difficult, since the methods at hand (hot

difficult, since the methods at hand (hot

wire anemometry, laser anemometry, PIV)

wire anemometry, laser anemometry, PIV)

only work at high sound levels.

only work at high sound levels.

Suggested reading



Beranek LL (1954) Acoustics. McGraw Hill

Beranek LL (1954) Acoustics. McGraw Hill



Fahy F (1995) Sound Intensity, 2.ed. Chapman and

Fahy F (1995) Sound Intensity, 2.ed. Chapman and

Hall

Hall



Gade S (1982) Sound Intensity, part 1: Theory.

Gade S (1982) Sound Intensity, part 1: Theory.

Brüel & Kjær Technical Review 3

Brüel & Kjær Technical Review 3



Kalmijn A (1988) Hydrodynamic and acoustic field

Kalmijn A (1988) Hydrodynamic and acoustic field

detection. In Atema J et al. (eds.) Sensory biology

detection. In Atema J et al. (eds.) Sensory biology

of aquatic animals. Springer, p. 83-130

of aquatic animals. Springer, p. 83-130



Larsen ON (1995) Acoustic equipment and sound

Larsen ON (1995) Acoustic equipment and sound

field calibration. In Klump GM et al (eds.) Methods

field calibration. In Klump GM et al (eds.) Methods

in comparative psychoacoustics. Birkhäuser

in comparative psychoacoustics. Birkhäuser

Verlag, p. 31-45

Verlag, p. 31-45