1

COMPLEX FUNCTIONS AND POLYNOMIALS

Lecture 3

2

COMPLEX FUNCTIONS

EXTRA

3

Definition of THE EXPONENTIAL FUNCTION

f(z)= e

z

For

z = x + iy  0

e

z

= e

x

(cos y + i∙sin y).

1.The function

e

z

is periodic:

e

z+2ki

= e

z

for k 

Integer

2. For a real number

z = x

, we obtain

e

z

= e

x

( cos 0 + i sin 0) = e

x

- the classical exponential function.

3. The modulus and the argument:

|e

z

| = e

x

, arg(e

z

) = y.

EXTRA

4

2

1

2

1

z

z

z

z

e

e

e

1







.

2

1

2

1

z

z

z

z

e

e

e

2





.

1

e

3

0



.

,

.

PROPERTIES OF THE EXPONENTIAL FUNCTION

Examples:
1)

-1·i = e

i

· e

i/2

= e

i3/2

= -i

2) Find the real and imaginary parts of

z = e

-i

:

e

-i

=cos(-1) + i sin(-1) = 0.540 + i

0.841
(

in radians)

5

The Logarithm

The logarithm of a complex variable is of form:

f(z) = log z

and

it is the inverse of the exponential function, i.e.
we want to have

log( e

z

) = z

and

e

log z

= z

Definition

z = r e

i 

, r > 0 (z0)

log z = { lnr + i ( + 2k): k  Z }

The logarithm

log z

is not in the strict sense a function

because the argument of a complex number is not uniquely defined,

The logarithm has infinitely many branches: every fixed k has one branch
which is a function in the strict meaning of the word.

EXTRA

6

The main branch is a function:

Log z = lnr + i∙Arg z (r > 0), π 

Arg z ≤ π

Log z = lnr + i∙ for z = r e

i

PROPERTIES

Log (z

1

∙z

2

) = Logz

1

+

Logz

2

,

Log1 = 0,

e

Logz

= z.

Examples:

2

i

= ?

i

i

= ?

EXTRA

7

2 = e

Log2

(the main branch of the logarithm

Log z

)

2

i

= e

i Log 2 =

cos(ln2) + i sin (ln2) ≈ 0.769239.. + i 0.638961..

The multivalued logarithm:

log i = ln 1 + i arg i = 0 + i (2k + ½ )π, k = 0, ±1, ±2, ±3, ...

The main branch:

Log i = ln 1 + i Arg i = 0 + i π/2,

i

i

= e

ii

Log i

= e

i

i

π/2

= e

– π/2

≈

0.20788....

FIND

2

i

FIND

i

i

i

i

= e

ii log i

= e

i

i (2k + ½) π

= e

-(2k + ½) π

,

k = 0, ±1, ±2, ±3, ...

ALL

i

i

ARE REAL !!!!!

EXTRA

8

For complex quantities THE RULE:

(x y)

a

= x

a

y

a

doesn't always

apply for complex numbers.

Example;

(2 z + z

2

)

a

≠ z

a

(2 + z)

a

e.g.

z = -3, a = i

(2 (-3) + (-3)

2

)

i

≠ (-3)

i

(2 + (-3))

i

LHS

:

(-6 + 9)

i

= 3

i

= e

iln3

 0.454832.. + i 1.09861...

RHS

:

(-3)

i

(2-3)

i

= (-3)

i

(-1)

i

= (3e

iπ

)

i

(e

iπ

)

i

= e

i Log(-3)

e

-π

= e

i

(ln3+iπ

) e

-π

 e

iln3

·

0.00186744..  0.000849374.. + i

0.0016631...

LHS  RHS

CAUTION !!!

9

POLYNOMIALS

10

Definitions

(i) A

polynomial in z

with real coefficients is the function W

n

(z)

of

the form:

W

n

(z) = a

n

z

n

+... + a

1

z + a

0

,

defined for zC, when nN.

If a

n

≠ 0 and a

m

= 0 for m>n then W

n

is a polynomial

of degree

n,

and the number n is called the degree of the polynomial W

n

.

e.g. W

4

(z) = 5 z

4

+ 1.05 z

3

+ 2 z

2

– 7 z + 0.5

(ii) The complex number x is called

the root

(zero) of the

polynomial W

n

(x)

iff W

n

(x) = 0, which means that

a

n

x

n

+... + a

1

x + a

0

= 0.

11

Example

a = -1 is the root of the polynomial W(x) = 3x

5

- 9x

2

- 2x +

10,

because W(-1) = 3(-1)

5

 9(-1)

2

 2(-1) + 10 = 0.

Please notice that polynomials

do not always have real

roots.
e.g.

W(x) = x

2

+ 1 and W(x) = x

4

+ x

2

+ 2

The theorem of Niels Abel and Evarist Galois tels us that there can
be no general algorithm (finite and involving only arithmetical
operations and radicals) to determine the roots of a polynomial of
degree n  5.

12

POLYNOMIALS WITH INTEGER

COEFFICIENTS

13

RATIONAL ROOT TEST

Consider a polynomial in x  R, with only integer coefficients:

f(x) = a

n

x

n

+ ... + a

o

The Rational Root Test tells you that if the polynomial has a rational root x

R

then it must be a fraction x

R

=

p

/

q

,

where

q

is a factor of the leading coefficient a

n

and

p

is a factor of the constant a

o

.

p(x) = 2x

4

− 11x

3

− 6x

2

+ 64x + 32

The factors of the leading coefficient '2' are 2 and 1 (the q 's).
The factors of the constant term '32' are 1, 2, 4, 8, 16, and 32 (the p 's).

Therefore the possible rational roots are ±1, 2, 4, 8, 16, or 32 divided by 2 or 1:
±1/2, 1/1, 2/2, 2/1, 4/2, 4/1, 8/2, 8/1, 16/2, 16/1, 32/2, 32/1
reduced: ± ½, 1, 2, 4, 8, 16, 32

Example:

14

Caution:

1. Don’t make the Rational Root Test out to be more than it is.

2. It doesn’t say that those rational numbers are roots, just that no other
rational
numbers can be roots.

3. And it doesn’t tell you anything about whether some irrational or even
complex roots
exist.

4. The Rational Root Test is only a starting point.

15

POLYNOMIALS WITH COMPLEX COEFFICIENTS

16

Theorem (dividing polynomials)

For every pair of polynomials Q(z) and P(z,) where the degree of Q(z) > 0,
there exist polynomials S(z) and R(z) such that

P(z) = S(z) ∙ Q(z) + R(z),

for z  C and the degree of R is smaller that the degree of Q.

The polynomials S(z) and R(z) are uniquely determined by Q(z) and P(z).

Example: (2x

5

+ 2x

4

- x

2

- 1) : (x

2

– x + 2) = (2x

3

+ 4x

2

- 9)(x

2

– x + 2) - 9x+17

17

Theorem
Let the coeffincients of the polynomial W(x)
W(z) = a

n

 z

n

+ a

n-1

 z

n-1

+ ..... + a

0

,

be real and the complex number z

R

= x + i y be a root of

it,

W(z

R

) = 0,

then the conjugate of z

R

= x - i y, is also a root of this

polynomial

W( z

R

) = 0

The Bezout Theorem

The value a is a root of the polynomial P(z) iff:
P(z) = (z – a)S(z).

Thus the fact that a is a root of the polynomial P(z)
is equivalent to the fact that the binomial (z – a) divides P(z).

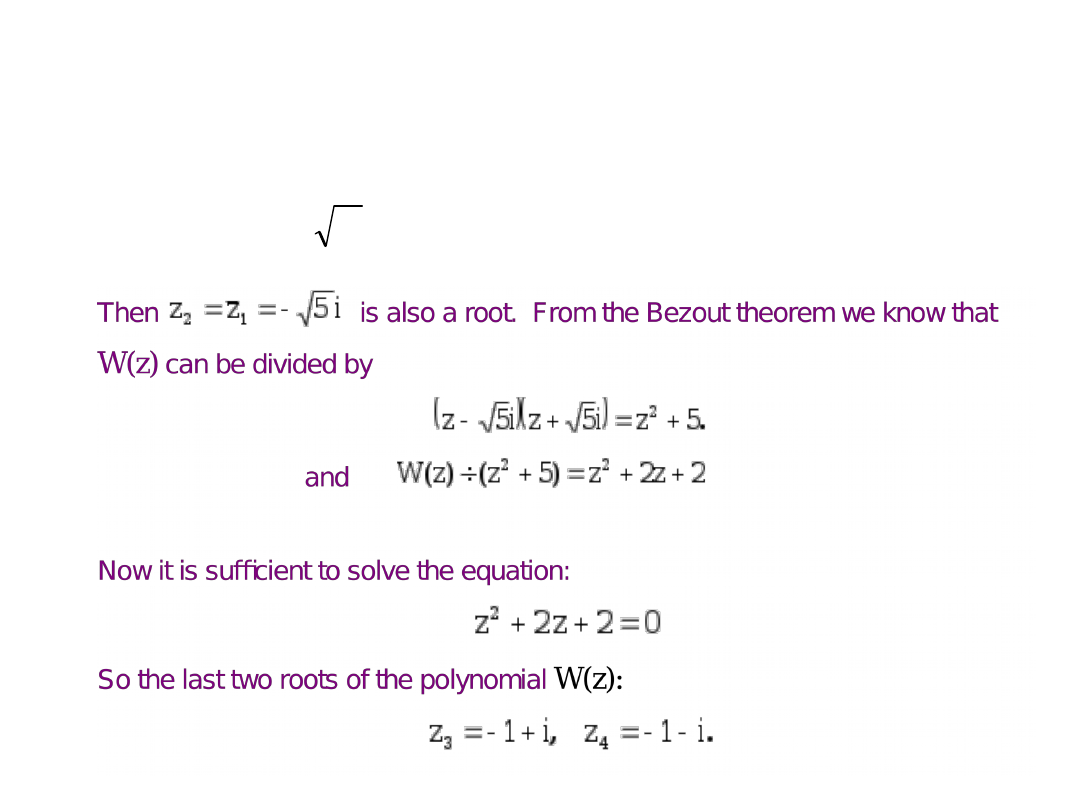
18

Example
Let us assume that you know one of the roots of the polynomial
l

,

)

(

10

z

10

z

7

z

2

z

z

W

2

3

4











.

i

5

z

1



which is

.

1

z

19

Fundamental Theorem of Algebra

Every polynomial W

n

(z) of degree n has exactly n complex roots

(taking into account the multiplicity of the roots)

Conclusion I
If the degree of the polynomial is odd, then it always has a real
root.

This is true because the number of

complex roots is even, (they

always appear in pairs ),

only real roots

can have an odd number.

)

,

( z

z

Example

20

Conclusion II

Let z

1

,......,z

m

be all the roots of W

n

(z) of order

respectively
k

1

, k

2

,......, k

m

(k

1

+k

2

+......+k

m

= n).

Then:

m

2

1

k

m

k

2

k

1

n

z

z

z

z

z

z

z

W

)

(

)

(

)

(

)

(











monomials