Optimization of Intake System and Filter of an 

Automobile using CFD analysis  

Ravinder Yerram

 and 

Nagendra Prasad

 

Quality Engineering and Software Technologies (QuEST), Bangalore 

 

  

 

   Prakash Rao Malathkar 

and 

Vasudeo Halbe

 

Mahindra & Mahindra Ltd., Nashik 

 

      

 

 

 

 

 

 

Shashidhara Murthy K

 

MNR Filters India Pvt. Ltd., Bangalore 

 
 
 
 

 
 

1. ABSTRACT 

Air intake system and filter play major role in getting 
good quality air into automobile engine. It improves the 
combustion efficiency and also reduces air pollution. 
This paper focuses on optimizing the geometry of an 
intake system in automobile industry to reduce the 
pressure drop and enhance the filter utilization area. 
3D viscous CFD analysis was carried out for an 
existing model to understand the flow behavior through 
the intake system, air filter geometry and filter media. 
Results obtained from CFD analysis of the existing 
model showed good correlation with experimental 
data. Based on existing model CFD results, 
geometrical changes like baffle placement in inlet 
plenum of the filter, inclusion of bell mouth in outlet 
plenum and dirty pipe , optimization of mesh size, 
removal of contraction in clean pipe of intake system 
etc are carried out, to improve the flow characteristics. 
The CFD analysis of the optimized model was again 
carried out and the results showed good improvement 
in flow behavior, better filter utilization with 
considerable reduction in pressure drop and significant 
reduction in re-circulation zones of the air filter 
geometry. By using 3D CFD analysis, optimal design 
of the intake system for an automobile engine is 
achieved with considerable reduction in development 
time and cost. 

2. INTRODUCTION 

The work of an air filter is to filter the dirt particles from 
the intake air and supply cleaner air to the automobile 
engine. Air enters the filter through dirty pipe and inlet 
side plenum, which guides the flow uniformly through 
the filter media. Optimum utilization of filter can 
significantly reduce the cost of filter replacements 
frequently and keep the filter in use for longer time. To 
optimize intake system and filter, thorough 

understanding of flows and pressure drop through the 
system is essential. Computational Fluid Dynamics 
(CFD) is considered to be the most cost effective 
solution for flow analysis of intake system along with 
filter media. This paper focuses on the optimization of 
the intake system and filter by CFD analysis results.     

3. GEOMETRY MODEL 

Figure (3.1) shows solid model of intake system and 
filter. In order to save the CFD computational time and 
cost, trivial geometric details that are unimportant from 
fluid flow point of view, such as fillets, blends, 
stiffeners and steps have been ignored. Ignoring all the 
above-mentioned, so called a cleaned geometry was 
obtained from solid model.  

 

 

 

 

 
 
 

 
Figure (3.1): Intake system solid model       

 

 Figure (3.2) shows the fluid volume for the existing 
intake system and filter and figure (3.3) shows the fluid 
volume for the modified intake system with baffles. 
where filter media is approximated to rectangular 
volume and considered as porous media. For mesh 
generation, all surfaces and curves were extracted 
from the cleaned model.  
 

 
 
 
 
 
 
 
 
 
 

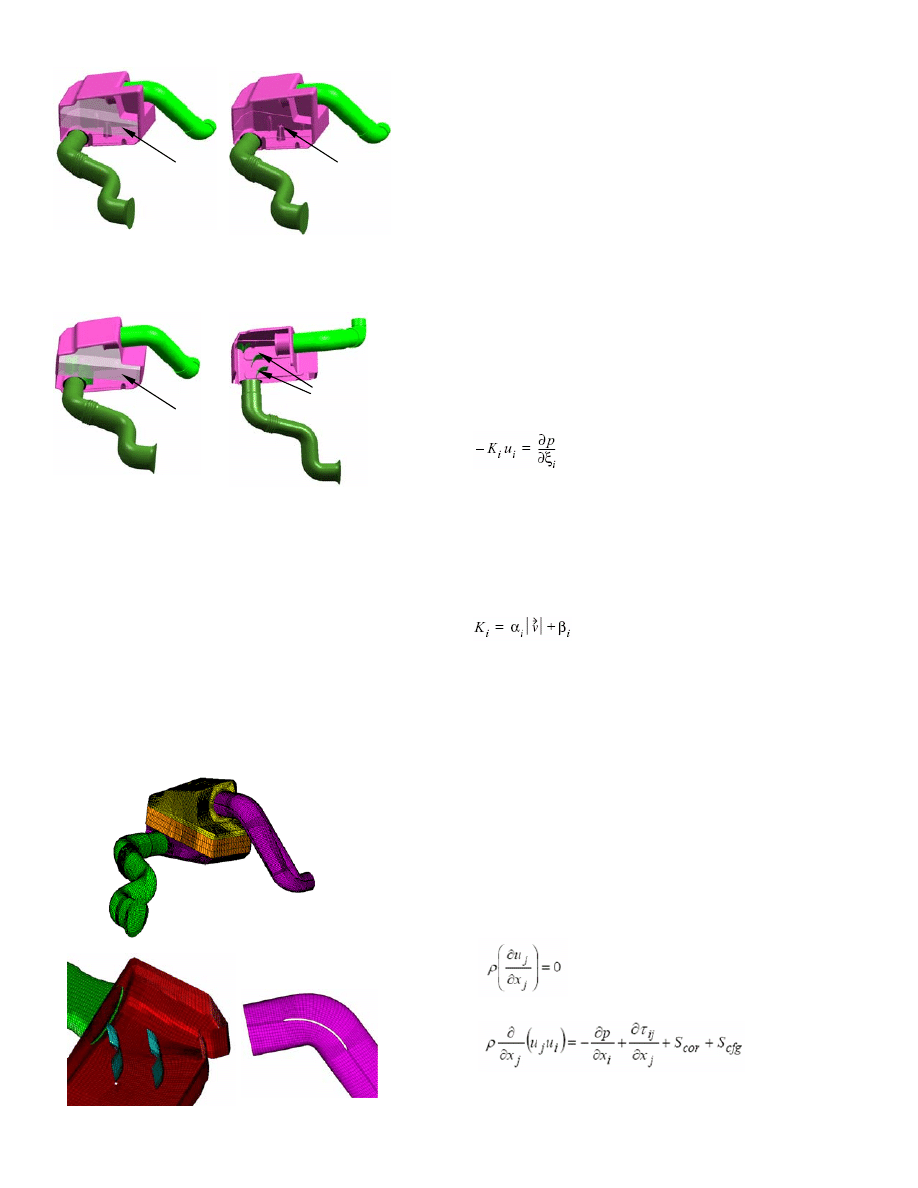
Figure (3.2): Fluid volume of existing intake system   
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 

Figure (3.3): Fluid volume of modified intake system 

      

4. CFD MESHING

 

 
To capture the three-dimensional flow inside the 
domain with reasonable accuracy, one needs good 
quality mesh. Multi-block structured hexagonal mesh 
was considered to be the best for this case and was 
created using commercial mesh generator (ICEM-
CFD). The model was approximately 0.55 million 
hexagonal fluid elements. Boundary layer was 
resolved for y+ of 40 to 200 to capture physics inside 
the complicated regions. Figure (4.1) shows 
hexahedral mesh of intake system fluid domain. Figure 
(4.2) shows hexahedral mesh near baffles and clean 
air pipe elbow.  
 
 
 
 
 
 
 
 
 
 

Figure (4.1): Intake system hexahedral mesh      

 

 
 
 
 
 
 
 
 
 

Figure (4.2): Hexahedral mesh near baffles and elbow 

 

5. CFD MODEL DESCRIPTION 
 

Air was used as fluid media, which was assumed to be 
steady and incompressible. High Reynolds number k-

ε 

turbulence model [2] was used in the CFD model. This 
turbulence model is widely used in industrial 
applications. The equations of mass and momentum 
were solved using SIMPLE algorithm [1] to get velocity 
and pressure in the fluid domain. The assumption of an 
isotropic turbulence field used in this turbulence model 
was valid for the current application. The near-wall cell 
thickness was calculated to satisfy the logarithmic law of 
the wall boundary. Other fluid properties were taken as 
constants. Filter media of intake system and air sensor 
were modeled as porous media using coefficients.  

Support 

Filter 

 
For porous media, it is assumed that, within the 
volume containing the distributed resistance [3], there 
exists a local balance everywhere between pressure 
and resistance forces such that  

Baffles 

Filter 

 
 

 

 

(1) 

 
Where 

ξ

I  

(i  = 1, 2, 3) represents the (mutually 

orthogonal) orthotropic directions.  
K

i

 is the permeability 

u

i

 is the superficial velocity in direction 

ξ

i

The permeability K

i

 is assumed to be a quasilinear 

function of the superficial velocity magnitude of the 
form 
 
                           

(2) 

 
Where 

α

i

 and 

β

i

 are user-defined coefficients [4].  

Superficial velocity at any cross section through the 
porous medium is defined as the volume flow rate 
divided by the total cross sectional area (i.e. area 
occupied by both fluid and solid). In this analysis, 

α

i

 

and 

β

i 

are assumed to be same.  

 

6. GOVERNING EQUATIONS 
 

Commercial CFD solver Star-CD was used for this 
study. It is a finite volume approach based solver 
which is widely used in the industries. Governing 
equations solved by the software for this study in 
tensor Cartesian form are following:  
 
Continuity:  
 
 
 

 

 

(3)

 

 

 

 

 

Momentum: 
 
 

 

 

 

 

 

(4)

 

 
 
Where  ρ is density, u

j 

is jth Cartesian velocity, p is 

static pressure, 

τ

ij 

is viscous stress tensor.   

 

7. BOUNDARY CONDITIONS  

 
Various boundary conditions for the different 
components applied to this study were as follows:  
For inlet, the mass flow rate was imposed using the 
fixed mass inlet boundary condition. The value of 
density (1 kg/m

3

), total pressure (1 atm) and 

turbulence intensity (5%) were specified at the inlet 
boundary. For outlet, outflow boundary condition was 
imposed with flow rate weighting of 1. No slip 
boundary condition was applied on all wall surfaces. 
For main filter media, porous media boundary was 
imposed with 

α

i

 =  

β

I 

= 3000. For air sensor, porous 

media boundary was imposed with 

α

i

 =  

β

I 

= 290. 

Whole domain was considered at 1 atm and at 298 K 
as initial condition.   
 

8. RESULTS AND DISCUSSION 

To

 

have effective cleaning of air from filter, it was 

suggested to have uniform velocity of air pass through 
filter. 

 

 
 
 
 
 
 
 
 
 
 
 
 
 

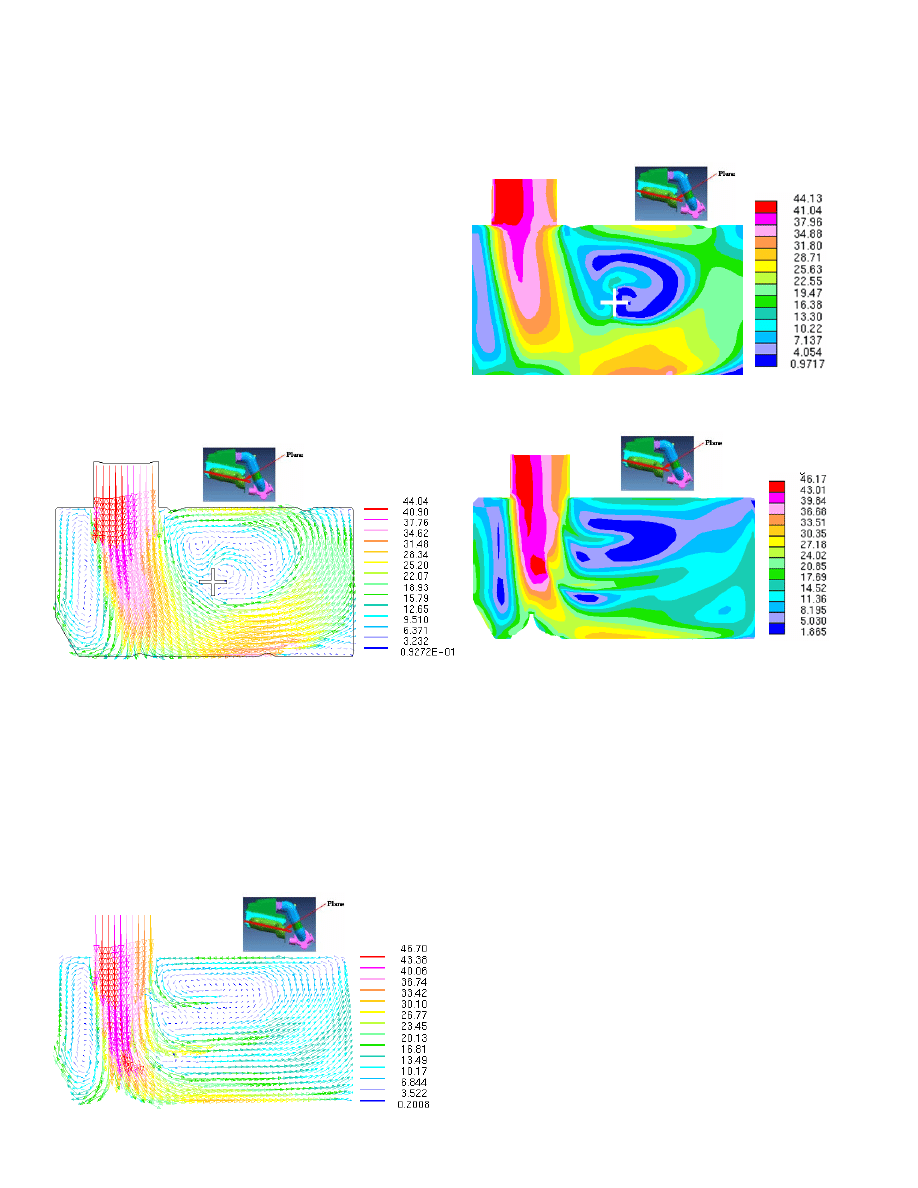
Figure (8.1-a): Velocity vector (m/s) plot for the existing model 

 

Figure (8.1-a) shows two recirculation zones right 
below the filter needed to be considered for 
optimization as the recirculation in flow field causes 
energy dissipation. In order to avoid the recirculation, 
introducing the baffle was suggested which would 
guide the flow to avoid recirculation.  
After the baffle was introduced in the existing model, 
CFD analysis was again carried out to decide the 
location and effect of baffle. Velocity vector plot of 
modified model in figure (8.1-b) below gives a clear 
picture of less recirculating flow field. 
 
 
 
 
 
 
 
 
 
 
 
 

  

   

Figure (8.1-b): Velocity vector (m/s) plot for the modified model 

 
 

It is worth to mention that introducing baffle in the inlet 
plenum below the filter has enhanced the efficiency by 
guiding the flow and reducing the pressure drop 
significantly that was present earlier. 
 
 
 
 
 
 
 
 
 
 
 
 
 
 

Figure (8.2-a): Velocity magnitude (m/s) contour plot for the existing 
model 

 
 
 
 
 
 
 
 
 
 
 
 
 
 
 
 

Figure (8.2-b): Velocity magnitude (m/s) contour plot for the existing 
model 

 

Figure (8.2-a) and figure (8.2-b) show the velocity 
magnitude contour plot in the critical region in the flow 
domain.  

In the figure (8.2-b) it can be seen the effect of baffle 
as the flow is relaxed and better flow distribution.  
 
Near outlet plenum exit, flow was separating and 
recirculating at both the ends. This phenomenon can 
be seen figure (8.3-a). To avoid separation and 
recirculation in this region, a bell-mouth was 
introduced. This can be clearly seen in figure (8.3-b) of 
velocity vectors.  
 
Figure (8.4) shows a separation zone at one side after 
the first bend of clean pipe. And more concentrated 
velocity magnitude contours were seen at the other 
end which is typical phenomenon that can be seen in 
bends. To overcome such phenomenon of separation, 
a baffle was introduced that guides the flow and make 
the flow uniform. This will possibly improve engine 
performance.  
 

Table (8.1) presents percentage improvement in total 
pressure drop (reduction) in the intake system with 
various design modifications. By changing mesh type 
(simplified rectangular grid) near entry to intake system 
and bell-mouth in dirty pipe inlet, pressure drop 
improved by 33%.  
 
By placement of baffles in inlet plenum before filter 
media the performance has improved by 28% that is 
significant in intake system. Bell-mouth and baffle 
inside the clean pipe improved the flow and pressure 
drop by 6.5%.     
    
 
 
 
 
 
 
 
 
 

Table (8.1): Percentage Improvements in total pressure drop 
(reduction) in various regions 

 

  

 

 

 

 

 

 

 

Figure (8.3-a): Velocity vectors plot near outlet plenum 
before modification 

 

 

 

 

 

 

 

 

Figure (8.3-b): Velocity vectors plot near outlet plenum 
after introducing bell-mouth 
 

 
 

 
 
 
 
 
 
 
 
 
 
 
 
          

a. Without baffle 

 

b. With baffle 

Figure (8.4): Velocity magnitude contours after clean pipe baffle 

 

9. CONCLUSION 

Percentage improvement (reduction) in Total 
Pressure drop with baffles and other modifications

CFD analysis was done using commercial CFD solver 
Star-CD to understand the flow phenomenon in an 
intake system. CFD results of the existing intake 
system had shown recirculation and separation zones 
before and after the filter media. Following design 
modifications were considered to improve the flow and 
pressure drop through the intake system 

• Changing of mesh type (simplified rectangular 
grid) in dirty pipe.  
•  Introduction of bell-mouth in dirty and clean pipe   
• Introduction of baffles inside inlet plenum just 
below filter media. 
•  Introduction of baffle in clean pipe bend 
 

All the above changes incorporated in the design 
improved overall pressure drop by 22%.  

 
10. ACKNOWLEDGMENTS 

The authors would like to sincerely thank Mr. Mihir 
Desai and Mr. Veerabathra Swamy for their support in 
CFD analysis in this project.  

11. REFERENCES 

(1) Patankar, S.V. 1980, “Numerical Heat Transfer and 
Fluid Flow”, Hemisphere, Washington, D.C. 
(2) Launder, B.E., and Spalding, D.B. 1974, “The 
Numerical Computation of Turbulent Flows”, Comp. 
Meth. in Appl. Mech. and Eng., 3, pp. 269-289. 
(3) STAR-CD Methodology 
(4) MNR Filters India Pvt. Ltd., experimental resources 
 

12. CONTACT 

Ravinder Yerram 
Senior Technical Leader, CFD Team 
Quality Engineering & Software Technologies (QuEST) 
#55 QuEST Towers, Whitefield Main Road, 
Mahadevapura, Bangalore-560 048 
Tel: +91-80-41190909 Extn. 313 
Fax: (91) 80-41190901 

 

Dirty pipe with mesh

33

take System, Filter 

d Air Sensor

28

ean Pipe 

6.5

hrough out the domain

22

In
an
Cl

T