Vector Controlled Doubly Fed Induction Generator for

Wind Applications

Ani Gole, Dept. of Electrical and Computer Eng.,
University of Manitoba.

This document discusses the theory of operation behind the doubly fed generator case
developed by Ani Gole (Univ. of Manitoba, Canada) and Om Nayak (Nayak Corporation,
Princeton, NJ). The controller concept is based on the paper by Pena et al [1].

Description of Rotor Current Generation Circuit (Generator PWM Connverter and
Controls)

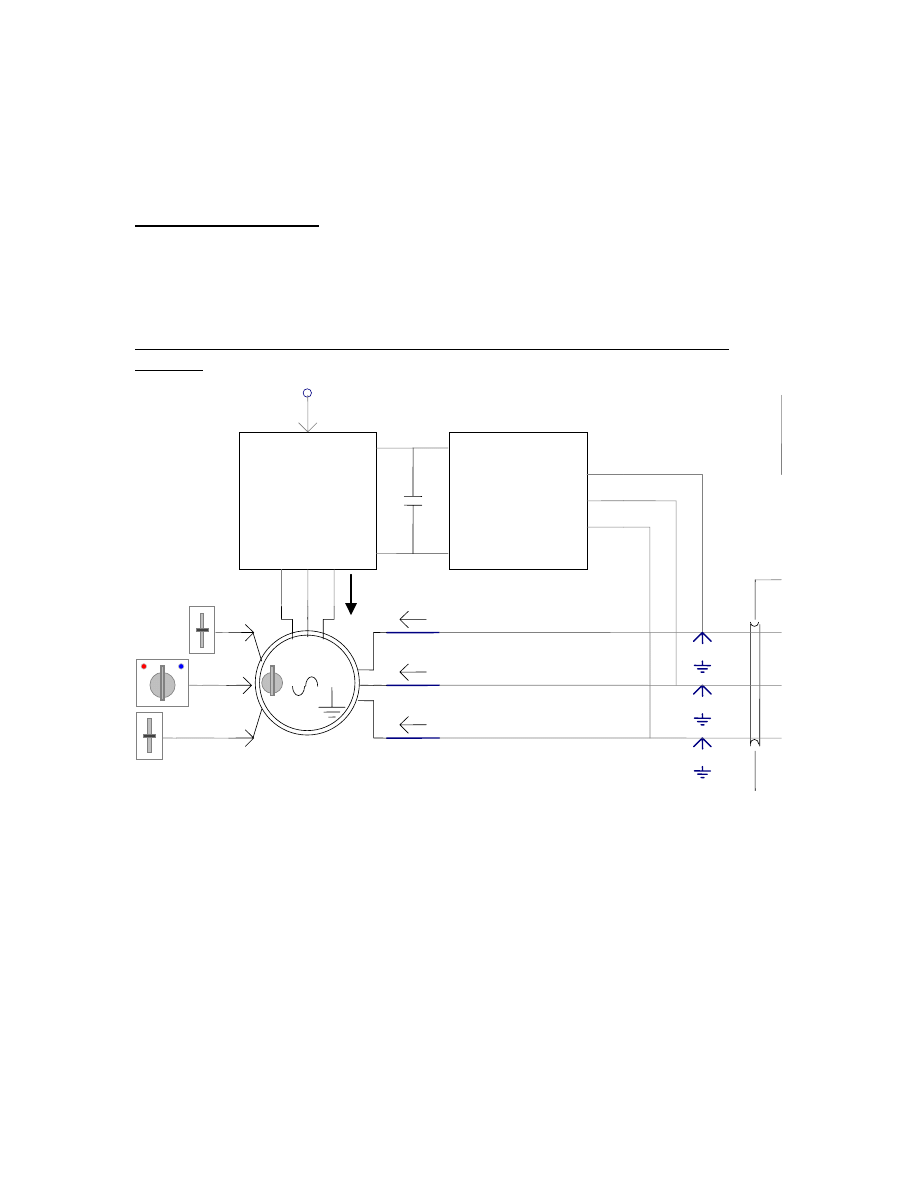
CTRL

a

GRID

GENERATOR

b

PWM Converter

PWM Converter

c

& Controls

& Controls

b

a

c

ira,irb,irc

b

a

c

W

A

A

Isa

Va

I M

S

B

B

Isb

Vb

C

TL

C

Isc

Vc

13.8 kV, 500 HP

INDUCTION GENERATOR

Fig 1: Doubly Fed Induction Generator

The Doubly fed induction generator/motor allows power output/input into the stattor
winding as well as the rotor winding of an induction machine with a wound rotor
winding. Using such a generator it is possible to get a good power factor even when the
machine speed is quite different from synchronous speed. Such machines can therefore
operate without the need for excessive shunt compensation.

1

The rotor currents (ira,irb,irc)of the machine can be resolved into the well known direct
and quadrature components i

d

and i

q

. The component i

d

produces a flux in the airgap

which is aligned with the rotating flux vector linking the stator; whereas the component i

q

produces flux at right angles to this vector. The torque in the machine is the vector cross
product of these two vectors, and hence only the component i

q

is contributes to the

machine torque and hence to the power. The component id then controls the reactive
power entering the machine. If id and iq can be controlled precisely, then so can the stator
side real and reactive powers.

The procedure for ensuring that the correct values of id and iq flow in the rotor is
achieved by generating the corresponding phase currents references ira_ref, irb_ref and
irc_ref, and then using a suitable voltage sourced converter (VSC) based current source to
force these currents into the rotor. The latter action is straightforward and can be
achieved using current-reference pulse width modulation (CRPWM) or other technique.
The crucial step is to obtain the instantaneous position of the rotating flux vector in space
in order to obtain the rotating reference frame. This can be achieved by realizing that on
account of Lenz’s law of electromagnetism, the stator voltage (after subtracting rotor
resistive drop) is simply the derivative of the stator flux linkage

λ

a

as in eqn. (1) which is

written for phase a.

a

a

a a

d

v

i R

dt

λ

−

=

…….(1)

The control structure shown in Fig. 2 can thus be used to determine the location (

φ

s

) of

the rotating flux vector.

Vbeta

Vsmag

Vc

Va

Isa

C

-

D +

Isa

Vb

C

-

D +

phisy

phisx

X

Y Y

r to p

X

mag

phi

phsmag

G

sT

1 + sT

*

0.467

*

0.467

Valfa

G

sT

1 + sT

1

sT

1

sT

phis

A

B

C

3 to 2

Transform

alfa

beta

*

0.467

Isa

C

-

D +

C

+

D -

Angle

Resolver

in

out

phis

rotor_angle

Very important signal -
present location ==>
of rotating stator flux

determining the relative difference between
stator flux and rotor position for resolving the
rotor currents

after removal of resistive drop. The washout filter removes any
dc component from the integrated flux without significantly
ffecting the phase

slpang

λ

α

λ

β

Ra

Fig 2: Determination of rotating mag. Flux vector location

2

In Fig. 2, the three phase stator voltages (after removal of resistive voltage drop) are
converted into the Clarke (

α

and

β

)components v

α

and v

β

, which are orthogonal in the

balanced steady state. This transformation is given by:

1

1/ 2

1/ 2

2 / 3

0

3 / 2

3 / 2

a

b

c

v

v

v

v

v

α

β

 

−

−









 

=









 









−







 

 

……(2)

Integrating v

α

and v

β

, we obtain

λ

α

and

λ

β

, the Clarke components of stator flux.

Converting to polar form

1

2

2

| |

,

tan (

/

)

s

α

β

β

α

λ

λ

λ

φ

λ λ

−

=

+

=

……(3)

The angle

φ

s

gives the instantaneous location of the stator’s rotating magnetic field. In

practical control circuits, as in Fig. 2, some filtering is required in order to rid the
quantities

λ

α

and

λ

β

of any residual dc component introduced in the integration process.

Now the rotor itself is rotating and is instantaneously located at angle

φ

r

(labeled “rotor

angle” in the figure). Thus, with a reference frame attached to the rotor, the stator’s
magnetic field field vector is at location

φ

s-

φ

r

, which we refer to the “slip angle”

φ

slip.

The instantaneous values for the desired rotor currents can then be readily calculated
using the inverse dq transformation, with respect to the slip angle, as shown in Fig. 4. The
equations for all transformations are shown in the appendix.

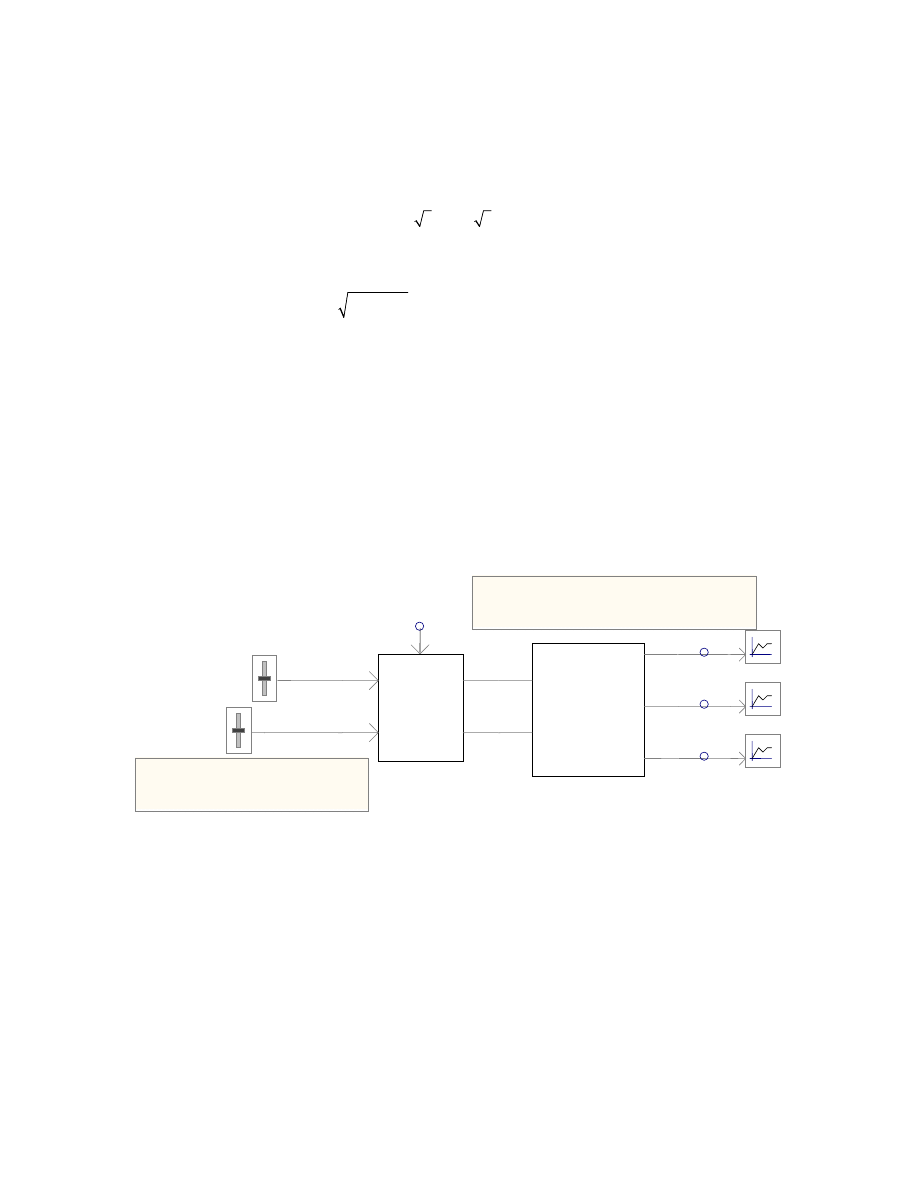
Generation of current references

slpang

A

to Stator

Rotor

alfa

Q

D

Ira_ref

Iraa

alfa

2 to 3

Transform

beta

B

Irb_ref

Irbb

beta

C

D and Q reference currents

Irc_ref

Ircc

Fig. 4: Final step in generation of rotor phase reference
currents

Once the reference currents are determined, they can be generated using a voltage
sourced converter operated with a technique such as current reference pulse width
modulation (CRPWM) as shown in Fig. 5. The Appendix gives a short introduction to
CRPWM.

3

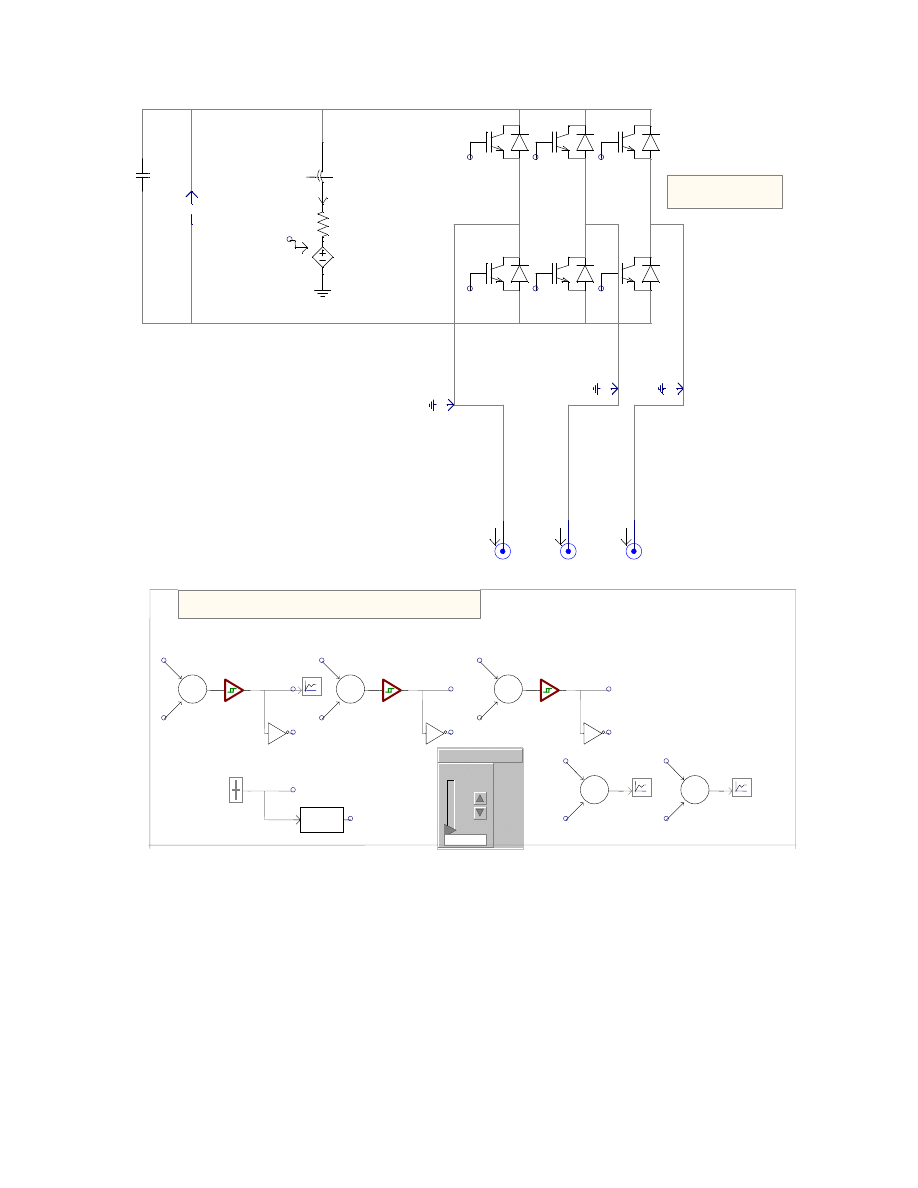
Ecap

10

00

0.0

Ecapref

B

R

K

Irc

Ir

b

T1

T1

D2

T1

T2

D1

T2

D2

T1

D1

T2

T4

T5

T6

T3

Er

c

Ira

Er

b

Er

a

T2

D1

D2

1.

0

V

CR-PWM based
Rotor-side converter

GA

GB

GC

Current-Reference PWM Controls. Hysteresis band can be adjusted

Ira

Irb

Irc

C

C

C

-

-

-

+

+

+

T1

T1

T3

T5

Ira_ref

E

E

Irb_ref

E

Irc_ref

T4

T6

CPanel

hysband

10

T2

ira_ref

ira_ref

C

C

+

+

hy

+

-

*

-1

E

E

nhy

hy

hy

0

0.1

Fig. 5: CRPWM Converter and Controller for rotor
currents

4

Grid PWM Converter and Controls:
As can be seen from Fig. 5, the rotor side VSC converter requires a dc power supply. The
dc voltage is usually generated using another voltage sourced converter connected to the
ac grid at the generator stator terminals. A dc capacitor is used in order to remove ripple
and keep the dc bus voltage relatively smooth. This grid PWM Converter is operated so
as to keep the dc voltage on the capacitor at a constant value. In effect, this means that the
Grid side converter is supplying the real power demands of the rotor side converter.

It is possible to operate this converter using a current reference approach used for the
rotor side converter. However, as mentioned earlier, CRPWM has the drawback that the
switching frequency and hence the losses are not predictable. Therefore, a feedback
controller is used in which the error between the desired and ordered currents is passed
through a proportional-integral controller which controls the output voltage of a
conventional Sinusoidal PWM Converter. The advantage of the SPWM controller is that
the number of switchings in a cycle is fixed, and so the losses can be easily estimated a-
priori.

It is possible to control the d axis current by controlling the d-component of the SPWM
output waveform and the q axis current via the q component. However, this leads to a
poor control system response, because attempting to change id also causes iq to change
transiently. Hence, modifications have to be made to the basic P-I controller structure so
that a decoupled response is possible, and a request to change id changes id and not iq;
and vice-versa.

If a voltage sourced converter with constant dc bus voltage is connected to an ac grid
through a (transformer) inductance L and resistance R, it can be shown that that:

t

d

d id

iq

R
L

----

–

ω

ω

–

R
L

----

–

id
iq

1
L

--- vd ed

–
eq

–

+

R
L

----

–

0

0

R
L

----

–

x1
x2

=

=

x1

vd ed

–

L

------------------

ω

id

+

=

x2

eq

L

------

–

ω

iq

–

=

…

ed

L

– x1 vd

ω

Lid

+

+

=

eq

– Lx2

ω

Liq

–

=

….(4)

Here v=vd is the voltage of the ac grid, and because this is chosen as the reference, vq is
by definition, zero. Ed and eq are the d and q components of the generated VSC voltage.
Eqn. 4 clearly shows that attempting to change id using ed will also cause a transient
change in iq. If instead, we use the quantities Lx1 and Lx2 to control the currents, the
resulting equations are decoupled. Using feedback PI control, we let the error in the id
loop affect L x1 and in the iq loop, L x2 as shown in Fig. 6.

5

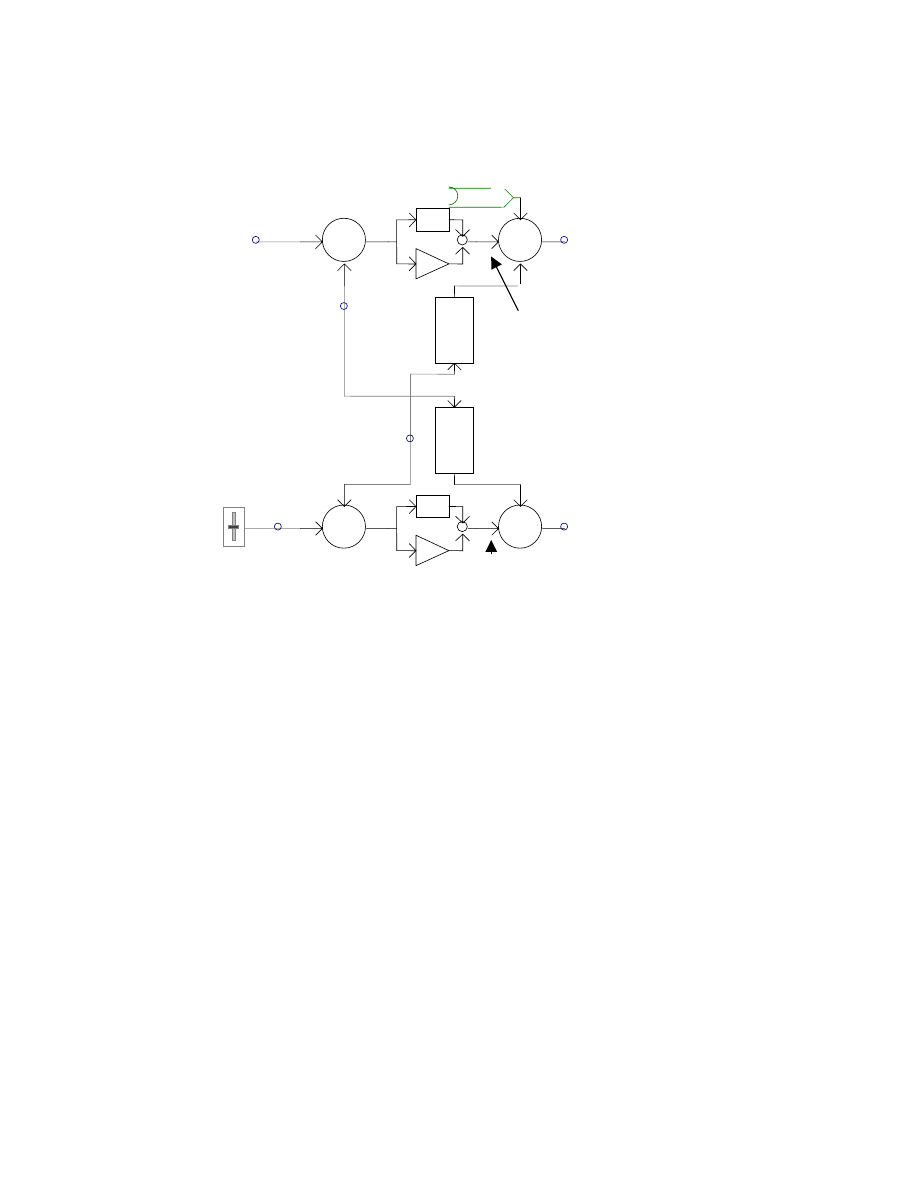
Vd=3.22 kV

3.266 B

+

P

idref

+

-

D

D

-

+

Vdref1

I

F

F

Lx1

1.6

i1d

ω

L

*

1.6

i1q

ω

L

F

F

P

-

-

iqref

D

D

+

-

Vqref1

I

Lx2

Fig 6: Decoupled Controller.

In the selected circuit, the grid transformer rating is 4 kV (secondary) , 1 MVA with 10%
leakage, giving an impedance

ω

L= 1.6

Ω

. Similarly a line-line voltage of 4 kV gives a

line to neutral voltage of 4/

√3

kV, and as we are using peak quantities in the dq conversion,

vd = (

4/

√3) √2

kV

= 3.26

kV.

The detection of the ac grid voltage refernce angle and and the generation of d and q
components of current (as required in Fig 6) are done in a straightforward manner using a
d-q transformation block as in Fig. 7.

The selection of idref for the grid side converter is through the control circuit shown in
Fig 8, which attempts to keep the capacitor voltage at its rated value by adjusting the
amount of real power. The reactive power order is dialed in, but could have been
generated by a similar controller whose objective would be to keep the ac voltage at some
setpoint.

If these reference voltages vdref1 and vqref1 (Fig. 6) are applied at the secondary of the
transformer, the desired currents idref and iqref will flow in the circuit. The remaining
part of the controls are standard PWM controls. The control blocks shown in Fig 9
convert the above references to phase and magnitude, taking care to limit the magnitude

6

to the maximum rating of the grid side VSC converter. The reference for each of the three
phase voltages is then generated by an inverse dq transformation.

Ea

A

Detection of system voltage

mag

X

Va

alfa

r to p

Vsmag

Valfas

Ed

3 to 2

Transform

beta

B

Y

phi

Y

Vb

X

Vbetas

phi

phivs

C

Vc

Detection of d-q components of currents. The washout
filters remove dc components. Phase change of 0.01326 rad
corrects washout filter phase error

i1a

i1alfa

phi

A

mag

X

mag

X

G

1 + sT

sT

1 + sT

i1a

Ecapref

Ecap

I

P

D +

F

+

G

sT

+ sT

Kpcvc

Ticvc

D +

F

-

Ecap

G

1 + sT

idref

1

Fig 8: Voltage controller

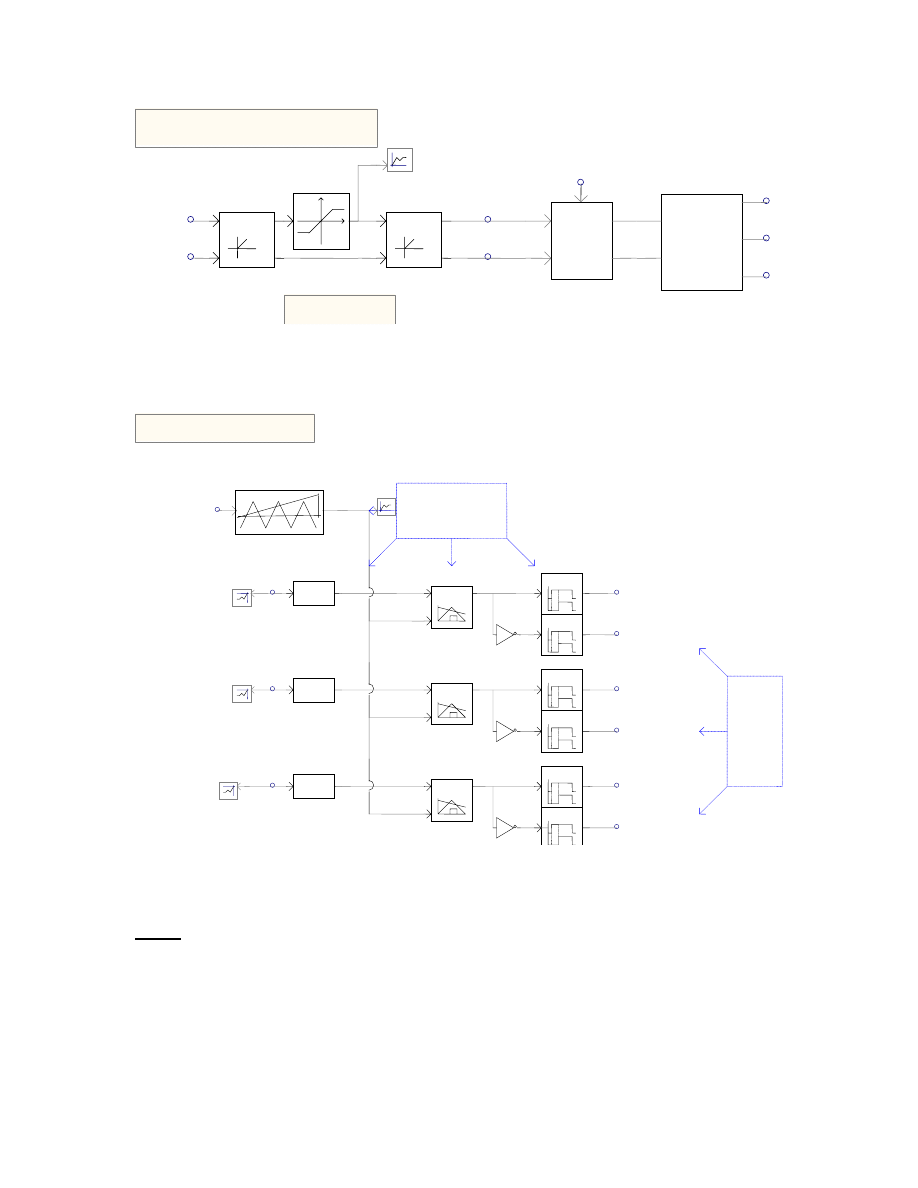
Fig.10 shows a standard sinusoidal PWM controller, in which each of the phase voltages
is compared with a high frequency triangle wave to determine the firing pulse patterns.

i1c

i1b

G

sT

1 + sT

i1beta

i1q

0.01326

Y

X

phi

Y

p_to_r

i1d

i1d

i1q

G

1 + sT

Stator

alfa

to Rotor

beta

Q

D

alfa

G

r to p

3 to 2

Transform

beta

B

Y

phi

Y

X

+

D

-

C

F

Fig 7: Generation of quantities required for the controller in Fig. 6

7

Generation of PWM Reference Voltages

mag_of_v

phi

mag

A

mag

X

X

to Stator

Rotor

alfa

Q

D

Varef

alfa

r to p

p_to_r

Vdref1

vdref

2 to 3

Transform

beta

B

Y

Y

phi

Y

Vbref

X

X

beta

phi

Y

Vqref1

vqref

C

Vcref

Magnitude Limiter

Fig 9: Phase reference voltage generator

1.26 kHz SPWM Firing Pulse generator

PWM and

phi

phi

tri

IGBT Firing

phi

Control

tri

Varef

Vamag

Delay

*

0.2

A

Compar-

ator

T

T1s

B

Delay

T

T4s

Vbmag

Delay

PULSES

FIRING

*

0.2

A

IGB
T

Co

r-

mpa

ator

T

T3s

Vbref

B

Delay

T

T6s

Vcref

Vcmag

Delay

*

0.2

A

Co

r-

mpa

ator

T

T5s

B

Delay

T

T2s

Fig 10: SPWM pulse generator

Tests:

The following tests can be conducted to check the operation. Set the generator on “speed
Control”, i.e., the machine will run at the speed designated by the slider. This is realistic
because any externally connected wind turbine model would interface to the machine
module through the “speed signal”. Set the speed to 0.8 pu.

8

Set idref=0.5 pu and iqref =0 pu for the rotor side converter and and vref = 10 kV and
iqref (Q order) for the grid side converter. Start the system. Observe that the powers are
indeed as expected. Increase idref (rotor converter) to 1 pu. The change should be
effected without any change in the reactive power. Similarly change iqref to 0.3 pu. And
observe that P does not change.

Change machine speed to 1.1 pu., with (rotor side) idref=0.5 pu and iqref =0. Notice that
the torque stays the same, but the power goes up with no change in reactive power. This
is because keeping idref constant maintains constant torque, and so P is proportional to
speed.

Monitor grid side converter currents. Observe that the dc capacitor voltage remains fixed
at its rated value and grid side currents are in phase with the ac voltage.

References:

1) R. Pena, J.C. Clare and G.M. Asher, “Doubly fed induction generator using back to back PWM converters and its
application to variable speed wind energy generation”, IEE Proc. Electrical Power Appliucations, Vol. 143., No.3.,
May 1996.

Appendix

A. Current Ref. PWM (CRPWM)
Current Reference PWM allows for the generation of any arbitrary current waveform in
an R-L load. As shown in Fig. A1, an upper and lower tolerance band is placed around
the desired reference waveform for the current as in the above figure. If the actual current
is below the lower threshold, the upper switch (T1/D1) is turned on which applies a
positive voltage(E/2) to the load. The current in the source thus rises in response to this
voltage. When the current rises above the upper threshold, the upper switch is turned off
and the lower switch (T2/D2) is turned on. This applies a negative voltage (-E/2) to the
load and causes the current to drop. Thus the difference between the desired and actual
currents is kept to within the tolerance band. By making the thresholds smaller, the
desired current can be approximated to any degree necessary. Note however, that there is
a limit to which this can be done, because the smaller the threshold, the smaller the
switching periods, i.e., the higher the switching frequency and losses.

Using this technique, any given current waveform can be synthesized. A method that
removes all harmonics can be constructed using the approach shown in Figure A1.
This approach suffers from the drawback that the switching frequency is not predictable
and can be very high making the circuit less attractive for larger ratings such as ac side
filters.

9

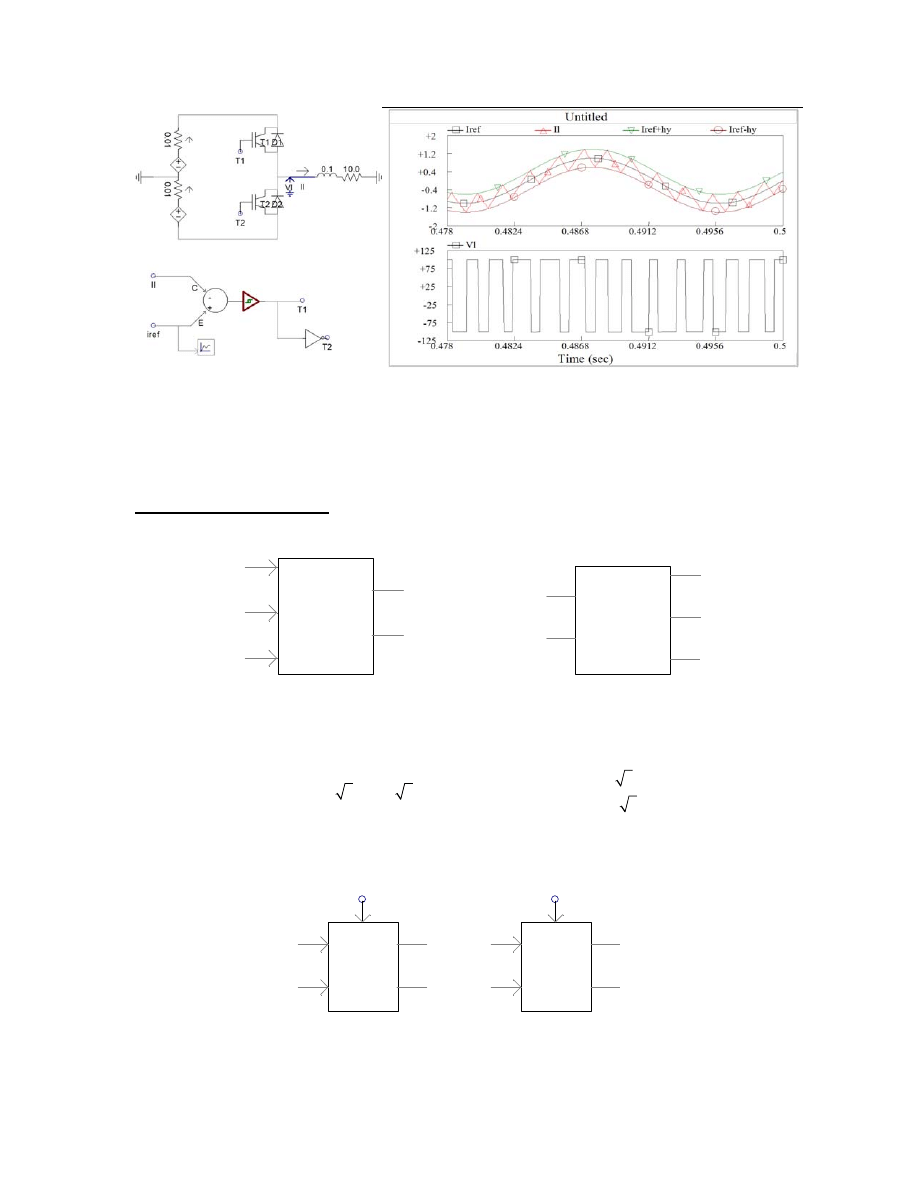
E/2

E/2

Fig A1: CRPWM Controller and Waveforms

B: Transform Equations:

•

Clarke’s Transformation

A

B

C

3 to 2

Transform

alfa

beta

A

B

C

2 to 3

Transform

alfa

beta

(

Forward (abc to

α

β )

Reverse

(α β

to abc)

1

1/ 2

1/ 2

2 / 3

0

3 / 2

3 / 2

a

b
c

α

β

 

−

−





 

 

=





 

 





−

 



 

 

1

0

1/ 2

3 / 2

1/ 2

3 / 2

a

b
c

α

β





 



  

 

= −



  

 

 





 

−

−

  



(A1)

•

Park’s Transformation

theta

theta

Stator

to Rotor

alfa

D

Q

beta

to Stator

D

Q

Rotor

alfa

beta

Forward (

α β

to dq) Reverse (

α β

to dq)

10

11

α

cos( )

sin(

sin( ) cos( )

d
q

θ

θ

θ

θ

β

 

 



  

=



  

−

  

  

cos( )

sin(

sin( ) cos( )

d
q

α

θ

θ

β

θ

θ

−

  

  

=





 

 

  

  

…….(A2)