A cage induction generator using back to back PWM converters for variable speed

grid connected wind energy system

Ruben Peña

Roberto Cardenas

Ramón Blasco

Greg Asher

Jon Clare

Department of

Electrical Engineering

Department of

Electrical Engineering

Department of System

Eng. And Automation

School of Electrical &

Electronic Engineering.

School of Electrical &

Electronic Engineering.

University of

Magallanes

University of

Magallanes

Polytechnic University

of Valencia

University of

Nottingham

University of

Nottingham

Casilla 113-D. Punta

Arenas

Casilla 113-D. Punta

Arenas

Camino de Vera, s/n.

Valencia

University Park. NG7

2RD. Nottingham

University Park. NG7

2RD. Nottingham

Chile

Chile

Spain

England

England

r p e @ o n a . f i . u m a g . c l r c d @ o n a . f i . u m a g . c l r b l a s co @i s a .u p v . e s . [e-mail] [e-mail]

Abstract: A new control scheme of a variable speed grid

connected wind energy generation system is presented. The
scheme uses a cage induction generator driven by an
emulated wind turbine with two back-to-back voltage-fed
PWM inverters to interface the generator and the grid. The
machine currents are controlled using an indirect vector
control technique. The generator torque is controlled to
drive the machine to the speed for maximum wind turbine
aerodynamic efficiency. The supply side converter currents
are also controlled using a vector approach using a
reference frame aligned with stator voltage vector. The DC
link voltage of the power electronics interface is controlled
acting upon the supply active power current component
using a non-linear control and a fuzzy based interpolation of
linear PI controllers to improve the disturbance rejection
and meet noise considerations in steady state. Experimental
validation of the proposed control scheme on an emulated
3.2kW wind energy system is shown.

I. INTRODUCTION.

Squirrel cage induction motors are the most commonly

used electrical machine in AC drives, because they are
robust, cheap and have low maintenance cost. These
advantages make the induction machine very attractive for
wind power applications both for fixed and variable speed
operation. To take advantage of the higher energy capture
and increase in the system compliance resulting from
variable speed operation a power electronic interface must be
provided between the machine terminals and the grid. The
back-to-back PWM inverter based power electronics
interface is a suitable option for cage induction machine in
wind power applications [1]. Vector control techniques are
used to control the machine currents allowing high current,
and therefore torque, dynamics and control of the excitation
or flux of the machine [2]. The supply side converter currents
are also controlled using a vector control approach leading to
an independent control of active and reactive power flow
between the supply converter and the grid [3,4]. The power
is therefore injected into the grid with low distortion currents
and close to unity power factor.

In a variable speed wind energy system, below rated wind

velocity, the electrical torque is controlled in order to drive
the system into an optimum speed for maximum energy
conversion [5,6]. In this paper a control strategy is presented

for a cage induction generator driven by a wind turbine and
supplying energy to the grid via a power electronics
interface. A strategy for optimum wind turbine speed
tracking is presented and the DC link voltage of the power
electronics interface is controlled using a non-linear
approach together with a fuzzy logic based interpolation of
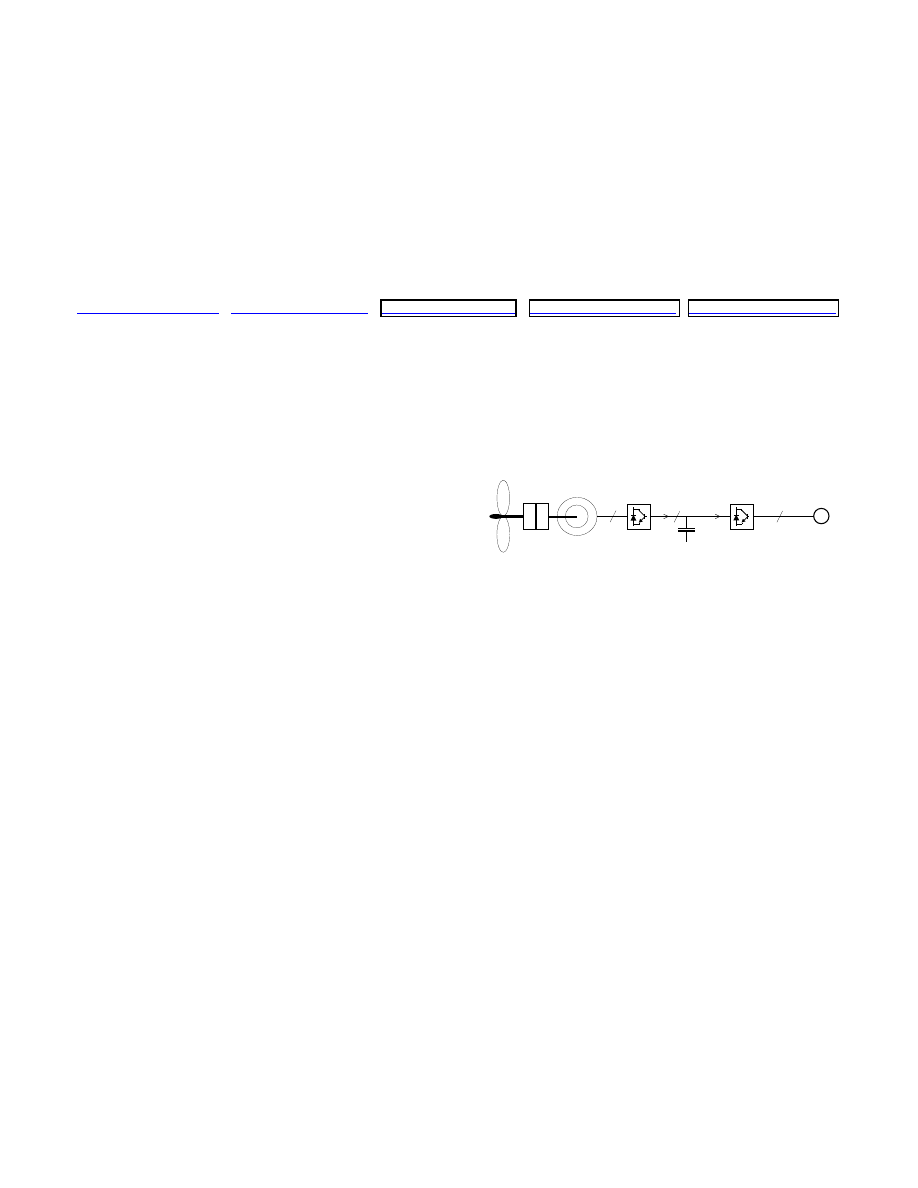
linear PI controllers. Fig.1 shows a schematic of the system..

Gearbox

Grid

3

1

i

G

i

L

3

Fig. 1. Schematic of the overall system

.

This paper is organised as follows. In section 2 a

description of the experimental set up is presented, section 3
describes the vector control of the induction machine and the
modelling of the wind turbine. In section 4 the DC link
voltage control strategy for the supply side converter is
presented. Section 5 shows experimental results and in
section 6 some final remarks are given.

II. EXPERIMENTAL SYSTEM

The system consists of two back-to-back PWM voltage

fed inverters connected between a 2.5kW, 1450 rpm Cage
Induction Generator (CIG) and the grid. The supply voltage
has been set to 250V via a three phase variac. The DC link
voltage is regulated at 550V providing enough modulation
index excursion during transients to avoid overmodulation
problems. Three 12mH filter inductances are connected
between the grid and the supply side converter. The
switching frequency of the PWM converters is 1kHz, the
position is measured with a 10000 ppr. encoder. The
sampling time of the current, voltages and position, as well
as the voltage and current control loops is set to 0.5ms unless
specified otherwise. Fig. 2 shows a schematic of the system.
The generator is driven by a four-quadrant DC drive
emulating a wind turbine. For a given wind velocity and
rotational speed a signal representing the wind turbine torque
is obtained using a look up table of the wind turbine
aerodynamics characteristics. This signal together with the
electrical torque are used to generated a reference speed

current
control

3-

φ

supply

Speed

Control

v

ω

r

d/dt

θ

r

optimal

tracking

control

i

sd

∗

i

sq

∗

i

sa,b

E

machine

vector

control

PWM

CIG

DCM

3

PWM

supply-side

vector

control

i

a,b

3-phase

variac

i

G

i

q

∗

E

∗

3

Turbine

Emulator

ω

r

∗

T

e

∗

Fig. 2. Experimental set-up

signal for the DC drive, emulating a 3.2 kW wind turbine
with given inertia and friction coefficient [7,8]. The entire
system is controlled using a microprocessor network [9]

III. INDUCTION MACHINE CONTROL AND WIND

TURBINE OPTIMUM SPEED TRACKING.

The CIG currents are controlled using a standard indirect

vector control, with the current and voltages referred to a d-q
synchronous frame aligned with the rotor flux vector [2]. The
flux

λ

dr

and the electrical torque T

e

are given by:

qs

mrd

r

o

e

r

sd

o

mrd

o

dr

i

i

L

L

p

k

T

s

T

i

L

i

L

2

1

2

1

=

+

=

=

λ

(1)

Where k

1

depends on the transformation used and i

sd

, i

sq

,

i

mrd

, are the d-q axis current and the magnetising current

respectively. L

0

, L

r

and T

r

are the magnetising and stator self-

inductances and the rotor time constant respectively. High
bandwidth current control for the d-q stator component
currents is achieved with the vector control approach.

For below rated wind velocity, the mechanical power, P

m

,

of a fixed-pitch wind turbine is a function of the effective
wind velocity trough the blades v, the air density

ρ

, the

blades radius R and the power coefficient C

p

. And is given

by [6]:

3

2

)

(

5

.

0

v

R

C

P

p

m

λ

ρ

=

(2)

Considering the rotational speed of the wind turbine

ω and

the torque coefficient C

t

, the analytical expressions for the

wind turbine mechanical torque is given by [6]:

)

(

)

(

)

(

5

.

0

2

3

λ

λ

λ

λ

ρ

t

p

t

m

C

C

v

R

C

T

=

=

(3)

Where

λ is the tip speed ratio defined by:

v

R

ω

λ =

(4)

Fig. 3 shows a typical wind turbine power coefficient

versus tip speed ratio.

λ

opt

C

p,max

C

p

λ

Fig. 3.

Power coefficient versus tip speed ratio

Therefore, the rotational speed

ω

opt

for maximum

aerodynamic efficiency for a given wind velocity is given by:

R

v

opt

opt

λ

ω

=

(5)

The mechanical torque at the optimum operating speed is

given by:

2

,

opt

opt

m

K

T

opt

ω

ω

ω

=

=

(6)

In this work, a first order model has been used to describe

the dynamic of the wind turbine given by:

(7)

Where J is the system inertia, T

e

is the electrical torque

and B the friction torque coefficient. By setting T

e

,

and the

reference i

sq

current as:

(8)

The mechanical equation becomes:

(9)

Therefore, the accelerating torque is equal to the

difference between the turbine mechanical torque and the
torque at optimum C

p

. Eventually the machine will reach the

optimum operating point.

Equation (7) and Fig. 3 are used to emulate the wind

turbine via a four-quadrant speed controlled DC drive. The
reference speed for the DC drive is given by:

J

T

k

B

k

T

k

T

k

k

w

e

m

r

r

))

(

)

(

)

(

(

)

(

)

1

(

*

*

ω

ω

ω

−

−

+

=

+

(10)

Where T

w

is the sampling time of the DC drive speed

control loop, set to 5ms, and J and B are the emulated system
inertia and the torque friction coefficient respectively. For a
given wind velocity, the wind turbine mechanical torque is
obtained using the wind turbine parameters, given in the
Appendix, together with the knowledge of the system speed
and a look-up table of the torque coefficient versus the tip
speed ratio, obtained from Fig 3.

IV.SUPPLY SIDE CONVERTER CONTROL

STRATEGY.

The supply side converter in Fig.1 is used injects the

generated power into the grid. By using vector control
techniques the currents in the ac side of the converter are
controlled with very high bandwidth. The orientation of the
reference frame is done along the supply voltage vector to
obtain a decoupled control of the active and reactive power.
Usually the reactive power component current is set to zero
for near unity power factor operation. The main aim of the

front-end converter control strategy is to keep the DC link
voltage E constant. It can be shown that the dynamic for the
DC link voltage E, under vector control and power factor
near to unity, is:

(11)

Where i

d

is the d-axis power current component, L

1

and R

1

are the line filter parameters, C is the DC link voltage
capacitor and v

d

is the supply voltage in d-q co-ordinates and

k is a constant which depends on the transformation used.
The effect of R

1

can be neglected and L

1

is usually small. The

term

2

1 d

i

R

is always positive and

2

1

5

.

0

d

pi

L

is bounded,

hence this simplification does not affect stability of the
closed loop system. The i

d

current is used to regulate the DC

link voltage. However, the relationship between E and i

d

is

non-linear. By choosing E

2

as the controlled variable

equation (11) becomes:

o

d

d

P

i

kv

pE

C

−

=

2

2

(12)

Because of the generally unknown generating conditions

the generated power P

o

is considered as a disturbance for the

DC link control loop. In order to have good DC link voltage
regulation, hence fast disturbance rejection, high open loop
gains are required. However, such high gains will cause
increased DC link voltage noise and, consequently, AC
current ripple. In this work a Fuzzy Logic Controller (FLC)
[10] is used to continuously change the bandwidth of the
voltage controller by interpolating between several linear PI
controllers. The strategy is as follows: Consider a digital
linear PI controller described by:

)

1

(

)

(

−

−

=

∆

k

ae

K

k

e

K

u

p

p

PI

(13)

This controller can be implemented using a Sugeno type

structure [11], with two membership functions, NEG and
POS, for both e(k) and e(k-1),

with

1

2

2

/

))

(

)

(

(

)

(

k

k

E

k

E

k

e

ref

−

=

, E

ref

the reference voltage

and k

1

a scaling factor for ensuring the membership domain

lies between

±1. The control signal

e

ref

k

k

E

k

E

k

e

/

)

1

(

)

(

)

(

−

−

=

, with k

e

again a scaling factor,

is used for the linear interpolation. In this work three PI
controllers are considered with three membership functions
for the control signal

)

(k

e

, S(Small), M(Medium) and

B(Big), corresponding to small, medium and big DC link
voltage error. The input membership functions are shown in
Fig. 4.

The rule base is shown in Table 1, where k

pi

and a

i

are the

proportional gain and zero of the i-th PI controller
respectively (i=1,2,3).

2

*

2

o

e

r

q

opt

e

L

T

L

k

i

B

K

T

=

−

=

ω

ω

2

ω

ω

opt

m

K

T

dt

d

J

−

=

G

o

d

d

d

d

Ei

P

P

i

R

dt

di

L

i

v

k

dt

dE

C

=

−

−

−

=

0

2

1

2

1

2

)

2

(

2

ω

ω

B

T

T

dt

d

J

e

m

−

−

=

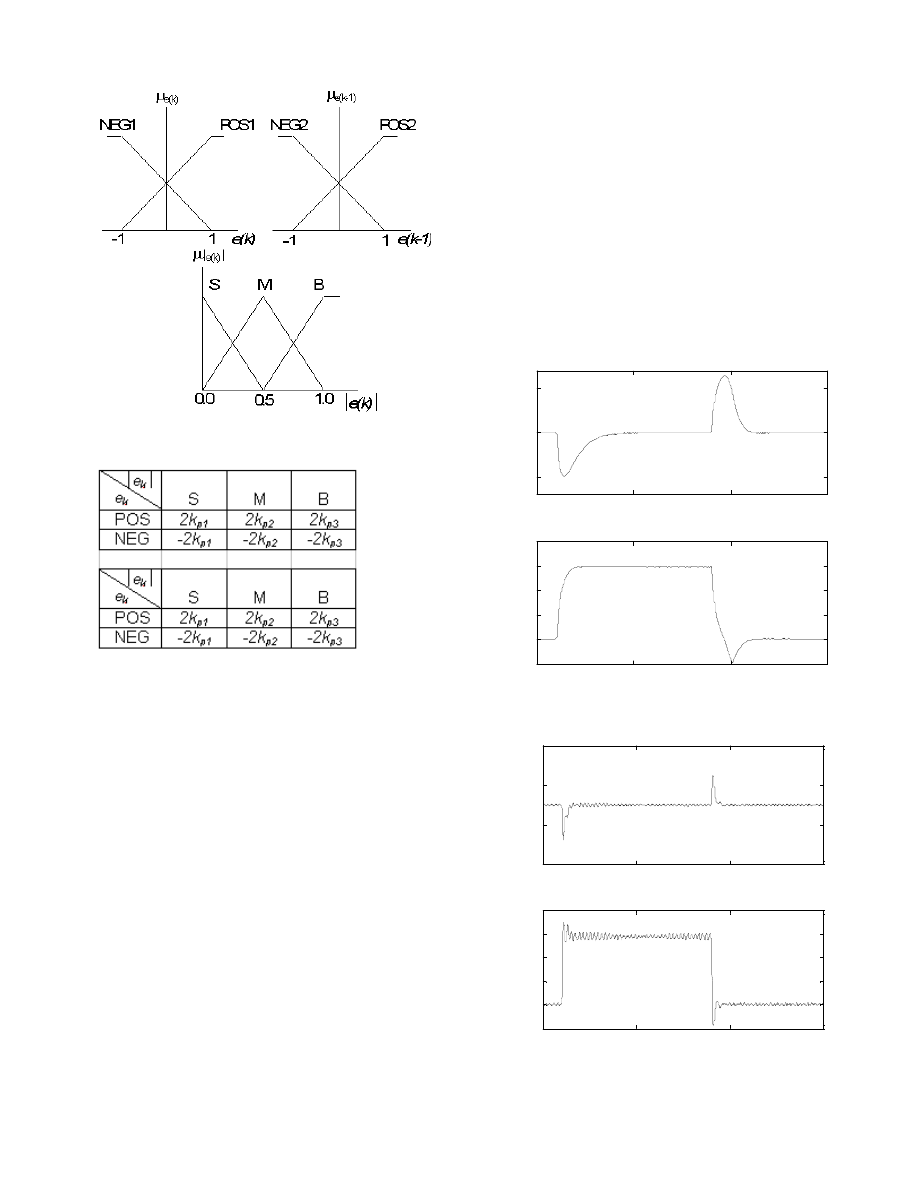
Fig. 4. Input membership functions

TABLA 1. Rule base

V. EXPERIMENTAL RESULTS.

The proposed control strategies have been experimentally

validated using the experimental set up shown in Fig. 2.

A. Experimental Results for the DC Link Voltage Control
Strategy.

The disturbance rejection performance of the DC link

voltage controller has been tested against load impacts using
a chopper controlled resistive load connected to the DC link.
Three DC link voltage PI controllers have been implemented
with closed loop natural frequencies of

ω

1

=20rads

-1

,

ω

2

=60rads

-1

,

ω

3

=120rad

-1

respectively, the DC link voltage

being regulated at 550V. As seen from Fig. 4, the high
bandwidth controller is used for large DC link voltage
excursions, while the low bandwidth controller reduces
system noise under steady state conditions. A 2.5kW load
impact has been applied to the DC link at t=0.1s and
disconnected at t=0.9s.

Fig. 5 shows the disturbance rejection performance of the

slowest PI controller. The top graphic shows the DC link
voltage and the bottom graphic shows the d-axis reference
current. The noise in the d-axis reference current is quite

small; however the voltage excursion is above 50V. Fig. 6
shows the DC link voltage performance for the same load
impact with the faster PI controller. The disturbance rejection
is very good with a maximum excursion of the DC link
voltage of about 20V, however the noise in the d-axis current
is noticeable

Fig. 7 shows the performance of the proposed fuzzy based

DC link voltage controller with a linear interpolation of PI
controllers, for the same load impacts. As seen from Fig. 7
the voltage regulation is also good, with a maximum
excursion of 22V, but the steady state noise in the d-axis
reference current is kept very low. The controller has low
closed loop bandwidth for small voltage errors, in order to
keep reduced noise, and high bandwidth for high errors
hence excellent load impact rejection is maintained.

0

0.5

1

1.5

500

550

600

Time(s)

D

C

L

in

k V

ol

tag

e (

V

)

0

0.5

1

1.5

-2

0

2

4

6

8

Time(s)

R

efe

re

nc

e Id

c

urr

en

t(

A

)

Fig. 5. Dc link voltage performance with a slow PI controller

0

0.5

1

1.5

520

540

560

580

Time(s)

DC

li

nk

vo

lta

ge

V

0

0.5

1

1.5

-2

0

2

4

6

8

Time(s)

R

efe

re

nc

e Id

c

ur

re

nt

(A

)

Fig. 6. DC link voltage disturbance rejection with a fast controller.

0

0.5

1

1.5

520

540

560

580

Time(s)

DC

lin

k vo

lta

ge

V

0

0.5

1

1.5

-2

0

2

4

6

8

Time(s)

R

efe

re

nc

e Id

c

urr

en

t(

A

)

Fig. 7. DC link voltage performance with the proposed fuzzy controller

B. Experimental Results for the Wind Turbine System.

The performance of the optimum wind turbine speed

tracking operation is shown in Figures 8 and 9 for realistic
wind profiles. In order to demonstrate the wind turbine
emulation scheme, two different wind turbines have been
considered. Fig 8 shows the speed tracking performance for a
wind turbine system with an inertia of 1kgm

2

and a friction

coefficient of B=0.002Nmsrad

-1

. The top graphics shows the

system rotational speed whereas the bottom graphics shows
the speed error. The speed error has been obtained using
considering the optimum speed operation given by (4)

0

50

100

150

600

800

1000

Time(s)

S

pe

ed

(rp

m

)

0

50

100

150

-400

-200

0

200

400

Time(s)

S

pe

ed

e

rro

r(rp

m

)

Fig. 8, Speed tracking for a system with small inertia.

Fig. 9 shows the performance of the speed tracking

strategy with an emulated wind energy system having an
inertia of 5kgm

2

. The top graphic shows the speed and the

bottom graphic shows the speed tracking error. As can be

seen form this figure, the tracking error increase because of
the higer inertia of the system.

0

50

100

150

600

800

1000

Time(s)

S

pe

ed(

rp

m

)

0

50

100

150

-400

-200

0

200

400

Time(s)

S

pe

ed

e

rr

or(rp

m

)

Fig. 9 Speed tracking with a higher inertia system.

Finally, Fig.10 shows the steady state phase voltage V

a

and supply current injected into the grid I

a

when the system

is operating at optimum speed with a wind velocity of
8.3m/s.

The q-axis supply reference current has been set to zero,

therefore the phase angle between the phase voltage and the
current is nearly 180

0

.

0

0.01

0.02

0.03

0.04

0.05

0.06

-200

-150

100

-100

-50

0

50

100

150

200

Time(s)

P

has

e V

ol

tage

(

V

)

0

0.01

0.02

0.03

0.04

0.05

0.06

-10

-8

-6

-4

-2

0

2

4

6

8

10

S

uppl

y C

ur

re

nt

(

A

)

Ia

Va

Fig. 10. Supply current and phase voltage for wind velocity of 8.3m/s

VI. FINAL REMARKS.

A new control system for a variable-speed cage induction

generator driven by wind turbine has been presented. Two
back-to-back voltage fed vector controlled inverters have
been used to interface the generator and the grid. The DC
link voltage is controlled using a fuzzy based controller. The
controller output is a fuzzy based interpolation of different PI
controllers. Experimental results have shown high dynamic

response for DC link disturbance rejection while keeping the
steady state control signal noise, i.e. the supply active current
component, very low.

The wind turbine has been emulated using a speed

controlled DC drive. The strategy is very flexible allowing
different wind turbines to be emulated with minimum
software modifications. Experimental results for tracking the
optimum operating speed of the wind turbine system have
been presented for two different wind turbine using a
realistic wind profile.

Experimental results have also shown the operation of the

system with low distortion currents injected into the grid
with close-to-unity power factor.

VII ACKNOWLEDGEMENTS

The financial support provided by Fondecyt, through

projects 1980688 and 7980077, is kindly acknowledged. The
financial support from The British Council via The
Academic Link Program is also acknowledged.

VIII REFERENCES.

[1]

Jones S.R., Jones R., "A Control Strategy for

Sinusoidal Supply Side Converters", IEE Colloquium
on Developments in Real Time Control for Induction
Motor Drives", DIGEST No. 1993/024, 1993.

[2]

Leonhard W, "Control of Electrical Drives", Spring

Verlag 1985.

[3]

Rim C T., et al, “A complete DC and AC Analysis of

Three-phase Current PWM Rectifier Using d-q
Transformation”, IEEE Trans. on Power Electronics,
Vol. 9, No. 4, 1994, pp. 390-396.

[4]

Zargary N, Joos G, “Performance Investigation of a

Current-controlled Voltage-regulated PWM Rectifier
in Rotating and Stationary Frames”, IEEE Trans. on
Industrial Electronics, Vol. 42, No. 4, 1995, pp. 396-
401.

[5]

Buchring J K, Freris L, "Control Policies for Wind-

energy conversion Systems", IEE Proc. C., Vol. 128,
No. 5, 1981, pp 253-261.

[6]

Freris L., “Wind Energy Conversion Systems”,

Prentice Hall, 1990.

[7]

Z. Hakan, G. Asher, J. Clare, “Dynamic Emulation of

Mechanical Loads Using a Vector-Controlled
Induction Motor-Generator Set”, IEEE Transaction on
Industrial Electronics, Vol 46, Nr. 2 April 1999.

[8]

R. Cardenas, R. Peña, G.M. Asher, J.C. Clare,”

Experimental emulation of wind turbines and
flywheels for wind energy applications”, European
Power Electronics and Applications Conference,
EPE2001, Graz Austria, August 2001, CD ROM

[9]

R. Peña, J.C. Clare, G.M. Asher, "A doubly fed

induction generator using back to back PWM
converters and its application to variable speed wind
energy generation", IEE-Proceeding part B (Electric
Power and Applications), pp. 231-241. May 1996.

[10]

D. Driankov, H. Hellendoorn, M. Reinsrank, “An

Introduction to Fuzzy Control”, Springer-Verlag, 1993.

[11]

Hakan A., Asher G., Clare J., “Equivalence of fuzzy

and classical controller: An approach to fuzzy control
design”. EPE’99, Lausanne. Switzerland. 1999.

APPENDIX.

Wind Turbine:

Power 3.2kW,
Blade radius R =2.26m
Rated speed=296rpm
Rated wind velocity=10ms

-1

Gearbox = 2.836

Cage Induction Machine:

Rated speed: 1450rpm
Rated field current I

d

=1.8A,

Rated torque = 4.6A
R

s

=2.1

Ω R

r

=1.7

Ω

L

s

=0.4186H, L

0

=0.4058H, L

r

=0.4186H.

Inverters:

Power: 5kW
Switching frequency: 1kHz
DC link Capacitor: 1200

µF