
Z. angew. Math. Phys. 53 (2002) 923–948
0044-2275/02/060923-26
c
° 2002 Birkh¨auser Verlag, Basel
Zeitschrift f¨
ur angewandte
Mathematik und Physik ZAMP
Modeling of the microwave drying process of
aqueous dielectrics
K. R. Rajagopal and L. Tao
Dedicated to the memory of Eugen So´
os
Abstract. We propose a thermodynamic framework for describing the microwave drying pro-
cess of aqueous dielectrics based on Maxwell-Lorentz field equations and mixture theory. Several
issues are discussed such as the form of entropy equation; the constitutive relations for the macro-
scopic electric polarization vectors, Cauchy stresses, heat fluxes, internal momentum supplies,
etc., for each component of the mixture: porous solid, water and gas in different regions; and the
interfacial jump conditions between different regions in the mixture. A brief examination of the
status of material frame indifference within the context of our framework is presented.
Mathematics Subject Classification (2000). 76A99, 76S05, 76T30, 78A25, 78A40, 80A17,
80A20.
Keywords. Aqueous dielectric drying, frame indifference, Maxwell’s equations, microwave heat-
ing, mixture theory.
1. Introduction
We derive most of our energy from electromagnetic radiation from our environ-
ment. The complete electromagnetic spectrum spans the gamut from exceedingly
short wave lengths associated with γ -rays of the order of 10
−5
µm , to X -rays
between 10
−4
µm to 10
−2
µm , the ultraviolet to the visible spectrum onto in-
frared rays of wave lengths between 1 µm and 10
2
µm and microwave radiation
with wave lengths greater than 10
2
µm . This electromagnetic radiation that is
received by us is converted into energy in various forms: chemical, mechanical
and thermal, and the nature of the conversion depends on the body that is being
irradiated as well as the wave length of the electromagnetic radiation. Energy
associated with certain wave lengths is not absorbed by certain bodies (molecules)
while that associated with other wave lengths is, in fact this is the basis for time
resolved spectroscopy. In this paper we are interested in the conversion of electro-
magnetic radiation of wave lengths in the range of microwaves into energy in the

924
K. R. Rajagopal and L. Tao
ZAMP
thermal form, commonly referred to as heat.
Microwave provides an effective means to dry aqueous dielectric materials due
to its ability to transfer energy directly to the interior of the materials which
generates internal heating through polarization. To date there is no comprehensive
model in place to describe this phenomenon and thus it offers an interesting and
challenging problem concerning the modeling of this drying process. The present
work attempts to propose a thermodynamic formulation regarding the modeling
within the context of Maxwell-Lorentz field equations and mixture theory under
the premise that such a continuum treatment is appropriate.
Any attempt at developing a model of the process of drying an aqueous di-
electric through microwave radiation has to come to grips with how to model the
supply of the electromagnetic radiation to the body. The mechanism by means
of which energy in the electromagnetic form is converted to heat is not yet well
understood for even reasonably simple molecules, though some understanding has
been achieved for simple monatomic gases. While dealing with continua we at
the very least need to provide a phenomenological continuum description of the
radiation received by the body, i.e., we need to provide a constitutive equation
for the radiant energy received or absorbed by a body. We realize this need for a
constitutive description for we recognize that for the class of “black bodies” and
for electromagnetic radiation in a certain range of wave lengths we do have such a
specification. However, for bodies other than this special class and for electromag-
netic radiation of different wave lengths, we know little concerning the constitutive
form.
In most thermodynamic studies in classical continuum thermomechanics, ra-
diation is essentially ignored, and even if it is not ignored it is eliminated from
consideration in the entropy equation in virtue of the following practice. Let us
consider the somewhat common scheme in classical continuum thermomechanics
where the energy equation is given for a single continuum in the local form
ρ
dU
dt
= tr (
τ L) − ∇·q + ρr,
where ρ denotes the density, U the specific internal energy,
τ the Cauchy
stress, L the velocity gradient, q the heat flux vector, r the radiant heating and
∇ denotes the eulerian spatial gradient. Let us suppose that the second law is
given through the Clausius-Duhem inequality ([13])
ρ
ds
dt
+
∇·
q
T
−
r
T
= σ
≥ 0, (∗)
where s , T and σ are, respectively, the entropy density, absolute temperature
and the internal source of the entropy besides r . We can now eliminate r from
the above equation by substituting for it from the energy equation to obtain what
is popularly referred to as the dissipation inequality, namely
σ = ρ
µ
ds
dt
−
1
T
dU
dt
¶
+
1
T
tr (
τ L) −
q
·∇T
ρT
2
≥ 0. (∗∗)

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
925
Next, the standard practice in continuum thermodynamics is to require that all
processes that the body can undergo meet the above inequality. This usually
places restrictions on the forms that the constitutive equations for the internal
energy, entropy, stress and heat flux can take. There are some problems with
regard to such an approach. First, (**) places no restrictions on the constitutive
representation for the radiant heating, though (*) indicates that it cannot be
arbitrary. Of course, not all forms of the radiant heating, when substituted into
the energy equation will lead to well-posed problems, but the way, in which the
second law is brought into play, namely (**), provides no restrictions with regard
to the entropy production due to radiant heating, directly. Second, it is common
to exploit (**) by assuming that (**) holds for arbitrary processes. However, it is
unreasonable to expect (**) to hold for any real material for all arbitrary processes
for the constitutive assumptions that we start with are only expected to hold for
a certain class of processes. For example, if we are trying to model rubber as an
elastic material, we cannot really subject it to arbitrary deformations, it will either
start behaving inelastically or fracture and fall apart. While mathematically we
can define an ideal elastic body, restrictions obtained from such a definition will
not have its intended meaning for a real body. To be more explicit, if (**) is
required to hold only for a class of processes, then the restrictions imposed are
far less severe and thus a larger class of models would be allowable. This point
cannot be overemphasized. Constitutive relations for bodies have meaning only
for a specified class of processes. To overcome the first difficulty we offer to include
the the radiant heating as a part of the internal source of entropy by redefining σ
which will allow the second law to place rather strigent restrictions on the form
of the heating. A similar scheme has also been adopted by Eringen and Maugin
[3], though they still employ a term r/T in the entropy equation to account for
some other forms of heating, which is not necessary based on the argument offered
above. The second problem can be avoided by seeking and exploring constitutive
relations that satisfy the entropy inequality automatically. Furthermore, there are
other important issues concerning the second law that are far from clear. For
instance, the exact form of the second law for open systems is a matter of much
debate. Also, whether the second law should be enforced as a global inequality for
a body (or for a system) or whether it could be enforced locally is far from obvious
especially as one deals, in the classical context, with sources and sinks. We shall
not get into a discussion of these issues here as they are beyond the intended scope
of this work. Here, we shall assume that the second law can be enforced pointwise.
We are yet faced with the onerous task of assuming a constitutive form for
the microwave heating in an aqueous dielectric. When a body is supplied with
the radiant energy, the vibrational energy, the rotational energy or the translation
energy of the molecules or the atoms can be changed. To try to capture the
manner in which the energy in its electromagnetic form converts into the energy
in its thermal form needs fundamentally an evaluation at the quantum mechanical
level. This is not the spirit of this presentation as we are primarily interested in

926
K. R. Rajagopal and L. Tao
ZAMP
trying to capture the form for the radiation from a phenomenological perspective.
Unfortunately, there is not sufficient amount of experimental data to do this. We
fill this lacuna with a judicious guess work for the radiation, following a statistical
scheme for the basis of the Maxwell-Lorentz field equations.
We are interested in describing microwave drying and we thus have to deal with
electromagnetic fields. Any attempt at incorporating electromagnetic effects nec-
essarily requires us to include Maxwell’s equations into the set of motion equations.
Thus, we need to decide whether we will enforce the requirement of material frame
indifference for this problem. We are aware of the controversy surrounding this
topic (Edelen and McLennan [1]; Wang [15]) and we will not pursue a thorough
discussion of it. We require Galilean invariance, instead of the more restrictive ma-
terial frame indifference, within the framework of classical non-relativistic theory,
for the sake of consistency to put the constitutive relations on the electromag-
netic and the thermodynamic quantities on the same footing. Also, this choice is
motivated by a short analysis to be presented in the Appendix.
We should mention that we do not pursue a scheme of volume-averaging here to
help close the mathematical model of microwave drying (for example, see Whitaker
[16] for details). This type of averaging scheme requires one to know the specific
constitutive relations for the motion of each constituent as a physical continuum
in the sub-domain it occupies in the mixture. With this information, an initial-
boundary value problem can be formally set up, the interactions between the
constituents are supposedly accounted for through some interfacial jump condi-
tions, and then the volume averaging can be carried out. There is some merit
to this scheme for suggesting forms of balance type equations, but it does not
resolve the issue of furnishing the constitutive relations necessary to make the
balance equations closed due to the following reasons. First, there are molecu-
lar interactions among the constituents that can significantly change the behavior
of each constituent and we may not have comprehensive information concerning
these molecular interactions. Second, each constituent will be treated as a math-
ematical continuum occupying the whole domain of the mixture, instead of the
sub-domain the constituent itself occupies physically. This treatment makes the
constitutive relations for the constituent as a mathematically homogenized con-
tinuum differ from the constitutive relations associated with the constituent in
the sub-domain it occupies, since they involve different types of field distributions.
Additional assumptions have to be introduced to overcome this problem in the
volume-averaging scheme, which is tantamount to constitutive assumptions. Fur-
thermore, a conventional scheme of volume averaging to develop the equations of
motion has some (conceptual) difficulties of its own. One difficulty occurs when
the spatial point is near a bounded boundary where a constant representative el-
ementary volume (REV) is not applicable. (A non-constant REV could be used
with complexities.) Another problem is the prescription of boundary conditions
consistent with the volume averaging scheme. An alternative is to seek the scheme
of ensemble averaging. And this issue has been discussed rather thoroughly in the

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
927
studies of turbulence modeling.
In the following section, we shall formulate, within the context of Maxwell-
Lorentz field equations and mixture theory, the electromagnetic field equations
and the equations of motion on the porous solid, water and gas in an aqueous
dielectric for the process of microwave drying. The microwave heating makes it
possible for the presence of several mixture regions inside an aqueous dielectric
body: mixture of a porous solid and water; mixture of a porous solid, water and
gas; and mixture of a porous solid and gas. And therefore, proper constitutive
relations of the macroscopic electric polarization vectors, Cauchy stresses, heat
fluxes, internal momentum supplies and so on have to be provided to characterize
the thermomechanical behavior of these mixtures, which is carried out on the basis
of continuum thermodynamics. The interfacial jump conditions between these
different mixture regions are treated in section 3. In the appendix we discuss
briefly an issue related to the requirement of material frame indifference. The
framework presented here is tentative and much more needs to be done to fix the
specific forms of the constitutive relations based on experimental data and the
solving of specific drying problems.
2. Formulation
Let us consider the microwave drying process of an aqueous dielectric. We have
a porous dielectric solid infused with water that is heated by an electromagnetic
wave of frequency ( ω ) in the range of about 300MHz to 3GHz. The mechanisms of
heating are supposedly orientation polarization and Maxwell-Wagner polarization
due to an alternating electric field (Hasted [6]; Metaxas and Meredith [9]) (these
polarizations are akin to a phenomenological description). In the initial stage
of the heating process, we have a mixture of a porous solid and water. As the
temperature of the mixture increases to some critical value T
c
, water starts to
evaporate (phase change) and a mixture of three constituents, a porous solid, water
and gas (dry steam), is present. Finally water will be converted into gas (dry
steam) completely, and we have a mixture of a porous solid and gas. These three
mixtures can co-exist simultaneously in different regions of the aqueous dielectric
body due to the non-uniform motion and heating. In the following, we will denote
the quantities associated with the three constituents by the superscripts s , w ,
and g , respectively.
Introducing the electric field E and the magnetic induction field B and fol-
lowing a statistical scheme on the basis of the Maxwell-Lorentz field equations
(Eringen and Maugin [3]; de Groot and Suttorp [5]), we can obtain the Maxwell
field equations for the mixture,
∇·E = −∇·
X
P
α
,
(1)

928
K. R. Rajagopal and L. Tao
ZAMP
−c
−1
∂E
∂t
+
∇×B = c
−1
X ·∂P
α
∂t
+
∇×(P
α
× v
α
)
¸
,
(2)
∇·B = 0,
(3)
c
−1
∂B
∂t
+
∇×E = 0,
(4)
where P
α
and v
α
are, respectively, the macroscopic electric polarization vector
and the velocity associated with constituent α , and c is the speed of light in
vacuum. In deriving these equations, we have assumed that the effect of magnetic
polarization is negligible and all the constituents are electrically neutral. Also,
these equations are formulated within the framework of classical, non-relativistic
theory and they are Galilean invariant up to the order of c
−1
. That is, the
equations retain the same form under the transformation
x
0
= x + Vt, E
0
= E
− c
−1
V
×B, B
0
= B + c
−1
V
×E,
P
α
0
= P
α
, α = s, w, g
(5)
where V is constant. The structure of P
α
will be given by constitutive as-
sumptions. We should remark that the above equations hold in all three kinds
of mixtures and the summation in the equations shall take proper terms in each
mixture.
We now formulate the equations of motion for the three mixtures, respectively,
within the context of mixture theory and on the basis of the scheme mentioned
above while ignoring the multi-poles other than dipoles.
2.1. Case 1: Mixture of a porous solid infused with water
When the temperature of the aqueous dielectric body is lower than the critical
temperature T
c
, we have a mixture of a porous solid infused with water. The
balance of mass equations for constituents s and w are
ρ
s
detF
s
= ρ
sR
, F
s
:=
∂x
s
∂X
s
,
(6)
∂ρ
w
∂t
+
∇·(ρ
w
v
w
) = 0,
(7)
where ρ
α
is the effective mass density in the present configuration, X
s
is a
reference configuration for the solid and ρ
sR
is the effective mass density of the
solid constituent in the reference configuration.
The balance of linear momentum equations, for α = s , w , are
∂
∂t
(ρ
α
v
α
) +
∇·(v
α
ρ
α
v
α
) =
∇·τ
α
+ f
α
em
+
X
β6=α
f
αβ
+ ρ
α
g,
(8)

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
929
f
α
em
:= c
−1
"
c (
∇E)·P
α
+ (
∇B)·(P
α
×v
α
)
+
∂
∂t
(P
α
×B) + ∇·(v
α
P
α
×B)
#
,
(9)
f
sw
+ f
ws
= 0.
(10)
Here
τ
α
is the Cauchy stress, f
α
em
is the electromagnetic force acting on the
constituent α , f
αβ
is the internal momentum supply to the constituent α from
the constituent β , and g is the gravitational acceleration. The stress
τ
α
is
asymmetric and meets
X h
τ
α
− (τ
α
)
T
i
=
X
(
E
α
⊗P
α
− P
α
⊗E
α
) ,
E
α
:= E + c
−1
v
α
×B.
(11)
Let U
α
be the internal energy density associated with the constituent α , then
we have for α = s , w ,
∂
∂t
·
ρ
α
µ
1
2
|v
α
|
2
+ U
α
¶¸
+
∇·
·
v
α
ρ
α
µ
1
2
|v
α
|
2
+ U
α
¶¸
=
∇·(τ
α
v
α
− q
α
) + h
α
em
+
X
β6=α
¡
f
αβ
·v
α
+ h
αβ
¢
+ ρ
α
g
·v
α
,
(12)
h
α
em
:=
∂P
α
∂t
·E − c
−1
(P
α
×v
α
)
·
∂B
∂t
+
∇·(v
α
P
α
·E) ,
(13)
f
sw
·v
s
+ h
sw
+ f
ws
·v
w
+ h
ws
= 0,
(14)
where q
α
is the diffusive flux, h
α
em
is the energy source due to the electromag-
netic field, and h
αβ
is the internal energy supply to the constituent α from the
constituent β . Assuming that the solid constituent and the water have the same
temperature field T ,† we can deduce from the above equations, with the help of
the balance of linear momentum equations, that
−
X
ρ
α
d
α
dt
U
α
+
X½
E
α
·
d
α
dt
P
α
− ∇·[q
α
+ P
α
E
α
·(v
α
− v
s
)]
¾
+
X
tr [(
E
τ
α
+ P
α
·E
α
1) L
α
] +
·
f
sw
+ (
∇P
w
)
E
w
−
³
∇P
w
´
E
w
+
∇·(P
w
⊗E
w
)
¸
·(v
w
− v
s
) = 0,
(15)
† Different temperature fields can be associated with the solid and the water, and the con-
vective heat transfer between the two constituents can be accounted for through a constitutive
relation. We do not get into this issue in the formulation here, both for the sake of simplicity
and due to the many uncertainties associated with the drying of the aque- ous dielectric.

930
K. R. Rajagopal and L. Tao
ZAMP
where
d
α
dt
:=
∂
∂t
+ v
α
·∇, L
α
ij
:= v
α
i ,j
,
E
α
:= F
sT
E
α
,
P
α
:= (F
s
)
−1
P
α
,
E
τ
α
:=
τ
α
+ P
α
⊗E
α
, [(
∇P
w
)
E
w
]
i
:= P
w
j,i
E
w
j
.
(16)
Here,
E
τ
α
is symmetric and ensures that
τ
α
satisfies (11).
A balance equation for the entropy for the mixture as a whole is adopted,
together with the non-negativity of the internal entropy production,
X · ∂
∂t
(ρ
α
s
α
) +
∇·(ρ
α
s
α
v
α
)
− ∇·J
α
¸
=
X
σ
α
= σ
≥ 0
(17)
where s
α
, J
α
and σ
α
are, respectively, the entropy density, the entropy flux
and the entropy production associated with the constituent α . This relation will
yield certain restrictions on the constitutive forms of P
α
,
τ
α
, f
αβ
, U
α
, q
α
,
s
α
and J
α
. To this end, we will assume that the solid constituent behaves like
an elastic body and
{U
α
, s
α
} = {U
α
, s
α
}
³
P
α
, F
s
, ρ
w
, T
´
, α = s, w.
(18)
We do not include the porosity υ explicitly in the above relation on the basis that
the true mass density of water ρ
wR
is supposedly a function of T only. Note that
υ = ρ
w
/ρ
wR
.
Following Liu’s scheme [8] which treats the above balance equations as con-
straints that can be appended to (17), we have
0
≤ σ =
X½
ρ
α
µ
∂s
α
∂T
− Z
∂U
α
∂T
¶
d
α
T
dt
− ∇·{J
α
+ Z [q
α
+ P
α
E
α
·(v
α
− v
s
)]
}
¾
+
X ½·
Z
E
α
+ ρ
α
µ
∂s
α
∂
P
α
− Z
∂U
α
∂
P
α
¶¸
·
d
α
dt
P
α
¾
+Ztr
("
E
τ
s
+ P
s
·E
s
1 +
1
Z
F
s
X
ρ
α
µ
∂s
α
∂F
s
− Z
∂U
α
∂F
s
¶
T
#
L
s
)
+Ztr
½·
E
τ
w
+ P
w
·E
w
1
−
1
Z
ρ
w
X
ρ
α
µ
∂s
α
∂ρ
w
− Z
∂U
α
∂ρ
w
¶
1
¸
L
w
¾
+Z
"
f
sw
+ (
∇P
w
)
E
w
−
³
∇P
w
´
E
w
+
∇·(P
w
⊗E
w
)
+
1
Z
E
w
P
w
·∇Z +
1
Z
ρ
w
(
∇F
s
) :
µ
∂s
w
∂F
s
− Z
∂U
w
∂F
s
¶
T
−
1
Z
ρ
s
µ
∂s
s
∂ρ
w
− Z
∂U
s
∂ρ
w
¶
∇ρ
w
#
·(v
w
− v
s
) +
X
q
α
·∇Z.
(19)
Here Z is the Lagrange multiplier related to the balance of energy equation (15).
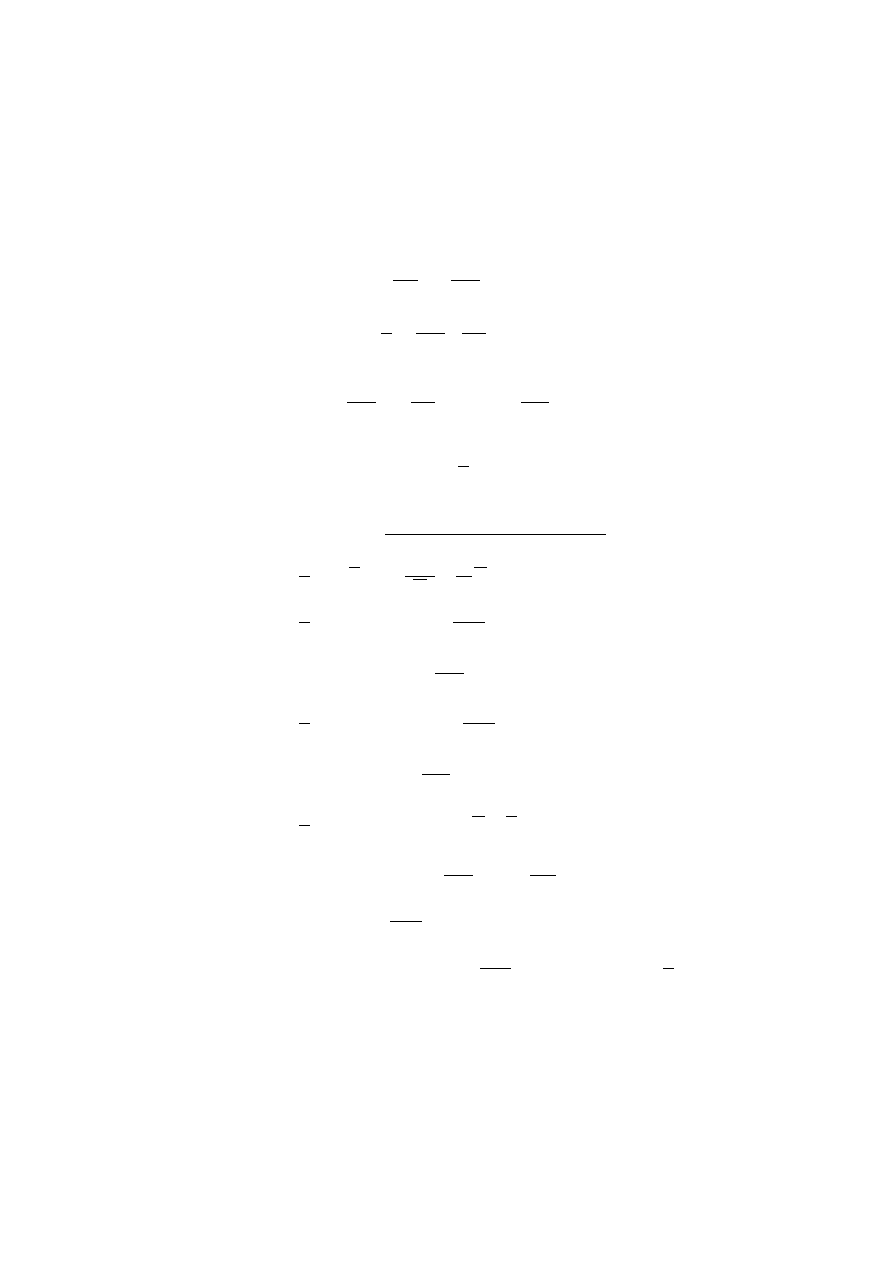
Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
931
Next, pick
∂s
α
∂T
− Z
∂U
α
∂T
= 0
or
1
Z
=
∂U
α
∂T
µ
∂s
α
∂T
¶
−1
.
Further, if the structures of U
α
and s
α
are such that
∂U
α
∂T
= T
∂s
α
∂T
, or s
α
=
−
∂A
α
∂T
,
(20)
with A
α
:= U
α
− T s
α
being the Helmholtz potential of constituent α , then,
Z =
1
T
,
(21)
and relation (19) can be recast as
0
≤ σ = −
X
∇·
·
J
α
+
q
α
+ ρ
α
N
α
u
α
+
E
α
·(v
α
− v
s
) P
α
T
¸
+
1
T
X µ
E
α
− ρ
α
∂A
α
∂
P
α
¶
·
d
α
dt
P
α
+
1
T
tr
("
E
τ
s
+ P
s
·E
s
1 +
ρ
s
ρ
w
ρ
(N
s
− N
w
)
−F
s
X
ρ
α
µ
∂A
α
∂F
s
¶
T
#
L
s
)
+
1
T
tr
("
E
τ
w
+ P
w
·E
w
1 +
ρ
w
ρ
s
ρ
(N
w
− N
s
)
+ρ
w
X
ρ
α
∂A
α
∂ρ
w
1
#
L
w
)
+
1
T
(
f
sw
+ (
∇P
w
)
E
w
−
³
∇P
w
´
E
w
+
∇·(P
w
⊗E
w
)
−ρ
w
(
∇F
s
) :
µ
∂A
w
∂F
s
¶
T
+ ρ
s
∂A
s
∂ρ
w
∇ρ
w
+
∇·
·
ρ
w
ρ
s
ρ
(N
w
− N
s
)
¸ )
·(v
w
− v
s
)
+
·X
q
α
+
E
w
·(v
w
−v
s
)P
w
+
ρ
w
ρ
s
ρ
(N
w
−N
s
)(v
w
−v
s
)
¸
·∇
1
T
. (22)
Here
ρ :=
X
ρ
α
, ρv :=
X
ρ
α
v
α
, u
α
:= v
α
− v,

932
K. R. Rajagopal and L. Tao
ZAMP
and N
α
is a second order tensor (with N
α
− N
β
being symmetric) to be deter-
mined that has the same unit as that of A
α
. We notice that there are other forms
to represent the entropy equation: For instance, we may adopt
P
α
= ρ
sR
/ρ
s
(F
s
)
−1
P
α
as in electromagnetic elastic solids, provided that corresponding mod-
ifications are made in the terms involving L
α
, v
α
− v
β
and so on in the above
equation. Certainly, these various forms are equivalent, but they may yield differ-
ent consequences in practice, as we may choose to meet these equivalent represen-
tations by different constitutive structures that are sufficient to meet the equivalent
forms. It is rather difficult to judge at this moment which form is better suited
to the problem under consideration, and we will proceed by adopting the form of
(22) and examine its consequences.
In what follows, we construct a model on the basis of the above relation. For
the entropy flux, we choose
J
α
=
−
q
α
+ ρ
α
N
α
u
α
+
E
α
·(v
α
− v
s
) P
α
T
, α = s, w,
(23)
to eliminate the divergence term from (22).
In the case of dielectric polarizations, we take (de Groot and Mazur [4])
d
α
dt
P
α
=
λ
α
µ
E
α
− ρ
α
∂A
α
∂
P
α
¶
, α = s, w,
(24)
where
λ
α
is positive definite and symmetric. It can be checked that this equa-
tion is Galilean invariant up to the order of c
−1
. Furthermore, we partition A
α
according to
A
α
= A
α
0
(C
s
, ρ
w
, T ) +
1
2
H
α
(C
s
, ρ
w
, T )
P
α
·P
α
, α = w, s,
(25)
where H
α
is positive definite and symmetric and C
s
:= F
sT
F
s
due to the re-
quirement of Galilean invariance on A
α
(Tao, Humphrey and Rajagopal [12]),
and we get
d
α
dt
P
α
=
λ
α
³
E
α
− ρ
α
H
α
P
α
´
, α = s, w.
(26)
We are able to determine H
α
and
λ
α
under the condition of small solid defor-
mations by resorting to the theory of dielectric polarizations in which P =
χ E
is employed under E = E
max
exp(iωt) (Hasted [6]; Hippel [7]). Here,
χ is the
complex electric susceptibility of a dielectric, (
χ is denoted as [re( χ )]−i[im( χ )]
with re(
χ ) and im( χ ) being real), ω is the frequency of the electromagnetic
wave field, and the material is at rest. Suppose that the susceptibility of the aque-
ous dielectric is obtained experimentally as
χ
M
=
χ
M
(ρ
s
, ρ
w
, T, ω) (some
data concerning susceptibility can be found in the book of Metaxas and Mered-
ith [9]), we may assume that P
s
=
χ
s
E with
χ
s
estimated by
χ
s
=
χ
M
(ρ
s
, 0, T, ω) and P
w
=
χ
w
E with
χ
w
=
χ
M
− χ
M
(ρ
s
, 0, T, ω) .
Substituting these expressions for P
s
and P
w
into (26), along with F
s
≈ 1 ,

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
933
v
α
= 0 and E = E
max
exp(iωt) results in, for α = s, w ,
λ
α
= ω [im (
χ
α
)] + ω [re (
χ
α
)] [im (
χ
α
)]
−1
[re (
χ
α
)] ,
(27)
H
α
=
ω
ρ
α
[
λ
α
]
−1
[re (
χ
α
)] [im (
χ
α
)]
−1
.
(28)
The Cauchy stresses, the internal momentum supply and the diffusive flux are
taken as
E
τ
s
=
−P
s
·E
s
1
−
ρ
s
ρ
w
ρ
(N
s
− N
w
) + F
s
X
ρ
α
µ
∂A
α
∂F
s
¶
T
,
E
τ
w
=
−P
w
·E
w
1
−
ρ
w
ρ
s
ρ
(N
w
− N
s
)
− ρ
w
X
ρ
α
∂A
α
∂ρ
w
1,
(29)
f
sw
=
− (∇P
w
)
E
w
+
³
∇P
w
´
E
w
− ∇·(P
w
⊗E
w
)+ρ
w
(
∇F
s
):
µ
∂A
w
∂F
s
¶
T
−ρ
s
∂A
s
∂ρ
w
∇ρ
w
−∇·
·
ρ
w
ρ
s
ρ
(N
w
− N
s
)
¸
+ b
sw
(v
w
− v
s
)+B
sw
∇T, (30)
and
X
q
α
=
−E
w
·(v
w
− v
s
) P
w
−
ρ
w
ρ
s
ρ
(N
w
− N
s
)(v
w
− v
s
)
−κ
sw
∇T + K
sw
(v
w
− v
s
) .
(31)
Here b
sw
is positive definite and symmetric, accounting for the effect of drag and
κ
sw
is the thermal conductivity of the mixture which is also positive definite and
symmetric. Both B
sw
and K
sw
are due to the cross effects associated with the
relative velocity and the temperature gradient.
With these constitutive relations, the entropy equation (22) reduces to
0
≤ σ =
1
T
X µ
E
α
− ρ
α
∂A
α
∂
P
α
¶
·λ
α
µ
E
α
− ρ
α
∂A
α
∂
P
α
¶
+
1
T
[b
sw
(v
w
− v
s
) + B
sw
∇T ]·(v
w
− v
s
)
+ [
−κ
sw
∇T + K
sw
(v
w
− v
s
)]
·∇
1
T
.
(32)
This inequality will impose restrictions on b
sw
, B
sw
,
κ
sw
and K
sw
. Certainly,
more comprehensive models can be suggested that include more cross effects.
2.2. Case 2: Mixture of a porous solid with gas
When the temperature of the aqueous dielectric is higher than the critical temper-
ature T
c
, there is a mixture of a porous solid with gas (dry steam). The balance
equations for constituents s and g and the mixture can be obtained as in the
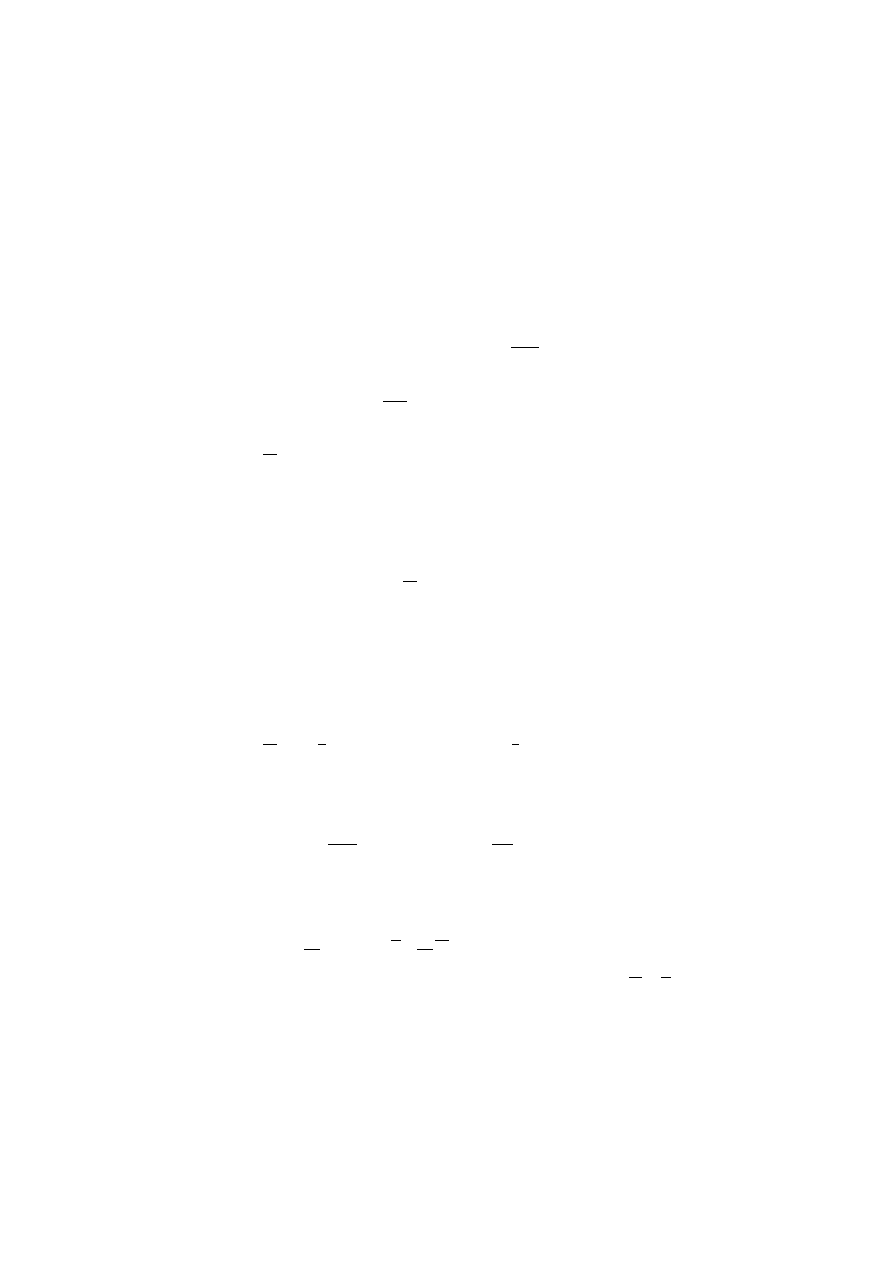
934
K. R. Rajagopal and L. Tao
ZAMP
above case of the mixture of a porous solid with water and they are recorded be-
low for the sake of completeness. One exception is that the porosity υ will be
included in formulating U
α
and s
α
since the true mass density of gas ρ
gR
is not
determined completely by T . Then,
ρ
s
detF
s
= ρ
sR
, F
s
:=
∂x
s
∂X
s
,
(33)
∂ρ
g
∂t
+
∇·(ρ
g
v
g
) = 0,
(34)
∂
∂t
(ρ
α
v
α
) +
∇·(v
α
ρ
α
v
α
) =
∇·τ
α
+ f
α
em
+
X
β6=α
f
αβ
+ ρ
α
g,
(35)
f
α
em
:= c
−1
"
c (
∇E)·P
α
+ (
∇B)·(P
α
×v
α
)
+
∂
∂t
(P
α
×B) + ∇·(v
α
P
α
×B)
#
,
(36)
f
sg
+ f
gs
= 0.
(37)
X h
τ
α
− (τ
α
)
T
i
=
X
(
E
α
⊗P
α
− P
α
⊗E
α
) ,
(38)
∂
∂t
·
ρ
α
µ
1
2
|v
α
|
2
+ U
α
¶¸
+
∇·
·
v
α
ρ
α
µ
1
2
|v
α
|
2
+ U
α
¶¸
=
∇·(τ
α
v
α
− q
α
) + h
α
em
+
X
β6=α
¡
f
αβ
·v
α
+ h
αβ
¢
+ ρ
α
g
·v
α
,
(39)
h
α
em
:=
∂P
α
∂t
·E − c
−1
(P
α
×v
α
)
·
∂B
∂t
+
∇·(v
α
P
α
·E) ,
(40)
f
sg
·v
s
+ h
sg
+ f
gs
·v
g
+ h
gs
= 0,
(41)
−
X
ρ
α
d
α
dt
U
α
+
X ½
E
α
·
d
α
dt
P
α
− ∇·[q
α
+ P
α
E
α
·(v
α
− v
s
)]
¾
+
X
tr [(
E
τ
α
+ P
α
·E
α
1) L
α
] +
h
f
sg
+ (
∇P
g
)
E
g
−
³
∇P
g
´
E
g
+
∇·(P
g
⊗ E
g
)
i
·(v
g
− v
s
) = 0,
(42)
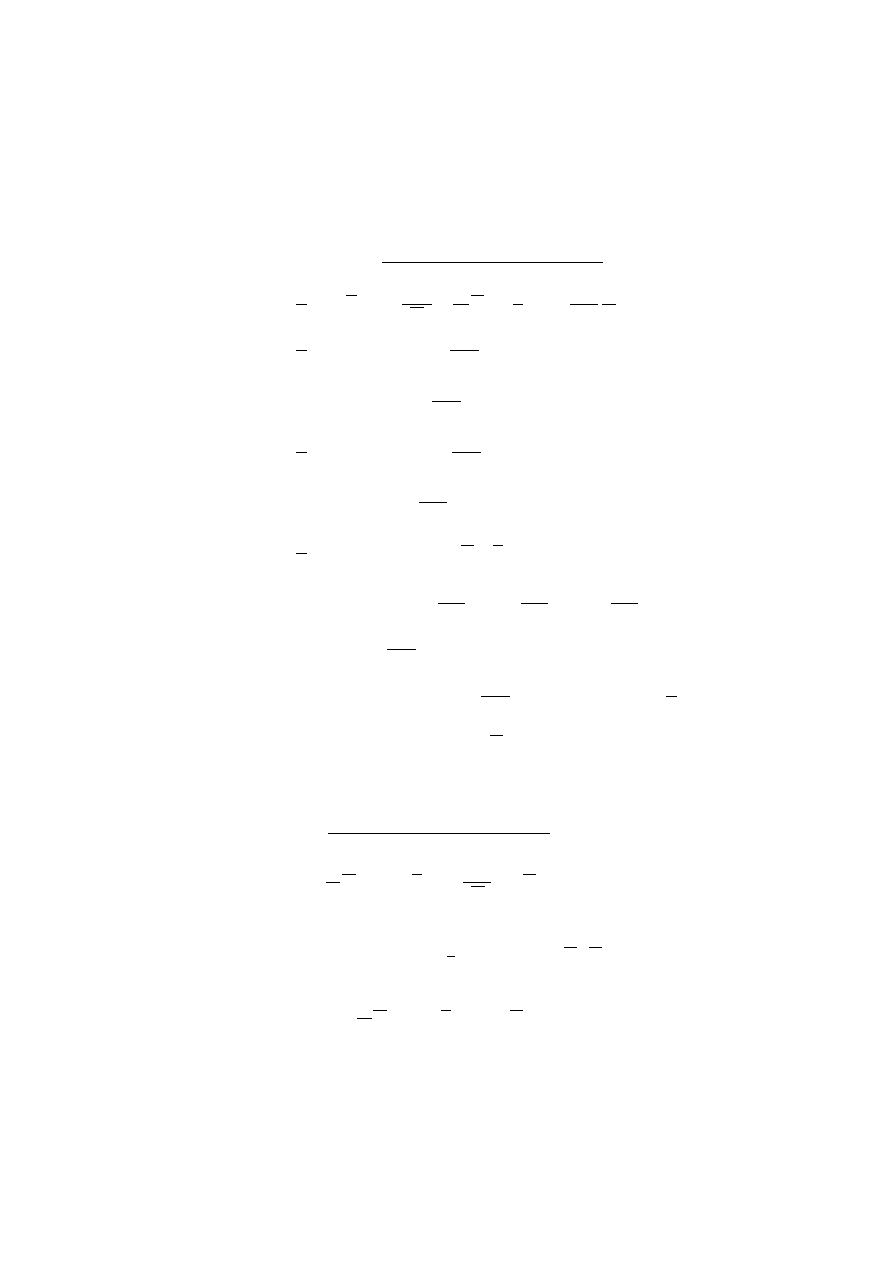
Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
935
0
≤ σ = −
X
∇·
·
J
α
+
q
α
+ ρ
α
N
α
u
α
+
E
α
·(v
α
− v
s
) P
α
T
¸
+
1
T
X µ
E
α
− ρ
α
∂A
α
∂
P
α
¶
·
d
α
dt
P
α
−
1
T
X
ρ
α
∂A
α
∂υ
d
s
dt
υ
+
1
T
tr
("
E
τ
s
+ P
s
·E
s
1 +
ρ
s
ρ
g
ρ
(N
s
− N
g
)
−F
s
X
ρ
α
µ
∂A
α
∂F
s
¶
T
#
L
s
)
+
1
T
tr
("
E
τ
g
+ P
g
·E
g
1 +
ρ
g
ρ
s
ρ
(N
g
− N
s
)
+ρ
g
X
ρ
α
∂A
α
∂ρ
g
1
#
L
g
)
+
1
T
(
f
sg
+ (
∇P
g
)
E
g
−
³
∇P
g
´
E
g
+
∇·(P
g
⊗E
g
)
−ρ
g
(
∇F
s
) :
µ
∂A
g
∂F
s
¶
T
+ ρ
s
∂A
s
∂ρ
g
∇ρ
g
− ρ
g
∂A
g
∂υ
∇υ
+
∇·
·
ρ
g
ρ
s
ρ
(N
g
− N
s
)
¸ )
·(v
g
− v
s
)
+
·X
q
α
+
E
g
·(v
g
− v
s
) P
g
+
ρ
g
ρ
s
ρ
(N
g
− N
s
)(v
g
− v
s
)
¸
·∇
1
T
, (43)
{U
α
, s
α
, A
α
} = {U
α
, s
α
, A
α
}
³
P
α
, F
s
, ρ
g
, υ, T
´
.
(44)
Based on relation (43), we choose the following model for the mixture of the
porous solid and gas. We take
J
α
=
−
q
α
+ ρ
α
N
α
u
α
+
E
α
·(v
α
− v
s
) P
α
T
, α = s, g,
(45)
d
s
dt
P
s
=
λ
s
µ
E
s
− ρ
s
∂A
s
∂
P
s
¶
,
P
g
= 0.
(46)
As indicated above, we partition A
s
in the form of
A
s
= A
s
0
(C
s
, ρ
g
, υ, T ) +
1
2
H
s
(C
s
, ρ
g
, υ, T )
P
s
·P
s
,
(47)
and we get
d
s
dt
P
s
=
λ
s
³
E
s
− ρ
s
H
s
P
s
´
.
(48)
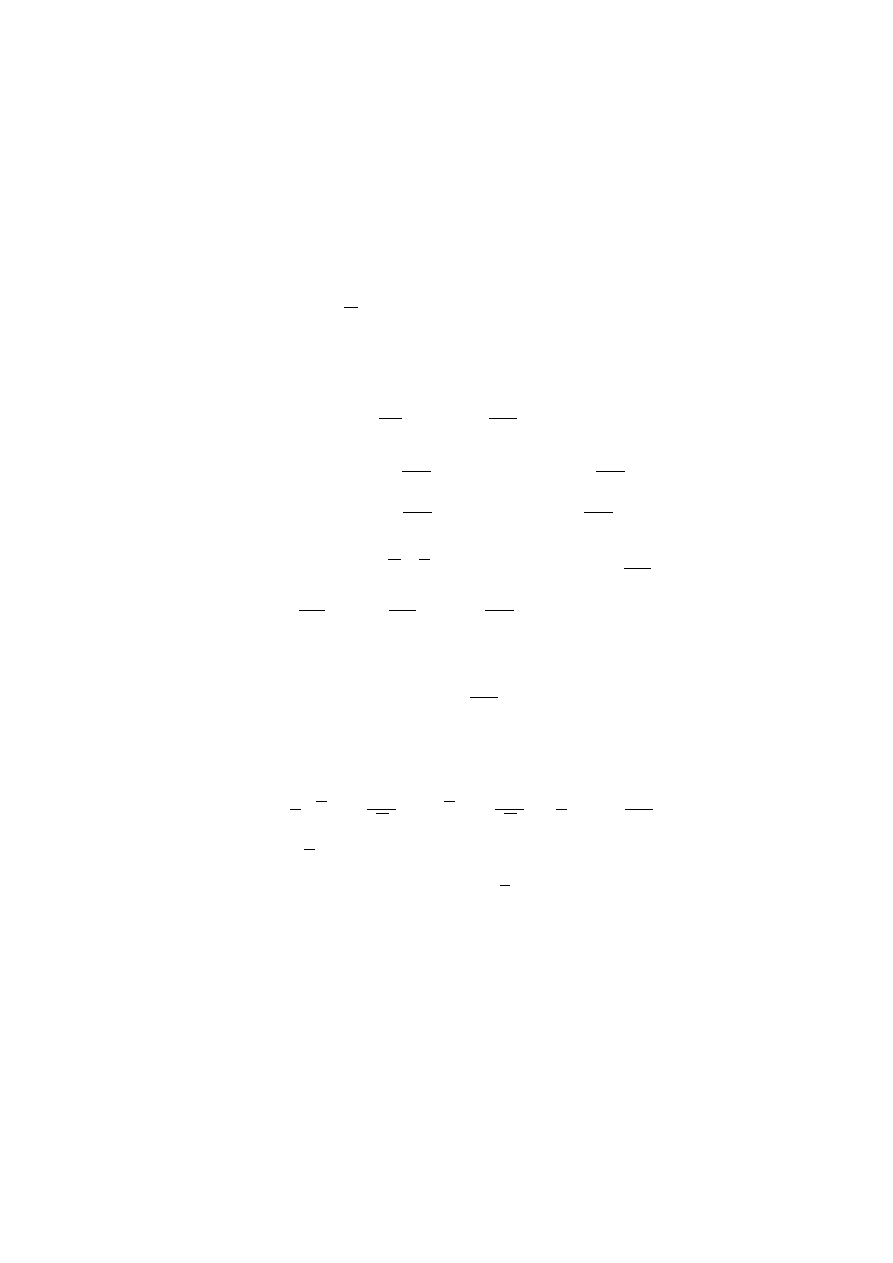
936
K. R. Rajagopal and L. Tao
ZAMP
We can estimate H
s
and
λ
s
in the same way as we did in Case 1,
λ
s
= ω [im (
χ
s
)] + ω [re (
χ
s
)] [im (
χ
s
)]
−1
[re (
χ
s
)] ,
H
s
=
ω
ρ
s
[
λ
s
]
−1
[re (
χ
s
)] [im (
χ
s
)]
−1
,
(49)
with
χ
s
=
χ
M
(ρ
s
, 0, T, ω) .
The evolution of porosity, the Cauchy stresses, the internal momentum supply
and the heat flux are taken to be
d
s
υ
dt
=
−γ
X
ρ
α
∂A
α
∂υ
,
(50)
E
τ
s
=
−P
s
·E
s
1
−
ρ
s
ρ
g
ρ
(N
s
− N
g
) + F
s
X
ρ
α
µ
∂A
α
∂F
s
¶
T
,
(51)
E
τ
g
=
−P
g
·E
g
1
−
ρ
g
ρ
s
ρ
(N
g
− N
s
)
− ρ
g
X
ρ
α
∂A
α
∂ρ
g
1,
(52)
f
sg
=
− (∇P
g
)
E
g
+
³
∇P
g
´
E
g
− ∇·(P
g
⊗E
g
) + ρ
g
(
∇F
s
):
µ
∂A
g
∂F
s
¶
T
−ρ
s
∂A
s
∂ρ
g
∇ρ
g
+ ρ
g
∂A
g
∂υ
∇υ − ∇·
·
ρ
g
ρ
s
ρ
(N
g
− N
s
)
¸
+b
sg
(v
g
− v
s
) + B
sg
∇T,
(53)
and
X
q
α
=
−E
g
·(v
g
− v
s
) P
g
−
ρ
g
ρ
s
ρ
(N
g
− N
s
)(v
g
− v
s
)
−κ
sg
∇T + K
sg
(v
g
− v
s
) ,
(54)
where γ
≥ 0 , b
sg
and
κ
sg
are positive definite and symmetric. The entropy
equation (43) reduces to
0
≤ σ =
1
T
µ
E
s
− ρ
s
∂A
s
∂
P
s
¶
·λ
s
µ
E
s
− ρ
s
∂A
s
∂
P
s
¶
+
γ
T
µX
ρ
α
∂A
α
∂υ
¶
2
+
1
T
[b
sg
(v
g
− v
s
) + B
sg
∇T ]·(v
g
− v
s
)
+ [
−κ
sg
∇T + K
sg
(v
g
− v
s
)]
·∇
1
T
.
(55)
This inequality will impose restrictions on b
sg
, B
sg
,
κ
sg
and K
sg
.
2.3. Case 3: Mixture of a porous solid with water and gas
Suppose that the temperature of the aqueous dielectric in some region reaches
the critical temperature T
c
due to the microwave heating, then water starts to

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
937
evaporate and gas (dry steam) comes into being. (It may also happen that the
gas condenses into water when the temperature drops.) There is now a mixture
of three constituents, the porous solid, the water and the gas, and the above
formulation needs to be extended to account for the mass conversion between the
water and the gas constituents due to the phase change. We will assume that the
constituents have the same temperature field T
c
which depends on the motion of
the mixture.
The balance equations for mass, linear momentum and angular momentum for
constituents s , w and g and the mixture as a whole are
ρ
s
detF
s
= ρ
sR
, F
s
:=
∂x
s
∂X
s
,
(56)
∂ρ
α
∂t
+
∇·(ρ
α
v
α
) = M
α
,
(57)
X
M
α
= 0, M
s
= 0,
(58)
where M
w
and M
g
characterize the mass conversion between the water and the
gas constituents, and
∂
∂t
(ρ
α
v
α
) +
∇·(v
α
ρ
α
v
α
) =
∇·τ
α
+ f
α
em
+
X
β6=α
f
αβ
+ M
α
v
α
+ ρ
α
g,
(59)
f
α
em
:= c
−1
"
c (
∇E)·P
α
+ (
∇B)·(P
α
×v
α
)
+
∂
∂t
(P
α
×B) + ∇·(v
α
P
α
×B)
#
,
(60)
f
sw
+ f
ws
= 0, f
sg
+ f
gs
= 0,
f
wg
+ M
w
v
w
+ f
gw
+ M
g
v
g
= 0,
(61)
X h
τ
α
− (τ
α
)
T
i
=
X
(
E
α
⊗P
α
− P
α
⊗E
α
) .
(62)
We have adopted three separate constraints in (61) to account for the interactions
between the three constituents and this might be relaxed by adopting only one
constraint for the mixture as a whole.
Since the temperature field of the mixture T
c
during the process of phase
change is supposedly known, the balance of energy for the mixture will be used to
determine the mass conversion rate M
g
(or M
w
). Let U
cα
denote the internal
938
K. R. Rajagopal and L. Tao
ZAMP
energy density of constituent α , we have, for α = s , w , g ,
∂
∂t
·
ρ
α
µ
1
2
|v
α
|
2
+ U
cα
¶¸
+
∇·
·
v
α
ρ
α
µ
1
2
|v
α
|
2
+ U
cα
¶¸
=
∇·(τ
α
v
α
− q
α
) + h
α
em
+ M
α
µ
U
cα
+
1
2
|v
α
|
2
¶
+
X
β6=α
¡
f
αβ
·v
α
+ h
αβ
¢
+ ρ
α
g
·v
α
,
(63)
h
α
em
:=
∂P
α
∂t
·E − c
−1
(P
α
×v
α
)
·
∂B
∂t
+
∇·(v
α
P
α
·E) ,
(64)
f
sw
·v
s
+ h
sw
+ f
ws
·v
w
+ h
ws
= 0, f
sg
·v
s
+ h
sg
+ f
gs
·v
g
+ h
gs
= 0,
f
wg
·v
w
+ h
wg
+ M
w
µ
U
cw
+
1
2
|v
w
|
2
¶
+f
gw
·v
g
+ h
gw
+ M
g
µ
U
cg
+
1
2
|v
g
|
2
¶
= 0.
(65)
Assuming that
U
cα
= U
cα
³
P
α
, F
s
, ρ
w
, ρ
g
, υ, T
c
´
, α = s, w, g,
(66)
summarizing equation (63) and eliminating the kinetic energy terms with the help
of (59), we obtain the expression for the mass conversion rate
M
g
=
·
1
2
|v
w
− v
g
|
2
+ U
cg
− U
cw
+
X
ρ
α
µ
∂U
cα
∂ρ
g
−
∂U
cα
∂ρ
w
¶¸
−1
×
(
X
"
− ρ
α
∂U
cα
∂T
c
d
α
T
c
dt
− ∇·[q
α
+ P
α
E
α
·(v
α
− v
s
)]
+
µ
E
α
− ρ
α
∂U
cα
∂
P
α
¶
·
d
α
dt
P
α
− ρ
α
∂U
cα
∂υ
d
α
υ
dt
#
+tr
"Ã
E
τ
s
+ P
s
·E
s
1
− F
s
X
ρ
α
µ
∂U
cα
∂F
s
¶
T
!
L
s
#
+tr
·µ
E
τ
w
+ P
w
·E
w
1 + ρ
w
X
ρ
α
∂U
cα
∂ρ
w
1
¶
L
w
¸
+tr
·µ
E
τ
g
+ P
g
·E
g
1 + ρ
g
X
ρ
α
∂U
cα
∂ρ
g
1
¶
L
g
¸
+
"
f
sw
+ (
∇P
w
)
E
w
−
³
∇P
w
´
E
w
+
∇·(P
w
⊗E
w
)
−ρ
w
(
∇F
s
):
µ
∂U
cw
∂F
s
¶
T
+ ρ
s
∂U
cs
∂ρ
w
∇ρ
w
#
·(v
w
− v
s
)
Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
939
+
"
f
sg
+ (
∇P
g
)
E
g
−
³
∇P
g
´
E
g
+
∇·(P
g
⊗E
g
)
−ρ
g
(
∇F
s
):
µ
∂U
cg
∂F
s
¶
T
+ ρ
s
∂U
cs
∂ρ
g
∇ρ
g
#
·(v
g
− v
s
)
+
"
f
gw
− ρ
w
∂U
cw
∂ρ
g
∇ρ
g
+ ρ
g
∂U
cg
∂ρ
w
∇ρ
w
#
·(v
w
− v
g
)
)
.
(67)
We adopt the following entropy equation for the mixture
0
≤ σ =
X · ∂
∂t
(ρ
α
s
cα
) +
∇·(ρ
α
s
cα
v
α
)
− ∇·J
α
¸
=
X µ
ρ
α
d
α
dt
s
cα
− ∇·J
α
¶
+ M
g
(s
cg
− s
cw
) ,
(68)
where s
cα
, J
α
are, respectively, the entropy density and the entropy flux associ-
ated with constituent α . Substituting (67) into the above equation and assuming
that
s
cα
= s
cα
³
P
α
, F
s
, ρ
w
, ρ
g
, υ, T
c
´
, α = s, w, g,
(69)
results in
0
≤ σ =
X½
ρ
α
µ
∂s
cα
∂T
c
−
1
Γ
∂U
cα
∂T
c
¶
d
α
T
c
dt
− ∇·
·
J
α
+
q
α
+ P
α
E
α
·(v
α
− v
s
)
Γ
¸¾
+
1
Γ
X ·
E
α
+ ρ
α
µ
Γ
∂s
cα
∂
P
α
−
∂U
cα
∂
P
α
¶¸
·
d
α
dt
P
α
+
1
Γ
X
ρ
α
µ
Γ
∂s
cα
∂υ
−
∂U
cα
∂υ
¶
d
α
υ
dt
+
1
Γ
tr
("
E
τ
s
+ P
s
·E
s
1 + F
s
X
ρ
α
µ
Γ
∂s
cα
∂F
s
−
∂U
cα
∂F
s
¶
T
#
L
s
)
+
1
Γ
tr
½·
E
τ
w
+ P
w
·E
w
1
− ρ
w
X
ρ
α
µ
Γ
∂s
cα
∂ρ
w
−
∂U
cα
∂ρ
w
¶
1
¸
L
w
¾
+
1
Γ
tr
½·
E
τ
g
+ P
g
·E
g
1
− ρ
g
X
ρ
α
µ
Γ
∂s
cα
∂ρ
g
−
∂U
cα
∂ρ
g
¶
1
¸
L
g
¾
+
1
Γ
"
f
sw
+ (
∇P
w
)
E
w
−
³
∇P
w
´
E
w
+
∇·(P
w
⊗E
w
)
−ρ
s
µ
Γ
∂s
cs
∂ρ
w
−
∂U
cs
∂ρ
w
¶
∇ρ
w
+ρ
w
(
∇F
s
) :
µ
Γ
∂s
cw
∂F
s
−
∂U
cw
∂F
s
¶
T
940
K. R. Rajagopal and L. Tao
ZAMP
+Γ
E
w
P
w
·∇
1
Γ
#
·(v
w
− v
s
)
+
1
Γ
"
f
sg
+ (
∇P
g
)
E
g
−
³
∇P
g
´
E
g
+
∇·(P
g
⊗E
g
)
−ρ
s
µ
Γ
∂s
cs
∂ρ
g
−
∂U
cs
∂ρ
g
¶
∇ρ
g
+ ρ
g
(
∇F
s
) :
µ
Γ
∂s
cg
∂F
s
−
∂U
cg
∂F
s
¶
T
+Γ
E
g
P
g
·∇
1
Γ
#
·(v
g
− v
s
)
+
1
Γ
"
f
gw
+ ρ
w
µ
Γ
∂s
cw
∂ρ
g
−
∂U
cw
∂ρ
g
¶
∇ρ
g
−ρ
g
µ
Γ
∂s
cg
∂ρ
w
−
∂U
cg
∂ρ
w
¶
∇ρ
w
#
·(v
w
− v
g
) +
X
q
α
·∇
1
Γ
,
(70)
where
Γ :=
·
s
cg
− s
cw
+
X
ρ
α
µ
∂s
cα
∂ρ
g
−
∂s
cα
∂ρ
w
¶¸
−1
·
1
2
|v
w
− v
g
|
2
+U
cg
− U
cw
+
X
ρ
α
µ
∂U
cα
∂ρ
g
−
∂U
cα
∂ρ
w
¶¸
.
(71)
To construct a model that satisfies the above relation, we take
∂s
cα
∂T
c
−
1
Γ
∂U
cα
∂T
c
= 0,
and assume that the structures of s
cα
and U
cα
are such that
∂U
cα
∂T
c
= T
c
∂s
cα
∂T
c
, or s
cα
=
−
∂A
cα
∂T
c
,
(72)
with A
cα
:= U
cα
− T
c
s
cα
being the Helmholtz potential associated with con-
stituent α . Then we have Γ = T
c
,
0
≤ σ = −
X
∇·
·
J
α
+
q
α
+ρ
α
N
α
u
α
+
E
α
·(v
α
− v
s
) P
α
T
c
¸
+
1
T
c
X µ
E
α
− ρ
α
∂A
cα
∂
P
α
¶
·
d
α
dt
P
α
−
1
T
c
X
ρ
α
∂A
cα
∂υ
d
s
υ
dt
+
1
T
c
tr
("
E
τ
s
+ P
s
·E
s
1 +
ρ
s
ρ
[ρ
w
(N
s
− N
w
) + ρ
g
(N
s
− N
g
)]
−F
s
X
ρ
α
µ
∂A
cα
∂F
s
¶
T
#
L
s
)

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
941
+
1
T
c
tr
("
E
τ
w
+ P
w
·E
w
1 +
ρ
w
ρ
[ρ
s
(N
w
− N
s
) + ρ
g
(N
w
− N
g
)]
+ρ
w
X
ρ
α
∂A
cα
∂ρ
w
1
#
L
w
)
+
1
T
c
tr
("
E
τ
g
+ P
g
·E
g
1 +
ρ
g
ρ
[ρ
s
(N
g
− N
s
) + ρ
w
(N
g
− N
w
)]
+ρ
g
X
ρ
α
∂A
cα
∂ρ
g
1
#
L
g
)
+
1
T
c
(
f
sw
+ (
∇P
w
)
E
w
−
³
∇P
w
´
E
w
+
∇·(P
w
⊗E
w
)
+ρ
s
∂A
cs
∂ρ
w
∇ρ
w
− ρ
w
(
∇F
s
):
µ
∂A
cw
∂F
s
¶
T
− ρ
w
∂A
cw
∂υ
∇υ
+
∇·
·
ρ
w
ρ
s
ρ
(N
w
− N
s
)
¸ )
·(v
w
− v
s
)
+
1
T
c
(
f
sg
+ (
∇P
g
)
E
g
−
³
∇P
g
´
E
g
+
∇·(P
g
⊗E
g
)
+ρ
s
∂A
cs
∂ρ
g
∇ρ
g
− ρ
g
(
∇F
s
):
µ
∂A
cg
∂F
s
¶
T
− ρ
g
∂A
cg
∂υ
∇υ
+
∇·
·
ρ
g
ρ
s
ρ
(N
g
− N
s
)
¸ )
·(v
g
− v
s
)
+
1
T
c
(
f
gw
− ρ
w
∂A
cw
∂ρ
g
∇ρ
g
+ ρ
g
∂A
cg
∂ρ
w
∇ρ
w
+
∇·
·
ρ
w
ρ
g
ρ
(N
w
− N
g
)
¸ )
·(v
w
− v
g
)
+
"
X
q
α
+
E
w
·(v
w
− v
s
) P
w
+
E
g
·(v
g
− v
s
) P
g
+
ρ
w
ρ
s
ρ
(N
w
− N
s
)(v
w
− v
s
)
+
ρ
g
ρ
s
ρ
(N
g
− N
s
)(v
g
− v
s
)
+
ρ
w
ρ
g
ρ
(N
w
− N
g
)(v
w
− v
g
)
#
·∇
1
T
c
,
(73)
942
K. R. Rajagopal and L. Tao
ZAMP
and
T
c
=
·
s
cg
− s
cw
+
X
ρ
α
µ
∂s
cα
∂ρ
g
−
∂s
cα
∂ρ
w
¶¸
−1
·
1
2
|v
w
− v
g
|
2
+U
cg
− U
cw
+
X
ρ
α
µ
∂U
cα
∂ρ
g
−
∂U
cα
∂ρ
w
¶¸
.
(74)
On the basis of (73), we will simply choose, for α = s, w, g ,
J
α
=
−
q
α
+ ρ
α
N
α
u
α
+
E
α
·(v
α
− v
s
) P
α
T
c
,
(75)
P
g
= 0,
(76)
d
s
dt
P
s
=
λ
s
µ
E
s
− ρ
s
∂A
cs
∂
P
s
¶
,
(77)
d
w
dt
P
w
=
λ
w
µ
E
w
− ρ
w
∂A
cw
∂
P
w
¶
,
(78)
d
s
υ
dt
=
−γ
X
ρ
α
∂A
cα
∂υ
,
(79)
E
τ
s
=
−P
s
·E
s
1
−
ρ
s
ρ
[ρ
w
(N
s
− N
w
) + ρ
g
(N
s
− N
g
)]
#
+F
s
X
ρ
α
µ
∂A
cα
∂F
s
¶
T
,
(80)
E
τ
w
=
−P
w
·E
w
1
−
ρ
w
ρ
[ρ
s
(N
w
− N
s
) + ρ
g
(N
w
− N
g
)]
−ρ
w
X
ρ
α
∂A
cα
∂ρ
w
1,
(81)
E
τ
g
=
−P
g
·E
g
1
−
ρ
g
ρ
[ρ
s
(N
g
− N
s
) + ρ
w
(N
g
− N
w
)]
−ρ
g
X
ρ
α
∂A
cα
∂ρ
g
1,
(82)
f
sw
=
− (∇P
w
)
E
w
+
³
∇P
w
´
E
w
− ∇·(P
w
⊗E
w
)
− ρ
s
∂A
cs
∂ρ
w
∇ρ
w
+ρ
w
(
∇F
s
) :
µ
∂A
cw
∂F
s
¶
T
+ ρ
w
∂A
cw
∂υ
∇υ − ∇·
·
ρ
w
ρ
s
ρ
(N
w
− N
s
)
¸
+b
sw
(v
w
− v
s
) + B
sw
∇T
c
,
(83)
f
sg
=
− (∇P
g
)
E
g
+
³
∇P
g
´
E
g
− ∇·(P
g
⊗E
g
)
− ρ
s
∂A
cs
∂ρ
g
∇ρ
g
Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
943
+ρ
g
(
∇F
s
) :
µ
∂A
cg
∂F
s
¶
T
+ ρ
g
∂A
cg
∂υ
∇υ − ∇·
·
ρ
g
ρ
s
ρ
(N
g
− N
s
)
¸
+b
sg
(v
g
− v
s
) + B
sg
∇T
c
,
(84)
f
gw
= ρ
w
∂A
cw
∂ρ
g
∇ρ
g
− ρ
g
∂A
cg
∂ρ
w
∇ρ
w
− ∇·
·
ρ
w
ρ
g
ρ
(N
w
− N
g
)
¸
+b
gw
(v
w
− v
g
) + B
gw
∇T
c
,
(85)
X
q
α
=
−E
w
·(v
w
− v
s
) P
w
− E
g
·(v
g
− v
s
) P
g
−
ρ
w
ρ
s
ρ
(N
w
− N
s
)(v
w
− v
s
)
−
ρ
g
ρ
s
ρ
(N
g
− N
s
)(v
g
− v
s
)
−
ρ
w
ρ
g
ρ
(N
w
− N
g
)(v
w
− v
g
)
− κ
swg
∇T
c
+K
sw
(v
w
− v
s
) + K
sg
(v
g
− v
s
) + K
gw
(v
w
− v
g
) .
(86)
Then, equation (73) reduces to
0
≤ σ =
1
T
c
X
α=s,w
λ
α
µ
E
α
−ρ
α
∂A
cα
∂
P
α
¶
·
µ
E
α
−ρ
α
∂A
cα
∂
P
α
¶
+
γ
T
c
µX
ρ
α
∂A
cα
∂υ
¶
2
+
1
T
c
[b
sw
(v
w
− v
s
) + B
sw
∇T
c
]
·(v
w
− v
s
)
+
1
T
c
[b
sg
(v
g
− v
s
) + B
sg
∇T
c
]
·(v
g
− v
s
)
+
1
T
c
[b
gw
(v
w
− v
g
) + B
gw
∇T
c
]
·(v
w
− v
g
)
+ [
−κ
swg
∇T
c
+ K
sw
(v
w
− v
s
) + K
sg
(v
g
− v
s
)
+K
gw
(v
w
− v
g
)]
·∇
1
T
c
,
(87)
which will impose restrictions on the coefficients.
As in the case of the mixture of s and w , we will adopt
A
cα
= A
cα
0
(C
s
, ρ
w
, ρ
g
, υ, T
c
) +
1
2
H
cα
(C
s
, ρ
w
, ρ
g
, υ, T
c
)
P
α
·P
α
, α = s, w, (88)
then, equations (77) and (78) become
d
α
dt
P
α
=
λ
α
³
E
α
− ρ
α
H
cα
P
α
´
, α = s, w.
(89)
Ignoring the polarization of the gas constituent, we may also estimate H
cα
and
λ
α
as above, by taking
χ
M
=
χ
M
(ρ
s
, ρ
w
, T
c
, ω) ,
χ
s
=
χ
M
(ρ
s
, 0, T
c
, ω)
and
χ
w
=
χ
M
− χ
M
(ρ
s
, 0, T
c
, ω) ,
λ
α
= ω [im (
χ
α
)] + ω [re (
χ
α
)] [im (
χ
α
)]
−1
[re (
χ
α
)] ,
H
cα
=
ω
ρ
α
[
λ
α
]
−1
[re (
χ
α
)] [im (
χ
α
)]
−1
.
(90)

944
K. R. Rajagopal and L. Tao
ZAMP
3. Interfacial jump conditions
To have a determinate model of the microwave drying process of an aqueous di-
electric body, we need to furnish the interfacial jump conditions. These interfaces
include the boundary of the body (relative to its environment) and the bound-
ary between any two mixture regions of
{s, w} , {s, w, g} and {s, g} inside the
body. Certainly, we notice the fuzzy nature related to the latter boundaries, and
we will idealize them as singular surfaces without any surface property attached
to them for the sake of simplicity. The jump conditions are obtained by following
the scheme illustrated in [2] and [14]. We may attach the velocity of the solid
constituent v
s
to the fields of E and B in deriving these conditions, though it
is not essential.
Let
S(t) be an interface with normal n and move with the velocity of ν and
let us define
φ
:= φ
+
− φ
−
.
(91)
Here φ
+
and φ
−
are the values of φ from positive and negative sides of n of
S(t) . Then, the jump conditions associated with the Maxwell field equations (1)
through (4) are
E +
X
P
α
·n = 0,
(92)
c
−1
³
E +
X
P
α
´
×ν + B + c
−1
X
v
α
×P
α
×n = 0,
(93)
B
·n = 0,
(94)
E − c
−1
B
×ν
×n = 0.
(95)
The balance of mass for the mixtures yields
X
ρ
α
(v
α
− ν)
·n = 0.
(96)
To derive the jump conditions associated with the balances of linear momenta and
energy for the mixtures, we rewrite the balance equations, with the help of (1) to
(4), in the forms
∂
∂t
³X
ρ
α
v
α
+ c
−1
E
×B
´
=
∇·
"
X
(
−v
α
ρ
α
v
α
+
τ
α
+ P
α
⊗E
α
)
−
1
2
³
|E|
2
+
|B|
2
´
1 + E
⊗E + B⊗B
#
+
X
ρ
α
g,
(97)
∂
∂t
"
X
ρ
α
µ
1
2
|v
α
|
2
+ U
α
¶
+
1
2
³
|E|
2
+
|B|
2
´ #
=
∇·
(
X
"
− v
α
ρ
α
µ
1
2
|v
α
|
2
+ U
α
¶
+
τ
α
v
α
− q
α
+ v
α
P
α
·E
#

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
945
− cE×
³
B
− c
−1
X
P
α
×v
α
´ )
+
X
ρ
α
v
α
·g.
(98)
Correspondingly, we will get the following jump conditions
X h
ρ
α
v
α
⊗(v
α
− ν) − (τ
α
)
T
− E
α
⊗P
α
i
− c
−1
(E
×B)⊗ν
+
1
2
³
|E|
2
+
|B|
2
´
1
− E⊗E − B⊗B
n = 0,
(99)
X ·
ρ
α
µ
1
2
|v
α
|
2
+ U
α
¶
(v
α
− ν) − τ
α
v
α
+ q
α
− P
α
v
α
·E
¸
−
1
2
³
|E|
2
+
|B|
2
´
ν + cE×B
·n = 0.
(100)
Several more sets of interfacial jump conditions can be generated. One results
from the continuity of displacement w
s
(:= x
s
− X
s
) and the balance of mass
for the solid constituent
w
s
= 0,
(101)
ρ
s
(v
s
− ν)
·n = 0.
(102)
However, we may not have the freedom to obtain similar jump conditions asso-
ciated with the balances of linear momenta and energy for the solid constituent
due to the problem associated with identifying the momentum and energy of the
electromagnetic field related to the solid constituent and the ambiguity of whether
to decompose some quantities into surface flux terms or source terms. The relation
(102) is met automatically when the interface is a part of the boundary ∂D of the
aqueous dielectric body since
ν = v
s
|
∂D
on one side and ρ
s
= 0 on the other
side. Also, the requirement can be imposed that the temperature is continuous
across the interfaces between the region of
{s, w} and the region of {s, w, g} and
between
{s, w, g} and {s, g} and this temperature equals T
c
. The other set of
conditions follows from the entropy equation for the mixture as a whole [12],
X
ρ
α
s
α
(v
α
− ν) −
X
J
α
·n = 0,
(103)
which is consistent with the assumption that the interface does not possess intrinsic
surface properties of its own, like surface mass density, surface entropy and so on.
4. Summary
We have presented, within the context of Maxwell-Lorentz field equations and mix-
ture theory, the electromagnetic field equations and the equations of motion for the

946
K. R. Rajagopal and L. Tao
ZAMP
porous solid, water and gas in an aqueous dielectric corresponding to the process
of microwave drying. Three mixtures are considered: mixture of a porous solid
and water; mixture of a porous solid, water and gas; and mixture of a porous solid
and gas. On the basis of continuum thermodynamics, constitutive relations are
proposed for the macroscopic electric polarization vectors, Cauchy stresses, heat
fluxes, internal momentum supplies and so on to characterize the thermomechan-
ical behaviors of these mixtures. The interfacial jump conditions between these
different mixture regions were derived. This framework is tentative at best, some
revisions might be needed upon further studies, and much more needs to be done
with regard to fixing the specific forms of the constitutive relations based on ex-
perimental data and by solving specific drying problems. The appendix discusses
briefly an issue related to the requirement of material frame indifference.
Appendix
Though a subject of controversy (Edelen and McLennan [1]; Wang [15]), the re-
quirement of material frame indifference (MFI) plays a major role in constitutive
theory (Noll [10]; Oldroyd [11]; Truesdell and Toupin [14]). Part of the disagree-
ment lies in whether MFI should be adopted or a less restrictive requirement, the
principle of Galilean invariance, should be used to formulate constitutive relations.
Confusion has also been caused in averaged turbulence modeling and mixture the-
ory, wherein the situation is worse in that there seems no sharp criterion to classify
some quantities as material properties, due to the averaged fields involving large
spatial and temporal scales. In this appendix, we examine a feature of MFI briefly
from a physical perspective, a perspective related to observers’ measuring of length
and time interval necessary in obtaining constitutive relations, not merely talking
about these material properties in a purely abstract and conceptual sense. And
the intended purpose is to provide some justification for our adoption of Galilean
invariance in this work.
Let
{x, t} be an inertial frame, and let a body in motion be represented in
this frame. Consider another frame x ,
x = Q(t)x + a(t), QQ
T
= Q
T
Q = 1
and assume that an observer is located at x
0
in the frame of x . We have to
restrict x
0
, Q and a such that
¯¯
T
(x
0
− a) + ˙a
¯¯
¯ < λc
(104)
where c is the speed of light in vacuum and λ << 1 . This is essential in order
that we may neglect relativistic effects. Otherwise, the observer’s measuring scales
of length and time would differ nontrivially from that of the observer’s located in
the frame of x , and we have to go beyond the framework of Newtonian mechanics
which is needed to establish the corresponding relations between the quantities

Vol. 53 (2002)
Modeling of the microwave drying process of aqueous dielectrics
947
associated with these two frames. Hence, there is the constraint that ˙
Q , a , ˙a
and x
0
have to meet.
We now take a = C (t + t
0
) with C and t
0
being constant in order to
simplify the analysis and we have
¯¯
T
(x
0
− C (t + t
0
)) + C
¯¯
¯ < λc.
(105)
The independence between Q , x
0
and C which is necessary for applying MFI
to formulate constitutive relations results in
|C| < λc
(106)
by picking ˙
Q = 0 ;
¯¯
T
x
0
¯¯
¯ < λc
(107)
by picking C = 0 ; and
¯¯
T
C (t + t
0
)
¯¯
¯ < 3λc
(108)
from (105) to (107) and the triangle inequality. Furthermore, as a proper estima-
tion, we can infer from (106) and (108) that
¯¯
T
¯¯
¯ |t + t
0
| ≤ 3
√
2.
(109)
That is, the product of the rotating speed
¯¯
T
/
√
2
¯¯
¯ and time t+t
0
is bounded
above. Specifically, if we choose t
0
= 12 (hours) (or both t
0
= 0 and
¯¯
T
¯¯
¯
constant) and t
∈ [0, 12] (hours), the rotating speed will be smaller than the spin
of Earth. This implies essentially that Q should be treated as constant or the
frame x should be approximated as not rotating, because we neglect the effect of
Earth’s spin in measuring material properties in most cases.
Acknowledgement
We thank the National Science Foundation and the National Institutes for Health
for support of this work.
References
[1] D. G. B. Edelen and J. A. McLennan, Material indifference: a principle or a convenience,
Int. J. Engng. Sci. 11 (1973), 813-817.
[2] A. C. Eringen, Mechanics of Continua. Wiley, New York, 1980.
[3] A. C. Eringen and G. A. Maugin, Electrodynamics of Continua I. Springer-Verlag, New
York, 1989.
[4] S. R. de Groot and P. Mazur, Non-Equilibrium Thermodynamics. Dover Publications, Inc.,
New York, 1984.
948
K. R. Rajagopal and L. Tao
ZAMP
[5] S. R. de Groot and L. G. Suttorp, Foundations of Electrodynamics. North-Holland Publish-
ing Co., Amsterdam, 1972.
[6] J. B. Hasted, Aqueous Dielectric. Chapman and Hall, London, 1973.
[7] A. R. von Hippel, Dielectrics and Waves. The MIT Press, Cambridge, Massachusetts, 1954.
[8] I-Shin Liu, Method of Lagrange multipliers for exploitation of the entropy principle, Arch.
Rat. Mech. Anal., 46 (1972), 131-148.
[9] A. C. Metaxas and R. J. Meredith, Industrial Microwave Heating. Peter Peregrinus Ltd,
London, 1983.
[10] W. Noll, On the continuity of the solid and fluid state, J. Ratl. Mech. Anal. 4 (1955), 13-81.
[11] J. G. Oldroyd, On the formulation of rheological equations of state, Proc. Roy. Soc. A200
(1950), 523-541.
[12] L. Tao, L., J. D. Humphrey and K. R. Rajagopal, A mixture theory for heat-induced alter-
ations in hydration and mechanical properties in soft tissues, Int. J. Engng. Sci. 39 (2001),
1535-1556.
[13] C. Truesdell, Rational Thermodynamics. Springer-Verlag, New York, 1984.
[14] C. Truesdell and R. Toupin, The Classical Field Theories. In Handbuch der Physik Vol.
III/1, W. Flugge, ed, Springer-Verlag, New York, 1960.
[15] C. C. Wang, On the concept of frame-indifference in continuum mechanics and in the kinetic
theory of gases, Arch. Ratl. Mech. Anal. 58 (1975), 381-393.
[16] S. Whitaker, Coupled transport in multiphase systems: A theory of drying. In Advances in
Heat Transfer, Vol. 31, 1-104. Academic Press, New York, 1998.
K. R. Rajagopal and L. Tao
Department of Mechanical Engineering
Texas A&M University
College Station, Texas 77843-3123
USA
e-mail: Krajagopal@mengr.tamu.edu
To access this journal online:
http://www.birkhauser.ch
Wyszukiwarka
Podobne podstrony:
Modeling with shrinkage during the vacuum drying of carrot (daucus carota) (Arévalo Pinedo, Xidieh M
Microwave drying characteristics of potato and the effect of different microwave powers on the dried
Modeling of Polymer Processing and Properties
Anatomy Based Modeling of the Human Musculature
Drying, shrinkage and rehydration characteristics of kiwifruits during hot air and microwave drying
Modeling Of The Wind Turbine With A Doubly Fed Induction Generator For Grid Integration Studies
Far infrared and microwave drying of peach (Jun Wang, Kuichuan Sheng)
Microwave–vacuum drying kinetics of carrot slices (Zheng Wei Cui, Shi Ying Xu, Da Wen Sun)
Modeling of Polymer Processing and Properties
Modelling of dehydration rehydration of orange slices in combined microwaveair drying
Additives for the Manufacture and Processing of Polymers dodatki do polimerów tworzyw sztucznych
Effect of vacuum microwave drying on selected mechanical and rheological properties of carrot
Microwave Drying of Parsley Modelling, Kinetics, and Energy Aspects
MODELING OF THE ACOUSTO ELECTROMAGNETIC METHOD FOR IONOSPHERE MONITORING EP 32(0275)
eBook Anatomy Based Modeling of the Human Musculature
Preparation of dry honey by microwave–vacuum drying
Microwave drying characteristics of spinach
więcej podobnych podstron