Influence of cyclic loading on the degradation
of mechanical

concrete properties

Rolf Breitenbücher, Hursit Ibuk, Hussein Alawieh

Ruhr-University of Bochum, Institute for Building Materials, Germany

Structures like bridges, concrete pavements, cooling- and wind towers are exposed
beside damaging environmental influences to cyclic mechanical loading scenarios.
Therefore, the reliability of such structures depends among other influences on the
degree of structural damage due to fatigue loading. In order to estimate the state of
a structure it is necessary to know the development of the crucial material proper-
ties during its service lifetime.

A basis for creating a lifetime-oriented design concept it is sufficient to con-

sider not only a general final failure, but also the information about the continuous
changes in the relevant material properties of a structure. Thus, within the frame-
work of the current Collaborative Research Center 398 (SFB 398) the purpose is
to investigate experimentally the degradation of material properties like compres-
sive strength, stiffness and fracture energy of plain concrete due to uniaxial cyclic
compression loading. The results of this investigation should validate approaches
of already developed material models. Various single-stage cyclic compression
tests were carried out with constant values for the lower stress level (10 percent of
the compressive strength) respectively 3 different upper stress levels between 60
and 70 percent of the compressive strength.

At the examined stress levels the development of fatigue damage obtained in

terms of drop of stiffness appears to be mainly governed by the evolution of the
fatigue strain.

By subtracting the initial strain (at N = 1) from the total strain (at N >> 1) the fa-

tigue strain (corresponding to the upper load level) can be experimentally deter-
mined. By plotting the decrease in stiffness against the corresponding fatigue strain
it can be shown that the curves run closely to each other even although they belong
to different load levels. The loading parameters (load level, number of cycles) that
lead to a certain state of fatigue strain seems to be negligible. Furthermore, the
results show significant changes in the shape of the stress-strain curves during
cyclic loading whereas the compressive strength remains unchanged.

318

R. Breitenbücher, H. Ibuk, H. Alawieh

1. Introduction

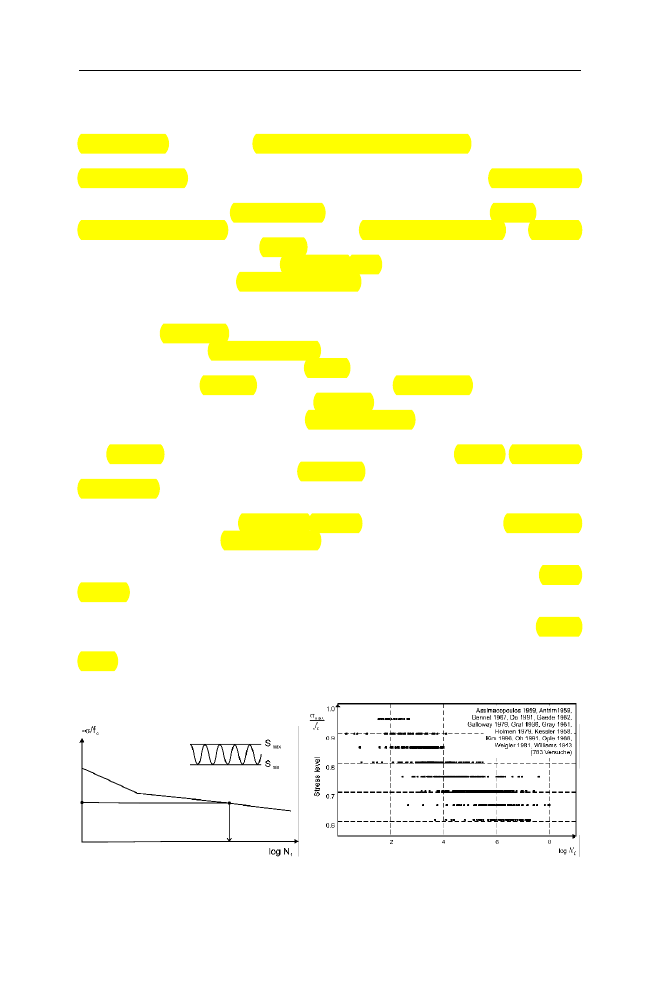
Investigations dealing with cyclic uniaxial compression loads are presented by
various scientists [1–6]. Those investigations mainly focused on describing the
fatigue failure of concrete in form of curves by Wöhler at various stress-strength
ratios (Fig. 1). However, the development of mechanical properties (e.g. stress-
strain relation, strength, ultimate strain) before the fatigue failure occurs almost
remained unconsidered. For fatigue problems, concerned with tension and flexural
loads a wide data base can be quoted [7, 8, 9]. But, these results cannot directly be
transferred to concrete behaviour exposed to pure cyclic compression loading.

In order to close these lack of information the here presented experimental in-

vestigation deal with the degradation of relevant concrete properties (see above).
Research works documented in current literature commonly represent single re-
sults and do not reveal any statistically verified descriptions of the degradation
processes based on a sufficient amount of data. In this context it has to be consid-
ered, that such degradation processes scatter within a large range (Fig. 2) [6, 10].

The reduction in stiffness (E) can be deduced to some extent from existing ex-

perimental data in the literature due to adequate cyclic loading tests. However, for
the other relevant material properties mentioned above, only a few research works
are available. Within Wöhler-tests the decrease of the compressive strength (f

c

)

was revealed by Bennett & Muir [11] only on the basis of ‘run-out’ specimens,
which were checked statically after sustaining more than one million load cycles.
Furthermore, systematic investigations were performed by Awad & Hilsdorf [12]
to determine the reduction in compressive strength (f

c

). However, these tests refer

only to a small number of specimens. Beyond that, the changes in the stress-strain
relation in most cases were examined only up to the upper stress levels of the
fatigue load (Mehmel & Kern [13]).

Beside describing the degradation process of concrete properties due to cyclic

loading it is also necessary for models within fatigue simulations (already devel-
oped within in the Collaborative Research Center 398) to divide the fatigue strain
(

ε

fat

) into two parts, namely into a “damage” and a “plastic” part, and to clarify

their changes during cyclic loading. That means that only a fraction of the fatigue
strain (

ε

fat,da

) determine the degradation process. In this context the parameter

β

fat

Fig. 1. Schematic S-N curve (Wöhler
lines)

Fig. 2. Variation of maximal bearable number of
load cycles to failure N

f

[10]

Influence of cyclic loading on the degradation of mechanical concrete properties

319

is introduced, which is defined as the relation between

ε

fat,da

and the fatigue strain

ε

fat

(Fig. 3). In order to formulate the fatigue damage in consideration of

β

fat

within

material models, the parameter

β

fat

is determined by evaluating experimental data

of different cyclic test series carried out by Holmen [6, 14]. Although different
load levels of the cyclic tests are evaluated a approximately constant value for

β

fat

can be quoted. That means, that

β

fat

remain constant up to the fatigue fracture

(N/N

f

= 1) and is independent of load levels of the cyclic loading.

2. Experimental investigation

Specimens

For the tests described in the following cylindrical specimens with a diameter d of
100 mm and a height h of 350 mm were used. These specimens were taken as
cores, drilled from concrete blocks. For these investigations normal-weight con-
cretes C30/37 and C70/85 were used. For monitoring the longitudinal strains two
strain gauges (50 mm in length) were applied in axial direction on opposite sides
of each specimen.

Experimental strategy and setups

Within the scope of the test series the specimens were loaded with a constant fre-
quency (f = 7 Hz) by a hydraulic cylinder within a defined stress-range S

max

/S

min

.

During these cyclic tests, starting at a concrete age of about 40 days, the longitu-
dinal strains were measured continuously. Before cyclic testing the specimens
remained on air until the conditions of the surrounding were reached. For all tests
the lower stress level S

min

was adjusted to 0.10 f

c

. The tests were carried out for

the 3 different upper stress levels S

max

= 0.75 f

c

, 0.675 f

c

and 0.60 f

c

.

Microdefects and their development were investigated by non-destructive ultra-

sonic (US) measurements after a defined number of load cycles. The Young’s
modulus was also measured at the same stages by carrying out an additional load

Fig. 3. Development of the parameter

β

fat

due to cyclic loading [14]

320

R. Breitenbücher, H. Ibuk, H. Alawieh

cycle with a S

max

/S

min

-ratio of 0.30 f

c

/0.10 f

c

and determining the corresponding

strains.

Additionally to these non-destructive testings, the development of the mechani-

cal properties – especially the changes in the stress-strain curve and strength –
were investigated by destructive tests on specimens taken out of the equipment
after certain defined numbers of cycles. By comparison of the various stress-strain
curves the development of the strength, stiffness and fracture energy of the respec-
tive concrete can be described.

3. Results

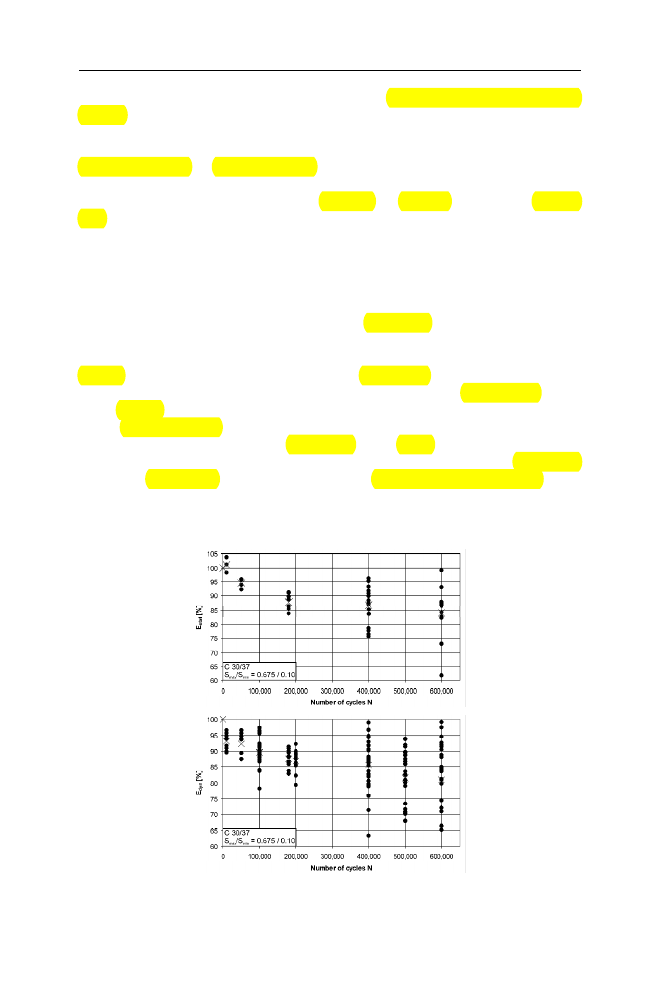
Degradation determined by decrease of stiffness

The state of the concrete damage is described by the degradation of the stiffness at
certain number of cycles (Fig. 4). For all the considered stress levels a sharp de-
crease could be observed after only a few number of cycles. Exemplarily at the
stress regime S

max

/S

min

= 0.675/0.10 the Young’s modulus E

stat

as well as the dy-

namic elastic modulus E

dyn

decreased within the first 180,000 number of cycles

(Fig. 4). A steady decline with a significant lower slope was observed between
180,000 and 400,000. After applying 400,000 number of cycles some specimens
showed an accelerated decrease in E

stat

and E

dyn

. The coefficient of variation of the

measured E

stat

and E

dyn

varied between 20% and 80%. Especially after about

Fig. 4. Decrease and scatter of E

stat

and E

dyn

at S

max

/S

min

= 0.675 f

c

/0.10 f

c

Influence of cyclic loading on the degradation of mechanical concrete properties

321

400,000 a significant increment in the standard deviation and coefficient of varia-
tion had to be considered.

As already mentioned the here obtained specimens were not loaded until fail-

ure. Therefore the results in Fig. 4 could not be referred to their specific failure
(N

f

). Moreover it is shown by a great many of Woehler tests that the maximal

bearable number of load cycles to failure (N

f

) vary widely. As a result it is not

suitable to predict the specific state of failure N

f

of the obtained specimens with

a adequate accuracy (Fig. 2).

Degradation determined by changes in stress-strain relation

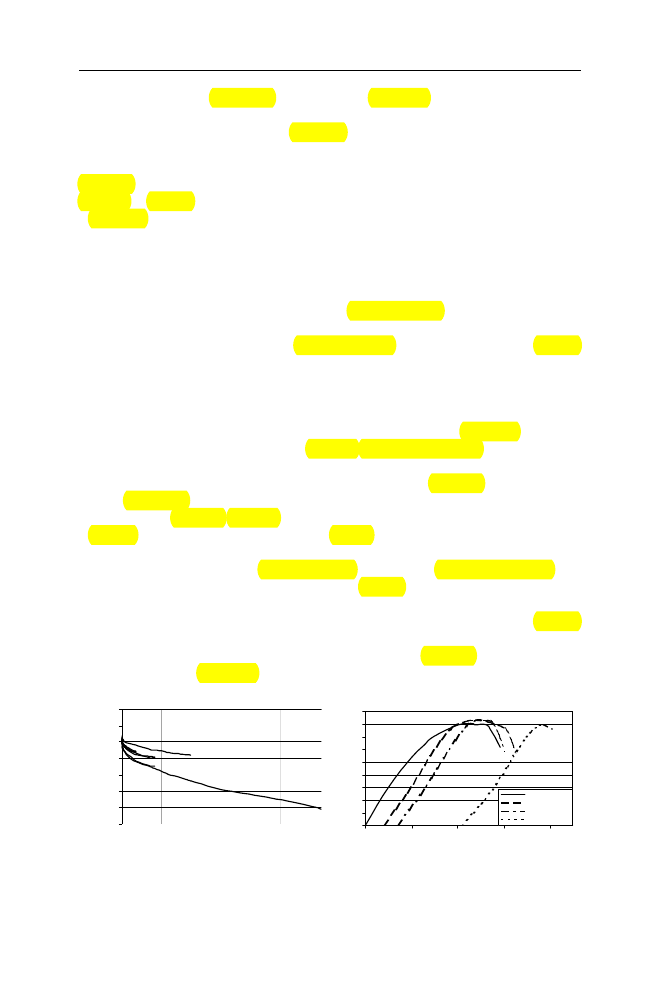
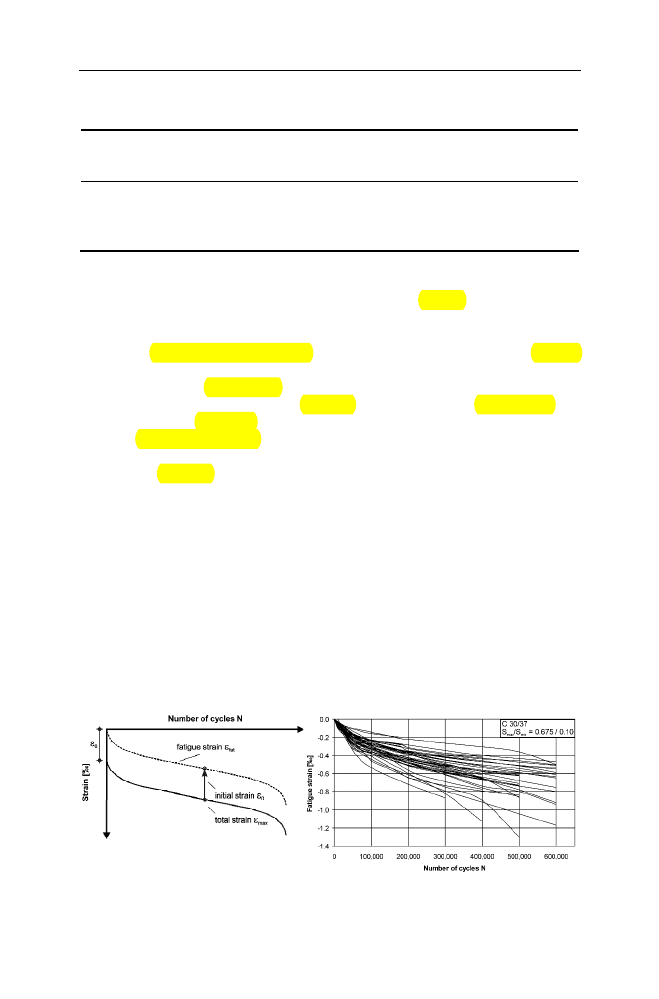
The deformation behaviour was acquired simultaneously to cyclic loading by
measuring the evolution of the longitudinal strain. The characteristic development
of longitudinal strain at

σ

max

is representatively shown at the stress regime

S

max

/S

min

= 0.60 f

c

/0.10 f

c

(Fig. 5). Until the first 2.0 millions of cycles the longitu-

dinal strain in general increased faster than the phase after 2.0 millions of cycles.
Up to 25.55 million cycles no failure due to the cyclic loading could be observed.

After reaching a defined number of cycles the degree of degradation of concrete

was examined by destructive tests (stress-strain relation). The averaged results of
these tests are shown in Fig. 5. The residual irreversible strains – caused by the
cyclic loading – after unloading are also integrated at the beginning of the stress-
strain curves. This deformations increased with increasing duration of loading.

The ascending branch of the stress-strain curves at different number of cycles

changed from concave towards the strain axis to a straight line and further to
a convex shape as shown in Fig. 5. The cracks in the microstructure of the con-
crete due to the cyclic load had an decreasing effect on the development of the
Young’s modulus (E

stat

), the fracture energy (g

c

) and the compressive strain (e

u

)

(Table 1). The fracture energy was calculated hereby as the area below the stress-
strain curve up to the compressive strain.

According to the increase of the longitudinal strain at the stress regime

S

max

/S

min

= 0.60 f

c

/0.10 f

c

the concrete properties E

stat

, g

c

and

ε

u

decreased also

faster up to the first 2.0 millions of cycles than after, whereas the compressive
strength (f

c

) almost remained constant.

-3.5

-3.0

-2.5

-2.0

-1.5

-1.0

-0.5

0.0

0

5

10

15

20

25

Number of cycles N [in million]

Tot

al

longit

udina

l s

tr

ain

ε

[‰

]

0

5

10

15

20

25

30

35

40

45

0.0

1.0

2.0

3.0

4.0

Strain

ε [‰ ]

S

tr

ess [

N

/m

m

2

]

N=0
N=1,800,000
N=4,150,000
N=25,550,000

Fig. 5. Measured longitudinal strain at

σ

max

and stress-strain curves at different number of

cycles (S

max

/S

min

= 0.60 f

c

/0.10 f

c

).

322

R. Breitenbücher, H. Ibuk, H. Alawieh

Table 1. Changes of concrete properties due to cyclic loading determined by changes in
stress-strain relation at S

max

/S

min

= 0.60 f

c

/0.10 f

c

Number

of cycles
N [Mio.]

Young’s
modulus

E

stat

[kN/mm

2

]

Compressive

strength

f

c

[N/mm

2

]

Compressive

strain

ε

u

[‰]

Fracture

energy

g

c

[kJ/m

3

]

0

28.4

40.2

2.3

63.8

1

.8

26.8

41.6

2.0

47.5

4

.15

25.6

42.0

1.9

43.8

25

.5

24.4

39.6

1.8

36.8

Adequate description of degradation by fatigue strain

Investigations carried out e.g. by Holmen [6] demonstrate the difficulties to for-
mulate the state of concrete damage depending on the ratio of already applied
number of cycles N to the maximal bearable number of load cycles until failure
N

f

. So for example, at a defined ratio of S

max

/S

min

(0.675/0.050) some specimens

failed already after only 10

5

cycles, whereas other ones of same composition and

loading conditions exceeded 3 x 10

6

cycles. This quite different behaviour shows

that each examined specimen has a specific value of N

f

. On the basis of these facts

a description of the degradation process of concrete subjected to load parameters
(N/N

f

) is not suitable.

Hence an alternative approach by material parameters was used to characterise

the state of damage more precisely. Starting from the measured total longitudinal
strains, which followed the typical S-shape, the development of the total strain can
be divided into two parts (Fig. 6, left). The first is given by the static loading at the
first cycle (initial strain) and the second part describes the fatigue strain. The fa-
tigue strain caused by the cyclic loading is derived by subtracting the initial strain
from the total strain (Fig. 6).

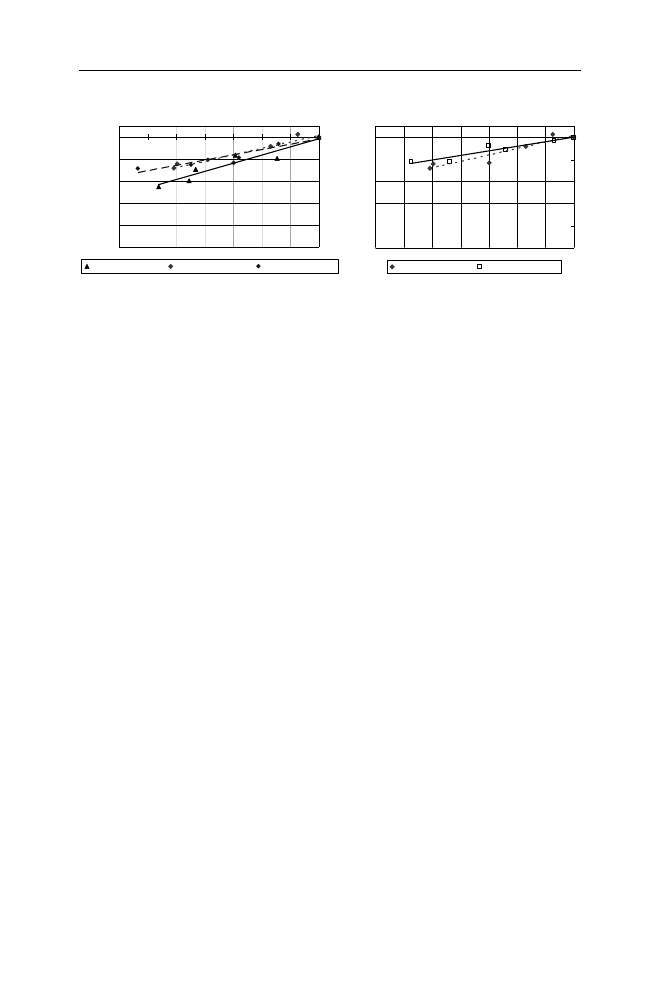
In Fig. 7 the averaged values of the residual Young’s modulus are plotted

against the corresponding fatigue strain. It becomes obvious that the approxima-
tion lines shift together. The trendlines for the lower stress levels almost lie on
each other. At lower stress levels it seems that the process of fatigue damage is
mainly coupled with the evolution of fatigue strain. The cause for the deformation,

Fig. 6. Calculation of fatigue strain and fatigue strain

Influence of cyclic loading on the degradation of mechanical concrete properties

323

i.e. stress level and number of cycles, appear to be negligible. That means, the
damage is governed only by the evolution of fatigue strain. The resulting values of
the residual Young’s modulus of all investigated stress levels resume to one trend-
line (Fig. 7, left). In comparison to the examinated normal strength concrete, the
results of the high strength concrete followed also the same trendline (Fig. 7,
right). Because of the linear relationship between fatigue strain and the decline of
the residual E-Modul it can concluded that the fraction of the damage strain re-
main constant due to cyclic loading.

4. Discussion, Conclusions

At a certain number of cycles the averaged degree of degradation of the Young’s
modulus and the dynamic elastic modulus were almost on a similar level. The
averaged development of E

stat

and E

dyn

as well as the determined longitudinal

strain resembled towards the abscissa the form of the first two phases of a typical
S-shape curve due to fatigue loading. By repetitive measurements the great scatter
in the cyclic test-results could be confirmed. The stress-strain relation is particu-
larly suitable for describing material behaviour. The obtained results showed sig-
nificant changes in the stress-strain curve in terms of the shape, magnitude of the
compressive strain and fracture energy. No influence on the compressive strength
could be observed. Furthermore it could be shown that the fatigue strain as a mate-
rial parameter is better suited for describing the degradation process of concrete
due to cyclic loading as load parameters like N/N

f

. Assumptions referring to the

degradation process within material models could be validated.

Acknowledgement

The authors acknowledge the financial support of the German Research Founda-
tion (DFG). The experimental work (A13) described in this paper was carried out

50

60

70

80

90

100

-0,7

-0,6

-0,5

-0,4

-0,3

-0,2

-0,1

0

fatigue strain [‰ ]

R

es

id

u

al E

-M

o

du

l [

%

]

Smax=0.75, C 30/37

Smax=0.675, C 30/37

Smax=0.60, C 30/37

50

60

70

80

90

100

-0,7

-0,6

-0,5

-0,4

-0,3

-0,2

-0,1

0

fatigue strain [‰ ]

R

es

idua

l E

-M

odu

l [%

]

Smax=0.675, C 30/37

Smax=0.675, C70/85

Fig. 7. Relationship between fatigue strain and degree of fatigue damage

324

R. Breitenbücher, H. Ibuk, H. Alawieh

at the Ruhr-University of Bochum as part of a programme of the Collaborative
Research Center 398 (SFB 398).

References

1.

Hsu TTC (1981) Fatigue of plain concrete. ACI Journal 78: 292

−305

2.

Klausen D (1978) Strength and damage of concrete by frequently repeated stress (in
German). Ph.D thesis, University of Technology Darmstadt

3.

Van Leeuwen J, Siemes AJM (1979) Miner's rule with respect to plain concrete.
Heron 24: 1

−34

4.

Tepfers R, Kutti T (1979) Fatigue strength of plain, ordinary and lightweight con-
crete. ACI Journal 76: 635

−652

5.

Weigler H, Klausen D (1979) Fatigue behaviour of concrete – Effect of loading in
the fatigue strength range. Betonwerk + Fertigteiltechnik 4: 159

−163

6.

Holmen JO (1979) Fatigue of concrete by constant and variable amplitude loading.
Ph.D thesis, Division of Concrete Structures, The Norwegian Institute of Technol-
ogy, Trondheim

7.

Zhang B, Wu K (1997) Residual fatigue strength and stiffness of ordinary concrete
under bending. Cement and Concrete Research 27: 115

−126.

8.

Kessler-Kramer C, Müller HS (2000) A Fracture Mechanical Investigation of the
Fatigue Behaviour of Concrete under Tension. In: Proceeding the 3rd International
PhD Symposium in Civil Engineering. Vienna, pp 107

−115.

9.

Kessler-Kramer C, Mechtcherine V, Müller HS (2001) Fatigue behaviour of concrete
in tension. In: de Borst et al. (eds) Fracture Mechanics of Concrete Structures. Sweets
& Zeitlinger, Lisse, pp 573

−578.

10. Pfanner D (2002) About degradation of reinforced concrete components due to fa-

tigue loading (in German). Ph.D thesis, Ruhr-University of Bochum

11. Bennett EW and Muir J (1967) Some fatigue tests of high-strength concrete in axial

compression. Magazine of Concrete Research 19: 113

−117.

12. Awad ME, Hilsdorf HK (1971) Strength and deformation characteristics of plain

concrete subjected to high repeated and sustained loads. Civil Engineering Studies,
Structural Research Service 372, Department of civil engineering, University of Illi-
nois.

13. Mehmel A, Kern E (1962) Elastische und plastische Stauchungen von Beton infolge

Druckschwell- und Standbelastung (in German). DAfStb, Heft 153.

14. Pfister T, Petryna Y, Stangenberg F (2006) Damage modelling of reinforced concrete

under multi-axial fatigue loading. In: Meschke G., de Borst R., Mang H, Bićanić N
(eds) Computational modelling of concrete structures. Balkema, Mayrhofen, pp
421

−429.