img221

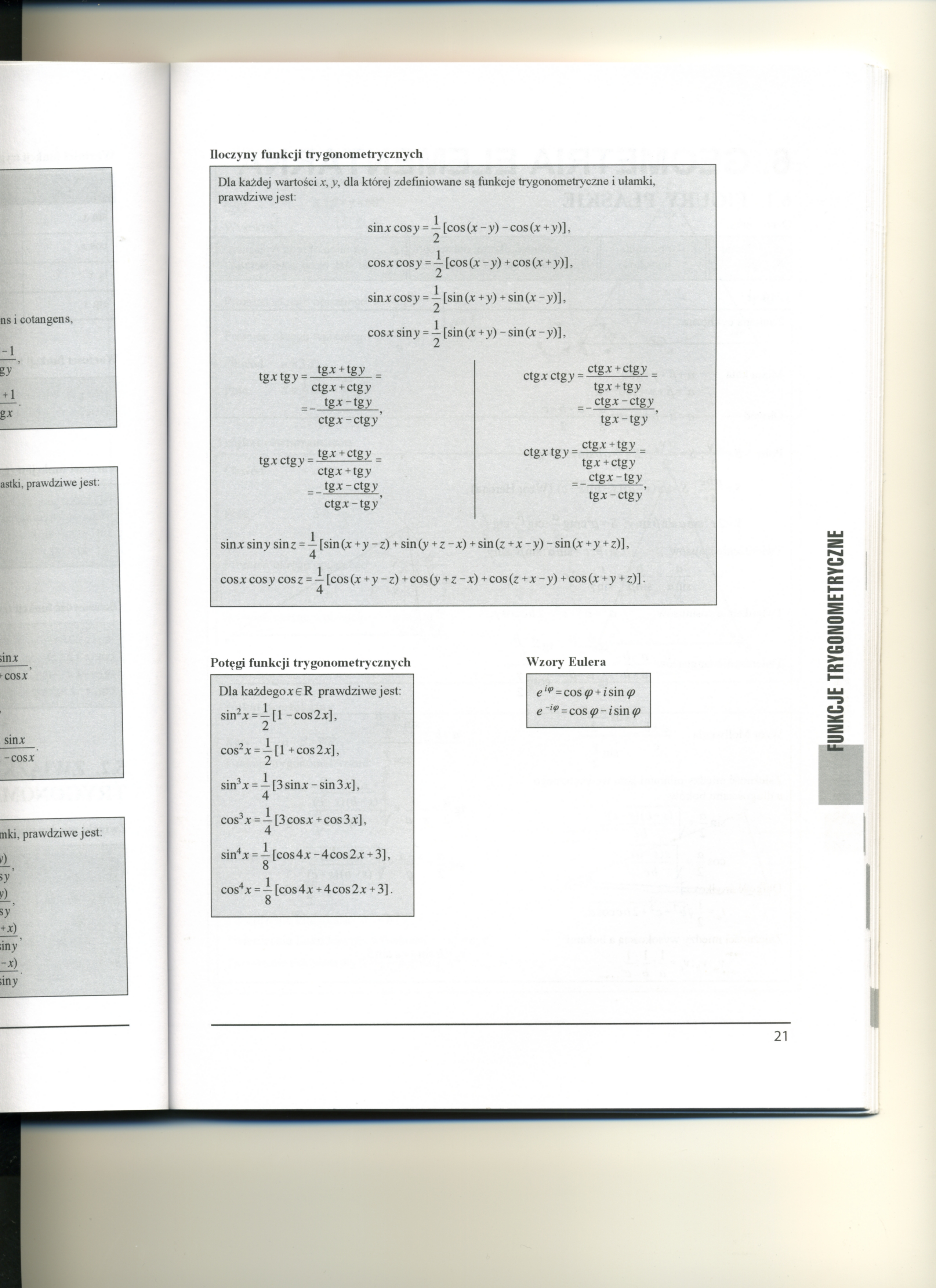
Iloczyny funkcji trygonometrycznych

Dla każdej wartości x, y, dla której zdefiniowane są funkcje trygonometryczne i ułamki, prawdziwe jest:

sin* cosy = ^ [cos (*-y) - cos (* + y)], cos* cosy = -i [cos (* -y) + cos (* + y)], sin* cosy =-i [sin (* +y) + sin(*-y)], cos* siny = — [sin (* + y) - sin (* - y)],

tg* + tgy

tg* ctgy

ctg* +	ctgy
- ‘g*	-tgy
ctg*	-ctgy’
+ * II	ctgy
ctg*	^+ tgy
tg-*	-ctgy

ctg* - tgy

ctg* ctgy =    ₌

tg* + tgy _ ctg*-ctgy

tg*-tgy ’

ctg* tgy

. ctg* + tgy _ tg* + ctgy . ctg*-tgy tg*-ctgy’

sin* siny sin z = — [sin(* +y - z) + sin(y + z -*) + sin (z +* -y) - sin(* +y + z)].

4

cos* cosy cosz = — [cos(* +y -z) +cos(y + z -*) + cos(z +* -y) +cos(* +y +z)]. 4

Potęgi funkcji trygonometrycznych

Dla każdego* eR prawdziwe jest: sin²* ⁼ ^ [1 - cos 2*],

cos²* = ^[l +cos2*].

sin³* = — [3 sin* - sin 3*],

cos³* = — [3 cos* + cos 3 *],

4

sin⁴* = -^[cos4*-4cos2* + 3],

cos⁴* = — [cos4* + 4cos2* + 3]. 8

Wzory Eulera

e‘^q, = cos<p+ińn<p e ^cosęz-isinę?

FUNKCJE TRYGONOMETRYCZNE

21