Modular Origami #2

In this exercise, we make a polyhedron using modular units which serve as 3-fold vertex 
units.  (In the previous modular origami exercise, the modular units served as edges.)  These 
units can be assembled as the vertices of a great many different polyhedra that have 3-way 
vertices.  It is best to start with a polyhedron in which all the faces have 5 or 6 sides, e.g., a 
dodecahedron.  But 4-sided and other faces can also be made.

The module was originally designed by Bennett Arnstein, and is one of many techniques 
described in these two books:

•

Rona Gurkewitz and Bennet Arnstein, 3-D Geometric Origami: Modular Polyhedra, 
Dover, 1995. 

•

Rona Gurkewitz and Bennet Arnstein, Multimodular Origami: Polyhedra, Dover, 
2003. 

A good initial exercise is to make a dodecahedron.  It has 20 vertices, so you will fold and 
assemble 20 triangle units.

A) Make triangles

You will start with many triangles of the same size.  A convenient size, which gets six 
triangles from an 8.5 x 11 inch sheet, is formed by putting three in each half of a sheet. Start 
by folding the sheet in half the long way and unfolding.  Then create a 60 degree angle at one 
end by bringing a corner to the center line and folding to a corner:

Then unfold and rip or cut the sheet in half into two long strips. In each half, use the initial 60 
degree angle as the basis for a zig-zag of three equilateral triangles, with some unused extra at 
each end. Given one line at 60 degrees, its supplement is 120 degrees, so just bisect it to make 
the next 60 degree angle, repeating until you have four lines outlining three triangles:

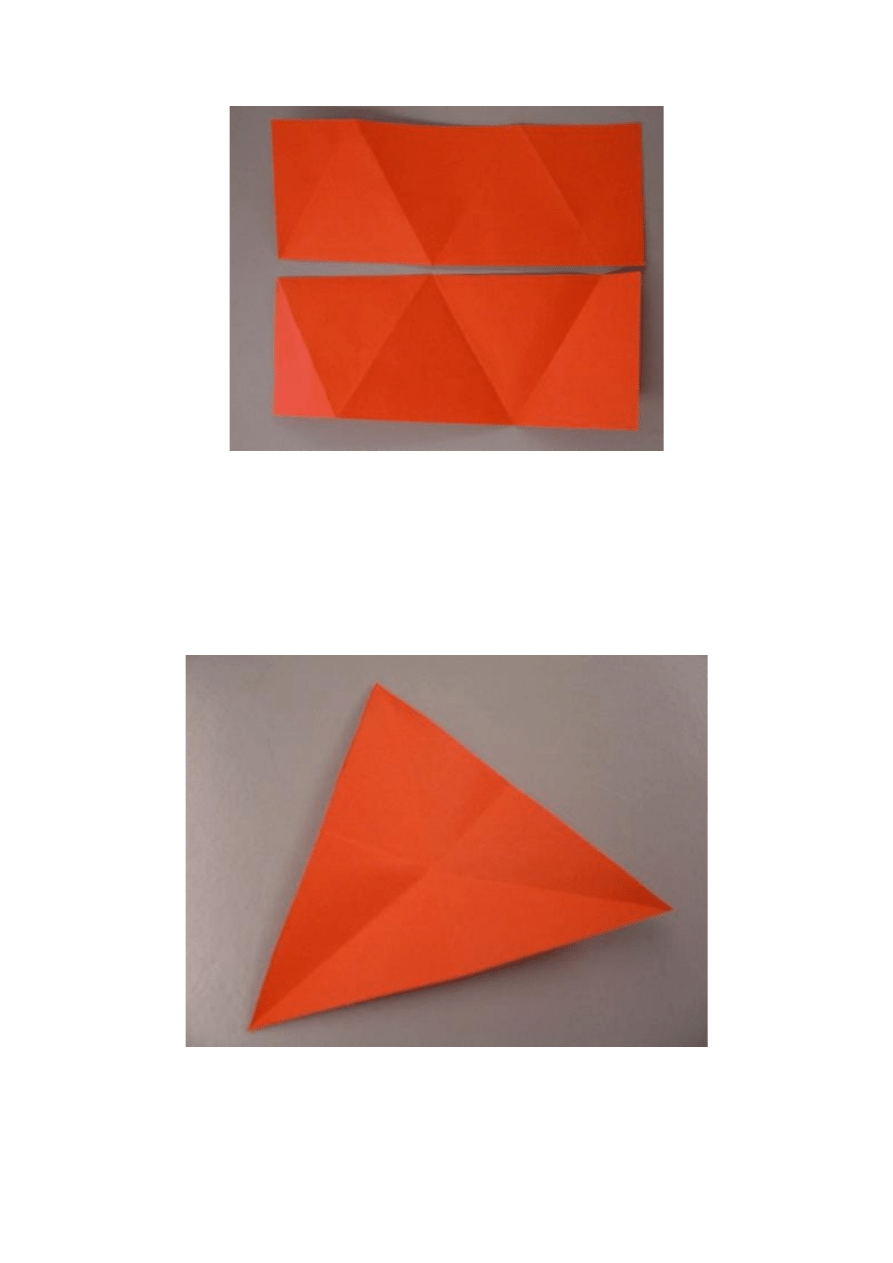
Rip or cut to make the three equilateral triangles from each half-sheet. Toss out the irregular 
ends.

B) Fold a triangle into a module

Do these steps separately for each triangle: 

Fold in half (valley folds) and unfold, all three ways:

Turn over and make 3 folds to bring all three vertices to the center.

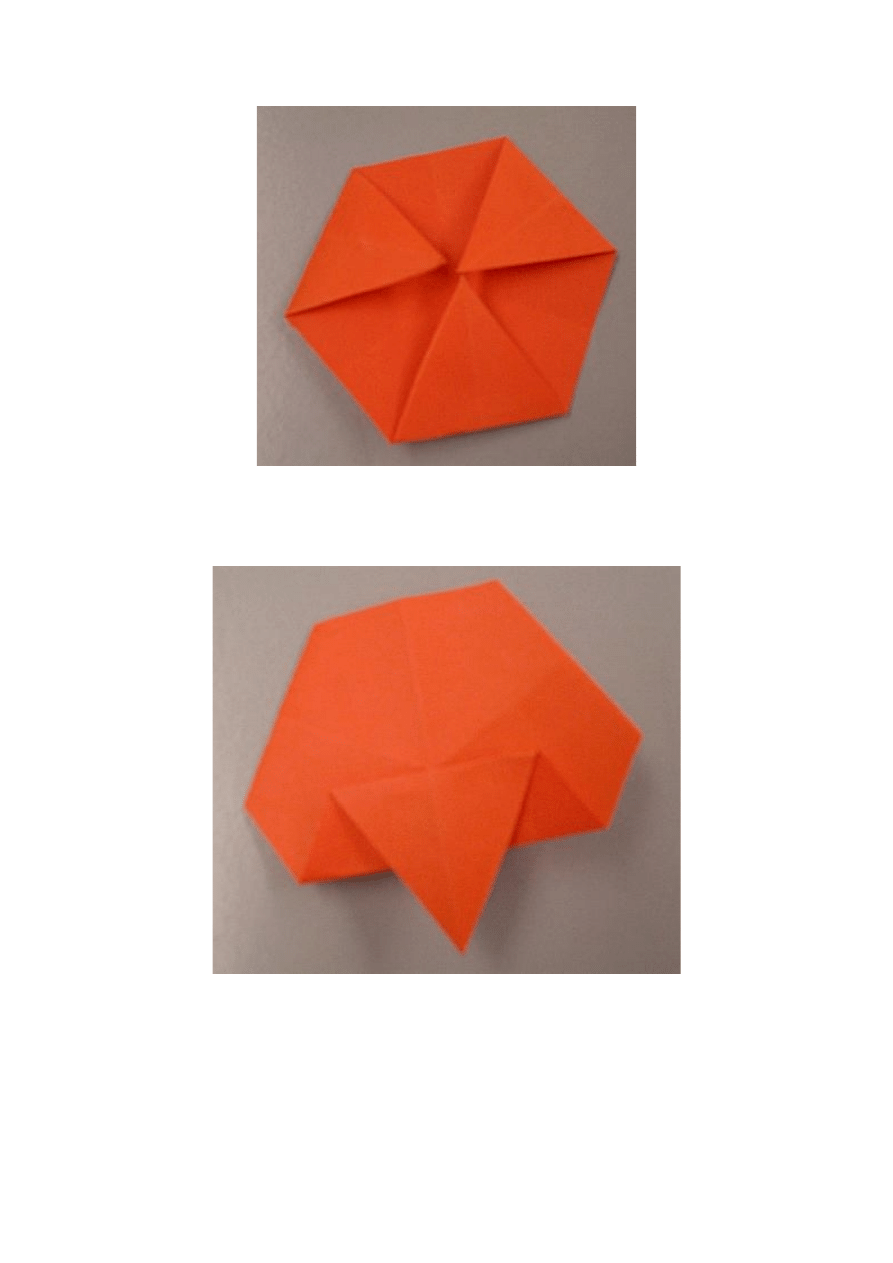
Turn over again and make a fold to bring the midpoint of a folded edge to the center.  As you 
do this fold, lift up so you only fold the top layer. The point which was at  the center on the 
bottom will pop out.

Repeat on the other two folded edges. Whichever side you did last will be on top; it is OK that 
it is not symmetric:

 

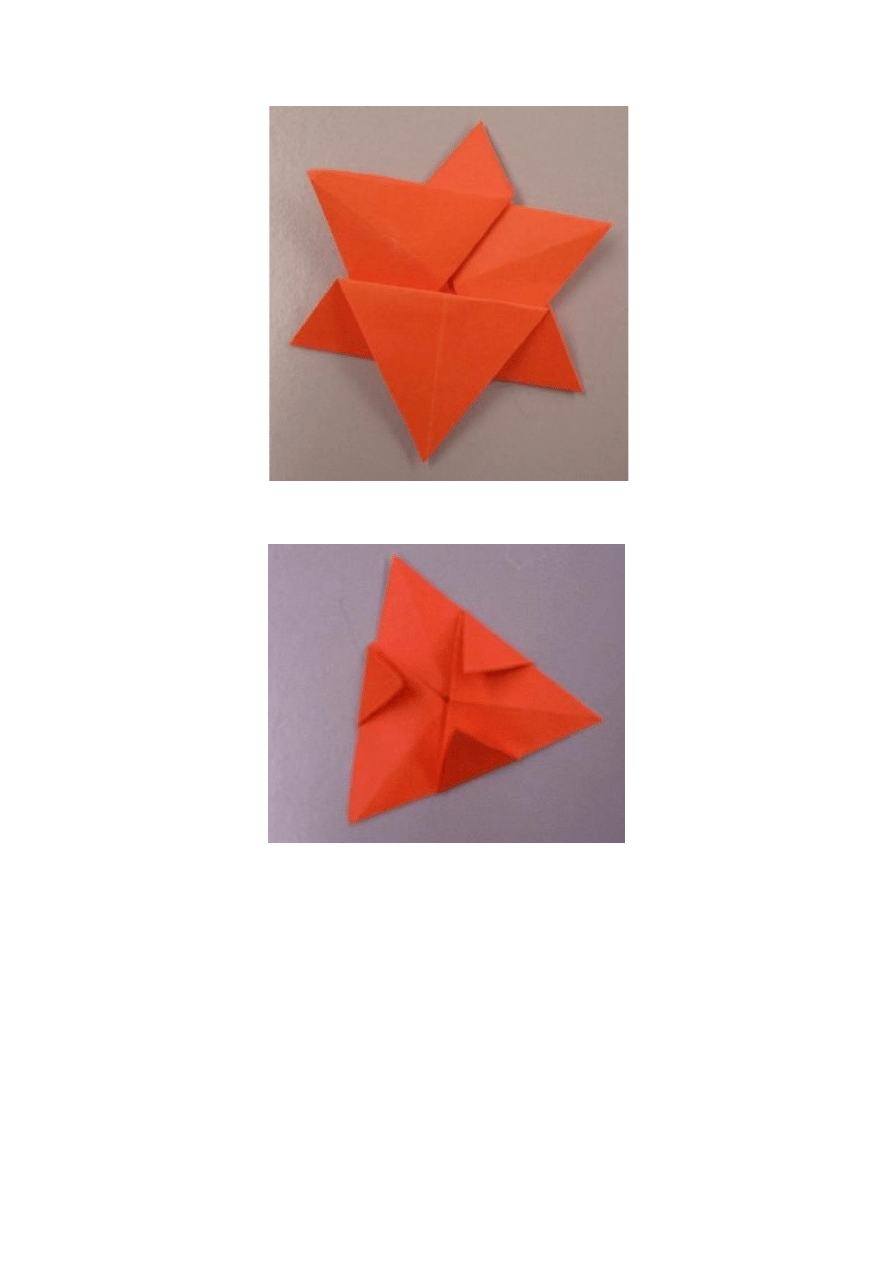
Fold the three thick corners to the center.

Turn over and fold the three long radii as mountains and the three short radii as valleys. (Be 
sure the central points on the bottom stay inside.) Crease well by squeezing in from the three 
sides. You have made one module:

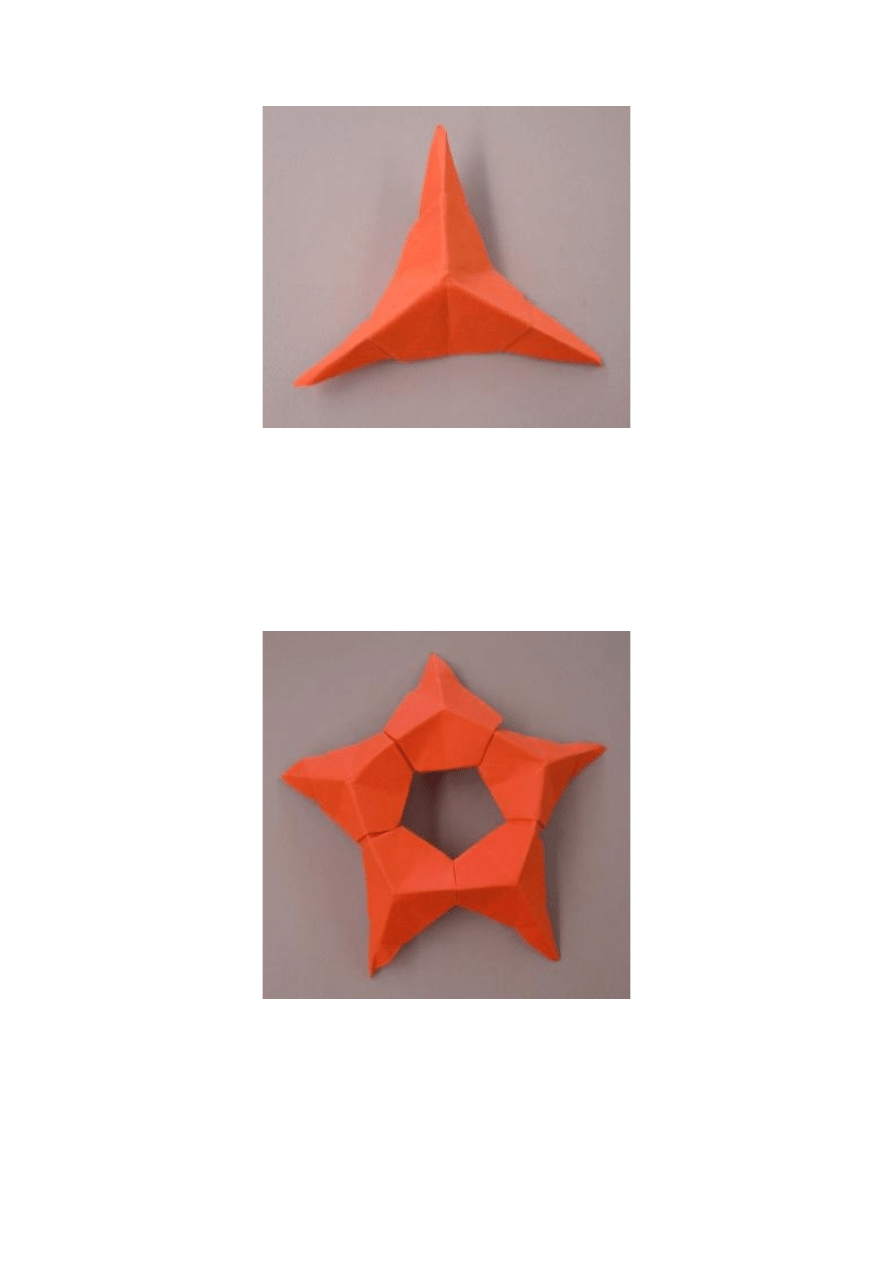
C) Assemble modules

After you have two or more modules you can begin to assemble them. Each module has three 
points and three slots.  (Immediately above each point is a slot.) Neighboring units join by 
putting the point of one into the slot of its neighbor.  Where two units join, either can be the 
"male" and the other will be the "female". Make a cycle of five, leaving a pentagon hole in the 
center.  

This extends in a natural way to make a dodecahedron. The last few connections take some 
creativity.

It will hold together without glue if you don't pull it apart, but the books cited above 
recommend a drop of glue be put on the points before sliding them into the slots. If you don't 
use glue, then you can reuse the modules to make other forms, as suggested below.

D) Make something different

These units have enough flex in them to make a wide range of shapes.  The only constraint is 
that each vertex must have three edges. The truncated octahedron has 6 square faces and eight 
hexagonal faces, all meeting a 3-way vertices.  There are 24 vertices total, so it is a good 
project to make next: