0-8493-1287-6/05/$0.00+$1.50
© 2005 by CRC Press LLC

What Is Coded in the
Primary Motor Cortex?

James Ashe

CONTENTS

Abstract
5.1 Introduction
5.2 Direction Tuning in Motor Cortex; Control of Kinematic Variables
5.3 Population Coding
5.4 What Do Direction Tuning and the Population Vector Represent?
5.5 Muscles or Movements: The Current Controversy
5.6 Statement of the Problem
5.7 The Case for Kinematic Control
5.8 The Case for Kinetic Control
5.9 Successive Coordinate Transformations
5.10 A Modest Proposal
5.11 The Challenge
References

ABSTRACT

The motor cortex is the most basic of cortical motor structures and is intimately
connected with the control of movement parameters. There has been a great deal of
debate as to whether the motor cortex codes for the spatial aspects (kinematics) of
motor output, such as direction, velocity, and position, or primarily relates to con-
trolling muscles and forces (kinetics). Although the weight of evidence is in favor
of the motor cortex controlling spatial output, the effect of limb biomechanics and
forces on motor cortex activity is incontrovertable. Here, I propose (1) that the motor
cortex codes for the most behaviorally relevant spatial variable, and (2) that both
spatial variables and limb biomechanics are reflected in motor cortex activity.

5.1 INTRODUCTION

Voluntary movement is an elemental function. Without movement we cannot com-
municate, walk, feed ourselves, or interact with the environment. It is fitting, therefore,
that the motor cortex was one of the first cortical areas to be explored experimentally.

1,2

Unfortunately, a deep understanding of motor cortex function still eludes us. In

what follows, I will deal with the motor cortex proper, i.e., Brodmann area 4, and
focus on the control of reaching movements of the upper limb. Also, I will not
consider in any detail issues such as how visual signals are integrated into motor
behavior, the putative coordinate transformations in other motor areas necessary to
convert retinal input into a reference frame meaningful for action, or how the
proprioceptive and motor systems interact. I am assuming that the final command
for a motor act comes from the motor cortex; this chapter will focus on the properties
(characteristics) of such a command.

Our understanding of the function of the motor cortex did not progress signifi-

cantly, despite the advances made in cataloguing its connectivity, from the detailed
experiments of Leyton and Sherrington

until the early 1960s, when extracellular

neural recording was introduced in studies of the motor system. The earlier studies,
notably those of Evarts,

4,5

Cheney and Fetz,

Hepp-Reymond,

7,8

and Thach,

showed

that there was a clear relation between neural activity and muscle force. These
experiments also found a relation between cell activity and limb position, and even
in those very constrained behavioral paradigms it was clear that the direction of
movement was an important controlled variable quite apart from muscle force or
posture.

Although activation of single muscles or movement at single joints appeared

to correlate relatively well with neural activity in motor cortex under very restricted
conditions, such explanations no longer held during more naturalistic behaviors. In
the first published experiment in which motor cortex cells were studied during free
arm movements to different visual targets, there was “no simple relation between
the electromyographic (EMG) and single precentral neuron” activity, and “the dis-
charge pattern of single precentral units may be temporally correlated equally well
with any number of joint rotations” (p. 144).

At about the same time an important

behavioral paper suggested that an ideal controller might more efficiently regulate
movement by coding for parameters at the endpoint of the limb.

5.2 DIRECTION TUNING IN MOTOR CORTEX;

CONTROL OF KINEMATIC VARIABLES

In what proved to be a very influential study, Georgopoulos and colleagues

showed

that the activity of cells in the motor cortex was best related to the direction of
movement. In that experiment, monkeys made visually instructed two-dimensional
movements to targets from a central starting point. The frequency of discharge of
the majority of cells varied in an orderly fashion with the direction of movement
(

Figure 5.1

); such cells were regarded as being directionally tuned, and their activity

could be expressed as a sinusoidal function of the direction of movement. The
preferred direction for tuned cells was that in which their neural discharge was
highest. Similar results were found for movements in three dimensions;

the neural

activity was related to the direction of movement

as follows:

(

)

(5.1)

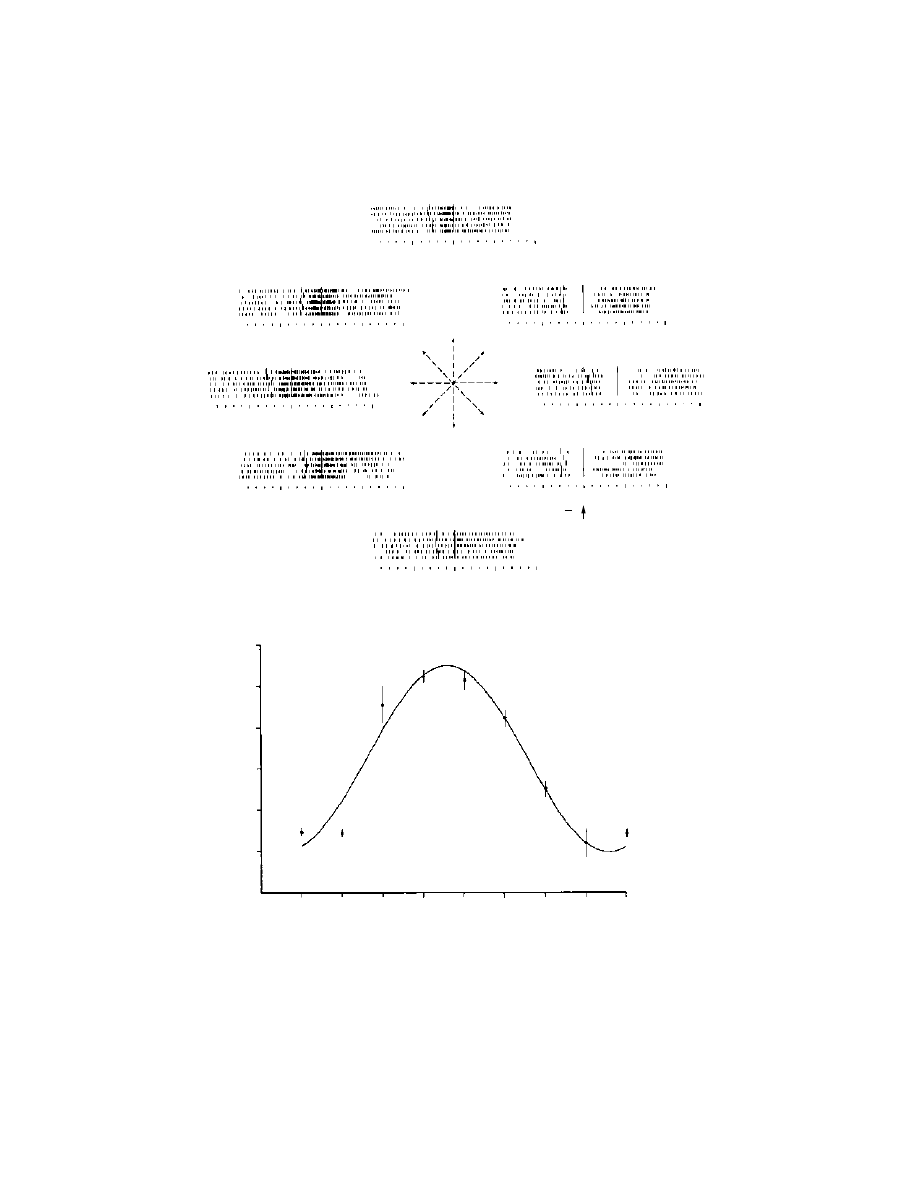
FIGURE 5.1

Variation in the frequency of discharge of a single motor cortex cell during movement in different

directions.

Upper half

: Each small tick indicates an action potential; the display shows impulse activity during

five repetitions (trials) of movements made in each of the eight directions indicated in the center diagram. Trials
are oriented to the onset of movement

Lower half

: Direction tuning curve of the same cell; the average

frequency of cell activity during the response time and movement time is plotted for each of the eight directions.
(From Reference 12, Figure 4, with permission.)

PCA110.S01

SIA

IMPULSES/SEC

135

DIRECTION OF MOVEMENT

225

315

−500

500

1000

−500

500

1000

−500

500

1000

−500

500

1000

−500

500

1000

−500

500

1000

−500

500

1000

−500

500

1000

T M

where

is the frequency of discharge,

is the intercept,

–b

are partial regression

coefficients, [

x, y, z

] are the direction cosines of the three-dimensional movement

vector, and

is an error term. Equation 5.1 implies that there is a direction

for

which the discharge of the cell is the highest, which is the preferred direction of the
cell. One can relate the discharge rate of a cell for movement in direction

relative

to the discharge for its preferred direction

using Equation 5.2:

(

) =

cos

(5.2)

where

and

are regression coefficients, and

is the angle formed by the cell’s

preferred direction

and a particular direction of movement

. In other words, if

we know the discharge of a cell for the preferred direction we can predict the
discharge for any other direction of movement.

The experiment was significant in a number of respects. It provided strong

evidence that, during naturalistic behaviors, neurons in the motor cortex were best
related to the direction of movement in space. It described the direction tuning
properties of these neurons, forming a link with much of the other literature on
direction coding and tuning in other systems.

14,15

It introduced the idea of population

coding of movement variables, forming the basis of later work on the population
vector. Finally, because the data presented in the paper provided strong support for
the coding of a kinematic movement variable, the paper inadvertently led to a
polarization of opinion in the motor control community into camps advocating
kinematic and kinetic coding of movement; this debate is as vibrant now as when
the paper was published.

5.3 POPULATION CODING

The population coding of movement direction was put forward first as a suggestion,

then as a formal hypothesis,

16,17

and finally with detailed neural data for movements

in two

and three dimensions.

18,19

The concept underlying population coding is as

follows: for a population of directionally tuned neurons, each neuron will make a
vectorial contribution to the code in its preferred direction, and at a magnitude that
is proportional to the angle between its preferred direction and the intended direction
of movement. In other words, cells with a preferred direction close to the direction
of movement make a greater contribution; those further away, a smaller one. The
vector sum of the contributions of a population of cells is used to form the population
vector that predicts the direction of the upcoming movement. In formal terms, the
population vector can be expressed as follows:

(5.3)

where

P(M)

is the neural population vector in movement direction

;

is the

preferred direction of the

th cell in the population; and

(

) is a weighting function

that reflects the magnitude of the contribution of

th cell to the population vector

for movement in direction

P M

w M C

(

)

(

)

∑

The population vector is an accurate reflection of the direction of movement

(Color

Figure 5.2

*). It can also be derived during the response time or in delay periods

before movement actually begins. In these contexts, the population vector may reflect
the “intention” of a population of motor cortex cells in relation to movement

FIGURE 5.2

(see color figure) An example of the population coding of movement direction.

The blue lines represent the vectorial contribution of individual cells in the population (

475). The actual movement direction is in yellow and the direction of the population vector
is in red. (From Reference 19, Figure 1, with permission.)

FIGURE 5.3

Evolution of the population vector in time, before and during an instructed arm

movement. A time series of population (P) and movement (M) vectors is shown. The instructed
movement direction is indicated by a small arrow on the far left. The population vector can
be seen to increase in size and point in the direction of the upcoming movement before the
movement occurs.

Stim

is onset of target instruction;

MOV

is onset of movement. (Adapted

from Reference 19, Figure 4, with permission.)

* See color insert following page 170.

.3 sec

−.5

Stim

Mov

(

Figure 5.3

). The population vector can be calculated in time (e.g., every 10 msec)

and therefore gives a continuous readout of the activity of cells that predicts motor
behavior in advance. For the population vector analysis to accurately predict the
direction of movement, the following three conditions need to be satisfied:

(1) the

directional tuning function is one of a broad category of functions that are radially
symmetric around a preferred direction; (2) the preferred directions of the tuned
cells are uniformly distributed; and (3) the values of the tuning parameters

and

are randomly distributed relative to the preferred directions. The accuracy of the
population vector is relatively resistant to cell loss and is a good predictor of
movement direction with as little as 20 tuned cells.

5.4 WHAT DO DIRECTION TUNING AND THE

POPULATION VECTOR REPRESENT?

Although the directional properties of both single cells and populations of cells are
strongly correlated with the direction of movement, this does not necessarily mean
that the cells code for the direction of movement alone. There are many other
variables, such as muscle activity, that also covary with movement direction and thus
might be reflected in cell activity. It is known that single cells in motor cortex generally
engage several motoneuronal pools

21–23

and thus influence the activity of many mus-

cles often distributed across more than one joint.

It can be reasonably assumed that

cells may engage different muscles at different strengths resulting in a set of muscles
with a preferred direction. The combinatorial possibilities of different muscles,
grouped together in distinct sets with different weights, would result in a very large
number of potential preferred directions to which motor cortex cells might relate.
Because the set of muscles to which a particular cell projects is likely to be active
for movements in many different directions, the direction tuning will be quite broad.
This view of the structure-function relation between motor cortex cells and muscles
is consistent with the results of experiments on preferred direction and population
vector. Therefore, the direction of movement in space is not the only interpretation
of the population vector derived from groups of cells, or the preferred direction in
individual motor cortex cells; nevertheless, it is perhaps the most parsimonious.

5.5 MUSCLES OR MOVEMENTS: THE

CURRENT CONTROVERSY

The issue of what is represented in the activity of motor cortex neurons is not new.
It dates back at least, and most famously, to the writings of Hughlins Jackson toward
the end of the 19th century. That the topic should still be hotly debated is just as
remarkable as if current geneticists and molecular biologists were battling it out
over some seminal statement of Gregor Mendel. Jackson wrote, “To speak figura-
tively, the central nervous system [read “motor cortex”] knows nothing of muscles,
it only knows movements.”

25,26

Unfortunately, the statement has been interpreted

literally and not “figuratively,” and thus has created two polarized groups within the
motor control community. One group holds that motor cortex “knows nothing of

muscles,” while the other, adopting an equally extreme position, believes that motor
cortex knows nothing of movements.

5.6 STATEMENT OF THE PROBLEM

It is obvious what the brain must do for us to successfully make voluntary movements
to a target. The target is initially represented within the visual system in retinotopic
coordinate space. We assume that the representation of the target is then transformed
into a coordinate frame that is relevant to the upper limb: either allocentric or “world
space,” or an egocentric frame that is anchored to the body. Finally, the brain
commands the arm to move toward the appropriately transformed target. What form
do these commands take? Does the motor cortex specify the exact activation of the
muscles around the shoulder and elbow joints, so that the appropriate torques are
produced to bring the arm to its target (the extreme kinetic position)? There is no
disagreement that these torques have to be specified at some level for movement to
occur; the issue is whether this specification takes place in the motor cortex. Alter-
natively, does the motor cortex plan the spatial trajectory of the movement alone
(the extreme kinematic position)? Movement kinetics refers to the forces produced
and their derivatives. At the lowest or most fundamental level, these are the forces
produced by individual muscles. However, the level of control could equally well
be that of the torques produced at specific joints or, indeed, the total force generated
by the limb. Kinematics refers to the spatial variables of movement, such as position,
velocity, acceleration, and direction. As is the case with kinetics, the kinematic
variables can be defined for muscles, joints, or the whole limb. The class of variable,
kinetic or kinematic variable, and the coordinate frame for movement control are
hypothetically independent. Nevertheless, kinetic coding is much more likely in
muscle or joint space. Similarly, kinematic coding is more probable in allocentric
(or extrinsic) space.

5.7 THE CASE FOR KINEMATIC CONTROL

More than two decades ago, Morasso

demonstrated what in retrospect seems

obvious: that it is computationally much less demanding to control the kinematics
of the endpoint than the kinematics at the component joints during movements of
the arm (

Figure 5.4

). There is compelling evidence from the psychophysical literature

that movement is indeed planned in terms of extrinsic coordinates,

27–30

although

there are other views.

However, though knowledge of the intrinsic geometry of the

limb may be a necessary part of the planning process.

32,33

Viviani and colleagues

have put forward a “vector coding” hypothesis which holds that during voluntary
movement the target information delivered to the motor system is in vector format
in extrinsic coordinates. Of course, the executive motor system, and particularly the
motor cortex, is under no obligation to operate directly on the information in this
vector format, although, again, it may be the most parsimonious approach.

The most compelling evidence in favor of the kinematic control of movement

comes not from the psychophysical literature, but from direct neural recording in

the motor cortex during a variety of motor behaviors. As mentioned above, there is
a large body of work demonstrating that cells in the motor cortex relate strongly to
the direction of arm movement in space.

12,18,34–39

Because direction of movement

varies along with several other movement variables, which are also potential control
variables, it was necessary to dissociate these variables systematically during neural
recording studies. Two sets of comprehensive dissociation experiments have been
performed. Alexander and Crutcher

40,41

dissociated the direction of arm movement

from the muscles used in a visually controlled task by applying loads to a one-
dimensional manipulandum. Approximately one third of cells in the monkey motor
cortex were related to muscle activation during the execution of movement

and an

even smaller proportion during a preparatory period before movement began.

Kakei

and colleagues

dissociated muscle activity, posture, and direction of movement in

space during a two-dimensional wrist movement task. They found that about 25%

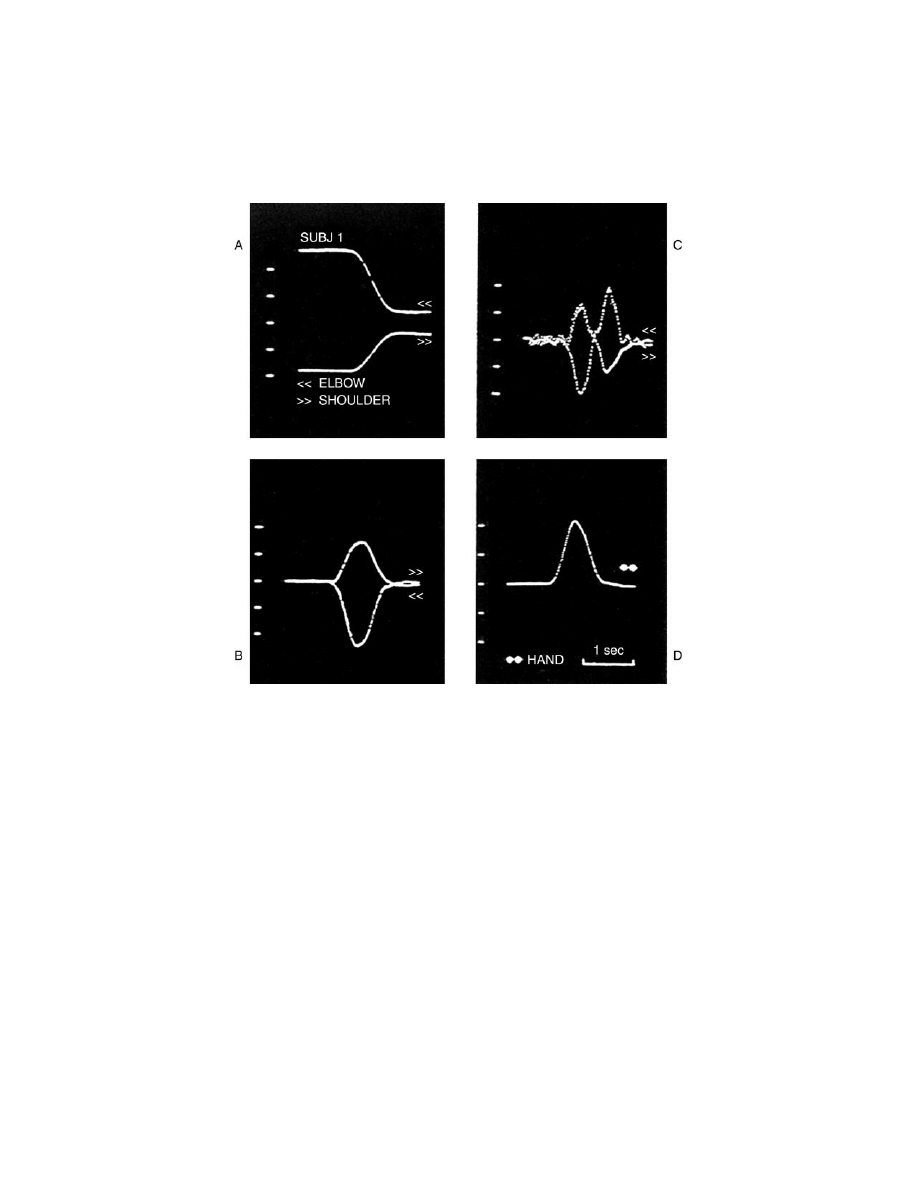
FIGURE 5.4

Records from one subject during a point-to-point movement on a two-dimen-

sional plane. (A) Change in position at the shoulder and elbow joints. (B) Velocity at the
shoulder and elbow joints. (C) Acceleration at the two joints. (D) Velocity at the hand. It is
obvious that the hand velocity would be the simplest variable to control. (Adapted from
Reference 11, Figure 3, with permission.)

of motor cortex cells had “muscle” properties, while approximately 50% related to
direction of movement in space, and reasonably concluded that both “muscles” and
“movements” were represented in the motor cortex (Figure 5.5).

The motor cortex not only seems to encode relatively static kinematic parameters

such as direction during point-to-point movements, but can also reflect parameters that
change continuously during straight movements such as position, velocity, and
acceleration.

The coding principles developed for simple, straight movements to

a target also hold true when applied to more complex ones. For example, direction

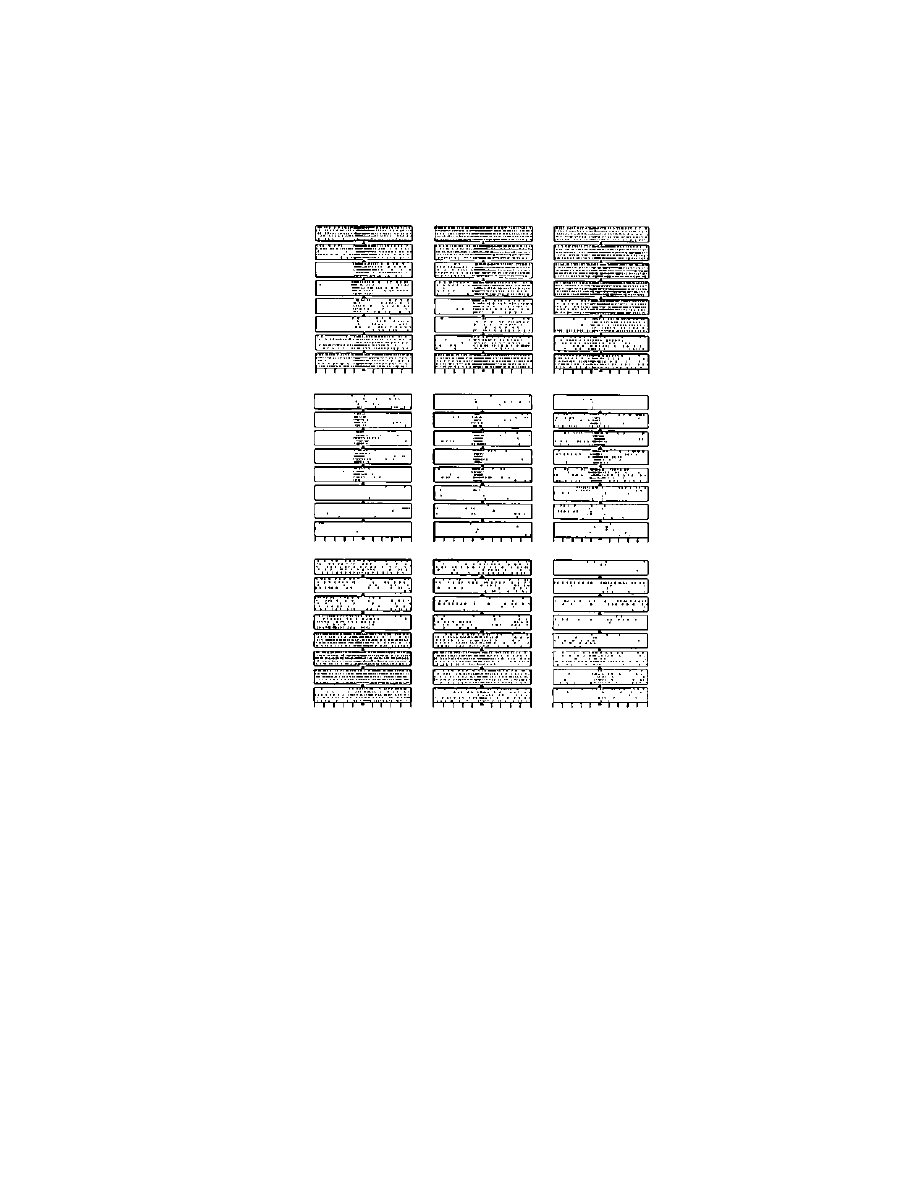
FIGURE 5.5

The records of three single neurons (A, B, C) in the motor cortex for movements

in six different directions (Up, Up and Rt, etc.) in each of three different wrist postures (Pro,
Mid, Sup). All the rasters are aligned to the onset of movement. The tuning of cell B does not
change across the different postures; therefore, it can be categorized as “extrinsic.” The activity
of cell A changes consiberably for the different postures, its preferred direction changing by
79° from Pro to Sup, and can be regarded as “muscle-like.” Cell C is an extrinsic-like neuron
that is also influenced by wrist posture. (From Reference 42, Figure 2, with permission.)

500

−500

500

−500

500

−500

Up+Rt:

Pro

Mid

Sup

Up: E

Dn+Rt:

Rt: U

Dn+Lf:

Dn: F

Up+Lf:

Lf: R

Up+Rt:

Up: E

Dn+Rt:

Rt: U

Dn+Lf:

Dn: F

Up+Lf:

Lf: R

Up+Rt:

Up: E

Dn+Rt:

Rt: U

Dn+Lf:

Dn: F

Up+Lf:

Lf: R

and speed, which vary continuously during drawing or spiral tracing movements,
are strongly reflected in the motor cortex

38,44–46

and the population vectors derived

from these parameters can be used to predict a complex hand trajectory accurately
(Figure 5.6).

In fact, Schwartz and colleagues have shown that models that take

both the direction and speed of movement into account provide more accurate
descriptions of motor cortex activity than those using direction alone. Fitting neural
activity to other time-varying movement parameters like EMG or joint-angle velocity
resulted in a much less accurate model of the data than that obtained for the trajectory
of the hand.

The obvious conclusion from these studies is that during drawing and

other continuous movements, it is the kinematics of movement in extrinsic space
that is primarily reflected in motor cortex activity.

5.8 THE CASE FOR KINETIC CONTROL

Cells in the motor cortex have prominent projections to the spinal cord, and some
have monosynaptic projections to motoneurons that in turn directly control muscle
activation. Muscles and their output, force, are the obvious control variable for cells
in motor cortex. Evarts

4,5

was the first to show a relation between motor cortex

activity and the force generated by the muscles. Since then, a large number of studies
have shown relations between motor cortex and the magnitude, direction, and rate

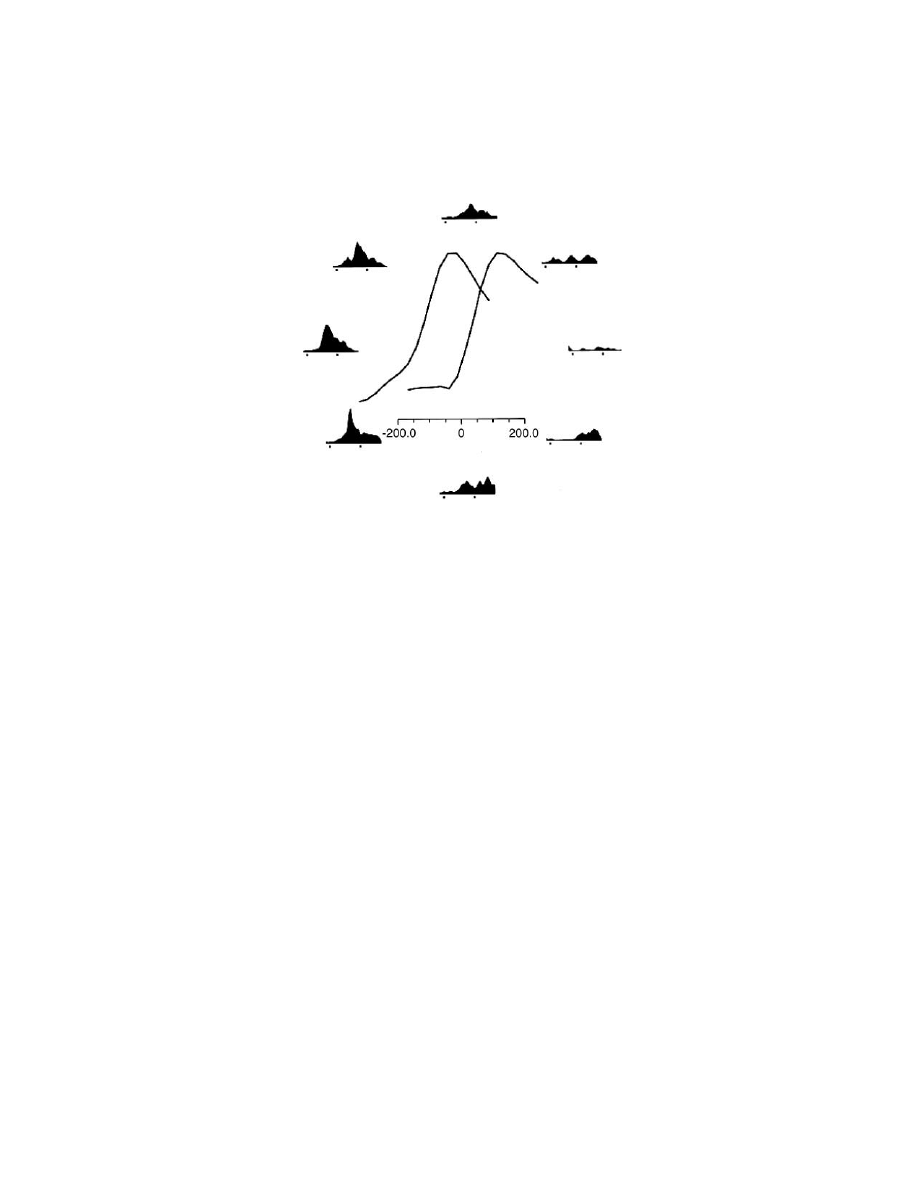
FIGURE 5.6 Representation of speed in a motor cortex cell. The radial histograms show the
averaged neural activity during center-out reaching movements in the respective directions.
The tick marks under each histogram represent 440 msec (the average response time plus
movement time) and indicate the portion used, through averaging across the 8 directions, to
generate the center-left waveform (nondirectional neural profile). There is remarkable con-
cordance between the nondirectional profile (left, center) and the average speed of the move-
ments (right, center). (From Reference 45, Figure 3, with permission.)

of change in force. (See References 47 and 48 for reviews.) However, with a relatively
simple behavior it is possible to find a relation between almost any motor variable
and motor cortex activity. Also, more than one variable may be encoded in the
activity of cells.

The case in favor of kinetic control has rested strongly on showing

that the coding of kinematic variables alone is not a complete explanation of the
variations in cell activity one sees in certain motor behaviors.

49,50

These last studies

showed that using different arm configurations to make movements to a set of spatial
targets resulted in changes in the activity of single cells in the motor cortex, which
suggested that the trajectory of the hand in space was not the only aspect of the
behavior being coded, as this did not change significantly for different arm config-
urations. In addition, other work has demonstrated that the location of the hand, and
hence the configuration of the arm, may have a systematic effect on the direction
tuning of cell activity during an isometric ramp and hold task,

in which no actual

movements were produced.

Despite the influence of arm posture on the activity of single cells in the motor

cortex, the direction of the population vector, based on the activity of these cells,
has been relatively resistent to changes in arm posture.

Nevertheless, in some

circumstances, such as when three-dimensional movements are made to targets from
different starting points, even the population vector appears to be modulated by limb
biomechanics.

Further evidence for the effect of biomechanics on the population

vector comes from experiments in which the biomechanics at the shoulder and elbow
joints were accurately measured, showing that the nonuniformity in the distribution
of population vectors was a function both of velocity and torque at the joints.

Taking these experiments as a whole, one cannot but conclude that the kinetic output
has a distinct influence on the activity of motor cortex cells, although the effect of
biomechanics on the population vector has been modest. It is obvious that arm
kinematics alone cannot account for the changes in neural activity that have been
observed. However, such a conclusion is quite different from stating that the motor
cortex codes primarily for the kinetics of motor output.

5.9 SUCCESSIVE COORDINATE TRANSFORMATIONS

Much has been made, particularly in the psychophysical literature, of the concept
of successive coordinate transformations to explain how visual targets, initially
defined in retinal coordinates, can be reached by the arm, for which the coordinate
frame is defined by the joints and muscles. As discussed above, there is clear evidence
that movement is first specified in terms of kinematics, but the actual movement is
ultimately produced by a weighted activation of groups of muscles (kinetics). The
hypothesis underlying successive coordinate transformations is that different motor
areas, including several sub-areas of parietal cortex, participate in the various stages
of this transformation from kinematics to kinetics. The common wisdom is that the
motor cortex would either be involved in the final stage of the kinematic to kinetic
transformation or would implement the kinetics on instructions from a “higher”
motor area such as the lateral premotor cortex or the supplementary motor area.

As mentioned above, the idea that the motor cortex implements kinetics alone is not
tenable on the basis of current evidence. There is more evidence in favor of the

motor cortex being instrumental in some kind of kinematic to kinetic transformation,
though the form of such a transformation is not at all clear.

34,49,51,53

5.10 A MODEST PROPOSAL

To paraphrase, or contort, the view of Hughlins Jackson: although the motor cortex
knows of both muscles and movements, it appears to be concerned primarily with
space. In other words, the motor cortex primarily codes for the most relevant spatial
aspects of motor output, both in the case of movement and during behaviors that
are purely isometric.

35,47,53–55

One simple experiment illustrates this point. Let us

imagine that one is required to make force pulses in different target directions in
the presence of opposing forces. The muscle forces exerted will not be in the direction
of the targets, because one has to neutralize the opposing forces. Will the activity
of motor cortex cells reflect the actual forces produced by the muscle or the resultant
force (a combination of the muscle force and the opposing force), which is inevitably
in the direction of the target? Using this behavioral paradigm in the monkey, it was
shown that both the single cell and population activity in the motor cortex related
to the resultant force, which was the most relevant spatial variable, and not to the
forces produced by the muscles.

For more than two decades, motor control has

been dominated by studies examining the relation between motor cortex cells and
the spatial aspects of motor output. If motor cortex codes for the spatial aspects of
behavior, in what coordinate framework does this coding occur? It is likely that the
coding occurs in the coordinate frame that is most relevant for the behavior. For
example, the majority of studies has used reaching movements and it is no surprise
that cell activity in those cases reflects an extrinsic reference frame anchored to the
hand. If instead, one were to perform the behavior using the elbow as a pointer, this
would likely be the reference point. Similarly, manipulation of objects by the hands
would be coded in a reference frame that might well be muscle or joint-based relative
to the hands.

However, a coherent theory of coding in the motor cortex must also account for

the clear effect of biomechanical factors on cell activity.

34,49–51,53

Just as gain fields

have been used to explain the interaction of several different frames of reference on
the activity of single neurons in the posterior parietal cortex,

we can perhaps use

a similar framework to explain findings in motor cortex neural recordings. The
composition of such a gain field is as yet uncertain. The available data suggests that
if such a gain field exists, it is comprised of unequal partners, the neural activity
relating to the spatial output predominating, but modulated in a systematic way
dependent on the biomechanics of the limb. For motor behaviors that are performed
in two dimensions — for example, the reaching movements in monkeys — one
might conceptualize such a field as a plane with a relatively shallow slope. Of course,
we currently lack the type of complete quantitative data that would be necessary in
order to construct such putative gain fields accurately, but systematic studies of this
issue are currently being conducted. We predict that the slope of such gain fields is
likely to be small and that the representation of space by the motor cortex, as in the
parietal cortex,

is likely to be distributed.

5.11 THE CHALLENGE

If such gain fields do in fact exist, then a major challenge for those concerned with
the cortical control of motor behavior will be to understand how a cortical repre-
sentation of space, modulated by limb biomechanics, is translated into the muscle
or joint coordinate frame that will ultimately be required for implementation of the
behavior. There are some intriguing possibilities. Bizzi and colleagues

57–60

have

shown, in experiments in the frog and rat, that a set of “motor primitives,” which
could form the basis of activating specific sets of muscles during multiple joint
movement, can be elicited through microsimulation of the spinal gray matter. These
primitives may form the building blocks for voluntary movement by translating
spatial signals from the motor cortex into appropriate muscle output. Recent data
from experiments using long trains of intracortical microstimulation suggest that the
motor cortex may be able to access such primitives directly.

In addition, other

spinal interneuronal systems such as the propriospinal system in the cat

have been

shown to be important in the patterned activation of the different muscles required
for reaching. These propriospinal interneurons may participate in the integration of
reaching movements at a spinal level, and may effectively translate signals from
cells in the motor cortex that relate to the direction of force output of the whole
limb

into appropriate patterns of muscle activation. Another question is how motor

cortex learns to access such motor primitives. It is likely that the association between
motor cortex cell activity and motor primitive “modules” at another level in the
motor system is established through learning and adaptation.

Though conceptually attractive, the idea of successive coordinate transforma-

tions in frontal motor areas culminating in a muscle or joint based coding of motor
output in motor cortex

does not have strong experimental support and should be

abandoned, at least as applied to skilled movements. The search for a direct reflection
of the motor periphery in the motor cortex is likely to be as futile as the quest for
the representation of the single muscle.

REFERENCES

1. Fritsch, G. and Hitzig, E., Über die elektrische Erregbarkeit des Grosshirns, Arch.

Anat. Physiol. Wis. Med., 37, 300, 1870.

2. Ferrier, D., Experiments in the brain of monkeys, Proc. R. Soc. Lond. (Biol.), 23,

409, 1875.

3. Leyton, A.S.F. and Sherrington, C.S., Observations on the excitable cortex of the

chimpanzee, orangutan and gorilla, Qu. J. Exp. Physiol., 11, 135, 1917.

4. Evarts, E.V., Relation of pyramidal tract to force exerted during voluntary movement,

J. Neurophysiol., 31, 14, 1968.

5. Evarts, E.V., Activity of pyramidal tract neurons during postural fixation, J. Neuro-

physiol., 32, 375, 1969.

6. Cheney, P.D. and Fetz, E.E., Functional classes of primate corticomotoneuronal cells

and their relation to active force, J. Neurophysiol., 44, 773, 1980.

7. Smith, A.M., Hepp-Reymond, M.C., and Wyss, U.R., Relation of activity in precentral

cortical neurons to force and rate of force change during isometric contractions of
finger muscles, Exp. Brain Res., 23, 315, 1975.

8. Hepp-Reymond, M.C., Wyss, U.R., and Anner, R., Neuronal coding of static force

in the primate motor cortex, J. Physiol., 74, 287, 1978.

9. Thach, W.T., Correlation of neural discharge with pattern and force of muscular

activity, joint position, and direction of intended next movement in motor cortex and
cerebellum, J. Neurophysiol., 41, 654, 1978.

10. Murphy, J.T., Kwan, H.C., MacKay, W.A., and Wong, Y.C., Activity of primate

precentral neurons during voluntary movements triggered by visual signals, Brain
Res., 236, 429, 1982.

11. Morasso, P., Spatial control of arm movements, Exp. Brain Res., 42, 223, 1981.
12. Georgopoulos, A.P., Kalaska, J.F., Caminiti, R., and Massey, J.T., On the relations

between the direction of two-dimensional arm movements and cell discharge in
primate motor cortex, J. Neurosci., 2, 1527, 1982.

13. Schwartz, A.B., Kettner, R.E., and Georgopoulos, A.P., Primate motor cortex and free

arm movements to visual targets in three-dimensional space. I. Relations between
single cell discharge and direction of movement, J. Neurosci., 8, 2913, 1988.

14. Henn, V. and Cohen, B., Coding of information about rapid eye movements in the

pontine reticular formation of alert monkeys, Brain Res., 108, 307, 1976.

15. Dykes, R.W., Afferent fibers from mystacial vibrissae of cats and seals, J. Neuro-

physiol., 38, 650, 1975.

16. Georgopoulos, A.P., Kalaska, J.F., Crutcher, M.D., Caminiti, R., and Massey, J.T.,

The representation of movement direction in the motor cortex: single cell and pop-
ulation studies, in Dynamic Aspects of Neocortical Function, Edelman, G.M., Gall,
W.E., and Cowan, W.M., Eds., John Wiley & Sons, New York, 1984, 501.

17. Georgopoulos, A.P., Caminiti, R., Kalaska, J.F., and Massey, J.T., Spatial coding of

movement: a hypothesis concerning the coding of movement direction by motor
cortical populations, Exp. Brain Res. Suppl., 7, 327, 1983.

18. Georgopoulos, A P., Schwartz, A.B., and Kettner, R.E., Neuronal population coding

of movement direction, Science, 233, 1416, 1986.

19. Georgopoulos, A.P., Kettner, R.E., and Schwartz, A.B., Primate motor cortex and free

arm movements to visual targets in three-dimensional space. II. Coding of the direc-
tion of movement by a neuronal population, J. Neurosci., 8, 2928, 1988.

20. Lukashin, A.V., Amirikian, B.R., Mozhaev, V.L., Wilcox, G.L., and Georgopoulos,

A.P., Modeling motor cortical operations by an attractor network of stochastic neu-
rons, Biol. Cybern., 74, 255, 1996.

21. Fetz, E.E. and Cheney, P.D., Postspike facilitation of forelimb muscle activity by

primate corticomotoneuronal cells, J. Neurophysiol., 44, 751, 1980.

22. Shinoda, Y., Yokota, J., and Futami, T., Divergent projection of individual corticospi-

nal axons to motoneurons of multiple muscles in the monkey, Neurosci. Lett., 23, 7,
1981.

23. Lemon, R.N., Mantel, G.W.H., and Muir, R.B., Corticospinal facilitation of hand

muscles during voluntary movement in the conscious monkey, J. Physiol. (London),
381, 497, 1986.

24. McKiernan, B.J., Marcario, J.K., Karrer, J.H., and Cheney, P.D., Corticomotoneuronal

postspike effects in shoulder, elbow, wrist, digit, and intrinsic hand muscles during
a reach and prehension task, J. Neurophysiol., 80, 1961, 1998.

25. Jackson, J.H., On the anatomical investigation of epilepsy and epileptiform convul-

sions, in Selected Writings of John Hughlings Jackson, Hodder & Stoughton, London,
1932, 113.

26. Jackson, J.H., On the comparative study of diseases of the nervous system, Br. Med. J.,

2, 355, 1889.

27. Vindras, P., Desmurget, M., Prablanc, C., and Viviani, P., Pointing errors reflect biases

in the perception of the initial hand position, J. Neurophysiol., 79, 3290, 1998.

28. Vindras, P. and Viviani, P., Altering the visuomotor gain. Evidence that motor plans

deal with vector quantities, Exp. Brain Res., 147, 280, 2002.

29. Rogosky, B.J. and Rosenbaum, D.A., Frames of reference for human perceptual-

motor coordination: space-based versus joint-based adaptation, J. Motor Behav., 32,
297, 2000.

30. Rosenbaum, D.A., Meulenbroek, R.G., and Vaughan, J., Planning reaching and grasping

movements: theoretical premises and practical implications, Motor Contr., 5, 99, 2001.

31. Soechting, J.F. and Flanders, M., Moving in three-dimensional space: frames of

reference, vectors, and coordinate systems, Annu. Rev. Neurosci., 15, 167, 1992.

32. Rossetti, Y., Desmurget, M., and Prablanc, C., Vectorial coding of movement: vision,

proprioception, or both? J. Neurophysiol., 74, 457, 1995.

33. Paillard, J. and Brouchon, M., A proprioceptive contribution to the spatial encoding

of position cues for ballistic movements, Brain Res., 71, 273, 1974.

34. Caminiti, R., Johnson, P.B., and Urbano, A., Making arm movements within different

parts of space: Dynamic aspects in the primate motor cortex, J. Neurosci., 10, 2039,
1990.

35. Kalaska, J.F., Cohen, D.A.D., Hyde, M.L., and Prud’homme, M., A comparison of

movement direction-related versus load direction-related activity in primate motor
cortex, using a two-dimensional reaching task, J. Neurosci., 9, 2080, 1989.

36. Hatsopoulos, N.G., Ojakangas, C.L., Paninski, L., and Donoghue, J.P., Information

about movement direction obtained from synchronous activity of motor cortical
neurons, Proc. Nat. Acad. Sci. U.S.A., 95, 15706, 1998.

37. Serruya, M.D., Hatsopoulos, N.G., Paninski, L., Fellows, M.R., and Donoghue, J.P.,

Instant neural control of a movement signal, Nature, 416, 141, 2002.

38. Schwartz, A.B., Direct cortical representation of drawing, Science, 265, 540, 1994.
39. Taylor, D.M., Tillery, S.I., and Schwartz, A.B., Direct cortical control of 3D neuro-

prosthetic devices, Science, 296, 1829, 2002.

40. Alexander, G.E. and Crutcher, M.D., Preparation for movement: neural representa-

tions of intended direction in three motor areas of the monkey, J. Neurophysiol., 64,
133, 1990.

41. Crutcher, M.D. and Alexander, G.E., Movement-related neuronal activity selectively

coding either direction or muscle pattern in three motor areas of the monkey,
J. Neurophysiol., 64, 151, 1990.

42. Kakei, S., Hoffman, D.S., and Strick, P.L., Muscle and movement representations in

the primary motor cortex, Science, 285, 2136, 1999.

43. Ashe, J. and Georgopoulos, A.P., Movement parameters and neural activity in motor

cortex and area 5, Cereb. Cortex, 4, 590, 1994.

44. Schwartz, A.B. and Moran, D.W., Motor cortical activity during drawing movements:

population representation during lemniscate tracing, J. Neurophysiol., 82, 2705, 1999.

45. Moran, D.W. and Schwartz, A. B., Motor cortical activity during drawing movements:

population representation during spiral tracing, J. Neurophysiol., 82, 2693, 1999.

46. Moran, D.W. and Schwartz, A.B., Motor cortical representation of speed and direction

during reaching, J. Neurophysiol., 82, 2676, 1999.

47. Ashe, J., Force and the motor cortex, Behav. Brain Res., 87, 255, 1997. [Corrected

and republished article originally printed in Behav. Brain Res., 86, 1, 1997.]

48. Hepp-Reymond, M.-C., Functional organization of motor cortex and its participation

in voluntary movements, in Comparative Primate Biology, Steklis, H.D. and Erwin,
J., Eds., Alan R. Liss, New York, 1988, 501.

49. Scott, S.H. and Kalaska, J.F., Changes in motor cortex activity during reaching

movements with similar hand paths but different arm postures, J. Neurophysiol., 73,
2563, 1995. [Published errata appear in J. Neurophysiol., 77, 1997, following table
of contents and 77, 2856, 1997.]

50. Scott, S.H. and Kalaska, J.F., Reaching movements with similar hand paths but

different arm orientations. I. Activity of individual cells in motor cortex, J. Neuro-
physiol., 77, 826, 1997.

51. Scott, S.H., Gribble, P.L., Graham, K.M., and Cabel, D.W., Dissociation between

hand motion and population vectors from neural activity in motor cortex, Nature,
413, 161, 2001.

52. Padoa-Schioppa, C., Li, C.S., and Bizzi, E., Neuronal correlates of kinematics-to-

dynamics transformation in the supplementary motor area, Neuron, 36, 751, 2002.

53. Sergio, L.E. and Kalaska, J.F., Systematic changes in motor cortex cell activity with

arm posture during directional isometric force generation, J. Neurophysiol., 89, 212,
2003.

54. Taira, M., Boline, J., Smyrnis, N., Georgopoulos, A.P., and Ashe, J., On the relations

between single cell activity in the motor cortex and the direction and magnitude of
three-dimensional static isometric force, Exp. Brain Res., 109, 367, 1996.

55. Georgopoulos, A.P., Ashe, J., Smyrnis, N., and Taira, M., Motor cortex and the coding

of force, Science, 256, 1692, 1992.

56. Andersen, R.A., Essick, G.K., and Siegel, R.M., Encoding of spatial location by

posterior parietal neurons, Science, 230, 456, 1985.

57. Bizzi, E., Mussa-Ivaldi, F., and Giszter, S., Computations underlying the execution

of movement: A biological perspective, Science, 253, 287, 1991.

58. Giszter, S.F., Mussa-Ivaldi, F.A., and Bizzi, E., Convergent force fields organized in

the frog’s spinal cord, J. Neurosci., 13, 467, 1993.

59. Tresch, M.C., Saltiel, P., and Bizzi, E., The construction of movement by the spinal

cord, Nat. Neurosci., 2, 162, 1999.

60. Bizzi, E., Tresch, M.C., Saltiel, P., and d’Avella, A., New perspectives on spinal motor

systems, Nat. Rev. Neurosci., 1, 101, 2000.

61. Graziano, M.S., Taylor, C.S., and Moore, T., Complex movements evoked by micro-

stimulation of precentral cortex, Neuron, 34, 841, 2002.

62. Lundberg, A., Integration in a propriospinal motor centre controlling the forelimb in

the cat, Igaku Shoin, Tokyo, 47, 1979.

63. Todorov, E., Direct cortical control of muscle activation in voluntary arm movements:

a model, Nat. Neurosci., 3, 391, 2000.

Document Outline

Motor Cortex in Voluntary Movements
- Table of Contents
  - Chapter 5: What Is Coded in the Primary Motor Cortex?

Wyszukiwarka

Podobne podstrony:
(eBook PL,matura, kompedium, nauka ) Matematyka liczby i zbiory maturalne kompedium fragmid 1287
Dz U 2008 nr 206 poz 1287 Tekst aktu
1287
CH05 2 id 110433 Nieznany
1287
1287
Ch05 Layers & Layer Groups
Genomes3e ppt ch05
ch05
czestosciomierz radiowy id 1287 Nieznany
CH05
ch05
9780735625891 SQLServer08AdminPocketConsult ch05
CH05
ch05 1 id 110434 Nieznany
1287
prawo geodezyjni kartograficzne Dz. U. z 2014 nr 193 poz. 1287, Geodezja
1287

więcej podobnych podstron

1287 ch05

Document Outline