
Courtney James Mewton
Page 1
GR, Tensor Analysis & Geometry
Tensor Analysis & Geometry
Spherical Coordinates
φ
θ
cos
sin
1
r
x
=
,
φ
θ
sin
sin
2
r
x
=
,
θ
cos
3
r
x
=
,
ct
x
=
4
3-dimensional line element:
(
)
2
2
2
2
2
2
sin
φ
θ
θ
d
d
r
dr
ds
+
+
=
Christoffel Symbols
Christoffel Symbol of the first kind:
[
]
∂
∂
−
∂
∂
+
∂
∂
=
=
Γ
r
mn
m
rn
n
rm
rmn
x
g
x
g
x
g
r
mn
2
1
,
.
Christoffel Symbol of the second kind:
mnr
rz
z
mn
g
mn
z
Γ
=
î
=
Γ
.
Derivation of the Riemann Curvature Tensor
In general, a second order differentiation on a covariant vector is independent of the order in which it is carried
out, i.e.:
j
k
i
k
j
i
x
x
V
x
x
V
∂
∂
∂
=
∂
∂
∂
2
2
.
However, the presence of Christoffel symbols can have an effect on this statement. We investigate this by first
finding the general second derivatives for both permutations of the differentiating parameters:
( )
r
i
r
jk
j
r
r
ik
jk
i
k
j
i
V
V
V
V
,
,
,
;
;
Γ
−
Γ
−
=
.
But
s
s
ij
j
i
j
i
V
V
V
Γ
−
=
,
;
,
( )
[
] [
]
s
s
ir
r
i
r
jk
s
s
rj
j
r
r
ik
k
s
s
ij
s
k
s
ij
jk
i
k
j
i
V
V
V
V
V
V
x
V
V
Γ
−
Γ
−
Γ
−
Γ
−
Γ
−
∂
Γ
∂
−
=
∴
,
,
,
,
:
:
( )
s
s
ir
r
jk
r
i
r
jk
s
s
rj
r
ik
j
r
r
ik
k
s
s
ij
s
k
s
ij
jk
i
k
j
i
V
V
V
V
V
V
x
V
V
Γ
Γ
+
Γ
−
Γ
Γ
+
Γ
−
Γ
−
∂
Γ
∂
−
=
⇔
,
,
,
,
:
:
Now we interchange j and k (which is the other possible way of determining this second derivative):
( )
s
s
ir
r
kj
r
i
r
kj
s
s
rk
r
ij
k
r
r
ij
j
s
s
ik
s
j
s
ik
kj
i
j
k
i
V
V
V
V
V
V
x
V
V
Γ
Γ
+
Γ
−
Γ
Γ
+
Γ
−
Γ
−
∂
Γ
∂
−
=
,
,
,
,
:
:
.
We now find the difference between these two. On the RHS, the first, third, fourth, sixth, and seventh terms
cancel out, thus giving the result:
s
s
rk
r
ij
s
rj
r
ik
k
s
ij
j
s
ik
s
s
rk
r
ij
s
j
s
ik
s
s
rj
r
ik
s
k
s
ij
kj
i
jk
i
V
x
x
V
V
x
V
V
x
V
V
Γ
Γ
−
Γ
Γ
+
∂
Γ
∂
−
∂
Γ
∂
=
Γ
Γ
−
∂
Γ
∂
+
Γ
Γ
+
∂
Γ
∂
−
=
−
:
:
.
We define the Riemann (or Riemann-Christoffel) Curvature tensor by:
s
rk
r
ij
s
rj
r
ik
k
s
ij
j
s
ik
s
ijk
x
x
R
Γ
Γ
−
Γ
Γ
+
∂
Γ
∂
−
∂
Γ
∂
=
.
The difference between the covariant derivatives can thus be written as
s
s
ijk
kj
i
jk
i
V
R
V
V
=
−
:
:
. The Riemann
tensor used in this equation is called the Riemann curvature tensor of the second kind. The curvature tensor of
the first kind is defined as:
r
jkl
ir
ijkl
R
g
R
=
.
Symmetry Properties:
First skew symmetry
jikl
ijkl
R
R
−
=
Second skew symmetry
ijlk
ijkl
R
R
−
=
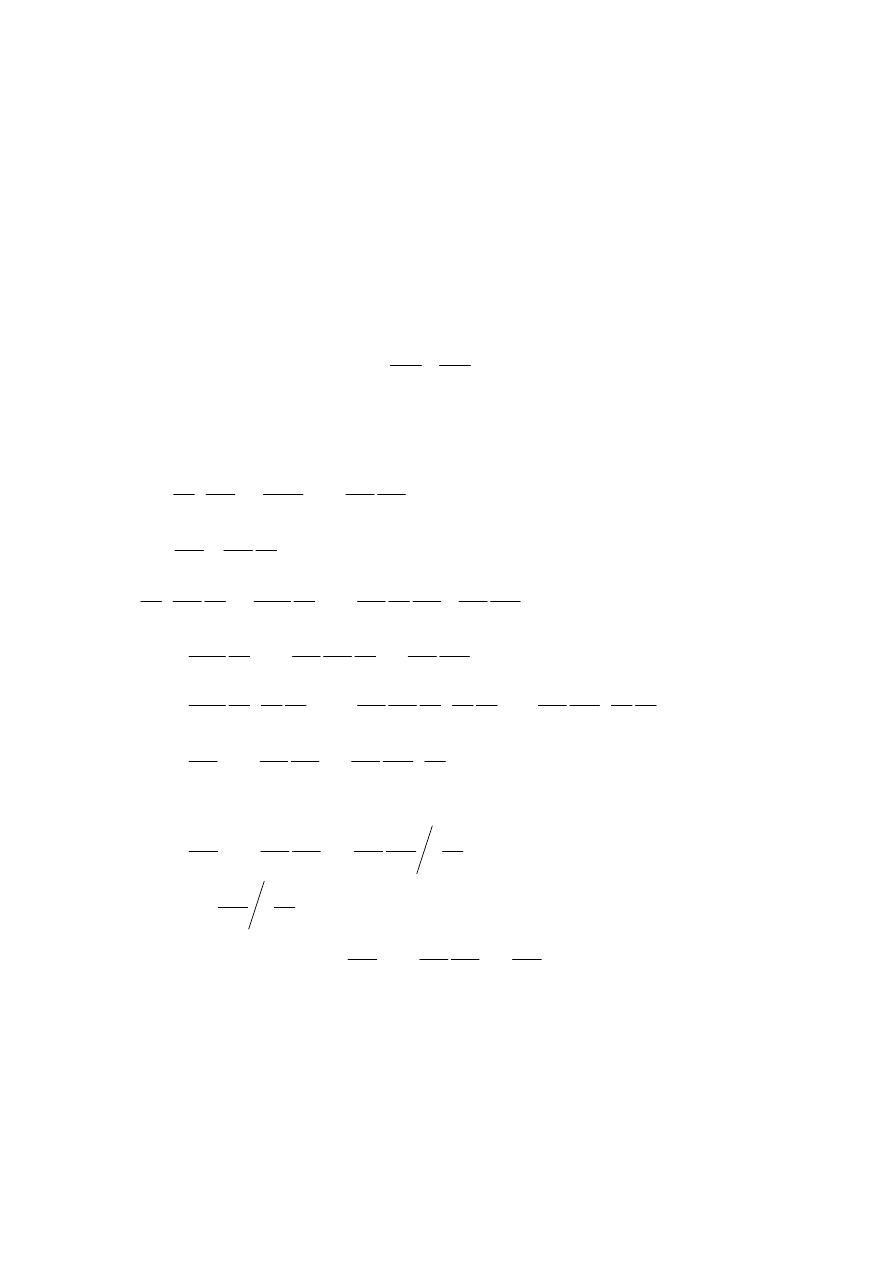
GENERAL RELATIVITY, TENSOR ANALYSIS AND GEOMETRY
Courtney James Mewton
Page 2
GR, Tensor Analysis & Geometry
Block symmetry
klij
ijkl
R
R
=
Bianchi’s identity
0
=
+
+
iljk
iklj
ijkl
R
R
R
The Ricci Tensor
The Ricci tensor of the first kind is simply a contraction of the Riemann tensor:
k
ijk
ij
R
R
=
.
The last index can be raised to yield the Ricci tensor of the second kind:
ki
ik
j
i
R
g
R
=
.
If this tensor is finally contracted by letting I = j, we get the Ricci curvature scalar. If it is zero, the space is flat.
From the first of the two equations above the Ricci tensor of the first kind can be calculated directly by:
k
rk
r
ij
k
rj
r
ik
k
k
ij
j
k
ik
k
ijk
ij
x
x
R
R
Γ
Γ
−
Γ
Γ
+
∂
Γ
∂
−
∂
Γ
∂
=
=
.
Transformation Of A Geodesic From Parameter u To v, Where v = f(u)
Given a particular geodesic in terms of a parameter u, in this section the geodesic equation will be transformed
so that it is in terms of a new parameter v.
Start with
0
2
2
=
Γ
+
=
du
dx
du
dx
du
x
d
du
dx
du
D
c
b
a
bc
a
a
.
Substitute
du
dv
dv
dx
du
dx
a
a
=
,
then
0
2
2
=
∂
∂
+
∂
∂
Γ
+
∂
∂
∂
=
∂
∂
du
v
d
v
x
du
dx
du
dv
v
x
du
dv
v
u
x
du
dv
v
x
du
D
a
c
b
a
bc
a
a
2
2
du
v
d
v
x
du
dv
du
dx
v
x
du
dv
v
u
x
a
c
b
a
bc
a
∂
∂
−
=
∂
∂
Γ
+
∂
∂
∂
⇔
∂
∂
−
=
∂
∂
Γ
+
∂
∂
∂
⇔
dv
du
dv
du
du
v
d
v
x
dv
du
dv
du
du
dv
du
dx
v
x
dv
du
dv
du
du
dv
v
u
x
a
c
b
a
bc
a
2
2
2
2
2
2
∂
∂
−
=
∂
∂
Γ
+
∂
∂
⇔
dv
du
du
v
d
v
x
dv
dx
v
x
v
x
a
c
b
a
bc
a
.
Since the LHS is now in terms of v, partial differentiation can be replaced by normal differentiation:
2
2
2
2
−
=
Γ
+
⇔
du
dv
du
v
d
dv
dx
dv
dx
dv
dx
dv
dx
a
c
b
a
bc
a
.
By letting
2
2
2
−
=
du
dv
du
v
d
λ
, we arrive at the final equation:
dv
dx
dv
dx
dv
dx
dv
dx
a
c
b
a
bc
a
λ
=
Γ
+
2
.
General Relativity
The Metric Tensor for Special Relativity
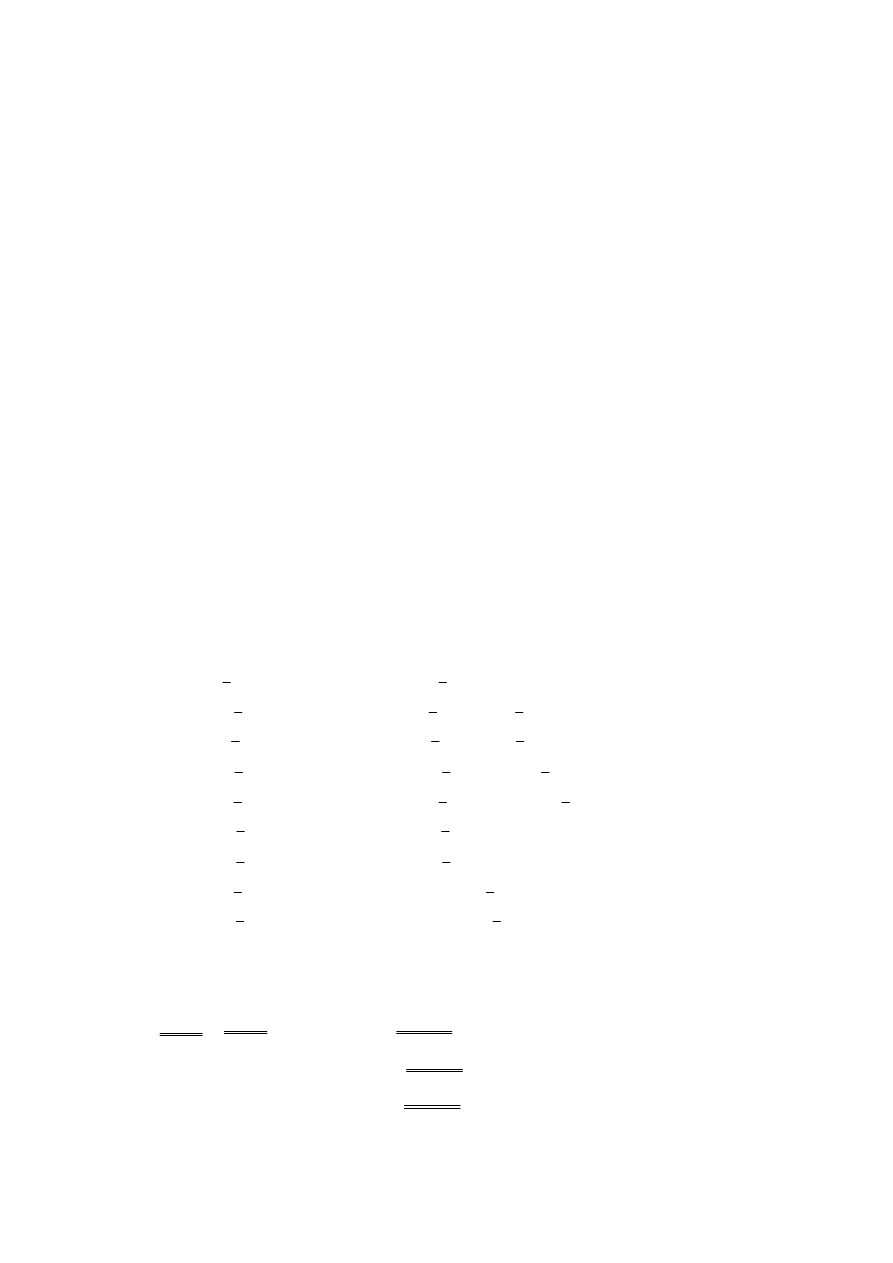
GENERAL RELATIVITY, TENSOR ANALYSIS AND GEOMETRY
Courtney James Mewton
Page 3
GR, Tensor Analysis & Geometry
−
−
−
=
1
0
0
0
0
1
0
0
0
0
1
0
0
0
0
1
µν
η
.
Einstein's Law of Gravitation
Simply stated, Einstein's law of gravitation is:
0
=
µν
R
.
This condition holds when the local space is completely devoid of all forms of matter and energy.
Derivation of the Schwarzchild Solution
In this section the line-element solution to the field equations for a quasi-static gravitational field produced by a
spherical body will be derived.
We start by setting up a general line element employing spherical coordinates:
(
)
2
2
2
2
2
2
2
2
2
sin
φ
θ
θ
τ
d
d
Wr
Bdr
dt
Ac
d
c
+
−
−
=
.
Before we continue, a few assumptions need to be made:
The space is asymptotically flat. This means that
1
→
=
B
A
as
∞
→
r
.
The gravitational field only affects time and radial distance, so W = 1.
We can immediately define the metric tensor:
2
00
Ac
g
=
,
B
g
−
=
11
,
2
22
r
g
−
=
and
θ
2
2
33
sin
r
g
−
=
.
Since we are dealing with the empty space surrounding the body, the Ricci tensor needs to equal zero. With this
in mind, the derivation begins. We first calculate the Christoffel symbols. Note that since a Christoffel symbol
of the second kind is defined as
mnr
rz
z
mn
g
mn
z
Γ
=
î
=
Γ
we need only calculate them for values when r = z.
[
]
A
A
g
g
g
c
A
g
′
=
−
+
=
Γ
=
Γ
−
−
−
1
2
1
0
,
10
0
,
10
1
,
00
2
1
2
1
100
00
0
10
[
]
2
1
2
1
1
,
00
1
2
1
1
,
00
0
,
01
0
,
10
1
2
1
001
11
1
00
c
A
B
g
B
g
g
g
B
g
′
=
=
−
+
−
=
Γ
=
Γ
−
−
−
[
]
B
B
g
B
g
g
g
B
g
′
=
−
=
−
+
−
=
Γ
=
Γ
−
−
−
1
2
1
1
,
11
1
2
1
1
,
11
1
,
11
1
,
11
1
2
1
111
11
1
11
[
]
( )
1
1
2
1
1
,
22
1
2
1
1
,
22
2
,
21
2
,
12
1
2
1
221
11
1
22
2
−
−
−
−
−
=
⋅
−
=
−
=
−
+
−
=
Γ
=
Γ
rB
r
B
g
B
g
g
g
B
g
[
]
(
)
(
)
θ
θ
2
1
2
1
2
1
1
,
33
1
2
1
1
,
33
3
,
31
3
,
13
1
2
1
331
11
1
33
sin
sin
2
−
−
−
−
−
=
−
=
−
−
=
−
+
−
=
Γ
=
Γ
rB
r
B
g
B
g
g
g
B
g
[
]
( )
1
2
2
1
2
,
21
1
,
22
2
,
21
2
2
1
212
22
2
21
2
−
−
−
=
−
⋅
−
=
−
+
−
=
Γ
=
Γ
r
r
r
g
g
g
r
g
[
]
θ
θ
θ
θ
sin
cos
sin
cos
2
2
2
2
1
2
,
33
3
,
32
3
,
23
2
2
1
332
22
2
33
−
=
⋅
−
=
−
+
−
=
Γ
=
Γ
−
−
r
r
g
g
g
r
g
[
]
(
)
1
2
2
2
2
1
3
,
13
3
,
13
1
,
33
2
2
2
1
133
33
3
13
sin
2
sin
sin
−
−
−
−
−
=
−
⋅
−
=
−
+
−
=
Γ
=
Γ
r
r
r
g
g
g
r
g
θ
θ
θ
[
]
(
)
θ
θ
θ
θ
θ
cot
sin
cos
2
sin
sin
2
2
2
2
1
3
,
23
3
,
23
2
,
33
2
2
2
1
233
33
3
23
=
−
⋅
−
=
−
+
−
=
Γ
=
Γ
−
−
−
−
r
r
g
g
g
r
g
We now solve the field equations:
[
(
) (
) (
) (
)
]
[
(
) (
)
(
) (
)
]
[
(
)
(
)
(
) (
)
]
[
(
)
(
)
(
) (
)
]
3
33
3
00
3
30
3
03
3
23
2
00
3
20
2
03
3
13
1
00
3
10
1
03
3
03
0
00
3
00
0
03
3
3
,
00
3
0
,
03
2
32
3
00
2
30
3
02
2
22
2
00
2
20
2
02
2
12
1
00
2
10
1
02
2
02
0
00
2
00
0
02
2
2
,
00
2
0
,
02
1
31
3
00
1
30
3
01
1
21
2
00
1
20
2
01
1
11
1
00
1
10
1
01
1
01
0
00
1
00
0
01
1
1
,
00
1
0
,
01
0
30
3
00
0
30
3
00
0
20
2
00
0
20
2
00
0
10
1
00
0
10
1
00
0
00
0
00
0
00
0
00
0
0
,
00
0
0
,
00
00
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
=
R

GENERAL RELATIVITY, TENSOR ANALYSIS AND GEOMETRY
Courtney James Mewton
Page 4
GR, Tensor Analysis & Geometry
(
) (
)
(
) (
)
]
[
(
) (
) (
) (
)
]
(
)
(
) (
)
(
)
]
(
)
(
)
(
)
(
)
]
3
33
3
11
3
31
3
13
3
23
2
11
3
21
2
13
3
13
1
11
3
11
1
13
3
03
0
11
3
01
0
13
3
3
,
11
3
1
,
13
2
32
3
11
2
31
3
12
2
22
2
11
2
21
2
12
2
12
1
11
2
11
1
12
2
02
0
11
2
01
0
12
2
2
,
11
2
1
,
12
1
31
3
11
1
31
3
11
1
21
2
11
1
21
2
11
1
11
1
11
1
11
1
11
1
01
0
11
1
01
0
11
1
1
,
11
1
1
,
11
0
30
3
11
0
31
3
10
0
20
2
11
0
21
2
10
0
10
1
11
0
11
1
10
0
00
0
11
0
01
0
10
0
0
,
11
0
1
,
10
11
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
=
R
[
(
)
(
)
(
) (
)
]
[
(
)
(
) (
)
(
)
]
[
(
)
(
)
(
) (
)
]
[
(
)
(
)
(
)
(
)
]
3
33
3
22
3
32
3
23
3
23
2
22
3
22
2
23
3
13
1
22
3
12
1
23
3
03
0
22
3
02
0
23
3
3
,
22
3
2
,
23
2
32
3
22
2
32
3
22
2
22
2
22
2
22
2
22
2
12
1
22
2
12
1
22
2
02
0
22
2
02
0
22
2
2
,
22
2
2
,
22
1
31
3
22
1
32
3
21
1
21
2
22
1
22
2
21
1
11
1
22
1
12
1
21
1
01
0
22
1
02
0
21
1
1
,
22
1
2
,
21
0
30
3
22
0
32
3
20
0
20
2
22
0
22
2
20
0
10
1
22
0
12
1
20
0
00
0
22
0
02
0
20
0
0
,
22
0
2
,
20
22
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
=
R
[
(
)
(
)
(
) (
)
]
[
(
)
(
)
(
)
(
)
]
[
(
)
(
)
(
)
(
)
]
[
(
) (
) (
) (
)
]
3
33
3
33
3
33
3
33
3
23
2
33
3
23
2
33
3
13
1
33
3
13
1
33
3
03
0
33
3
03
0
33
3
3
,
33
3
3
,
33
2
32
3
33
2
33
3
32
2
22
2
33
2
23
2
32
2
12
1
33
2
13
1
32
2
02
0
33
2
03
0
32
2
2
,
33
2
3
,
32
1
31
3
33
1
33
3
31
1
21
2
33
1
23
2
31
1
11
1
33
1
13
1
31
1
01
0
33
1
03
0
31
1
1
,
33
1
3
,
31
0
30
3
33
0
33
3
30
0
20
2
33
0
23
2
30
0
10
1
33
0
13
1
30
0
00
0
33
0
03
0
30
0
0
,
33
0
3
,
30
33
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
−
Γ
=
R
We are left with
3
13
1
00
2
12
1
00
1
11
1
00
1
00
0
01
1
1
,
00
00
Γ
Γ
−
Γ
Γ
−
Γ
Γ
−
Γ
Γ
+
Γ
−
=
R
,
3
31
3
13
3
13
1
11
3
1
,
13
2
21
2
12
2
12
1
11
2
1
,
12
0
10
1
11
0
01
0
10
0
1
,
10
11
Γ
Γ
+
Γ
Γ
−
Γ
+
Γ
Γ
+
Γ
Γ
−
Γ
+
Γ
Γ
−
Γ
Γ
+
Γ
=
R
,
3
32
3
23
3
13
1
22
3
2
,
23
1
22
2
21
1
11
1
22
1
1
,
22
0
10
1
22
22
Γ
Γ
+
Γ
Γ
−
Γ
+
Γ
Γ
+
Γ
Γ
−
Γ
−
Γ
Γ
−
=
R
,
2
33
3
32
2
12
1
33
2
2
,
33
1
33
3
31
1
11
1
33
1
1
,
33
0
10
1
33
33
Γ
Γ
+
Γ
Γ
−
Γ
−
Γ
Γ
+
Γ
Γ
−
Γ
−
Γ
Γ
−
=
R
.
These equations must equal zero, thus after substitution, we have:
B
r
A
A
A
A
A
B
A
B
R
1
4
2
4
00
′
−
′
′
+
′′
−
′
′
=
,
(1)
Br
A
B
B
B
A
A
A
A
R
′
−
′
′
−
′′
+
′
−
=
4
2
4
2
11
,
(2)
1
1
2
2
2
22
−
+
′
−
′
=
B
B
r
B
AB
r
A
R
,
(3)
θ
θ
2
22
2
2
33
sin
sin
1
1
2
2
R
B
B
r
B
AB
r
A
R
=
−
+
′
−
′
=
.
(4)
Equation 2 becomes:
B
B
A
A
A
A
Br
A
B
Br
A
B
B
B
A
A
A
A
4
2
4
4
2
4
0
2
2
′
′
−
′′
+
′
−
=
′
⇒
′
−
′
′
−
′′
+
′
−
=
.
This can be substituted into equation 1 to give:
A
A
B
B
r
A
Br
A
B
B
r
A
Br
A
B
′
−
=
′
⇒
′
−
=
′
⇒
′
−
′
−
=
1
0
.
This can also be written as:

GENERAL RELATIVITY, TENSOR ANALYSIS AND GEOMETRY
Courtney James Mewton
Page 5
GR, Tensor Analysis & Geometry
1
1
=
⇒
=
⇒
−
=
⇔
−
=
AB
A
B
A
dA
B
dB
Adr
dA
Bdr
dB
,
upon solving the simple differential equation. We use this solution to simplify equation 3:
( )
′
=
′
+
′
=
+
′
=
⇔
−
+
′
+
′
=
Ar
r
A
r
A
A
r
A
A
A
r
A
r
A
1
1
2
2
0
We now integrate:
( )
Ar
k
r
dr
Ar
dr
d
dr
=
+
⇒
=
∫
∫
,
where k is an integration constant. The equation can be rearranged to find A:
r
k
A
+
=
1
.
Since
1
−
=
A
B
,
1
1
−
+
=
r
k
B
.
The value of k is the last thing to obtain. In the next section on the approximation of Newtonian gravitation,
00
00
1 h
g
+
=
. It can immediately be seen that the
00
h
is equivalent to k. In the Newtonian approximation, it
is required that the Newtonian gravitational potential
00
2
2
1
h
c
V
=
. Using the Newtonian potential
r
GM
V
−
=
, this gives a value of
2
2
rc
GM
k
−
=
. By substituting A and B back into the original line-
element equation at the start of this section, we have the Schwarzchild solution:
(
)
2
2
2
2
2
1
2
2
2
2
2
2
sin
2
1
2
1
φ
θ
θ
τ
d
d
r
dr
rc
GM
dt
c
rc
GM
d
c
+
−
−
−
−
=
−
.
Utilising Geodesic Equation to Find GR Approximation of Newtonian Gravity
A particle travels through spacetime along a geodesic, given by the equation:
0
2
=
Γ
+
τ
τ
τ
σ
ν
µ
νσ
µ
d
dx
d
dx
d
dx
.
τ
is the time experienced relative to the particle. The equation simply states that relative to a free particle, it
experiences no net acceleration (though other objects appear to accelerate if a gravitational field is present).
We wish to determine the motion of the particle relative to coordinate time, denoted by t. The equation would
give the path of the particle in accordance to what other observers would see if they thought they were in a
gravitational field.
With the above said, the following equation can be immediately written, transforming from proper time to
coordinate time:
dt
dx
d
dt
d
t
d
dt
dx
dt
dx
dt
x
d
µ
σ
ν
µ
νσ
µ
τ
τ
=
Γ
+
2
2
2
2
2
2
.
First, we expand and consider its spatial components:
dt
dx
d
dt
d
t
d
dt
dx
dt
dx
dt
dx
dt
dx
dt
dx
dt
dx
dt
x
d
i
i
k
i
k
k
j
i
jk
i
=
Γ
+
Γ
+
Γ
+
2
2
2
2
0
0
00
0
0
2
2
2
τ
τ
.
One of the Christoffel symbols has a coefficient of two since
dt
dx
dt
dx
dt
dx
dt
dx
k
i
k
j
i
j
0
0
0
0
Γ
=
Γ
. We simplify the
equation:
dt
dx
d
dt
d
t
d
c
dt
dx
c
dt
dx
dt
dx
dt
x
d
i
i
k
i
k
k
j
i
jk
i
=
Γ
+
Γ
+
Γ
+
2
2
2
2
00
2
0
2
2
2
τ
τ
.
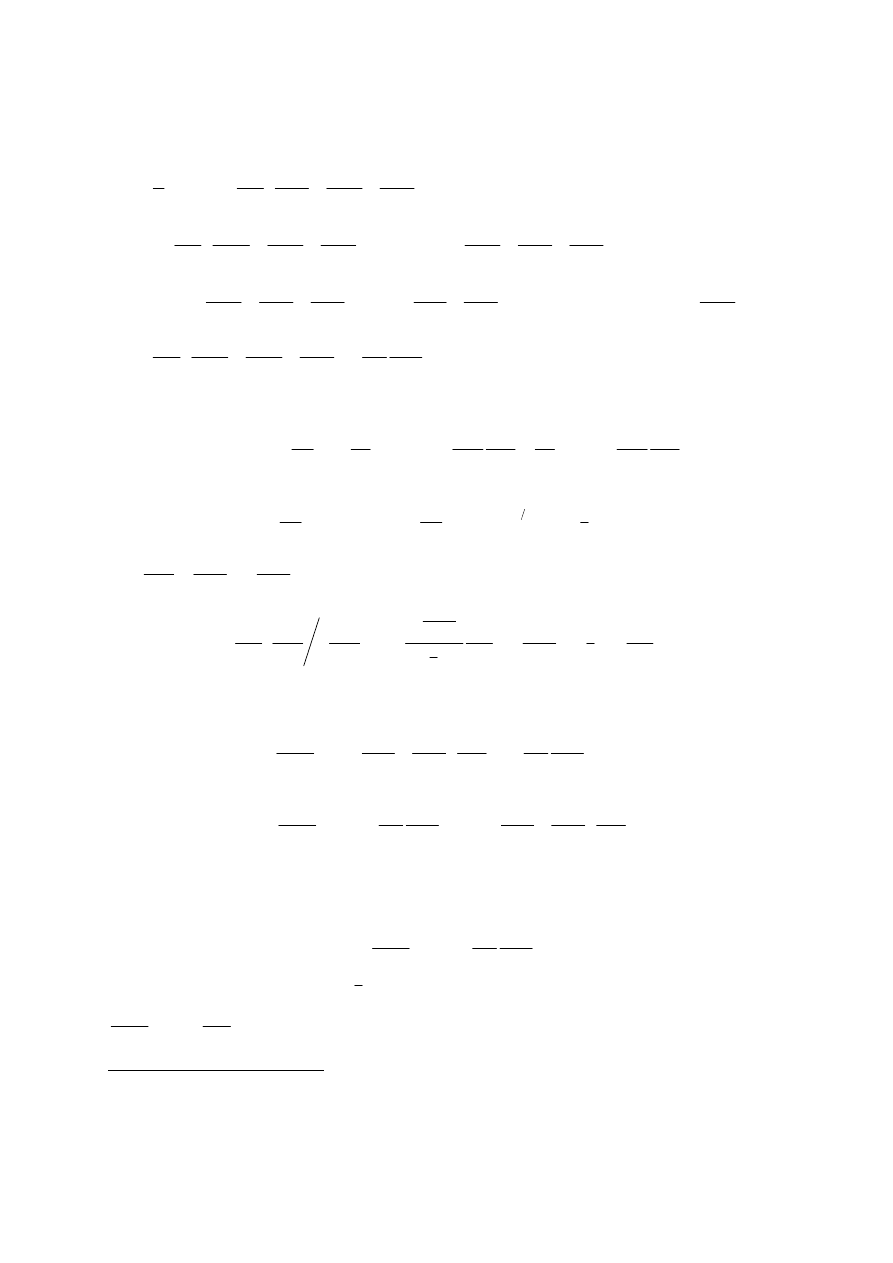
GENERAL RELATIVITY, TENSOR ANALYSIS AND GEOMETRY
Courtney James Mewton
Page 6
GR, Tensor Analysis & Geometry
We assume that the gravitational field is quasi-static, i.e. that it doesn't change with respect to time. Therefore,
any derivatives of the metric tensor with respect to time can be left out. Now we evaluate the connection
coefficients:
∂
∂
−
∂
∂
+
∂
∂
=
Γ
=
Γ
ρ
ρ
ρ
ρ
ρ
ρ
x
g
x
g
x
g
g
g
jk
j
k
k
j
i
jk
i
i
jk
2
2
1
. These derivatives are quite small, and can be neglected.
1
(
)
∂
∂
−
∂
∂
+
∂
∂
+
≈
∂
∂
−
∂
∂
+
∂
∂
=
Γ
ρ
ρ
ρ
ρ
ρ
ρ
ρ
ρ
ρ
η
x
h
x
h
x
h
h
x
g
x
g
x
g
g
k
k
k
i
i
k
k
k
i
i
k
0
0
0
0
0
0
0
2
2
2
(
)
∂
∂
−
∂
∂
−
≈
∂
∂
−
∂
∂
+
∂
∂
+
≈
s
k
k
s
is
k
k
k
i
i
x
h
x
h
x
h
x
h
x
h
h
0
0
0
0
0
δ
η
ρ
ρ
ρ
ρ
ρ
on neglecting terms involving
0
x
h
∂
∂
µν
.
z
iz
i
i
x
h
x
g
x
g
x
g
g
∂
∂
≈
∂
∂
−
∂
∂
+
∂
∂
=
Γ
00
00
0
0
0
0
00
2
2
δ
κ
κ
κ
κ
.
Now we need to evaluate the RHS of the equation. We start by first looking at the following line element:
(
)
(
)
dt
dx
dt
dx
h
c
dt
dx
dt
dx
h
c
dt
d
dx
dx
g
d
c
ν
µ
µν
ν
µ
µν
µν
ν
µ
µν
η
τ
τ
+
=
+
=
⇔
=
1
1
1
2
2
2
2
2
. Neglecting
terms involving the spatial components, which are small in comparison to the temporal components, we get:
(
)
(
)
(
)
00
2
1
2
1
00
00
2
1
1
1
h
h
dt
d
h
dt
d
+
≈
+
=
⇒
+
=
τ
τ
.
Now
0
00
00
2
2
dx
dh
c
dt
dh
dt
d
=
=
τ
, so the RHS of our major equation becomes:
(
)
dt
dx
h
dx
dh
c
dt
dx
h
dx
dh
c
d
dt
d
t
d
dt
dx
i
i
i
00
2
1
0
00
00
2
1
0
00
2
2
2
2
1
1
−
≈
+
≈
τ
τ
.
This is negligible since it involves temporal derivatives of the gravitational field.
By plugging everything into our equation, we get:
0
2
00
2
0
0
2
2
=
∂
∂
+
∂
∂
−
∂
∂
−
z
iz
k
s
k
k
s
is
i
x
h
c
dt
dx
x
h
x
h
dt
x
d
δ
δ
.
Through multiplying by mass m and rearranging the equation, we get:
dt
dx
x
h
x
h
m
x
h
mc
dt
x
d
m
k
s
k
k
s
is
z
iz
i
∂
∂
−
∂
∂
+
∂
∂
−
=
0
0
00
2
2
2
2
δ
δ
.
The term on the left is the force that the particle appears to experience. The first term on the right is some kind
of potential of the field, since its temporal component is involved. The last term, which involves perpendicular
velocities, is indicative of some sort of Coriolis force. We are not interested in the Coriolis effects, so we shall
assume that we are in a non-rotating frame, so we get:
z
iz
i
x
h
mc
dt
x
d
m
∂
∂
−
=
00
2
2
2
2
δ
.
If we denote a potential by
00
2
2
1
h
c
V
=
, then the equation simply becomes
V
m
F
∇
−
=
, or
z
iz
i
x
V
dt
x
d
∂
∂
−
= δ
2
2
. We want the metric tensor to be flat when there is no gravitational field present. The
1
The metric tensor
µν
µν
µν
η
h
g
+
≈
, where
µν
η
is the familiar metric tensor of Special Relativity, and
µν
h
are small terms which include the action of any gravitational fields which may be present, and are small in
comparison to the
µν
η
in weak gravitational fields, as opposed to the awesome sucking power of a black hole!

GENERAL RELATIVITY, TENSOR ANALYSIS AND GEOMETRY
Courtney James Mewton
Page 7
GR, Tensor Analysis & Geometry
equation for the potential leads us to the expression
2
00
00
00
2
1
c
V
h
g
+
=
+
= η
. To finally get the actual
expression for the potential in terms of mass, we need to use the line element from the Schwarzchild solution.
The temporal component gives
r
GM
V
−
=
. With this expression, we can easily obtain an approximation of
the gravitational force:
2
r
GMm
V
m
F
−
=
∇
−
=
.
Field Equations in the Presence of Matter: The Poisson Approximation
Let us write the equation:
µν
µν
µν
κ
T
g
R
=
−
2
1
,
or, more compactly as:
µν
µν
κ
T
G
=
,
where G is the Einstein tensor.
As a test for General Relativity, at velocities which are small in comparison to the speed of light, there must be
an approximation to Poisson’s equation:
ρ
π
G
V
4
2
=
∇
. To achieve this requires the weak field
approximation by leaving out negligible terms in the Ricci tensor.
2
µν
µν
κ
T
G
=
µν
µν
µν
κ
T
R
g
R
=
−
2
1
µν
µν
µν
µν
µν
µν
κ
g
T
R
g
g
R
g
=
−
2
1
µν
µν
κ
g
T
R
R
=
−
2
µν
µν
κ
g
T
R
−
=
∴
We substitute this back into our original equation:
µν
µν
µν
µν
µν
µν
µν
κ
κ
κ
g
T
g
T
R
g
T
R
2
1
2
1
−
=
+
=
We are approximating that the material energy tensor has a negligible value in for all
µ
,
ν
except when
µ
=
ν
=
0, so we get:
00
2
1
00
00
00
2
1
00
00
T
g
T
g
T
R
κ
κ
κ
=
−
=
.
We now make the Ricci tensor covariant:
00
2
1
00
00
00
00
2
1
00
00
00
T
R
g
g
T
R
g
g
κ
κ
=
⇔
=
Weak field approximation.
(
)
αα
µν
αα
µν
να
µα
µα
να
µν
αα
µν
,
2
1
,
,
,
,
2
1
g
g
g
g
g
R
≈
+
−
−
≈
V
c
g
g
R
2
2
00
2
2
1
,
00
2
1
00
1
∇
=
∇
≈
≈
∴
αα
0
0
2
1
00
2
1
2
2
1
v
v
T
V
c
κρ
κ
=
=
∇
∴
The particles are traveling at the travelling at the speed of light through time, so we get:
4
2
1
2
c
V
κρ
=
∇
This must equal Poisson’s equation, stated earlier
This gives a value
4
8
c
G
π
κ =
.
2
Note that this particular calculation would be shorter if we took the Einstein tensor and the material energy
tensor to be covariant as opposed to contravariant, but due to the actual form of the material energy tensor, I
prefer it to be contravariant.
Wyszukiwarka
Podobne podstrony:
Quaternion Math (small article) WW
Math Differential Geometry Tensor Analysis In Differentiable Manifolds
501 Geometry Questions (LearningExpress, 2002) WW
Vector Calculus [small handout] J Dougherty (1998) WW
Classical Geometry (Harvard lecture notes) WW
Real Analysis, Quantitative Topology, and Geometric Complexity S Semmes (2000) WW
A Geometric Approach to Differential Forms D Bachman (2003) WW
Geometric Approach to Differential Forms D Bachman (2003) WW
Intro to Braided Geometry & Q Minkowski Space [jnl article] S Majid (1994) WW
geometria w płaszczyźnie
GEOMETRIA
GEOMETRIA OBLICZENIOWA I
lec6a Geometric and Brightness Image Interpolation 17
Geometria wykreślna Ćwiczenie 8
więcej podobnych podstron