Modelling the inactivation of Bacillus

subtilis spores by ethylene oxide processing

Gisela Cristina Mendes

Teresa Ribeiro da Silva Brandão

Cristina Luisa Miranda Silva

CBQF - Centro de Biotecnologia e Química Fina

Escola Superior de Biotecnologia

Universidade Católica Portuguesa,

Rua Dr. António Bernardino de Almeida

4200-072 Porto, Portugal

This study was supported by Bastos Viegas, S.A.

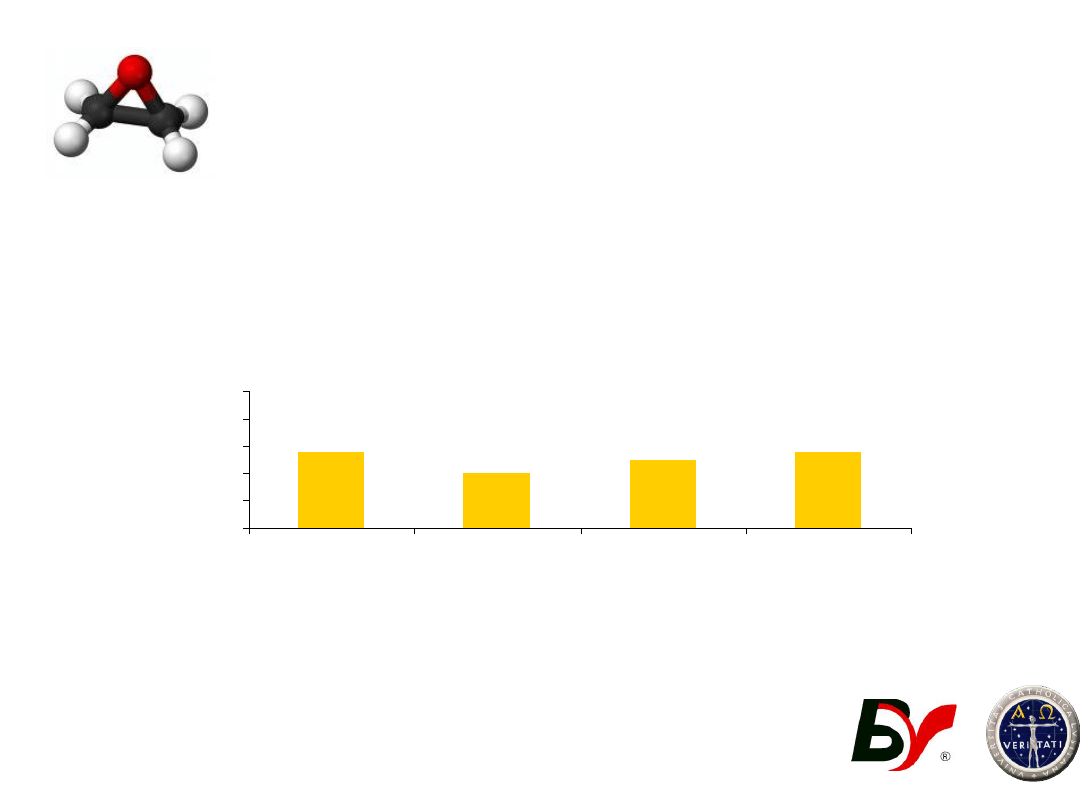
ETHYLENE OXIDE IS CURRENTLY A

DOMINANT STERILIZATION AGENT USED

IN MEDICAL DEVICES INDUSTRY

EO sterilization consumption for

medical devices

0

20

40

60

80

100

70

's

80

's

90

's

No

wa

da

ys

Decade

%

Advantages / Disadvantages



Advantages

Effectiveness Diffusivity

Bactericidal, fungicidal and virucidal properties

Compatibility with most materials

Process flexibility

Low temperature sterilization



Disadvantages

Toxicity of the sterilizing agent

Process complexity

Process cost

Processing time

Advantages / Disadvantages

Objectives

Understanding the full dynamics of the sterilization allows

design optimization / efficient control of the process -

Parametric release

Screen the most significant

variables on B. subtilis

inactivation by EO sterilization

Model the inactivation kinetics

of B. subtilis, including

the variables’ effects

Provide a method of

integrating lethality

Modelling microorganisms inactivation

Experimental design

Bacillus subtilis, var. niger or Bacillus atrophaeus spores (ATCC

9372) inoculated in strips (biological indicators, BIs)

Matrix: Drapes

Temperature and humidity sensors

EO sensor (

Infrared analyser in the sterilizer chamber headspace

)

Sterilization cycles

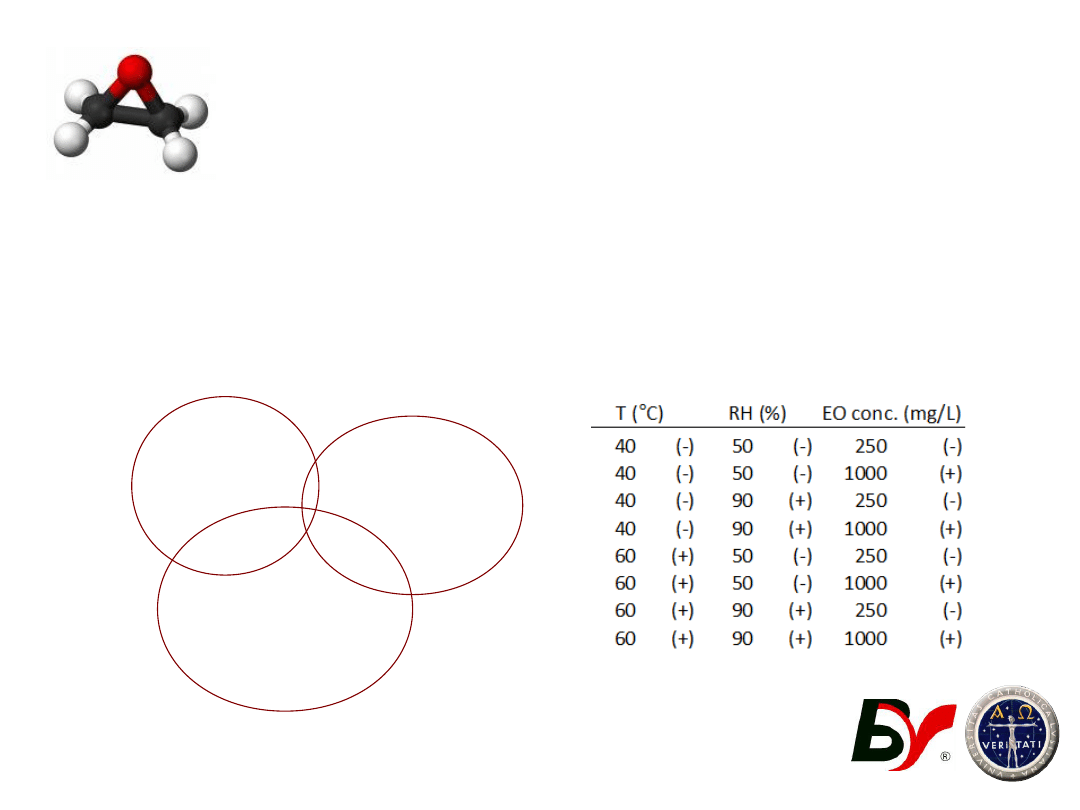
Modelling microorganisms inactivation

- Conditions defined according to the 2

3

factorial design -

Sterilization cycles

- Target exposure conditions –

40 and 60 ºC 50 and 90 %RH

250 and 1000

mg(EO)/L

Modelling microorganisms inactivation

Survival curves construction
1

st

order kinetics

Gompertz model

Gompertz function has the ability of modelling
both linear and asymmetrical sigmoidal data

(

)

























+

−

λ

−

−

⋅

=









1

t

A

e

k

exp

exp

A

N

N

log

max

0

0

N

log

t.

k

N

log

+

−

=

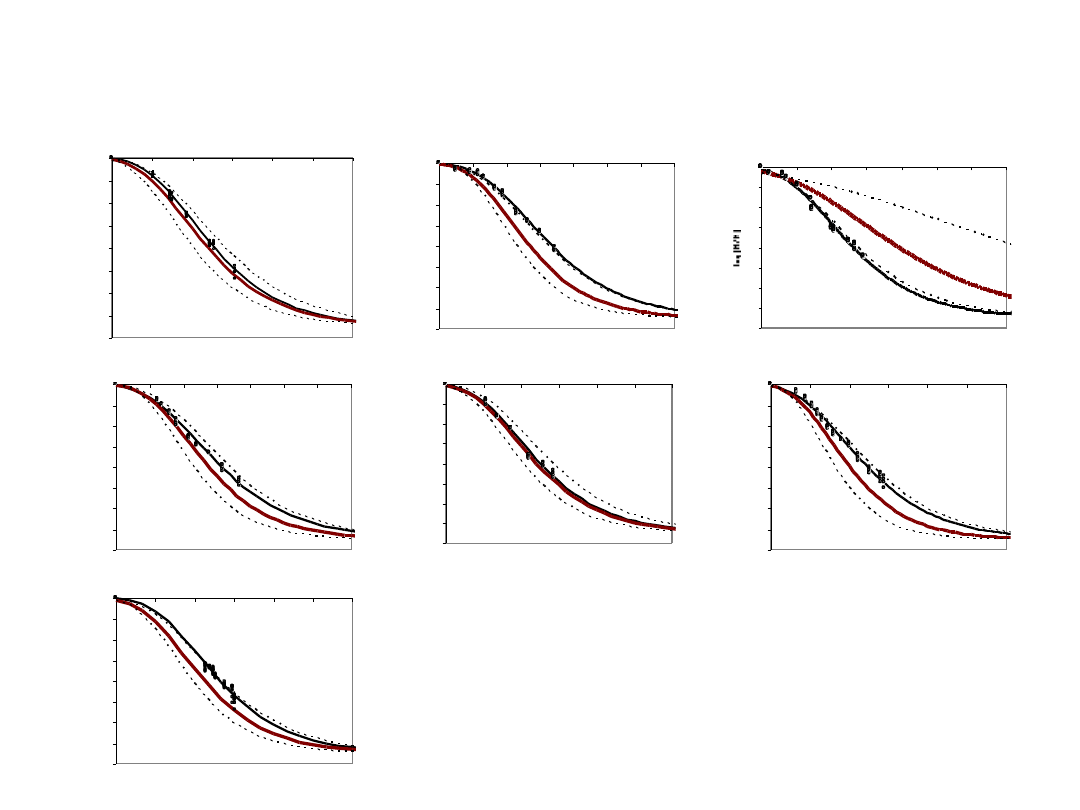
Inactivation of B. subtilis spores by EO sterilization

- Conditions defined according to the 2

3

factorial design -

-8

-7

-6

-5

-4

-3

-2

-1

0

0

500

1000

1500

2000

2500

3000

U (s)

lo

g

(

N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

1000

2000 3000 4000 5000 6000 7000

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

2000

4000

6000

8000

10000 12000 14000

U (s)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

500

1000

1500 2000 2500 3000 3500

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

500

1000

1500

2000

2500

3000

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

1000

2000

3000

4000

5000

6000

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

300

600

900

1200

1500

1800

U (s)

lo

g

(N

/N

0

)

Legend
⁰ Experimental data
___ Fitted Gompertz model

___

Predicted data

- - - Upper and lower limits of predicted data (considering the

maximum fluctuations of temperature and EO concentration)

T=60 °C; EO=233 mg/L;
RH=63 %

T=44 °C; EO=257 mg/L;

RH=86 %

T=34 °C; EO=222 mg/L;

RH=60 %

T=40 °C; EO=980 mg/L;
RH=90 %

T=59 °C; EO=266 mg/L;

RH=85 %

T=33 °C; EO=940 mg/L;

RH=61 %

T=59 °C; EO=1004 mg/L;

RH=98 %

Data analysis

The

non-linear regression analysis

was carried in

Statistica© 6.0 software (StatSoft, USA), using the Levenberg-
Marquardt algorithm to minimize the sum of the squares of the
differences between the predicted and experimental values.

Data analysis

The experimental inactivation data were successfully fitted with
the Gompertz model:

- High precision of k

max

and

λ

estimates, since low errors were

attained (SHW

95%

);

- Residuals randomness and normality;
- Coefficient of determination (R

2

>0.98);

Data analysis and planning future work

The analysis of variance (ANOVA) allowed to identify the most
significant parameters affecting B. subtilis inactivation -
temperature and EO concentration

Additional experiments considering intermediate conditions of
these parameters were defined in order to model their effects
and combined effects on the lethality (runs 9 to 15)

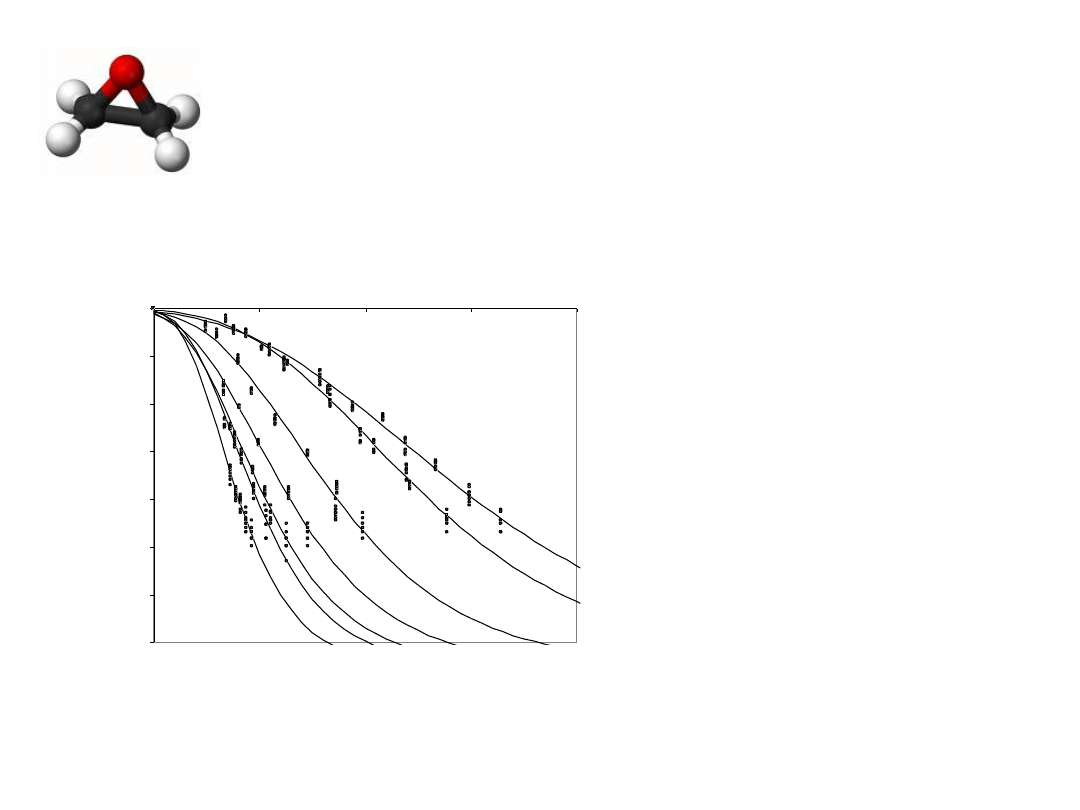
Inactivation of B. subtilis spores by EO sterilization at

the additional experimental conditions

-7

-6

-5

-4

-3

-2

-1

0

0

1000

2000

3000

4000

U (s)

lo

g

(

N

/N

0

)

Legend

⁰ Experimental data

___ Fitted Gompertz model

Run 9 to Run 15

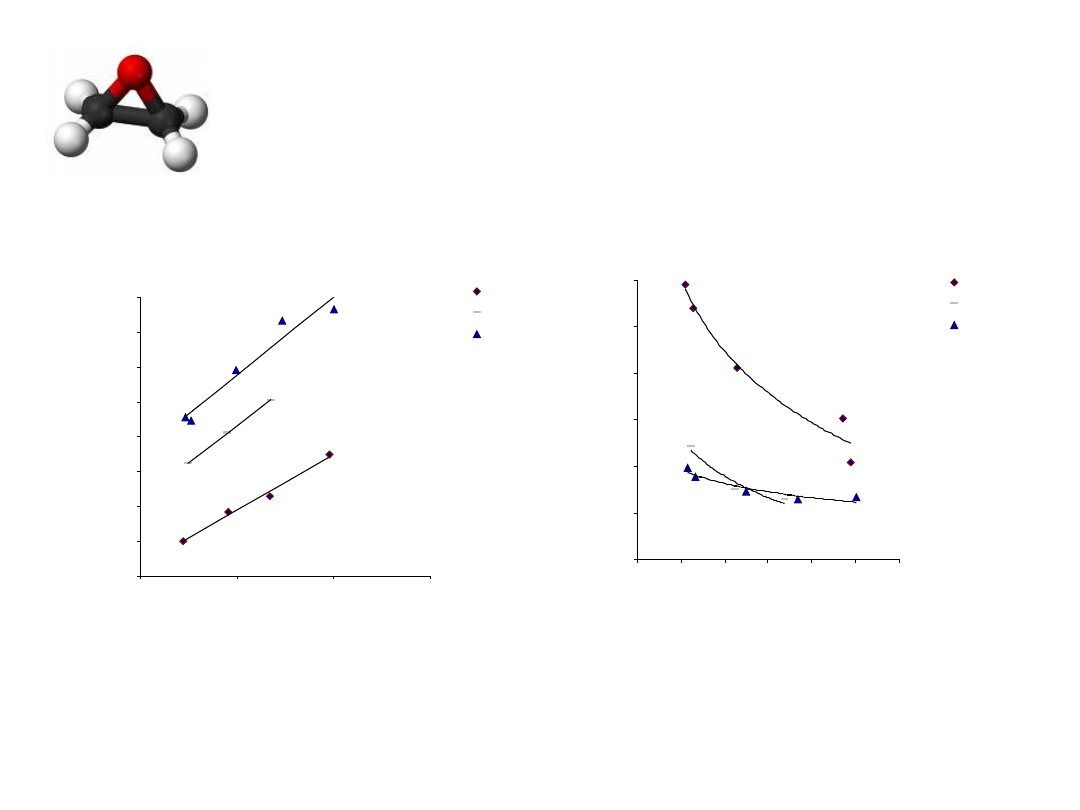
Estimated k

max

and l parameters of B. subtilis inactivation at the

temperature, EO concentration and relative humidity conditions tested

EO concentration influence on k

max

and

λ

k

max

= 3.22x10

-6

[EO] + 2.74x10

-4

k

max

= 4.25x10

-6

[EO] + 2.18x10-3

k

max

= 4.46x10

-6

[EO] + 3.53x10

-3

0

1

2

3

4

5

6

7

8

0

500

1000

1500

[EO] (mg/L)

k

m

a

x

x

1

0

3

(s

-1

)

37.0 ºC

50.5 ºC

60.0 ºC

λ

= -88.076ln[EO] + 853.05

λ

= -229.66ln[EO] + 1733.7

λ

= -446.4ln[EO] + 3570.8

0

200

400

600

800

1000

1200

0

200

400

600

800

1000

1200

[EO] (mg/L)

λ

(s

)

38.0 ºC

50.5 ºC

60.0 ºC

Influence of EO concentration on k

max

at

37.0

,

50.5

e

60.0

°C

Influence of EO concentration on

λ

at

38.0

,

50.5

and

60.0

°C

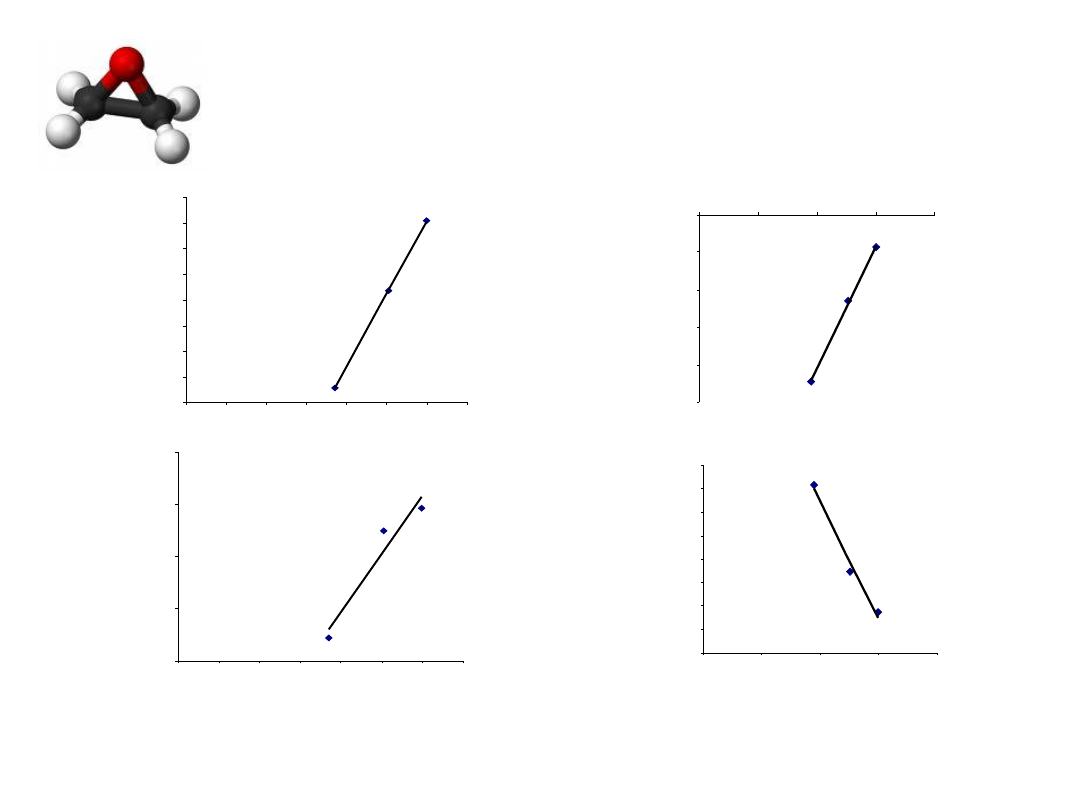
T influence on parameters

a

k

/

b

k

and

a

λ

/

b

λ

a

k

= 1.42x10

-4

T - 4.96x10

-3

0.0

0.5

1.0

1.5

2.0

2.5

3.0

3.5

4.0

0

10

20

30

40

50

60

70

T (ºC)

a

k

x

1

0

3

(

s

-1

)

b

k

= 5.54x10

-8

T + 1.25x10

-6

3.0

3.5

4.0

4.5

5.0

0

10

20

30

40

50

60

70

T (ºC)

b

k

x

1

0

6

(

L

m

g

-1

s

-1

)

a

λ

= 16.34T - 1063.61

-500

-400

-300

-200

-100

0

0

20

40

60

80

T (ºC)

a

λ

(

s

)

b

λ

= -124.74T + 8227.00

0

500

1000

1500

2000

2500

3000

3500

4000

0

20

40

60

80

T (ºC)

b

λ

(

s)

Influence of T on

a

k

e

b

k

parameters

Influence of T on

a

k

e

b

k

parameters

Data analysis

T and EO concentration have a negative effect on

λ

and a

positive effect on k

max

:

- Higher temperatures and EO concentration imply narrow
shoulder times and higher inactivation rates;
- Lower inactivation rates and more evident shoulder phases

were observed at the lowest temperature and EO
concentration;

Mathematical model resulting from the integration

of the T and EO concentration parameters for

lethality calculation of the EO sterilization process

[ ]

























×

















−

×

+

−

×

+









−

×

−

−

×

−

−

−

=















7.5

-

e

EO

6

10

1.25

T

8

10

5.54

3

10

4.96

T

4

10

1.42

exp

7.5)exp

(

0

N

N

log

[ ]













+









−









×

+

×

−

+









×

−

×

×

1

U

3

10

8.23

T

2

10

1.25

)

EO

ln(

3

10

1.06

T

1

10

1.63

Inactivation of B. subtilis spores by EO sterilization

- Conditions defined according to the 2

3

factorial design -

-8

-7

-6

-5

-4

-3

-2

-1

0

0

500

1000

1500

2000

2500

3000

U (s)

lo

g

(

N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

1000

2000 3000 4000 5000 6000 7000

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

2000

4000

6000

8000

10000 12000 14000

U (s)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

500

1000

1500 2000 2500 3000 3500

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

500

1000

1500

2000

2500

3000

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

1000

2000

3000

4000

5000

6000

U (s)

lo

g

(N

/N

0

)

-8

-7

-6

-5

-4

-3

-2

-1

0

0

300

600

900

1200

1500

1800

U (s)

lo

g

(N

/N

0

)

Legend
⁰ Experimental data
___ Fitted Gompertz model

___

Predicted data

- - - Upper and lower limits of predicted data (considering the

maximum fluctuations of temperature and EO concentration)

T=60 °C; EO=233 mg/L;
RH=63 %

T=44 °C; EO=257 mg/L;

RH=86 %

T=34 °C; EO=222 mg/L;

RH=60 %

T=40 °C; EO=980 mg/L;
RH=90 %

T=59 °C; EO=266 mg/L;

RH=85 %

T=33 °C; EO=940 mg/L;

RH=61 %

T=59 °C; EO=1004 mg/L;

RH=98 %

In conclusion

[ ]

























×

















−

×

+

−

×

+









−

×

−

−

×

−

−

−

=















7.5

-

e

EO

6

10

1.25

T

8

10

5.54

3

10

4.96

T

4

10

1.42

exp

7.5)exp

(

0

N

N

log

A mathematical inactivation model expressed only in terms of the
relevant process variables (T and EO concentration) was
achieved.

The conventional design of EO sterilization cycles usually involves
a significant amount of experimental work, which is time
consuming and also expensive. The results of this work are
certainly a contribution for an efficient control, design and
optimization of the EO sterilization process.

[ ]













+









−









×

+

×

−

+









×

−

×

×

1

U

3

10

8.23

T

2

10

1.25

)

EO

ln(

3

10

1.06

T

1

10

1.63

Thanks’