Biomaterials 21 (2000) 1461}1469

Adhesion of bioactive glass coating to Ti6Al4V oral implant

J. Schrooten*, J.A. Helsen

Department of Metallurgy & Materials Engineering, Katholieke Universiteit Leuven, de Croylaan 2, B-3001 Leuven, Belgium

Received 8 September 1999; accepted 18 January 2000

Abstract

Bioactive glass (BAG) is a bioactive material with a high potential as implant material. Reactive plasma spraying produces an

economically feasible BAG-coating for Ti6Al4V oral implants. This coating is only functional if it adheres well to the metal substrate
and if it is strong enough to transfer all loads. To examine these two properties an appropriate mechanical adhesion test, the moment
test, is developed. This test quanti"es under a realistic loading condition the corresponding functional adhesion strength to be

'

84 MPa in tensile. To get a qualitative insight in the BAG-coating behavior during loading the mechanical test was combined with

"nite element analysis, acoustic emission and microscopic analysis. These analyses showed that the coating withstands without any

damage an externally generated tensile stress of 47 MPa. Not only the initial adhesion is determining for the implant quality, but more
important is the coating functionality after reaction of the BAG. Adhesion testing after two months of in vitro reaction in a simulated
body #uid showed that coating adhesion strength decreased with 10%, but the implant system was still adequate for load-bearing
applications.

2000 Elsevier Science Ltd. All rights reserved.

Keywords: Bioactive glass; Coating; Adhesion test; Oral implant; Acoustic emission; In vitro

1. Introduction

The search for better implant "xation is evolving from

a mechanical "xation by screws or cement towards a
physico-chemical bond. The latter is established by bi-
oactive materials, in most cases ceramics, that react in
a compatible way with the surrounding tissue, thus cre-
ating a tight bond [1}3]. Due to their limited mechanical
properties bioactive ceramics cannot be used as a bulk
material in load-bearing applications [1]. To avoid this
restriction metal implants are coated with a bioactive
layer. During the last 10 yr coating medical implants
became a &hot topic' in the biomaterials research. One of
the most accepted and commercialized bioactive coating
materials is thermally sprayed hydroxyapatite (HA)
[4,5]. These HA-coated implants still have some un-
avoidable drawbacks and no major improvements in the
HA research can be expected [6]. Thus, a search for new
or improved techniques or new materials is required.
Among all bioactive materials bioactive glass (BAG), that
consists of a family of glass compositions that bond in

* Correspondence address: de Croylaan 2, B-3001 Leuven, Belgium.

Tel.: #[phone]; fax: #[phone].

E-mail address: [e-mail] (J. Schrooten).

a few hours to hard and soft tissue, shows the best-known
bioactive behavior [7,8]. Like all bioactive ceramics the
brittleness of BAG limits the use of bulk-BAG to a few,
non-load-bearing applications [9]. Due to very high pro-
duction cost no commercial BAG-coatings are available.
Recently however, our laboratory developed an eco-
nomically feasible BAG-coating production process, re-
active plasma spraying, that transforms crystalline-base
products during plasma spraying into a glass-coating
[10]. The new technique was "rst applied to coat
Ti6Al4V oral implants with a 50

lm BAG-layer of the

following composition (in wt%): 52.0 SiO, 30.5 CaO, 9.8

NaO, 6.2 PO and 1.5 CaF.

Because the BAG-coating is interfacing the implant

and the surrounding tissue has to adhere well to both,
and maintain this adhesion over a long period of time. It
is known that the adhesion quality of BAG to bone is
su$cient to withstand all loads [11,12]. Moreover, the
BAG-coating has to form a substantial bond with the
metal substrate. To quantitatively measure the adhesion
strength of a coating a lot of adhesion tests exist [13].
Most tests supply results that are not representative for
the adhesion strength in a real implant [14]. It seems
reasonable to try to measure the adhesion strength under
conditions close to reality [14,15]. A "rst test of this kind
(shear test) was introduced, but it still contained some

0142-9612/00/$ - see front matter

2000 Elsevier Science Ltd. All rights reserved.

PII: S 0 1 4 2 - 9 6 1 2 ( 0 0 ) 0 0 0 2 7 - 2

Fig. 1. BAG-coated Ti6Al4V oral implant.

Fig. 2. BAG-coated Ti6Al4V test rod (A), shear test sample (B) and
moment test sample (C).

drawbacks [16]. This led to the newly proposed adhesion
test (moment test) that is based on the following rationale:
`An adhesion test should generate a realistic stress distri-
bution with one dominant stress component that reaches
its maximum at the interface of interest enabling the
calculation of a quantitatively reliable adhesion strength

a.

The mechanical test on its own can validate the ad-

hesion quality of the BAG-coating in a quantitative man-
ner. To fully understand the fracture behavior of the
BAG-coating additional quantitative and qualitative in-
formation about the coating behavior under loading is
needed. To simulate the real-stress conditions during
mechanical testing a "nite element analysis was per-
formed. The combination of acoustic emission during
loading [14,17,18] and microscopic analysis of the tested
and cross-sectioned samples can add information about
the failure mode of the plasma-sprayed BAG-coating.

The combination of realistic mechanical testing, FEA

and AE makes it possible to describe both in a quantita-
tive and qualitative way the adhesion strength of a plasma
sprayed BAG-coating [19,20]. The complete adhesion
testing was performed on as-sprayed BAG-coatings and
on two months in vitro reacted BAG-coatings. The latter
was carried out to predict the strength of BAG as func-
tion of the reaction time.

2. Materials and methods

Due to a too complicated implant geometry (Fig. 1) to

perform a quantitative adhesion test and in order to test
the adhesion of the BAG-coating in a representative way,
a simpli"ed geometry for the adhesion test samples
(Fig. 2A), based on that of the oral implant (Fig. 1), is
chosen. The oral and test substrate have the same ther-
mal mass to guarantee that both substrates will have
a comparable coating when using identical spraying
parameters.

2.1. Theoretical and practical set-up moment test

A realistic oral implant loading generates shear stres-

ses [14,15] instead of tensile stresses, like in most
adhesion tests. The coating}substrate interface of a
cylindrical implant can be subjected to a shear stress in
two ways (Fig. 3). One can generate a shear stress in the
plane of the interface in the direction of the central axis or
in a plane perpendicular to the central axis, along a cir-
cumferential direction. The former shear stress is gener-
ated in the shear test [16]. The latter can be generated by
applying a moment (M) using a force (F) and a cantilever
(R). The relation between the applied moment and the
resulting shear stress is the following

q"

FR

2

nrl

(1)

where r is the radius of the cylindrical sample and l the
height the tested surface.

Due to its relation to the radius (&1/r

), the maximum

shear stress is generated at the coating}substrate inter-
face. Using Eq. (1) to calculate the adhesion strength the
assumption is made that the stress distribution is homo-
geneous throughout the whole sample.

In practice the shear stress in the sample is generated in

the following way. A rectangular stainless-steel counter-
part with a central cylindrical hole is glued around the
sample. In the shear test the counterpart was "xed at one
end of the test sample (Fig. 2B) and both parts were
pulled in opposite directions. In the moment test the
counterpart is glued around the center of the sample,
leaving the end parts free (Fig. 2C). By fully constraining
both end parts and rotating the counterpart around the
central axis with the moment M by using an appropriate
cantilever (R"100 mm) the circular shear stress (

q)

is generated. The dimensions of the whole test sample are

1462

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

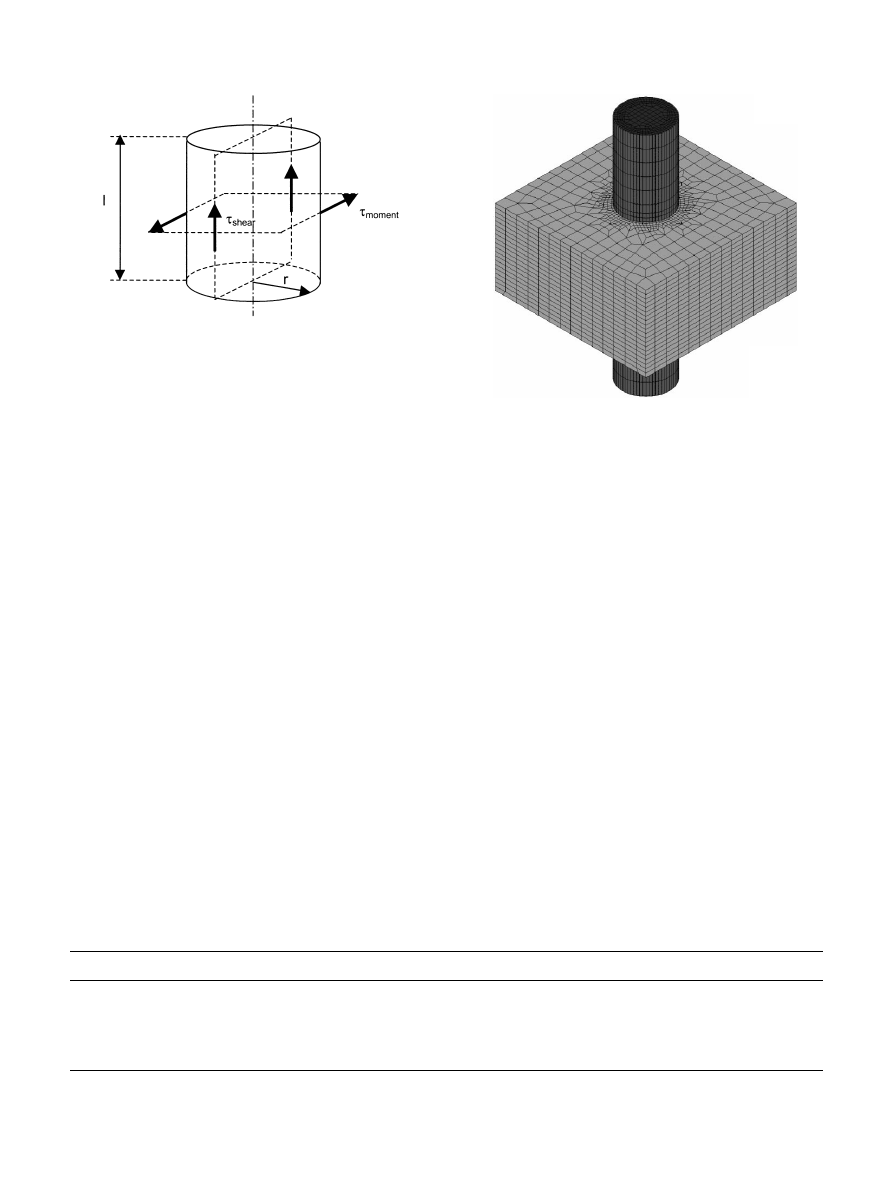
Fig. 3. 3D presentation of two perpendicular shear stresses generated
at a cylindrical surface.

Table 1
Mechanical and geometrical properties of all materials used in the moment test

Ti6A14V

BAG

Epoxy

Stainless steel

E(GPa)

113.8

10/30/78

3.2

193

l

0.34

0.21

0.36

0.22

Geometry

H

3 mm

Thickness 50

lm

Thickness 100

lm

10

;10;5 mm

Length 12 mm

Length 10 mm

Length 5 mm

Central hole

H

3.3 mm

In#uence of coating porosity, 78 GPa"fully dense BAG.

Fig. 4. 3D "nite element grid used for analysis of the moment test.

collated in Table 1. This test set-up together with Eq. (1)
will be used to measure the quantitative adhesion
strength of the BAG-coating before and after two months
in vitro.

2.2. Finite element analysis (FEA)

To evaluate the moment test and to simulate the stress

distribution a 3D "nite element model of the test set-up
was made. The FE-grid, shown on Fig. 4, is loaded with
an evenly distributed moment that generates, according
to Eq. (1), a shear stress of 40 MPa at the coating}sub-
strate interface. This value was chosen based on former
results and approximates the adhesion strength of the
BAG-coating [16]. All materials, whose characteristics
are contained in Table 1, are assumed to behave in
a linear elastic manner. For the brittle materials (BAG
and epoxy glue) this assumption is always correct. The
dimensional design of the test set-up is made up in such
a way that all stresses in both metals (Ti6Al4V-substrate
and stainless-steel counterpart) are limited within the
elastic deformation area. To take into account the in#u-
ence of closed porosity in the coating [21,22] di!erent
BAG Young's moduli are used in the model.

The quantitative calculation of the adhesion strength

only makes use of one stress component (

q). In

reality, a material is subjected to di!erent stress compo-
nents. All six possible stress components, in cylindrical
co-ordinates, working on a cylindrical surface are shown
in Fig. 5. The FE model will evaluate these six stress
components along an axial and a radial path in order to
evaluate the quantitative correctness of the simpli"cation
made by using Eq. (1).

2.3. Acoustic emission (AE) and microscopic analysis

During adhesion testing AE sensors (piezo electrical

crystals) were attached to the test set-up as close as
possible to the BAG-coating. Table 2 contains the AE-
signal analysis parameters. The AE software (Mistras)
o!ers the possibility to analyze as a function of time
a wide range of AE-parameters. For the BAG-coating the
following four time-dependent parameters were selected:
the average sound level (ASL) (dB), the number of hits,
the amplitude (dB) and the energy. Preliminary experi-
ments proved that all AE activity originated from the
BAG-coating. To examine more closely the BAG-coating
behavior during stress build-up, experiments from low

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

1463

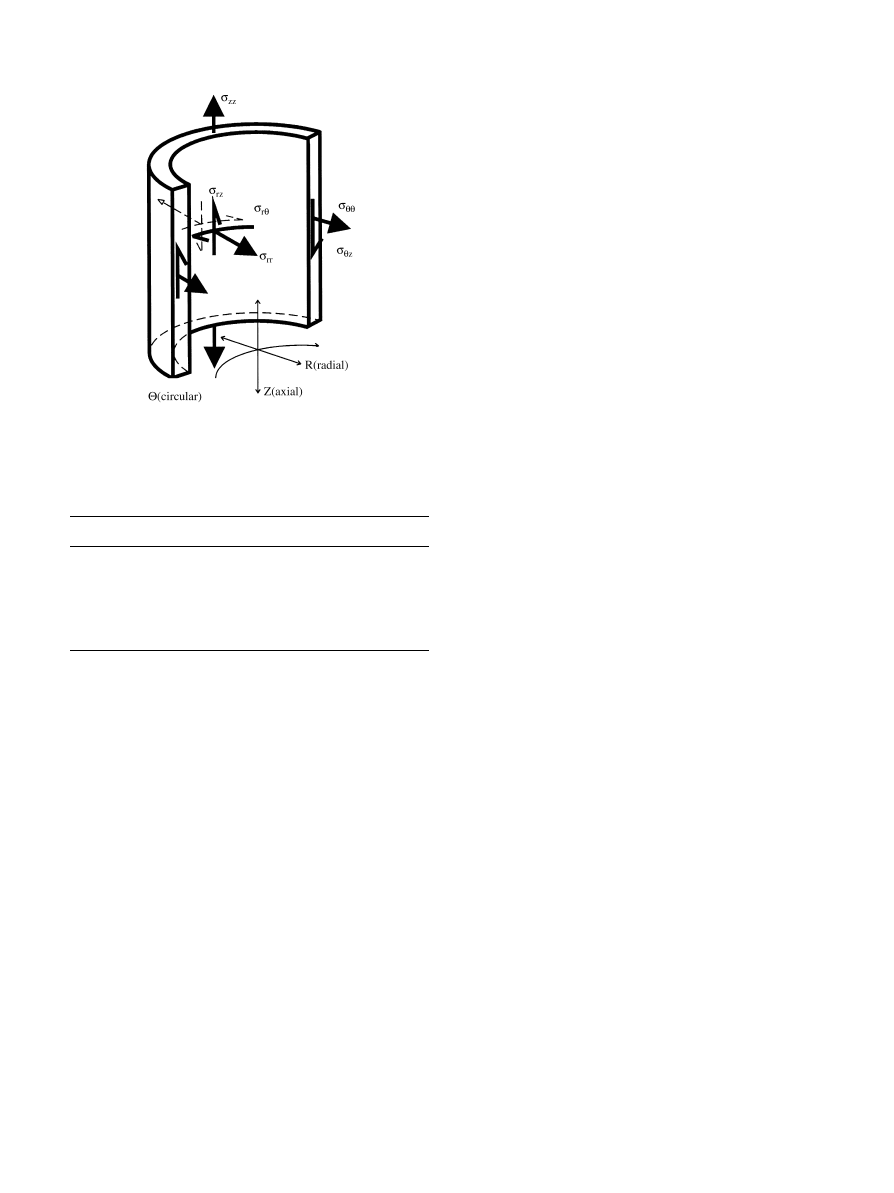
Fig. 5. 3D presentation in cylindrical co-ordinates of all six stress
components.

Table 2
Parameters used for acoustic emission signal analysis

AE-signal analysis

Value

Channels

2

Threshold (dB)

35

Pre-ampli"er gain (dB)

40

Sample rate (MHz)

4

Pre trigger (

ls)

20

Broad band sensor

2

stress levels to stresses beyond the maximum were per-
formed.

After testing all samples were imbedded in a resin

(Epo"x) and sawn in half. The cross-sections were wet
ground and polished. Using optical and scanning micro-
scopy (SEM-FEG, Philips) these cross-sections were
examined and related to the AE-results.

2.4. Combination FEA}AE-microscopy

Knowing all six stress components, the value and the

direction of the principal stresses working on the BAG-
coating can be calculated. Because BAG is a glass, pos-
sible cracks will grow from the surface in the material
along a direction perpendicular to the maximum princi-
pal normal stress [23,24]. Thus from the actual stress
distribution one can calculate the strength of the mate-
rial. In the case of the BAG-coating the following reaso-
ning is followed. If through AE and microscopy
subcritical crack initiation and growth can be detected in
the BAG-coating during loading, than the responsible
critical load (F) can be related to stresses from which

the maximum principal normal stress or material
strength can be calculated.

2.5. In vitro experiment

It is not only the initial adhesion strength of the BAG-

coating that determines the implant quality but, and to
a greater extend, the maintenance of this adhesion for
a longer period of time. It is believed that a bioactive
glass coating (50

lm) in the long run completely trans-

forms into or is replaced by a CaP-layer [25,26]. During
this transformation the bioactive glass consists of a con-
tinuously changing structure that has to preserve a good
adhesion to the substrate and that has to withstand
substantial loading.

To examine the in#uence of the reacting BAG-coating

on its adhesion strength in vitro experiments on the
BAG-coated cylindrical test samples were carried out.
This was done by placing coated samples in a stirred
simulated body #uid (Hanks') with S/<"0.1 cm

\ at

a constant body temperature of 373C during a period of
two months. Afterwards all samples were submitted to
the moment adhesion test, AE and microscopy in the
same way as non-reacted samples.

3. Results

3.1. Adhesion results

In general adhesion strength is expressed by a tensile

stress. The moment test, using Eq. (1), calculates the ad-
hesion strength in terms of a shear stress. To compare the
test results with values in literature a relationship be-
tween shear and tensile stress has to be found. In this
particular case, it is found that the shear stress can be
extrapolated to a tensile stress using the Von Mises
criterium [16].

For the processing of the experimental data a normal

and a Weibull distribution are assumed. Because BAG is
a brittle material the former is valid as long as the
material is fully intact. From the point the material starts
to deteriorate the latter distribution is the only valid one
[27].

All quantitative and extrapolated results of unreacted

and in vitro reacted BAG-coatings are combined in
Table 3. The shear adhesion strength is calculated by
introducing the maximum force (F ) from the

force}displacement curve of the moment test (Fig. 6) into
Eq. (1). Due to the small number of post in vitro experi-
ments no Weibull distribution was calculated.

3.2. FEA

The distribution of all six stress components during

loading of the test sample with a moment is evaluated in

1464

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

Table 3
Shear and tensile adhesion of BAG-coating to Ti6A14V core before and
after in vitro testing using the moment test, assuming a homogeneous
stress distribution

Unreacted BAG

In vitro BAG

Average shear stress (MPa)

48.6

43.6

SD

7.9

1.1

Number of tests

8

4

Normalized Weibull stress (MPa)

49.6

*

Weibull modulus

7

*

Tensile adhesion strength (MPa)

84.2

75.5

Fig. 6. Typical force}displacement curve of a moment test sample.

Fig. 7. Axial distribution of all stress components along the BAG}
Ti6Al4V interface.

Fig. 8. Radial distribution of all stress components along a radial path
in the middle of the sample.

two directions. The six stress components consist of three
normal stresses (

pPP, pFF, pXX) and three shear stresses (pPF,

pFX, pXP). The stress pPF is the same as the shear stress

(

q) of Eq. (1). Aiming at the adhesion strength of the

BAG-coating to the Ti6Al4V-substrate the axial distribu-
tion of all stress components is calculated at the most
important interface (BAG}Ti6Al4V) (Fig. 7). In order to

"nd

out that the test set-up meets its rationale also

a radial stress distribution along a path in the middle of
the sample is calculated (Fig. 8). Further analysis re-
vealed that the stress distribution along similar paths
shows an identical evolution.

3.3. AE and microscopy

Fig. 9 contains the typical AE result in combination

with the corresponding force}displacement curve of
a BAG-coating subjected to loading beyond the maxi-
mum. Beyond a certain load (F) the AE activity is

initiated and gradually increases up till the maximum
load (F ) is reached. The critical load (F) is cal-

culated to be 0.21F . The combination of AE activity

prior to the maximal loading with the microscopic analy-
sis shows that AE originates from cracks in the BAG-
coating that grow from the surface into the coating
(Fig. 10). The BAG}Ti6Al4V interface itself remains free

from damage even when the maximum load is reached
(Fig. 11).

The AE behavior (Fig. 12) and crack features (Fig. 13)

of an in vitro reacted BAG-coating are similar to those of
an unreacted sample. But in comparison to the unreacted
samples the cross-sectioned in vitro BAG-coatings show
a totally di!erent structural build-up [28].

3.4. Combination FEA}AE-microscopy

Due to the fact that the subcritical AE originates from

coating fracture the critical load (F"0.21F ) can be

used to calculate the strength of the BAG-coating or in
other words the total external load the coating can with-
stand without damage. By expressing the critical force
through FEA in terms of stresses and by calculating the
corresponding maximum principal stress it is found that
the strength of the BAG-coating is 47 MPa.

The post-plasma cooling of the two bonded mater-

ials (BAG, Ti6Al4V) with a di!erent thermal expansion

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

1465

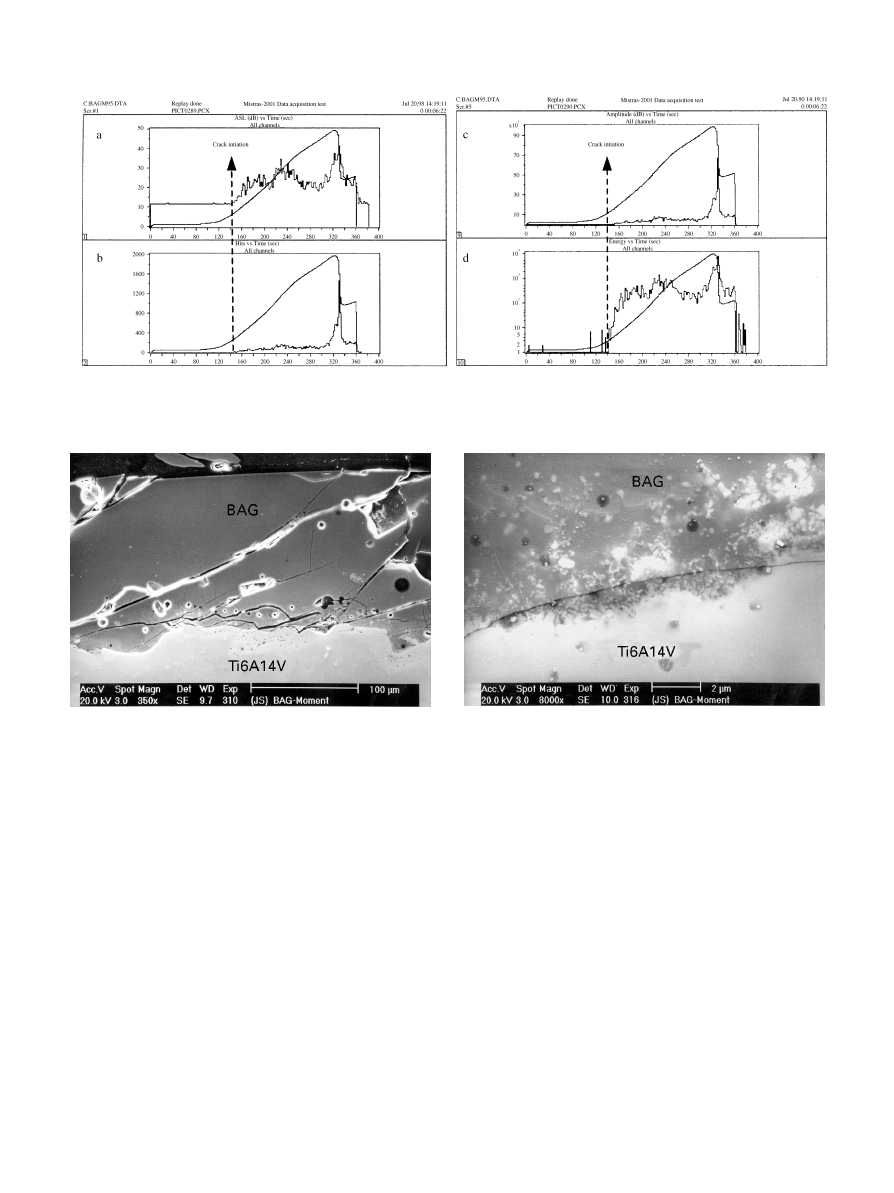
Fig. 9. Typical acoustic emission results (ASL (a), hits (b), amplitude (c) and energy (d)) versus time together with the force}displacement curve of
a tested BAG-coated moment test sample.

Fig. 10. SEM-micrograph of the coating cross-section of a BAG-coated
moment test sample, tested beyond its functionality.

Fig. 11. SEM-micrograph of the BAG}Ti6Al4V interface after ad-
hesion testing.

coe$cient

(

a %"10.3;10\ K\, a24"8.6;

10

\ K\) from a temperature above the BAG transition

temperature induces a residual tensile stress of 51 MPa in
the BAG-coating. Taking into account this residual
stress in the FEA calculation the maximum principal
stress is found to be 97 MPa.

4. Discussion

4.1. Quantitative adhesion quality

The quantitative results of the moment test, expressed

in shear or tensile strength (Table 3), show that the BAG-
coating can withstand a substantial amount of loading
before it looses its functionality. Compared to the ad-
hesion of all other bioactive coatings, the reactive

plasma-sprayed BAG is one of the best adherent coatings
[12,29}32]. The measured adhesion ('48 MPa in shear
or '84 MPa in tensile) is more than su$cient for
a load-bearing biomedical application [33]. The adhesive
quality of the coating is not only determined by the
average adhesion strength, but also by the scatter. For
a brittle material, like BAG, a scatter of 16% is low and
compared to other coatings the BAG-coating belongs to
the more reliable ones [27]. This reliable behavior of the
BAG-coating is con"rmed by the high Weibull modulus.
A modulus of 7 points out that the moment test is reliable
and that the strength distribution can be regarded as
symmetrical. Thus, a Gaussian approach is realistic
[34].

The changes in the BAG-coating during the in vitro

reaction in#uence as expected the coating strength in
a negative way. However, a 10% loss in adhesion

1466

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

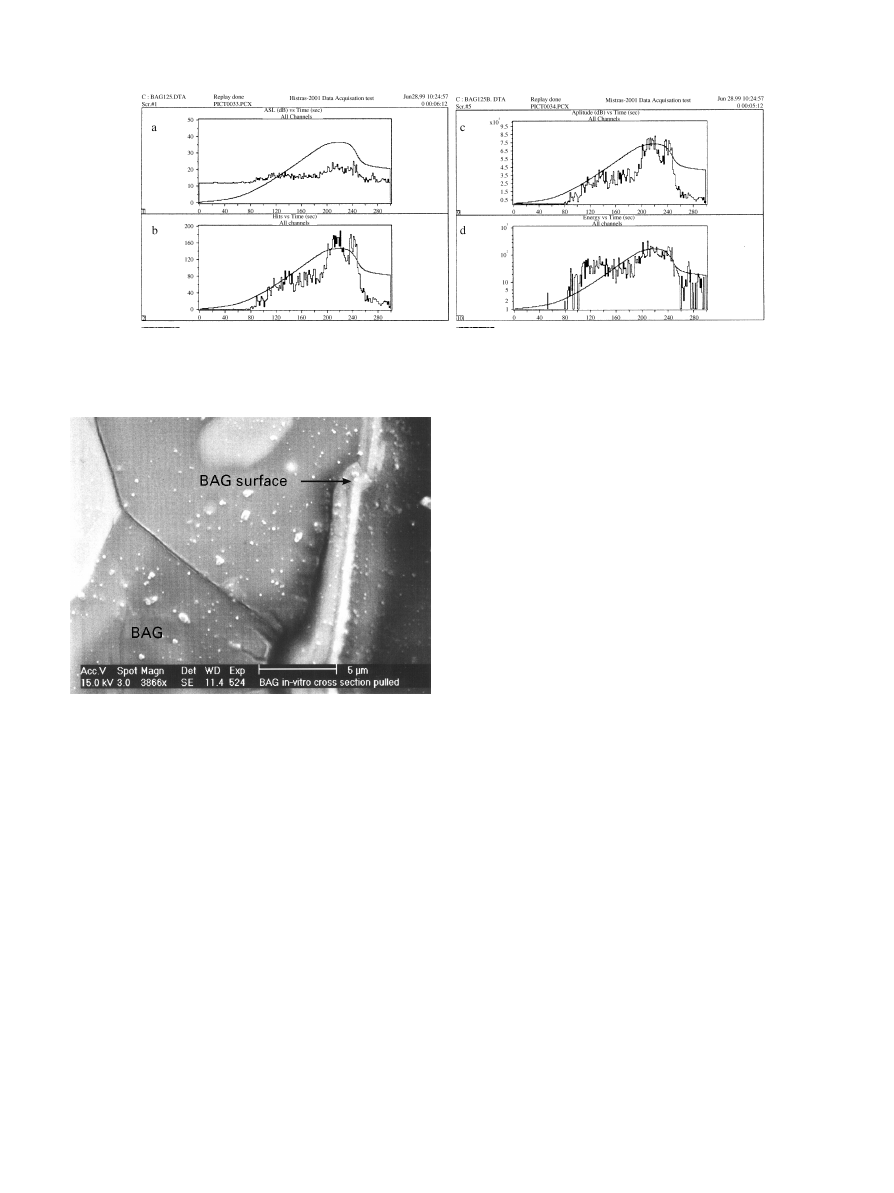
Fig. 12. Typical acoustic emission results (ASL (a), hits (b), amplitude (c) and energy (d)) versus time together with the force}displacement curve of
a post in vitro tested BAG-coated moment test sample.

Fig. 13. SEM-micrograph of the coating cross section of a post in vitro
tested BAG-coated moment test sample.

strength after two months still leaves a su$ciently func-
tional BAG-coating.

The accuracy of the adhesion values is evaluated by

FEA. The axial stress distribution along the BAG}
Ti6Al4V interface (Fig. 7) shows that the interface is only
stressed by two shear stresses. The torsion component
(

pFX) only becomes important at the sample edges and the

shear stress used for the quantitative calculations
(

pPF"q) is the dominant stress component

throughout the whole sample. Thus the moment test has,
instead of the shear test [16], one dominant stress com-
ponent. The radial stress distribution (Fig. 8) points out
that the dominant stress component reaches its max-
imum at the interface of interest and that the BAG-
coating is by far the most loaded material. The two stress
distributions prove that the moment test meets its ration-
ale to a great extend, but due to axial stress concentra-
tions at the sample edges and radial stress discontinuities

the simpli"ed Eq. (1) is still an underestimate of the
quantitative shear adhesion strength. The coating poros-
ity has no in#uence on the stress distribution. Additional
research introduced a new technique to measure directly
and non-destructively the Young's modulus and the po-
rosity of the BAG-coating [35]. The new method showed
that the plasma-sprayed BAG-coating has a Young's
modulus of 51 GPa and a porosity of 17%. This value
falls within the assumed sti!nesses, thus con"rming the
correctness of the calculated stress distribution.

4.2. Fracture behavior BAG-coating

The AE and microscopic analysis revealed that the

BAG already was subjected to substantial cracking be-
fore the coating lost its functionality. Microscopic analy-
sis showed that prior to adhesion testing no cracks were
found in both the unreacted and the in vitro reacted
BAG-coating. The subcritical failure consists initially of
cracks that grow from the coating surface into the BAG.
This cracking behavior is generally accepted for glass
materials [23,24]. In the "nal stadium the coating is
being crushed and looses its functionality, but the actual
BAG}Ti6Al4V interface stays intact. Thus, the adhesive
quality of the BAG-coating to the Ti6Al4V substrate is
more than su$cient because the interfacial strength is
higher than the bulk strength of the coating.

Detailed analysis of the in vitro reacted BAG-coating

shows that the BAG}Ti6Al4V interface is still present
over the entire sample and that it remains intact after
adhesion testing. Combining these two facts with the AE
and the microscopic analyses of the in vitro samples it is
clear that the lower adhesion strength is a result of the
lower strength of the reacted BAG. Because the reaction
starts at the BAG surface and proceeds to the metal
interface, the weakest part of the coating is still its surface
and a failure behavior similar to the unreacted samples at
a lower stress level will occur. Thus also for a reacted

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

1467

Table 4
The total adhesion quality of the BAG-coating to the Ti6A14V substra-
te before and after in vitro testing

BAG-coating property

Unreacted

In vitro reacted

Functional strength (MPa,

p, q)

'

48

'

43

'

84

'

75

Crack-free external loading (MPa,

p)

47

*

Coating strength (MPa,

p)

'

80

*

Load-bearing quality

##

#

Shear stress.

BAG-coating the BAG is weaker than the BAG}Ti6Al4V
interface and the highest possible adhesion strength is
reached once again.

4.3. Strength of plasma sprayed BAG

The combination of FEA, AE and microscopic analy-

sis makes it possible to obtain a better insight in the
failure behavior of the BAG-coating. The AE already
proved that the coating is submitted to substantial crack-
ing before it reaches its maximum load. Because it is
known that AE detects the initiation of macroscopic
cracking [36}38] the force F can be regarded as the

maximum load the plasma-sprayed BAG can withstand.
The possibility to express this load in terms of stresses is
o!ered by the FEA because prior to crack initiation the
BAG-coating behaves like an intact material as sugges-
ted in the FE model. Taking into account the residual
tensile stresses, the BAG strength of 97 MPa is of the
same order of magnitude as the strength of conventional,
by melting produced, bulk-BAG. This proves that react-
ive plasma spraying has no negative in#uence on the
BAG strength. It also shows that the closed porosity does
not participate in the strength behavior of the coating,
what is con"rmed in the literature [39]. But the thermal
mismatch is responsible for the early failure of the glass at
about 50% of its strength. The value of 47 MPa is the
maximum tensile stress the coating can withstand with-
out cracking. Because the presence of cracks can alter the
reaction path in the BAG-coating all loads should be
kept under this stress level in order to guarantee a uni-
form coating reaction front. Even taking into account
this limitation the BAG-coating is still su$cient for
load-bearing applications.

5. Conclusions

To evaluate the quality of the reactive plasma-sprayed

BAG-coating before and after in vitro testing, all aspects
of a new mechanical test were investigated. The moment
test o!ers the possibility to generate, at the interface of
interest, the maximum of one dominant stress compo-
nent. This way a valid quantitative adhesion strength can
be calculated using a classical equation. Due to stress
discontinuities and topological stress concentrations the
adhesion strength is still an underestimate. Finite ele-
ment analysis supports the test results and shows that the
generated stress condition is complicated.

By combining mechanical testing with FEA, AE and

microscopic analysis the coating quality, being a combi-
nation of adhesive and cohesive strength, can be evalu-
ated more closely. The coating itself has to be strong
enough to transfer all loads and the coating}substrate
interface has to consist of a strong bond to maintain the
implant functionality. Only if both demands are met an

implant coating can be regarded as functional. This is the
case for the reactive plasma-sprayed BAG-coating on the
Ti6Al4V oral implant (Table 4). The coating is functional,
meaning that it can support loads, up to levels that are
more than su$cient for a biomedical load-bearing ap-
plication. The gradual coating-breakdown of unreacted
and reacted BAG starts with subcritical cracking up to
the point were the coating is crushed and is no more
functional. The BAG}Ti6Al4V interface remains fully
intact at all times.

In general, it is concluded that the adhesion test is

valid and that the adhesion of the reactive plasma-
sprayed BAG-coating, tested under realistic loading con-
ditions and evaluated by a set of analyses, is su$cient for
a load-bearing oral application even after an in vitro
reaction time of two months.

Acknowledgements

Jan Schrooten gratefully acknowledges the Flemish

Institute for Scienti"c Research (IWT) for the IWT-grant
951022.

References

[1] Tsuru K, Ohtsuki C, Osaka A. Bioactivity of sol}gel derived

organically modi"ed silicates. J Mater Sci: Mater Med 1997;
8:157}61.

[2] de Bruijn JD, van Blitterswijk CA, Davies JE. Initial bone matrix

formation at the hydroxyapatite interface in vivo. J Biomed Mater
Res 1995;29:89}99.

[3] Hench LL. Bioceramics and the origin of life. J Biomed Mater Res

1989;23:685}703.

[4] Eberhardt AW, Zhou C, Rigney Jr ED. Bending and thermal

stresses in fatigue experiments of hydroxyapatite coated titanium
rods. In: Proceedings of the Seventh National Spray Conference,
June 20}24, Boston, MA, 1992. p. 165}9.

[5] Piliar RM, Filiaggi MJ. Mechanical characterisation of plasma-

sprayed hydroxyapatite-titanium alloy interfaces. In: Bon"eld W,
Hastings GW, Tanner KE, editors. Bioceramics, vol. 4. London:
Butterworth-Heinemann Ltd, 1991. p. 343}50.

1468

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

[6] Leali Tranquilli P, Merolli A, Palmacci O. Evaluation of di!erent

preparations of plasma-spray hydroxyapatite coating on titanium
alloy and duplex stainless steel in the rabbit. J Mater Sci: Mater
Med 1994;5:345}9.

[7] El-Ghannam A, Ducheyne P, Shapiro IM. Bioactive material

template for in vitro synthesis of bone. J Biomed Mater Res 1995;
29:359}70.

[8] Hydroxyapatite, Acta Orthop Scand 1993;255(suppl):64.
[9] Gianni S, Moroni A, Pompili M, Ceccarelli F, Cantagalli S,

Pezzuto V, et al. Bioceramics in orthopaedic surgery: state of the
art and preliminary results. Italian Soc Orthop Traumat 1992;
18:431}41.

[10] Helsen JA, Proost J, Schrooten J, Timmermans G, Brauns E,

Vanderstraeten J. Glasses and bioglasses: synthesis and coatings.
J Eur Ceram Soc 1997;17:147}52.

[11] Nakamura T, Yamamuro T, Higashi S, Kokubo T, Itoo S. A new

glass-ceramic for bone replacement: evaluation of its bonding to
bone tissue. J Biomed Mater Res 1985;19:685}98.

[12] Li Z, Kitsugi T, Yamamuro T, Higashi S, Chang Y, Senaha Y,

et al. Bone-bonding behavior under load-bearing conditions of an
alumina ceramic implant incorporating beads coated with glass-
ceramic containing apatite and wollastonite. J Biomed Mater Res
1995;29:1081}8.

[13] Ambroz O, Krejcova J. Determination of the adhesive and cohe-

sive fracture modes the adhesion tensile test. In: Proceedings of
the International Thermal Spray Conference, May 28}June, Or-
lando, FL, USA, 1992. p. 921}7.

[14] Berndt CC, Lin CK. Measurement of adhesion for thermally

sprayed materials. J Adhesion Sci Technol 1993;7:1235}64.

[15] GruKtzner H, Weiss H. A novel shear test for plasma-sprayed

coatings. Surf Coatings Technol 1991;45:317}23.

[16] Schrooten J, Van Oosterwyck H, Vander Sloten J, Helsen JA.

Adhesion of a new bioactive glass coating. J Biomed Mater Res
1999;44:243}52.

[17] Lin C, Berndt CC. Acoustic emission studies on thermal spray

materials. Surf Coatings Technol 1998;102:1}7.

[18] Chalker PR, Bull SJ, Rickerby DS. A review of the methods for

the evaluation of coating-substrate adhesion. Mater Sci Engng
1991;A140:583}92.

[19] Greving DJ, Shadley JR, Rybicki EF. E!ects of coating thickness

and residual stresses on the bond strength of ASTM C633-79
thermal spray coating test specimens. J Thermal Spray Technol
1994;3:371}8.

[20] Strawbridge A, Evans HE. Mechanical failure of thin brittle

coatings. Engng Failure Anal 1995;2:85}103.

[21] Lauschmann H, Moravcora M, Neufuss K, ChraHska P. Elastic

Young modulus of plasma sprayed materials, In: Proceedings of
the Seventh National Thermal Spray Conference, June 20}24,
Boston, MA, 1994. p. 699}701.

[22] Robertson J, Manning MI. Limits to adherence of oxide scales.

Mater Sci Technol 1990;6:81}91.

[23] Ernsberger FM. Origin and detection of micro#aws in glass. In:

Bradt RC, Hasselman DPH, Lange FF, editors. Fracture mech-
anics of ceramics, vol. 1. New York: Plenum Press, 1974.
p. 161}73.

[24] Evans AG. Fracture mechanics determinations. In: Bradt RC

et al., editors. Fracture mechanics of ceramics, vol. 1. New York:
Plenum Press, 1974. p. 17}37.

[25] PajamaKki KJJ, Lindholm TS, Andersson OGH, Karlsson KH,

Vedel E, Yli-Urpo A, Happonen RP. Bioactive glass and glass-
ceramic-coated hip endoprothesis: experimental study in rabbit.
J Mater Sci: Mater Med 1995;6:14}8.

[26] Gabbi C, Cacchioli A, Locardi B, Guadagnino E. Bioactive glass

coating: physicochemical aspects and biological "ndings. Bio-
materials 1995;16:515}20.

[27] Berndt CC. Tensile adhesion testing methodology for thermally

sprayed coatings. J Mater Engng 1990;12:151}8.

[28] Helsen JA, Schrooten J, Vichev R. New interpretation of bioactive

glass depth pro"les. In: Proceedings of ECASIA 99, October 4}8,
Sevilla, Spain, 1999, in preparation.

[29] Lace"eld WR, Hench LL. The bonding of Bioglass

威 to

a cobalt}chromium surgical implant alloy. Biomaterials 1986;
7:104}8.

[30] Krajewski A, Ravaglioli A, Perugini G. Bioglass composition as

coating for metal protheses. Sci Ceramics 1981;11:85}90.

[31] Krajewski A, Ravaglioli A, Mazzocchi M, Fini M. Coating of

ZrO supports with a biological glass. J Mater Sci: Mater Med

1998;9:309}16.

[32] Barth E, Hero H. Bioactive glass ceramic on titanium substrate:

the e!ect of molybdenum as an intermediate bond coating. Bio-
materials 1986;7:273}6.

[33] Kameyama T, Ueda M, Onuma K, Motoe A, Ohsaki K, Tanizaki

H, Iwasaki K. Characteristics, of a radio-frequency thermal
plasma spraying method for the coating of hydroxyapatite. In:
Proceedings of ITSC 1995, Kobe, Japan, p. 187}92.

[34] Leigh SH, Berndt CC, Wu CL, Nakamura T. Tensile adhesion of

thermal spray coatings on #at substrates, In: Proceedings of the
Seventh Thermal Spray Conference, June 20}24, Boston, MA,
1994. p. 655}62.

[35] Schrooten J, Roebben G, Helsen JA. Young's modulus of bioac-

tive glass coated oral implants: porosity corrected bulk modulus
versus resonance frequency analysis. Scripta Mater 1999; 41:1047}53.

[36] Becher PF, Newell WL, Halen SA. Applications of failure mech-

anics to the adherence of thick "lms and ceramic braze joints. In:
Bradt RC, Hasselman DPH, Lange FF, editors. Fracture mech-
anics of ceramics, vol. 3. New York: Plenum Press, 1978.
p. 463}71.

[37] Wakayama S, Nishimura H. Critical stress of microcracking in

alumina evaluated by acoustic emission. In: Bradt RC et al.,
editors. Fracture mechanics of ceramics, vol. 10. New York:
Plenum Press, 1992. p. 59}71.

[38] Tetelman AS, Evans AG. Failure prediction in brittle materials

using fracture mechanics and acoustic emission. In: Bradt RC,
Hasselman DPH, Lange FF, editors. Fracture mechanics of cer-
amics, vol. 2. New York: Plenum Press, 1974. p. 895}923.

[39] Kostopoulos V, Peteves SD, Steen M. Mixed-mode fracture

toughness of hot-presses SiN a comparative study. In: Bradt

RC, Hasselman DPH, Munz D, Sakai M, Shevchenko VY, edi-
tors. Fracture mechanics of ceramics, vol. 11. New York: Plenum
Press, 1996. p. 273}88.

J. Schrooten, J.A. Helsen / Biomaterials 21 (2000) 1461}1469

1469