Influence of airflow velocity on kinetics of convection apple drying

D. Veli

c

*

, M. Planini

c, S. Tomas, M. Bili

c

Department of Process Engineering, Faculty of Food Technology, University J.J. Strossmayer of Osijek, F. Kuhaca 18,

P.O. Box 709, 31000 Osijek, Croatia

Received 30 May 2003; accepted 13 September 2003

Abstract

The aim of this experiment was to investigate airflow velocity influence (0.64, 1.00, 1.50, 2.00, 2.50 and 2.75 m s

1

) on the kinetics

of convection drying of Jonagold apple, heat transfer and average effective diffusion coefficients. Drying was conducted in a con-
vection tray drier at drying temperature of 60

C using rectangle-shaped (20 · 20 · 5 mm) apple samples. Temperature changes of

dried samples, as well as relative humidity and temperature of drying air were measured during the drying process. Rehydratation
ratio was used as a parameter for the dried sample quality. Kinetic equations were estimated by using an exponential mathematical
model.

The results of calculations corresponded well with experimental data. Two well-defined falling rate periods and a very short

constant rate period at lower air velocities were observed. With an increase of the airflow velocity an increase of heat transfer
coefficient and effective diffusion coefficient was found. During rehydratation, about 72% of water removed by the drying process
was returned.
2003 Elsevier Ltd. All rights reserved.

Keywords: Airflow velocity; Convection apple drying; Exponential drying model; Effective diffusion coefficient; Heat transfer coefficient

Apple is an important raw material for many food

products and apple plantations are cultivated all over
the world in many countries. Thus, it is very important
to define the conditions under which the characteristics
of fresh apples can be preserved and to define optimal
parameters for their storage and reuse.

Drying is a frequently and used procedure for food

preservation. Convection drying as well as other tech-
niques for drying are used in order to preserve the
original characteristics of apples. Dried apples can be
consumed directly or treated as a secondary raw mate-
rial.

High temperatures and long drying times required to

remove the water from the fruit material in convection
air drying may cause serious damage in flavour, colour,
nutrients and can reduce the bulk density and rehydra-
tation capacity of the dried product (Lin, Durance, &
Scaman, 1998).

There is a growing interest in the food industry in

the development of economical methods for food

production with high organoleptic and nutritional
value. The purpose of this study was to study con-
vection drying of apple in laboratory conditions and
to investigate the influence of airflow velocities on
drying kinetics, heat transfer coefficient and effective
diffusion coefficient.

1. Materials and methods

1.1. Drying equipment

Drying was performed in a pilot plant tray dryer

(UOP 8 Tray Dryer, Armfield, UK). The dryer operates
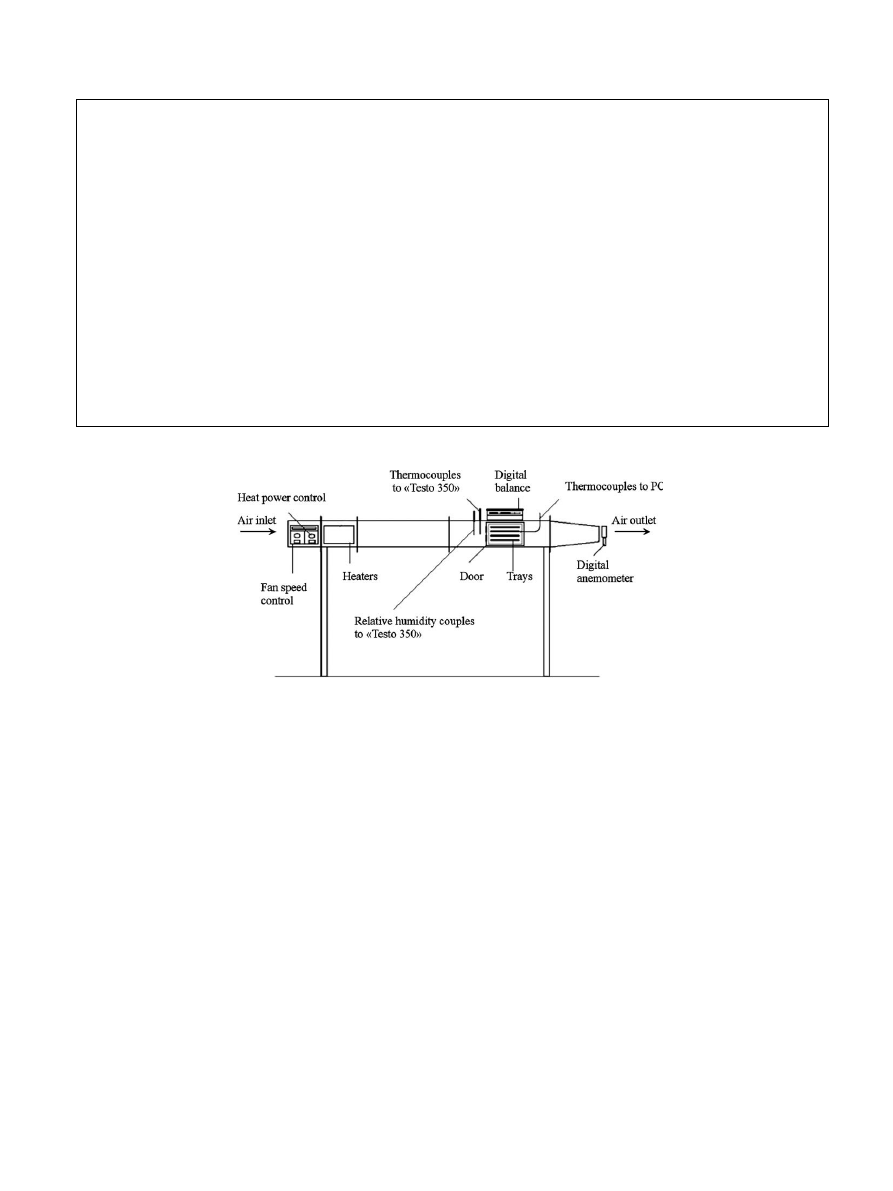
on the thermogravimetric principle. The dryer (Fig. 1) is
equipped with controllers for controlling the tempera-
ture and airflow velocity. Air was drawn into the duct
through a diffuser by a motor driven axial flow fan
impeller. In the tunnel of the dryer there were carriers
for trays with samples, which were connected to a bal-
ance. The balance was placed outside the dryer and
continuously determined and displayed the sample
weight. A digital anemometer at the end of the tunnel
measured airflow velocity.

Journal of Food Engineering 64 (2004) 97–102

www.elsevier.com/locate/jfoodeng

*

Corresponding author. Tel.: [phone]; fax: [phone]-

115.

E-mail address:

[e-mail]

(D. Veli

c).

0260-8774/$ - see front matter

2003 Elsevier Ltd. All rights reserved.

doi:10.1016/j.jfoodeng.2003.09.016

1.2. Material

Apples (Jonagold) were obtained from a local super-

market and stored at +4

C. After 2-h stabilization at

the ambient temperature, apples were hand peeled and
cut to the rectangle-shaped slices, dimensions: 20

· 20 · 5

mm.

1.3. Drying procedure

The dryer was operated at air velocities 0.64, 1.0, 1.5,

2.0, 2.5 and 2.75 m s

1

, with 60

C dry bulb temperature

and average relative humidity of 9%. Air flowed parallel
to the horizontal drying surfaces of the samples. Drying
process started when drying conditions were achieved
(60

C and constant air velocity). The apple samples on

trays were placed into the tunnel of the dryer and the
measurement started from this point. During the drying
process temperature changes of dried samples were
continuously recorded by thermocouples connected to a

PC. ‘‘Testo 350’’ probes placed into the drying chamber
measured relative humidity and drying air temperature.
Sample weight loss was recorded every 5 min during the
drying process using a digital balance (with precision of
±0.01 g). Dehydration lasted until a moisture content of
about 20% (wet base) was achieved. Airflow velocity was
measured every 5 min with a digital anemometer that
was placed at the end of the tunnel. Dried samples were
kept in airtight glass jars until the beginning of rehy-
dratation experiments.

1.4. Determination of the total solid/moisture content

The moisture content of the dried samples was de-

termined by using a standard laboratory. Small quanti-
ties of each sample were dried in a vacuum oven (6 h at of
70

C and 30 mbar pressure). Time dependent moisture

content of the samples was calculated from the sample
weight and dry basis weight. Weight loss data allowed
the moisture content to be calculated such as follows:

Nomenclature

a

, K

parameters in model (8)

c

specific heat (J kg

1

K

1

)

CP

critical point

h

heat transfer coefficient (W m

2

K

1

)

k

, n

parameters in model (2)

L

length (m)

‘

sample thickness (m)

m

weight (kg)

Nu

Nusselt number

Pr

Prandtl number

Re

Reynolds number

t

drying time (min)

T

temperature (K)

X

moisture (kg

w

kg

1
db

)

X

0

dimensionless moisture

dX

0

=

dt drying rate (min

1

)

v

air velocity (m s

1

)

k

heat conductivity (W m

1

K

1

)

l

dynamic viscosity of air (Pa s)

#

temperature (

C)

q

density of air (kg m

3

)

Subscripts
db

dry basis

w

water

0

initial

a

air

e

equilibrium

f

film

s

surface

Fig. 1. Schematic diagram of the convection drying equipment (UOP 8 Tray Dryer, Armfield, UK).

98

D. Veli

c et al. / Journal of Food Engineering 64 (2004) 97–102

X

ðtÞ ¼ m

w

=m

db

1.5. Rehydratation

The rehydratation characteristics were used as a

quality index of a dried product. Approximately 3 g
(±0.01 g) of dried samples were placed in a 250 ml
laboratory glass (2 parallels for each sample), 150 ml
distilled water was added, the glass was covered and
heated up to the boiling point within 3 min. The content
of the laboratory glass was then cooked for 10 min by
mild boiling and cooled. Cooled content was filtered for
5 min under vacuum, and weighed.

The rehydratation ratio (R) was used to express

ability of the dried material to absorb water (Lewicki,
1998). It was determined by the following equation:

Rehydratation ratio%

¼

mass of water absorbed during rehydratation

mass of water removed during drying

100

ð1Þ

1.6. Drying rate curve determination

The exponential model successfully describes the

drying kinetics of some porous materials, such as clay
(Skansi & Tomas, 1995; Tomas, Skansi, & Sokele,
1994), Al–Ni catalyst (Sander, Tomas, & Skansi, 1998)
and food materials (Tomas & Skansi, 1996). The au-
thors also used this model to describe the changes of
moisture content and drying rates. The time dependent
weight of samples was converted for the given time de-
pendent to moisture content.

To avoid some ambiguity in results because of the

differences in initial sample moisture, the sample mois-
ture was expressed as dimensionless moisture ratio
(X

0

¼ X ðtÞ=X

0

). The drying curve for each experiment

was obtained by plotting the dimensionless moisture of
the sample vs. the drying time. For the approximation
of experimental data and calculating drying curves
(Eq. (2)) and drying rate curves (Eq. (3)), the simplified
model was used, as follows:

X

0

ðtÞ ¼ exp

ðkt

n

Þ

ð2Þ

dX

0

dt

¼ k n t

ðn1Þ

X

0

ðtÞ

ð3Þ

The parameters k and n were calculated by non-linear
regression method (Quasi-Newton) using Statistica 6.0
computer program. The correlation coefficient (r

2

) was

used as a measure of model adequation. The first and
second critical points were determined as a maximum
and point of inflexion of the function (

dX =dt) (Tomas

& Skansi, 1996).

1.7. Calculation of the heat transfer coefficient

Convective heat transfer occurs between a moving

fluid and a solid surface. This work investigated con-
vective heat transfer for forced convection flow over a
flat plate. The viscosity of the fluid requires that the fluid
has zero velocity at the plate’s surface. Because a
boundary layer exists, the flow is initially laminar but
can proceed to turbulence once the Reynolds number of
the flow is sufficiently high (Pitts & Sissom, 1977).

It was assumed that the plate (sample) was main-

tained at constant temperature (T

s

) and the plate length

(L) was sufficiently short so that turbulent flow was
never triggered (Fig. 2).

Average heat transfer coefficient was calculated using

Pohllhausen equation (4) for laminar flow and other
correlations (5) and (6) that are given below:

Nu

lam

¼ 0:664 Re

1=2
lam

Pr

1=3

ðvalid for Re < 2 10

5

Þ

ð4Þ

Nu

¼

h

L

k

;

Re

¼

L

v q

l

;

Pr

¼

l

c

k

ð5Þ

All calculations were performed at the average film
temperature (T

f

):

T

f

¼

T

a

þ T

s

2

½K

ð6Þ

where are: T

a

––air temperature [K], T

s

––average tem-

perature of sample surface [K].

1.8. Determination of the effective diffusion coefficient

The simplified method (Zogozsa, Maroulis, & Mari-

nos-Kouris, 1994) was used for determination of the
effective diffusion coefficient. For a thin plate the solu-
tion of Fick’s law of diffusion, with assumptions of
moisture migrating only by diffusion, negligible shrink-
ing, constant temperature and diffusion coefficients and
long drying times, are given below (Baroni & Hubinger,
1998):

X

X

e

X

0

X

e

¼

X

n

¼1

n

¼0

8

ð2n þ 1Þ

2

p

2

exp

D

eff

ð2n þ 1Þ

2

p

2

t

4‘

2

!

ð7Þ

where X

e

and X

0

represent equilibrium and initial

moisture contents, and ‘ is the slab thickness. The value

Fig. 2. Convection heat transfer for forced flow over a flat plate.

D. Veli

c et al. / Journal of Food Engineering 64 (2004) 97–102

99

of the equilibrium moisture content is relatively small
(low air relative humidity) compared to X or X

0

. Thus

ðX X

e

Þ=ðX

0

X

e

Þ is simplified to X

0

¼ X =X

0

(dimen-

sionless moisture ratio) (Doymaz & Pala, 2002).

Where sample thickness is small (0.005 m) and drying

time is relatively large, only the first term of Fickan’s
solution series is need, and Eq. (7) becomes:

X

0

¼ a expðK tÞ

ð8Þ

where K

¼ ðD

eff

p

2

Þ=ð4‘

2

Þ is represent the slope of X

0

vs. t

plotting on the semi-logarithmic diagram.

2. Results and discussion

The results of numerical adoptions of experimental

data are summarized in Table 1. The moisture contents
(experimental and modelled data) vs. drying time at dif-
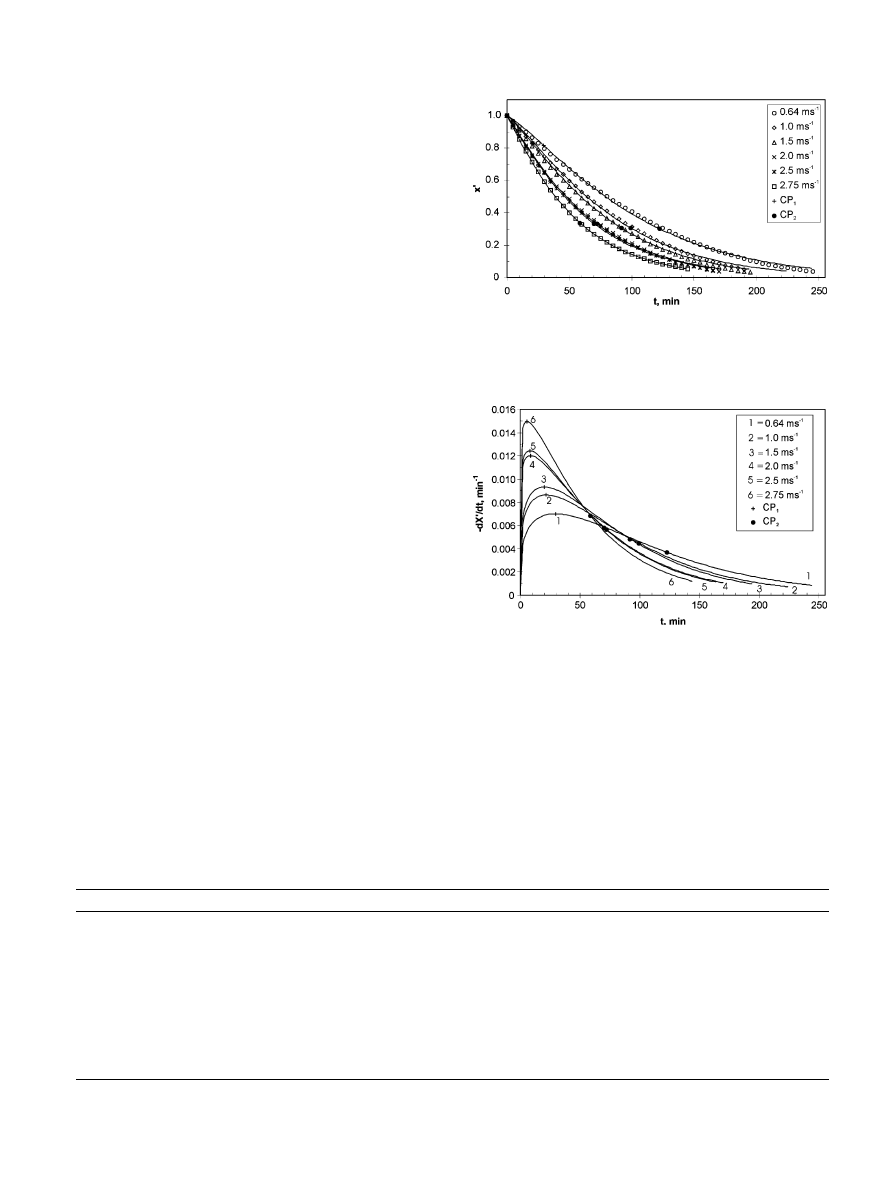
ferent airflow velocities are shown in Fig. 3. It can be seen
that a good agreement between experimental data and
chosen mathematical model exists, which is confirmed by
high values of correlation coefficient (0.9991–0.9995).
Results show that the airflow rate had a significant effect
on drying rates of apple. With the increase of the air flows
velocity, the time required to achieve certain moisture
content decreased.

Fig. 4 shows typical drying curves, which are char-

acterised by two falling rate periods with no undoubt-
edly apparent constant rate period. However, it might
be possible to have a very short constant rate period at
lower airflow velocities (0.64, 1.0 and 1.5 m s

1

) followed

after the initial period of increasing drying rate. In this
period samples retained almost constant temperature
(Fig. 5) and then kept growing. After the first critical
point (in interval from 0.8176 to 0.9186 kg

w

kg

1
db

), the

internal resistance of product increase, resulted in the
first falling rate period. The second falling rate period
started after the second critical point (around 0.3
kg

w

kg

1
db

according to air velocity). If the slope of tan-

gent to the drying curve (

dX =dt) was considered as the

drying rate of the sample, the results suggested that in
the second period drying was faster than in the first
falling rate period. Similar results were obtained during
the drying of sweet potato slices (Diamante, 1994).

Rehydratation did not show a clear dependence of

rehydratation ability of dried apple on airflow velocity.
During the rehydratation, dried sample absorbed be-

Table 1
Results of numerical analyses [model (2)]. Time, dimensionless moisture and drying rate in the first and second critical points at different airflow rate

v

(m s

1

)

0.64

1.0

1.5

2.0

2.5

2.75

k

0.002917

0.004230

0.005829

0.009298

0.009846

0.012683

n

1.252203

1.225387

1.201714

1.116508

1.110101

1.092722

r

2

0.999140

0.999309

0.999186

0.999486

0.999516

0.999185

t

(min)

CP

1

29.4

21.7

20.2

8.7

8.0

5.7

CP

2

122.5

99.3

91.9

72.1

69.9

58.6

X

0

CP

1

0.81758

0.83199

0.83170

0.90091

0.90558

0.91865

CP

2

0.30077

0.30607

0.30596

0.33143

0.33314

0.33795

dX

0

=

dt

(min

1

)

CP

1

0.00701

0.00863

0.00932

0.01204

0.01245

0.01496

CP

2

0.00369

0.00447

0.00483

0.00566

0.00581

0.00683

CP

1

––first critical point; CP

2

––second critical point.

Fig. 3. Experimental and calculated moisture contents vs. drying time.

Fig. 4. Drying rate vs. drying time for different airflow velocities with
first and second critical points.

100

D. Veli

c et al. / Journal of Food Engineering 64 (2004) 97–102

tween 63.80% and 79.25% of water, which was removed,
by drying (Table 2).

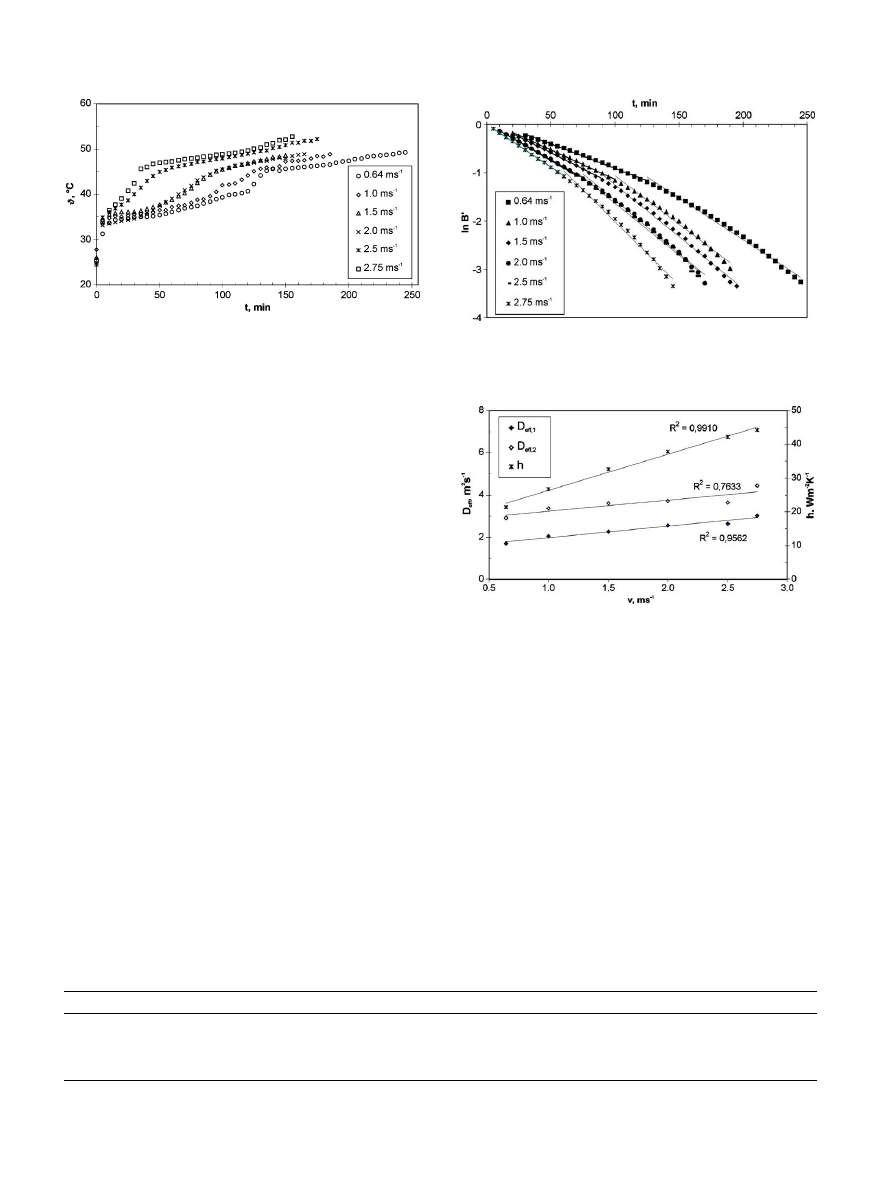
As the airflow velocity increased, the heat transfer

coefficient for drying apples also increased almost pro-
portionally (Table 2; Fig. 7).

The semi-logarithmic dimensionless moisture vs.

drying time plot for falling rate period at different air
velocities is shown in Fig. 6.

Two well-defined falling rate periods are observed,

each corresponding to an approximately constant slope
from which the effective diffusion coefficients are calcu-
lated. With increasing the airflow rate, D

eff

increases too

in the both periods. For the examined airflow rate, the
value of the average effective diffusion coefficient in the
first falling rate period ranged from 1.7

· 10

9

to

3.0

· 10

9

m

2

s

1

(Table 2). That accords with the liter-

ature data for food products such as: vegetable wastes
(Lopez, Iguaz, Esnoz, & Virseda, 2000), carrot and po-
tatoes (Mulet, 1994), apple cubes (Simal, Dey

a, Frau, &

Rossel

o, 1997) and apple tissues (Feng, Tang, & Dixon-

Werren, 2000). Whereas, in the second falling rate pe-
riod average D

eff

was around 1.6 times greater than in

the first period, and it ranged from 2.9

· 10

9

to

4.4

· 10

9

m

2

s

1

. This is corresponds with conclusions

that the rate of diffusion is proportional to the sample
temperature (Diamante, 1994), which in this case de-
pends on the airflow velocities and heat transfer coeffi-
cient (Fig. 7), and that the value of D

eff

increases in time

(Simal, Rossello, Berna, & Mulet, 1994).

3. Conclusion

The drying kinetics of Jonagold apple, average heat

transfer coefficient and average effective diffusion coef-
ficient at airflow rate: 0.64, 1.0, 1.5, 2.0, 2.5 and 2.75
m s

1

were obtained by a thermogravimetric method.

As can be observed, by using the exponential model it

is possible to accurately simulate the drying kinetics of
apple at different air velocities. An increase of airflow
velocity resulted in increase of moisture removal rate.
Two well-defined falling rate periods with different
drying rates and effective diffusion coefficients were ob-
served at all examined air rate.

Table 2
Rehydratation ratio, heat transfer coefficient and effective diffusion coefficient for drying apple at different airflow ratio

v

(m s

1

)

0.64

1.0

1.5

2.0

2.5

2.75

R

(%)

79.15

63.80

72.20

79.25

72.00

75.95

h

(W m

2

K

1

)

21.43

26.73

32.72

37.87

42.28

44.30

D

eff;1

(m

2

s

1

)

1.70

· 10

9

2.06

· 10

9

2.26

· 10

9

2.56

· 10

9

2.64

· 10

9

3.02

· 10

9

D

eff;2

(m

2

s

1

)

2.91

· 10

9

3.37

· 10

9

3.61

· 10

9

3.72

· 10

9

3.62

· 10

9

4.45

· 10

9

D

eff;1

––effective diffusion coefficient in the first falling rate period; D

eff;2

––effective diffusion coefficient in the second falling rate period.

Fig. 5. Temperature of sample vs. drying time for different airflow
velocities.

Fig. 6. Semi-logarithmic dimensionless moisture ratio vs. drying time
in the falling rate period for different airflow velocities.

Fig. 7. The effect of airflow velocity on the heat transfer coefficient and
effective diffusion coefficients.

D. Veli

c et al. / Journal of Food Engineering 64 (2004) 97–102

101

The average D

eff

increased with airflow rate, and

ranged form 1.7

· 10

9

to 3.0

· 10

9

m

2

s

1

for the first

and from 2.9

· 10

9

to 4.4

· 10

9

m

2

s

1

for the second

falling rate period.

With the increase of the airflow velocity, heat transfer

coefficient increased too, and it ranged between 21.4 and
44.3 W m

2

K

1

.

During rehydratation of dried apples, 63.80–79.25%

of water removed by the drying process was returned.

References

Baroni, A. F., & Hubinger, M. D. (1998). Drying of onion: effects of

pretretmant on moisture transport. Drying Technology, 16(9&10),
2083–2094.

Diamante, L. M. (1994). Drying characteristics of sweet potato slices.

In Proceedings of the international conference of preservation and
security (pp. 187–199).

Doymaz, I., & Pala, M. (2002). The effects of dipping pretreatments on

air-drying rates of the seedless grapes. Journal of Food Engineering,
52(4), 413–417.

Feng, H., Tang, J., & Dixon-Werren, S. J. (2000). Determination of

moisture diffusivity of red delicious apple tissues by thermogravi-
metric analysis. Drying Technology, 18(6), 1183–1199.

Lewicki, P. P. (1998). Some remarks on rehydration of dried foods.

Journal of Food Engineering, 36, 81–87.

Lin, T. M., Durance, T. D., & Scaman, C. H. (1998). Characterization

of vacuum microwave air and freeze dried carrot slices. Food
Research International, 4, 111–117.

Lopez, A., Iguaz, A., Esnoz, A., & Virseda, P. (2000). Thin-layer

drying behaviour of vegetable wastes from wholesale market.
Drying Technology, 18(4&5), 995–1006.

Mulet, A. (1994). Drying modelling and water diffusivity in carrota

and potatoes. Journal of Food Engineering, 22, 329–348.

Pitts, D. R., & Sissom, L. E. (1977). Schaum’s outline of theory and

problems of heat transfer (pp. 614–638). New York: McGraw-Hill
Inc.

Sander, A., Tomas, S., & Skansi, D. (1998). The influence of air

temperature on effective diffusion coefficient of moisture in the
falling rate period. Drying Technology, 16(7), 1487–1499.

Simal, S., Dey

a, E., Frau, M., & Rossel

o, C. (1997). Simple modelling

of air drying curves of fresh and osmotically pre-dehydrated apple
cubes. Journal of Food Engineering, 33, 139–150.

Simal, S., Rossello, C., Berna, A., & Mulet, A. (1994). Heat and mass

transfer model for potato drying. Chemical Engineering Science,
22(49), 3739–3744.

Skansi, D., & Tomas, S. (1995). Microwave drying kinetics of a clay-

plate. Ceramics International, 21, 207–211.

Tomas, S., & Skansi, D. (1996). Numerical interpretation of drying

curve of food products. Journal of Chemical Engineering of Japan,
29(2), 367–370.

Tomas, S., Skansi, D., & Sokele, M. (1994). Convection drying of

porous material. Cheramics International, 20, 9–16.

Zogozsa, N. P., Maroulis, Z. B., & Marinos-Kouris, D. (1994).

Moisture diffusivity methods of experimental determination a
review. Drying Technology, 12(3), 483–515.

102

D. Veli

c et al. / Journal of Food Engineering 64 (2004) 97–102