Pma2 jpeg

International Tables for Crystallography (2006). Voł. A, Space group 28, pp. 224-225.

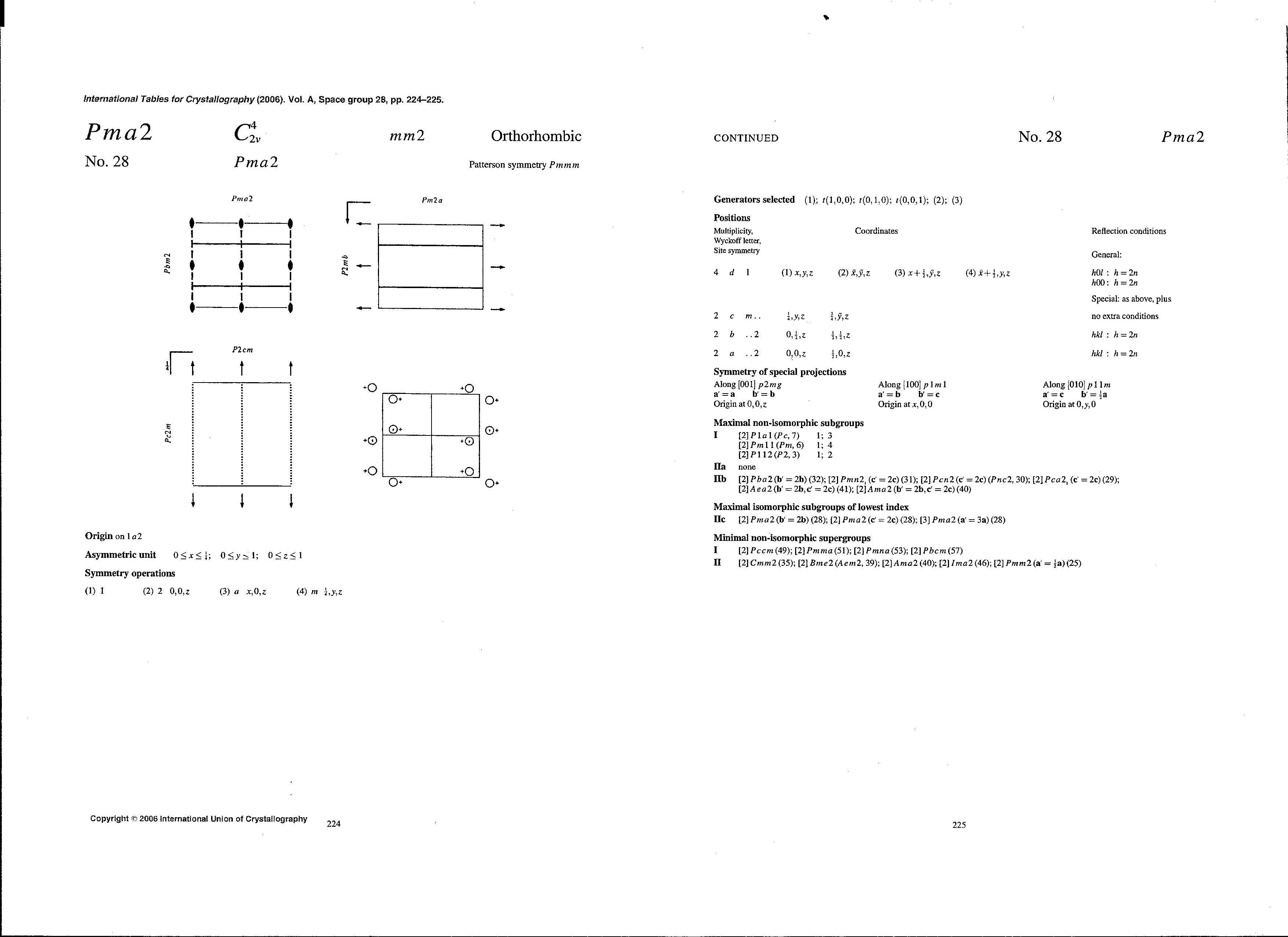
Pma 2    Ct

mm 2    Orthorhombic

No. 28

Pma 2

Patterson symmetry Pmmm

Pma2

♦— 1	—♦— i	—♦
1 i ^£ ?	i ♦ i	1 ♦ i
1 ♦—	1 —♦—	I —t
n	PI cm f	f
£ j : : i i i i
i	1	I

r

*o		*o
	o*
	o*
-o		*0
♦o		*o

o    o*

Origin on lo2

Asymmetric unit 0 <* < J; 0 < y < 1; 0 < z < 1 Symmetry operations

(1) 1    (2) 2 0,0,z    (3) a x,0,z    (4) m ),y,z

CONTINUED

No. 28

Pma 2

Generatora selected (1); f(l,0,0); /(0,1,0); /(0,0,1); (2); (3) Positions

Multiplicity,    Coordinates

Wyckoff letter,

Site symmetry

Symmetry of special projections

Along [001] p2mg a' = a b = b

Origin at 0,0, z

Along [100] plml a' = b b’ = c Origin at *,0,0

Along [010] pllm a' = c b' = Origin at0,y,0

Reflection conditions General:

4	d 1	(D*,y,z	(2) x,y,z	(3)	(t)Z+i,y,z	II II S|
						Special: as above, plus
2	c m..		!,y>z			no extra conditions
2	b ..2	o,i,z	5, Z			hkl : h = 2n
2	a ..2	0,0,Z	5,0, Z			hkl : h = 2n

Maximal non-isomorphic subgroups

I [2] Pio 1 (Pc, 7)    1; 3

[2]Pmll(Pm,6) 1; 4 [2]PI 12(P2,3)    1; 2

na nonę

nb    [2] Pba2<b' = 2b) (32); [2] Pmn2_x (t = 2c) (31); [2] Pcn2 (ć = 2c) (Pnc2,30); [2]Pco2, (c' = 2c) (29);

[2]Aea2(b' = 2b,ć = 2c)(41); [2]Amo2(b' = 2b,c' = 2c)(40)

Maximal isomorphic subgroups of lowest index

He [2] Pma 2 (b' = 2b) (28); [2] Pm o 2 (C = 2c) (28); [3] Pma2 (a' = 3a) (28)

Minimal non-isomorphic supergroups

I    [2] Pccm(49); [2]Pmma(51); [2] Pmna(53); [2] Pbcm(57)

D    [2] Cmm2 (35); [2J Bme2 (Aem2, 39); [2] Amo2 (40); [2] Ima2 (46); [2] Pmm2 (a' = ±a)(25)

225