trygonometria

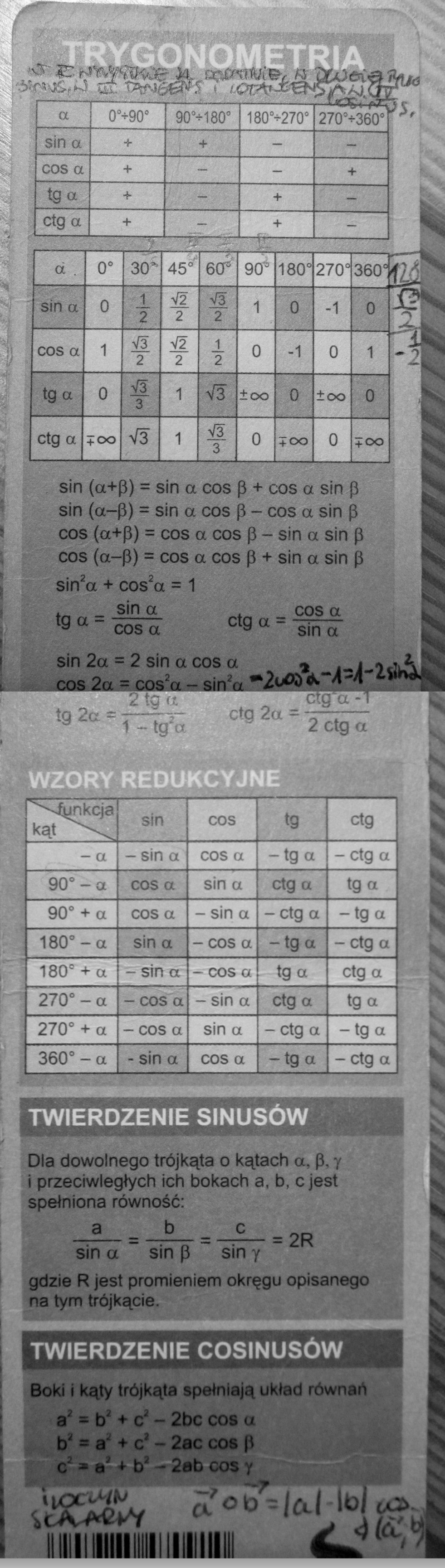
'&fc

f ' i 0D*? -

i

| a	0°+90°		90°^ 180°		180°+270°		i-**'*>» r* 270°+360°		n.
sin a	+		+		-		-
cos a	+		-		-		+
tg a			-		+		-
ctga	+		-		+		-
a .	0°	30"	45*	60*	90*	180°	270°	360‘	1
sin a	0	1 2	V2 2	<1 2	1	0	-1	0	i ■
cos a	1	V3 2	V2 2	1 2	0	-1	0	1	1
tg a	0	V3 3	1	V3	±oo	0	±oo	0
ctga	+oo	V3	1	V3 3	0	+ oo	0	+oo

sin (a+P) = sin a cos p + cos a sin p sin (a-P) = sin a cos p - cos a sin p cos (a+p) = cos a cos p - sin a sin p cos (a-p) = cos a cos p + sin a sin p sin²a + cos²a = 1

.    _ sin a

*^{g a} ~ cos a

_ COS a ^{ctg a} "slrTBT

sin 2a = 2 sin a cos a ,    . . «

cos 2a m cos²a- sin²a S&fcfK

2tga" ctga -1

•8^'Trss ^c,g2a=2^

^\fjjnkcjs ? kąt^\^	sin	cos	tg	ctg
- a	- sin a	cos a I	-tg a	- ctg a
] 90° - a	cos a	sin a	ctg a	tg a
| 90° + a	cos a	- sin a	-ctga	-tg a
S 180°-a	sin a	- cos a	- tg a	-ctg a
| 180° + a	- sin a	- cos a	tg a	ctg a
| 270°-a	- cos a	- sin a	ctg a	tg a
| 270° +a	-cos a	sin a	-ctg a	- tg a
j 360°-a	- sin a	cos a	-tg a	- ctg a

TWIERDZENIE SINUSÓW

Dla dowolnego trójkąta o kątach a, p, y i przeciwległych ich bokach a, b, cjest spełniona równość:

a ₌ b ₌ c _{= 2R} sin a sin p sin y

gdzie R jest promieniem okręgu opisanego na tym trójkącie.

TWIERDZENIE COSINUSÓW

Boki i kąty trójkąta spełniają układ

’ = b² + c² - 2bc cos u b² = a² + c² - 2ac cos p c² * a² + b² - 2ab cos y

im