7

Laminar and Turbulent Flow

Il y a toujours sur ma strophe ou sur ma page
un peu de l’ombre du nuage et de la salive de la mer;
ma pens´

ee flotte et va et vient, comme d´

enou´

ee par toute

cette gigantesque oscillations de l’infini. (Victor Hugo)

In my boyhood I had the advantage of the constant guidance of my father,
also a lover of mechanics, and a man of no mean attainments in mathematics
and its application to physics. (Reynolds)

7.1 Reynolds

The NS equations give an accurate description of a great variety of fluid flows
including both laminar flow with ordered flow features and turbulent flow
with unordered seemingly chaotic fluid dynamics.

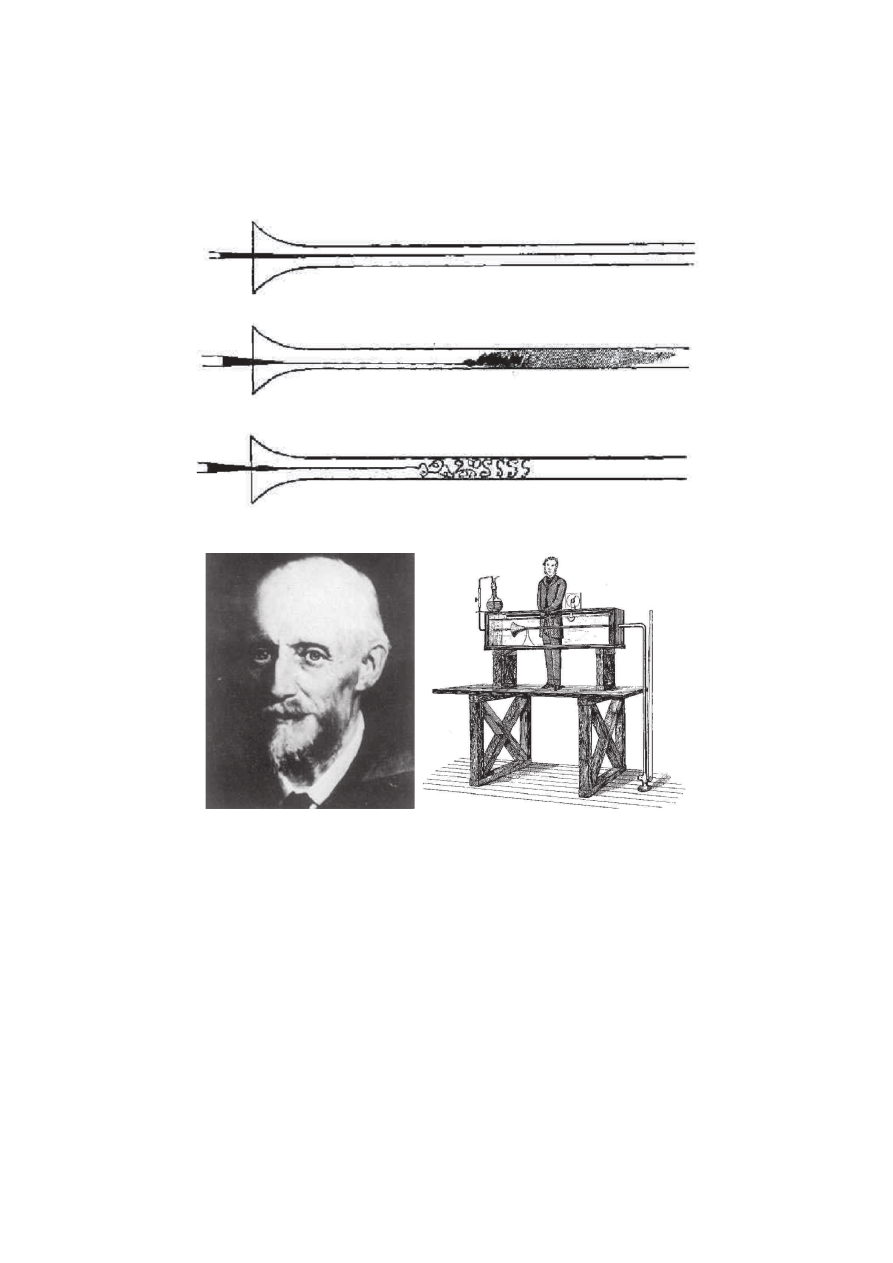
The onset of turbulence in laminar flow was studied experimentally by

Osborne Reynolds in the 1880s. By injecting dye in a flow through a trans-
parent pipe of a certain length, Reynolds could trace streamlines of the flow
through the pipe, and thus observe the straight streamlines of laminar inlet
flow starting to fluctuate into irregular motion downstream. Reynolds thus
could study transition from laminar to turbulent flow, see Fig. 7.1.

Reynolds tried to find a connection between transition and the Reynolds

number Re

≡

U L

ν

, where U represents a characteristic flow velocity and L

a characteristic length scale. Reynolds found that transition occurred if Re
was large enough (usually in the range 10

2

− 10

3

), but his hope to determine

a critical value of Re, above which transition would always occur and never
below, turned out to be elusive. We will explain in Chapter 36 below in a
detailed study of transition, why this is impossible. In short, the reason is
that transition occurs if a product of perturbation growth and perturbation
level is above a certain threshold, and only the perturbation growth can be
connected to Re. In Reynolds’ experiments the perturbation level varied from
one day to the other, and thus the transition clould occur at a certain Re one

52

7 Laminar and Turbulent Flow

Fig. 7.1. “This is a definite relation of the exact kind for which I was in search.
Of course without integration the equations only gave the relation without showing
at all in what way the motion might depend upon it. It seemed, however, to be
certain, if the eddies were due to one particular cause, that integration would show
the birth of eddies to depend on some definite value of U L/ν” (Osborne Reynolds,
1842–1912).

7.2 Applications and Reynolds Numbers

53

day, but not the next. Therefore Reynolds’ idea of a critical value of Re for
transition will have to be abandoned.

As a consequence, there is no precise value of Re indicating the presence

of turbulence in a given flow, but most flows exhibit turbulent flow features
for Re

≥ 10

3

, because perturbations are always present in both practice and

controlled experiments, albeit on different levels. Kolmogorov conjectured in
his famous 1941-articles [75, 76, 74] that turbulent flow features occur on a
range of length scales down to a smallest scale, which may be estimated to
be of size Re

−3/4

(or ν

3/4

), normalizing to U = L = 1. We can thus use the

rough size of Re to indicate the qualitative nature of a given flow, such as the
presence and scale features of turbulent flow.

7.2 Applications and Reynolds Numbers

Important applications concern fluid flow around bluff bodies, such as the flow
of air around a car, a jumbo-jet at take off/landing or the sail of a sailing boat,
or the flow of water around a super-tanker or a sailing boat, which all represent
incompressible partly turbulent flows at large Reynolds numbers: Re

≈ 10

6

for a car traveling at 60 mph, Re

≈ 10

8

for a jumbo jet or a super-tanker

at cruising speed, while Re

≈ 10

10

might be relevant in meteorology. In bluff

body flow, turbulence typically appears in a boundary layer close to the surface
of the body, and in a wake attaching to the rear of the body, while the flow
elsewhere is laminar, see Fig. 7.2. Typically the boundary layer is laminar on
the body surface facing the flow, with streamlines following the surface, until
separation away from the surface into recirculating turbulent flow. For very
high Re (

∼ 10

6

) the boundary layer may undergo transition to turbulence

before separation, resulting in a delayed separation of the boundary layer,
corresponding to a drastically reduced volume of the wake, and thus also a
reduction of the drag force, referred to as drag crisis.

In everyday life, we can observe separating laminar/turbulent bluff body

flow around a boat, a stone in a river, or a car in the case of light rain or mist
when the flow pattern becomes visible, see Fig. 7.2–7.3.

In this book we focus on flows with medium (say Re

≈ 10

2

− 10

4

) over

large (say Re

≈ 10

4

− 10

6

) to very large (say Re > 10

7

) Reynolds numbers

involving both laminar and turbulent flow features, which appear in many
important applications. For short we refer to such flows as turbulent flows.
Such flows typically have surfaces separating laminar and turbulent flow, see
Fig. 7.2. For very large Reynolds numbers we use the Euler equations, formally
corresponding to Re =

∞.

Normalizing to U = L = 1, we thus focus on flows with medium small

viscosity (ν

≈ 10

−2

− 10

−4

), over small (ν

≈ 10

−4

− 10

−6

) to very small

viscosity or zero viscosity (ν = 0), that is, we focus on small viscosity. We
shall see that the precise value of the small viscosity, or the large Reynold’s
number, in many cases is irrelevant. As indicated, this relieves us from the

54

7 Laminar and Turbulent Flow

Fig. 7.2. Lockheed L-1011 and F1 racing car (upper), vorticity in computations
of flow past square and circular cylinders and a sphere, transversal velocities in
a boundary layer computation, and in a computation of flow past cylinder rolling
along ground (modeling a wheel).

7.2 Applications and Reynolds Numbers

55

difficult (or simply impossible) task of determining a precise value of the
viscosity ν to put into the NS equations.

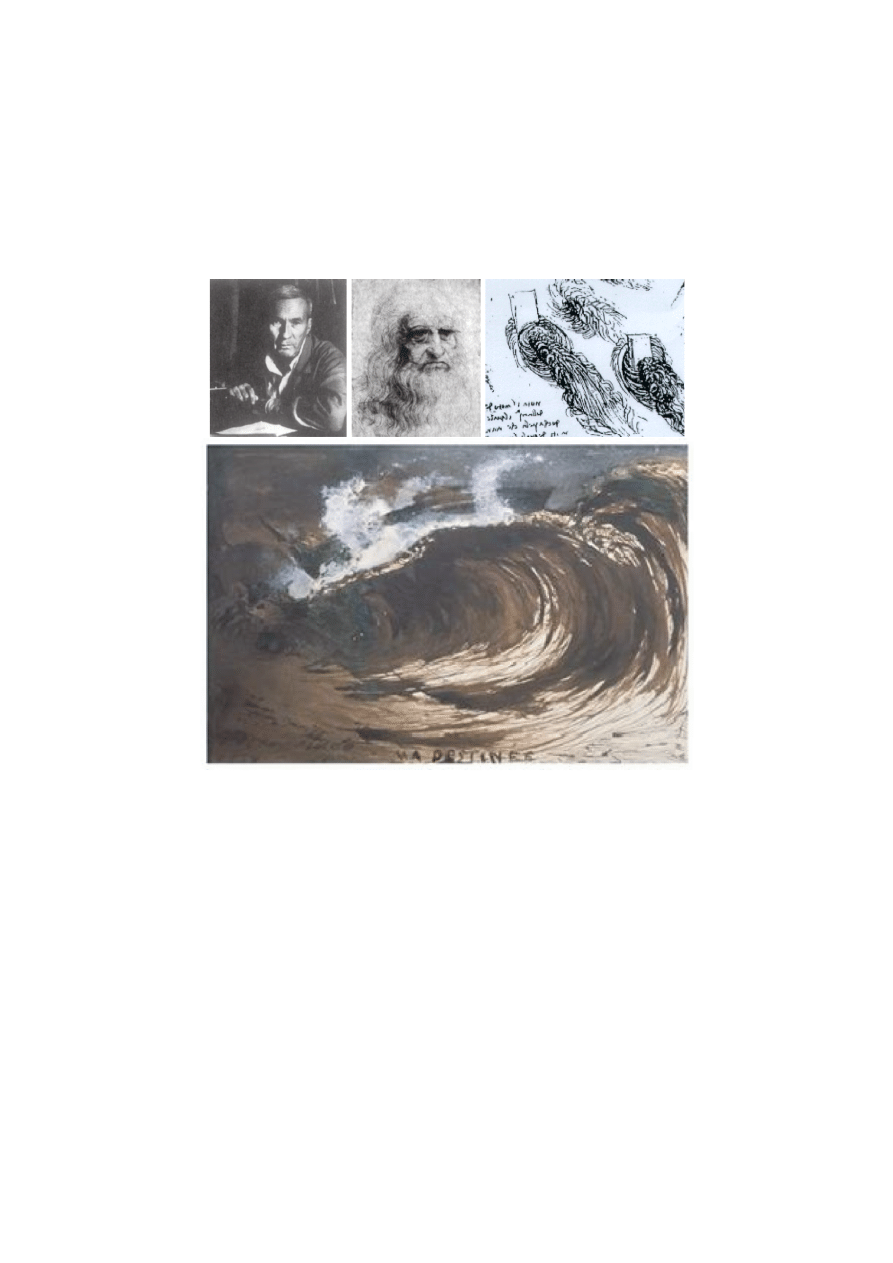
Fig. 7.3. Andrey Nikolaevich Kolmogorov (1903–1987), and Leonardo da Vinci
(1452–1519) with a sketch of turbulent wakes behind bluff bodies, and “My Destiny”
by Victor Hugo. Inscription on the ship; FRACTA SED INVICTA.