Flow Measurement

• Direct Method
• Restriction Flow Meter

– Orifice Plate
– Flow Nozzle
– Venturi

• Linear Flow Meters
• Traversing Methods

Direct Method

Liquid

Gas

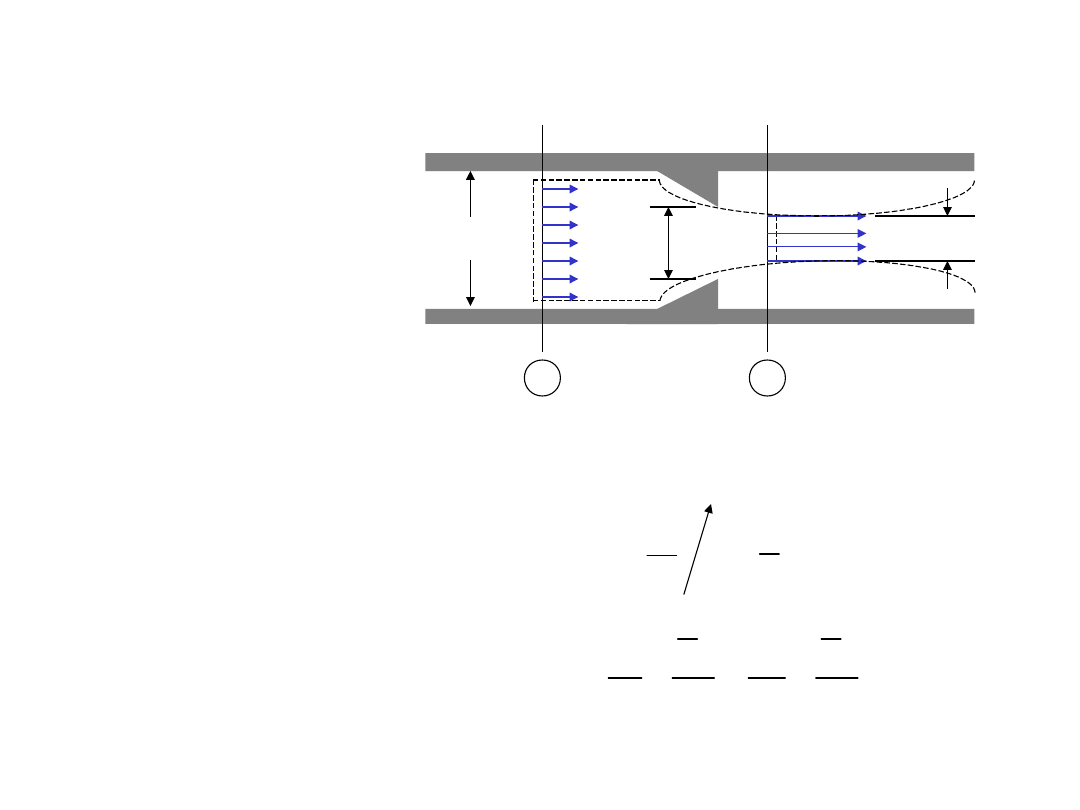
Restriction Flow
Meters

1

2

V

1

V

2

D

1

D

2

D

t

Vena contracta

Assumptions:
(1)

Steady flow

(2)

Incompressible flow

(3)

Flow along a streamline

(4)

No friction

(5)

Uniform velocity at 1

and 2

(6)

Uniform pressure at 1

and 2

(7)

z

1

=z

2

Theoretical Flow Rate

Basic equations:

2

2

2

2

2

2

1

1

V

p

V

p





























CS

CV

A

d

V

V

d

t





0

= 0

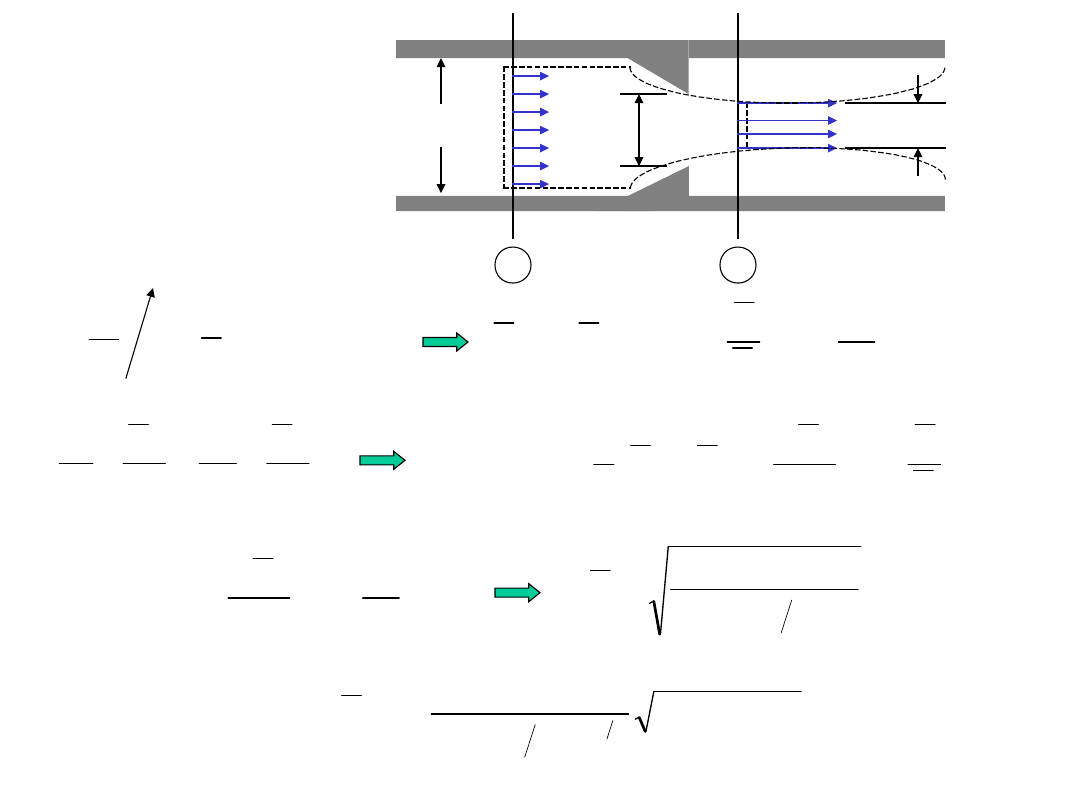
1

2

V

1

V

2

D

1

D

2

D

t

Vena contracta

2

2

2

2

2

2

1

1

V

p

V

p





























CS

CV

A

d

V

V

d

t





0

= 0

2

2

1

1

A

V

A

V















































2

2

1

2

2

2

1

2

2

2

1

1

2

2

V

V

V

V

V

p

p

2

1

2

2

2

1

or



























A

A

V

V



































2

1

2

2

2

2

1

1

2

A

A

V

p

p

Theoretical Flow Rate









]

1

[

2

2

1

2

2

1

2

A

A

p

p

V

















2

1

2

1

2

1

2

2

2

2

l

theoretica

2

]

1

[

p

p

A

A

A

A

V

m















Theoretical Flow Rate vs(versus) Actual Flow Rate









2

1

2

1

2

1

2

2

l

theoretica

2

]

1

[

p

p

A

A

A

m



















2

1

2

1

2

1

actual

2

]

1

[

p

p

A

A

CA

m

t

t











C: discharge coefficient

Let  = D

t

/D

1

,

then (A

t

/ A

1

)

2

= (D

t

/D

1

)

4

=



4





2

1

2

1

4

actual

2

]

1

[

p

p

CA

m

t













factor

-approach

elocity-of







:

1

1

4





2

1

actual

2

p

p

KA

m

t









2

1

4

]

1

[ 





C

K

C: discharge coefficient

For the turbulent flow regime,

n

D

b

C

C

1

Re







Flow-coefficient equation has the form of

n

D

b

K

K

1

Re

1

1

4











Subscript  denotes the coefficient at infinite Reynolds number.

Flow Meter Installation

• Flow meter coefficients are measured

with fully developed turbulent velocity
distributions at the meter inlet.

• If installed downstream from a valve, a

fitting, or other disturbance, a straight
section pipe must be placed in front of the
meter.

– For venturi meters, L

straight pipe

 10 D

– For orifice-plate or flow nozzle meters,

L

straight pipe

 40 D

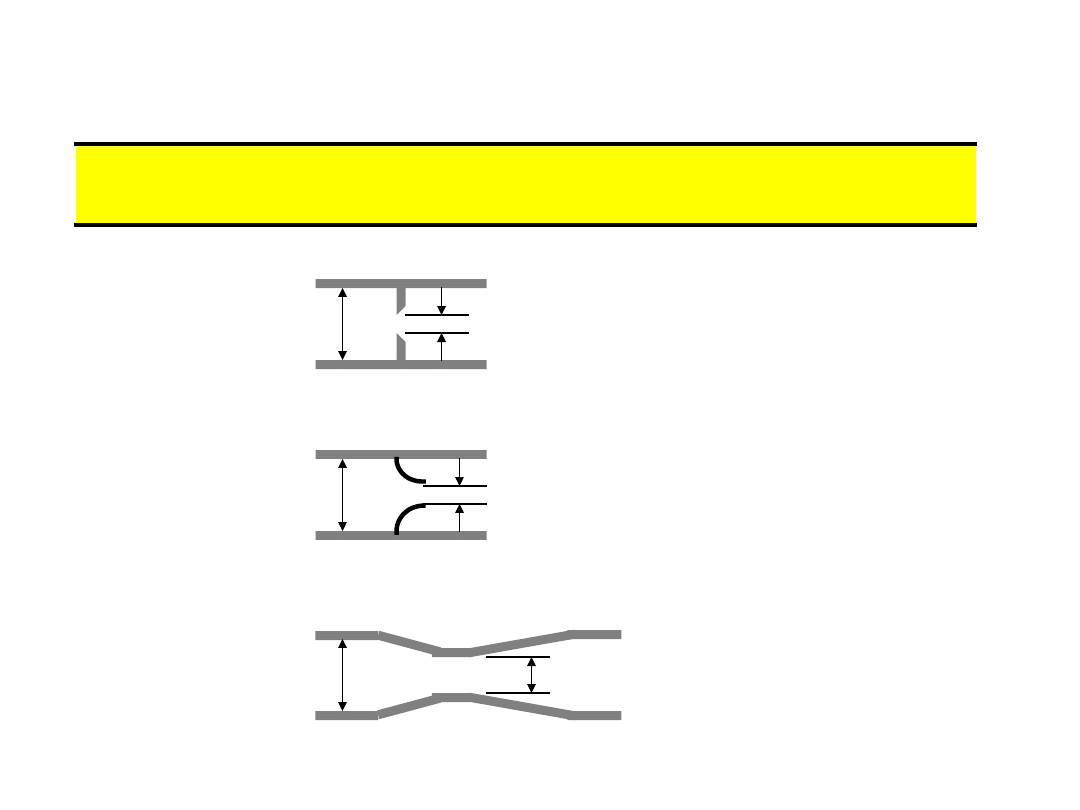
Flow Meter Type

Head Loss

Cost

D

1

D

t

Orifice

High

Low

D

1

D

t

Flow Nozzle

IntermediateIntermediate

D

1

D

t

Venturi

Low

High

Characteristics of Restriction Flow Meters

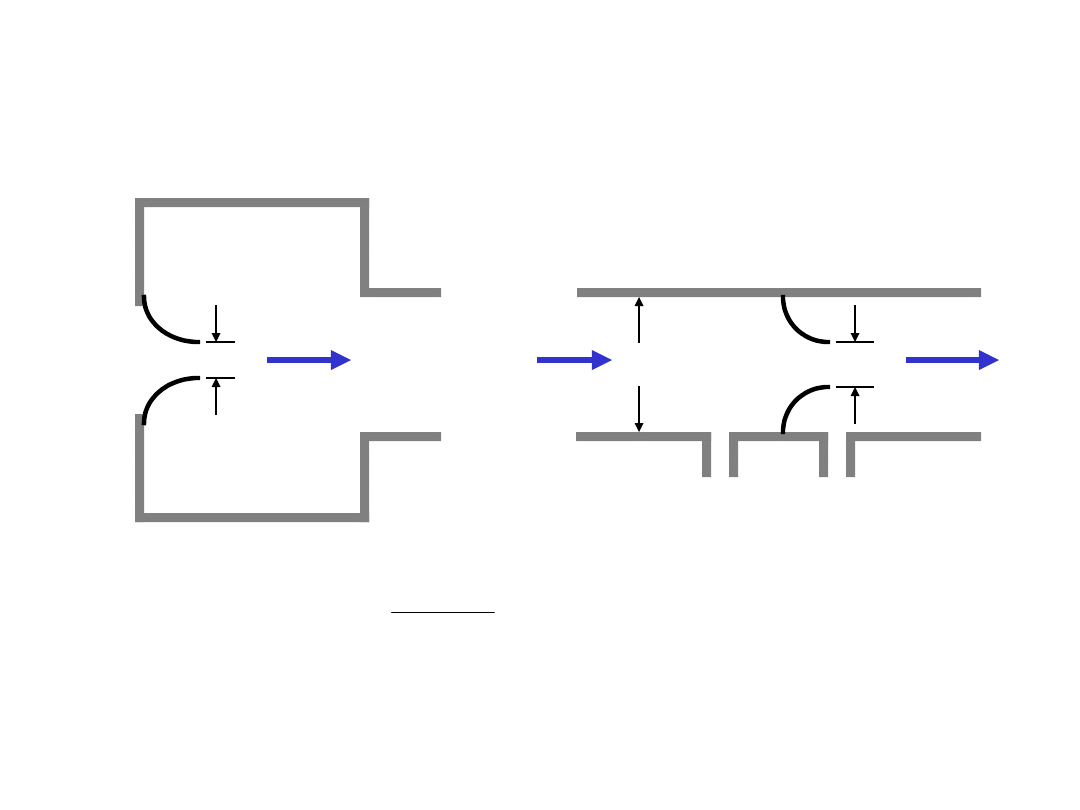
The Orifice Plate

D

2

D

''

1

''

1

Flow

D and D/2 taps

Corner taps

Flange taps

75

.

0

5

.

2

8

1

.

2

1

Re

71

.

91

184

.

0

0312

.

0

5959

.

0

D

C















7

4

10

Re

10

and

75

.

0

2

.

0

For

1











D

For corner taps

The Flow Nozzle

Nozzle

2

D

1

p

0

1



V

Flow

Plenum chamber

Flow

2

D

1

D

1

V

1

p

2

p

5

.

0

5

.

0

1

Re

53

.

6

9975

.

0

D

C







7

4

10

Re

10

and

75

.

0

2

.

0

For

1











D

ASME long-radius nozzle

The Venturi

• Discharge coefficients range from 0.98

to 0.995 at high Reynolds numbers.

• All restriction flow meters produce

pressure differentials proportional to
the square of the flow rate.

• A meter must be chosen to

accommodate the highest expected
flow rate.

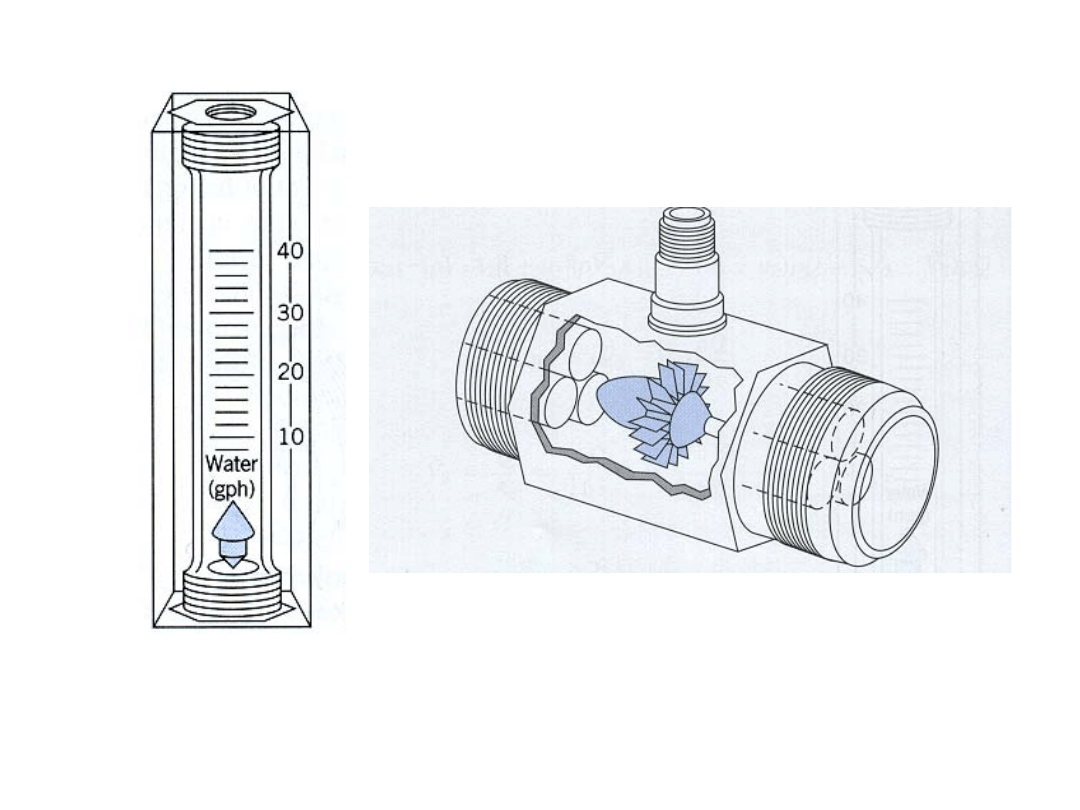
Linear Flow Meters

• Flow meters produce outputs that

are directly proportional to flow
rate.

• Examples of linear flow meter

– Float meter (rotameter), turbine flow

meter, vortex flow meter,
electromagnetic flow meter,
ultrasonic flow meter.

Traversing Methods

• The duct cross section is subdivided

into segments of equal area; the
velocity is measured at the center of
each area segment using a pitot tube
or a suitable anemometer.

• Flow meter can be used for traversing

method

– Pitot tube, thermal anemometer, Laser

Doppler anemometer.

Homework: 8.88, 8.145, 8.159, 8.167