1

Lecture 15

Geometry of Space

Dot Product - the Euclidean space,

Vector Product,

Points in a space: Line, Plane.

2

DOT (INNER, SCALAR)
PRODUCT IN R

n

Definition
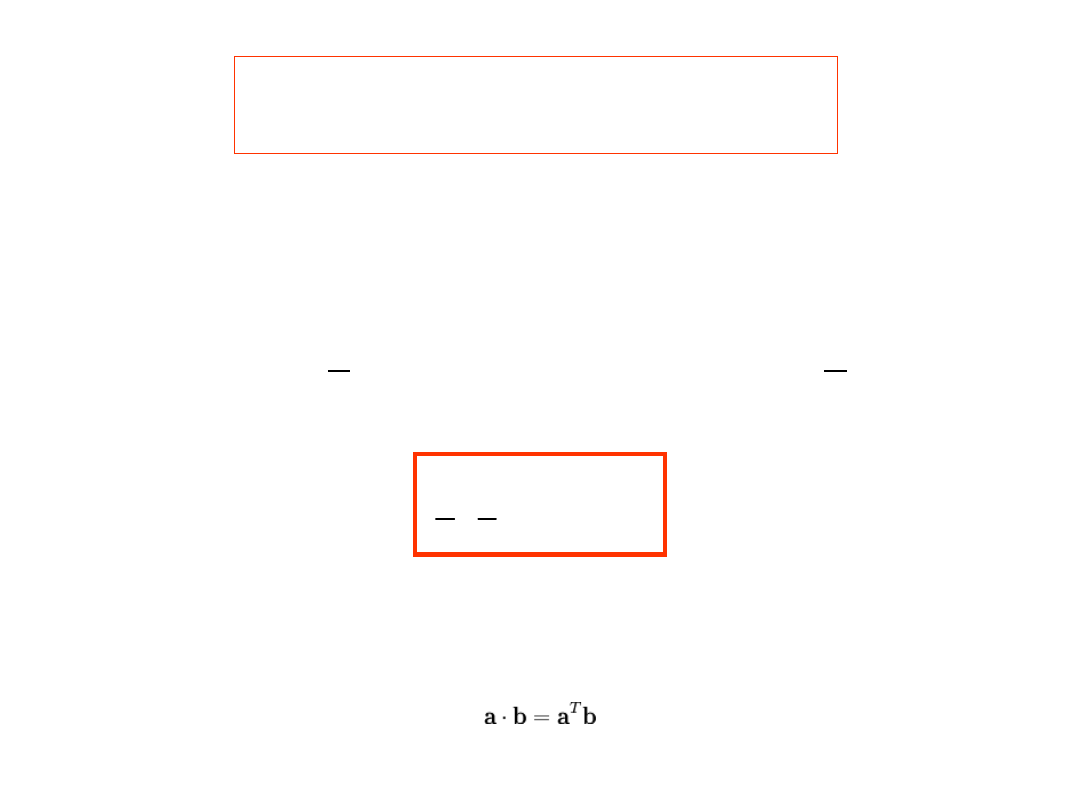
The dot product (scalar, inner product) is a function
which assigns to two vectors, one real number.

For two vectors a = ( a

1

, a

2

, ....., a

n

) and b = ( b

1

,

b

2

, ....., b

n

) we define the dot product • : R

n

x R

n

 R

as:

.

1









n

i

i

i

b

a

b

a

Using matrix multiplication and treating the (column) vectors as n×1
matrices, the dot product can also be written as:

where a

T

denotes the transpose of the matrix a.

3

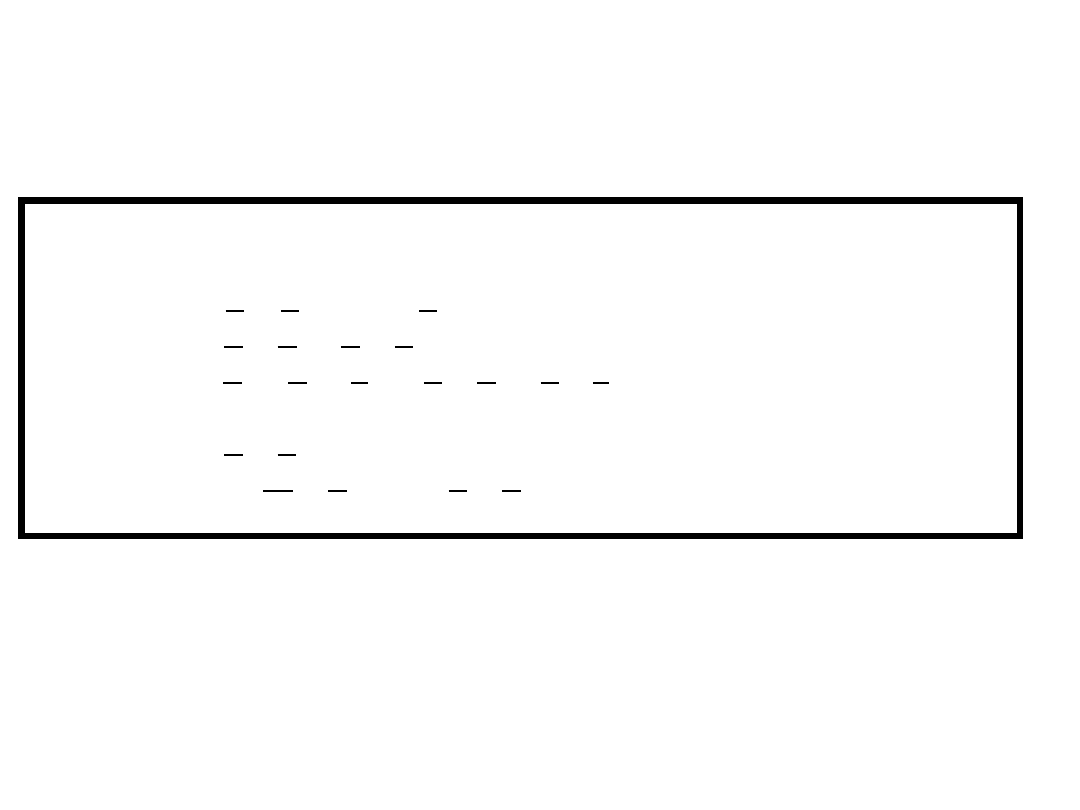
Properties of the scalar product:

(i)                a • a = 0  a = 0,

(ii)                a

•

b = b

•

a (commutation),

(iii)               a

•

(b + c) = a

•

b + a

•

c (distribution with respect

to addition),
(iv)               a

•

a  0,

(v)               (  a)

•

b =  ( a

•

b).

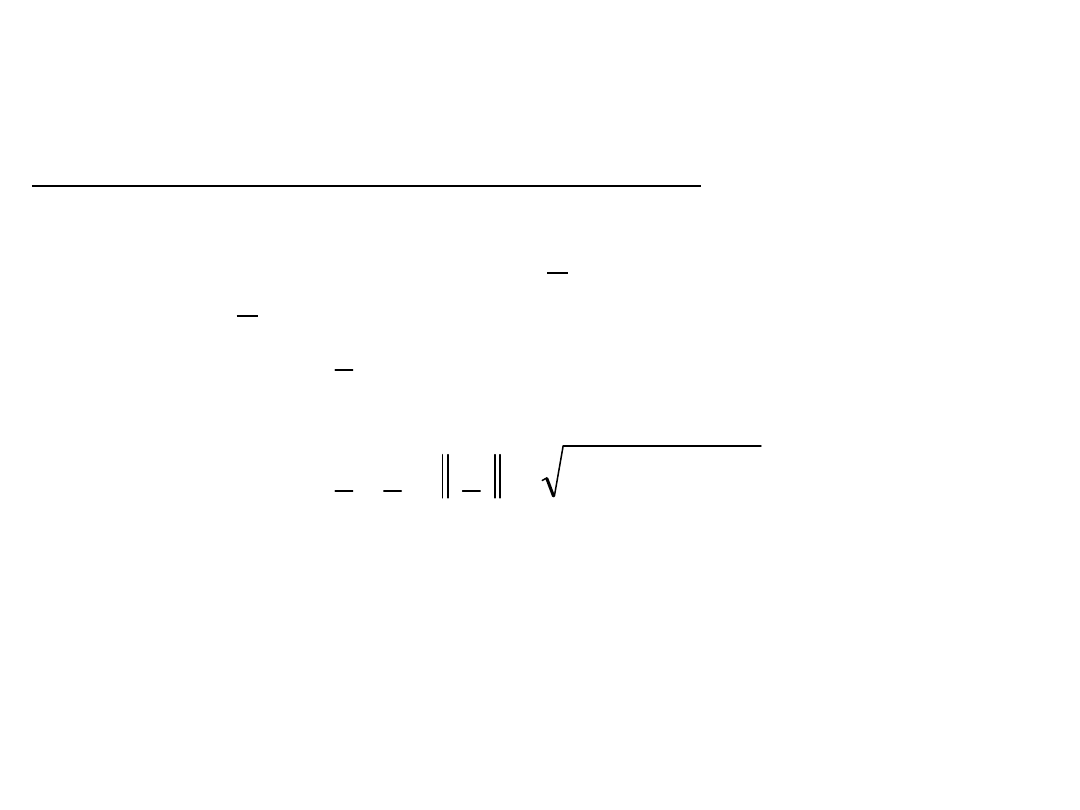
4

1. Length/Magnitude of a Vector:
                                                      
The dot product of a vector v with itself is equal to
its length ||v||:

Geometrical interpretations in R

3

2

3

2
2

2

1

3

2

1

)

,

,

(

v

v

v

v

v

v

v

v

v

v













2. The dot product does not change under isometric
changes of the basis: rotations, reflections, and
combinations, keeping the origin fixed.

5







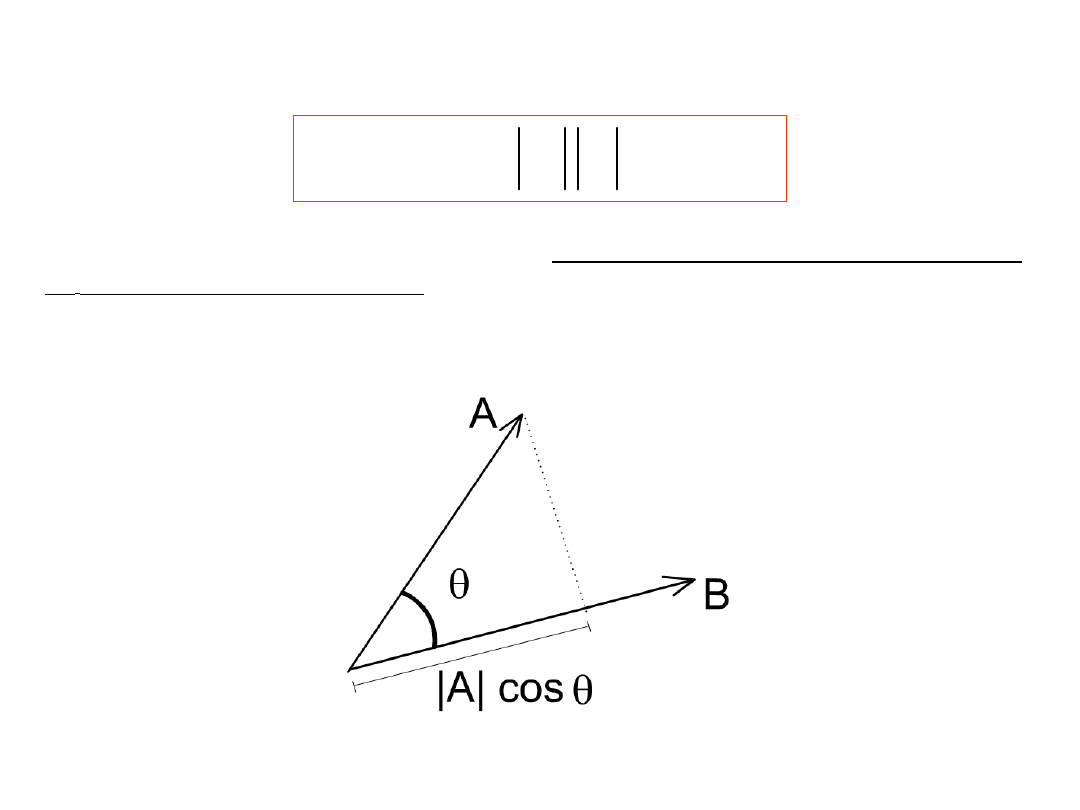
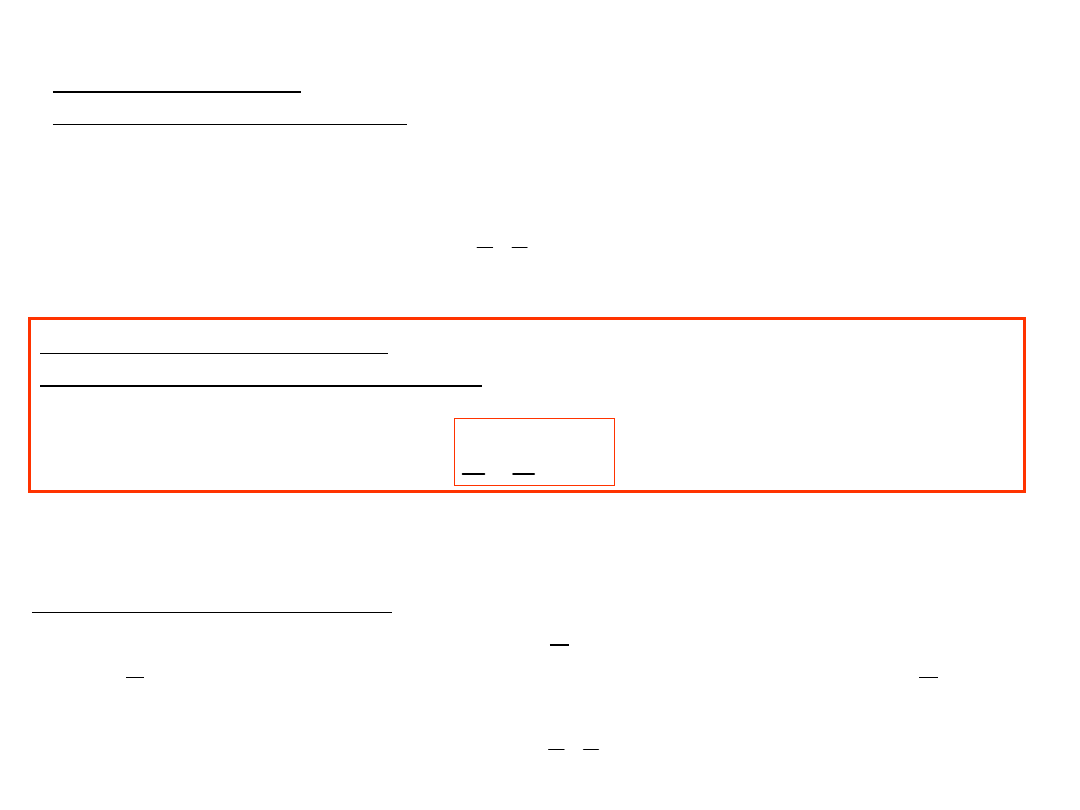
cos

B

A

B

A

4. The dot product of two vectors is the length of the projection of
A

 

onto the unit vector B when the two vectors are placed so that

their tails coincide.

3. For R

3

it is easliy shown that

6

Parallel Vectors:

 

                 

When a and b are parallel to each other, their dot product is identical
to the ordinary multiplication of their sizes, since  = 0

o

and cos 0

o

= 1.

Perpendicular Vectors:

 

             

When a and b are perpendicular to each other, their dot product is
always zero, since = 90

o

and cos 90

o

= 0.

Component of a Vector:

 

                

The component of a along a direction d is equal to the dot product of the
vector a and the unit vector which points along the direction of d .

ab

b

a





0



b

a

d

a

a

d





7

Definition MEASURE OF ANGLE BETWEEN
VECTORS
Let a, b be non-zero vectors from R

n

.

Definition
The measure of the angle between vectors a and b is a real
number φ 

0 which satisfies the equality:

.

b

a

b

,

a

cos







8

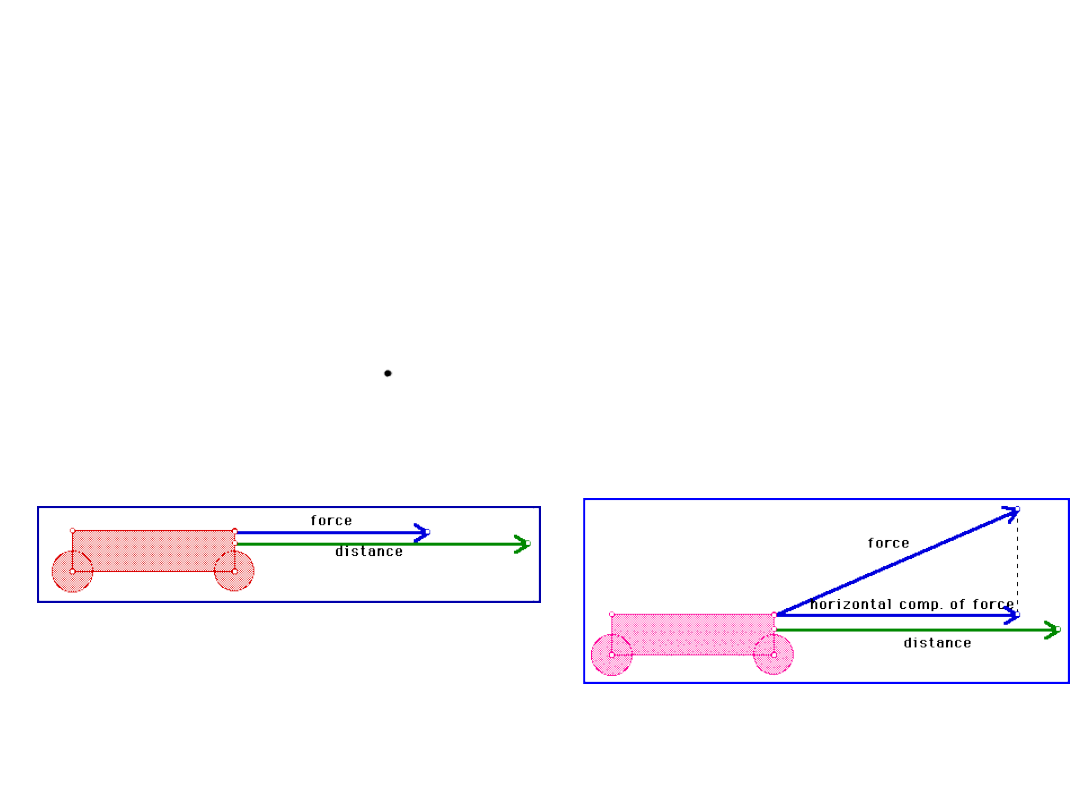
What Good Are Dot Products?

•The dot product formula is easy to compute and gives you information
about the angle between vectors, to know whether or not two vectors
are perpendicular .

•The dot product tells you about work. If you have a constant force
applied directly in the direction an object is moved, then
the amount of work = (force) (distance).   

9

Backface culling
When deciding if a polygon is facing the camera, you need only calculate
the dot product of the normal vector of that polygon, with a vector from
the camera to one of the polygon's vertices. If the dot product is less than
zero, the polygon is facing the camera. If the value is greater than zero, it
is facing away from the camera.

The dot product is used in the lighting calculations and backface
removal in 3D graphics. It is also used in mechanics

10

Definition

The distance between vectors u and v  E is the value

d (u , v,) defined as

.

)

,

(

v

u

v

u

d





11

An n-dimensional space R

n

with notions of

distance and angle is called an n-
dimensional Euclidean space.

DEFINITION
The space R

n

with the scalar product

where a = [ a

1

, ....., a

n

] and b = [ b

1

, ....., b

n

] is a Euclidean space

and will be denoted as E

n

.

Definition
Let v be a vector from E

n

. The norm (length) of vector v is

the value of

v

v

v





.

1









n

i

i

i

b

a

b

a

12

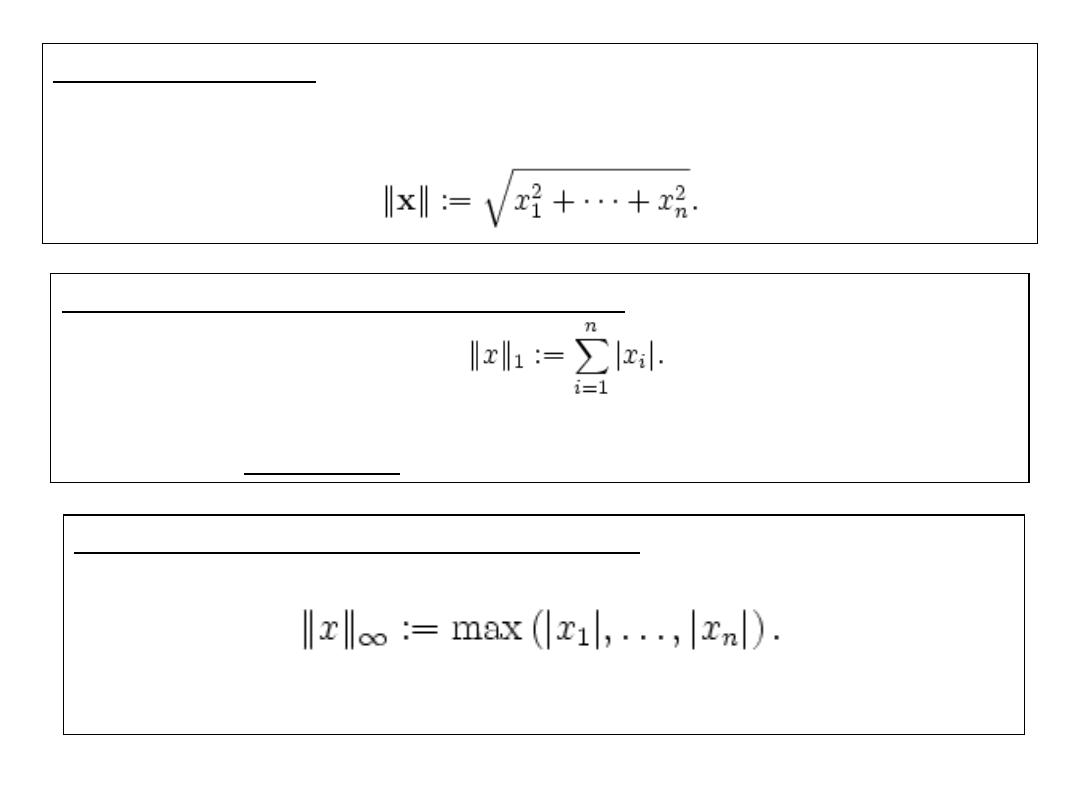
Euclidean norm
On R

n

, the intuitive notion of length of the vector x = [x

1

, x

2

, ...,

x

n

] is captured by the formula

  

                            

Taxicab norm or Manhattan norm

 

                   

The name relates to the distance a taxi has to drive in a
rectangular street grid to get from the origin to the point x.

Maximum norm chessboard norm

 

                                    

The distance between squares,in terms of moves necessary
for a king

13

 

                       
Illustrations

of

unit circles

in different

norms

.

DEFINITION:
A circle is a set of points which
are at a fixed distance R from
the centre.

14

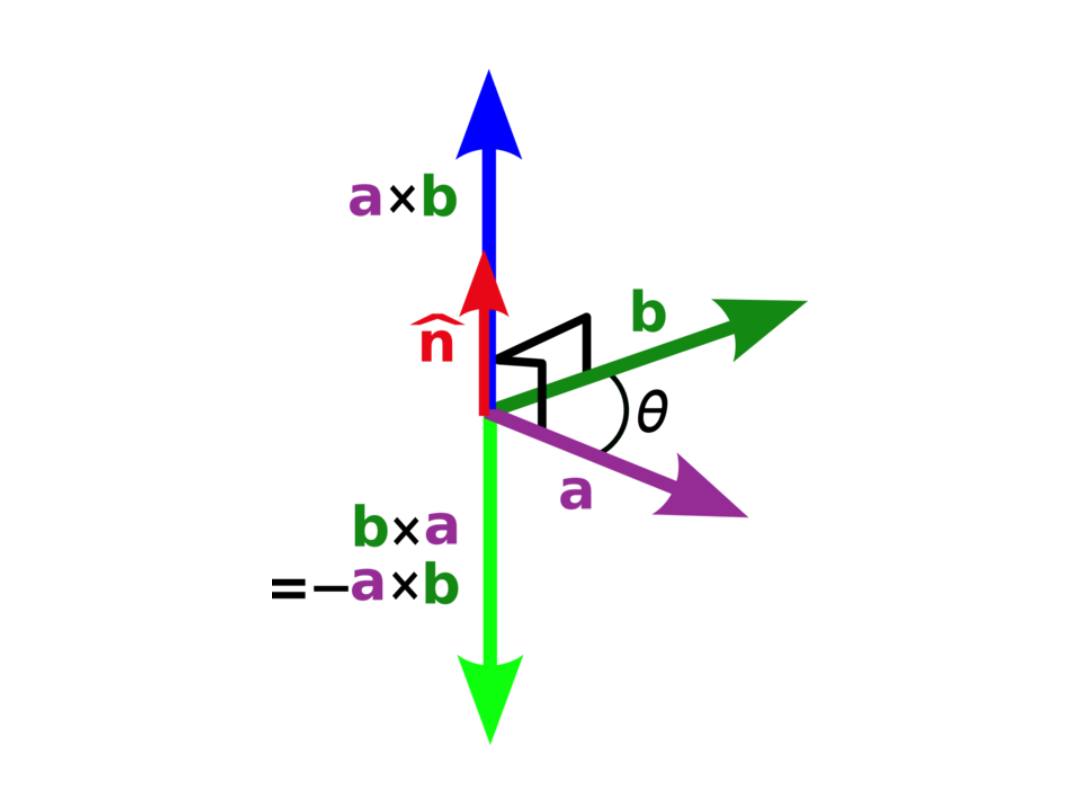
Vector Product

15

VECTOR PRODUCT IN E

3

A vector product in E

3

is a transformation which

assigns to two vectors

a, b

from E

3

, a vector from

E

3

.

3

3

3

:

E

E

E

b

a







16

17

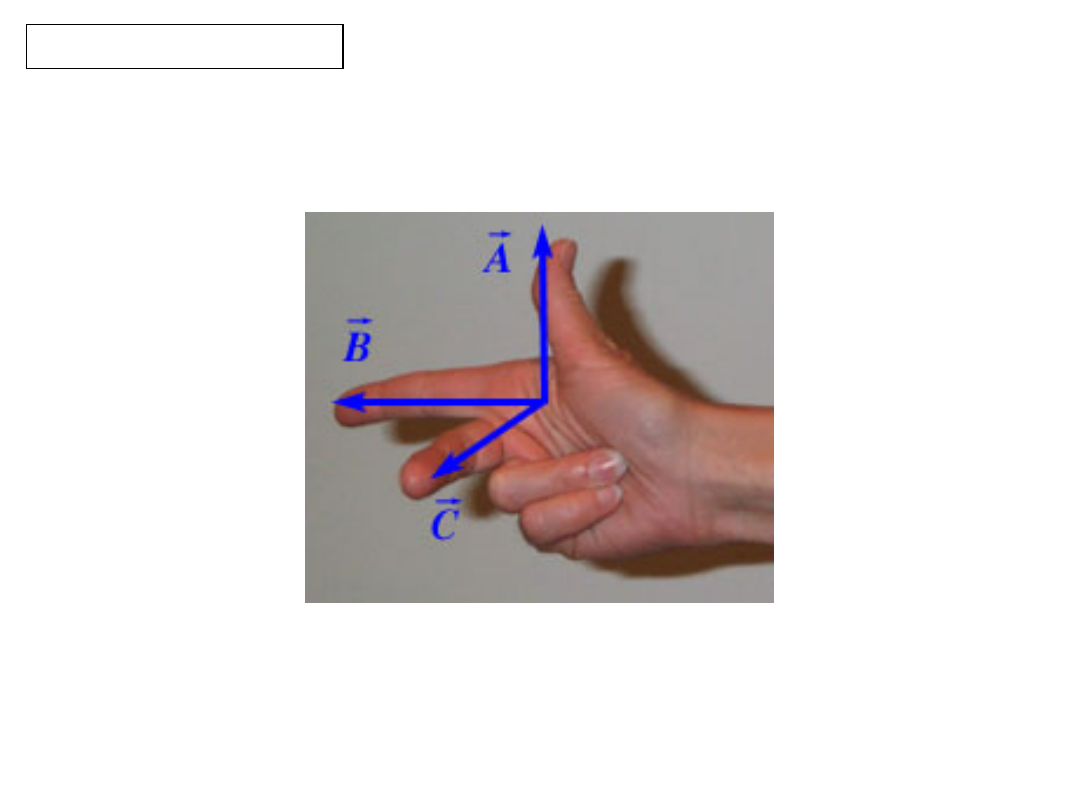
C

B

A











The length of is equal to the area of the rectangle formed by

A



C

and

B





Rule of the right hand

18

Definition

Let a = (a

1

,a

2

,a

3

) and b = (b

1

,b

2

,b

3

), we denote the vector

(outer) product as:
a x b

and we call it a vector product (outer product) of vectors a i b.





















































2

1

2

1

1

3

1

3

3

2

3

2

b

b

a

a

det

,

b

b

a

a

det

,

b

b

a

a

det

b

a

Example:

Find the vector product of the
vectors

According to the definition:









.

5

,

0

,

4

,

1

,

2

,

3









b

a





8

,

11

,

10

0

4

2

3

det

,

4

5

3

1

det

,

5

0

1

2

det































































b

a

19

a) a x b = - ( b x a )

anticommutativity
b) a x ( b + c ) = a x b + a x c

distributivity over

addition
c) ( a ) x b =  ( a x b )
d) < a, b  c > = < b, c  a > = < c, a x b >.

PROPERTIES

20

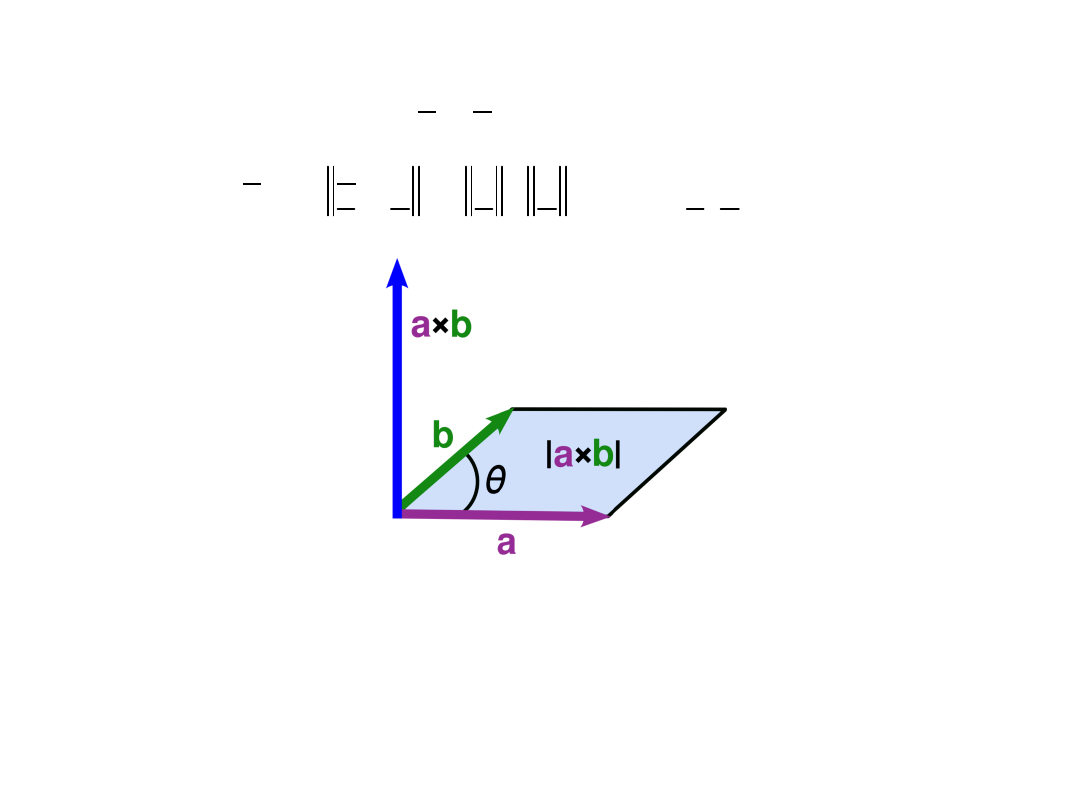
1. The length of a vector a x b equals the area of a parallelogram
defined
by vectors a and b.

 

b

,

a

sin

b

a

b

a











2. The cross product of any two vectors in the xy-plane will be
parallel to
the z axis.

21

.

3

2

1

3

2

1

3

2

1

























b

b

b

a

a

a

e

e

e

b

a

Can also be written as

In the Cartesian coordinate system the vector product of
vectors:

),

,

,

(

)

,

,

(

3

2

1

3

2

1

b

b

b

b

a

a

a

a





22

It should be emphasized that unlike the dot product
of two vectors, which is a number, the vector
product is a vector.

Let us note that for any two linearly dependent
vectors
a i b we have
a x b = 0
(see definition).

In particular a x a = 0.

23

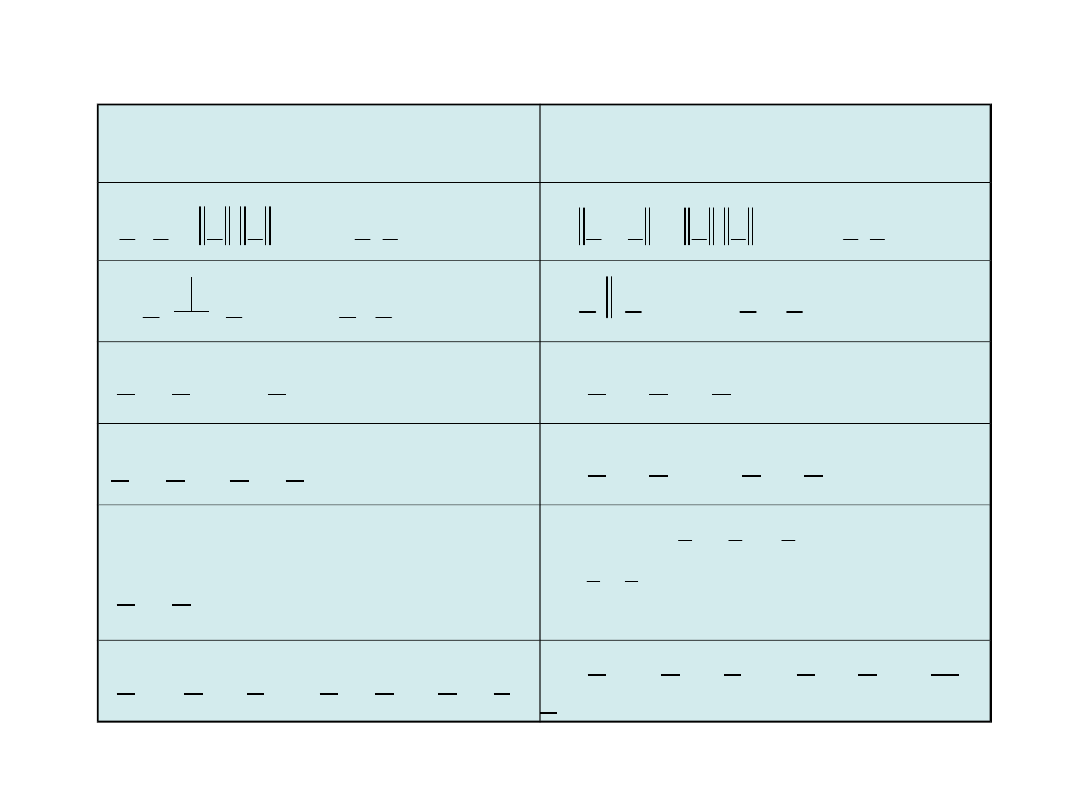
dot product

vector product

a • a =  a 

2

a  a = 0

 

a • b = b • a

a  b = - b  a

a • b = a

1

b

1

+ a

2

b

2

+ a

3

b

3

a • (b + c) = a • b + a • c

a  (b + c) = a  b + a 
c

 

b

a

b

a

b

a

,

cos





 

b

a

b

a

b

a

,

sin









.

3

2

1

3

2

1

3

2

1

























b

b

b

a

a

a

e

e

e

b

a

0







b

a

b

a

0







b

a

b

a

24

A Plane in E

3

25

Let us consider a linear space not only with vectors but also with points.

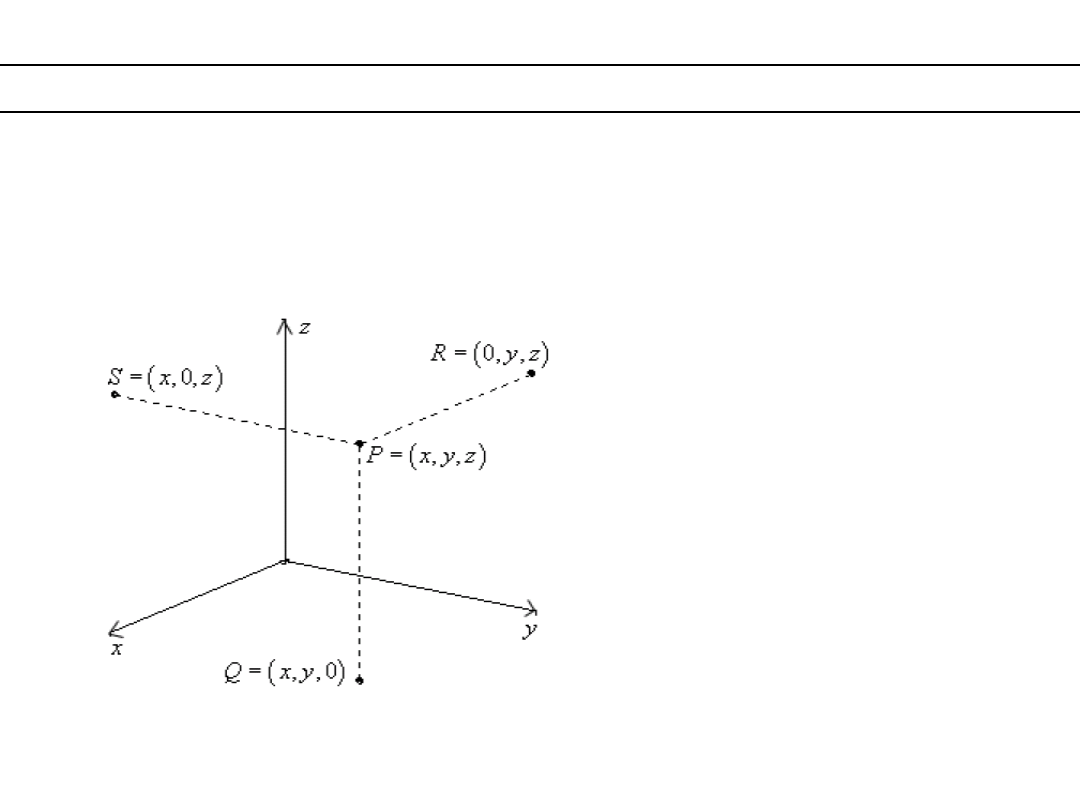
26

Point P with coordinates (x,y,z)

Let us consider a linear space not only with vectors but also with points.

27

Let us consider a point

P

and a vector v from the

space R

n

:

P

= (p

1

,p

2

,...,p

n

) and v = [v

1

,v

2

,...,v

n

].

Definition
A result of an action of the vector v on the point P is a
point
Q = (q

1

,q

2

,...,q

n

) E

n

such that:

[ q

1

– p

1

, q

2

– p

2

,...,q

n

- p

n

] = [ v

1

, v

2

, ….., v

n

] ,

Such action is denoted as:

Q = P

 v and we say that the

vector v is attached to the point

P

and has its end in the

point

Q

.



28

].

,

,

,

[

2

2

1

1

n

n

p

q

p

q

p

q

PQ











So , we take

P

= (p

1

, p

2

,..., p

n

) as the beginning (head) and

Q

= (q

1

, q

2

,..., q

n

) as the end of vector

PQ

v 

Q

= (q

1

,q

2

,...,q

n

)

P

= (p

1

, p

2

,..., p

n

)

PQ

v 

29

A straight line in the space R

n

is a subspace

generated by a single,

nonzero vector.

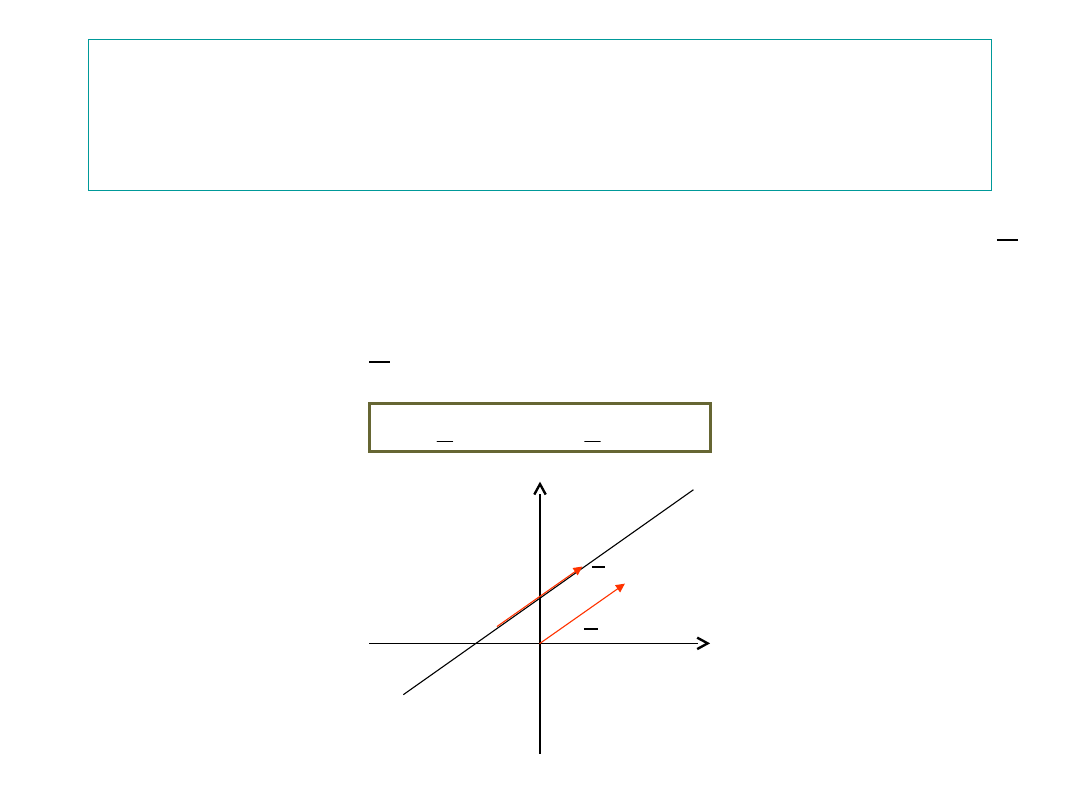
30

The equations of a line

.

In 3D the line is the intersection of two planes, or the
'variation' of a vector.

A line

L

in is determined by a point

P

and a direction vector

v

.

A point

Q

lies on a line exactly when it is a translation from

P

by some multiple of

v

.





R

t

v

t

v

L







:

P

)

,

P

(

P

P + v

v

31

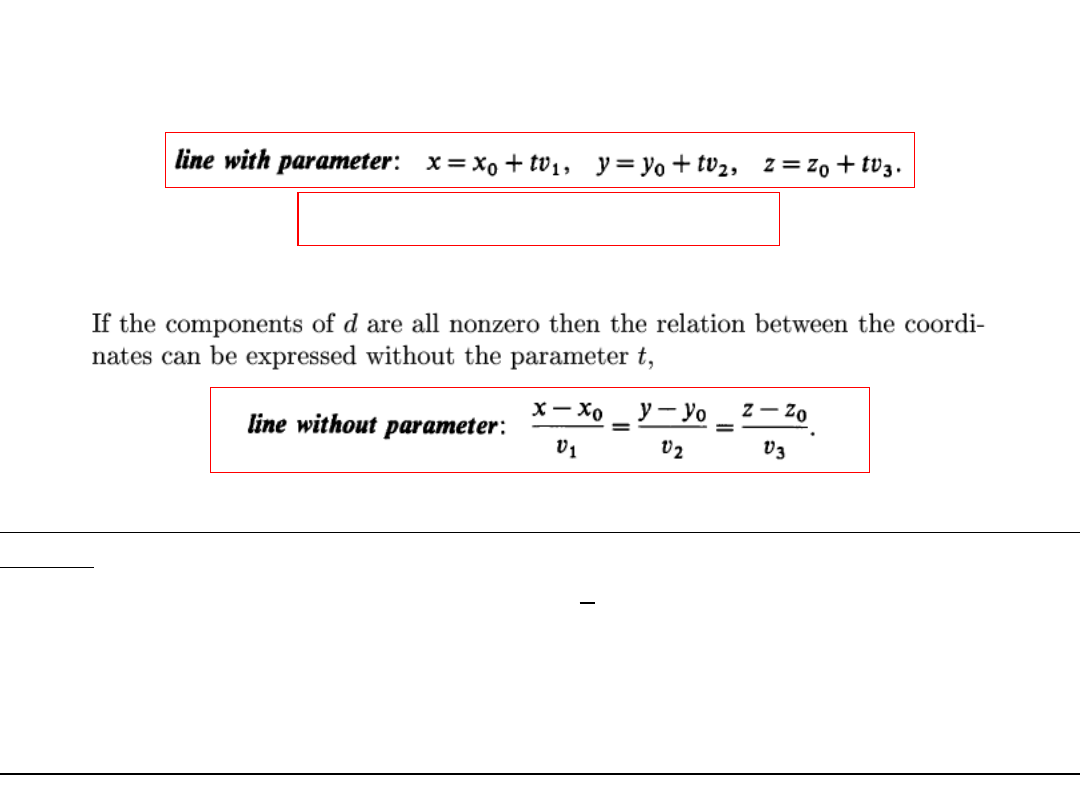
In coordinates a point

(x,y,z)

lies on a line L

((x

0

,y

0

,z

0

), ((v

1

,v

2

,v

3

))

when

for some

t

in

R

.

(x,y,z) = (x

0

,y

0

,z

0

) + t (v

1

,v

2

,v

3

)

Example
The line through

P

= (1,1,1) in the direction v = (1,2,3) consists of all points (x,y,z)

satisfying
(x,y,z) = (1,1,1) + t (1,2,3) for any t  R,

or satisfying the two equations
x -1 = (y -1)/2 and (y -1)/2 = (z -1)/3.

32

Suppose a, b  R

n

are linearly independent

vectors.

Definition

A plane P(a,b) generated by a and b
is the set of vectors v of the form: v = t a + w b,
for some real numbers t, w.

P(a, b) = { v: v = t  a + w  b ; t, w

 R }.

33

Theorem
The point Q lies on the line P ( a, b ) if and only if
there exist real numbers t and w such that:

.

n

,

,

,

i

for

,

b

w

a

t

p

q

i

i

i

i



2

1

0













,

b

w

a

t

p

q

1

1

0

1

1











,

b

w

a

t

p

q

2

2

0

2

2











.

b

w

a

t

p

q

3

3

0

3

3











These equations are called the parametric equations of the plane

When we eliminate the parameters t, w from the above equations we obtain:

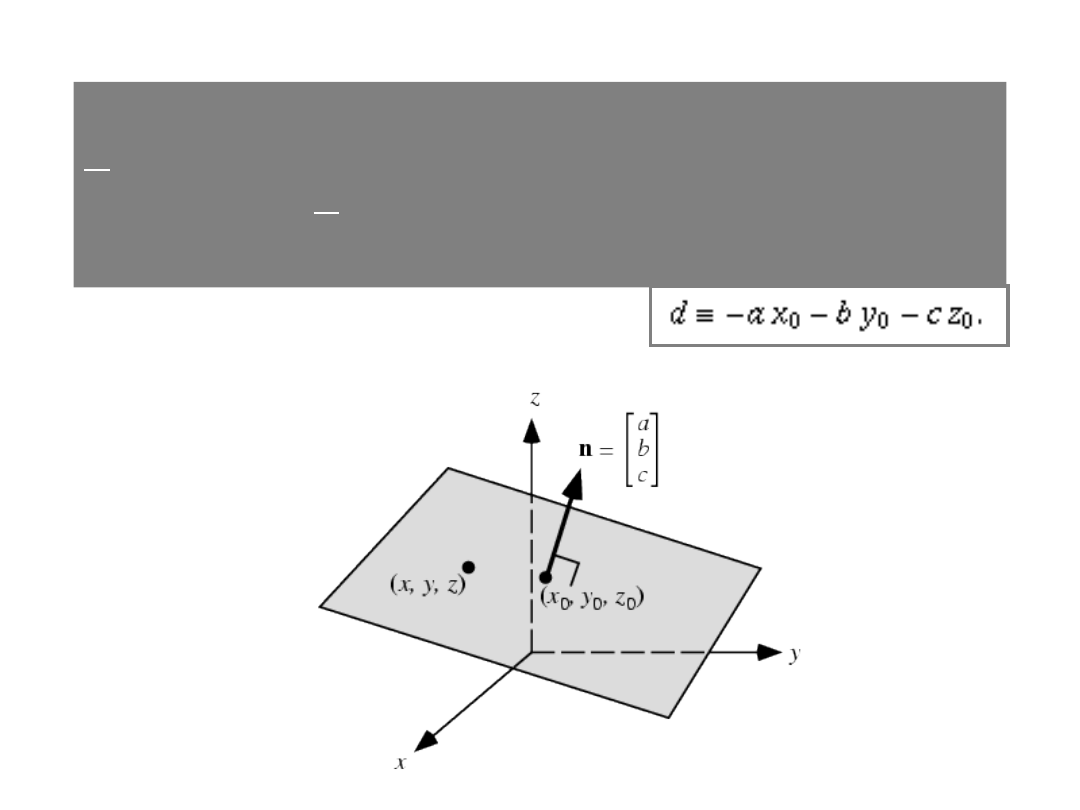
34

The equation of a plane perpendicular to a vector
n with
coefficients n = (a, b, c) is

ax + by + cz + d =0