!"$#%!

&

#%!'

)(*

+,

!"

Venice 2001

Beyond
Conventional
Adaptive
Optics

Julien Charton

a

, Eric Stadler

a

, Wilfrid Schwartz

a,b

a

CNRS-LAOG

b

LETI

ABSTRACT

The emerging MOEMS technology applied to electrostatic actuators is one of the most
promising solutions to meet the needs of deformable mirrors for future adaptive op-
tics systems in astronomy. But the low stroke and high non-linearity of "classical"
parallel plate electrostatic actuators make them difficult to use. This paper presents 2
ideas to improve electrostatic actuators using non-linear restoring force and L-C net-
work. Simulation results show that these solutions can keep parallel-plate electrostatic
actuators in the race for future deformable mirrors.

1. ASSUMPTIONS

1.1. Base line specifications

The performances required for future Multi-Conjugate Adaptive Optics Systems (MCAO) and/or Extremely Large Tele-
scopes (ELTs) are still under discussion among AO specialists. The base-line specifications assumed in this paper are
summarized in Table 1.1. But the actuator stroke required for ELTs may be lower than written here, and this question
must be clarified ASAP.

Parameter

Value

Mirror diameter

1cm to 10cm

Actuator size

around 1mm

Number of actuator

1E2 to 1E6

Actuator stroke

+/-10 micrometers

Differential stroke for

2 neighboring actuators

+/-5 microns

Table 1. Mirror specifications assumed in this paper.

These specifications are intentionally imprecise and are intended to match as much applications as possible in the field

of astronomy.

2. IMPROVING ELECTROSTATIC ACTUATORS

Classical parallel-plate actuators have to main drawbacks:

-

They are higly non-linear.

-

The force density is very low.

Two solutions are presented here to improve this kind of actuator:

-

A new structure able to provide a non-linear restoring force.

-

A new layout used to adapt L-C network to DMMs.

Further author information: send an e-mail to

[e-mail]

0

2e–06

4e–06

6e–06

8e–06

1e–05

1.2e–05

1.4e–05

1.6e–05

1.8e–05

2e–05

2.2e–05

2.4e–05

FORCE (N)

2e–06

4e–06

6e–06

8e–06

1e–05

1.2e–05 1.4e–05

GAP (m)

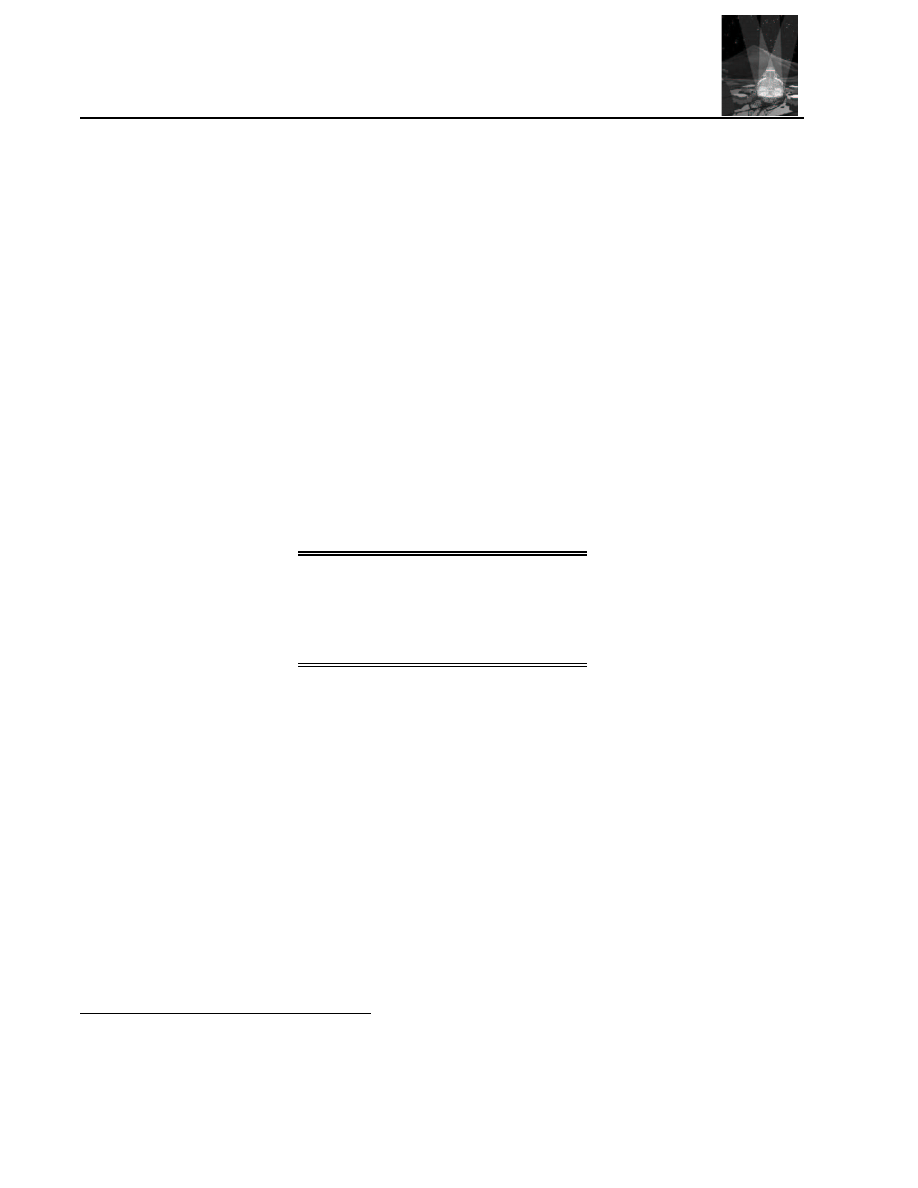
Figure 1. Electrostatic forces and restoring forces.

2.1. Non-linear restoring force

The non-linear behaviour of the classical EA can be explained as follow: We are trying to equilibrate a (highly) non-linear
electrostatic force with an (almost) linear restoring force. The Fig. 1 illustrates this concept:

The thin curves are representing the electrostatic force for different values of the control voltage. The dotted line

represents a linear restoring force. In most case, this restoring force is provided by a spring-like device, such as bending
arms or a deformable membrane. One can see that when the voltage increases, the equilibrium position (defined by the
intersection between the actuating and restoring curves) is not moving linearly. Below 2/3 of the nominal gap, there is no
more stable equilibrium and the moveable electrode collapses on the bottom electrode. If the restoring force was changing
with gap as shown by the sick continuous line, the equilibrium position will move linearly from nominal-gap to zero-gap.

2.1.1. Restoring force required to linearize the actuator

This ideal non-linear restoring force Fr can be determined by combining the expression of the required linear behavior

.0/2143657895%:

with the expressions of the electrostatic force Fe (Eq (1)

;<=/?>A@

.=B

C

5

B

(1)

This gives the expression of the required restoring force Fr:

;=D/FE

@

1,BG3IHAJK8L5%:

B

C

5

B

(2)

We must now find a mechanical device able to provide the force define by Eq (2). Fig. 2 shows an example of such a
device: A beam is attached at point A, and the force is applied to the point C. An underlying support with a special shape
provides an additional contact force in point B. By adjusting the shape of the support, it is possible to modify the stiffness
curve seen at point C. This could be used as a flexure beam in an EA, where the moving electrode would be attached to
the point C. One can note that a strict compliance with Eq (2) is impossible, because it would require a null stiffness for
g=0. But this device can match Eq (2) when g is increasing.

2.1.2. Shape of the vertical support

To simplify the expressions, we make the following change of variables:

MKN/25O8957

(3)

P

/

E

@

1,B

C

(4)

The required restoring force becomes Eq (5), and its derivative with respect to Yc gives the required stiffness (Eq (6))

Q4R"/

PS

R

B

3

S

RTHVUW:

B

(5)

A

B

C

x

y

Fc

Fb

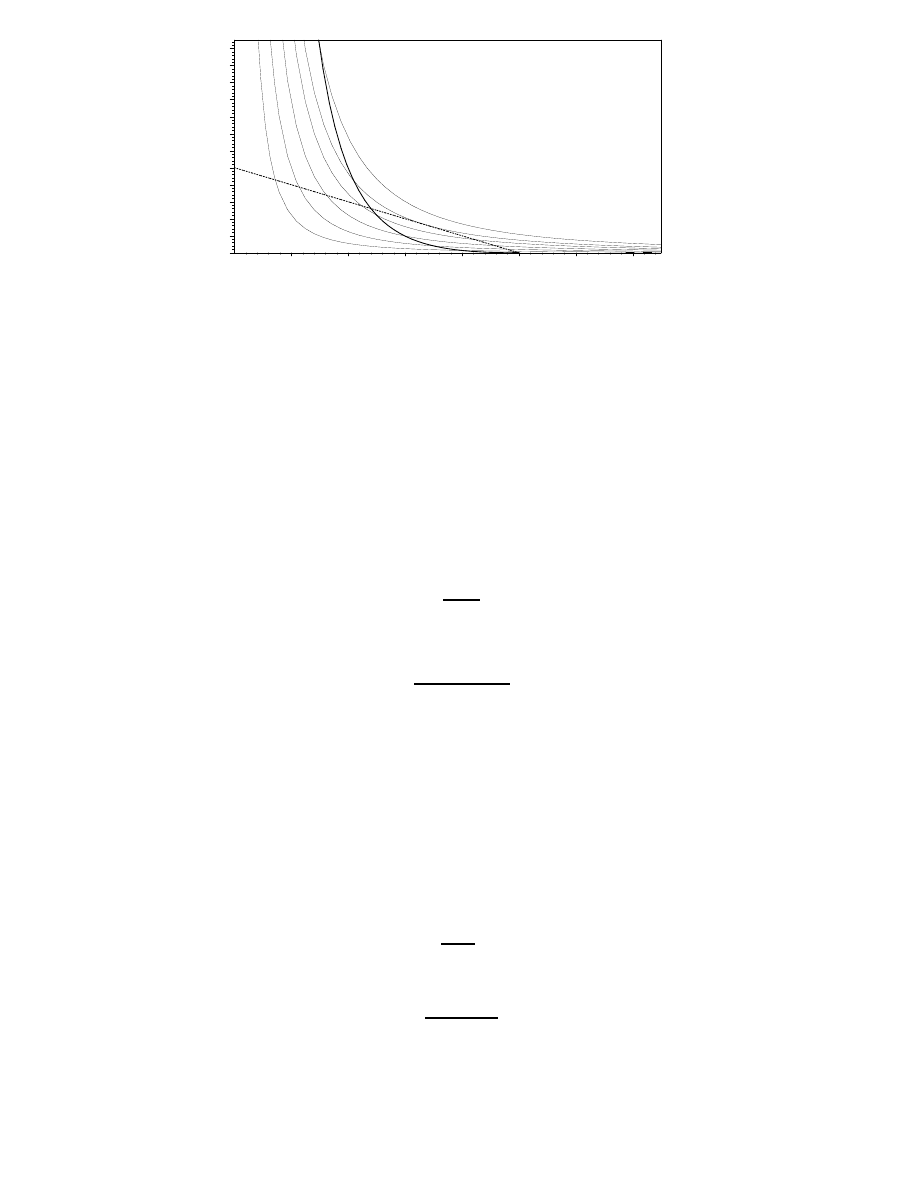
Figure 2. Non-linear restoring force: example of device.

–20

–15

–10

–5

0

Yb

100

200

300

400

500

Xb

Figure 3. Example of profile for

X

NY/[Z\]\^`_bac5

7

/

C

\A^`_9aedKfO/hgjiAk!^`_9l

B

.

mR/

C

PS

R,H]U

3

S

RTH]Un:co

(6)

Basic beam theory shows that the coordinates of any point on the beam is given by Eq (7) between A and B, and by Eq (8)
between B and C

Mp/

grqs3IQ4R8tQ4uj:

X

o

T[grq

C

3IvOu,Q4u8wvxR,Q4Ry:

X

B

z|{

(7)

Mp/

grqs4Q}R

X

o

8gq

C

vxR~Q4R

X

B

T[grq

C

Q}uvOu

B

X

8gqs}Q4u~vxu

o

z|{

(8)

The combination of Eq (7) and Eq (8) applied at point B can be used to eliminate Fb. This result applied at point C gives
an expression of Fc which depends only of Xb and Yb (Eq (9)).

Q}R'/h8

C

z|{€

C

S

R`vxu

o

TvOR

o

S

uY8wvOR

B

S

uvOuj‚

vOR

B

vxu

B

€

8

C

vxR~vOuTvOR

B

TvOu

B

‚

(9)

The derivative of Eq (9) with respect to Yc gives the stiffness of the beam seen at point C (Eq (10)). The good news is
that this stiffness does not depend on Yb.

mR'/08Yƒ

vxu,z|{

vOR

B

€„8

C

vOR`vxu}TvOR

B

T…vxu

B

‚

(10)

Combining the required stiffness in C (Eq (6)) and the actual beam stiffness (Eq (10)) gives Xb (Eq (11))

vOu"/

PS

RHVU"vOR

o

8z|{93

S

R}T…HVUn:

o

T‡†

8'zG{L3

S

RTH]Un:

o'ˆ

C

PS

R~HVU'vxR

o

8z|{93

S

R4T…HVUW:

oy‰

PLS

R~HVUŠvOR

B

(11)

Combining the required force in C (Eq (5)) and the actual beam load (Eq (9)) gives Yb (Eq (12))

S

u"/

S

R

ˆ

ƒ4vOuz|{93

S

RTH]UW:

B

T

PLS

RvxR

B

3‹vOu8wvxRŒ:

B

‰

vOu

B

C

z|{vOR

B

3

S

RTH]Un:

B

3c8"vxRT}vOuŒ:

(12)

Eq (11) and Eq (12) give the shape of the profile as a parametric function of Yc. Fig. 3 shows a typical profile computed
for

X

N)/ŽZA\A\^`_bac5A7)/

C

\A^`_9aedKfb/gjiAk!^`_

l

B

As expected, the left part of the profile is not defined, because it’s

impossible to have a null stiffness. There will be no contact point for small bends, and the stiffness will be constant until
the beam touches the support profile.

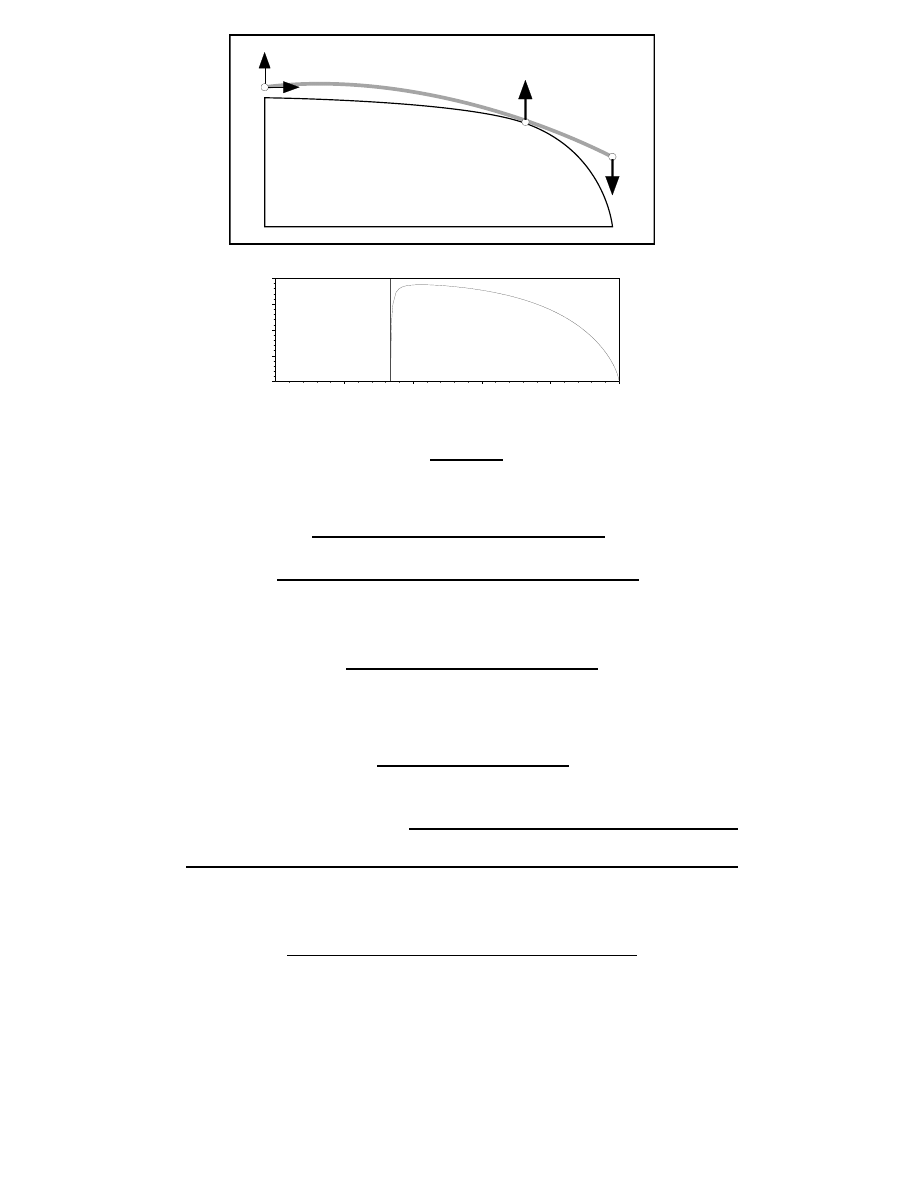
V-groove

Flexible
Beam

Figure 4. Simple structure providing a non-linear restoring force.

Flexible beams

(above the V-grooves)

Movable

electrode

Figure 5. More realistic mask for the flexible beams and the electrode.

2.1.3. Physical implementation

The idea of providing a non-linear restoring force to linearize an electrostatic actuator is not new, but the physical imple-
mentation previously proposed made this idea difficult to apply. We propose a new solution using a V-groove structure,
easily manufactured with common anysotropic etching (KOH or TMAH). The basic idea is shown in Fig. 4: A cantilever
beam with a surf shape is located just above a V-groove etched in the substrate. This structure is functionally equivalent
to the Fig. 4, but the inclined sidewall of the V-groove structure transfers the need for a very accurate profile from the
vertical plan (where profile control is difficult) to the horizontal plan (where geometry is easily controlled by the mask
layout). But several modifications must be done on this over-simplified design to make it more realistic:

-

Some holes must be made in the top layer so that the V-groove can be etched through the top flexible layer.

-

To use the analytic equations Eq (11) and Eq (12), the moment of inertia of the flexible beam must be constant along
its length. The beam geometry must therefore be modified so that its equivalent section is constant, and transversal
stiffener may be added to prevent parasitic deformations.

-

The equations Eq (11) and Eq (12) are valid for a true vertical profile. To get the equivalent shape using the V-
groove trick, this profile must be projected on the V-groove sidewall. In the case of common silicon V-groove, the
Y-axis of Fig. 3 must be divided by tan(54deg).

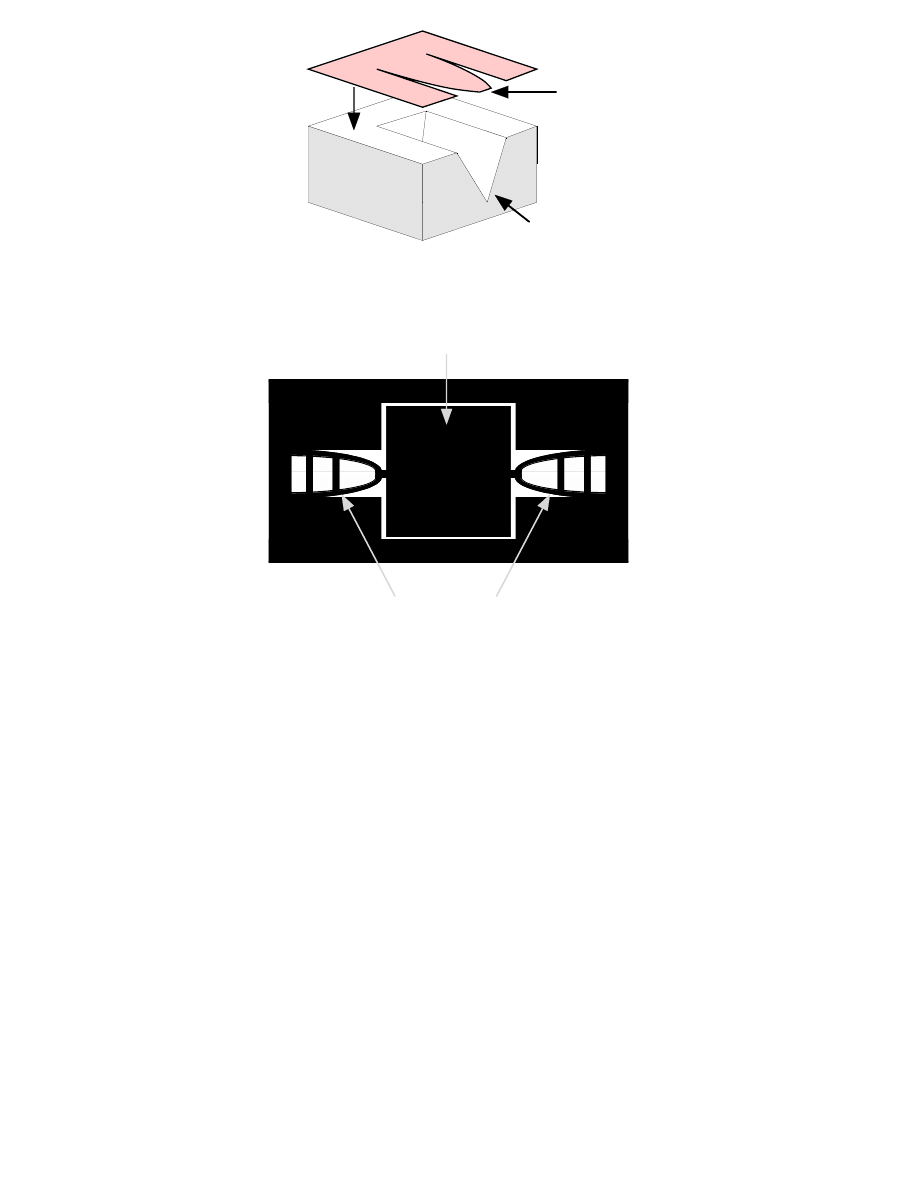
Botom

electrode

Movable electrode

Planar inductor

V

R,L

C

Figure 6. Simple L-C actuator.

2.2. Using a L-C network

The force provided by a classical parallel plate actuator is low, but it increases with the square of the applied voltage.
Unfortunately, the electronic industry is always trying to decrease the voltage used in integrated circuits to increase the
speed and reduce the power consumption. Even if some manufacturer offer special "high-voltage" process, it is difficult
to go beyond 100V without an external discrete amplifier. We present here a novel idea to increase the voltage applied on
the actuator without any high voltage active amplifier.

2.2.1. Basic idea

If we add an inductor in series with the electrostatic actuator (which mainly behaves like a capacitor), we obtain an L-C
network. Such a network exhibits a well-known resonant frequency, where the voltage across the capacitor can be much
larger than the power supply voltage. The electrostatic force due to this high-frequency source is proportional to the rms
value of this voltage. The idea is simple, but several problems exists:

-

As the capacitor value of a typical electrostatic actuator is very small (a few pF max), the resonant frequency of
the L-C network may be very high and therefore difficult to control with simple electronic circuitry. An inductance
value as high as possible is required to keep the resonant frequency as low as possible.

-

One inductor is needed for each actuator. The inductors and the actuators should therefore be co-fabricated on the
same substrate, using the same kind of process. Unfortunately, only planar spiral inductor are easy to fabricate on
silicon, and the value of their inductance is limited at high frequency (mainly due to mirror currents flowing in the
substrate)

But a solution exists in the particular case of parallel plate actuator: If we put the inductor on top of the movable electrode,
we obtain two advantages:

-

One planar inductor can be made for each actuator without losing any surface on the wafer.

-

As there is no substrate under the movable electrode (or at least it is at a distance equal to the gap from the inductor),
the mirror currents are reduced, and the inductor can be used at higher frequency without loosing too much of its
inductance. The top electrode can be segmented (with some radial cuts around the center contact point) to reduce
the mirror currents into it.

Fig. 6 show an electrostatic actuator together with its inductor. We will call this actuator an ”L-C actuator”. (To improve
clarity, the device providing the restoring force is not shown, the inductor is made of only a few turns, and the radial cuts
in the top electrode are not made)

Vs

12

10

8

6

4

2

0

g

1e-05

8e-06

6e-06

4e-06

2e-06

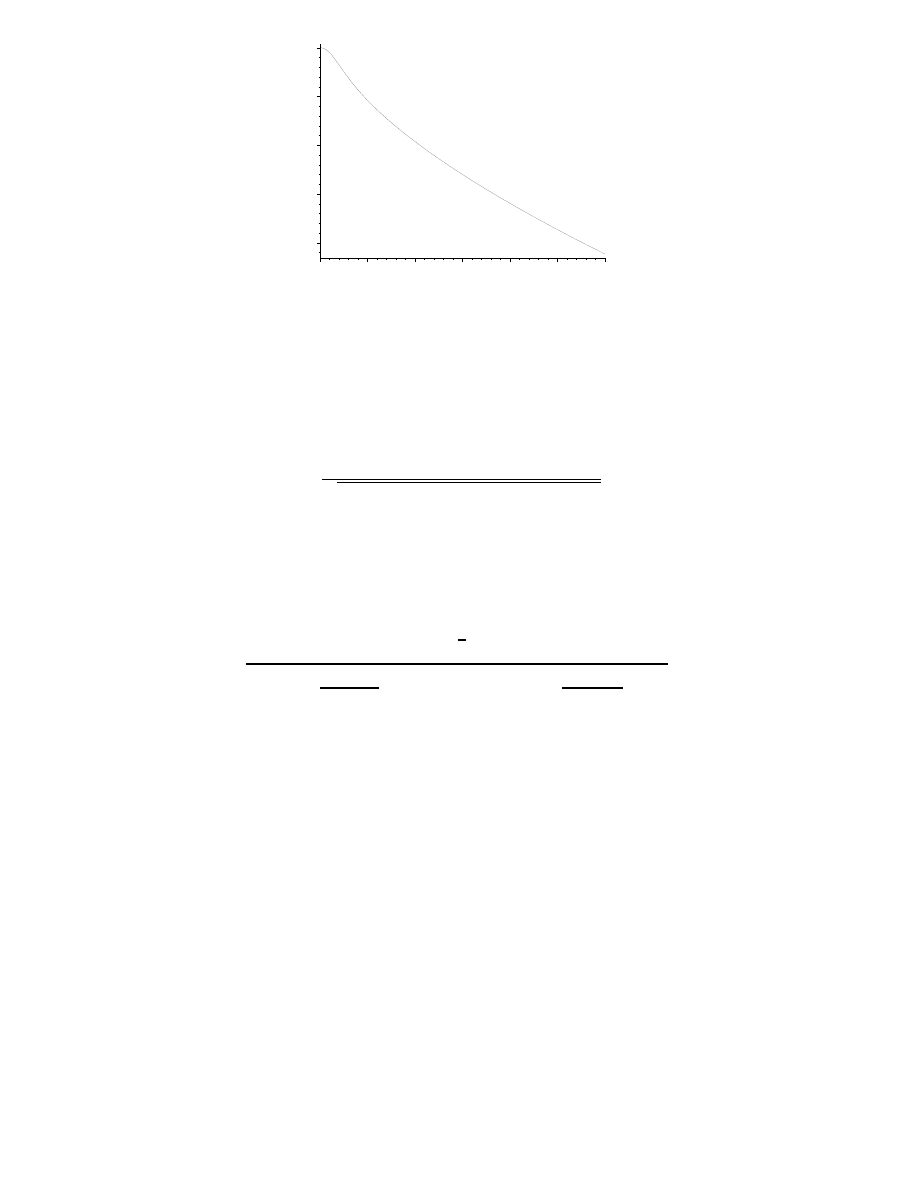
Figure 7. Equilibrium position versus supply voltage amplitude.

2.2.2. Automatic feedback

The L-C actuator has another exiting property: The electrostatic force is large because the actuator is powered at its
resonant frequency. But as the top electrodes moves down, the capacitor value increases (because the gap decreases).
The natural resonant frequency is then changed, and the power supply is no more tuned to the L-C network. As a result,
the amplitude of the voltage across the electrodes decreases when the top electrodes moves down. We can hope that this
feedback effect will tend to enlarge the stable travel range of the actuator beyond the typical value of 1/3 of the nominal
gap. The mathematical expression of the voltage across the electrodes when the gap can change is:

N/

‘~’

5

“

”

B

<

B

@

B–•"B

T…—

BŒ•˜

<

B

@

B

8

C

—

•"B

<

@

5ŠTw5

B

(13)

Where Vc is the amplitude of the voltage across the electrode, Vs and w are respectively the amplitude and frequency of
the supply voltage, R and L are respectively the (parasitic) resistance and inductance of the inductor, S is the area of the
electrodes, e is the permittivity and g is the gap between the two electrodes. If we assume a linear restoring force Fr as in
Eq (14), the equilibrium gap g for a given input voltage Vs is given by Eq (16) (at the nominal resonant frequency)

;=DK/[™=3‹HVU8L5%:

(14)

;=D/

C



(15)

xš'/h›

8

C

<

@

™)œ`8

HVU

B

”

B

<

@

—

8wHVU

o

THVU

B

5O8wHVU5

B

T

5

”

B

<

@

HVU

—

Tt5

oŒ

q<

@

(16)

Where g0 is the nominal gap and k the stiffness constant of the restoring spring. We can use a numerical example with
S=1mm*1mm, L=1uH, R=200Ohm, g0=10um and k=1 (these values are consistent with a spiral inductor made with 40
turn of 2um thick aluminum). With these values, Eq (16) gives Fig. 7.

The Fig. 7 shows that we can theoretically obtain full gap stable positioning, with a quasi-linear response (at least

compared to the response of classical actuators). Depending on the parasitic resistance of the inductor, a force at least 4
to 10 times greater can be achieved. But several issues are not taken into account by this simple model, and still have to
be studied:

-

When the gap is close to zero, even a small error in the planarity of one of the electrode will concentrate the force
around it, and the model is no longer valid. Some margins must be taken, and full gap positioning is actually not
possible.

-

To keep a high inductance value at high frequency, the moveable electrode must be segmented. But this is possible
without reducing the electrode stiffness if a thick dielectric layer is inserted just under the spiral inductor.

-

When the top electrode moves closer to the bottom one, the spiral inductor is also closer to the substrate. Mirror
currents flowing in the substrate will increase, and this will decrease the actual inductance value. This will add
some positive feedback in the system, and will probably reduce the stable positioning range. But this effect can be
suppressed if the substrate is etched (from the back side of the wafer) under the bottom electrode (in this case, the
bottom electrode must also be segmented).

3. CONCLUSION

Two new ideas have been shown to improve the behavior of parallel plate electrostatic actuators. One is using a new
structure to provide a non-linear restoring force. The other adds a planar spiral inductor to increase the voltage across the
electrodes and to provide a negative feedback on this voltage when the gap is reduced. This feedback suppress the pull-in
effect, and increases the stable positioning range. But the mathematical models used to validate these ideas are probably
over-simplified. More complete simulations and the some prototypes are still needed.

REFERENCES

David M.Burns,”Nonlinear flexures for stable deflection of aelectrostatically actuated micromirror” in SPIE Vol. 3226,

p125-136.

Renato P.Ribas, ”Micromachined Microwave Planar Spiral Inductors and Transformers” in IEEE transaction on Mi-

crowave Theory and Techniques, Vol 48, no8, August 2000.

Sunderarajan,”Simple Acurate Expressions for Planar Spiral Inductance” in IEEE Journal Of Solid-State Circuits, Vol

34, No. 10, October 1999.

Suresh Kumar, ”Electrostatically levitated microactuators” in J. Micromech and Microeng. 2 (1992) p96-103.