TECHNICAL NOTE

Exergetic ef

ficiency of high-temperature-lift chemical

heat pump (CHP) based on CaO/CO

2

and CaO/H

2

O

working pairs

Mehdi Arjmand

1,2,

*

,

†

, Longcheng Liu

1

and Ivars Neretnieks

1

1

Division of Chemical Engineering, Department of Chemical Engineering and Technology, Royal Institute of Technology (KTH),

Stockholm SE-100 44, Sweden

2

Division of Environmental Inorganic Chemistry, Department of Chemical and Biological Engineering, Chalmers University of

Technology, Göteborg SE-412 96, Sweden

SUMMARY

The use of reversible chemical reactions in recuperation of heat has gained signi

ficant interest due to higher magnitude of

reaction heat compared to that of the latent or sensible heat. To implement chemical reactions for upgrading heat, a
chemical heat pump (CHP) may be used. A CHP uses a reversible chemical reaction where the forward and the reverse
reactions take place at two different temperatures, thus allowing heat to be upgraded or degraded depending on the mode
of operation. In this work, an exergetic ef

ficiency model for a CHP operating in the temperature-level amplification mode

has been developed. The

first law and the exergetic efficiencies are compared for two working pairs, namely, CaO/CO

2

and

CaO/H

2

O for high-temperature high-lift CHPs. The exergetic ef

ficiency increases for both working pairs with increase in

task, T

H

, decrease in heat source, T

M

, and increase in condenser, T

L

, temperatures. It is also observed that the difference in

reaction enthalpies and speci

fic heats of the involving reactants affects the extent of increase or decrease in the exergetic

ef

ficiency of the CHP operating for temperature-level amplification. Copyright © 2012 John Wiley & Sons, Ltd.

KEY WORDS

chemical heat pump (CHP);

first law efficiency; second law (exergetic) efficiency; temperature amplification; heat transformer; CaO/

CO

2

; CaO/H

2

O

Correspondence

*Mehdi Arjmand, Division of Chemical Engineering, Department of Chemical Engineering and Technology, Royal Institute of Technology

(KTH), Stockholm SE-100 44, Sweden.

†

E-mail: [e-mail]

Received 25 September 2011; Revised 18 February 2012; Accepted 1 March 2012

1. INTRODUCTION

Depending on the type of the process, waste heat may be
released at any temperature in the range of chilled cooling
water to high-temperature gases from an industrial furnace
[1]. High-temperature waste heat provides a higher recov-
ery rate and thus can be often effectively recovered using
conventional and physical recovery solutions (e.g. using
a series of heat exchangers). However, most waste heat
streams in the industry have a low temperature (

<400 K)

and therefore are called low-grade waste heat [2]. In this
case, physical recovery may not be effective in retrieving
the lost energy.

A low-temperature waste heat may be upgraded using a

vapor compression heat pump, which requires electricity,
and/or sorption heat pumps, which uses the heat of (de)
sorption of a medium [3

–10]. In recent years, however,

engaging reversible chemical reactions for recuperation
of heat has gained signi

ficant interest because of the higher

magnitude of reaction heat compared with that of the latent
or sensible heat as retrieved in physical recovery techni-
ques or vapor compression and sorption heat pumps [11].
To use chemical reactions for upgrading low-grade waste
heat, a chemical heat pump (CHP) may be used which
offers a wider range of operating temperature and versatil-
ity in comparison with the conventional vapor compression
or (de)sorption heat pump. In practice, temperatures as low
as 230 K in refrigeration or freezing systems, and up to 870
K in heat generation systems can be supplied using CHPs
[12

–20].

In theory, a CHP operates in either of three different

modes: (a) heat generation, (b) refrigeration, or (c)
temperature-level ampli

fication; also known as chemical

heat transformer (CHT) [21

–23]. First and/or second

INTERNATIONAL JOURNAL OF ENERGY RESEARCH

Int. J. Energy Res. (2012)

Published online in Wiley Online Library (wileyonlinelibrary.com). DOI: 10.1002/er.2918

Copyright © 2012 John Wiley & Sons, Ltd.

law (exergetic) ef

ficiency analyses of a range of CHPs

with different working pairs (working

fluid or medium)

operating mainly for heat generation and refrigeration,
that is, modes a and b, have been carried out
[12,20,24

–31]. The kinetic aspects of some of the work-

ing pairs have also been investigated [14,17,20,32

–34].

However, the work on exergetic ef

ficiency analysis of

a CHP operating in the temperature-level ampli

fication

mode, that is, mode c, is still limited [27,35].

The temperature-level ampli

fication mode may be used

to upgrade low-grade waste heat. It also shapes the basis
for high-temperature lift CHPs particularly for power gen-
eration or other industrial applications [36], an area where
progress is to be seen [23,34,37

–40]. It is well known that

the conventional heat balance for evaluation of losses and
system ef

ficiency does not fully represent the effectiveness

of a system. On the other hand, an estimation of available
energy (exergy) is advantageous for a closer measurement
of losses and thus effective conservation of energy during
design and operation of such systems. In addition, the eval-
uation of the exergetic ef

ficiency for this mode of operation

offers a criterion to discern heat transformers that are ther-
modynamically effective from those that are not. More-
over, exergetic ef

ficiency can be used to identify systems

that have potential for improvements. A signi

ficant differ-

ence between the exergetic and the practical ef

ficiency sug-

gests that there may be room for possible performance
enhancements [41].

Thus, an exergetic ef

ficiency model for a CHP operating

in the temperature-level ampli

fication mode has been devel-

oped. The model is then used to compare the ef

ficiencies of

two working pairs, namely, CaO/CO

2

and CaO/H

2

O for

high-temperature-lift CHPs.

2. CHEMICAL HEAT PUMP

In contrast to the heat engine de

fined by Carnot where

work is delivered between a high-temperature heat source
and a low-temperature heat sink, the CHP uses three (or
four) temperature levels of high, medium, and low to con-
sume or produce thermochemical energy. Thus, for a CHP,
a closed cycle in which the forward reaction is endothermic
and the reverse reaction is exothermic may be considered.
The endothermic reaction occurs at a lower temperature,
whereas the exothermic reaction is carried out at a higher
temperature. As a result, a low-temperature heat may be
absorbed by the endothermic reaction and released at a
higher temperature by the exothermic reaction.

In principal, the general reaction in a CHP may be

assumed as follows:

A

þ B↔C

(1)

It should be noted that the reaction is reversible and that

the forward and the reverse reactions are assumed to take
place at two different temperatures, thus allowing heat to
be upgraded or degraded depending on the mode of

operation (i.e. refrigeration, heat generation, or tempera-
ture-level ampli

fication) [19]. By absorbing heat through

the reverse endothermic reaction in Equation (1), C is
decomposed to A (a nonvolatile compound) and B (a vola-
tile compound). On the other hand, the forward reaction in
Equation (1) is exothermic, during which C is formed
again. Thus, in the simplest form, a CHP comprises a
decomposition reactor, a condenser, an evaporator, and a
synthesis reactor [18]. The evaporator is the source of the
volatile compound B for later formation of C in the synthe-
sis reactor. B is eventually condensed in the condenser
after C is decomposed into A and B in the decomposition
reactor. Because the chemical compound C and the con-
densate are pure phases, the pressure of the volatile com-
pound B in the reactors and the evaporator depend only
on the temperature. Thus, the operation is monovariant,
that is by specifying the pressure, the temperature will also
be determined [42,43].

The modes of heat generation or refrigeration have sim-

ilar fundamentals in using the low temperature heat from
the environment or surrounding to achieve the output
effect, that is, producing heat or cold, respectively. How-
ever, they differ in the de

finition of the user as demanding

cold (refrigerating) or hot (heat generating) streams. Such
CHPs have been thoroughly studied, and the evaluation
of both thermal ef

ficiencies has been reported in several

publications [12,20,24,27

–31].

The temperature-level ampli

fication mode on the

other hand operates on a somewhat different basis that
uses an intermediate-level heat source to generate a
high-temperature-level heat [9,43]. In order for this to
occur, the forward and the reverse directions of the
reversible reaction (Equation (1)) must both take place
at temperatures higher than that of the environment. To
further distinguish between the heat ampli

fication and

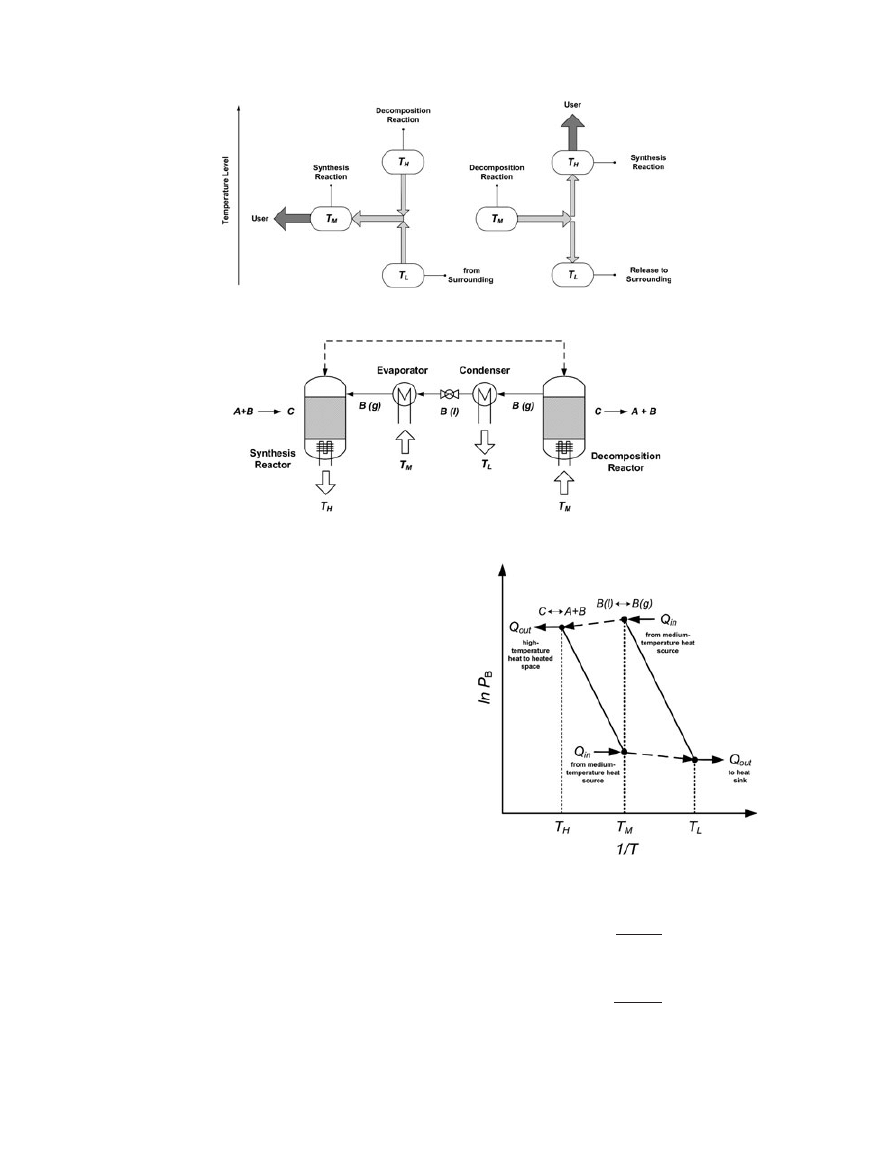
the heat generation and cooling modes, consider T

M

and

T

L

representing different media as outlined in Figure 1.

In the case of a CHP for heat generation, T

M

represents

the temperature of the synthesis reaction that releases
the useful heat to the user, which is generated with the
help of heat from the endothermic reaction at the higher
temperature, T

H

, (e.g. using surplus heat) and heat from

the environment at the lower temperature, T

L

. By

contrast, in the case of a CHP operating for temperature
ampli

fication, T

L

represents the condenser, which releases

nonuseful heat to the environment (from part of the sup-
plied heat) while offering the upgraded heat at the higher
temperature, T

H

, with the help of the medium-temperature

heat source, T

M

, (e.g. from surplus heat).

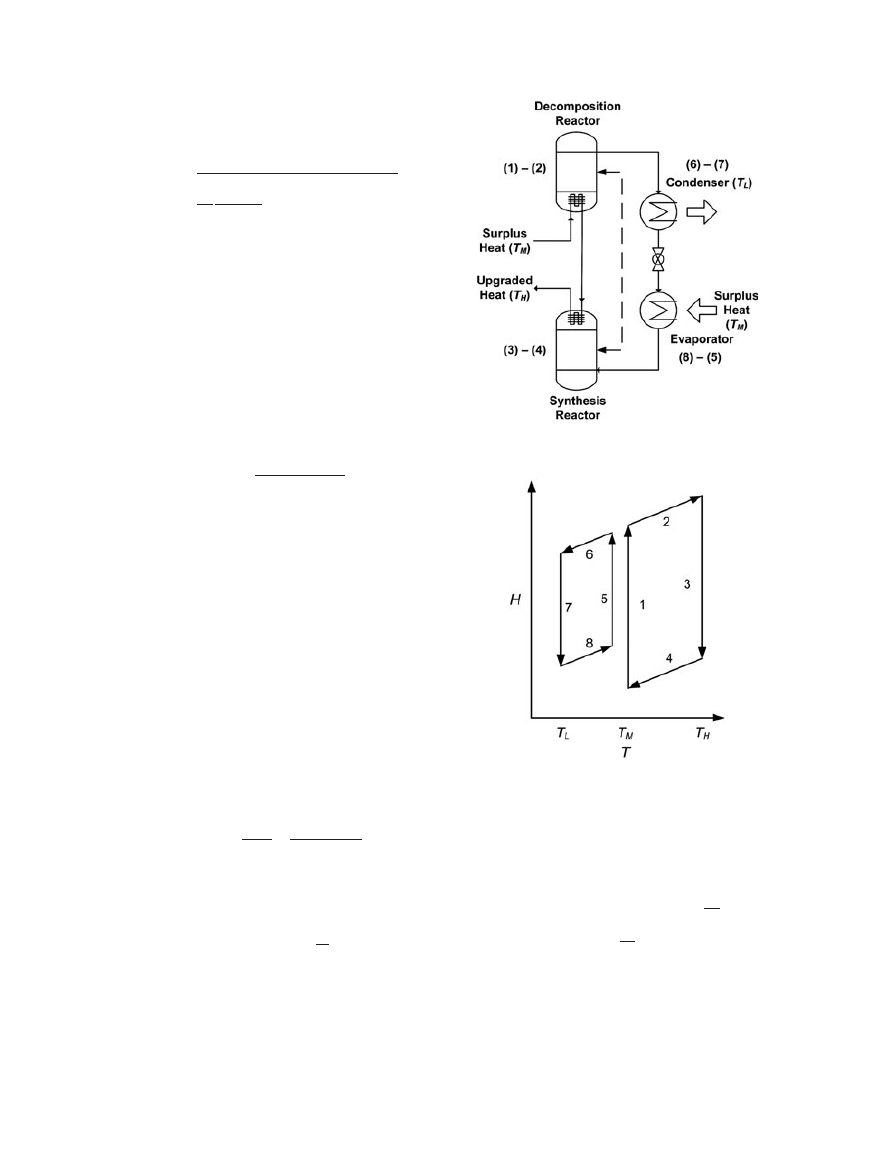
Figure 2 shows the schematic of a CHP operating in the

temperature-level ampli

fication mode. Here, an intermediate-

level heat (e.g. waste heat) at T

M

is supplied to both the

decomposition reactor to breakup C into its constituents and
later to the evaporator to vaporize the working

fluid, B. The

vapor B generated from the decomposition reactor condenses
in the condenser during the charging phase. During the dis-
charging phase, the working

fluid B is admitted to the evap-

orator, where it is vaporized and allowed to react with A in

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.
DOI: 10.1002/er

the synthesis reactor. Thus, compound C is formed at a
higher temperature-level, T

H

, and with a higher quality than

that of the original source supplied to the system. The con-
denser is used to partly remove the low-temperature heat at
ambient, T

L

, and to complete the cycle. The result is that a

medium-temperature heat, T

M

(e.g. from waste heat), is

absorbed by the system through the reverse endothermic re-
action and evaporation of the working

fluid and is upgraded

to a high-temperature heat, T

H

, by the exothermic heat of

the forward reaction. Figure 3 shows the energy

flows of a

CHP operating in the temperature-level ampli

fication mode.

It can be noticed that the intermediate temperature-level heat,
T

M

, is required during both charging and discharging

modes, that is, decomposition of C and evaporation of
B. If this source is not always available, two CHPs may
be integrated [43].

3. THERMAL EFFICIENCIES

3.1. First law ef

ficiency

The maximum ef

ficiency of any heat engine, heat pump, or

refrigerator can be derived for cyclic reversible processes
using the well-known Carnot ef

ficiency. To derive the

Carnot ef

ficiency of a CHP, two cycles consisting of one heat

pump (operating in the higher temperature interval) and one
heat engine (operating in the lower temperature interval) may
be considered [9,42]. The maximum (or Carnot) ef

ficiency of

the heat engine may then be expressed as

max

HE

¼

T

M

T

L

T

M

(2)

and the maximum ef

ficiency of the heat pump may be

written as

max
HP

¼

T

H

T

H

T

M

(3)

The overall maximum ef

ficiency of the CHP is defined as

the ratio of the heat obtained to the heat supplied. Thus, the

Figure 1. Operation principle of a CHP operating in heat generation mode (left) and temperature-level ampli

fication mode (right).

Figure 2. Schematic of a CHP operating in the temperature-level ampli

fication mode.

Figure 3. Energy

flow of a CHP operating in the temperature-

level ampli

fication mode.

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.

DOI: 10.1002/er

maximum ef

ficiency of a CHP operating as a temperature

ampli

fier is as follows

max

CHP

¼

Utilized heat at higher temperature

Supplied heat

¼

T

H

T

M

T

M

T

L

T

H

T

L

(4)

Equation (4) shows that the ideal performance of a CHP

depends only on the cycle temperature boundaries [19]. An
investigation of different working pairs and optimum operat-
ing temperatures has also been reported. An overview of the
working pairs used for CHPs can be found in the reviews by
Wongsuwan et al. [18] and Aristov et al. [19].

However, the actual ef

ficiency of a CHP is not depen-

dent on the temperature levels but on the enthalpy changes
in the high and low temperature cycle. Thus, assuming
negligible variation in reaction enthalpy with temperature
and neglecting sensible heat, the actual ef

ficiency of a CHP

in operation for temperature-level ampli

fication is [43]

CHP

;I

¼

ΔH

H

H

ΔH

L

M

þ Δ

H

H

M

(5)

In Equation (5), the superscripts refer to either the high- or

the low-temperature reaction (or reactor) and the subscripts
indicate the temperature level. It should be mentioned that
the temperatures of the CHP cycle are not independent of
each other, and with the determination of one, the other
two temperatures are also set. Consequently, the actual ef

fi-

ciency of the CHP (Equation (5)) can theoretically reach
the Carnot ef

ficiency (Equation (4)), irrespective of the

chemical nature of the working pair [19].

3.2. Second law (exergetic) ef

ficiency

In contrast to the

first law, the second law analysis uses the

concept of available energy (exergy) and irreversibility
[44]. Exergy analysis provides the means for evaluation
of the degree of thermodynamic perfection of a process.
On the basis of the exergy function, the ef

ficiency of a heat

pump can be expressed as [45]

CHP

;II

¼

E

X

out

E

X

avail

:

¼

E

X

avail

:

E

X

loss

E

X

avail

:

(6)

For a heat pump receiving heat at T

i

, the available

exergy is given as per the following equation [45]:

E

X

avail

:

¼ ΔH

i

1

T

0

T

i

(7)

where T

0

represents the reference (or ambient) temperature

in Equation (7).

Figure 4 shows the scheme of the processes con

figura-

tion for a CHP operating in the temperature ampli

fication

mode, and Figure 5 represents the corresponding cycle

path of such CHP. It can be observed in Figure 5 that the
available exergy is provided as heat input during processes
1, 2, 5 and 8; thus,

E

X

avail

:

¼ ΔH

1

þ ΔH

5

þ ΔH

8

ð

Þ 1

T

L

T

M

þ ΔH

2

ð

Þ 1

T

L

T

H

(8)

To account for the exergy losses, the irreversibilities of

the process should also be determined. For this, irrevers-
ibility can be expressed in terms of entropy and enthalpy
change with respect to the reference temperature (T

0

) for

each cycle path as [46]

Figure 4. Process con

figuration of a CHP operating in the

temperature-level ampli

fication mode.

Figure 5. Cycle path of a CHP operating in the temperature-

level ampli

fication mode.

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.
DOI: 10.1002/er

I

¼ T

0

ΔS

i

ΔH

i

T

sink

=source

(9)

In Equation (9), i refers to the ith cycle path and T

sink/source

is the temperature at the end of the cycle path of the process,
which can either be a sink or a source depending on the cycle
path.

Considering Equation (1) for the CHP, the cycle paths

in the processes involved can be described as follows:

(1) Dissociation of compound C at T

M

,

ΔH

H

M

is supplied

ΔH

1

¼ ΔH

H

M

(10)

I

1

¼ T

0

ΔS

1

ΔH

1

T

M

(11)

(2) Temperature of the constituting compounds A and B

increases to T

H

, absorbing sensible heat

ΔH

2

ΔH

2

¼ C

P

A

þ C

P

B

ð

Þ T

H

T

M

ð

Þ

(12)

and

I

2

¼ T

0

ΔS

2

ΔH

2

T

H

(13)

(3) Formation of compound C at T

H

,

ΔH

H

H

is released

ΔH

3

¼ ΔH

H

H

(14)

and

I

3

¼ T

0

ΔS

3

ΔH

3

T

H

(15)

(4) Temperature of compound C decreases to T

M

, releasing

sensible heat

ΔH

4

ΔH

4

¼ C

P

C

T

H

T

M

ð

Þ

(16)

and

I

4

¼ T

0

ΔS

4

ΔH

4

T

M

(17)

(5) B evaporates at T

M

, absorbing latent heat

ΔH

5

ΔH

5

¼ ΔH

L

M

(18)

and

I

5

¼ T

0

ΔS

5

ΔH

5

T

M

(19)

(6) Temperature of B decreases from T

M

to T

L

, releasing

sensible heat

ΔH

6

ΔH

6

¼ C

P

B

T

M

T

L

ð

Þ

(20)

and

I

6

¼ T

0

ΔS

6

ΔH

6

T

L

(21)

(7) B condenses at T

L

, releasing latent heat

ΔH

7

ΔH

7

¼ ΔH

L

L

(22)

and

I

7

¼ T

0

ΔS

7

ΔH

7

T

L

(23)

(8) B absorbs sensible heat from the medium at T

M

ΔH

8

¼ C

P

B

T

M

T

L

ð

Þ

(24)

and

I

8

¼ T

0

ΔS

8

ΔH

8

T

M

(25)

because for the entire process, it is known that

X

ΔH

i

¼ 0 ;

X

ΔS

i

¼ 0

(26)

the total irreversibility of the process can be written as

E

X

loss

¼

X

I

i

¼ T

L

ΔH

1

T

M

þ

ΔH

2

T

H

þ

ΔH

3

T

H

þ

ΔH

4

T

M

þ

ΔH

5

T

M

þ

ΔH

8

T

M

ΔH

6

ΔH

7

(27)

Considering that the overall enthalpy change of a cycle is

zero, that is,

ΔH

1

þ ΔH

2

¼ ΔH

3

ð

Þ þ ΔH

4

ð

Þ

(28)

using Equations (8) and (27), Equation (6) can be written as

CHP

;II

¼

ΔH

3

ð

Þ 1

T

L

T

H

þ ΔH

4

ð

Þ 1

T

L

T

M

ΔH

1

þ ΔH

5

þ ΔH

8

ð

Þ 1

T

L

T

M

þ ΔH

2

ð

Þ 1

T

L

T

H

(29)

which by substituting

ΔH

i

, the

CHP,II

is obtained as

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.

DOI: 10.1002/er

where subscript II indicates the second law (exergetic) ef

fi-

ciency of the CHP operating for temperature-level
ampli

fication.

4. CASE STUDY

In what follows, the results from the

first law efficiency

and the exergetic ef

ficiency model developed here for a

CHP operating in the temperature-level ampli

fication mode

will be presented and discussed for two working pairs,
namely, CaO/CO

2

and CaO/H

2

O. These working pairs

were selected among a series of potential sets by the crite-
rion that they offer a high-lift temperature increase (773 to
1273 K and 673 to 873 K, respectively) [26,29].

4.1. CaO/CO

2

working pair

The principle of operation using the CaO/CO

2

working

pair is similar to what is shown in Figure 2. Energy is
absorbed with the dissociation of CaCO

3

and is released

again with the reaction of dissociated products, that is,
CaO and CO

2

. If the pressure of CO

2

during the carbon-

ation process is higher than the pressure of CO

2

during

the de-carbonation process, then the former reaction can
occur at a higher temperature than the latter. Thus, to
increase the CO

2

pressure, it can either be (i) reacted with

another metal oxide and stored in the form of a metal car-
bonate (i.e. using chemical looping [47], (ii) compressed
and stored, (iii) adsorbed (e.g. using zeolites or activated
carbon), or (iv) absorbed with an appropriate solvent (e.g.
using amines) [26].

The compression of the CO

2

gas is less attractive be-

cause of additional energy penalty and the fact that it is
only a modi

fication of the conventional vapor compression

heat pump. CO

2

separation by chemical looping increases

the complexity of the process, and absorption using amine
is an existing process with associated energy cost. There-
fore, this work further investigates the process involving
the adsorption of CO

2

gas. Restuccia et al. [22] showed

that the zeolite 13X is the most suitable adsorbent for
CO

2

in this case because of the highest energy density of

this particular zeolite. Thus, the data for the zeolite 13X
are used in the following. The process description is as
follows: heat is absorbed at the intermediate temperature
level, T

M

, in the decomposition reactor thus producing

CO

2

as CaCO

3

decomposes. The produced CO

2

gas is

cooled and is removed by the zeolite at the lower tempera-
ture, T

L

. The vessel is sealed and is heat to the intermediate

temperature, T

M

. This increases the partial pressure of the

CO

2

above the zeolite as more CO

2

is desorbed. The

CO

2

gas is then sent to the synthesis reactor to form

CaCO

3

, producing a higher-temperature heat, T

H

.

The parameters and values were analyzed using

MATLAB

W

to evaluate the ef

ficiencies based on the previ-

ously mentioned formulation (summarized in Table I). It
should be noted that for the CaO/CO

2

working pair, CO

2

is assumed to be stored at an average temperature of 273
K, which is equivalent to storing energy at 773 K [26]. It
is also known that regardless of the number of moles
adsorbed, an isosteric enthalpy of

40 kJ/mol may be

assumed [48]. Given this and other data sets [25,49

–51]

as listed in Table I, the results for the

first law and exergetic

ef

ficiencies obtained using Equations (5) and (30) are

shown in Figure 6.

To observe the effect of heat source, condenser and task

temperatures on the ef

ficiencies, a range of temperatures

has to be considered. As shown in Figure 6, it can be inferred
that the exergetic ef

ficiency is dependent on the temperatures

of the heat source, T

M

, condenser, T

L

, and the task, T

H

,

whereas the

first law efficiency remains constant irrespective

of the temperatures. Calculated

first law efficiency is ap-

proximated at 0.82, which is slightly higher than the
corresponding value obtained experimentally [26] for an
upgraded temperature of 1173 K. This justi

fies the validity

of the

first law efficiency (Equation (5)).

However, the interpretation of the exergetic ef

ficiency

should not be carried out as equivalent to the

first law effi-

ciency or performance but rather only to understand the
degree of the available energy to the system for the desired
task (in this case, upgrading heat from a lower to a higher
temperature). As shown in Figure 6, a higher condenser
temperature, T

L

, offers a higher availability of energy to

CHP

;II

¼

ΔH

H

H

1

T

L

T

H

þ C

P

C

T

H

T

M

ð

Þ 1

T

L

T

M

½

ΔH

H

M

þ ΔH

L

M

þ C

P

B

T

M

T

L

ð

Þ

1

T

L

T

M

þ C

P

A

þ C

P

B

ð

Þ T

H

T

M

ð

Þ

½

1

T

L

T

H

(1)

(30)

Table I. Summary of the parameters used for the ef

ficiencies of the CaO/CO

2

and CaO/H

2

O working pairs.

Reactant

Reaction temperature (K)

Speci

fic heat (C

P

) (kJ/mol K)

Reaction

Reaction enthalpy (kJ/mol)

CaO

773

0.0522 [49]

–

–

673

0.0513 [49]

CaCO

3

1273

0.1230 [51]

CaO

(s)

+ CO

2 (g)

↔ CaCO

3(s)

178.3

CO

2

237

0.0314 [50]

CO

2 (g)

+ zeolite 13X

↔ CO

2 (ads.)

42

Ca(OH)

2

873

0.0930 [25]

CaO

(s)

+ H

2

O

(g)

↔ Ca(OH)

2(s)

104

H

2

O

288

0.0757 [50]

H

2

O

(g)

↔ H

2

O

(l)

40

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.
DOI: 10.1002/er

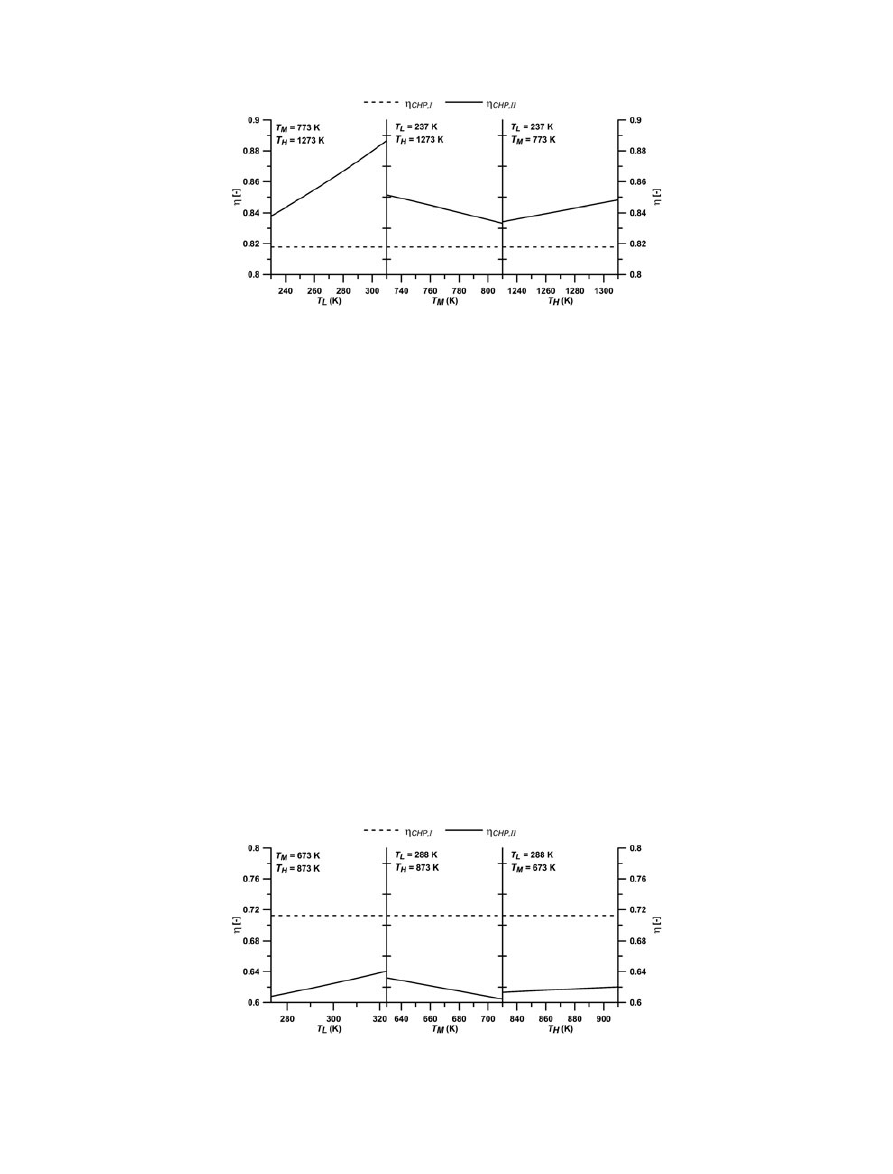
the system for a constant heat source, T

M

, and task, T

H

,

temperatures, which is expected as the condensation in
the cooler imposes that a higher degree of energy is to be
removed from the system. On the contrary, with the
increase of heat source temperature, T

M

, a lower amount

of energy will be available to the system as the exergetic
ef

ficiency is reduced. In other words, if the quality of the

heat source, T

M

, is increased while the task temperature,

T

H

, is remained constant, the system ef

ficiency will

decrease. Accordingly,

CHP,II

increases by increasing the

task temperature, T

H

, under

fixed condenser, T

L

, and heat

source, T

M

, temperatures. This can also be interpreted as

that at lower task temperature, T

H

, the system ef

ficiency

will also be lower.

4.2. CaO/H

2

O working pair

The basis of operation for the CaO/H

2

O set does not differ

from the con

figuration of the CHP operating for tempera-

ture ampli

fication as described earlier. In this case, the

difference in the partial pressure of water in the liquid
and the gaseous form is the driving force between the reac-
tors. The ef

ficiencies of the CaO/H

2

O working pair operat-

ing in the temperature-level ampli

fication mode are shown

in Figure 7. Here, the

first law efficiency obtained is also in

agreement with the corresponding experimental value [29].
The trends of dependencies on the heat source and task

temperatures are rather similar to that of the CaO/CO

2

working pair. However, the extent in increase or decrease
in the exergetic ef

ficiency of the CaO/H

2

O working pair

differs from that of the CaO/CO

2

. It is worth noting that

the speci

fic heats of CaCO

3

and Ca(OH)

2

compounds are

only slightly different (0.1230 and 0.093 kJ/mol K, respec-
tively), whereas a larger difference exists for the respective
values of CO

2

and H

2

O in addition to the endothermic re-

action enthalpies of the involving pairs (

ΔH = 104kJ/mol

for CaO/H

2

O versus

ΔH = 178.3kJ/mol for CaO/CO

2

).

5. CONCLUSIONS

Analyses of the

first law and exergetic efficiencies of a

CHP operating in the temperature-level ampli

fication mode

have been carried out. The exergetic ef

ficiency model de-

rived was used for two working pairs, namely, CaO/CO

2

and CaO/H

2

O for high-temperature high-lift CHPs. The

exergetic ef

ficiency increases for both working pairs with

increase in task, T

H

, decrease in heat source, T

M

, and

increase in condenser, T

L

, temperatures. However, the

difference in reaction enthalpies and speci

fic heats of the

involving reactants affects the extent of increase or
decrease in the exergetic ef

ficiency of the CHP operating

in the temperature-level ampli

fication mode.

Figure 6. Dependencies of

first law (

CHP,I

) and exergetic (

CHP,II

) ef

ficiency on T

H

, T

L

and T

M

for the CaO/CO

2

working pair.

Figure 7. Dependencies of

first law (

CHP,I

) and exergetic (

CHP,II

) ef

ficiency on T

H

, T

L

and T

M

for the CaO/H

2

O working pair.

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.

DOI: 10.1002/er

NOMENCLATURE

A, B, C

= reactants

HE

= heat engine

HP

= heat pump

CHP

= chemical heat pump

C

P

= speci

fic heat

E

X

= exergy

H

= enthalpy

P

= pressure

S

= entropy

T

= temperature

= ef

ficiency

Superscripts

H

= high-temperature reactor

L

= low-temperature reactor

Subscripts

avail.

= available quantity

loss

= lost quantity

H

= high-temperature level

M

= medium-temperature level

L

= low-temperature level

g

= gas state

l

= liquid state

s

= solid state

0

= reference state

I

=

first law

II

= second law (exergetic)

ACKNOWLEDGEMENT

The authors express their gratitude to Vattenfall AB for the

financial support of this work.

REFERENCES

1. Sternlicht B. Waste energy recovery: An excellent

investment opportunity. Energy Conversion and
Management 1982;

22:361–373.

2. Spoelstra S, Haije WG, Dijkstra JW. Techno-

economic feasibility of high-temperature high-lift chem-
ical heat pumps for upgrading industrial waste heat.
Applied Thermal Engineering 2002;

22:1619–1630.

3. Cheng C-S, Shih Y-S. Exergy and energy analyses of

absorption heat pumps. International Journal of
Energy Research 1988;

12:189–203.

4. Demir H, Mobedi M, Ülkü S. A review on adsorption

heat pump: Problems and solutions. Renewable and
Sustainable Energy Reviews 2008;

12:2381–2403.

5. Tahat MA, Babushaq RF, O

’Callaghan PW, Probert

SD. Integrated thermochemical heat-pump / energy-
store. International Journal of Energy Research
1995;

19:603–613.

6. Altini

şik K, Veziroglu TN. Metal hydride heat pumps.

International

Journal

of

Energy

Research

1991;

15:549–560.

7. Dawoud B, Amer EH, Gross DM. Experimental

investigation

of

an

adsorptive

thermal

energy

storage. International Journal of Energy Research
2007;

31:135–147.

8. Morawetz E. Sorption-compression heat pumps. Inter-

national Journal of Energy Research 1989;

13:83–102.

9. Bjurström H, Raldow W. The absorption process for

heating, cooling and energy storage

—an historical

survey. International Journal of Energy Research
1981;

5:43–59.

10. Ahachad M, Charia M, Bernatchou A. Solar absorp-

tion heat transformer applications to absorption refrig-
erating machines. International Journal of Energy
Research 1993;

17:719–726.

11. Kato Y, Sasaki Y, Yoshizawa Y. Magnesium oxide/

water chemical heat pump to enhance energy utilization
of a cogeneration system. Energy 2005;

30:2144–2155.

12. Saito Y, Kameyama H, Yoshida K. Catalyst-assisted

chemical heat pump with reaction couple of acetone
hydrogenation/2

–propanol dehydrogenation for upgrad-

ing low-level thermal energy: Proposal and evaluation.
International Journal of Energy Research 1987;
11:549–558.

13. Andersson J, Azoulay M, de Pablo J. Chemical heat

pumping

—A rapid experimental procedure for investi-

gating the suitability of salt hydrates under dynamic
conditions. International Journal of Energy Research
1988;

12:137–145.

14. Matsumura Y, Yoshida K. Heat pump characteristics

of sodium carbonate dehydration/hydration system.
International

Journal

of

Energy

Research

1995;

19:253–261.

15. Cacciola G, Anikeev V, Recupero V, Kirillov V,

Parmon V. Chemical heat pump using heat of revers-
ible catalytic reactions. International Journal of
Energy Research 1987;

11:519–529.

16. Wentworth WE, Chen E. Simple thermal decomposi-

tion reactions for storage of solar thermal energy.
Solar Energy 1976;

18:205–214.

17. Mooksuwan W, Kumar S. Study on 2-propanol/

acetone/hydrogen chemical heat pump: endothermic
dehydrogenation of 2-propanol. International Journal
of Energy Research 2000;

24:1109–1122.

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.
DOI: 10.1002/er

18. Wongsuwan W, Kumar S, Neveu P, Meunier F. A

review of chemical heat pump technology and
applications. Applied Thermal Engineering 2001;
21:1489–1519.

19. Aristov Y. Chemical and adsorption heat pumps:

Cycle ef

ficiency and boundary temperatures. Theoreti-

cal Foundations of Chemical Engineering 2008;
42:873–881.

20. Kim TG, Yeo YK, Song HK. Chemical heat pump

based on dehydrogenation and hydrogenation of i-
propanol and acetone. International Journal of Energy
Research 1992;

16:897–916.

21. Gandia LM, Montes M. Effect of the design variables

on the energy performance and size parameters of a
heat transformer based on the system acetone/H

2

/2-

propanol. International Journal of Energy Research
1992;

16:851–864.

22. Restuccia G, Cacciola G, Quagliata R. Identi

fication of

zeolites for heat transformer, chemical heat pump and
cooling systems. International Journal of Energy
Research 1988;

12:101–111.

23. Aristov YI, Parmon VN, Cacciola G, Giordano N.

High-temperature chemical heat pump based on
reversible

catalytic

reactions

of

cyclohexane-

dehydrogenation/benzene-hydrogenation: Comparison
of the potentialities of different

flow diagrams.

International Journal of Energy Research 1993;
17:293–303.

24. Fujii I, Tsuchiya K, Higano M, Yamada J. Studies of

an energy storage system by use of the reversible
chemical reaction: CaO + H

2

O

⇌ Ca(OH)

2

. Solar

Energy 1985;

34:367–377.

25. Azpiazu MN, Morquillas JM, Vazquez A. Heat

recovery from a thermal energy storage based on the
Ca(OH)

2

/CaO cycle. Applied Thermal Engineering

2003;

23:733–741.

26. Kyaw K, Shibata T, Watanabe F, Matsuda H,

Hasatani M. Applicability of zeolite for CO

2

storage

in a CaO

–CO

2

high temperature energy storage

system. Energy Conversion and Management 1997;
38:1025–1033.

27. Chen KS, Hwang WC. On the chemical heat pump

system and its second-law ef

ficiency. Energy Conver-

sion and Management 1988;

28:123–127.

28. Chung Y, Kim B-J, Yeo Y-K, Song HK. Optimal

design of a chemical heat pump using the 2-
propanol/acetone/hydrogen system. Energy 1997;
22:525–536.

29. Ogura H, Yamamoto T, Kage H. Ef

ficiencies of CaO/

H

2

O/Ca(OH)

2

chemical heat pump for heat storing and

heating/cooling. Energy 2003;

28:1479–1493.

30. Meng N, Shinoda S, Saito Y. Improvements on

thermal ef

ficiency of chemical heat pump involving

the reaction couple of 2-propanol dehydrogenation
and acetone hydrogenation. International Journal of
Hydrogen Energy 1997;

22:361–367.

31. Fujimoto S, Bilgen E, Ogura H. Dynamic simulation

of CaO/Ca(OH)

2

chemical heat pump systems.

Exergy, An International Journal 2002;

2:6–14.

32. Kato Y, Honda T, Kanzawa A. Kinetic measurement

on the isobutene/water/tert-butanol chemical heat
pump; dehydration of tert-butanol. International
Journal of Energy Research 1996;

20:681–692.

33. Chadda D, Ford JD, Fahim MA. Chemical Energy

Storage by the Reaction Cycle CuO/Cu

2

O. Inter-

national

Journal

of

Energy

Research 1989;

13:63–73.

34. Kato Y, Harada N, Yoshizawa Y. Kinetic feasibility of

a chemical heat pump for heat utilization of high-
temperature processes. Applied Thermal Engineering
1999;

19:239–254.

35. Izquierdo M, Aroca S. Lithium bromide high-temperature

absorption heat pump: Coef

ficient of performance and

exergetic ef

ficiency. International Journal of Energy

Research 1990;

14:281–291.

36. Arjmand M. Thermochemical Recovery of Heat:

Conceptual Integration of Chemically Recuperative

Cycle and Chemcial Heat Pump. LAP LAMBERT
Academic Publishing GmbH &Co. KG: Saarbrücken,
2011; 1

–84.

37. Olszewski M, Zaltash A. High-Lift Chemical Heat

Pump Technologies for Industrial Processes. ASME
International Congress and Exposition, 1995. US
Department of Energy. Available from: www.osti.
gov/bridge/servlets/purl/100092-7tELqq/webviewable/,
Accessed 2010-10-26.

38. Kato Y, O-shima T, Yoshizawa Y. Thermal perfor-

mance of a packed bed reactor for a high-temperature
chemical heat pump. International Journal of Energy
Research 2001;

25:577–589.

39. Kato Y, Yoshizawa Y. Application of a chemical heat

pump to a cogeneration system. International Journal
of Energy Research 2001;

25:129–140.

40. Zam

firescu C, Naterer GF, Dincer I. Upgrading of

Waste Heat for Combined Power and Hydrogen
Production

With

Nuclear

Reactors.

Journal

of

Engineering for Gas Turbines and Power 2010;
132:102911–102919.

41. Smith JM, Van Ness HC, Abbott MM. Introduction To

Chemical Engineering Thermodynamics. Mc-Graw
Hill: New York, 2001; 169

–176.

42. Raldow WM, Wentworth WE. Chemical heat pumps--

A basic thermodynamic analysis. Solar Energy
1979;

23:75–79.

43. Raldow W. Thermal ef

ficiencies of chemical heat

pump con

figurations. Solar Energy 1981; 27:307–311.

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.

DOI: 10.1002/er

44. Moran MJ, Shapiro HN. Fundamentals of Engineering

Thermodynamics. John Wiley & Sons Inc.: West
Sussex, 2006; 303

–306.

45. Ahern JE. The Exergy Method of Energy Systems Anal-

ysis. John Wiley & Sons Inc.: New York, 1980; 45

–52.

46. Jones JB, Hawkins GA. Engineering Thermodynamics:

An Introductory Textbook. John Wiley & Sons Inc.:
New York, 1986.

47. Ishida M, Zheng D, Akehata T. Evaluation of a chemi-

cal-looping-combustion power-generation system by
graphic exergy analysis. Energy 1987;

12:147–154.

48. Lee J-S, Kim J-H, Kim J-T, Suh J-K, Lee J-M, Lee

C-H. Adsorption Equilibria of CO

2

on Zeolite 13X

and Zeolite X/Activated Carbon Composite. Jour-
nal

of

Chemical

&

Engineering

Data

2002;

47:1237–1242.

49. Lander JJ. Experimental Heat Contents of SrO,

BaO, CaO, BaCO

3

and SrCO

3

at High Tempera-

tures. Dissociation Pressures of BaCO3 and SrCO3.
Journal of the American Chemical Society 1951;
73:5794–5797.

50. Perry RH, Green DW. Perry

’s Chemical Engineering

Handbook. McGraw-Hill: New York, 1997.

51. Jacobs GK, Kerrick DM, Krupka KM. The high-

temperature heat capacity of natural calcite (CaCO

3

).

Physics and Chemistry of Minerals 1981;

7:55–59.

Exergetic efficiency of high-temperature high-lift chemical heat pump

M. Arjmand, L. Liu and I. Neretnieks

Int. J. Energy Res. (2012) © 2012 John Wiley & Sons, Ltd.
DOI: 10.1002/er