Control of auditory distance perception based

on the auditory parallax model

Hae-Young Kim *, YoÃiti Suzuki, Shouichi Takane, Toshio Sone

Research Institute of Electrical Communication and Graduate School of Information Sciences,

Tohoku University, 2-1-1 Katahira, Aoba-ku, Sendai 980-8577, Japan

Received 27 November 1999; received in revised form 17 February 2000; accepted 21 March 2000

Abstract

Simulation of the distance of a sound image within 2 m from a listener, in the absence of re¯ec-

tions and a loudness cue, was investigated. To do this, a model named the ``auditory parallax

model'', which focuses on the role of parallax angle information involved in head-related transfer

functions (HRTFs), was examined with psychoacoustical experiments. For purposes of com-

parison, experiments were also done on an actual sound source, sound with digitally synthe-

sized HRTFs, and sound with the interaural time di€erence (ITD) and interaural level

di€erence (ILD) synthesized by the Hirsch±Tahara model. The perceived distance of a sound

image by the actual sound source monotonically increased with the physical distance of the

source up to 1±1.5 m without any cues of sound pressure level and re¯ections from walls. The

perceived distance of a sound image simulated with the auditory parallax model and that with

synthesized HRTFs showed tendencies very similar to those with the actual sound source. On the

other hand, for a sound image produced by the Hirsch±Tahara model, the perceived distance of

the sound image increased up to around 40 cm and then became saturated. These results show

that simple synthesis of the ITD and ILD as a function of distance is insucient to explain auditory

distance perception. However, the results of the experiment in which HRTFs were simulated

based on the auditory parallax model showed that the cues provided by the new model were

almost sucient to control the perception of auditory distance from an actual sound source

located within about 2 m. Possible reasons for the good performance of the auditory parallax

Applied Acoustics 62 (2001) 245±270

www.elsevier.com/locate/apacoust

0003-682X/01/$ - see front matter # 2001 Elsevier Science Ltd. All rights reserved.

PII: S0003-682X(00)00023-2

* Corresponding author at current address: Akita Prefectural University, Faculty of Systems Science

and Technology, Department of Electronics and Information Systems, 84-4 Ebinokuch, Tsuchiya, Honjo,

015-0055 Japan. Tel.: [phone]; fax: [phone].

E-mail address: [e-mail] (H.-Y. Kim).

model are the resemblance between the relative frequency characteristics (shape) as well as the

shape of ILD as a function of frequency simulated by the auditory parallax model and those

of the actual HRTFs. # 2001 Elsevier Science Ltd. All rights reserved.

Keywords: Sound localization; Auditory distance perception; Modeling of sound image; Sound image

control; Auditory parallax

1. Introduction

The direction of a sound source yields very important information at a listener's

eardrum for the perception of the location of the sound source. Therefore, the

notion of head-related transfer function (HRTF), which represents the transfer

characteristics of a sound path from a sound source in a free ®eld to the listener's

external ear, has been introduced [1]. HRTF is generally important when consider-

ing sound localization because it is useful for expressing sound pressure at the liste-

ner's eardrum over the whole frequency range in question. Moreover, the interaural

di€erence in HRTFs is also important since it involves the ITD (interaural time

di€erence) and the ILD (interaural level di€erence) collectively as a function of fre-

quency. The sound localization on the horizontal plane is primarily related to the

interaural di€erence of HRTFs [2±4], while some spectral features of the HRTFs

(spectral cues) play some role in determination of azimuthal localization [5]. In the

median plane localization, spectral cues seem to play an important role, and many

studies have been done to elucidate the role of such spectral cues [6±11].

As compared with the auditory perception of direction, however, auditory dis-

tance perception has not been suciently investigated. Most of the earlier investi-

gations have focused on distance perception under anechoic conditions [12±16].

Distance perception under reverberant conditions has also been investigated by

some researchers [16±19]. Reviews of the literature can be found in references [1,20].

According to those investigations, distance perception is in¯uenced by many factors

such as loudness [21-23], binaural di€erence [12], the ratio of early re¯ections from

the room surface to direct sound [6,17,24], and the decay of high frequency compo-

nents by air absorption [25]. In the absence of re¯ections, the change in loudness as a

function of distance (loudness cue) plays an important role in the auditory distance

perception [22,26]. With re¯ections, it is known that the greater the ratio of re¯ec-

tion to direct sound, the farther the perceived distance. Furthermore, the perceived

distance also depends on the familiarity of sound, e.g. a whisper is localized very

close to a listener and shouting is localized farther than a voice at a normal level of

loudness if they are equally loud [14].

There have been several studies on distance perception in the absence of re¯ections

and loudness cue [22, 26±28]. The perceived distance of a sound image increases when

the actual distance increases to around 1±3 m and then the perceived distance

becomes saturated. Haustein [22], Nielsen [26], von BeÂkeÂsy [27], and Sone et al. [28]

have reported that it becomes saturated at 2±3, 1±2, 1±2 and 1.2±1.5 m, respectively.

This phenomenon of the existence of an upper limit in how far an auditory perceived

distance can be, was termed ``acoustical horizon'' by von BeÂkeÂsy [27]. Morimoto

246

H.-Y. Kim et al. / 62 (2001) 245±270

showed that HRTF systematically changes as a function of distance up to around 1

m [29]. This indicates that the change in the HRTF and its interaural di€erence as a

function of distance may be used as a perceptual cue for auditory distance perception.

In the present study, we focused on the role of the HRTF as well as its interaural dif-

ference in controlling distance perception. To do this, we conducted experiments in

the absence of re¯ections and the loudness cue because, in this condition, the HRTF

may be the only possible source of physical perceptual cues for distance perception.

To examine the e€ectiveness of the HRTF and its interaural di€erence in con-

trolling auditory distance perception for a sound source up to 2 m, four experiments

were conducted. The ®rst experiment was concerned with the perceived distance for

an actual small sound source. In the second one, the HRTF was synthesized as

precisely as possible. In the third experiment, the HRTF was modeled so as to have

only simple ITD and ILD cues as proposed by Hirsch [30] and Tahara [31]. In that

model, the ITD and ILD are constant over the whole frequency range and are calcu-

lated by the di€erence between the two distances from a sound source to the listener's

two ears. In the last experiment, HRTFs for the listener's two ears were modeled so

that only the information due to the auditory parallax angle [42,43] could be realized.

2. HRTF and its synthesis

2.1. Description of HRTF

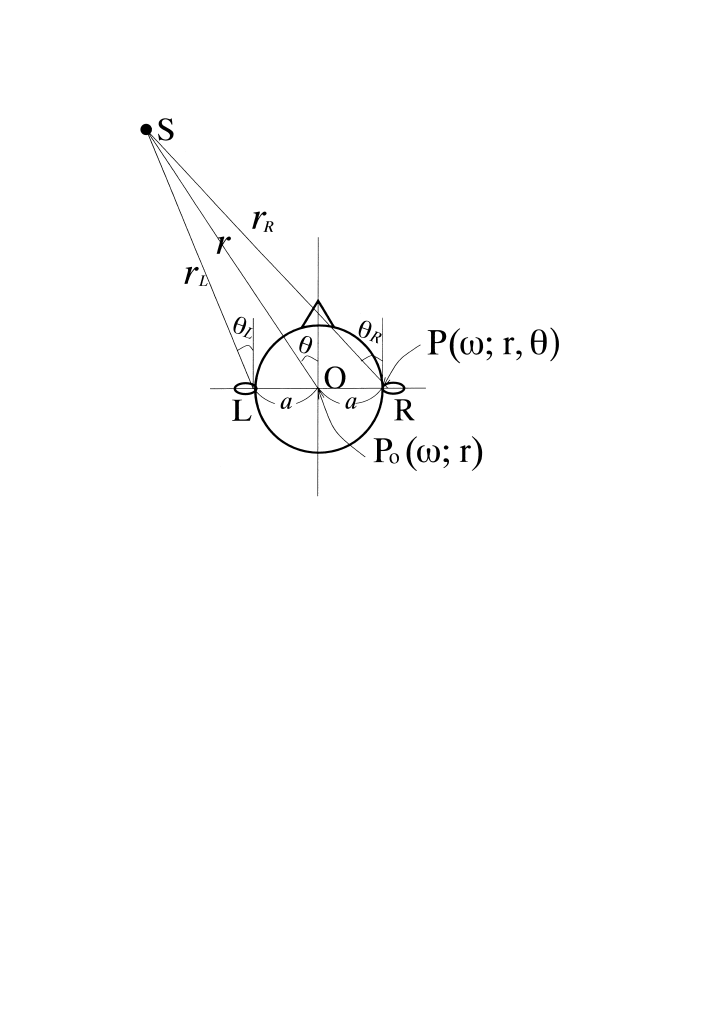
The geometric con®guration between a sound source and the left and right ears of

a listener is shown in Fig. 1. As indicated in that ®gure, the distance from the sound

source to the midpoint of the listener's head is denoted as r, the distances from the

sound source to both ears are r

L

and r

R

, respectively, and the azimuthal angles from

both ears to the sound source are

L

and

R

, where the suxed L and R indicate the

left and the right ears, respectively. The sound pressure at a point corresponding to

the center of the head while the subject is absent, is expressed as P

0

…t; r†. The sound

pressure at the listener's ear canal is expressed as P…t; r; †. Their Fourier transforms

are shown as P

0

…!; r† and P…!; r; †. The HRTF, H…!; r; †, de®ned by Blauert [1] is

expressed as follows:

H…!; r; † ˆ

P…!; r; †

P

0

…!; r†

:

…1†

In order to simplify the explanation of the auditory parallax model in a later sec-

tion, the HRTFs corresponding to both ears, H

L

…!; r; † and H

R

…!; r; †, are

expressed with the points of both ears as a reference. Hence, H

L

…!; r; † and

H

R

…!; r; † are rewritten as G

L

and G

R

, which are expressed as follows:

G

L

…!; r

L

;

L

† ˆ H

L

…!; r; †;

‰

Š

…2†

G

R

…!; r

R

;

R

† ˆ H

R

…!; r; †:

‰

Š

…3†

H.-Y. Kim et al. / 62 (2001) 245±270

247

2.2. Method to synthesize HRTFs using two loudspeakers

Since the HRTF involves comprehensive information on sound localization, it is

quite natural to consider that the localization of a sound source can be controlled by

simulating HRTFs. Schroeder et al. [32] developed the basic theory for simulating

HRTFs and Ando et al. [33] generalized Schroeder's theory. Blauert et al. [34]

approximated this method by use of analogue ®lters and headphones. Morimoto et

al. [35] realized this method by using a digital signal processing technique and con-

ducted some psychoacoustical experiments. They reported that the listener could

localize a sound image synthesized by simulated HRTFs.

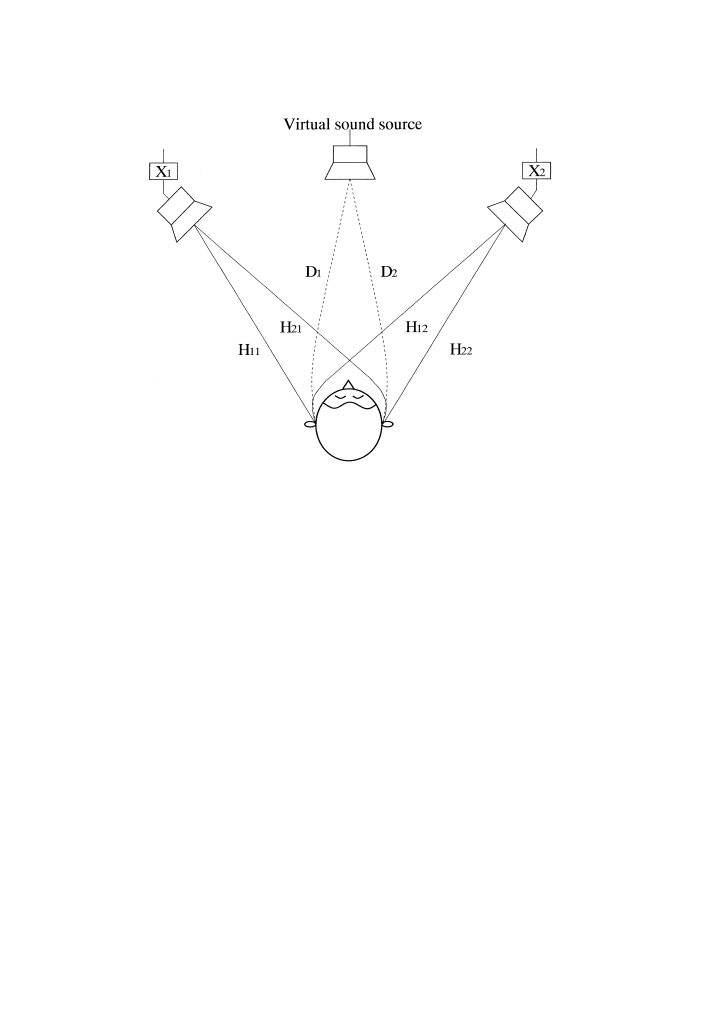
A schematic diagram of the present method is shown in Fig. 2. The transfer

functions for paths from an electric input terminal of a virtual sound source to the

output terminal of microphones at a listener's eardrums of the right and the left ears

are denoted by D

1

…!† and D

2

…!†, respectively. The transfer functions for the four

paths from the electric input terminals of two loudspeakers to the output terminals

of microphones located at the listener's right and left eardrums are indicated by

H

11

…!†, H

12

…!†, H

21

…!† and H

22

…!†, as shown in the ®gure. To reproduce the sound

pressures at the listener's eardrums as those produced by the virtual sound source

using the two loudspeakers, ®lters X

1

…!† and X

2

…!† connected with the electric input

to each loudspeaker are given by the following equation:

Fig. 1. Geometric relationship between the sound source and the left and right ears.

248

H.-Y. Kim et al. / 62 (2001) 245±270

H

11

…!† H

12

…!†

H

21

…!† H

22

…!†

X

1

…!†

X

2

…!†

ˆ D

1

…!†

D

2

…!†

:

…4†

In practice, the two transfer functions, D

1

and D

2

are synthesized by the time-

domain deconvolution technique [36]. Eq. (4) is transformed into the time-domain

as the following equation:

HX ˆ D

…5†

With, H, X and D being expressed as follows:

H ˆ h

11

h

12

h

21

h

22

; X ˆ x

1

…!†

x

2

…!†

; D ˆ d

1

d

2

:

…6†

Here, h

ij

is the matrix consisting of the impulse response between the sound source

j and the control point i, i.e.

h

ij

ˆ

h

ij

0

… †

0

...

..

.

h

ij

M ÿ 1

…

†

h

ij

0

… †

..

.

...

0

h

ij

M ÿ 1

…

†

2
6

6

6

6

6

6

4

3
7

7

7

7

7

7

5

:

…7†

Fig. 2. Block diagram for HRTF simulation using two loudspeakers.

H.-Y. Kim et al. / 62 (2001) 245±270

249

Moreover, x

j

is the ®lter coecient connected to the jth loudspeaker and is

expressed as

x

j

ˆ x

j

…0† . . . x

j

…L ÿ 1†

T

h

i

:

…8†

d

i

is the desired impulse response at point i, that is

d

i

ˆ d

i

…0† d

i

…N ÿ 1†

‰

Š

T

;

…9†

and L, M and N are the lengths of samples of the corresponding impulse responses,

respectively. The ®lter coecients are determined by Eq. (6). Usually, there are more

equations than the unknowns, i.e. this system is overdetermined. An overdetermined

system has no exact solution except for the case when the vector D is an element of

range H. Thus, we strove to minimize HX ÿ D

k

k

2

to solve this equation. The least-

square-error solution X

LS

is then obtained by the following equation:

X

LS

ˆ

H

T

D

H

T

H

:

…10†

X

LS

is the solution which minimizes residual energy at each control point.

3. Modeling of head-related transfer function to control distance perception

3.1. Hirsch±Tahara model

A simple model to control the distance perception for a sound source placed in a

free ®eld is given by Hirsch [30]. The model is based on the level and time di€erences

between two ear signals caused by the di€erence of the distances from a sound

source to the listener's two ears. Tahara proposed a model very similar to Hirsch's

model [31]. His model is based on the assumption that the distance perception is

based on the simultaneity of the changes of the interaural time di€erence and the

interaural level di€erence as a function of distance as well as azimuthal angle. This

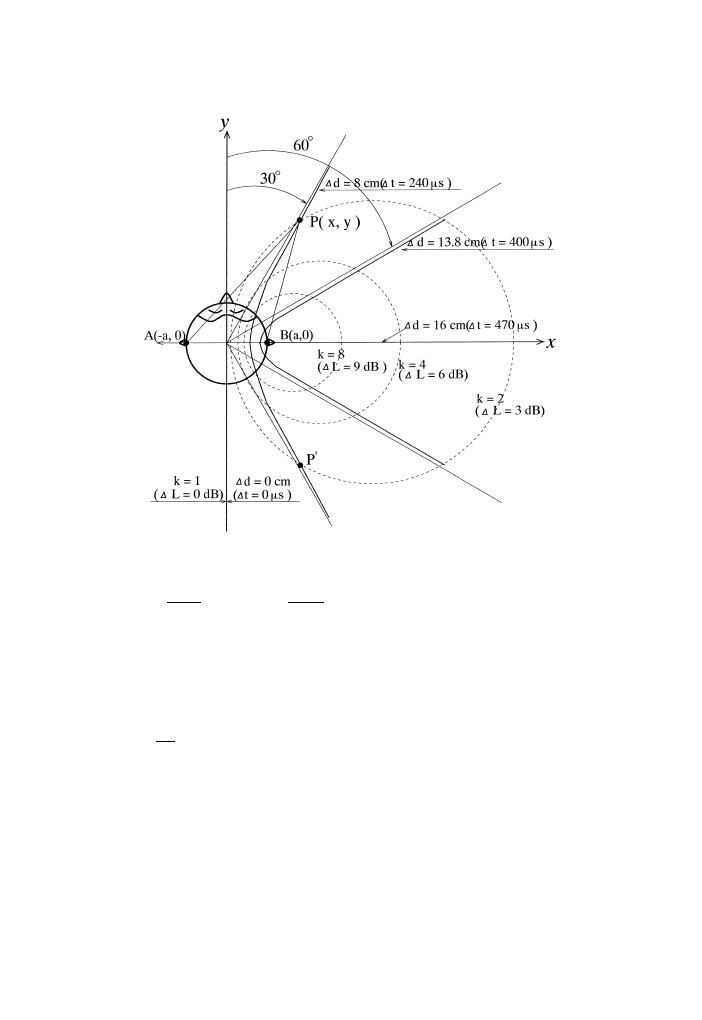
model is referred to as the ``Hirsch±Tahara model'' in this paper. The relationship of

interaural time di€erence and interaural level di€erence for a sound image is shown

in Fig. 3. This model assumes that the location of both ears, A…ÿa; 0† and B…a; 0†,

and the location of a sound source, P…x; y†, are on the horizontal plane. If the

acoustical in¯uence of a head is neglected, the loci of point P…x; y > 0† at which the

distance di€erence d…ˆ PA ÿ PB† between both ears and the square ratio of dis-

tance k ˆ PA

2

=PB

2

are constant, are described by the hyperbolic function of Eq.

(11) and the circular function of Eq. (12), respectively.

x

2

ÿ

d

2

…2a ‡ d†…2a ÿ d†

y

2

ˆ

d

2

2

"

#

;

…11†

250

H.-Y. Kim et al. / 62 (2001) 245±270

x ÿ

k ‡ 1
k ÿ 1

a

2

‡y

2

ˆ

2



ka

p

k ÿ 1

!

2

2
4

3
5:

…12†

Therefore, d and k can be determined, if P…x; y† is given. Then the interaural

time di€erence t and interaural level di€erence L can be calculated using Eqs.

(13) and (14), respectively.

t ˆ

d

c

;

…13†

L ˆ 10 log

10

k

;

…14†

where c is the velocity of sound.

Therefore, if a listener uses t and L as cues for distance perception, a sound

image is perceived at the crossover of the loci of Eqs. (11) and (12). On the contrary,

if this model were valid, subjects perceive a sound image at either location of P or P

0

when speci®c t and L are given. The loci of t and L are actually much more

complicated than described in the above discussion due to the e€ect of head and

Fig. 3. Relation of interaural time di€erence and level di€erence of the sound shown by Tahara [31].

H.-Y. Kim et al. / 62 (2001) 245±270

251

pinnae. In this paper, however, we examine whether the overall tendency in t and

L might be explained by the loci in Fig. 3. It should be noted that this model

cannot explain whether a sound source is in front of or behind the listener because

the e€ect of head and pinnae is disregarded.

3.2. Auditory parallax model

The HRTFs depend on the sound source direction and the distance of the sound

source [20,29,37±41]. Distance localization is concerned with the directional di€er-

ence from both ears to the sound source, since the characteristics of HRTFs mainly

depend on the direction of the sound source. For the purpose of modeling, we focus

on the directional component of the HRTF and ignore the dependence on the dis-

tance. From this point of view, another model of simulating HRTFs for distance

perception has been proposed [42,43]. This model is called the ``auditory parallax

model'' and is based on the assumption that the parallax angle calculated from the

di€erence between the directions from the two ears to the sound source may be

e€ective in controlling the auditory distance perception (Fig. 4).

This model can be explained for the frontal incidence as follows. Suppose there

are two special virtual sound sources as shown in Fig. 5. Virtual sound source A

should realize a transfer function of a sound propagation path from a real sound

Fig. 4. Change of auditory parallax with sound source distance.

252

H.-Y. Kim et al. / 62 (2001) 245±270

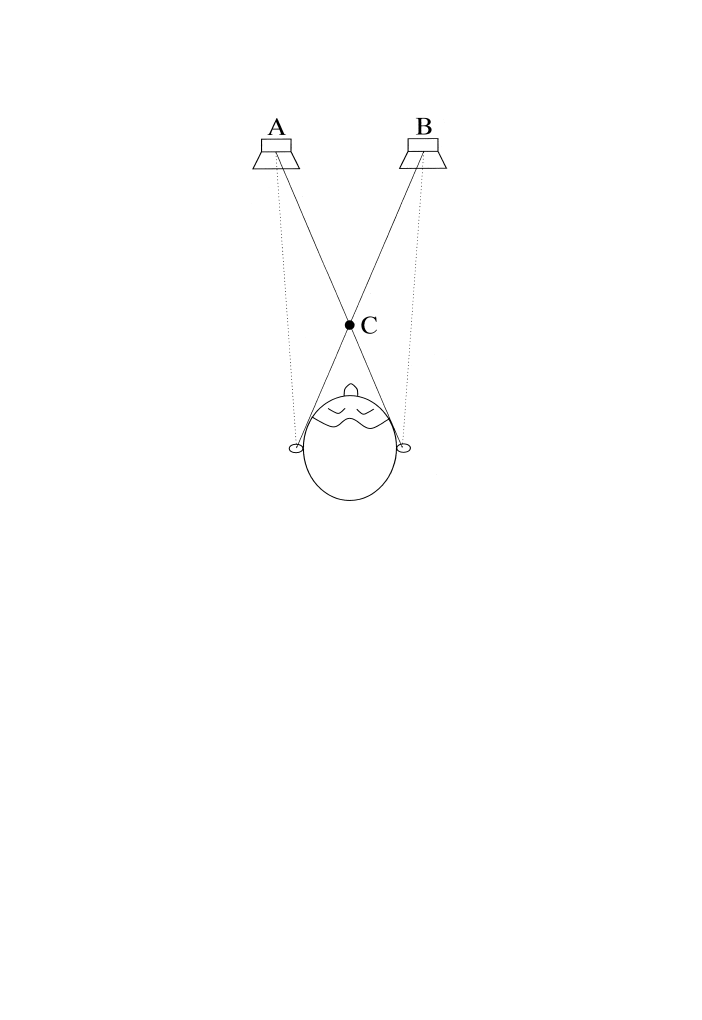
source at the position of A to the right ear (solid line), while for the left ear, a null

transfer function (dotted line) should be realized. Virtual sound source B should

realize a transfer function from a real sound source at the position of B to the left

ear, while for the right ear, a null transfer function should be realized. Under this

condition, it can be expected that a sound image can be perceived at the crosspoint

C if the parallax angle is e€ective in simulating the distance-dependent factors

involved in HRTFs. Such transfer functions are never realized by any real sound

image but may be virtually synthesized by using a digital signal processing technique

as described in detail below. It should be noted that this model cannot be applied to

the lateral directions (90

and 270

) because the directions from both ears to the

sound source are identical. The relationship between the sound source position and

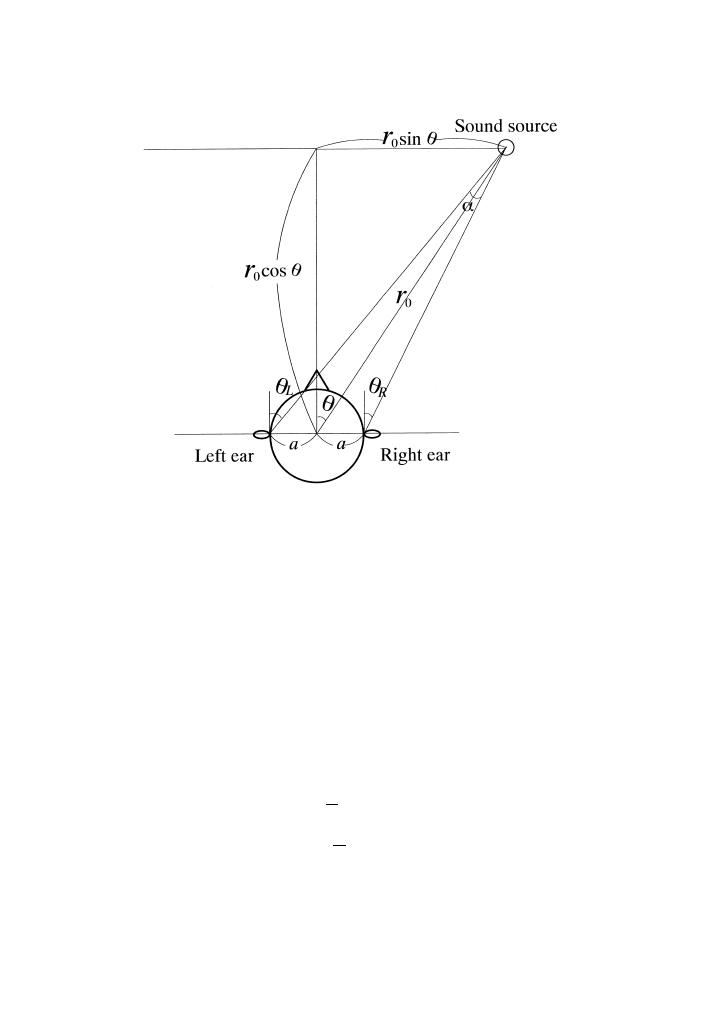
the parallax angle are illustrated in Fig. 6. The azimuthal angles from both ears to

sound sources

L

and

R

are expressed by

L

ˆ tan

ÿ1

……r

0

sin ‡ a†=r

0

cos †

;

…15†

R

ˆ tan

ÿ1

……r

0

sin ÿ a†=r

0

cos †

;

…16†

where r

0

and are the distance and azimuthal angle from the midpoint of the head

to the sound source, respectively. The parallax angle is given as the di€erence in

directions from both ears to the sound source, i.e. ˆ j

L

ÿ

R

j. The value

Fig. 5. Conceptual illustration of auditory parallax model.

H.-Y. Kim et al. / 62 (2001) 245±270

253

decreases as the sound source distance r

0

increases. The change of would yield

some perceivable changes in the di€erence of directional information in HRTFs,

resulting in perceptual cues for the distance perception. In other words, it is assumed

that the sound image can be perceived at the crossing point of simulated HRTFs,

which are synthesized for the two ears independently.

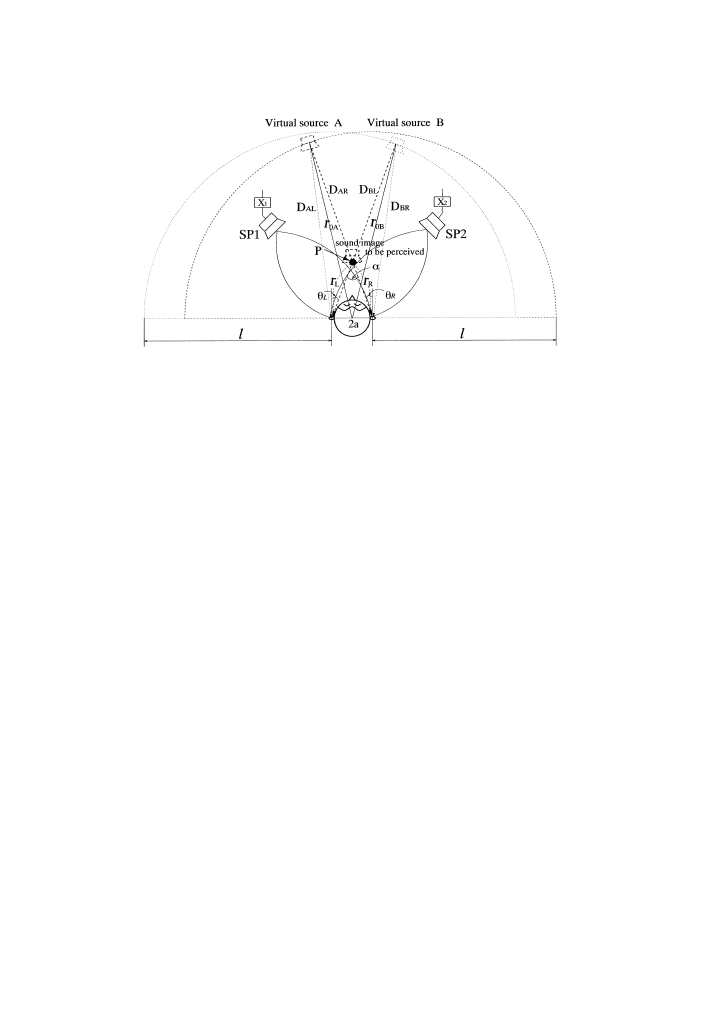
Fig. 7 shows a schematic con®guration of a system for simulating the distance of a

sound image based on the auditory parallax model using two loudspeakers. The

HRTFs for two ears are expressed as G

L

…!; r

L

;

L

† and G

R

…!; r

R

;

R

† as stated in

Section 2.1, where the distances from both ears to the sound source are r

L

and r

R

.

The distance from virtual sound sources A and B to the midpoint of the listener's

head are r

oA

and r

oB

, respectively. If the phase delay due to the di€erence between

the distances from the sound source to the center of the head and the ear is sepa-

rated from G, the HTRFs for the left and right ears can be expressed as Eqs. (17)

and (18), respectively.

G

L

…!; r

L

;

L

† ˆ G

0

L

…!; r

L

;

L

†e

ÿj!…

r0B

c

†

;

…17†

G

R

…!; r

R

;

R

† ˆ G

0

R

…!; r

R

;

R

†e

ÿj!…

r0A

c

†

:

…18†

When a sound image based on this auditory parallax model is synthesized, the

interaural time di€erence is eliminated, i.e. G

0

L

and G

0

R

, rather than G

L

and G

R

, are

Fig. 6. Con®guration of the sound source and listener's ears.

254

H.-Y. Kim et al. / 62 (2001) 245±270

synthesized. In addition, in order to focus on the directional characteristics of

HRTF, both r

L

and r

R

are assumed to be l. That is, only directional information in

HRTFs are synthesized. The HRTF of the virtual sound source A for the listener's

right ear, D

AR

, and that of the virtual sound source B for the listener's left ear, D

BL

,

which are schematically shown by the dashed lines in Fig. 7, are described by Eqs.

(19) and (20), respectively.

D

AR

ˆ G

0

L

…!; l;

L

†

;

…19†

D

BL

ˆ G

0

R

…!; l;

R

†

:

…20†

On the other hand, D

AL

and D

BR

are set to be null transfer functions. In the pre-

sent study, in order to control the auditory distance perception based on this audi-

tory parallax model, the procedure for synthesizing HRTF using two loudspeakers,

described in Section 2.2, was applied; four transfer functions, D

AL

, D

AR

, D

BL

and

D

BR

, were synthesized by the time-domain deconvolution technique. It should be

noted that the sound sources A and B are not real but rather virtual ones which can

only be realized by digital signal processing. According to this model, a sound image

is expected to be localized at the point P where the line from the virtual sound

source A to the right ear and the line from the virtual sound source B to the left ear

cross. These four transfer functions, D

AR

, D

BL

, D

AL

and D

BR

, are synthesized with

digital ®lters X

1

and X

2

combined with loudspeakers SP

1

and SP

2

, respectively, as

shown in Fig. 2.

4. Subjective experiments on distance perception

Four psychoacoustical experiments on auditory distance perception were con-

ducted in this study. First, two experiments with an actual sound source and with a

Fig. 7. Schematic diagram of the simulation system based on the auditory parallax model.

H.-Y. Kim et al. / 62 (2001) 245±270

255

simulated sound source by use of digitally synthesized HRTFs were conducted to

con®rm the human ability of distance perception. Furthermore, two other experi-

ments were carried out to examine the validity and/or limitations of the Hirsch±

Tahara model and the auditory parallax model.

4.1. Common experimental conditions

In all the experiments, a subject was seated in an anechoic room (7.04.62.5 m)

with his head ®xed on a small headrest. The subjects who participated in the experi-

ments were four young males with normal hearing who had previously participated in

other localization experiments. Pink noise up to 20 kHz was used as the signal

sound. The sound pressure level at the location of the center of the subject's head

was always kept constant at 60 dBSPL. The subject judged the perceived distance of

an auditory sound image and reported it using a response sheet by marking a mark

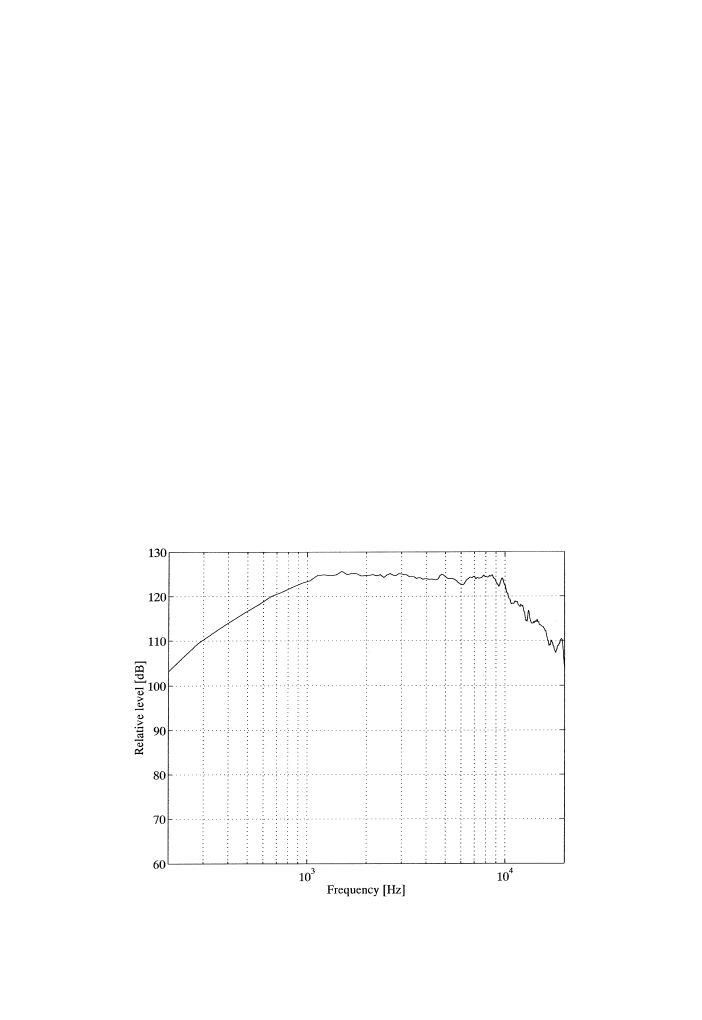
on a line corresponding to 0 cm to 5 m with 10-cm intervals. The frequency char-

acteristics of the loudspeaker is shown in Fig. 8. The stimulus for one experimental

condition was given six times to each subject and all the stimuli were presented in

random order.

4.2. Experiment on distance perception with an actual sound source (Exp. 1)

Auditory distance perception of an actual sound source was examined in the ®rst

experiment. A schematic diagram of this experiment is shown in Fig. 9. The sound

Fig. 8. Frequency characteristics of the loudspeaker.

256

H.-Y. Kim et al. / 62 (2001) 245±270

source was a small movable loudspeaker, 8 cm in diameter, in a 101020 cm

enclosure, the position of the loudspeaker being controlled by a computer. The

loudspeaker was placed at the level of the subject's ears. A curtain was hung

between the loudspeaker and the subject so as to avoid providing any visual infor-

mation about the source distance. Mechanical noise due to the movement of the

loudspeaker was masked by noise radiated from another loudspeaker located behind

the subject. The loudspeaker was positioned at distances of 25, 50, 75, 100, 125, 150,

175 and 200 cm from subject. The direction of the sound source was at one of the

four azimuthal angles of 0

, 45

, 135

and 180

, where 0

was de®ned as the position

in front of a subject, 90

being directly to the left.

4.3. Experiment on distance perception with simulated HRTFs (Exp. 2)

In this experiment, HRTFs at the listener's ears were simulated as precisely as

possible by two loudspeakers placed in an anechoic room. The experimental setup is

represented in Fig. 10. The HRTFs of all subjects were measured individually prior

to the experiment and then simulated by the procedure described in Section 2.2

[32,33,36]. The two loudspeakers were located at 45

on the horizontal plane and

the loudspeaker was placed at the level of the subject's ears.

4.4. Experiment on distance perception based on the Hirsch±Tahara model (Exp. 3)

In this experiment, the interaural time di€erence (ITD) and interaural level dif-

ference (ILD) given by the Hirsch±Tahara model (Section 3.1) were digitally syn-

thesized with the procedure described in Section 2.2. The experimental setup was the

same as in Exp. 2 (see Fig. 10). The ITD and ILD were synthesized for three azi-

muthal angles of 30

45

and 60

at 10, 20, 30, 40, 50, 60, 75, 100, 150 and 200 cm.

Fig. 9. Schematic diagram of the experimental arrangement for distance perception with an actual sound

source (Exp. 1).

H.-Y. Kim et al. / 62 (2001) 245±270

257

The ITD and ILD were set to be constant as de®ned by Eqs. (13) and (14), respec-

tively, over the whole frequency range. The desired transfer functions, D

1

…!† and

D

2

…!†, were also set to be ¯at functions over the whole frequency range.

4.5. Experiment on distance perception based on the auditory parallax model (Exp. 4)

The validity and limitation of the auditory parallax model were examined in this

experiment. The experimental setup is shown in Fig. 7. The procedure described in

Section 3.2 was used to synthesize a sound image. The virtual sound sources A and

B were synthesized on a semicircle whose radius l was 300 cm as shown in Fig. 7.

The HRTF for the virtual sound source A at the listener's right ear, G

0

AR

; and that

for the virtual sound source B at the listener's left ear, G

0

BL

, were used for D

AR

and

D

BL

, respectively, as speci®ed in Section 3.2. The point at which D

AR

and D

BL

cross,

i.e. the point where the sound image is expected to be perceived, was set at 10, 20,

25, 30, 40, 50, 60, 75, 100, 125, 150, 175 and 200 cm. The synthesized directions were

four azimuthal angles of 0

, 45

, 135

and 180

since 90

cannot be realized with

the auditory parallax model.

5. Results

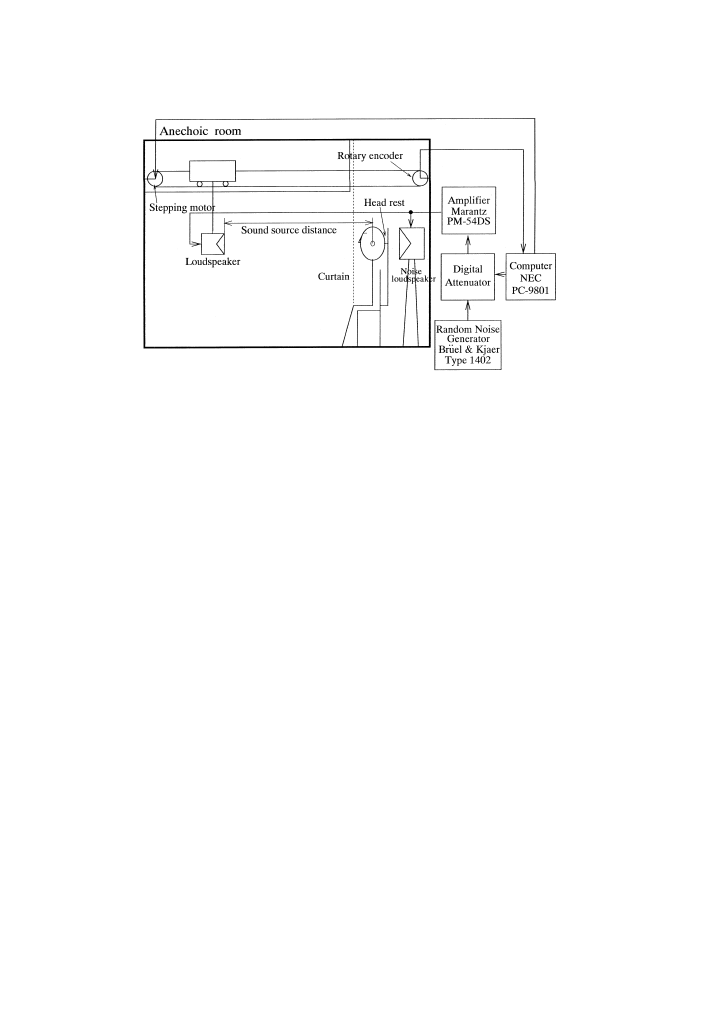
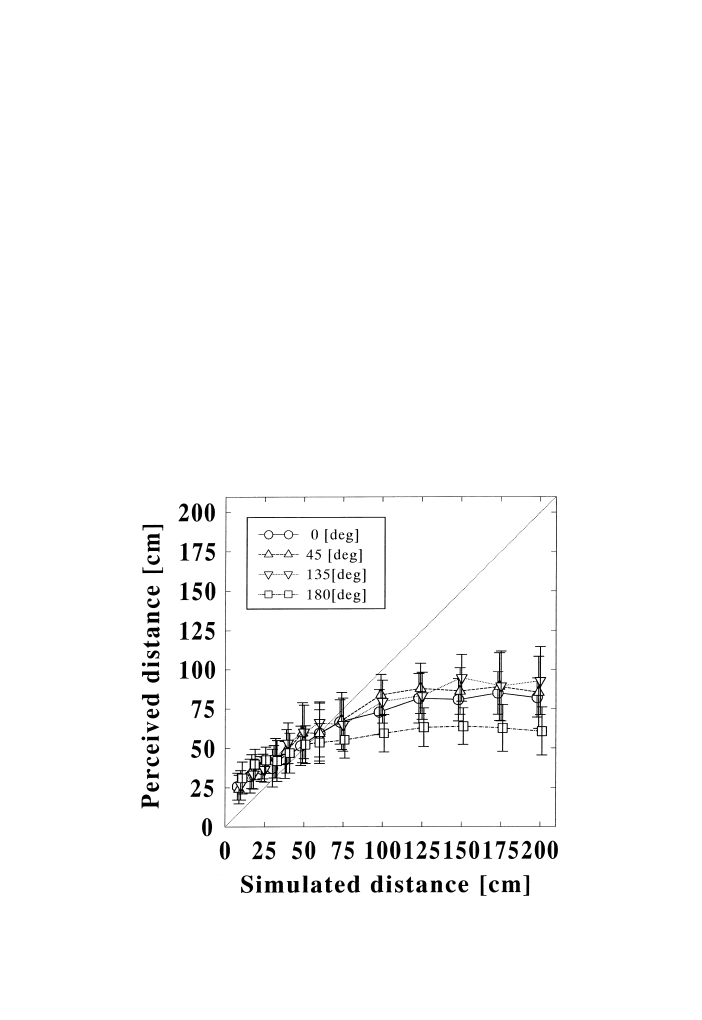
Fig. 11 shows the results of Exp. 1. The abscissa shows the physical distance of the

sound source and the ordinate shows the perceived distance of the sound image. The

results of Exps. 2, 3 and 4 are shown in Figs. 12, 13 and 14, respectively. In each

®gure, the abscissa represents the simulated physical distance and the ordinate

represents the perceived distance of the sound image. The plots are the mean of the

results from four subjects and error bars show standard deviation.

Fig. 11 shows that the perceived distance of a sound source is closer to the lis-

teners than the physical one beyond 50 cm. Fig. 11 also shows that the perceived

Fig. 10. Schematic diagram of the HRTF simulation system using two loudspeakers (Exp. 2).

258

H.-Y. Kim et al. / 62 (2001) 245±270

distance increases up to 75 cm±1 m as the actual distance increases until the actual

distance reaches 1±1.5 m. These results mean that a distance less than about 1.5 m is

perceived due to some spectral change of HRTFs without re¯ections and loudness

cue.

We applied the analysis of variance to the data by three-way ANOVA with the

sound source distances, the sound source directions, and the subjects as sources. The

main e€ects of the sound source distance (F

…7;96†

ˆ 160:52; P < 0:0001), the sound

source direction (F

…3;192†

ˆ 27:46; P < 0:0001), and the subject (F

…3;192†

ˆ 3:85;

P ˆ 0:0095) were statistically signi®cant beyond P ˆ 0:01, respectively. The e€ects

of interaction (sound source distance) (sound source direction) (subject)

(F

…63;6†

ˆ 0:77; P ˆ 0:9007) and (sound source distance) (subject) (F

…21;24†

ˆ 0:89;

P ˆ 0:5999) were not signi®cant, while the interactions, (sound source distance)

(sound source direction) (F

…21;24†

ˆ 2:46; P ˆ 0:0003) and (sound source direction)

(subject) (F

…9;48†

ˆ 3:95; P < 0:0001) were signi®cant beyond P ˆ 0:01. Furthermore,

we used a multiple comparison test (Fisher's LSD: least signi®cant di€erence test)

with a signi®cance level of P ˆ 0:01 to examine the di€erence in the perceived dis-

tances between the sound source distances and the sound source directions. The

results showed that the listener's perceived distance of an actual sound source at 1 m

is judged as being signi®cantly closer to him than those at 1.25±2.0 m. When the

Fig. 11. Perceived distance from an actual sound source when the sound level at the subject's position was

kept constant.

H.-Y. Kim et al. / 62 (2001) 245±270

259

sound source distance exceeded 1 m, no signi®cant di€erence was found. This means

that the perceived distance increases as the physical sound source distance increases

up to 1.25 m. As to the azimuthal angle, no statistical signi®cance was found

between 0

and 180

, nor between 45

and 135

, while the perceived distances within

other pairs of directions were found to be signi®cant. The perceived distance for

direction of 45

and 135

were found to be slightly greater than that for 0

and 180

.

This suggests that the change in the interaural di€erences as a function of distance

may be an e€ective cue for the auditory distance perception.

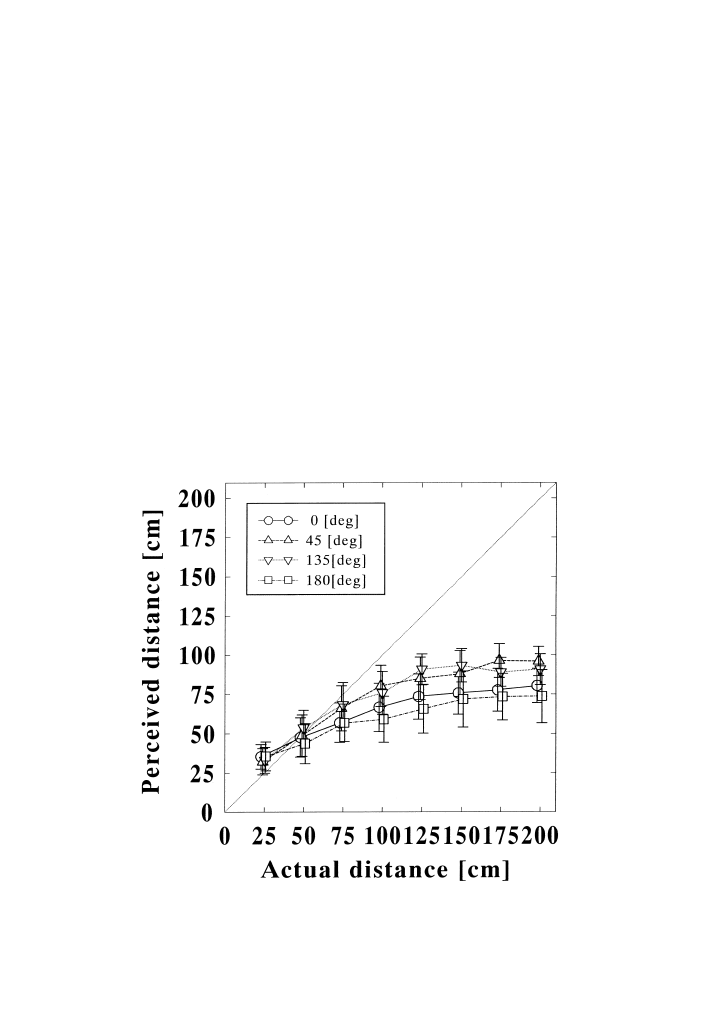
The experimental results of Exp. 2 for various source azimuthal angles are shown

in Fig. 12. This ®gure shows that the perceived distances of a synthesized sound

image with simulation of HRTFs increase up to the simulated distance of 1±1.5 m.

This tendency is very similar to that in Exp. 1. The results recon®rm that some cues

included in HRTFs are useful for auditory distance perception even in the absence

of re¯ections and the loudness cue as long as the distance is closer than 1±1.5 m.

We also applied three-way ANOVA to the results with the same sources as in

the results of Exp. 1. The main e€ects of the sound source distance

(F

…7;96†

ˆ 173:59; P < 0:0001), the sound source direction (F

…3;192†

ˆ 6:12;

P ˆ 0:0040) and subject (F

…3;192†

ˆ 16:56; P < 0:0001) were signi®cant beyond

P ˆ 0:01. The interactions, (sound source distance) (source direction) (subject)

(F

…63;6†

ˆ 0:78; P ˆ 0:9591), (sound source distance) (sound source direction)

Fig. 12. Perceived distance from a simulated sound source with synthesized HRTF.

260

H.-Y. Kim et al. / 62 (2001) 245±270

(F

…21;24†

ˆ 0:71; P ˆ 0:2052), and (sound source direction) (subject) (F

…9;48†

ˆ 1:50;

P ˆ 0:4250) were not found to be signi®cant, while the interaction of (sound source

distance) (subject) (F

…21;24†

ˆ 2:33; P ˆ 0:0007) was found to be signi®cant. Fur-

thermore, we performed the multiple comparison tests (LSD) with the signi®cance

level of P ˆ 0:01 for the sound source distances and the sound source directions.

The results showed that the perceived point of the source with simulation of HRTFs

at 1 m is signi®cantly closer than those at 1.25±2.0 m. When the simulated distance

exceeded 1 m, no signi®cant di€erence was found. This means that the perceived

distance increases as the synthesized distance with simulation of HRTFs increases

up to 1±1.5 m. Moreover, it was found that the perceived distances for 45

are sig-

ni®cantly greater than those for 0

, and that the perceived distance for other pairs of

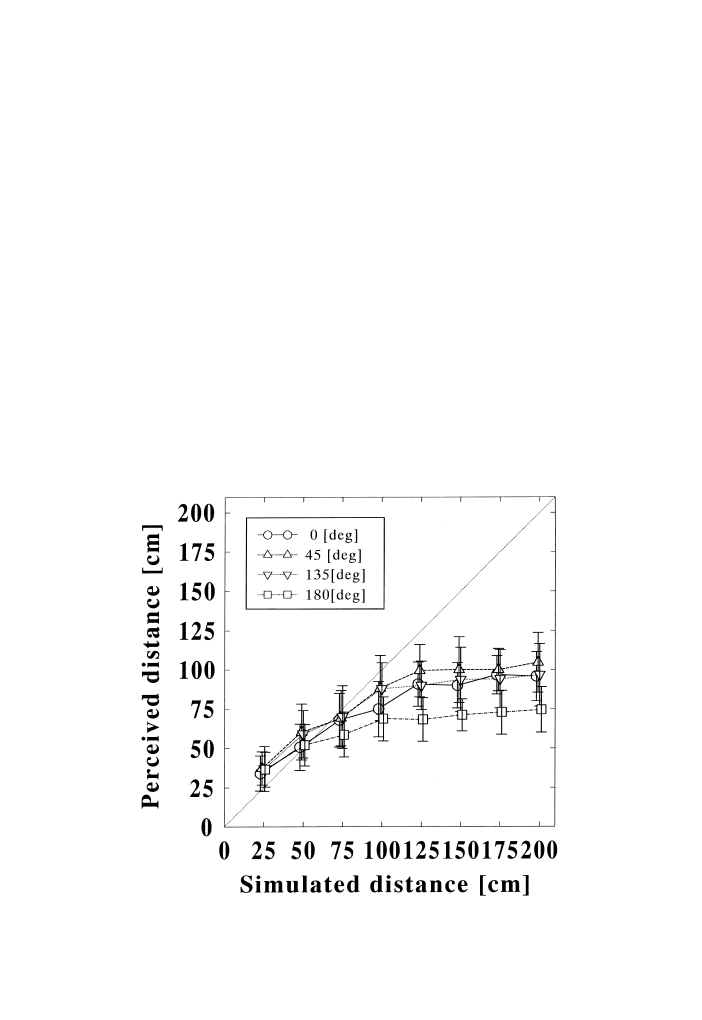
directions were also signi®cant. Fig. 13 shows the results with the Hirsch±Tahara

model. With a sound image generated by this model, the perceived distance of the

sound image increases up to about 40 cm and becomes saturated. This means that

subjects did not perceive the change of ITD and ILD as cues for the distance per-

ception over 40 cm. The change in ILD is noticeable when it exceeds a certain value

(0.5±1.0 dB) [44]. Therefore, if the change in ILD is less than this value, it cannot be

perceived even as a change in distance, as mentioned by Tahara [31]. The above

Fig. 13. Perceived distance from a simulated sound source with the interaural time di€erence and inter-

aural level di€erence of the sound.

H.-Y. Kim et al. / 62 (2001) 245±270

261

results mean that the spectral cues provided by the di€raction and re¯ection from

the head and pinnae are necessary for the perception of distance beyond 40 cm.

From the results of three-way ANOVA, the main e€ect of the sound source dis-

tance (F

…7;72†

ˆ 3:12; P ˆ 0:0031) was signi®cant beyond P ˆ 0:01, but the main

e€ect of the sound source direction (F

…2;192†

ˆ 0:19; P ˆ 0:2962) and that of the

subject (F

…3;144†

ˆ 3:25; P ˆ 0:2151) were not signi®cant (P > 0:01). The e€ect of

interactions (sound source distance) (source direction) (subject) (F

…42;6†

ˆ 0:32;

P ˆ 1:0000), (sound source distance) (subject) (F

…21;18†

ˆ 0:42; P ˆ 0:8910) and

(sound source direction) (subject) (F

…6;48†

ˆ 0:90; P ˆ 0:9582) were not signi®cant,

while the interaction of (sound source distance) (sound source direction)

(F

…14;24†

ˆ 4:04; P < 0:0001) was signi®cant. The result of multiple comparison tests

(LSD) with the signi®cance level of P ˆ 0:01 showed that the perceived distance for

the synthesized source of a distance of 10 cm is signi®cantly closer to a listener than

that for distances between 40 and 200 cm. Moreover, the perceived distances for the

synthesized sources for distances between 40 and 200 cm, except for the perceived

distance of 100 cm, were not signi®cantly more distant than those for 20 and 30 cm.

In short, the perceived distance with the Hirsch±Tahara model increases as the syn-

thesized distance increases only up to 40 cm.

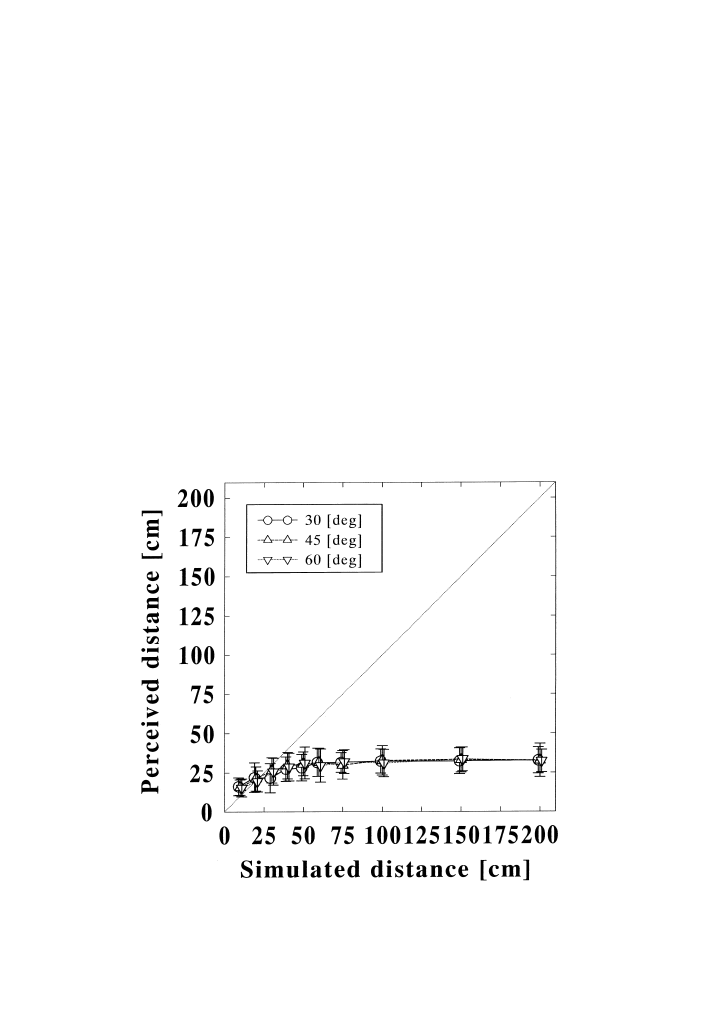
Fig. 14 presents the results with the auditory parallax model. This ®gure shows

that the distance perception with this model is very similar to those for actual sound

Fig. 14. Perceived distance from simulated sound source with parallax information.

262

H.-Y. Kim et al. / 62 (2001) 245±270

source and simulated HRTFs. This suggests that the auditory parallax angles seem

to provide important information when the synthesized distance is within 1±1.5 m in

the absence of loudness cues and re¯ections. Instead, some factors in HRTFs,

depending on the incident direction, may provide information on the distance of a

sound image.

From the results of three-way ANOVA, the main e€ects of the sound source dis-

tance (F

…7;96†

ˆ 116:57; P < 0:0001), the sound source direction (F

…3;192†

ˆ 15:21;

P < 0:0001), and subject (F

…3;192†

ˆ 15:21; P < 0:0001) were found to be signi®cant.

The e€ects of interactions (sound source distance) (source direction) (subject)

(F

…63;6†

ˆ 1:12; P ˆ 0:5200) and (sound source distance) (subject) (F

…21;24†

ˆ 1:49;

P ˆ 0:7363), were not signi®cant (P > 0:01), while the interaction of (sound source

distance) (sound source direction) (F

…21;24†

ˆ 4:53; P < 0:0001) and (sound source

direction) (subject) (F

…9;48†

ˆ 5:59; P < 0:0001) were signi®cant (P < 0:01). The

result of multiple comparison tests (LSD) with the signi®cance level of 0.01 showed

that the perceived distance for the synthesized sound image based on the auditory

parallax model at 1 m is signi®cantly closer than those at 1.25±2.0 m. When the

simulated distance exceeded 1 m, no signi®cant di€erence was found in the perceived

distance. This means that the perceived distance increases as the synthesized distance

with the auditory parallax model increases up to 1.25 m.

The perceived distances between 45

and 135

were not signi®cantly di€erent,

while those between the other pair of directions were signi®cant. Moreover, the

perceived distances for direction of 45

and 135

were signi®cantly greater than

those for 0

and 180

, and the perceived distance of direction of 0

was slightly more

distant than that for 180

. This again suggests that the change of the interaural dif-

ferences as a function of distance could be a cue for the auditory distance perception.

6. Discussion

6.1. Comparison between actual sound source and the auditory parallax model

The results of Exps. 1, 2 and 4 showed a very similar tendency, namely that the

perceived distance monotonically increased up to around 1 m as the simulated dis-

tance increased up to 1±1.5 m and then clearly became saturated. This is the same

tendency previously reported by Morimoto and Nomachi [45] for a sound source

placed in an anechoic room with a sound source distance of 0.2±3 m. Nielsen [16]

also showed similar tendencies when a voice signal was played back in an anechoic

room with a sound source distance of 1±5 m on the horizontal plane. Similar results

were also found by Haustein [22], von BeÂkeÂsy [27], and Sone et al. [28]; the perceived

distance of the sound image becomes saturated at 2±3, 1±2 and 1.2±1.5 m, respec-

tively. Beyond about 1 m, HRTFs hardly change as a function of distance [20,29].

Therefore, the relative change in the spectral shape of HRTFs may be an important

cue for distance judgment up to about 1 m.

A comparison between perceived distances of an actual sound source and those

based on the auditory parallax model is presented in Fig. 15. This ®gure shows that

H.-Y. Kim et al. / 62 (2001) 245±270

263

the perceived distances with the auditory parallax model are almost identical to the

distance of an actual sound source. This suggests that the cues provided by the

parallax angle are useful for the distance perception of a sound source within 1±1.5

m at least from a listener in the absence of re¯ections and the loudness cue.

As mentioned above, the distance perceived by a listener for a sound image syn-

thesized with the auditory parallax model showed tendencies very similar to those

with an actual sound source. In order to discuss the similarity/di€erence of the per-

ceived distances between the two methods in more detail, we performed three-way

ANOVA with the sound source directions, the experimental methods and the sub-

jects chosen as variables for each sound source distance. The results showed that the

main e€ect of the sound source direction was signi®cant beyond P ˆ 0:01 when the

sound source distance was equal to or above 75 cm (for 75 cm, F

…3;48†

ˆ 6:62;

P ˆ 0:0003; for 100 cm, F

…3;48†

ˆ 3:79; P < 0:0001; for 125 cm, F

…3;48†

ˆ 16:48;

P < 0:0001; for 150 cm, F

…3;48†

ˆ 20:35; P < 0:001; for 175 cm, F

…3;48†

ˆ 18:04;

P < 0:001; for 200 cm, F

…3;48†

ˆ 37:04; P < 0:001), while it was not signi®cant

beyond P ˆ 0:01 when the sound source distances were 25 cm (F

…3;48†

ˆ 3:79;

P ˆ 0:0117) and 50 cm (F

…3;48†

ˆ 1:14; P ˆ 0:3342). The main e€ect of the subjects was

not signi®cant beyond P ˆ 0:01 for all the sound source distances (for 25 cm, F

…3;48†

ˆ

Fig. 15. Comparison of the perceived distances from actual sound source and simulated sound source

with parallax information.

264

H.-Y. Kim et al. / 62 (2001) 245±270

0:23; P ˆ 0:8746; for 50 cm, F

…3;48†

ˆ 0:51; P ˆ 0:6726; for 75 cm, F

…3;48†

ˆ 0:69;

P ˆ 0:5587; for 100 cm, F

…3;48†

ˆ 0:64; P ˆ 0:5932; for 125 cm, F

…3;48†

ˆ 1:61;

P ˆ 0:1886; for 150 cm, F

…3;48†

ˆ 3:58; P ˆ 0:0153; for 175 cm, F

…3;48†

ˆ 2:30;

P ˆ 0:0794; for 200 cm, F

…3;48†

ˆ 1:20; P ˆ 0:3112). The main e€ect of the experimental

methods was signi®cant beyond P ˆ 0:01 when the sound source distance were 25 cm

(F

…1;96†

ˆ 29:94; P < 0:001), 50 cm (F

…1;96†

ˆ 11:45; P ˆ 0:0009) and 100 cm (F

…1;96†

ˆ

13:52; P ˆ 0:0003), but not signi®cant for other sound source distances (for 75 cm,

F

…1;96†

ˆ 1:19; P ˆ 0:2771; for 125 cm, F

…1;96†

ˆ 1:08; P ˆ 0:3010; for 150 cm, F

…1;96†

ˆ

3:83; P ˆ 0:0520; for 175 cm, F

…1;96†

ˆ 3:63; P ˆ 0:8731; for 150 cm, F

…1;96†

ˆ 0:09;

P ˆ 0:9787). When the sound source distances were 25, 50 and 100 cm, and the perceived

distances with the auditory parallax model were more distant than those judged for the

actual sound source.

Furthermore, we conducted LSD multiple comparison tests with the signi®cance

level of 0.01 to investigate whether the sound source direction yields signi®cant dif-

ferences in the perceived distance. For the sound source distances from 75 to 125 cm,

the perceived distances of 180

were signi®cantly closer than those for the other

three directions (0

, 45

and 135

) while no signi®cant di€erences were found among

these three directions. When the sound source distance was from 150 to 200 cm, the

perceived distances for 45

and 135

were not signi®cantly di€erent while those for

0

were signi®cantly closer than those for the two directions. Moreover, the per-

ceived distances for 180

were far closer than those for the other three directions.

6.2. Analysis of spectral features involved in HRTF

From the fact that the results of Exps. 1, 2 and 4 showed very similar tendencies,

we focused on the similarity of HRTFs as well as the similarity of their interaural

di€erence to consider ``what parameter of HRTFs could be a cue for distance per-

ception?'' As mentioned in Section 3.2, ITD and ILD due to the di€erence of the

length of sound propagation paths for both ears of the listener is not involved in the

present auditory parallax model. Thus, we consider only spectral factors in HRTFs

and their interaural di€erence.

To investigate the similarity between the measured HRTFs at each actual sound

source distance and the synthesized transfer functions used in Exp. 2 with the two-

loudspeaker method, average level di€erences between the measured HRTFs and

the transfer functions synthesized by the three methods were calculated with the 1/3

octave bands levels. The 1/3 octave band analysis was adopted because 1/3 octave is

close to the critical bandwidth (ca. 1/4 octave). The abscissa in Fig. 16 represents the

sound source distance and the ordinate represents the average of the relative level

di€erence in every 1/3 octave band. The measured HRTFs at each distance and the

synthesized HRTFs for each simulated distance with each method, which were

superposed as their average squared di€erences in 1/3 octave band levels were

minimum. The results showed that the average rms level di€erences between the

measured HRTFs for all the distances and the HRTFs synthesized by the simulation

method with two loudspeakers were below 0.5 dB for all the distances. The level

di€erences between the measured HRTFs and the transfer functions synthesized

H.-Y. Kim et al. / 62 (2001) 245±270

265

based on the auditory parallax model were within around 1±2 dB. These results

show that the transfer functions synthesized with the auditory parallax model as well

as the HRTFs which were directly synthesized well simulate the measured HRTFs,

though the latter are slightly better than the former. This may be a reason for the

Fig. 16. Examples of level di€erence in 1/3 octave band analysis. (Simulation: measured HRTFs vs syn-

thesized HRTFs; parallax: measured HRTFs vs synthesized HRTFs based on the parallax model; Tahara:

measured HRTFs vs synthesized based on the Hirsch-Tahara model.)

266

H.-Y. Kim et al. / 62 (2001) 245±270

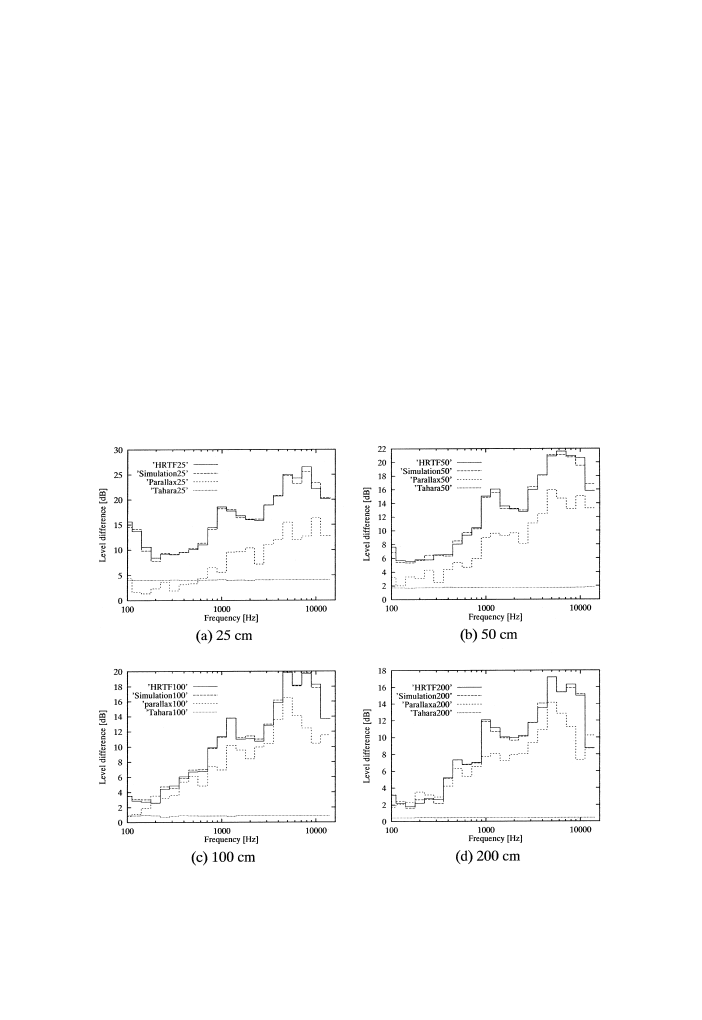
similarity among the experimental results. On the other hand, the level di€erence

between the measured HRTFs and the transfer functions synthesized based on the

Hirsch±Tahara model is around 7±8 dB, because there are no spectral peaks and

dips over all of the frequency bands. Thus, the distance perception of a sound image

may be dicult with the Hirsch±Tahara model.

As described in the previous section, the sound sources at the azimuthal angles of

45

and 135

were localized at a distance farther than in those at 0

and 180

. These

results are in agreement with Haustein [22], Sone et al. [28] and Nielsen [16]; they

found that the ability to judge distance when the sources were placed in a lateral

direction was better than when the sources were in the front or back. This suggests

that the change in the interaural di€erences as a function of distance may be a cue

for the distance perception. Thus, we calculated the interaural level di€erence (ILD)

of the HRTFs and synthesized transfer functions; which were analyzed every 1/3

octave band for each distance. Fig. 17 shows the results. The abscissa shows fre-

quency and the ordinate indicates the ILD for every 1/3 octave with the mean of

four subjects. This ®gure shows that the di€erence in the absolute values of ILD is

rather large between those with the auditory parallax model and those with

Fig. 17. Examples of interaural level di€erence in 1/3 octave band analysis. (Azimuthal angle: 45

,

HRTF: measured HRTFs for each distance; simulation: synthesized HRTFs; parallax: synthesized

HRTFs based on the parallax model; Tahara: synthesized based on the Hirsch±Tahara model.)

H.-Y. Kim et al. / 62 (2001) 245±270

267

measured HRTFs, while the di€erence between those with the simulated HRTFs

and those with measured HRTFs is almost negligible. However, as a function of

frequency, the relative shape of the ILD based on the auditory parallax model is

quite similar to the actual ILD and this may be a reason for the similarity among the

experimental results.

7. Conclusion

Methods to simulate the distance of a sound image within 2 m from a listener in

the absence of re¯ections and the loudness cue was investigated. To do this, a model

named the ``auditory parallax model,'' which focuses on the role of parallax angle

information involved in HRTFs, was examined. Psychoacoustic experiments were

conducted to examine the e€ectiveness in simulating the distance of a sound image.

Experiments with an actual sound source, with digitally synthesized HRTFs and

with ITD and ILD synthesis as proposed by Hirsch and Tahara were also made for

purposes of comparison.

The experiments with an actual sound source showed that distance localization is

possible for a distance of about 1.5 m or less without any cues of sound pressure

level and re¯ections from walls. The perceived distance of a sound image mono-

tonically increased with the physical distance of the source up to 1±1.5 m. If the

presented distance exceeded this, the perceived distance was almost completely

saturated. The distance of a sound image simulated with the auditory parallax

model and that with synthesized HRTFs showed tendencies very similar to those

with the actual sound source. On the other hand, for a sound image yielded due to

the Hirsch±Tahara model, the perceived distance of the sound image increased up to

around 40 cm and then became saturated. These results show that simple synthesis

of the change of the ITD and ILD as a function of distance is insucient to simulate

the distance of a sound image. On the contrary, the results of the experiment in

which HRTFs were simulated based on the auditory parallax model showed very

similar tendencies to those with an actual sound source.

Possible explanations of the similarity between the results with an actual sound source

and those simulated by the auditory parallax model were then examined by comparing

the realized transfer functions. The result showed that the relative frequency char-

acteristic (shape) of the actual HRTFs and that simulated by the auditory parallax

model were quite similar. Moreover, the shape of ILD as a function of frequency was

quite similar between the two methods. These similarities of the frequency character-

istics may be the reason for the good performance of the auditory parallax model.

Acknowledgements

The authors wish to thank Professor Henrik Mùller for meaningful discussion on

the perceptual cues for distance localization and the auditory parallax model. This

268

H.-Y. Kim et al. / 62 (2001) 245±270

research was partially supported by the Ministry of Education, Science, Sports and

Culture, Grants-in-Aid for Scienti®c Research (B), 10450143, 1998 and Scienti®c

Research (C), 10835001, 1998. Part of this work was carried out under the Coop-

erative Research Project Program of the Research Institute of Electrical Commu-

nication, Tohoku University.

References

[1] Blauert J. Spatial hearing. Rev. ed. Cambridge, MA: The MIT Press, 1995.

[2] Damaske P, Wagener B. RichtungshoÈrversuche uÈber einen nachgebildeten Kopf. Acustica

1969;21:30±5.

[3] Shaw EAG. Transformation of sound pressure level from the free ®eld to the eardrum in the hor-

izontal plane. J Acoust Soc Am 1974;56:1848±61.

[4] Kurosawa A, Takagi T, Yamaguchi Z. On transfer function of human ear and auditory localization.

J Acoust Soc Jpn(J) 1981;38:145±9 (in Japanese).

[5] Gotoh T. Can the acoustic head-related transfer function explain every phenomenon in sound loca-

lization? A Symposium Convened at the University of Guelph. 1979:244±9.

[6] Blauert J. Sound localization in the median plane. Acustica 1969;22:205±13.

[7] Shaw EAG, Teranishi R. Sound pressure generated in an external-ear replica and real human ears by

a nearby sound source. J Acoust Soc Am 1968;44:240±9.

[8] Hebrank J, Wright D. Spectral cues used in the localization of sound sources on the median plane. J

Acoust Soc Am 1974;56:1829±1834.

[9] Mehrgardt S, Mellert V. Transformation characteristics of the external human ear. J Acoust Soc Am

1977;61:1567±76.

[10] Morimoto M, Nomachi K. Binaural disparity cues in the median plane localization. J Acoust Soc

Jpn(E) 1982;3:99±103.

[11] Asano F, Suzuki Y, Sone T. Role of spectral cues in median plane localization. J Acoust Soc Am

1990;88:159±68.

[12] Coleman PD. Failure to localize the source distance of an unfamiliar sound. J Acoust Soc Am

1962;34:345±6.

[13] Cochran P, Throop J, Simpson WE. Estimation of distance of a source of sound. Am J Psychol

1968;81:198±206.

[14] Gardner MB. Distance estimation of 0 degree or apparent 0 degree oriented speech signal in anec-

hoic space. J Acoust Soc Am 1969;45:47±53.

[15] Petersen J. Estimation of loudness and apparent distance of pure tones in a free ®eld. Acustica

1990;70:61±5.

[16] Nielsen SH. Auditory distance perception in di€erent rooms. J Audio Eng Soc 1993;41:755±70.

[17] Mershon DH, King LE. Intensity and reverberation as factors in the auditory perception of ego-

centric distance. Perception and Psychophysics 1975;18:409±15.

[18] Mershon DH, Bowers JN. Absolute and relative cues for the auditory perception of egocentric dis-

tance. Perception 1979;8:311±22.

[19] Wagenaars WM. Localization of sound in a room with re¯ecting walls. J Audio Eng Soc 1990;38:99±110.

[20] Begault DR. 3-D Sound for virtual reality and multimedia. Cambridge, MA: AP Professional, 1994.

[21] Stevens SS, Guiro M. Loudness, reciprocality and partition scales. J Acoust Soc Amer 1962;34:1466±

71.

[22] Haustein BG. Hypothesen uÈber die einohrige Entfernungswahrnehmung des menschlichen GehoÈrs.

Hochfrequenztech u Elektroakustik 1969;79:46±57.

[23] Begault DR. Control of auditory distance. PhD dissertation, University of California, San Diego,

1987.

H.-Y. Kim et al. / 62 (2001) 245±270

269

[24] Gotoh T, Kimura Y, Akira A, Yamada A. A consideration of distance perception in binaural hear-

ing. J Acoust Soc Jpn 1977;33:667±71.

[25] Coleman PD. Dual role of frequency spectrum in determination of auditory distance. J Acoust Soc

Am 1968;44:631±2.

[26] Nielsen SH. Distance perception in hearing, Doctoral dissertation. Aalborg University, 1991.

[27] von BeÂkeÂsy G. The moon illusion and similar auditory phenomena. Am J Psychol 1949;62:540±52.

[28] Sone T, Suzuki Y, Takane S, Suzuki H. Distance perception in sound localization and its control by

simulation of head-related transfer functions. Proc 14th Intnl Cong Acoust L7-4, 1992.

[29] Morimoto M, Ando Y, Maekawa Z. On head-related transfer function in distance perception. Proc

Cong of Acoust Soc Jpn 1975;137-8 (in Japanese).

[30] Hirsch HR. Perception of the range of a sound source of unknown strength. J Acoust Soc Am

1968;43:373±4.

[31] Tahara Y, Sakurai H. A tentative model for the localization of sound based on simultaneity of time

di€erence and level di€erence of the sound between ears. Proc Cong of Acoust Soc Jpn 1974;161±2

(in Japanese).

[32] Schroeder MR, Atal BS. Computer simulation of sound transmission in rooms. IEEE Int Conv Part

7, 1963;150±5.

[33] Ando Y, Shidara S, Maekawa Z, Kido K. Some basic studies on the acoustic design of room by

computer. J Acoust Soc Jpn 1973;29:151±9 (in Japanese).

[34] Blauert J, Laws P. Verfahren zur orts- und klanggetreuen Simulation von Lautsprecherbeschallungen

mit Hilfe von KopfhoÈrern. Acustica 1973;29:273±7.

[35] Johren M, Morimoto M, Ando Y. Localization of sound source simulated by a digital computer.

Proc Cong of Acoust Soc Jpn 1977;87±8 (in Japanese).

[36] Yamakoshi K, Watanabe K, Yoshikawa S. Sound localization control system using FIR-®lter.

IEICE Technical Report EA81-34, 1981 (in Japanese).

[37] Batteau DW. The role of the pinna in human localization. Proc Roy Soc Lodon, B168:158±80

[38] Laws P, Platte HJ. Ein spezielles Konzept zur Realisierung eines Kunstkopfes fuÈr die kopfbezogene

stereophone Aufnahmetechnik [A speci®c concept related to the construction of a dummy head for

head-related stereophonic sound pickup]. Rundfunktech Mitt 1978;22:28±31.

[39] Kleiner M. Problems in the design and use of ``dummy heads''. Acustica 1978;41:183±93.

[40] Mùller H, Sùrensen MF, Hammershùi D, Jensen CB. Head-related transfer function of human sub-

jects. J Audio Eng Soc 1995;43:300±21.

[41] Brungart DS, Rabinowitz WM. Auditory localization of nearby sources. Head-related transfer

functions. J Acoust Soc Am 1999;106:1465±79.

[42] Suzuki K, Ozawa K, Suzuki Y, Sone T. A Consideration on the factor of the short distance locali-

zation. Technical Report of IEICE, EA90-29, 1990 (in Japanese).

[43] Suzuki Y, Kim H-Y, Takane S, Sone T. A modeling of distance perception based on auditory par-

allax model. Proc of the 16th Intern. Cong. on Acoust. and 135th Meeting of the Acoust Soc of Am

1998;IV:2903±4.

[44] Rodenburg M. Sensitivity of the auditory system to di€erences in intensity. PhD thesis, Medical

Faculty, Rotterdam. 1972.

[45] Morimoto M, Nomachi K. Distance perception of sound sources simulated by a digital computer.

Proc Cong of Acoust Soc Jpn 1978;287±8 (in Japanese).

270

H.-Y. Kim et al. / 62 (2001) 245±270