E€ect of a novel physical pretreatment process on the drying kinetics

of seedless grapes

Marisa Di Matteo

*

, Luciano Cinquanta

1

, Gianni Galiero, Silvestro Crescitelli

2

Chemical and Food Engineering Department, Salerno University, Via Ponte Don Melillo, 84064 Fisciano, Italy

Received 26 March 1999; accepted 3 April 2000

Abstract

In this paper an alternative physical method for enhancing the drying rate of seedless grapes is proposed. It consists of the

super®cial abrasion of the grape peel using an inert abrasive material. The e€ectiveness of this novel process was compared to that of

the traditional ethyl oleate dipping process by analysing not only their respective drying times, but also the peel surfaces by scanning

electron microscopy. Moreover, the drying kinetics of the above two treatments was reconstructed by using a mathematical model in

which the grape pretreatment used was assumed to a€ect the water di€usivity in the grape peel, but not in the grape pulp. Even

though the abrasion method was found to be as e€ective as the traditional method and gave rise to a darker ®nal product, which is

less attractive to consumers, it would allow grape pretreatment thus avoiding the use of chemical additives, and permit safer raisins

to be produced. Ó 2000 Elsevier Science Ltd. All rights reserved.

Keywords: Grape drying; Abrasion; Dipping; Modelling; Browning

1. Introduction

Grape drying to produce raisins is a very slow process

(King, 1977; Peri & Riva, 1984; Rizvi, 1986; Tutuncu &

Labuza, 1996; Labuza & Hyman, 1998), due to the pe-

culiar structure of grape peel, that is covered by a waxy

layer (Chambers & Possingham, 1963; Aguilera, Op-

permann & Sanchez, 1987; Mahmutoglu, Emõr & Saygi,

1996). Its removal has been so far carried out by using

several chemical pretreatments (Pointing & Mc Bean,

1970; Bolin, Petrucci & Fuller, 1975; Bolin & Sta€ord,

1980; Riva & Peri, 1986; Saravacos & Marousis, 1988).

For example, when grapes are dipped into an alkaline

solution containing, for instance, ethyl oleate, this

component penetrates into the waxy layer and causes

the formation of many small pores. As a consequence,

the drying time of pretreated grapes is up to four times

shorter than the drying time of untreated grapes.

In view of the increasing interest in minimally pro-

cessed food products, the main aim of this work was to

develop an alternative physical pretreatment consisting

of a preliminary abrasion of the grape peel so as to ac-

celerate grape drying to almost the same of that of the

traditional ethyl oleate dipping process. At this point in

time, the feasibility of this process on an industrial scale

was not considered. The aim was to study the e€ec-

tiveness of the process under laboratory conditions.

2. Materials and methods

Seedless white grapes (var. Nevado), harvested from

Trinitapoli in the Puglia region (Italy) and stored at 5°C

for two days before testing, to simulate long distance

transport conditions usually adopted, were used

throughout all experiments. Table 1 shows the main

chemical and physical parameters of about 100 fresh

grape berries.

The abrasion of the grape peel was carried out in a

shaker the walls of which were covered by coating with

abrasive sheets (USM ± Canada, grit range 60±80) as

reported before (Di Matteo, Donsõ, Ferrari, Cinquanta

& La Notte, 1997). Drying experiments were carried out

in a convection oven at 50°C, with an air speed of 0.5 m/

s, so as to reduce the average moisture of grapes to

about 20% w/w. Before drying, samples of about 50

grape berries were submitted to one of the following

pretreatments (TR):

Journal of Food Engineering 46 (2000) 83±89

www.elsevier.com/locate/jfoodeng

*

Corresponding author.

1

Present address: DI.S.T.A.A.M., Molise University, Via F. De

Sanctis, 86100 Campobasso, Italy.

2

Present address: Chemical Engineering Department, Federico II

University, P.le V. Tecchio, 80125 Napoli, Italy.

0260-8774/00/$ - see front matter Ó 2000 Elsevier Science Ltd. All rights reserved.

PII: S 0 2 6 0 - 8 7 7 4 ( 0 0 ) 0 0 0 7 1 - 6

1. immersion in an aqueous solution at 2% (v/v) ethyl

oleate and 2.5% (v/v) K

2

CO

3

at 40°C for 3 min

(EtOl);

2. abrasion in the shaker for 10 min (Abr);

while untreated samples (UT) were used as reference.

Moisture and reducing sugars content of fresh sam-

ples, as well their acidity expressed as equivalent tartaric

acid (by titration with NaOH solution), were measured

according to AOAC (1989). All measurements were

performed in triplicate.

During and after drying, the colour of the berries was

determined three times on 20 di€erent samples by means

of a colorimeter (chroma-meter type CR-200b, Minolta,

Japan) using the Hunter colorimetric system (L, light-

ness; a

, redness; b

, yellowness, as de®ned by Hunter,

1979) and by measuring the absorbance (OD) at 425 nm

(Peri & Riva, 1984) with a spectrophotometer (Varian

DMS 100S).

Before drying, the peel of untreated and pretreated

samples was removed, washed with ethanol and dehy-

drated by means of aqueous solutions at 40±100% (w/w)

acetone. Then, after immersion in liquid nitrogen and

vacuum plating, the peel was examined using a scanning

electron microscope (Stereoscan 90, Cambridge Instru-

ments, UK) to evaluate the e€ectiveness of the physical

and chemical methods at removing the waxy layer from

the grape surface.

During the drying process, at each time t, in addition

to the water content, the axes of the grapes (considered

as ellipsoids) were measured; from these values it was

possible to compute the equivalent radius, R

i

, as the

radius of the sphere having the same volume of the el-

lipsoid. To take into account the volume reduction of

the grape berries that takes place during the dehydration

process, a staircase function was considered for the

equivalent radius R

i

in the mathematical model de-

scribed in the following paragraphs; the value of each

step of the function was computed as the mean between

the initial and the ®nal equivalent radius of each mea-

suring interval.

3. Results and discussion

At the end of the drying process the original structure

of the berries was maintained independently of the

pretreatment used. The abrasion was quite uniform over

the entire surface of the grapes and did not involve any

loss of juice since not one crack was observed both after

the physical pretreatment and after drying. Moreover,

after abrasion the grapes were rougher and had a slight

tissue softening. The drying curves of pretreated and

control grape berries are shown in Fig. 1. Not only was

the drying time needed to reduce the average moisture of

the grape berries from 84% to 20% w/w at 50°C in the

order of 35 h, but also the pattern of the drying curves

was quite similar for both the chemically and physically

treated samples. This drying time was about one third of

the time required to dry the untreated grape berries.

Notation

B

parameter de®ned by Eq. (5)

c

1

water concentration in the grape pulp (moles/m

3

)

c

2

water concentration in the grape peel (moles/m

3

)

c

3

water vapour concentration in air (moles/m

3

)

k

mass transfer coecient, de®ned by Eq. (8)

D

i

water di€usivity in the grape pulp …i ˆ 1† and peel …i ˆ 2†

(m

2

/h)

h

convective mass transfer coecient (m/h)

H

grape humidity, (%) dry matter

K

1

equilibrium constant, de®ned by the ratio between the

equilibrium concentrations of water in the grape pulp and in

the grape peel

K

2

equilibrium constant, de®ned by the ratio between the

equilibrium concentrations of water in the grape pulp and in

the grape peel

K

3

equilibrium constant, de®ned by the ratio between the

equilibrium concentrations of water in the grape pulp and

vapour in air

L

parameter de®ned by Eq. (12)

N

total test number

r

distance from the grape center (m)

R

1

pulp radius (m)

R

2

overall grape berry radius (m)

t

time (h)

Greek symbols

b

n

nth root of Eq. (13)

d

peel thickness …d ˆ R

2

ÿ R

1

† (m)

Subscripts

0

refers to time t ˆ 0

1

refers to grape pulp

2

refers to grape peel

3

refers to air surrounding the grape berry

eq

refers to equilibrium conditions …t ! ‡1†

1

refers to air bulk

Superscripts

TR

refers to treated grape berries: abraded (Abr) or dipped

(EtOl)

UT

refers to untreated grape berries

WP

refers to grape berries with no peel

Table 1

Main chemical composition and dimensions of 100 fresh grape berries

of var. Nevado

Moisture (% w/w)

84:0 1:6

Acidity (% w/w)

0:2 0:01

Reducing sugar (% w/w)

14:1 0:4

Average radius of grape

berries (m)

1:1 0:1…10

ÿ2

†

Average peel thickness

a

(m)

15 0:2…10

ÿ6

†

a

Measured with scanning electron microscope.

84

M. Di Matteo et al. / Journal of Food Engineering 46 (2000) 83±89

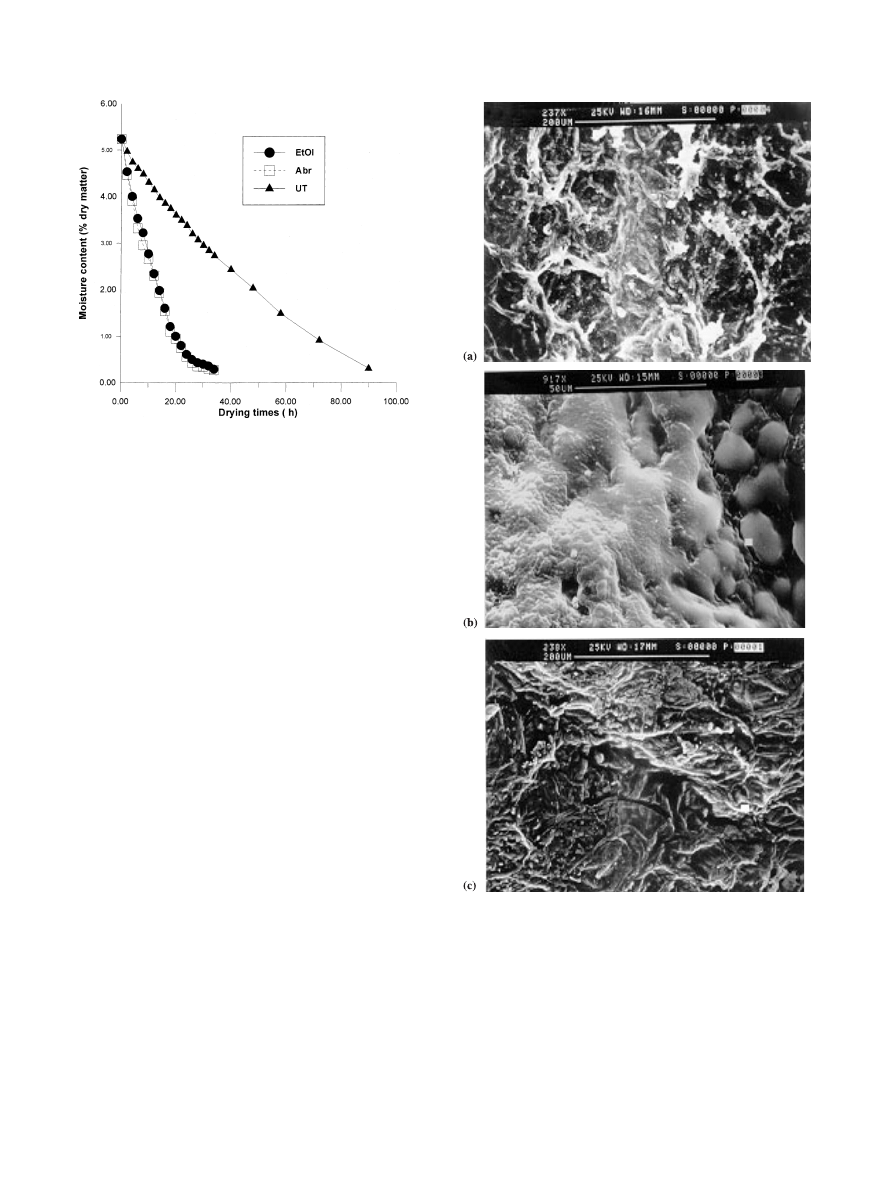
The e€ect of these methods on the waxy layer re-

moval from the grape peel was analysed by scanning

electron microscopy, as shown in Fig. 2.

In the untreated berries, Fig. 2(a) clearly shows the

waxy network. Fig. 2(b) refers to the peel of a sample

dipped in the ethyl oleate solution. As a result of waxy

solubilization by ethyl oleate, a number of micropores

in the waxy layer was formed, but this was accompa-

nied by a non-uniform redistribution of the waxy

component on the berry surface. Finally, in the peel of

the abraded sample, the waxy layer was almost com-

pletely removed in a quite uniform way (Fig. 2(c)).

The total sugar content, measured on a dry basis, did

not vary after drying and berries showed no sticky

surface.

The quality of grape berries and raisins was assessed

by measuring their colour in terms of the Hunter scale

variables (Table 2). In particular, lightness L and the

yellow chroma component (b

) were in¯uenced by the

pre-treatment used. As a result of drying, the variable L

tended to decrease slightly in all samples, its values being

always greater in pretreated samples than in the un-

treated ones; while the variable b

increased in all pre-

treated grape berries, being greater for the chemically

treated samples. After drying the OD values at 425 nm

were lower in chemically treated samples than in phys-

ically and untreated ones.

Generally, the colour of the abraded grape berries

was darker than that of the chemically treated samples,

which showed a lighter colour more appreciated

by consumers (Grncarevic & Hawker, 1971). Since

browning in white grape berries occurs by enzymatic

and non-enzymatic browning reactions (the former be-

ing due to the polyphenoloxidase mainly located in the

peel, Aguilera et al., 1987), the overall e€ect of the grape

peel abrasion appeared to be that of enhancing the de-

gree of enzymatic browning.

Fig. 2. Scanning electron micrograph of the peel of a grape berry

untreated (a), or pre-treated by dipping in ethyl oleate (b), or by

abrasion (c).

Fig. 1. Experimental values of humidity (% dry matter) vs drying times

for grape berries the peel of which was untreated (UT) or pre-treated

by dipping into ethyl oleate (EtOl) or by abrasion (Abr).

M. Di Matteo et al. / Journal of Food Engineering 46 (2000) 83±89

85

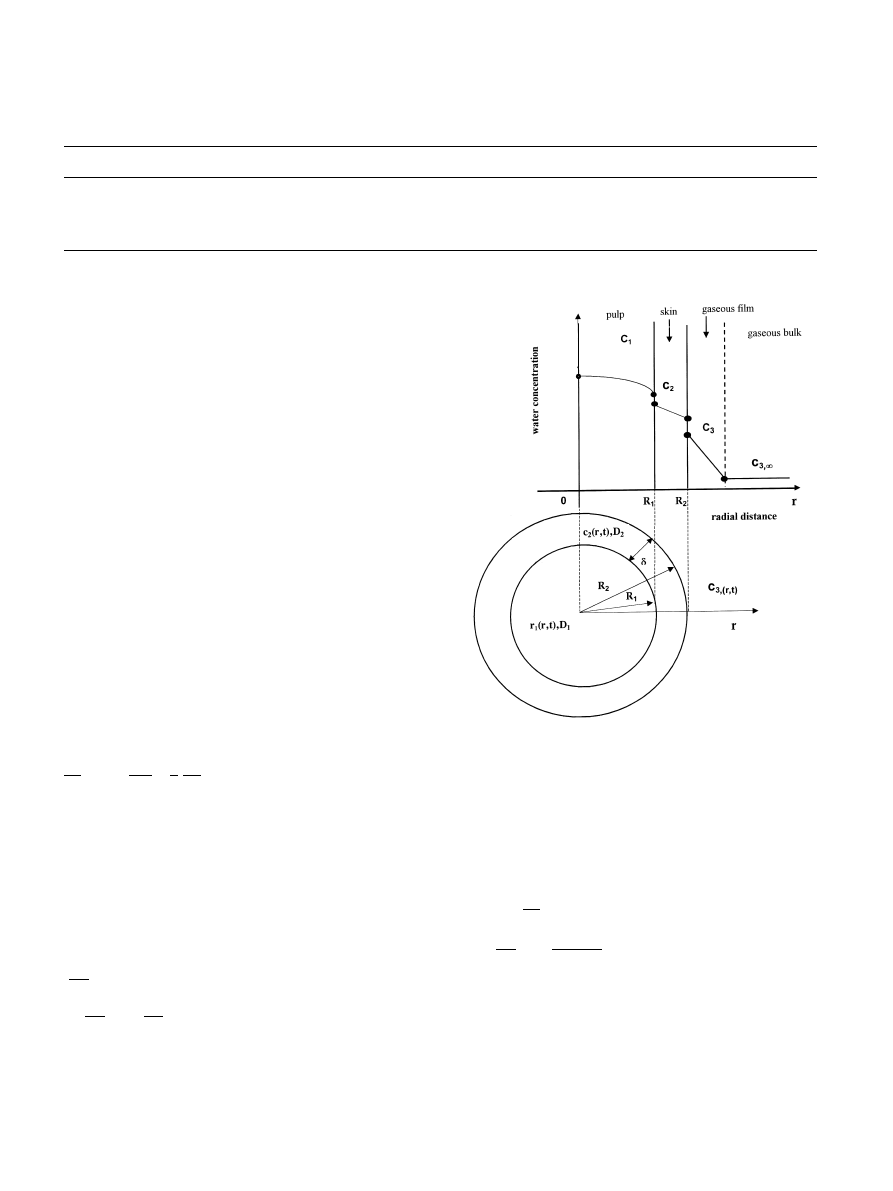
4. Mathematical model of grape dehydration

In the dehydration process of grape berries by means

of warm air, simultaneous heat and water (liquid and

vapour) transport in the pulp, in the peel (if present) and

in the gaseous ®lm surrounding the grapes, take place.

Since the duration of the thermal transient was generally

found to be far less than the duration of the dehydration

process, mass transport may be regarded as taking place

under isothermal conditions. In other words, the whole

drying process is controlled by mass transport only

(Bird, Stewart & Lightfoot, 1960; Peri & Riva, 1984).

Under the assumptions that pulp and peel (if present)

are uniform and isotropic, and the grape berries are

spherical, the mathematical model of grape dehydration

can be reduced to that of mass di€usion from a spherical

body (Bird et al., 1960; Luikov, 1968; Crank, 1975;

Carslaw & Jaeger, 1980).

Fig. 3 shows the schematic water concentration pro-

®les in the grape pulp and peel, as well as in the gaseous

®lm surrounding each grape berry.

The mathematical model that describes the di€usion

of water through whole grape berries must account for

its di€usion both in the pulp and in the peel. Both the

processes, are described by the model:
oc

i

ot

ˆ D

i

o

2

c

i

or

2

‡

2

r

oc

i

or

;

…1†

where the index i ˆ 1 refers to the pulp [i.e., for

r 2 …0; R

1

†] and i ˆ 2 to the peel [i.e., for r 2 …R

1

; R

2

†],

D

1

is the water di€usivity in the grape pulp, which is

much higher than that in the grape peel …D

2

†.

Water concentration within grape pulp can be esti-

mated by solving di€erential equation (1) for i ˆ 1 with

the following initial and boundary conditions:

c

1

…r; 0† ˆ c

10

; r 2 ‰0; R

1

Š; t ˆ 0;

oc

1

or

ˆ 0; r ˆ 0; t > 0;

D

1

oc

1

or

ˆ D

2

oc

2

or

; r ˆ R

1

; t > 0;

c

1

ˆ K

1

c

2

; r ˆ R

1

; t > 0;

…2†

where the equilibrium±distribution curve relating water

concentrations in the pulp/peel interface was assumed to

be a linear one, characterised by the equilibrium con-

stant K

1

. Similarly, water concentration within the grape

peel can be obtained by solving Eq. (1) for i ˆ 2 with the

following initial and boundary conditions:

c

2

…r; 0† ˆ

c

10

K

1

; r 2 ‰R

1

; R

2

Š; t ˆ 0;

ÿ D

2

oc

2

or

ˆ h

c

2

…R

2

; t†

K

2

ÿ c

31

; r ˆ R

2

; t > 0 if h 6ˆ 1;

c

2

…R

2

; t† ˆ K

2

c

31

; r ˆ R

2

; t > 0 if h ! 1;

…3†

where the equilibrium±distribution curve relating water

concentrations at peel/outer environment interface was

assumed to be a linear one, characterised by the

equilibrium constant K

2

. Water di€usion in the gaseous

Fig. 3. Schematic diagram of water (or steam) concentration pro®les in

the grape pulp and peel, and in the gaseous ®lm surrounding each

grape berry.

Table 2

Colour parameters of grape berries as fresh product or dried after 48 h when using no pretreatment (UT), ethyl oleate dipping (EtOl) or abrasion

(Abr)

Grape berries

Samples pretreatment

L

a

b

OD at 425 nm

Fresh

UT

42:3 7:2

)3.3 ‹ 0.4

5.6 ‹ 0.7

0:163 0:02

Dried

UT

37:1 6:5

)3.1 ‹ 0.3

5.4 ‹ 0.6

0:528 0:04

Dried

EtOl

41:8 6:8

2.5 ‹ 0.2

13.8 ‹ 0.9

0:331 0:02

Dried

Abr

38:2 5:2

2.7 ‹ 0.2

9.3 ‹ 0.7

0:493 0:03

86

M. Di Matteo et al. / Journal of Food Engineering 46 (2000) 83±89

®lm around the grape berry was described by means of

the convective mass transfer coecient h (Bird et al.,

1960).

Owing to the thinness of grape (Table 1), it is possible

to neglect water accumulation in the peel, thus consid-

ering a steady-state distribution of c

2

…r; t† in the peel for

any time. In this case, the second boundary condi-

tion for the di€usion in the pulp (if h 6ˆ 1) (Eq. (2))

becomes:

ÿD

1

oc

1

or

j

rˆR

1

ˆ B

c

1

…R

1

; t†

K

1

ÿ c

2eq

;

…4†

with

B ˆ

D

2

d

1

ÿ

D

2

=d

h=K

2

‡ D

2

=d

;

…5†

c

2eq

ˆ K

2

c

31

:

…6†

For h ! 1, Eq. (4) reduces to

ÿD

1

oc

1

or

j

rˆR

1

ˆ k…c

1

…R

1

; t† ÿ c

1eq

†

…7†

with

k ˆ

D

2

dK

1

…8†

and
c

1eq

ˆ K

1

K

2

c

31

:

…9†

In the experiments described here, the gaseous ve-

locity was quite high, thus allowing the resistance to the

mass transport out of the grape to be neglected (i.e.

h ! 1). Under this hypothesis, an analytical solution

(in series) exists (Luikov, 1968; Crank, 1975):
c

1

ÿ c

1eq

c

0

ÿ c

1eq

ˆ

2LR

2

r

X

1

nˆ1

exp…ÿD

1

b

2

n

t=R

2

2

†

b

2

n

‡ L…L ÿ 1†

sin…b

n

r=R

2

†

sin…b

n

†

:

…10†

By integrating Eq. (10) over the whole volume of any

grape berry, it was possible to derive the following time

distribution for its humidity:

H ÿ H

eq

H

0

ÿ H

eq

ˆ 6L

2

X

1

nˆ1

exp…ÿD

1

b

2

n

t=R

2

2

†

b

2

n

…b

2

n

‡ L…L ÿ 1††

;

…11†

with

L ˆ

R

2

k

D

1

;

…12†

when b

n

is the nth root of the following trascendental

equation:
b

n

cot…b

n

† ‡ L ÿ 1 ˆ 0:

…13†

When any grape berry is peeled, its dehydration

process can be mathematically described by accounting

only for the di€usion of water in the grape pulp and

steam in the gaseous ®lm surrounding the grape berry.

The di€usion of water in the pulp is described by dif-

ferential equation (1) with i ˆ 1 together with the fol-

lowing initial and boundary conditions:

c

1

…r; 0† ˆ c

10

; r 2 ‰0; R

1

Š; t ˆ 0;

oc

1

or

ˆ 0; r ˆ 0; t > 0;

D

1

oc

1

or

ˆ h‰c

3

…R

1

; t† ÿ c

31

Š; r ˆ R

1

; t > 0 if h 6ˆ 1;

c

1

…R

1

; t† ˆ c

1eq

; r ˆ R

1

; t > 0 if h ! 1:

…14†

Under the assumptions that in each instant there is a

thermodynamic equilibrium at the interface gas/grape

pulp and that this equilibrium is expressed as a linear

law, it follows that

c

3

…R

1

; t† ˆ

c

1

…R

1

; t†

K

3

if h 6ˆ 1;

…15†

or

c

1eq

ˆ K

3

c

31

if h ! 1:

…16†

Again, since the gaseous velocity was quite high, it is

possible to neglect the resistance to the mass transport

out of the grape (i.e. h ! 1). In these circumstances, an

analytic solution (in series) (Carslaw & Jaeger, 1980) can

be used to estimate water di€usion in a spherical peeled

grape berry (Carslaw & Jaeger, 1980):

c

1

ÿ c

1eq

c

10

ÿ c

1eq

ˆ

X

1

nˆ1

2…ÿ1†

n‡1

R

1

sin…

npr

R

1

†

npr

exp

ÿ

n

2

p

2

D

1

t

R

2

1

:

…17†

By integrating Eq. (17) over the whole volume of any

grape berry devoid of its peel, it was possible to derive

the following time distribution for its humidity:

H ÿ H

eq

H

0

ÿ H

eq

ˆ

6

p

2

X

1

nˆ1

1

n

2

exp

ÿ

n

2

p

2

D

1

t

R

2

1

:

…18†

By considering just the ®rst term of the above series,

it is possible to describe the logarithmic reduction of the

®rst term of Eq. (18) as a linear function of time, as

previously proposed by Peri and Riva (1984):

ln

H ÿ H

eq

H

0

ÿ H

eq

ˆ ln

6

p

2

ÿ

p

2

D

1

R

2

1

t:

…19†

It has to be pointed out that even if this equation has

often been used in the literature to estimate the pa-

rameter D

1

, it should be used only for large values of

t; …t ! 1†: in fact for t ˆ 0 it does not forecast

H ! H

0

.

M. Di Matteo et al. / Journal of Food Engineering 46 (2000) 83±89

87

5. Parameter estimation

The values of unknown parameters of the models

described here were estimated by ®tting the experimental

drying data collected when using peeled, untreated and

chemically and physically treated grape berries. As can

be seen from Fig. 1, humidity±time data for abraded and

dipped berries practically coincided.

The unknown parameters to be estimated are the

following: D

1

and c

1eq

, for all three kinds of grape berries

used, and k for the untreated or treated ones.

A ®rst assessment of such parameters was performed

as follows:

(1) The equilibrium values of the moisture content

…H

1eq

† for all grape berries used was estimated by aver-

aging the experimental humidity determined at the end

of each drying process on the assumption that such

mean values coincided with the equilibrium ones. From

these values …H

WP

eq

; H

UT

eq

; H

TR

eq

†c

1eq

for grapes without

peel …c

WP

1eq

† for untreated grapes …c

UT

1eq

† and for treated

grapes …c

TR

1eq

† can be easily estimated by considering a

suitable spherical volume.

(2) The di€usivity of water in the grape pulp …D

1

† can

be easily estimated via Eq. (19) using the above H

WP

eq

end

value and the experimental H±t data pertaining to

peeled grape berries. Such a parameter was also used to

describe water di€usivity in the grape pulp of all the

samples tested, these being always composed of grape

berries of the same variety, that were almost simulta-

neously harvested in the same region.

(3) The starting value of the mass transfer coecient

k, which accounts for water di€usivity in the untreated

or treated grape peel …D

2

† and equilibrium constant at

the pulp/peel interface …K

1

†, was obtained by plotting

the corresponding experimental humidity ratio

…H ÿ H

eq

†=…H

0

ÿ H

eq

† vs time, by estimating numerically

its slope for t ˆ 0 and by equating such a slope to the

derivative of the above humidity ratio with respect to

time as calculated from Eq. (11) and computed by ac-

counting for the ®rst term of the series only and for

t ˆ 0:

d

dt

H ÿ H

UT

eq

H

0

ÿ H

UT

eq

!

ˆ 6L

2

ÿ D

1

b

2

1

R

2

2

1

b

2

1

…b

2

1

‡ L…L ÿ 1††

!

:

…20†

Since the parameter k is used to de®ne L and thus im-

plicitly b

1

, its estimate has to be obtained by means of

successive iterations.

The optimal estimates of all the unknown parameters

…D

1

; k

UT

; K

TR

; c

WP

1eq

; c

UT

1eq

; c

TR

1eq

† were derived by mini-

mising the following performance index:

U ˆ

X

N

iˆ1

H

S

i

ÿ

ÿ H

T

i

2

…21†

which represents the sum of the squared di€erences

among the experimental humidity H

S

i

data referred to

peeled, untreated and treated grape berries and those

calculated via their corresponding H

T

i

models. More

speci®cally, for the grapes without peel H

T

i

was com-

puted from Eq. (19), thus obtaining H

T

i

ˆ H…D

1

; c

WP

1eq

†;

for the untreated berries H

T

i

was estimated via Eq. (11)

obtaining H

T

i

ˆ H…D

1

; k

UT

; c

UT

1eq

†; and ®nally for chemi-

cally or physically treated grape berries H

T

i

was com-

puted from Eq. (11) obtaining H

T

i

ˆ H…D

1

; k

TR

; c

TR

1eq

†.

Moreover, any water content was forecasted by con-

sidering the ®rst n terms of any series only, n being

suciently high to assure a relative error between the

generic n and …n ‡ 1† terms summation of less than 1%.

In all the forecasts computed a value of n ˆ 2±3 was

sucient.

The minimisation exercise was carried out by using a

non-linear estimation method based on a mixed (direct/

gradient) algorithm (Buzzi Ferraris, 1972) thus leading

to the optimal values of the above unknown parame-

ters. Their covariance matrix was also computed to

determine the con®dence intervals of all the estimates at

a 95% con®dence level (Buzzi Ferraris, 1972; Bard,

1974).

During the minimisation exercise, the progressive

reduction of the dimensions of any grape berries (due

to their loss of water) was taken into account by

considering their equivalent radius, R

i

, as described

before.

The continuous and broken lines in Fig. 1 show the

calculated time variation in moisture content for the

grape berries tested and shows quite a good agreement

with the corresponding experimental values. As previ-

ously stated, it was impossible to detect any moisture

content variation between physically and chemically

treated grape berries.

The optimal value of the water di€usion coecient in

the pulp D

1

equal to

D

1

ˆ 0:4 10

ÿ4

0:1 10

ÿ4

…m

2

=h†;

was found to be larger than the values reported else-

where (e.g. Riva & Peri, 1986), which referred to the

average water di€usivity in the whole grape berry, that is

in its pulp and peel. Therefore, the value determined

here represents the true water di€usivity in the grape

pulp only.

As far as the optimal values are concerned, that is

k

TR

ˆ 2:817 10

ÿ4

2 10

ÿ5

…m=h†;

k

UT

ˆ 7:067 10

ÿ5

1:13 10

ÿ6

…m=h†;

k

TR

was obviously found to be greater than k

UT

and

measures the greater capability of the pre-treatments

used to enhance water di€usivity in the grape skin rel-

ative to that in the untreated samples.

88

M. Di Matteo et al. / Journal of Food Engineering 46 (2000) 83±89

6. Conclusions

Removal of the waxy layer from the grape peel by

abrasion was found to be as e€ective as the traditional

chemical dipping method, as con®rmed by the mass

transport coecient k

TR

which was about 4 times greater

than k

UT

determined for untreated samples. Not only

the drying time but also the pattern of the drying curves

was quite similar for both the chemically and physically

treated samples. Despite the fact that the physical

method gives rise to a more coloured ®nal product than

the chemical one, it makes no use of chemical additives

and therefore allows safer raisins to be produced.

References

Aguilera, J. M., Oppermann, K., & Sanchez, F. (1987). Kinetics of

browning of sultana grapes. Journal of Food Science, 52 (4), 990±

993.

AOAC, 1989. Ocial methods of analysis (15th ed.). Washington, DC:

Association of Ocial Analytical Chemists.

Bard, Y. (1974). Non-linear parameter estimation. New York: Aca-

demic Press.

Bird, B. R., Stewart, W. E., & Lightfoot, E. N. (1960). Transport

phenomena. New York: Wiley.

Bolin, H. R., Petrucci, V., & Fuller, G. (1975). Characteristics of

mechanically harvested raisins produced by dehydration and by

®eld drying. Journal of Food Science, 40, 1036±1038.

Bolin, H. R., & Sta€ord, A. E. (1980). Fatty acid esters and carbonates

in grape drying. Journal of Food Science, 45, 754±755.

Buzzi Ferraris, G. (1972). Experience with an algorithm for

model ®tting and discrimination. Ingegneria Chimica Italiana, 8,

261.

Chambers, T. C., & Possingham, J. V. (1963). Studies on the ®ne

structure of the wax layer of sultana grapes. Australian Journal of

Biological Science, 16, 818±825.

Crank, J. (1975). The mathematics of di€usion. Oxford: Clarendon

Press.

Carslaw, H. S., & Jaeger, J. C. (1980). Conduction of heat in solids.

Oxford: Clarendon Press.

Hunter, R. S. (1979). Scales for the measurement of colour di€erence in

the measurement of appearance. New York: Wiley.

Di Matteo, M., Donsõ, G., Ferrari, G., Cinquanta, L., & La Notte, E.

(1997). The e€ects of pretreatments on the drying kinetics of grapes

and on the quality of raisins. Engineering & Food at ICEF 7,

Sheeld Academic Press, G69±G72.

Grncarevic, M., & Hawker, J. J. (1971). Browning of Sultana grapes

berries during drying. Journal of the Science of Food and Agricul-

ture, 22, 270±272.

Labuza, T. P., & Hyman, C. R. (1998). Moisture migration and

control in multi-domain foods. Trends in Food Science & Technol-

ogy, 9, 47±55.

Luikov, A. V. (1968). Analytical heat di€usion theory. New York:

Academic Press.

King, C. J. (1977). Heat and mass transfer fundamentals applied to

food engineering. Journal of Food Process Engineering, 1, 3±14.

Mahmutoglu, T., Emõr, F., & Saygi, Y. B. (1996). Sun/solar drying of

di€erently treated grapes and storage stability of dried grapes.

Journal of Food Engineering, 29, 289±300.

Peri, C., & Riva, M. (1984). Etude du sechage des raisins 2: E€et des

traitments de modi®cation de la surface sur la qualite du produit.

Sciences des Alimentes, 4, 273±286.

Pointing, J. D., & Mc Bean, D. M. (1970). Temperature and dipping

treatment e€ects on drying times of grapes prunes and other waxy

fruits. Food Technology, 24, 1403±1406.

Riva, M., & Peri, C. (1986). Kinetics of sun and air drying of di€erent

varieties of seedless grapes. Journal of Food Technology, 21, 199±

208.

Rizvi, S. S. H. (1986). Thermodynamic properties of food in

dehydration. In M. A. Rao, & S. S. H. Rizvi, Engineering

properties of foods. New York: Marcel Dekker.

Saravacos, G. D., & Marousis, S. M. (1988). E€ect of ethyloleate on

the rate of air-drying of foods. Journal of Food Engineering, 7, 263±

270.

Tutuncu, M. A., & Labuza, T. B. (1996). E€ect of geometry on the

e€ective moisture transfer di€usion coecient. Journal of Food

Engineering, 30, 433±447.

M. Di Matteo et al. / Journal of Food Engineering 46 (2000) 83±89

89