1

1 Non-Linear Mathematical Steady State Model of the EPN

For EPN (electric power network) there are usually given the active and

reactive powers in the load nodes.

A steady state in the power system is

uniquely

determined

, if in all nodes

these values are known :

- absolute voltage value

U

,

- voltage angle



,

- active power

P

,

- reactive power

Q

.

Usually two values in the nodes are given and the others can be

determined by solution of steady state calculation.

The nodes of the EPN are divided according to values, which are given for

individual node, as follows:

2

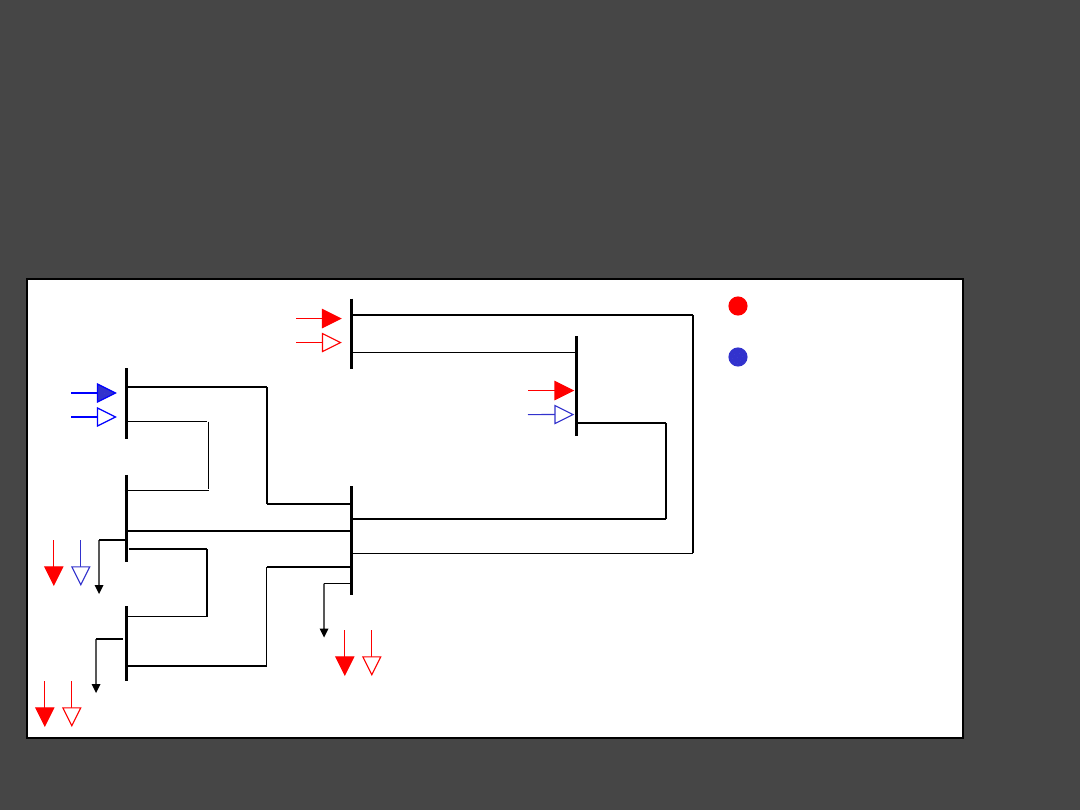
1.1 Node Classification in the EPN

1. Type (

U

,



) – the balance node

This node is usually marked in the equivalent network as the first one. By solution

of steady state we receive the active

P

and reactive power

Q

.

2. Type (

P

,

Q

) – the supply, or load nodes with the given active and reactive power.

The power of supply node and load node differs in a sign. The solution of steady

state are the voltages

U

and their angles



.

3. Type (

P

,

U

) – so called regulation or compensation nodes.

The absolute voltage values and the active power values are given in these nodes.

The solution of steady state is the reactive power

Q

required for keeping the

desired voltage of a node and its angle



.

P

,

U

nodes are used to represent:

- generation nodes with voltage control (by the reactive power),

- nodes with synchronous compensator

- it is synchronous machine without any active

power generation or load (except for losses) used for voltage control (by reactive power)

3

In practical, EPN (with total number of the nodes

n

) is usually given by:

• one balance node (

U

,



),

•

k

nodes (

P

,

Q

),

•

n-k-

1

nodes (

U

,

P

).

given parameters

result of the steady

state calculation

P6

Q6

P1

Q1

P4 Q4

P2

Q2

U1,



1

P5

Q5

U2,



2

U5,



5

U6,



6

P3 Q3

U3,



3

U4,



4

4

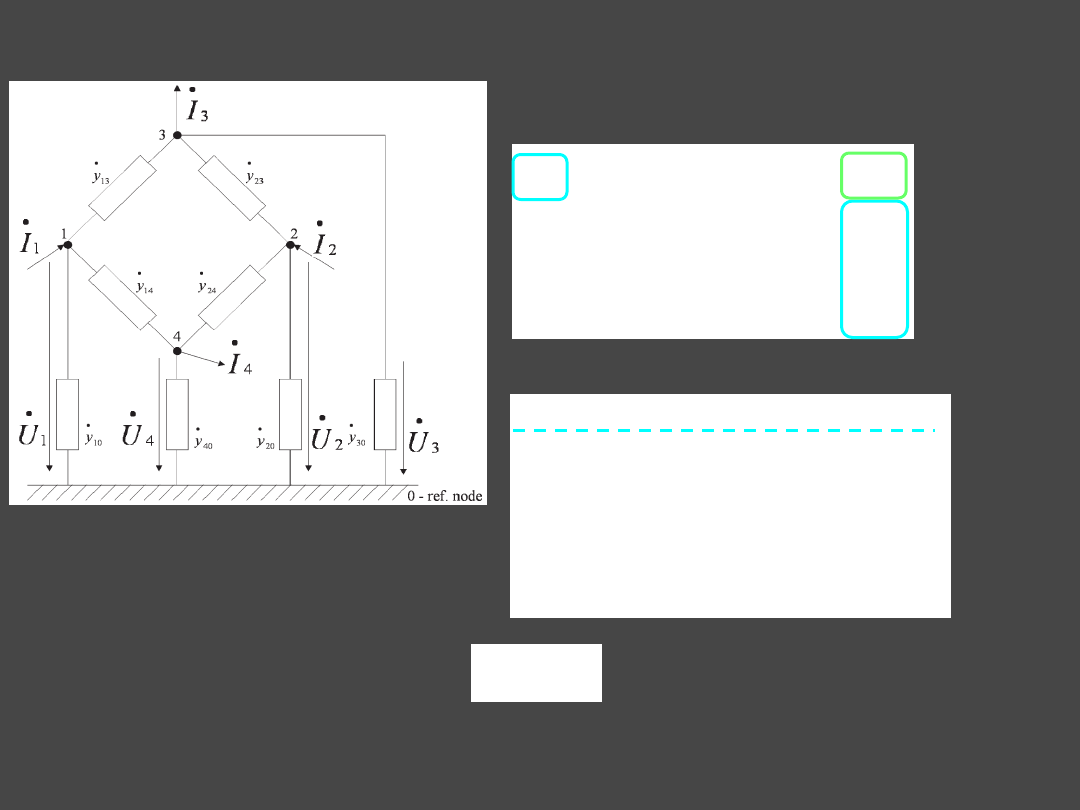
1.2 Formulation of the Equations for Steady State Calculation of EPN

With application of the node voltages method
for the given network we can write:















































































































































4

3

2

1

44

43

42

41

34

33

32

31

24

23

22

21

14

13

12

11

4

3

2

1

.

U

U

U

U

Y

Y

Y

Y

Y

Y

Y

Y

Y

Y

Y

Y

Y

Y

Y

Y

I

I

I

I

By multiplication of this equation we obtain:

4

44

3

43

2

42

1

41

4

4

34

3

33

2

32

1

31

3

4

24

3

23

2

22

1

21

2

4

14

3

13

2

12

1

11

1

.

.

.

.

.

.

.

.

.

.

.

.

.

.

.

.









































































































U

Y

U

Y

U

Y

U

Y

I

U

Y

U

Y

U

Y

U

Y

I

U

Y

U

Y

U

Y

U

Y

I

U

Y

U

Y

U

Y

U

Y

I

unknown

variables

If we can determine unknown variables , it is possible to eliminate the first
equation. We obtain 3 equations with 3 unknown variables.

4

3

2

,

,







U

U

U

known voltage

of the balance

node

5

4

44

3

43

2

42

1

41

4

4

34

3

33

2

32

1

31

3

4

24

3

23

2

22

1

21

2

.

.

.

.

.

.

.

.

.

.

.

.















































































U

Y

U

Y

U

Y

U

Y

I

U

Y

U

Y

U

Y

U

Y

I

U

Y

U

Y

U

Y

U

Y

I

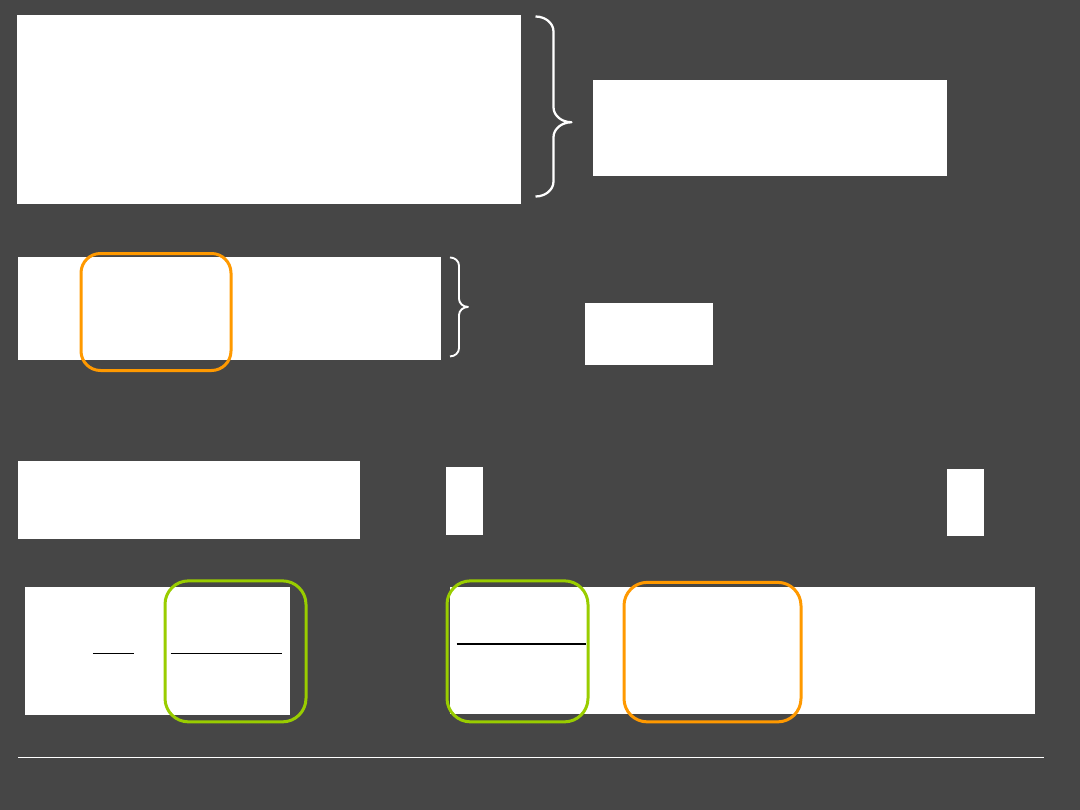
These equations can be written in form:

4

,

3

,

2

.

4

1















i

U

Y

I

j

j

ij

i

Generally, for the network with

n

nodes we can write:

n

i

U

Y

I

n

j

j

ij

i

,

,

3

,

2

.

1

















n

-1 equations with

n

-1 unknown

variables

n

U

U





...,

,

2

In case, that in the

i –

th node is given the active and reactive power, equation for this

power in one phase

*

of the network is:

i

i

i

i

i

I

U

Q

P

S

*

.

j











i

I

*

where is the complex conjugate of the current

i

I



i

i

i

i

i

i

U

Q

P

U

S

I

*

*

*

j











n

i

U

Y

U

Q

P

n

j

j

ij

i

i

i

,

,

3

,

2

.

j

1

*

















*

In the next calculation, we suppose that the system (sources, transmission elements and loads) is symmetric, therefore the

problem can be solved as the one-phase network.

6













n

j

j

ij

i

i

i

U

Y

U

Q

P

1

*

.

j

This model is called

Non-Linear mathematical steady state model of EPN

(the powers are given in the nodes).

This expression presents system of

n

-1 non-linear equations with

n

-1 unknown variables.













n

j

j

ij

i

i

i

U

Y

U

Q

P

1

*

.

.

j



In practise, the non-linear equation system is most often solved by: Gauss-Seidl and

Newton iterative method.

n

i

,

,

3

,

2





n

i

,

,

3

,

2



