SHORT
SHORT
-
-
CIRCUITS IN ELECTRICAL
CIRCUITS IN ELECTRICAL
POWER SYSTEMS
POWER SYSTEMS
dr hab. Irena Wasiak, prof. nadzw.
Institute of Electrical Power Engineering
SHORT
SHORT
-
-
CIRCUITS IN ELECTRICAL
CIRCUITS IN ELECTRICAL
POWER SYSTEMS
POWER SYSTEMS
dr hab. Irena Wasiak, prof. nadzw.
Institute of Electrical Power Engineering
2 /36
Subject program
Subject program
Lecture
1.
General information on short-circuits, short-circuit
current time course
2.
Principles of calculating asymmetrical short-circuits
3.
Equipment impedance in symmetrical components
system
4.
Line-to-earth short-circuits in networks with an
ineffective grounded neutral point
Project
1.
Per unit method
2.
Normalized method of short-circuit calculations
3 /36
Principles of credit
Principles of credit
¾
The lecture is passed based on a written test (in English).
¾
The project is passed based on individual work concerning
calculating short-circuit quantities in a selected electrical power
system.
¾
The final mark is an average from these two forms.
4 /36
Literature
Literature
Basic
1. Notes from the lecture
2. Kanicki A.: Wyznaczanie wielkości zwarciowych w systemie
elektroenergetycznym. Available in e-format.
Additional
1. Kacejko P., Machowski J.: Zwarcia w sieciach
elektroenergetycznych. WNT, Warszawa 1993, 2002
2. Schlabbach J.: Short-circuit currents, IEE, London, 2005
Basic information
Basic information
Short-circuit currents in power systems
Lecture 1
6 /36
Importance of short
Importance of short
-
-
circuit currents
circuit currents
Electrical power systems have to be planned, projected and
constructed in such a way to enable a safe, reliable and economic
supply of loads.
The knowledge about the loading of the equipment is necessary for
the design and determination of the equipment rating.
Short-circuits during the system operation cannot be avoided despite
careful planning and good maintenance of the system. Therefore,
short-circuit currents have an important influence on the design and
operation of equipment and the power system a whole.
Equipment and installations must withstand the expected thermal
and electromagnetic effects of short-circuits. Switchgear and fuses
have to switch-off short-circuit currents in a safe way.
7 /36
Short
Short
-
-
circuit classification
circuit classification
Based on the number of connected points –
symmetrical and asymmetrical
Based on fault impedance –
metallic
(direct) i
resistant
(occurring through impedance, e.g.
electrical arc)
Based on the short-circuit location –
far-from-generator
short-circuit and
near-to-generator
short-circuit
Based on the number of short-circuit places –
single
and multiplace
Based on the location of short circuit places –
internal and
exterior
Based on the moment of short-circuit origin –
simultaneous
and non-simultaneous
Based on the short-circuit duration –
lasting (durable)
and going by
8 /36
Short
Short
-
-
circuit statistics
circuit statistics
Frequency of short-circuit occurrence:
Line-to-earth short-circuit –65% av.(from 30% to 97%)
Double line-to-earth short-circuit and line-to-line short-circuit with
earth –20% av. (from 0% to 55%)
Line-to-line short-circuit 10% av. (from 0% to 55%)
Three-phase short-circuit - 5% av. (from 0% to 35%)
Frequency of short-circuit occurrence depends on nominal voltage of
the network and the type of line. The bigger voltage level and the
bigger share of overhead lines in the network the bigger frequency of
line-to-earth short-circuits.
9 /36
Causes of short
Causes of short
-
-
circuits
circuits
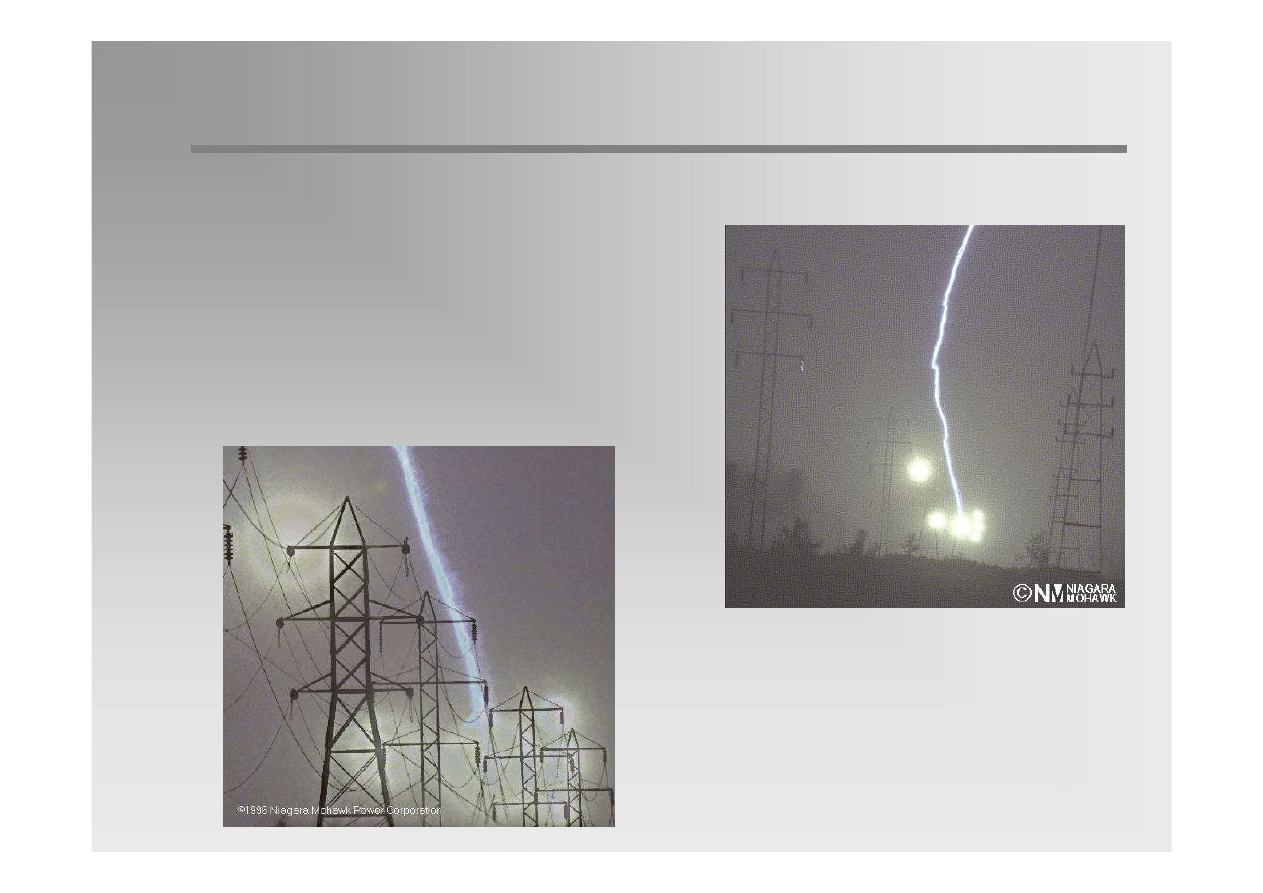
Electrical causes:
Lighting strokes
Switching overvoltages
Switching mistakes
Long-lasting current overloading
10 /36
Causes of short circuits
Causes of short circuits
Non-electrical causes:
Humidity and contamination of the insulation of lines, devices
Ageing of insulation material
Mechanical damages of cables, poles, isolators
Device factory defect
Interference of animals e.g. birds, rodents
Falling over or too high trees
Bringing conductors closer during wind
11 /36
Short
Short
-
-
circuit currents effects
circuit currents effects
Thermal effects
A short-circuit causes large current overloading, which are accompanied by
thermal energy proportional to short-circuit duration. The short-circuit
duration depends on the duration of protection operation.
Dynamic effects
Short-circuit currents cause mechanical forces that affect current conductors;
this may lead to mechanical destruction of equipment. Short-circuits
stimulate mechanical oscillations of generators which can cause problems
with power transfer stability.
Electric shock threat
Short-circuit currents flowing through earth can induce impermissible touch
and step voltages.
Voltage dips and overvoltages
The high value of short-circuit current causes the high voltage of voltage
drop in the network, which results in voltage decreasing in the network
nodes. Overvoltages accompany line-to-earth short-circuits.
Displacement of the voltage neutral-to-earth
12 /36
Short
Short
-
-
circuit currents effects
circuit currents effects
Accidental contact of overhead line conductors with a crane.
Network and system effects
Resulting from switching off the parts of the network being embraced with fault; economic
effects
Threats caused by an electric arc
•
Cable melting-down
•
Insulating materials ignition (oil, paper-oil insulation), emission of smoke and toxic gasses
•
Thermal and ultraviolet radiation of the arc
•
Air heating and blowing-out from the arc space
•
Reducing oxygen in the place where the arc is burning
13 /36
Minimal and maximal short
Minimal and maximal short
-
-
circuits
circuits
Depending on the purpose of engineering studies the maximal and
minimal short-circuit currents are calculated.
The maximal current is the main design criteria for the rating of
equipment to withstand the effects of short-circuit currents, thermal
and electromagnetic.
The minimal short-circuit current is needed for the design of
protection and selection of settings of protection relays.
The short-circuit current depends on various parameters: voltage
level, impedance of the network between any generator unit and
the short-circuit location, number of generation units, fault
impedance, etc. Determination of short-circuit currents requires
detailed knowledge about the elements of electrical power system.
14 /36
(
)
ω + γ = +
0
di
2 Esin
t
Ri L
dt
( )
(
)
(
)
−
=
ω + γ − ϕ −
⋅
⋅
γ − ϕ
R
t
L
0
z
0
z
2 E
2 E
i t
sin
t
e
sin
Z
Z
( )
2
2
Z
R
L
=
+ ω
ω
ϕ =
z
L
arctg
R
Short
Short
-
-
circuit current time course
circuit current time course
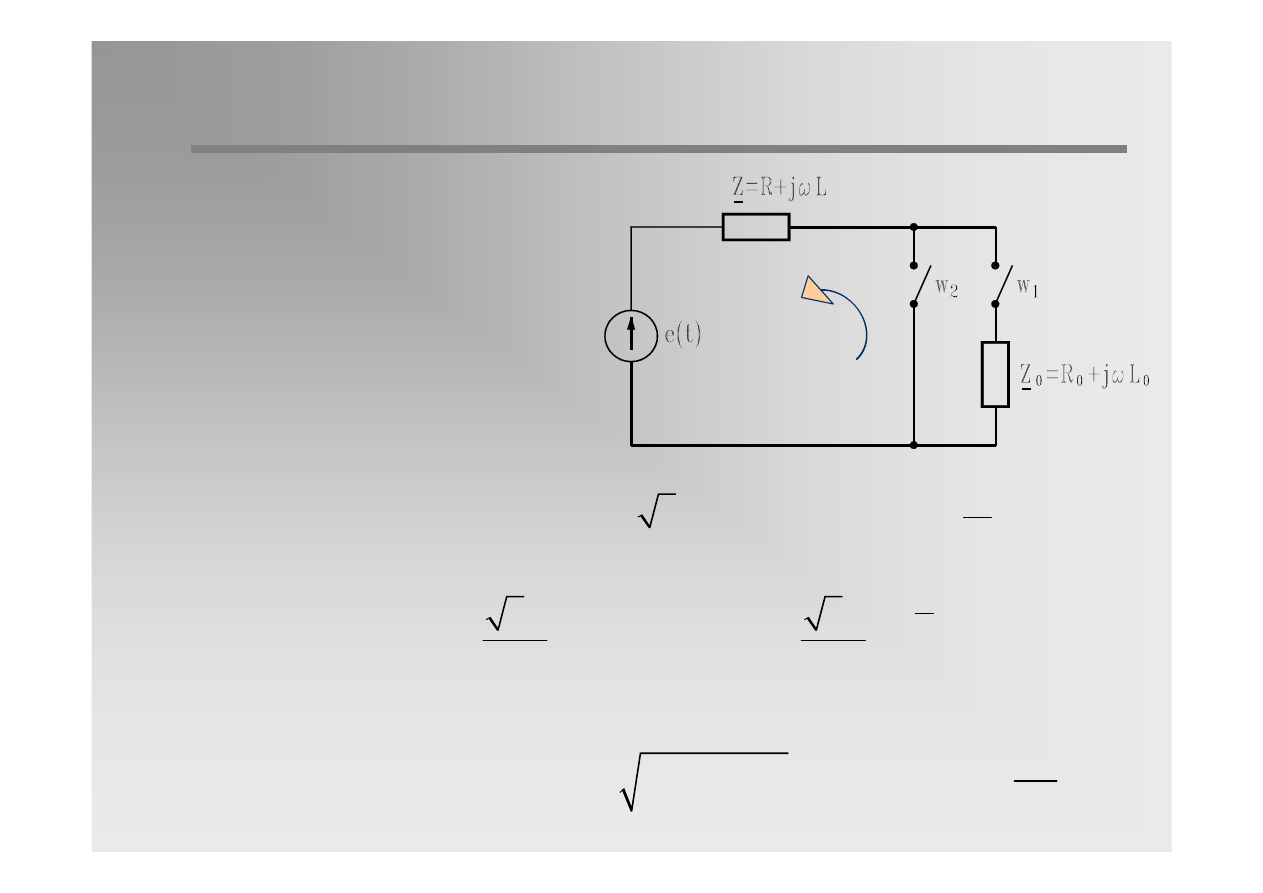
Case 1: W1 open –
short-circuit from unloaded
state
Initial condition:
−
=
=
i(t 0 ) 0
E – voltage
R – resistance
L – inductance
Z – impedance
γ
0
– initial voltage phase angle
ϕ
z
– short-circuit impedance angle
15 /36
( )
( )
( )
ok
nok
i t
i
t
i
t
=
+
( )
(
)
(
)
=
ω + γ − ϕ =
ω + γ − ϕ
ok
0
z
ok
0
z
2 E
i
t
sin t
2I sin t
Z
( )
(
)
−
−
−
= −
⋅
⋅
γ − ϕ =
⋅
=
⋅
a
t
R
R
t
t
T
L
L
nok
0
z
nokm
nokm
2 E
i
t
e
sin
i
e
i
e
Z
For the moment t=0:
i
ok
(0)= - i
nok
(0)
Short
Short
-
-
circuit current time course
circuit current time course
−
= = =
i(0) 0 i(t 0 )
-1,5
-1
-0,5
0
0,5
1
1,5
2
i
ok
i
onk
i
ok
– periodic component of short-circuit current
i
nok
– aperiodic component of short-circuit current
The principle of current continuity in RL circuit
16 /36
Short
Short
-
-
circuit current time course
circuit current time course
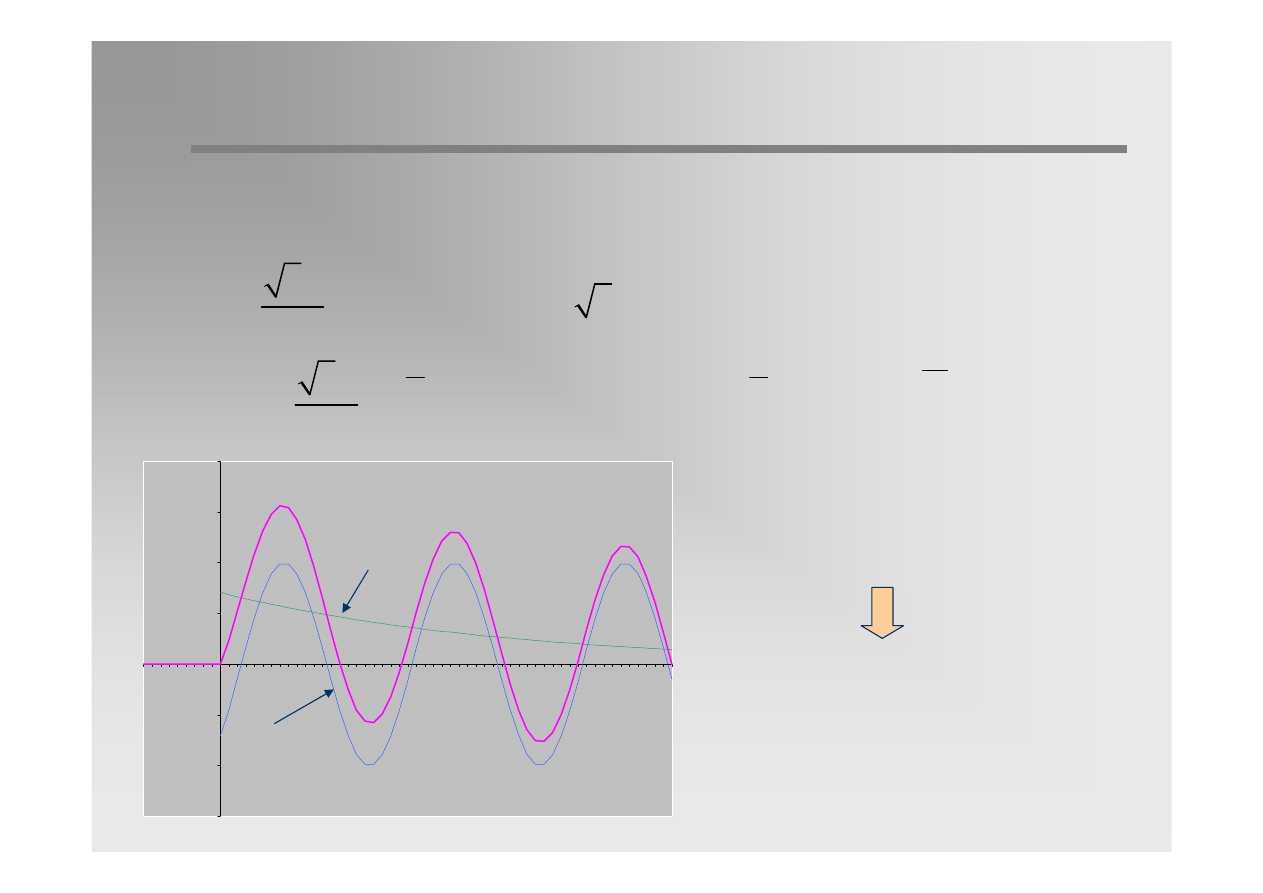
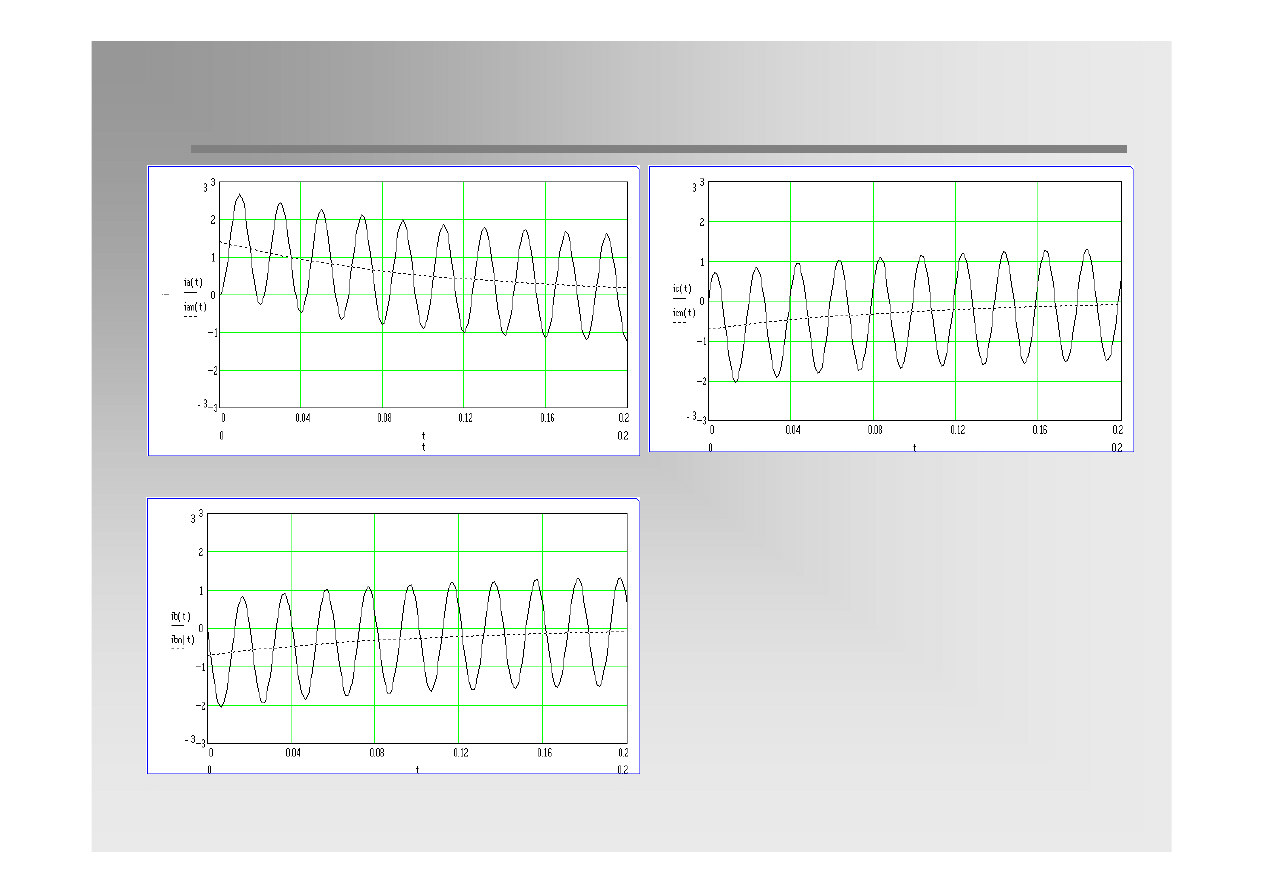
Short-circuit current time course in three
phases of the three-phase system,
when
ɣ
0
=0, φ
z
=90°
Phase R
Phase T
Phase S
17 /36
( )
(
)
ob
0
ob
ob
2 E
i
t
sin t
Z
=
⋅
ω + γ − ϕ
(
) (
)
=
+
+
+
2
2
ob
o
o
Z
R R
X X
+
ϕ =
+
o
ob
o
X X
arctg
R R
( )
( )
( )
( )
−
=
=
=
=
+
ob
ok
nok
i 0
i(0 ) i
0
i
0
i
0
( )
( )
( )
=
=
= −⎡
−
⎤
⎣
⎦
nok
nokm
ok
ob
i
0
i
i
0
i
0
-1,5
-1
-0,5
0
0,5
1
1,5
2
Short
Short
-
-
circuit current time course
circuit current time course
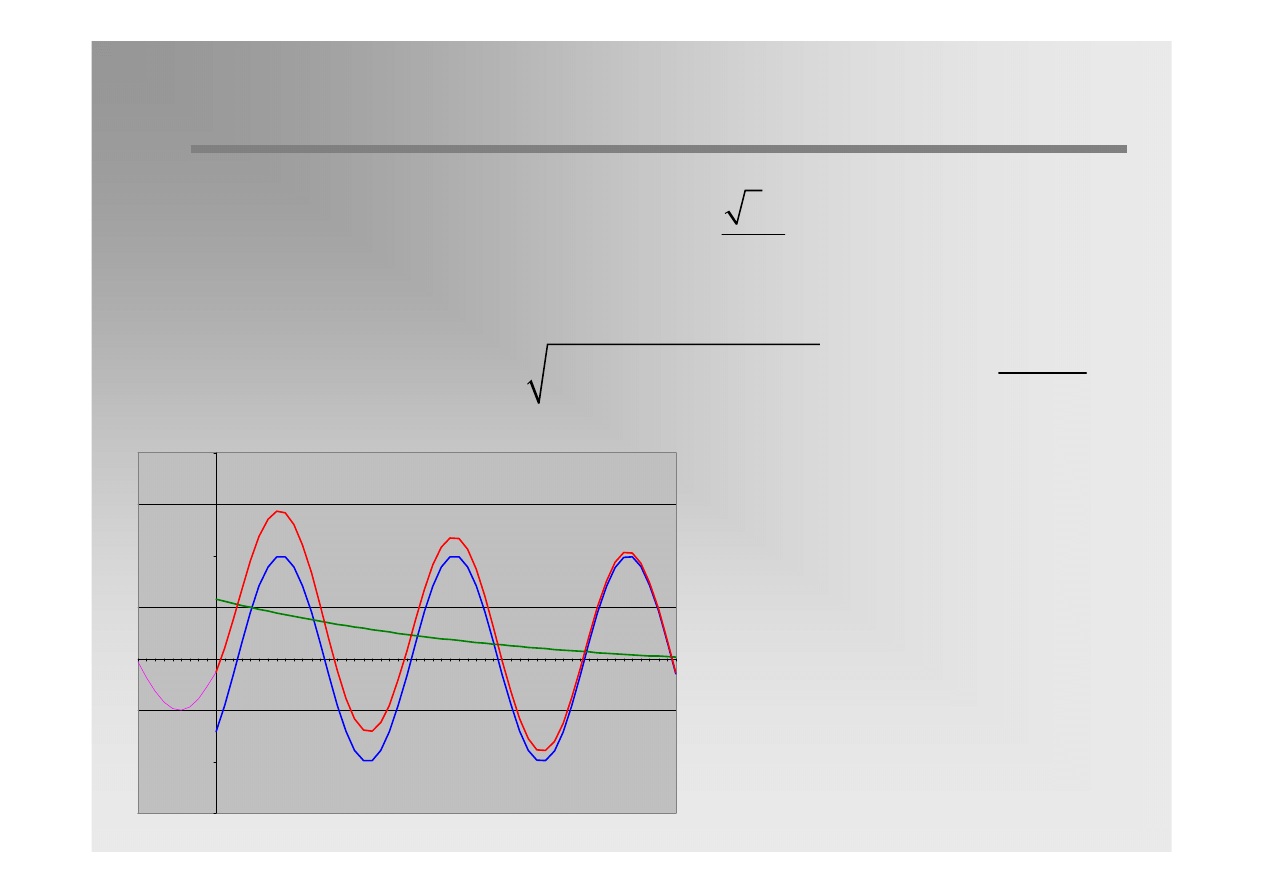
Case 2: W1 closed –
short-circuit from loaded state
Initial condition:
−
=
=
ob
i(t 0 ) i
18 /36
Voltage time course during short
Voltage time course during short
-
-
circuit
circuit
(
)
(
)
−
⎤
⎡
=
ω + γ − ϕ + ϕ −
γ − ϕ
⎥
⎣
⎥⎦
a
t
T
P
P
0
z
b
0
z
u
2 U
sin
t
K sin
e
i
t=0
U
P
P
a
a
a
L
j
R
Z
ω
+
=
b
b
b
L
j
R
Z
ω
+
=
(
)
o
t
sin
E
2
e
γ
+
ω
=
T
a
– time constant
Voltage at the P point:
(
) (
)
+
=
+
+
+
2
2
b
b
P
2
2
a
b
a
b
R
X
U
E
R
R
X
X
+
ϕ =
+
a
b
z
a
b
X
X
arc tan
R
R
ϕ =
b
b
b
X
arc tan
R
19 /36
Voltage time course during short
Voltage time course during short
-
-
circuit
circuit
+
=
+
a
b
a
a
b
L
L
T
R
R
⎛
⎞
+
=
−
⎜
⎟
+
⎝
⎠
+
b
a
b
b
2
2
b
a
b
b
b
R
R
R
L
K
L
L
L
R
X
0
0.01
0.02
0.03
0.04
0.05
0.06
0.07
0.08
0.09
0.1
2
1
0
1
2
When R
a
/L
a
=R
b
/L
b
, K=0, and the voltage time course does not include an
aperiodic component. In practice, the K coefficient has a small value, and the
aperiodic component is omitted.
γ
0
= 90°
γ
0
= 0°
Coefficient K:
20 /36
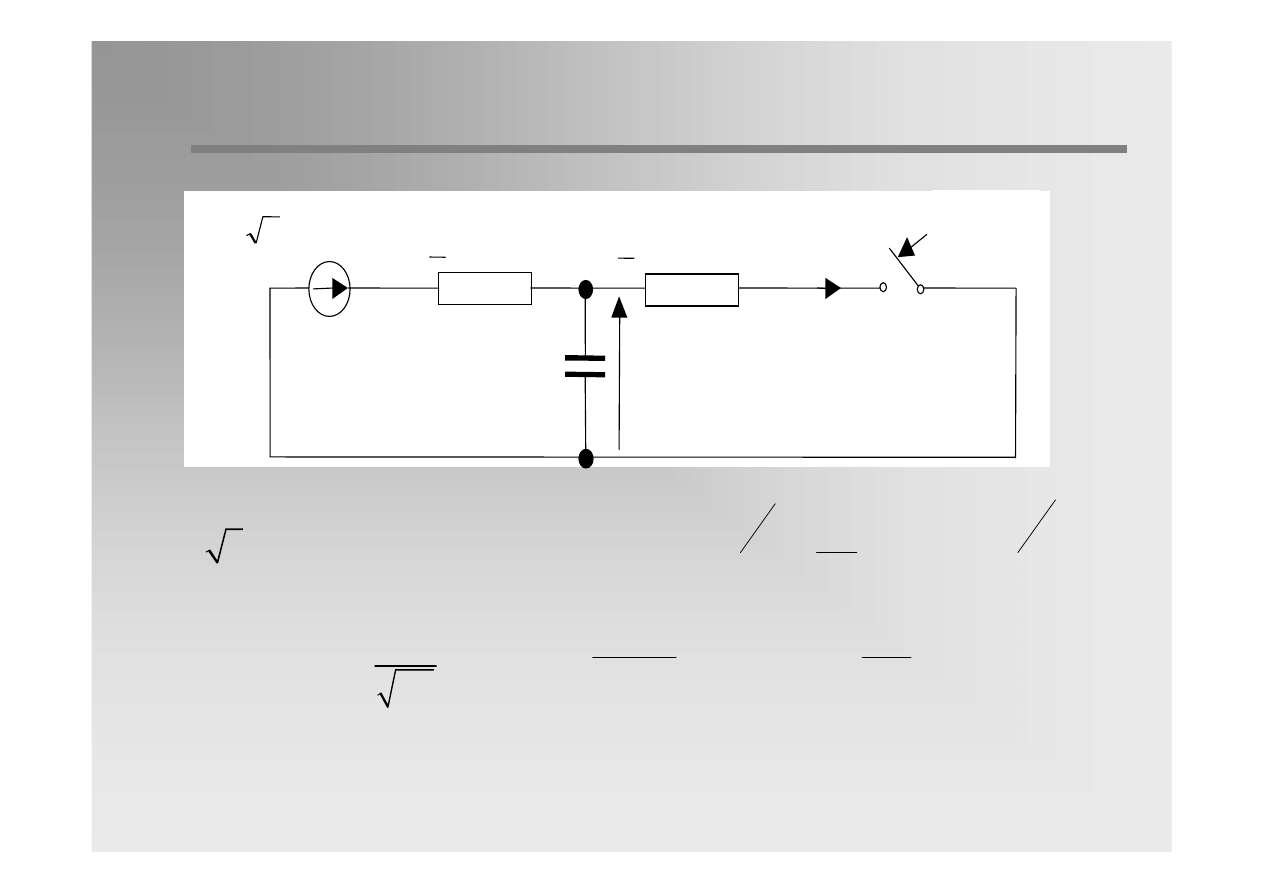
Taking into account the network capacitance
Taking into account the network capacitance
(
)
(
)
( )
−
−
⎡
⎤
ω
⎢
⎥
=
ω + γ − ϕ −
γ − ϕ
−
ω
ω
⎢
⎥
⎣
⎦
p
a
t
t
T
T
ok
o
z
o
z
p
p
i
2I
sin
t
sin
e
sin
t e
ω = π =
p
p
1
2 f
L C
≅
+
a b
a
b
L L
L
L
L
=
b
P
b
2 L
T
R
f
p
– the frequency of the circuit self-oscillations is from couple of hundred Hz to
couple kHz, the efficient value of that component does not go over 20% I
ok
.
i
t=0
C
u
)
t
Esin(
2
e
0
γ
+
ω
=
a
a
a
L
j
R
Z
ω
+
=
b
b
b
L
j
R
Z
ω
+
=
21 /36
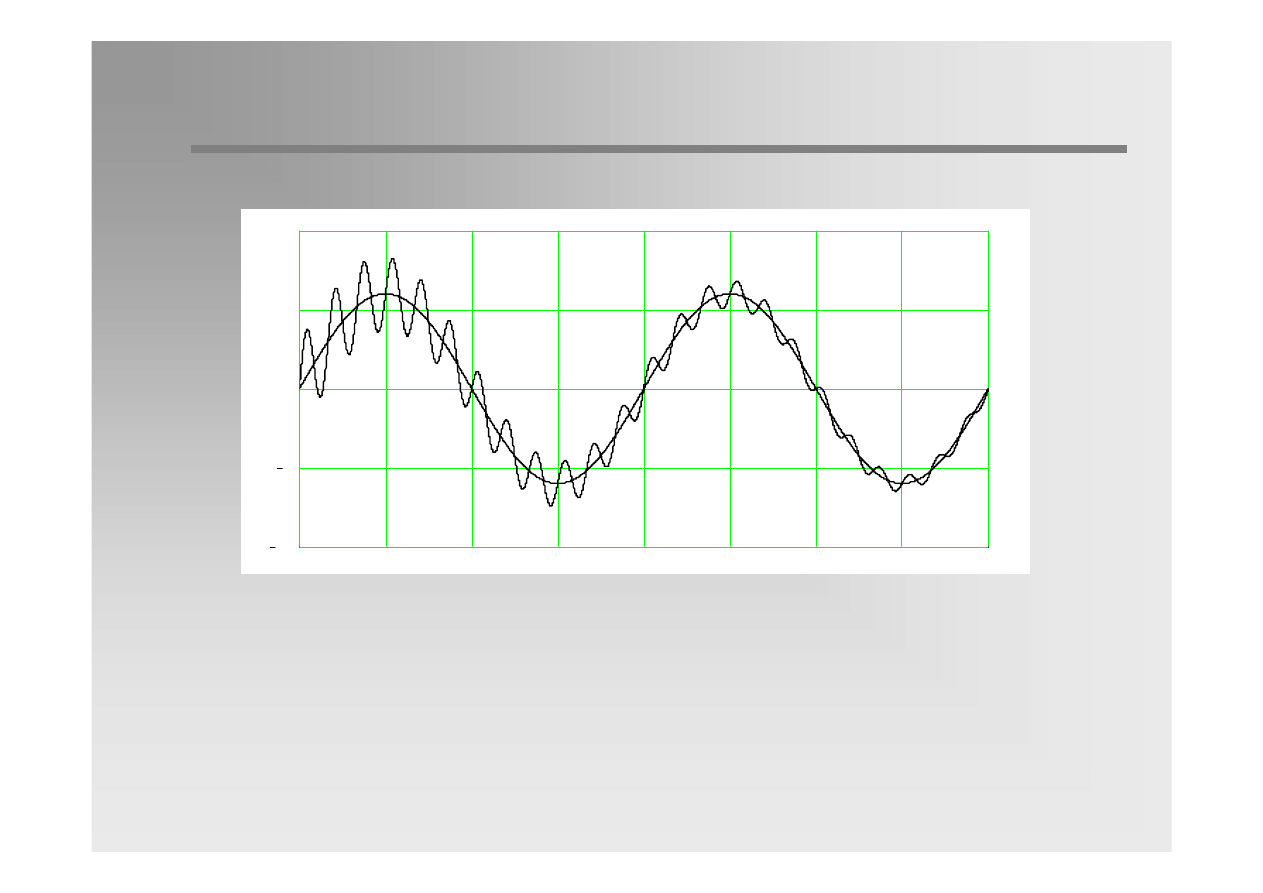
Taking into account the network capacitance
Taking into account the network capacitance
0
0.005
0.01
0.015
0.02
0.025
0.03
0.035
0.04
10
5
0
5
10
The short-circuit current time course for the short-circuit with voltage
phase angle of 90°.
22 /36
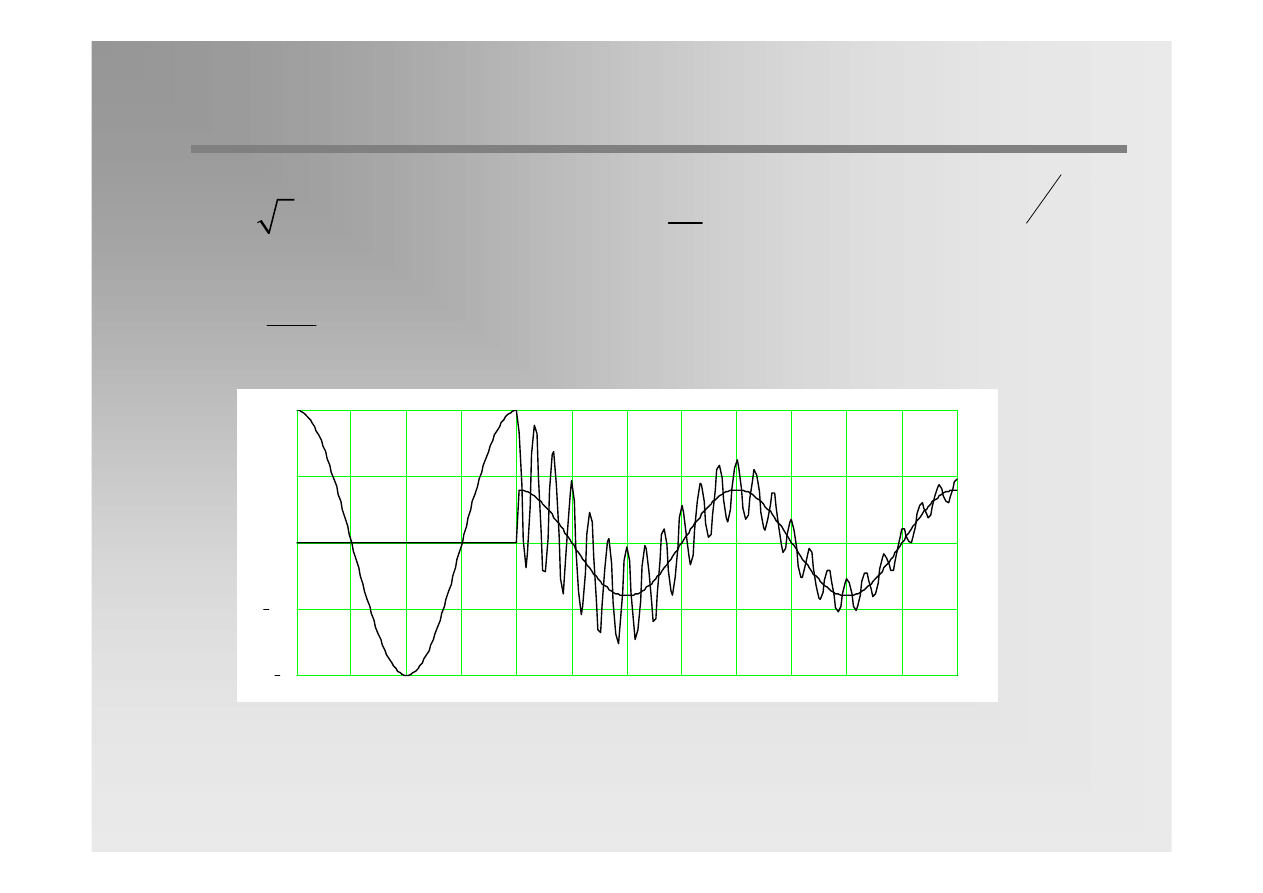
Voltage time course during short
Voltage time course during short
-
-
circuit
circuit
(
)
(
) (
)
−
⎤
⎡
=
ω + γ − ϕ + ϕ +
γ − ϕ
ω
⎥
⎣
⎥⎦
P
t
T
a
P
P
0
z
b
0
z
P
b
L
u
2 U
sin
t
sin
sin
t e
L
=
b
P
b
2 L
T
R
0
0.005
0.01
0.015
0.02
0.025
0.03
0.035
0.04
0.045
0.05
0.055
0.06
1
0.5
0
0.5
1
The voltage time course during a short-circuit with the initial short-circuit angle of 90°.
23 /36
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
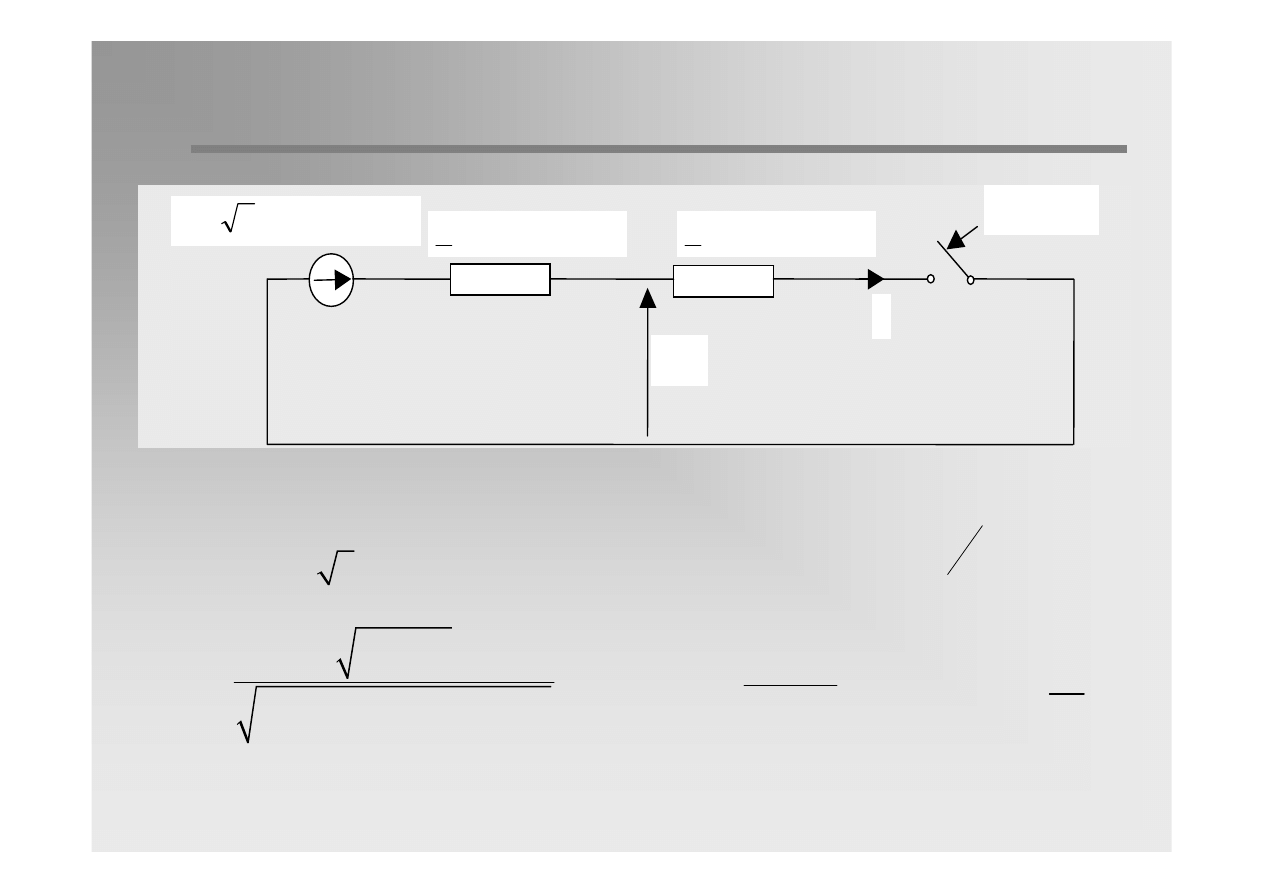
g
U
E
d
X
d
g
I
=
+
d
r
ad
X
X
X
=
+
d
d
g
g
E
U
j X I
=
d
ud
d
E
I
X
Generator is the source of short circuit current.
The equivalent circuit
diagram of the
generator in steady-
state
X
r
– leakage reactance of
stator windings
X
ad
– mutual reactance of
stator and rotor
I
ud
– steady-state component of short-circuit current
d-axis synchronous reactance
24 /36
Consider the sequence of events associated with a three-phase short-
circuit on the unloaded generator. Before the fault occurs, the field
produces an air gap flux entering the armature and produces time-varying
flux linkages for all mutually coupled circuits consisting of three phase
windings (a, b, c), a field winding (F) and two damper windings (D, Q).
A the instant t=0 the fault is applied. This forces flux linkages to change. By
the principle of constant flux linkages the step change is not possible and
all flux linkages must remained fixed at least for an instant.
(According to magnetic inertia principle the step change of flux linkages
would mean a step change of energy accumulated in the magnetic field of
the winding.
To counteract sudden flux changes transient dc fluxes are induced in each
winding which maintain flux linkages constant. These transient fluxes decay
to zero with time constants depending on the resistance and inductance of
each circuit.
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
25 /36
The existence of additional fluxes in the generator circuits changes its
magnetic state and an equivalent reactance which represents the
generator at this state.
At the first moment of the fault transient fluxes appear in all generator
windings; this state is called subtransient and the generator is
represented by so called subtransient reactance X”
d
. After the
decaying of transient dc flux in rotor damper windings (time period of
(
0,01-0,05
s) the generator passes on to the transient state and is
represented by transient reactance. Then, when the flux at the field
winding decays (
0,6-1)
s the generator reaches steady state and the
representing reactance is X
d
.
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
26 /36
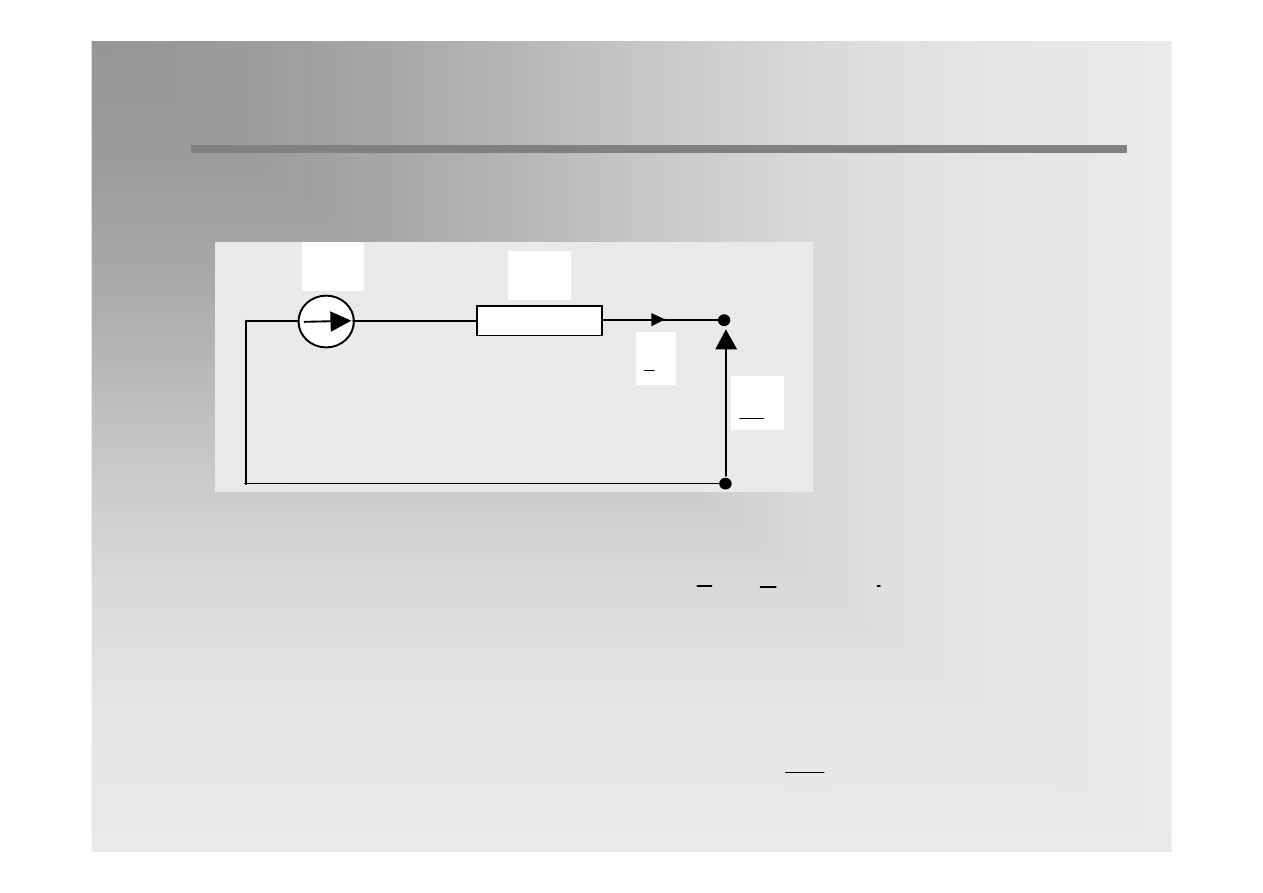
In short-circuit calculations
we will analyze the currents at the initial
moment of the short-circuit. This is a subtransient state for
generator, so it can be represented by the reactance X
d
”
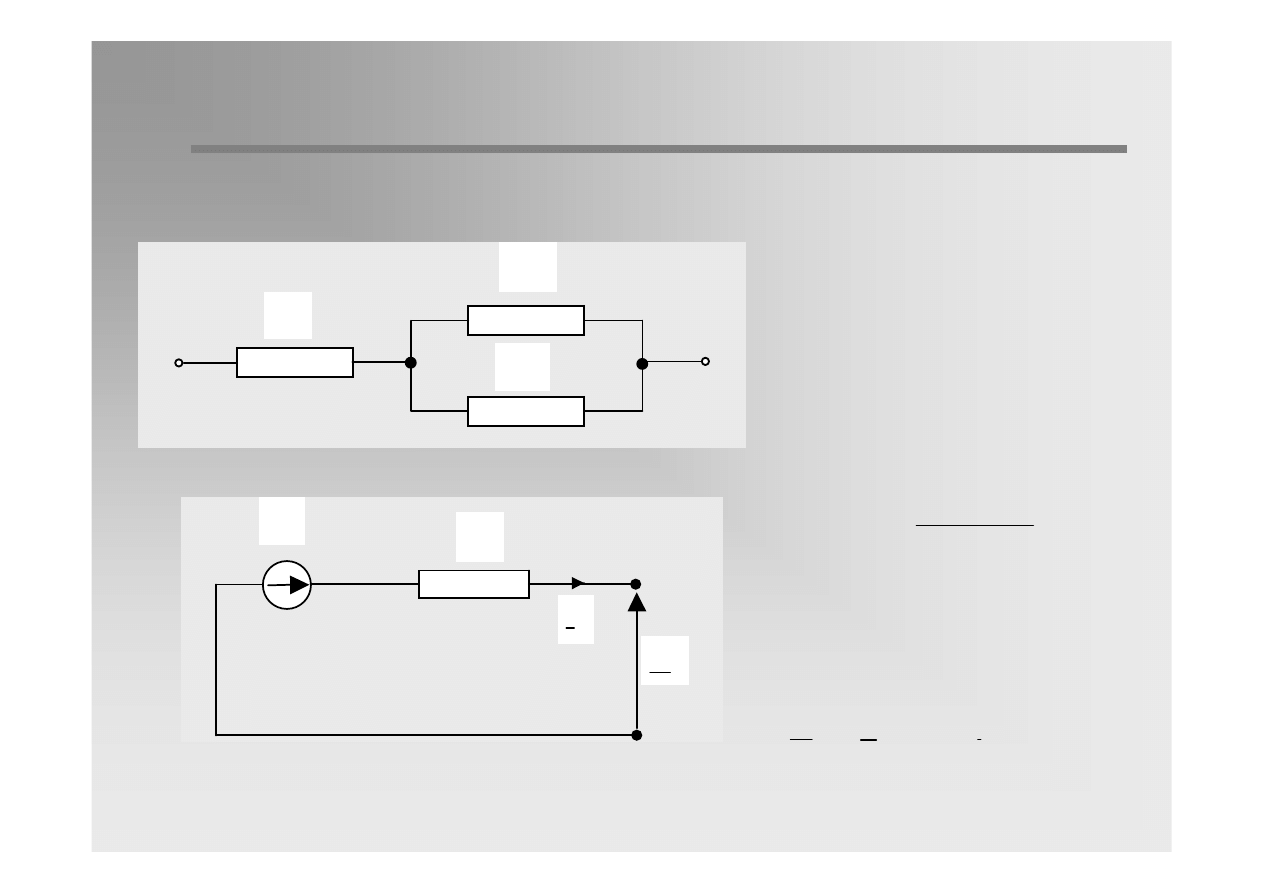
Generator equivalent circuit diagram
Generator equivalent circuit diagram
"
2
d%
1n
g
n
X
U
X
100 S
=
⋅
U
n
– rated generator voltage [kV]
S
n
- rated generator power [MVA ]
[
Ω ]
27 /36
r
X
ad
X
rf
X
′ =
+
+
rf
ad
d
r
rf
ad
X X
X
X
X
X
g
U
d
E′
g
I
'
d
X
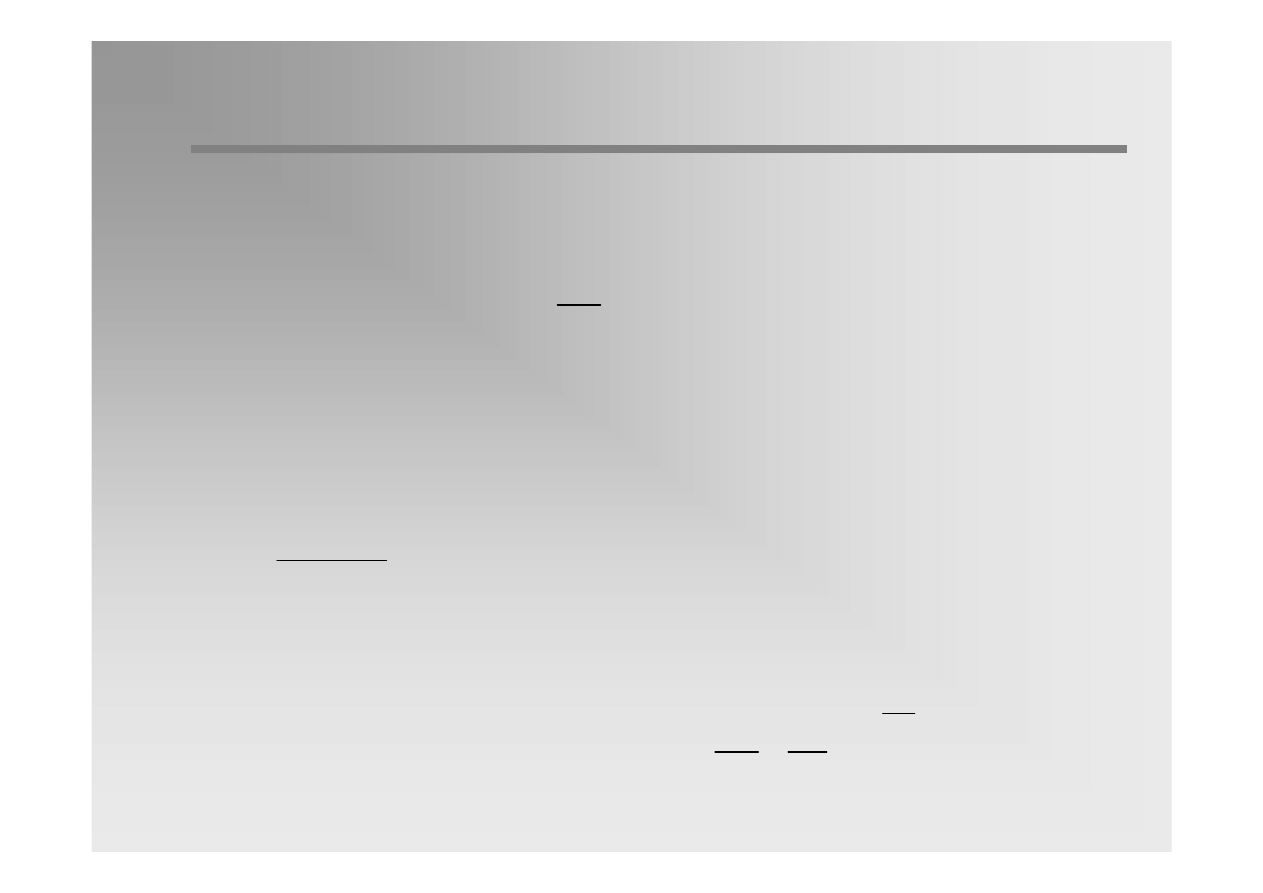
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
Transient state
X
rf
– leakage reactance of the
field winding
X
r
– leakage reactance of the
armature winding
′
′
=
+
d
d
g
g
E
U
j X I
Transient electromotive force
(EMF)
28 /36
'
d
d
d0
d
X
T
T
X
′
′
=
'
d
Z
d
d0
d
Z
X
X
T
T
X
X
+
′
′
=
+
−
⎛
⎞
Δ =
−
⎜
⎟
⎜
⎟
⎝
⎠
'
d
t
'
T
'
d
d
d
'
d
d
E
E
I
e
X
X
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
An additional transient flux in the magnetizing winding decays with the
time-constant T’
d
.
T’
d0
– time-constant with the
armature circuits open
(5-12) s
Typically, T’
d
is about ¼ that of T’
d0.
If the short-circuit occurs behind an outer reactance:
An additional direct flux in the field winding
causes a positive sequence additional
current component in the armature
29 /36
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
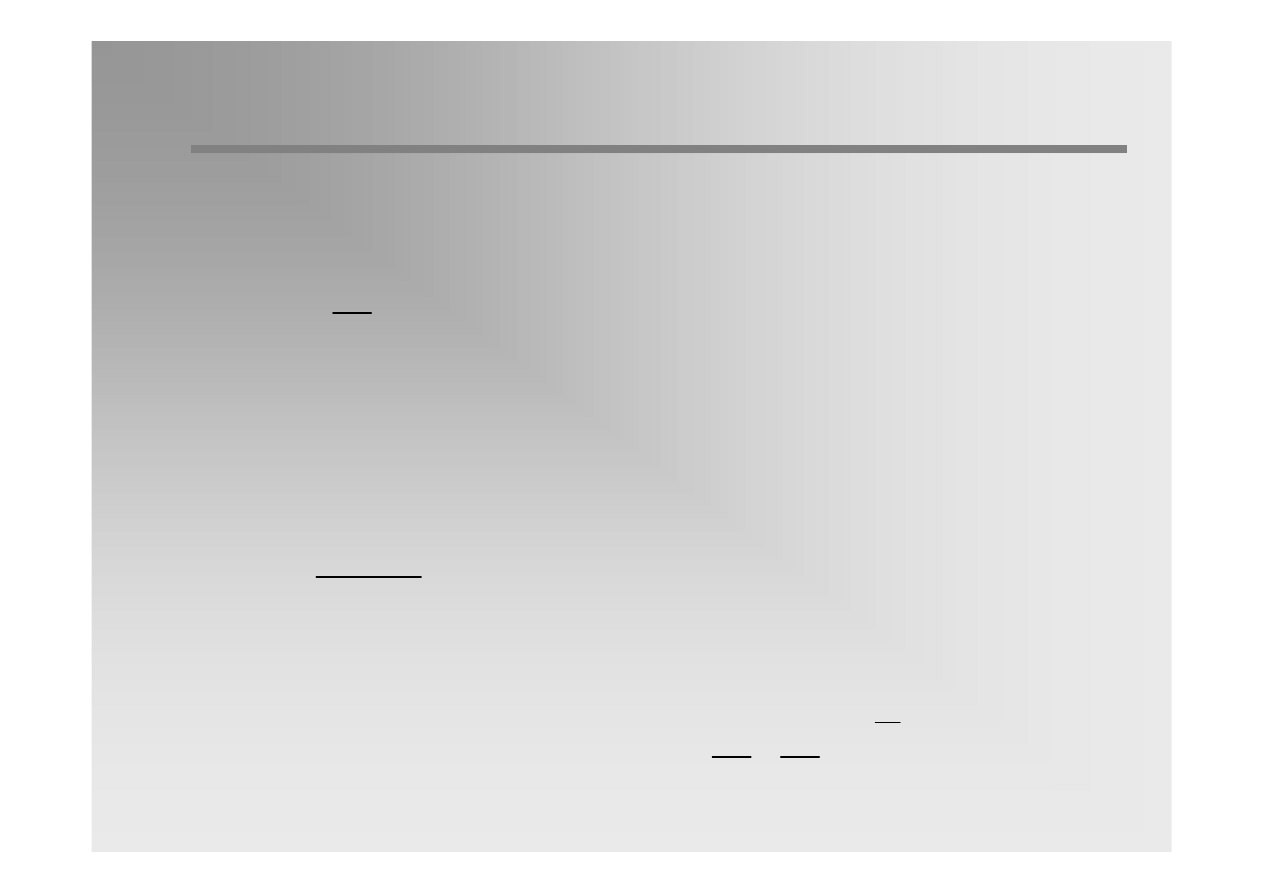
Subtransient state
r
X
rf
X
rD
X
ad
X
′′ =
+
+
+
d
r
rD
rf
ad
1
X
X
1
1
1
X
X
X
X
rD
– leakage reactance
of the rotor dumper
winding
g
U
d
E ′′
g
I
d
X ′′
d q
d
q
E
U
j X I
″
′′
=
+
Subtransient electromotive
force (EMF)
30 /36
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
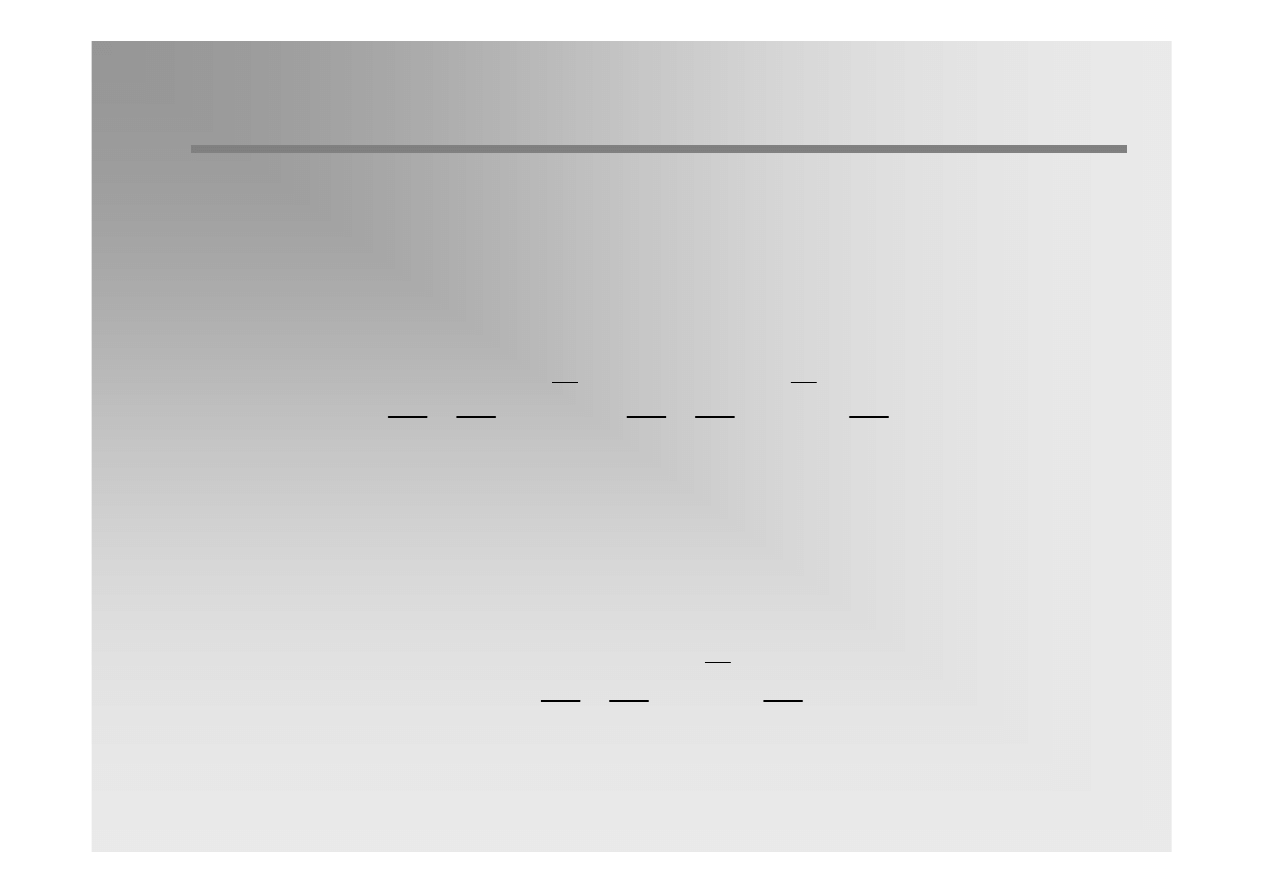
An additional direct flux in the damper winding decays with time-
constant T”
d
.
d
d
d0
d
X
T
T
X
′′
′′
′′
=
′
′′ +
′′
′′
=
′ +
d
Z
d
d0
d
Z
X
X
T
T
X
X
If the short-circuit occurs behind an outer reactance:
T”
d0
– time-constant with the armature
circuits open (0,02-0,2) s
−
′′
⎛
⎞
′′
′
′′
Δ =
−
⎜
⎟
′′
′
⎝
⎠
d
t
T
d
d
d
d
d
E
E
I
e
X
X
An additional direct flux in the damper winding
causes a positive sequence additional current
component in the armature
Typical value of T”
d
is 2 cycles.
31 /36
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
Alternating short-circuit current in d-axis is the sum of steady and
transient components:
′′
′
= Δ + Δ +
okd
d
d
ud
I
I
I
I
−
−
′′
′
⎛
⎞
⎛
⎞
′′
′
′
=
−
+
−
+
⎜
⎟
⎜
⎟
′′
′
′
⎝
⎠
⎝
⎠
d
d
t
t
T
T
d
d
d
d
d
okd
d
d
d
d
d
E
E
E
E
E
I
e
e
X
X
X
X
X
−
′′
⎛
⎞
′′
=
−
+
⎜
⎟
⎜
⎟
′′
⎝
⎠
q
t
T
q
q
q
okq
q
q
q
E
E
E
I
e
X
X
X
Alternating short-circuit current in g-axis does not include the field
component (no field winding in the q-axis)
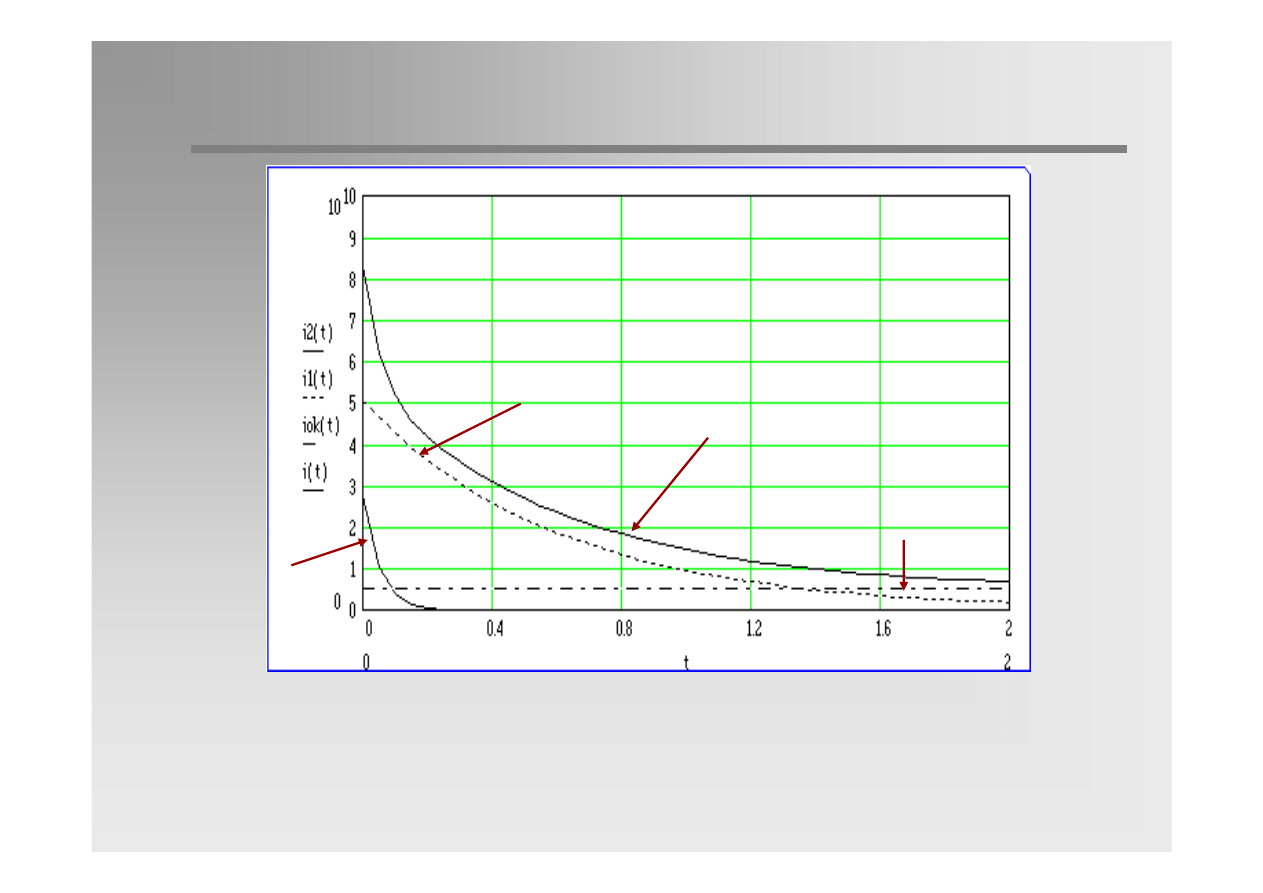
32 /36
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
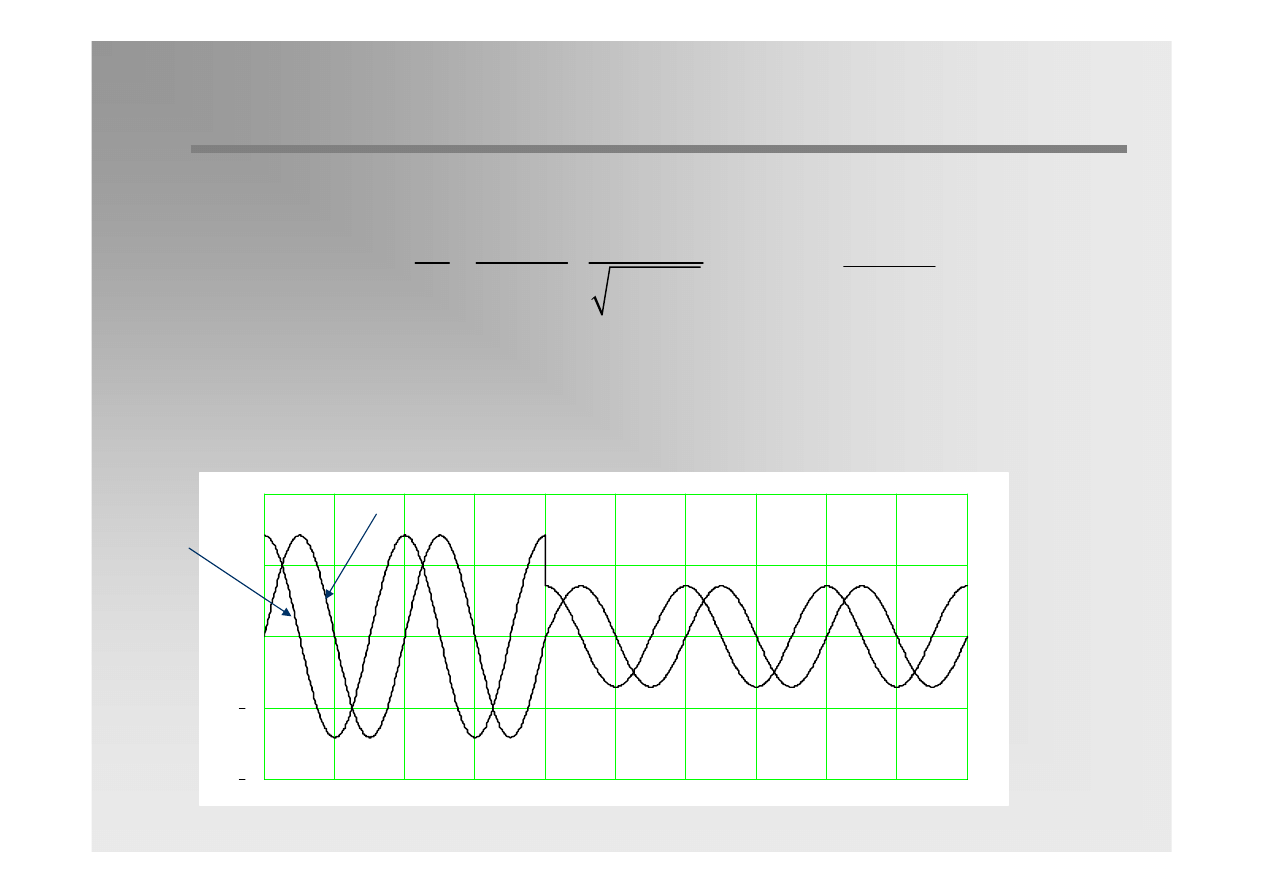
The time course of rms values of short-circuit ac current components.
Short-circuit at the terminals of unloaded generator.
Subtransient
component
Transient
component
AC current
Steady state
component
33 /36
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
The time course of rms values of short-circuit ac current components.
Short-circuit at the terminals of generator rating loaded.
Subtransient
component
Transient
component
AC current
Steady state
component
34 /36
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
Alternating-current component of short-circuit current for t=0 is called the initial current:
(
)
(
)
(
)
⎛
⎞
′′
⎛
⎞
′′
⎡
⎤
=
=
= ⎡
=
⎤ +
=
=
+ ⎜
⎟
⎜
⎟
⎣
⎦
⎣
⎦
⎜
⎟
′′
′′
⎝
⎠
⎝
⎠
2
2
2
2
q
d
p
ok
okd
okq
d
q
E
E
I
I
t 0s
I
t 0s
I
t 0s
X
X
The forward wave moves at twice synchronous speed with
respect to the armature and induces a second harmonic
current in the armature circuit; amplitude (5-10)% I
n
.
ω
+
ω
-
ω
In the armature windings an aperiodic components appear as the result of direct
transient flux occurrence (by the principle of constant stator flux linkage). It decays with
the time-constant T
a
= (0,3-5) s. It induces additional ac currents in the field winding,
decaying to zero with time-constant T
a
. Such an ac current produces a pulsating flux
which is stationary with respect to waves, one is going forward and one backward. The
backward is such as to oppose the stationary armature field.
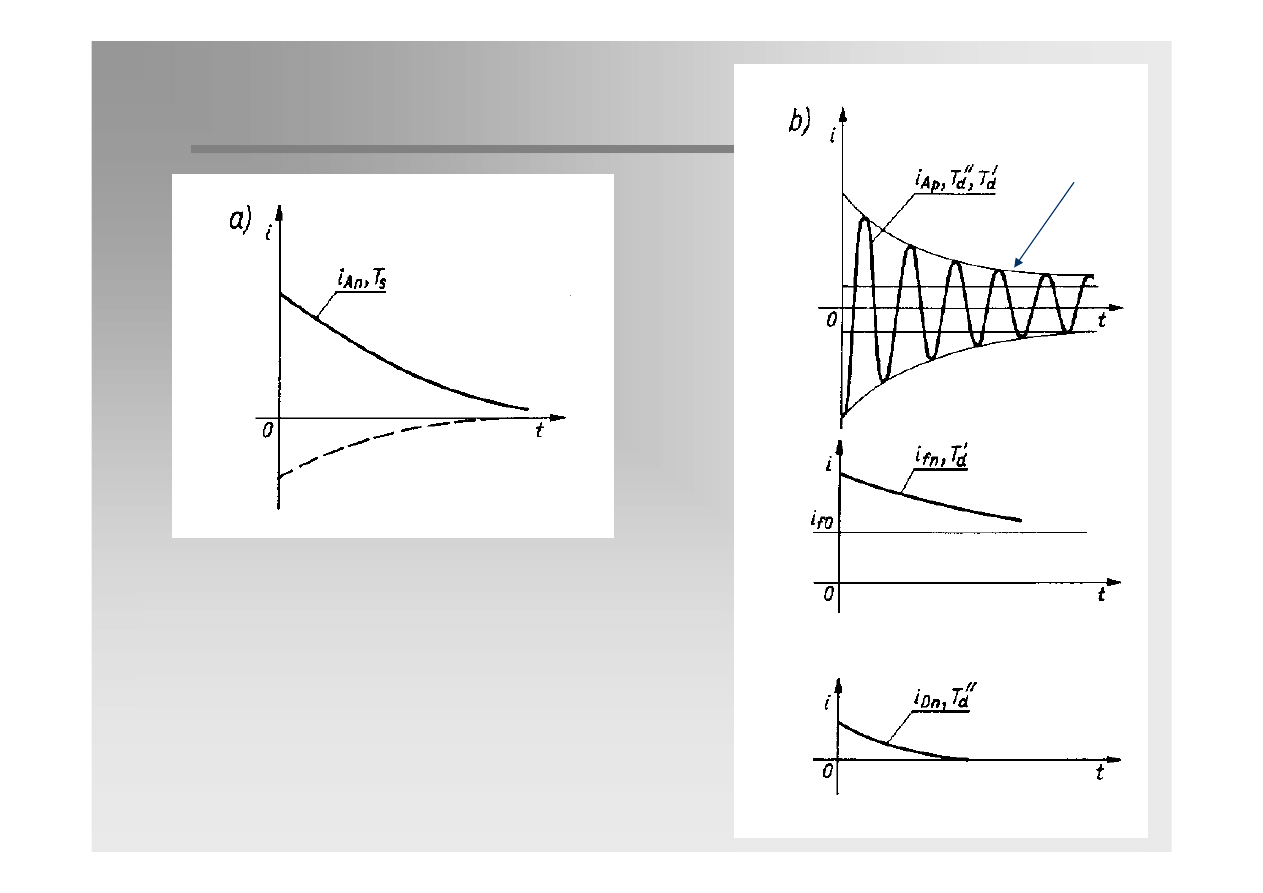
35 /36
a) Armature dc current component
b) Armature ac current component and
transient dc currents in rotor windings
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit
Transient
current in the
field winding
Transient
current in
damper
winding
AC component
envelope
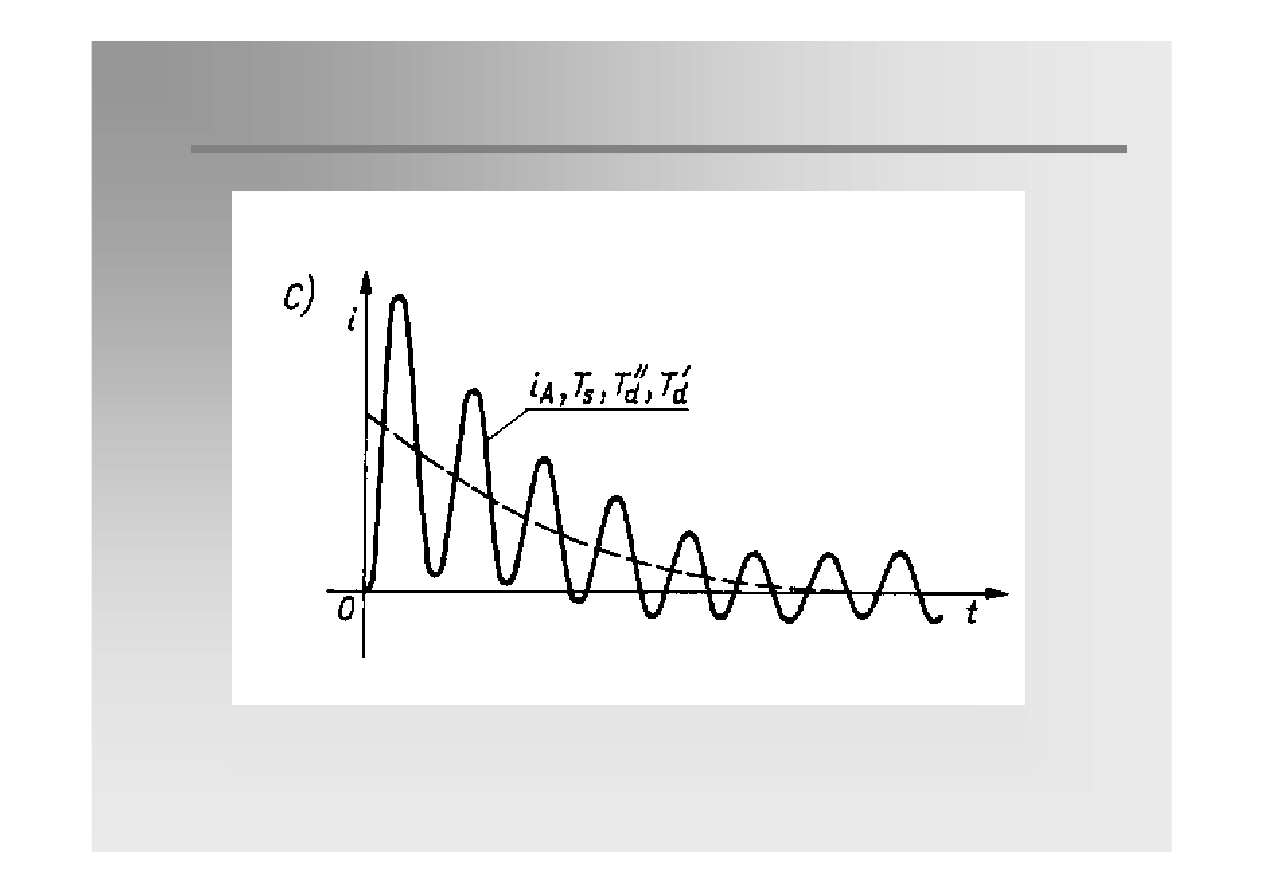
36 /36
The time course of the total stator current
Near
Near
-
-
to
to
-
-
generator short circuit
generator short circuit