Measurement of flow rate, friction
Factor, and velocity Profile in Pipe
Flow

57:020 mechanics of Fluids and Transfer

Processes

Experimental Laboratory #2

Purpose

Measure

Flow rate in a pipe (smooth)
Friction factor
Velocity profile
Specify the turbulent-flow Reynolds Number
Compare the results with benchmark data
Uncertainty analysis for:



Friction factor



Velocity profile

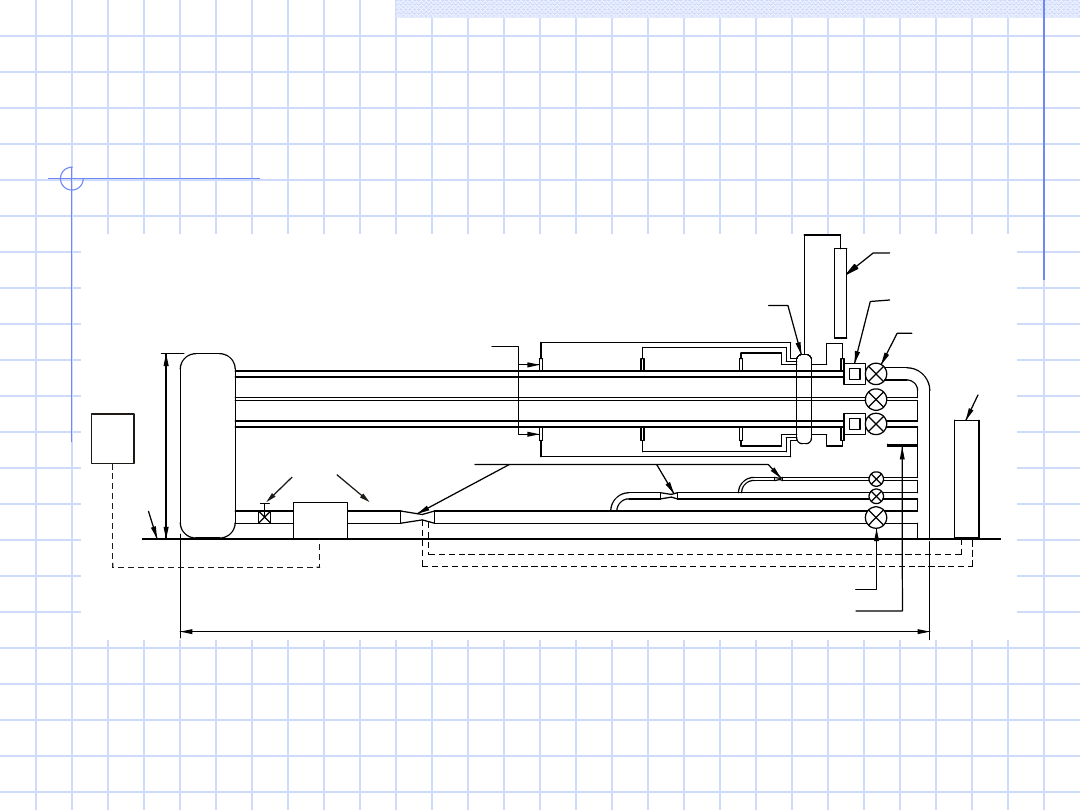
Test Design

The facility consists of:

Closed pipe network
Fan
Reservoir

Instruments used:

3 Venturi meters

Simple water Manometer
Differential Water manometer
Pitot Probe
Digital Micrometer

(Accurate radial positioning)

Contraction Diameters
(mm):

12.7

25.4

52.9
3

Flow Coefficient, K

0.915

0.93
7

0.93
5

Air Flow Pipe facility

P r e s s u r e

ta p s

M o to r

c o n tr o lle r

F lo o r

6’

-6

”

R

es

er

vo

ir

2 . 0 ” s m o o th

0 . 5 ” s m o o th

2 . 0 ” r o u g h

R e lie f

v a lv e s

B lo w e r

D = 2 . 0 ”

D = 1 . 0 ”

D = 0 . 5 ”

t

t

t

3 6 ’

V e n tu r i m e te r g a te v a lv e s

T h e r m o m e te r

1

2

3

4

V a lv e m a n if o ld

S im p le

m a n o m e te r

P ito t tu b e

h o u s in g s

V a lv e s

D iff e r e n tia l

m a n o m e te r

V e n tu r i m e te r s

Test Design

(Continue)

Reservoir:

To build up pressure and force the air

to flow downstream through any of the
three straight experiment pipes.

Digital Micrometer:

Allow the measurement of the position

of the Pitot probe at different locations
along the cross section of the pipe tested

Pitot Probe:

Located in the glass-wall box
Used to measure the Stagnation

pressure and calculate the velocity
profile in pipe

Venturi meters:

Located on each pipe type
Used to measure flow rate Q along the

differential water manometer

Pressure Taps:

Located along each pipe, they are

connected to the simple water
manometer to evaluate the head
measurement

They are used to calculate the friction

factor

Manometers:

To measure the head at each pressure

Tap along the pipe and to make the Pitot-
tube measurements (simple Manometer)

To measure head drops across the

venturi meters (differential Manometer)

Pressure tap manifold and Pitot-tube
housing

Pressure tap manifold

Pitot-tube housing

Measurement Systems

:

The equipment used in the experiment includes:

Digital thermometer with a range of – 40 to 450 F and a

smallest reading of 0.1 F for measurement of the

environment temperature.
Digital micrometer with least significant digit 0.01 mm for
positioning the Pitot-tube inside the pipe.
Simple water manometer with a range of 2.5 ft and a least
scale division of 0.001 ft for measurement of the head at each
pressure tap along the pipes and for measurement of
velocities using the Pitot-tube arrangement .
Differential water manometer with a range 3 ft and a least
scale division of 0.001ft for measurement of the head drop
across the Venturi meters.

Measurement Systems

(continue)

For the flow rate and friction
factor, the individual
measurement are performed
for:



Ambient air temperature (A.3)



Pipe air temperature (A.5)



Pipe pressure head



Venturi meter pressure head drop

The experimental Results
are:



Manometer water density



Air density



Kinematic viscosity



Flow rate



Reynolds number



Friction factor

Data reduction equations
are:

)

(

o

w

w

T

f





)

(

o

air

air

T

f





)

(

o

air

air

T

f





air

w

DM

t

Z

g

KA

Q









2

air

e

D

Q

R





4







j

i

SM

SM

air

w

Z

Z

LQ

D

g

f











2

5

2

8

Measurement Systems

(continue)

For the velocity profile, the individual measurement systems
are for:



the ambient temperature



pipe air temperature



pitot stagnation and static pressure heads.

The experimental results are for:



manometer water density (A.3)



Air density (A.5)



Velocity profile (below)

Data reduction equation:

(using the Bernoulli equation along the manometer

equation)





















static

stag

SM

SM

a

w

Z

r

Z

g

r

u

)

(

2

)

(





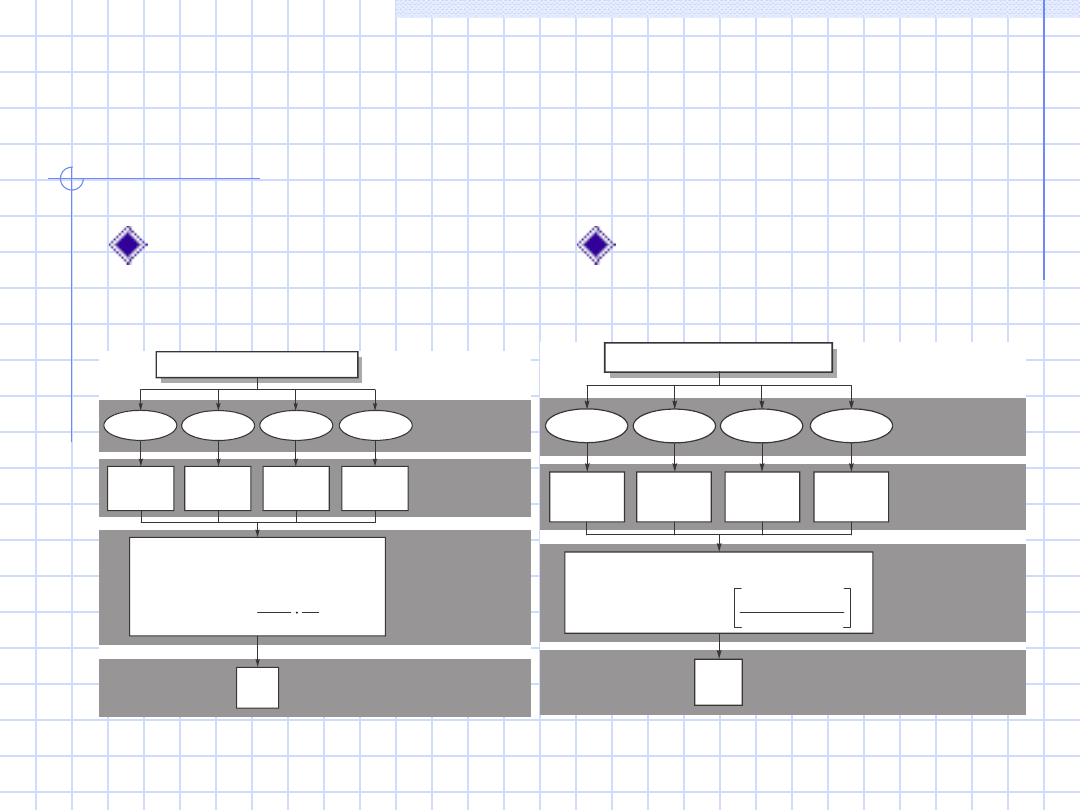
Flow rate, Friction factor and velocity
profile measurement systems

Block diagram of the
experimental determination of
the Friction

Block diagram of the Velocity
measurement

E X P E R IM E N T A L

R E S U L T S

E X P E R IM E N T A L E R R O R S O U R C E S

IN D IV ID U A L

M E A S U R E M E N T

S Y S T E M S

M E A S U R E M E N T

O F IN D IV ID U A L

V A R IA B L E S

D A T A R E D U C T IO N

E Q U A T IO N S

T E M P E R A T U R E

W A T E R

T E M P E R A T U R E

A IR

f

B , P

V E N T U R I

P R E S S U R E

P IP E

P R E S S U R E

f = F ( , , z , Q =





)

a

a

w

g

D

 

8 L Q




Q = F ( z )



w

w

T

T

B

T

, P

z

z

B , P

f

f

S M

S M

w

w

D M

S M

2

2

5

a

T

T

B

T

, P

a

a

z

S M

z

z

B , P

D M

D M

z

D M

= F (T )




( )

w

= F (T )

a

z

S M i

- z

S M j

w

a

E X P E R IM E N T A L

R E S U L T

w

w

T

T

B

T

, P

S T A G N A T IO N

P R E S S U R E

S T A T IC

P R E S S U R E

E X P E R IM E N T A L E R R O R S O U R C E S

IN D IV ID U A L

M E A S U R E M E N T

S Y S T E M S

M E A S U R E M E N T

O F IN D IV ID U A L

V A R IA B L E S

D A T A R E D U C T IO N

E Q U A T IO N S

z

B

, P

S M

B , P

u

u

u

= F ( T )




u = F ( , , z , z )





2 ( )

g

 



½

=

T E M P E R A T U R E

W A T E R

T E M P E R A T U R E

A IR

w

a

s ta g

a

T

T

B

T

, P

a

z

w

w

w

S M

s t a g

z

S M

s t a g

z

B

, P

S M

s ta t

z

S M

s t a t

z

S M

s t a t

= F ( T )

a

a

a

S M

s ta g

S M

s ta t

z

S M

s ta g

- z

S M

s ta t

w

a

Data Acquisition and reduction

The procedures for data acquisition and reduction are described as follow:

1.

Use the appropriate Venturi meter, (2” smooth pipe) measure the head
drop

2.

Take reading for ambient air (manometer water) and pipe air
temperatures.

3.

To obtain velocity data, measure in the appropriate Pitot-tube box, the
ambient head and stagnation heads across the full diameter. Measure
the stagnation heads at radial intervals. The recommended radial
spacing for one half of the diameter is 0, 5, 10, 15, 20, 23, and 24 mm.

4.

Maintaining the discharge, measure the head along the pipe by means
of the simple water manometer connected to the pressure taps located
along the pipe being studied (10 times for uncertainty analysis)

5.

Repeat step 2

6.

Execute data reduction for data analysis and uncertainty analysis using
equation above

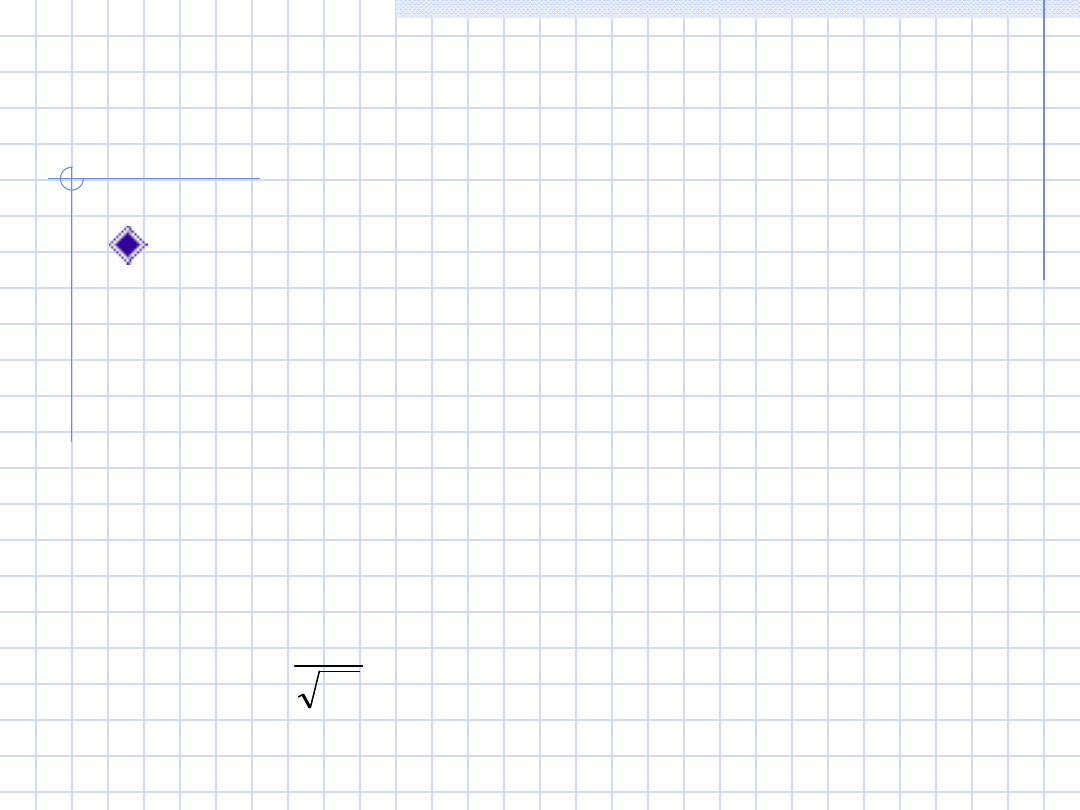
Uncertainty Analysis

The data reduction equation for the friction factor is:

However here we will only consider bias limits for Z

SM i

and Z

SM j .

The total

uncertainty for the friction is:

The Bias Limit, B

f

and the precision limit, P

f

, for the result are given by:

)

,

,

,

,

,

,

,

(

j

i

SM

SM

a

w

Z

Z

Q

L

D

g

F

f







2

2

2

f

f

f

P

B

U





2

2

2

2

1

2

2

2

j

SM

SMj

i

SM

i

SM

Z

Z

Z

Z

j

i

i

i

f

B

B

B

B

















M

tS

P

f

f



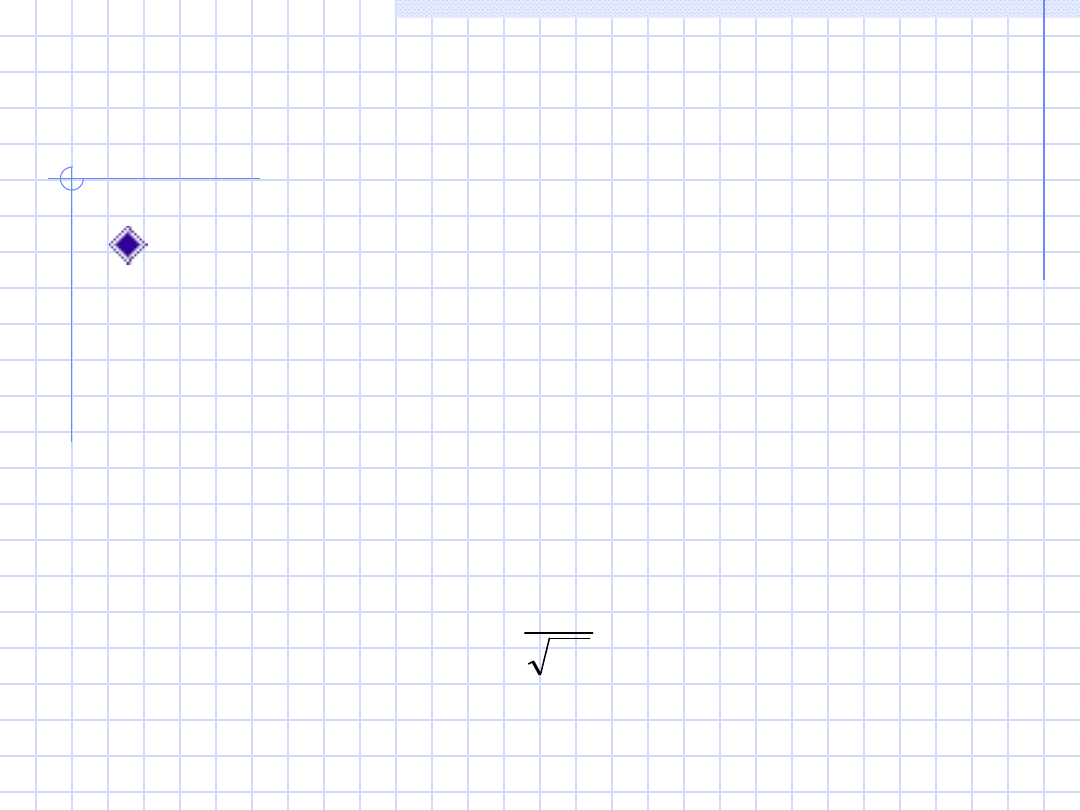
Uncertainty Analysis

(continue)

Data Reduction equation for the velocity profile is as follow:

2

2

2

u

u

u

P

B

U





2

2

2

2

1

2

2

2

stat

SM

stat

SM

SMstagn

stagn

SM

Z

Z

Z

Z

j

i

i

i

u

B

B

B

B

















M

tS

P

u

u



)

,

,

,

,

(

static

stagnation

SM

SM

a

w

Z

Z

g

F

f







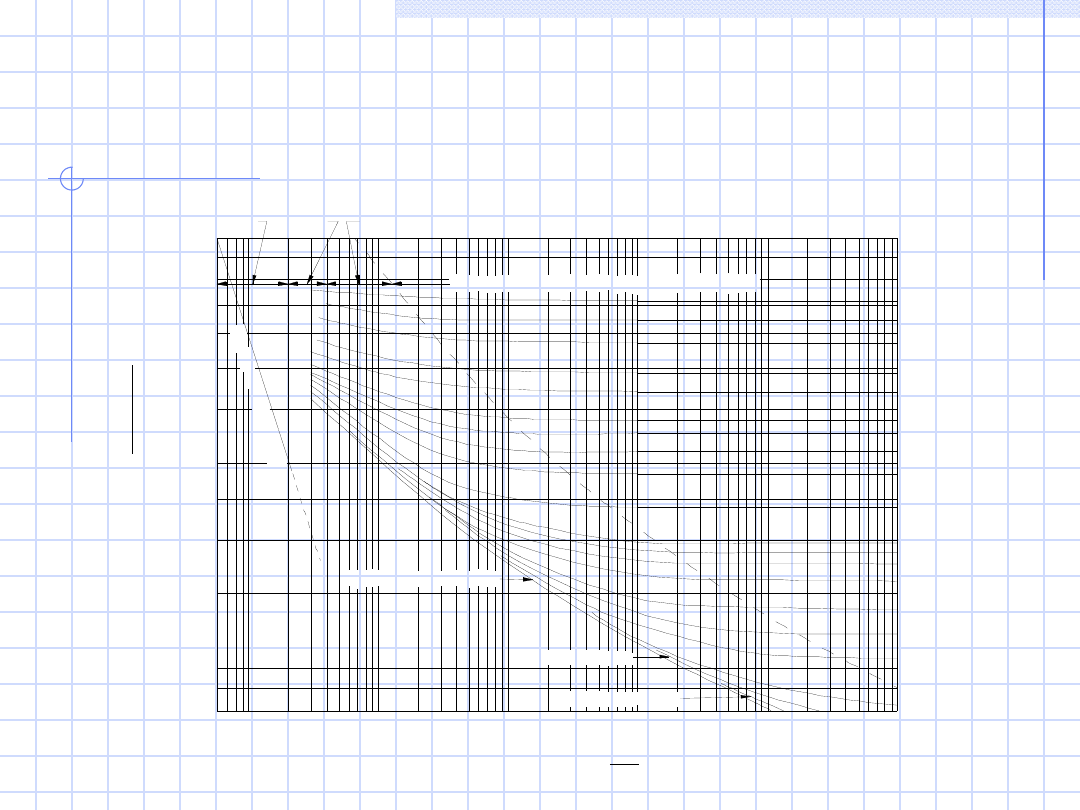
Moody Chart for pipe friction with
smooth and rough walls

1 0

1 0

4

1 0

1 0

1 0

1 0

5

6

7

8

3

0 . 0 0 8

0 . 0 0 9

0 . 0 1 5

0 . 0 2 5

0 . 0 2 0

0 . 0 1 0

0 . 0 3 0

0 . 0 4 0

0 . 0 5 0

0 . 0 6 0

0 . 0 7 0

0 . 0 8 0

0 . 0 9 0

0 . 1 0

R e y n o ld s N u m b e r , R e =

V D

F

ri

ct

io

n

F

a

ct

o

r

f

=

h

f

(L

/D

)V

/(

2

g

)

2

0 . 0 0 0 0 1

0 . 0 0 0 0 5

0 . 0 0 0 1

0 . 0 0 0 2

0 . 0 0 0 4

0 . 0 0 0 6

0 . 0 0 0 8

0 . 0 0 1

0 . 0 5
0 . 0 4
0 . 0 3

0 . 0 2

0 . 0 1

0 . 0 1 5

0 . 0 0 8
0 . 0 0 6
0 . 0 0 4

0 . 0 0 2

R

e

la

tiv

e

R

o

u

g

h

n

e

ss

,

/D

L a m i n a r

F l o w

C r i ti c a l

Z o n e

T r a n s i ti o n

Z o n e

La

m

in

a

r F

lo

w

f =

6

4/R

e

/D = 0 . 0 0 0 0 0 5

/D = 0 . 0 0 0 0 0 1

C o m p l e te T u r b u l e n c e , H y d r a u l i c a l l y R o u g h

H y d r a u l i c a l l y S m o o th

k



k

k