1

CALCULUS I

DIFFERENTIAL CALCULUS

Mathematical Analysis I

2

2

3

2

Pythagoreans

a school

,

in some ways a brotherhood, and in

some ways a monastery.

Pythagoras of Samos

between 580 and 572 BC
(between 500 and 490 BC)

4

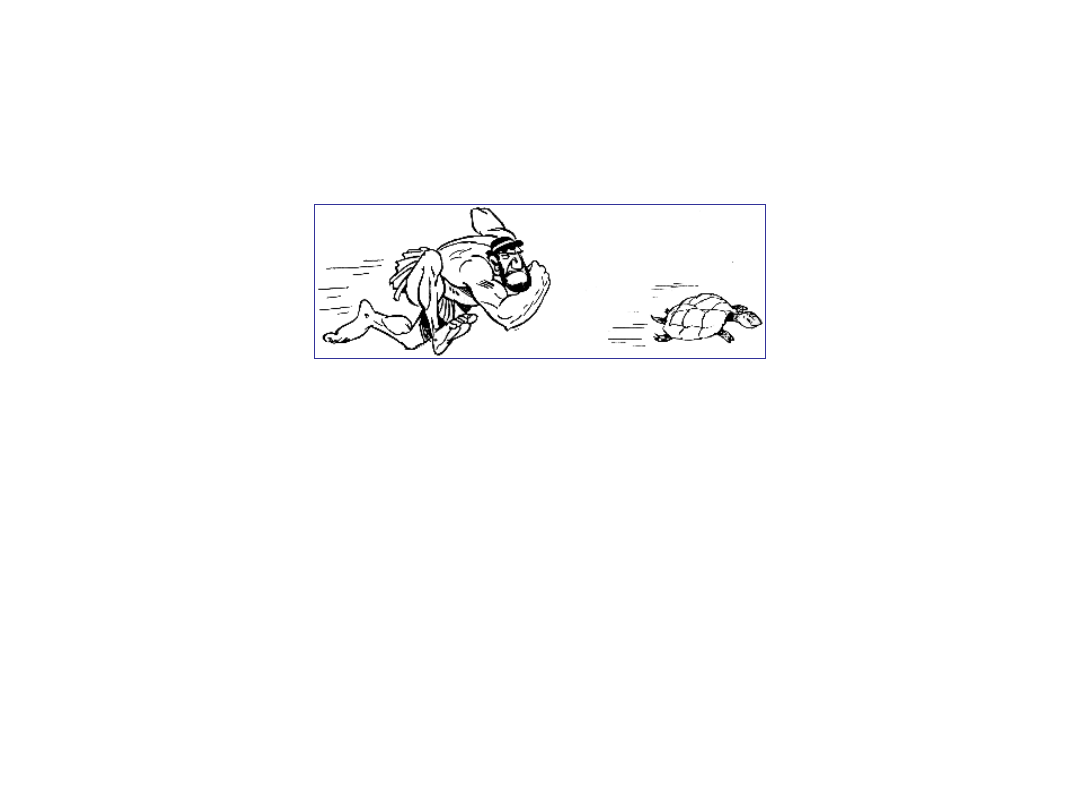
Zeno of Elea (490-430 BC): On the paradox of motion

5

Achilles

Tortoise

6

7

 

                    

             

ISAAC NEWTON 1643 – 1727, England

8

Gottfried Leibniz, 1647-1716, Germany

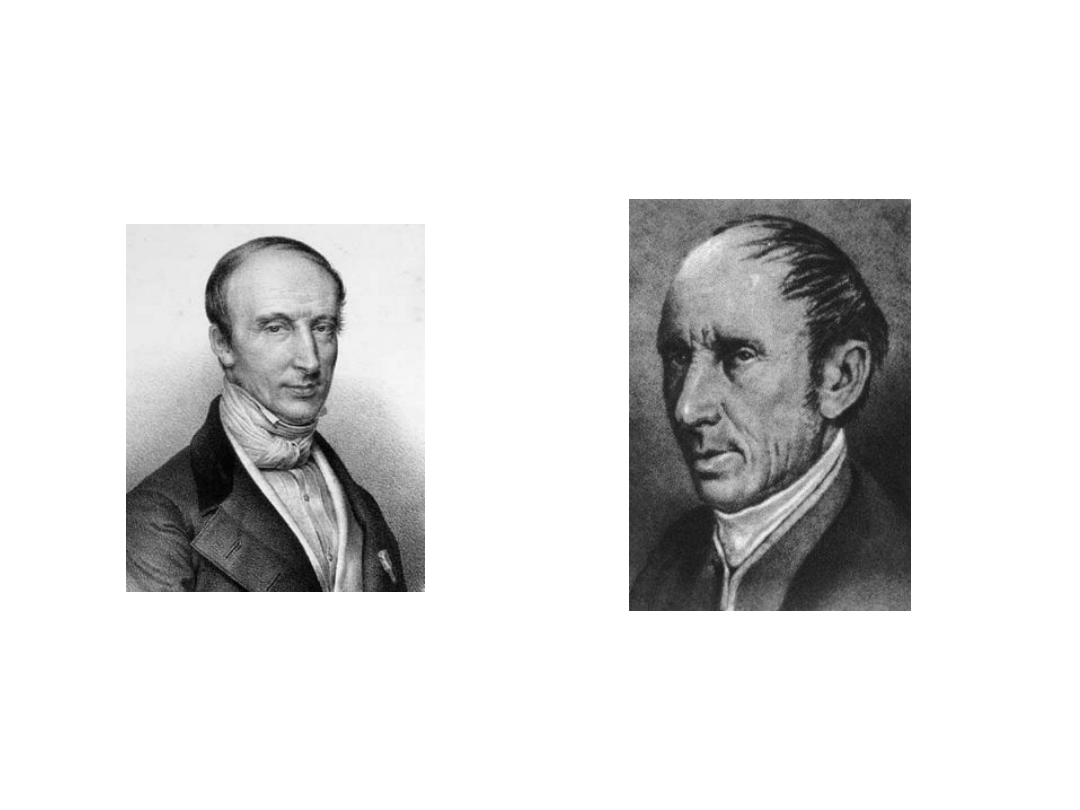
9

Augustin Louis Cauchy, 1789 -1857, France

10

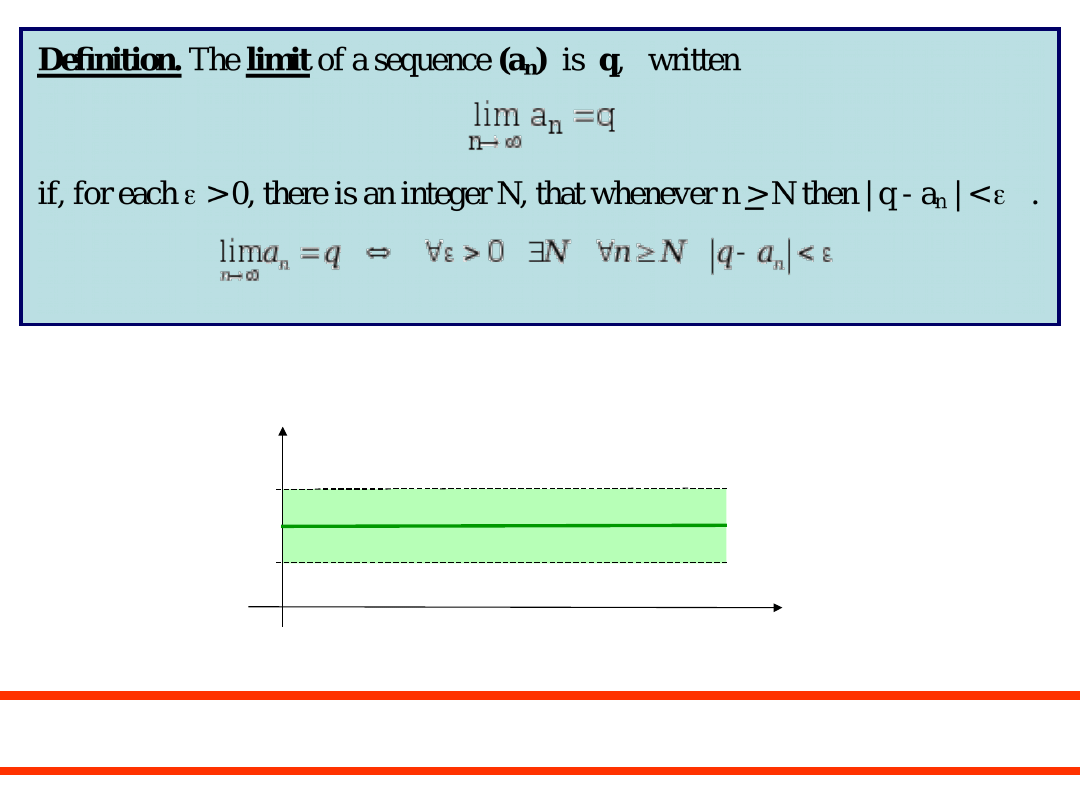
LIMIT OF A SEQUENCE

Lecture 1

11

A SEQUENCE

12

A SEQUENCE

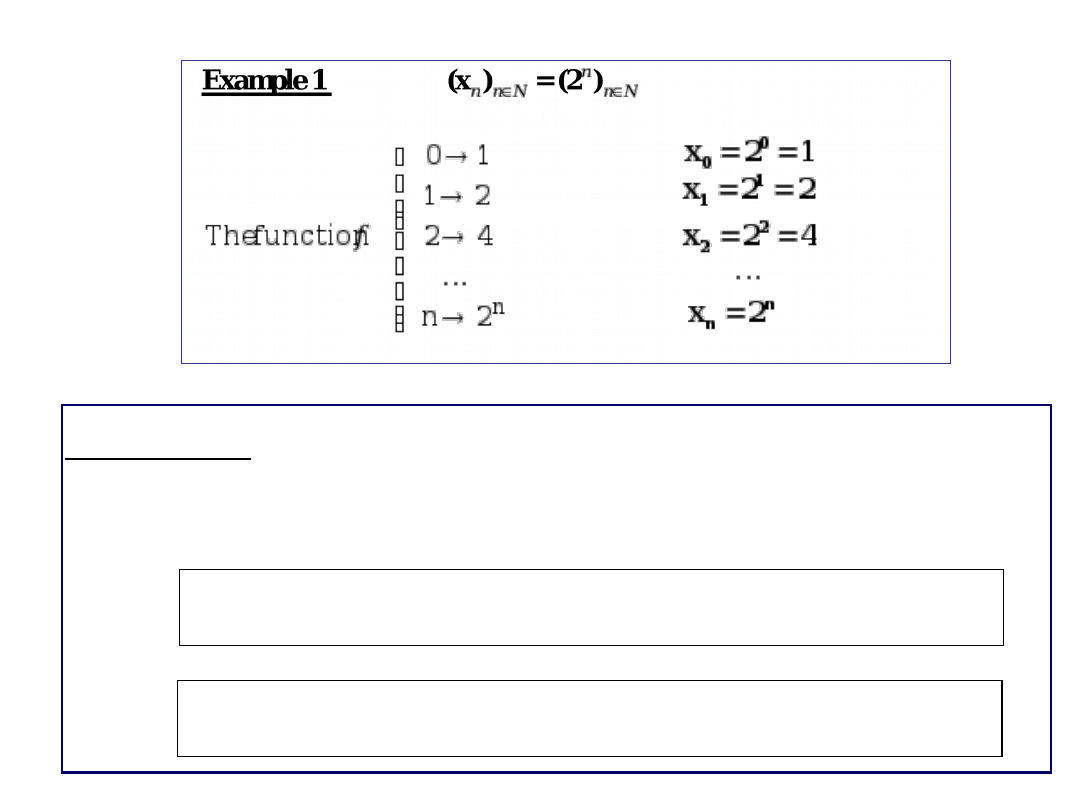
13

,

x

x

0







...

,

1

,

0

n

,

x

,

,

x

,

x

F

x

0

1

n

n

1

n











r

x

x

n

1

n







n

1

n

x

q

x





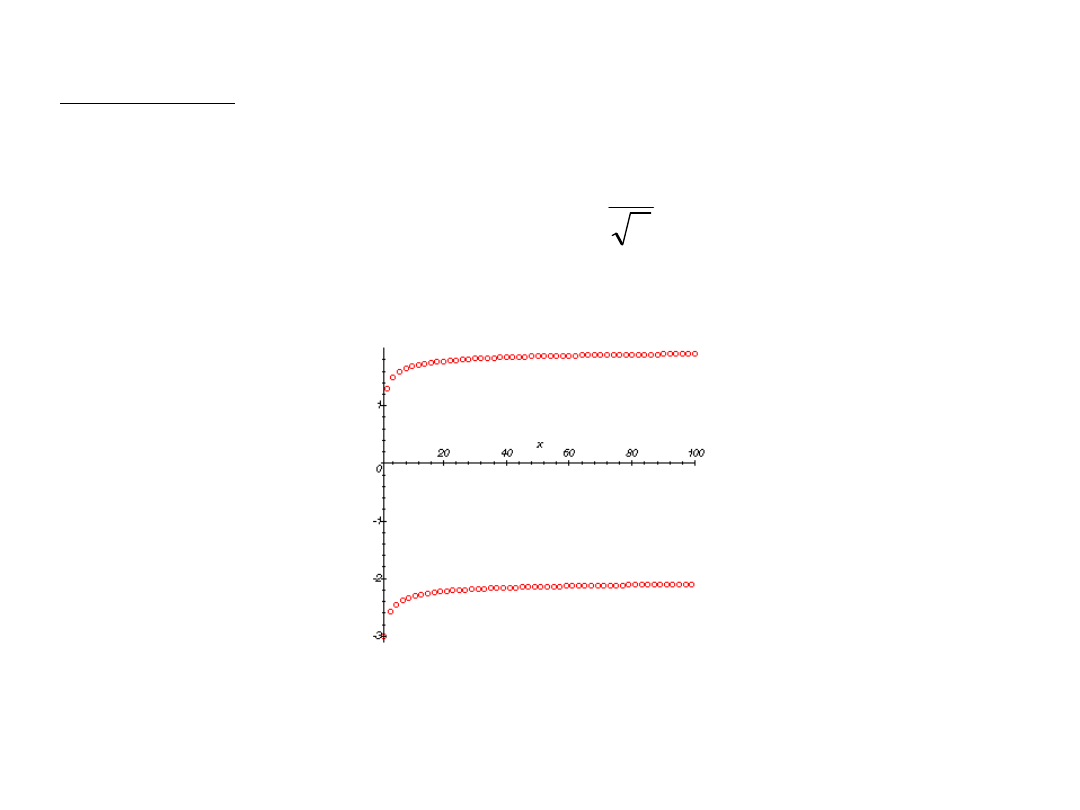
Example 2

recursive definition

arithmetic sequence - common difference

geometric sequence - common ratio

14

15

n

1

2

1

a

n

n







)

(

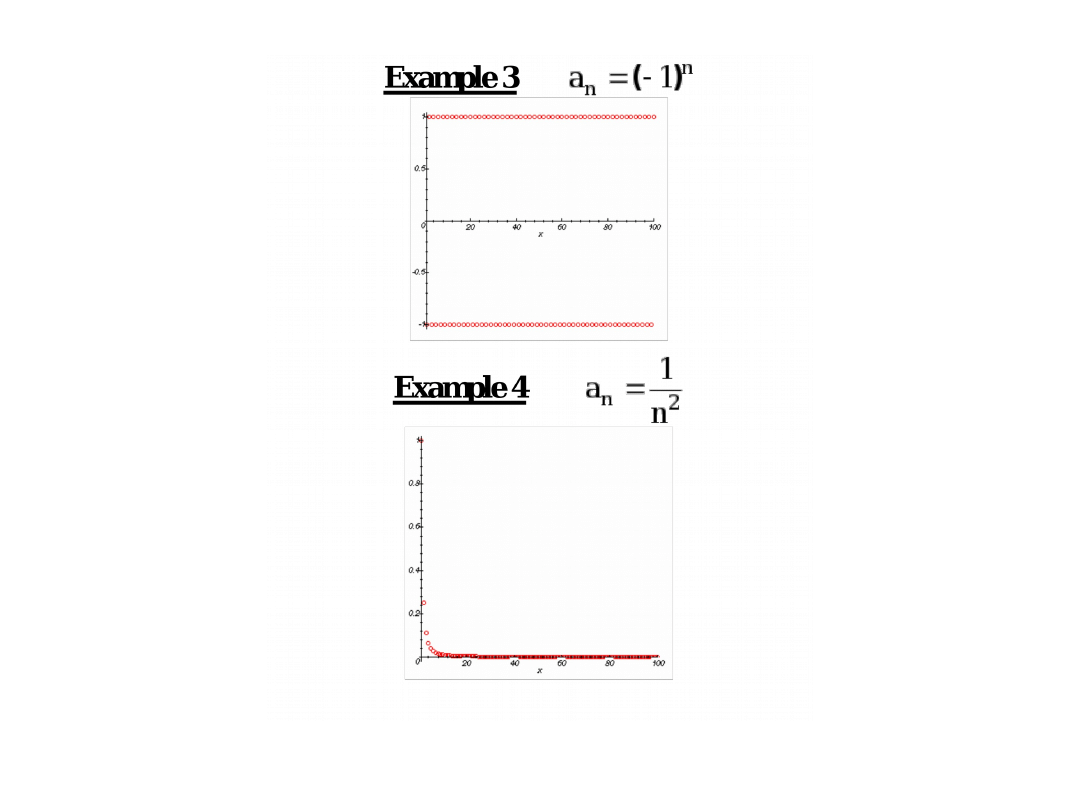
is divergent

Example 4

16

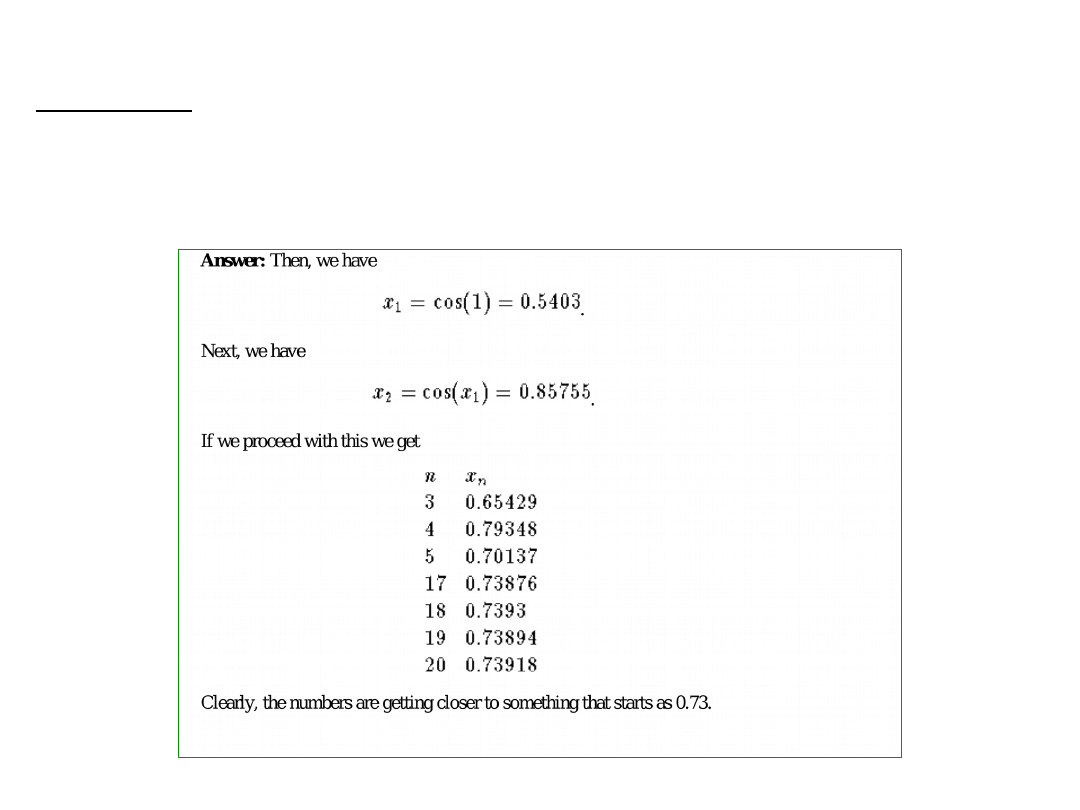
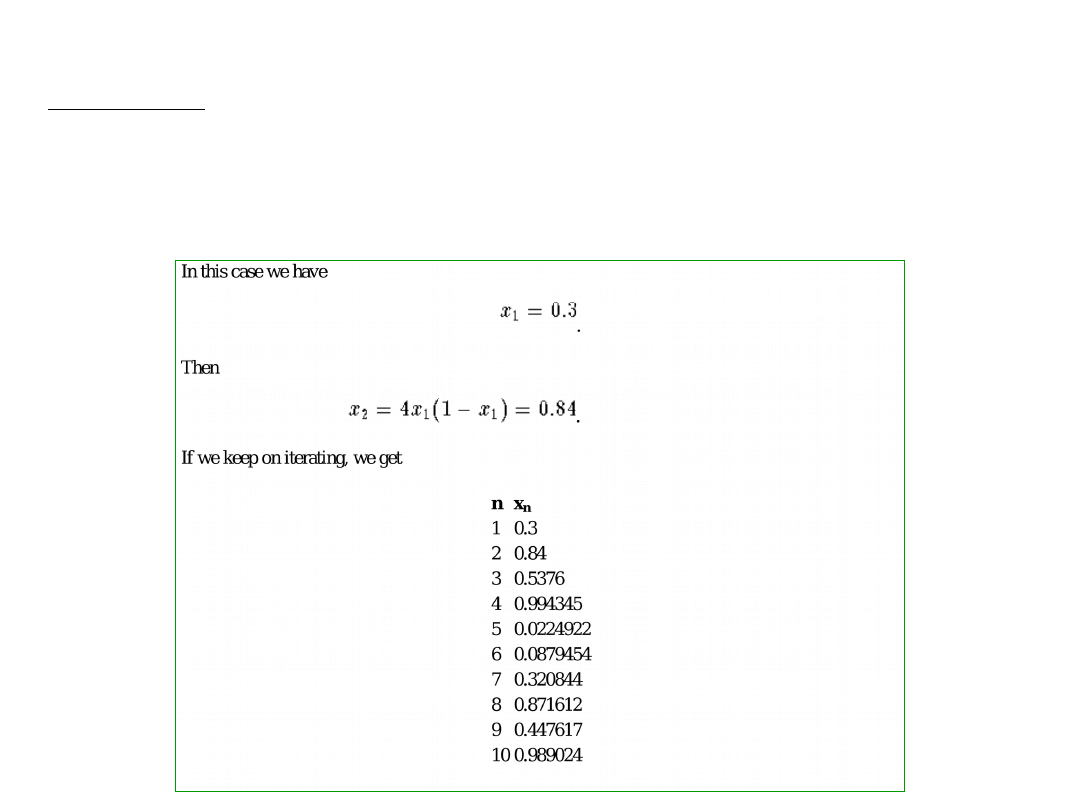
Example 5 Take a calculator, set it to "radian mode" and enter the
number 1. Then, hit the function cosine over and over again. Analyse the
output of this experiment.

17

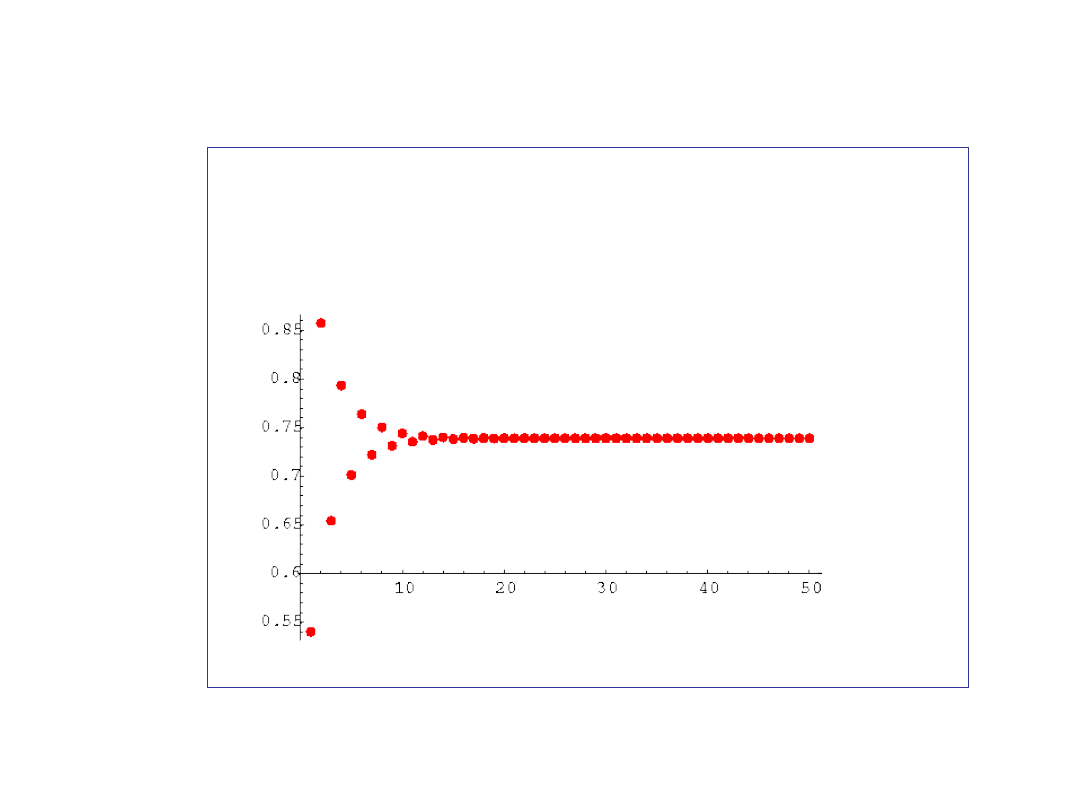
We graph the points (n, x

n

) on a plane

The points ‘converge’ to about 0.73

18

Example 6 As before, take your calculator and enter the number 0.3.
Second, program your machine to compute y = f (x) = 4(1- x) x.

Then, keep on doing the same as you did in the previous two examples.
Finally, analyse the output.

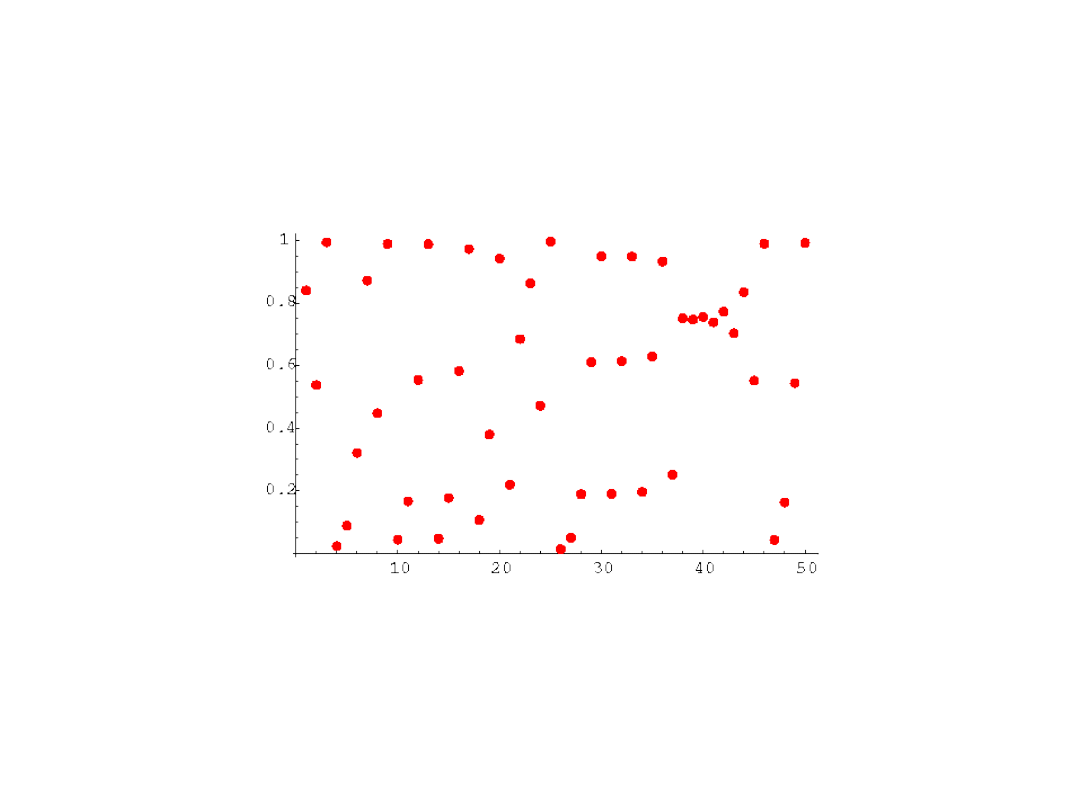
19

The plot of the sequence x

n

= 4(1- x

n-1

) x

n-1

(the first 50-ty terms)

Completely chaotic - divergent

20

LIMIT OF A SEQUENCE

21

If the limit of the sequence (a

n

) exists then we say the sequence (a

n

) is

convergent; otherwise, we say the sequence (a

n

) is divergent.

No matter how small the number is, at some ‘moment’ the terms of the sequence
enter the band of width and stay there





n

a

n

q

q+ε

q-ε

x

x

x

x

x

x

x

x

x

x x x

x

x

x

x

x

x

22

Suppose that the sequences (a

n

)

nN

and (b

n

)

nN

are convergent then for :

.

,

,

,

,

,

,

0

0

0

1

0

0

0



















INDETERMINATE FORMS:

,

b

b

lim

,

a

a

lim

n

n

n

n













,

0

A

A

)

A

(

lim

.

6

R

C

,

a

)

a

(

lim

.

5

0

b

lim

if

b

a

b

a

lim

.

4

b

a

)

b

a

(

lim

.

3

,

b

a

)

b

a

(

lim

.

2

a

C

)

a

C

(

lim

.

1

a

a

n

C

C

n

n

n

n

n

n

n

n

n

n

n

n

n

n

n

n



























































R

C

23

How to use the definition to find the limit.

0

n

)

1

(

lim

n

n









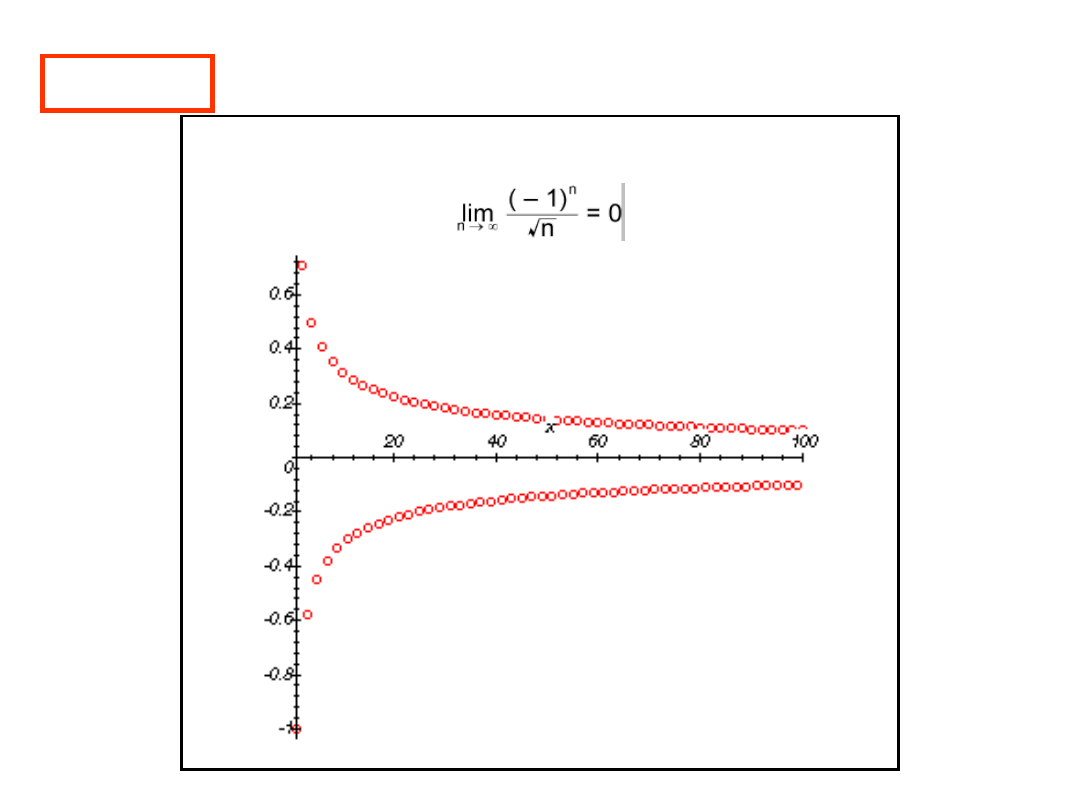
24

1.

Guess

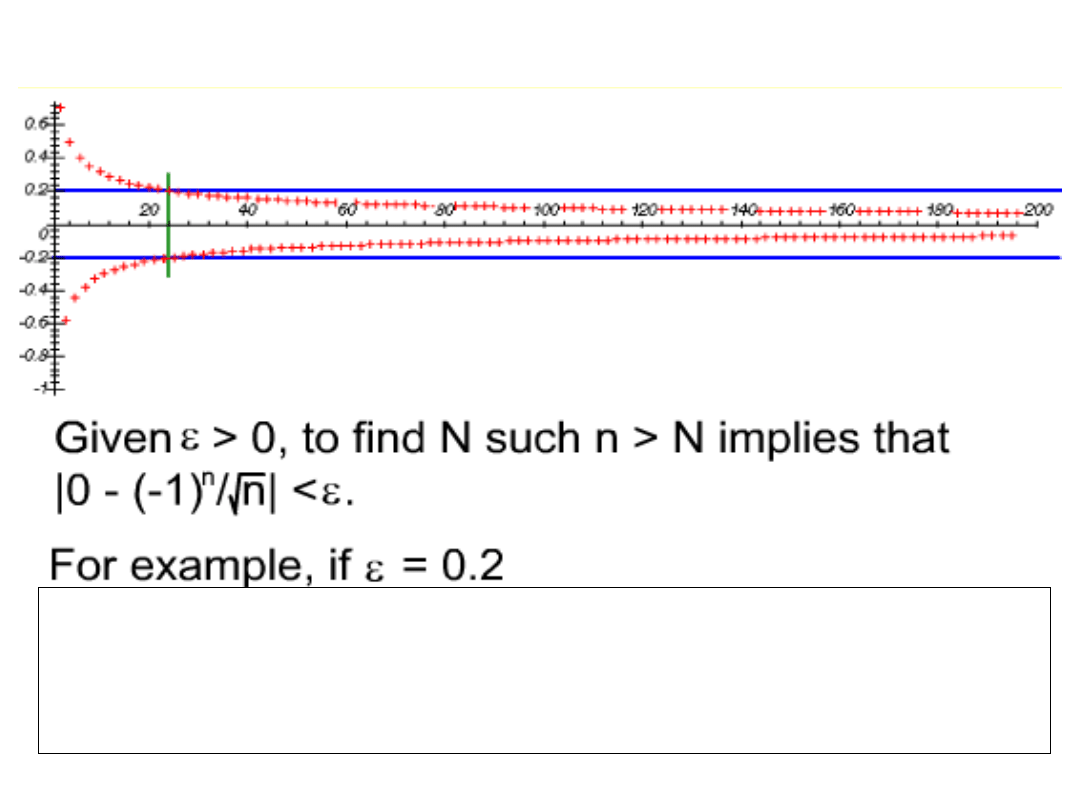
25

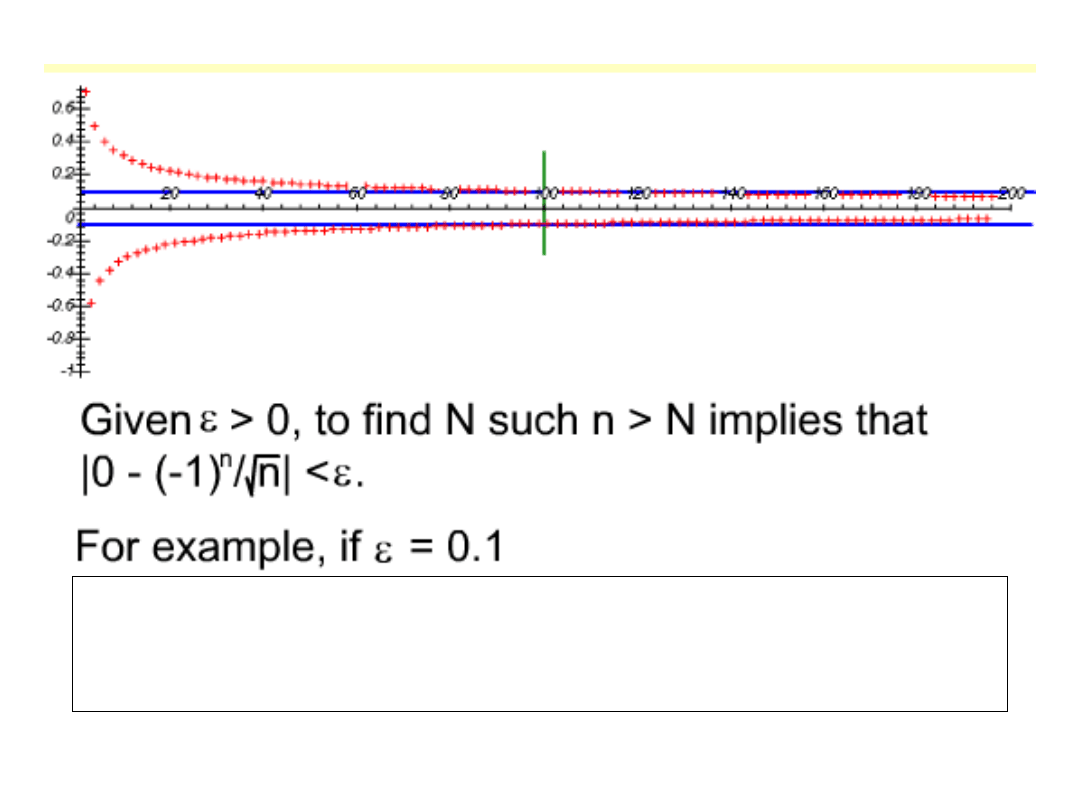
26

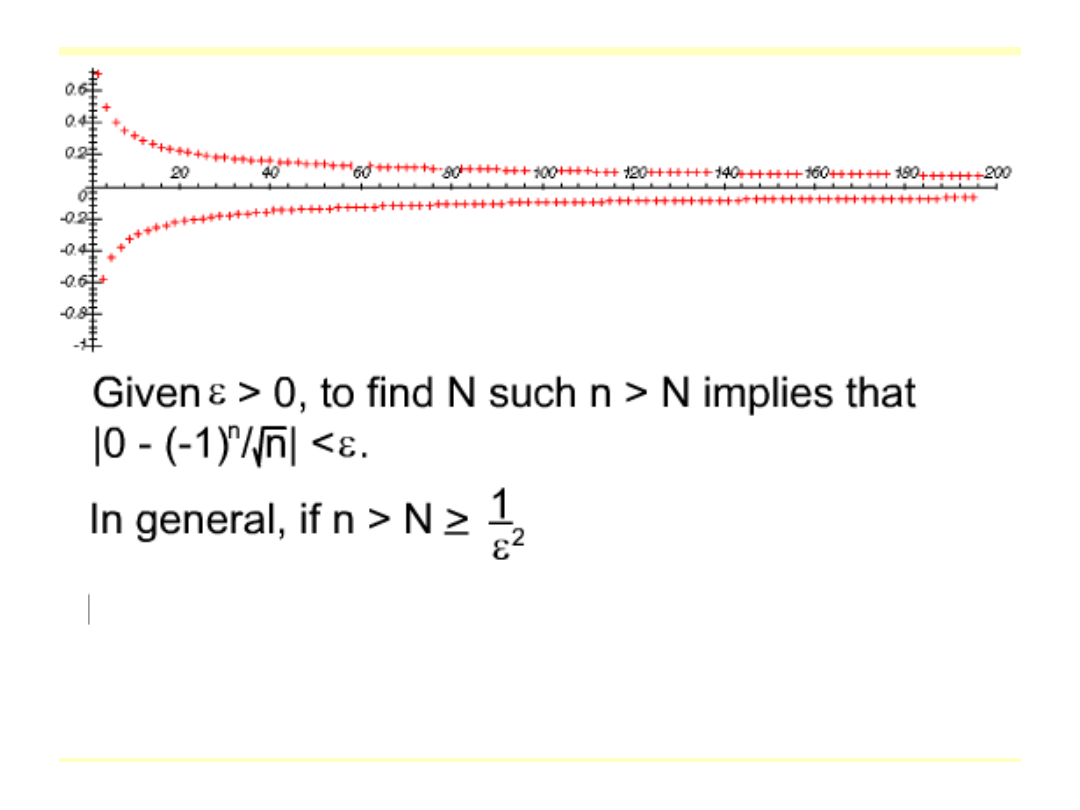
27

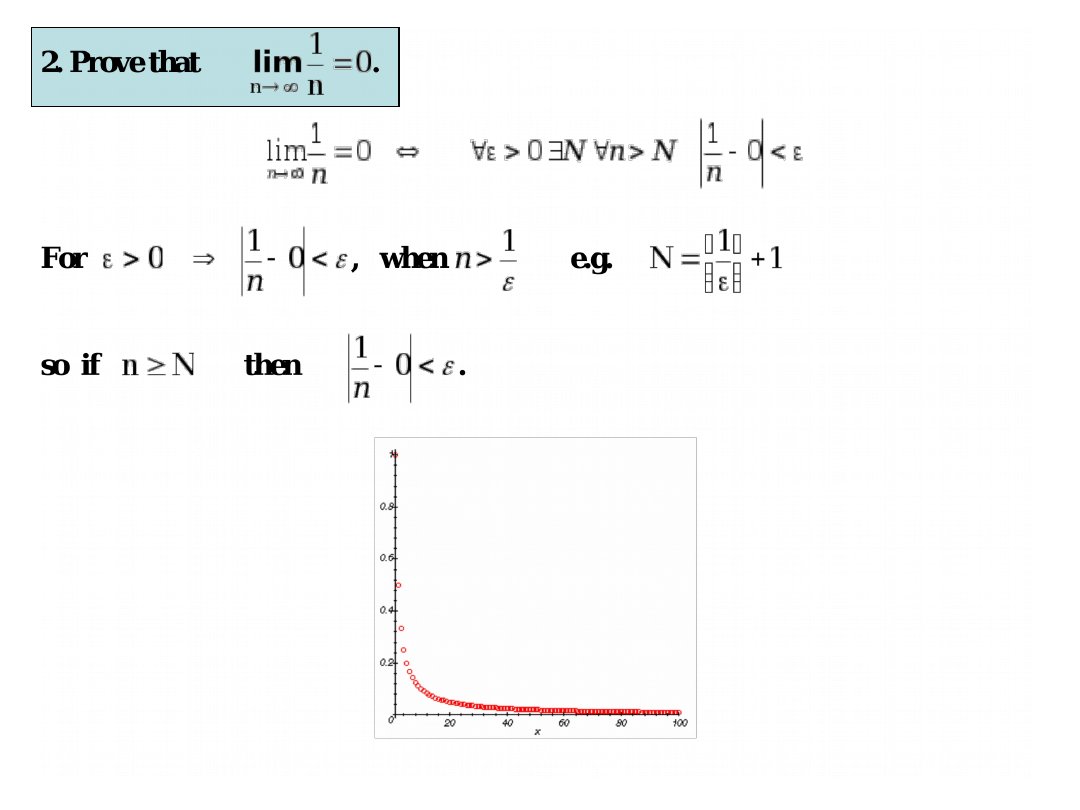
28

29

Theorem

If the limit exists, it must be unique.

If a

n

is convergent then it has only one limit.

Proof
Suppose that q’, q’’ are limits of (a

n

) and that

.Let N’ be a natural number such that if then

Let N” be a natural number such that if then









"

q

'

q

and

''

q

'

q

,'

N

n







3

1

'

q

a

n

,

"

N

n







3

1

"

q

a

n

Let N = sup{N’,N”}, so that































3

2

3

1

3

1

"

q

a

a

'

q

"

q

a

a

'

q

"

q

'

q

n

n

n

n













3

2

"

q

'

q

and

"

q

'

q

We obtain a contradiction

QED

n

n

a

lim





30

0

q

n

n







lim

1

q 





 0

q

n

q

n

log

log



1

q

n

0























log

log

IMPORTANT LIMITS

1.

,for

Sketch of proof:



so

QED

1

a

lim

n

n







1

a









2

n

x

2

1

n

n

nx

1

x

1











R

x

N

n



 ,

,for

For proof we need the Bernoulli Inequality

,

where

2.

QE

D

31

Theorem SQUEEZE PRINCIPLE
( Sandwich, Policeman Theorem )

     

n

n

n

c

,

b

,

a

n

n

n

c

b

a





q

c

lim

a

lim

n

n

n

n













q

b

n

n







lim

Assume that the sequences

satisfy

.

then

0

n

n

some

for



an
d

32

n

n

n

n

n

5

4

3









lim

n

n

n

n

n

n

n

n

5

3

5

4

3

5











EXAMPL
E

n

n

n

5

3

n

n

5

5

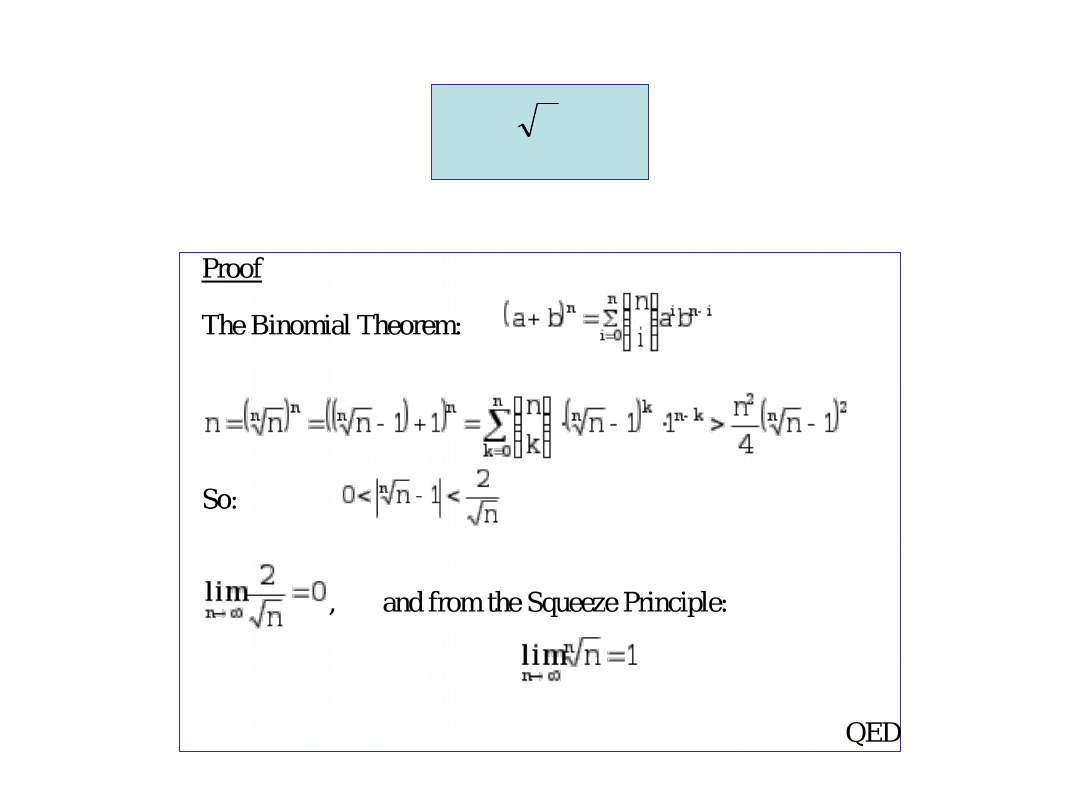
33

.

lim

1

n

n

n







34

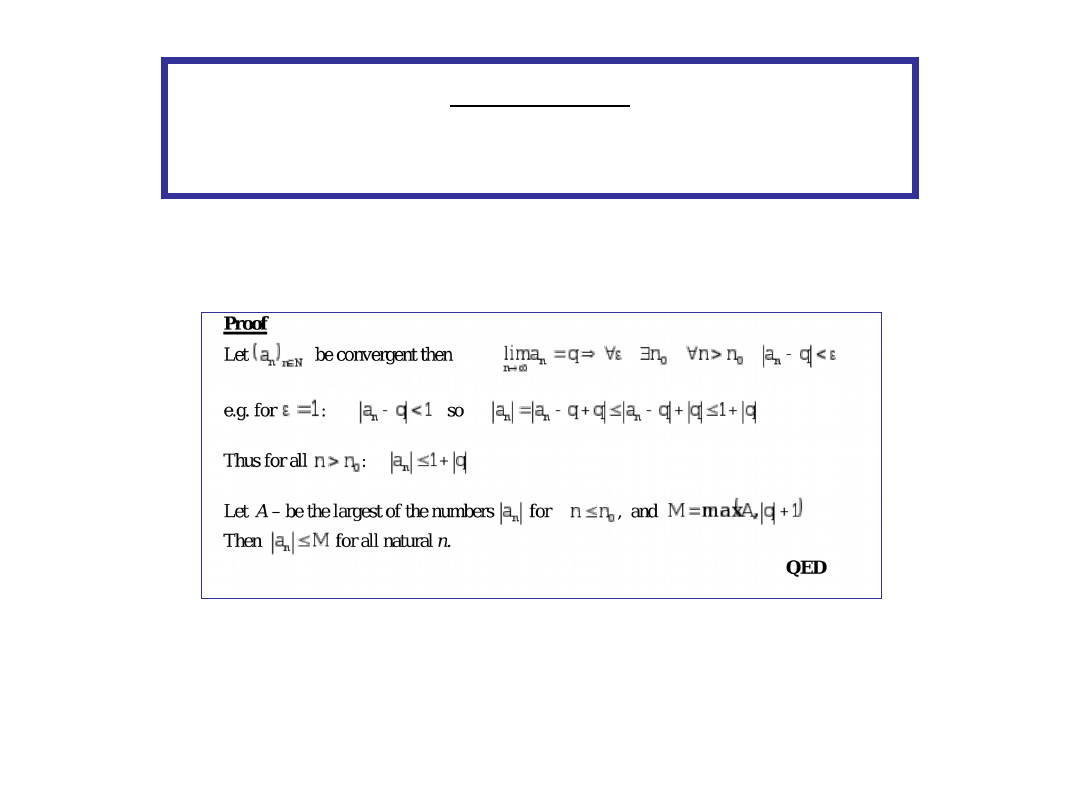
Theorem

Every convergent sequence is bounded.

35

Note:

Boundedness is necessary but not sufficient to guaranty convergence.

Example:

(-1)

n

 bounded,

divergent

36

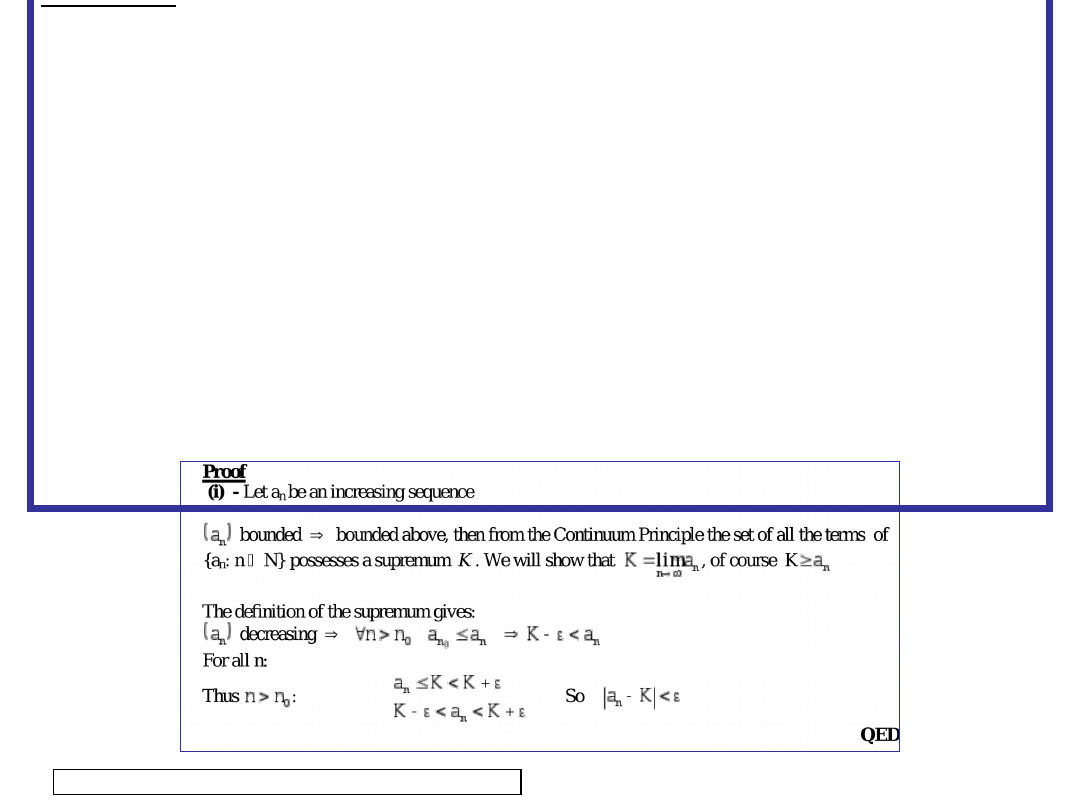
Theorem
BOUNDED MONOTONE SEQUENCE
THEOREM

Let a

n

be a monotone sequence

( increasing a

n

≤ a

n+1

or

decreasing a

n+1

≤ a

n

for all a

n

, except maybe some first terms a

1

, a

2

,

a

3

,... a

k

)

(i)

If a

n

is bounded

(appropriately:a) bounded above i.e. there

exists an upper bound U such that a

n

≤ U

or b)bounded below i.e.

there exists a lower bound L such that L

≤ a

n+1

)

then a

n

it is

convergent.

(ii)

If a

n

is unbounded then it is divergent

to either .





http://demonstrations.wolfram.com/ConvergenceOfAMonotonicSequence/

37

.

!

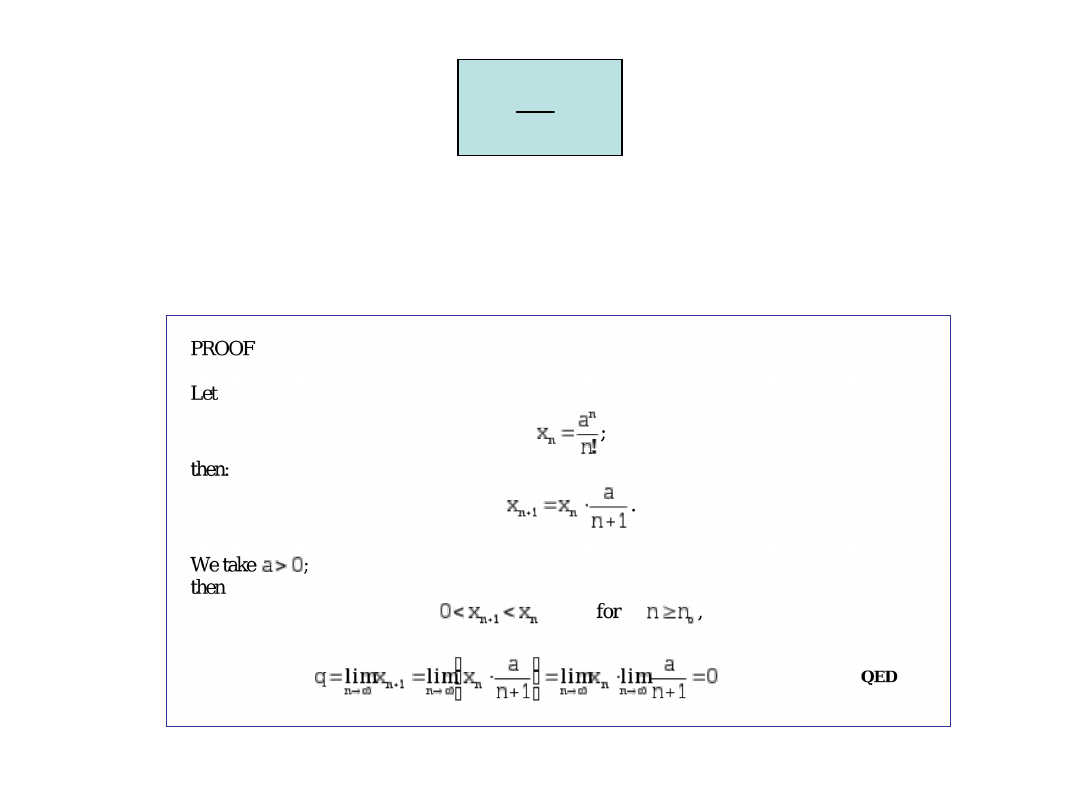
lim

0

n

a

n

n







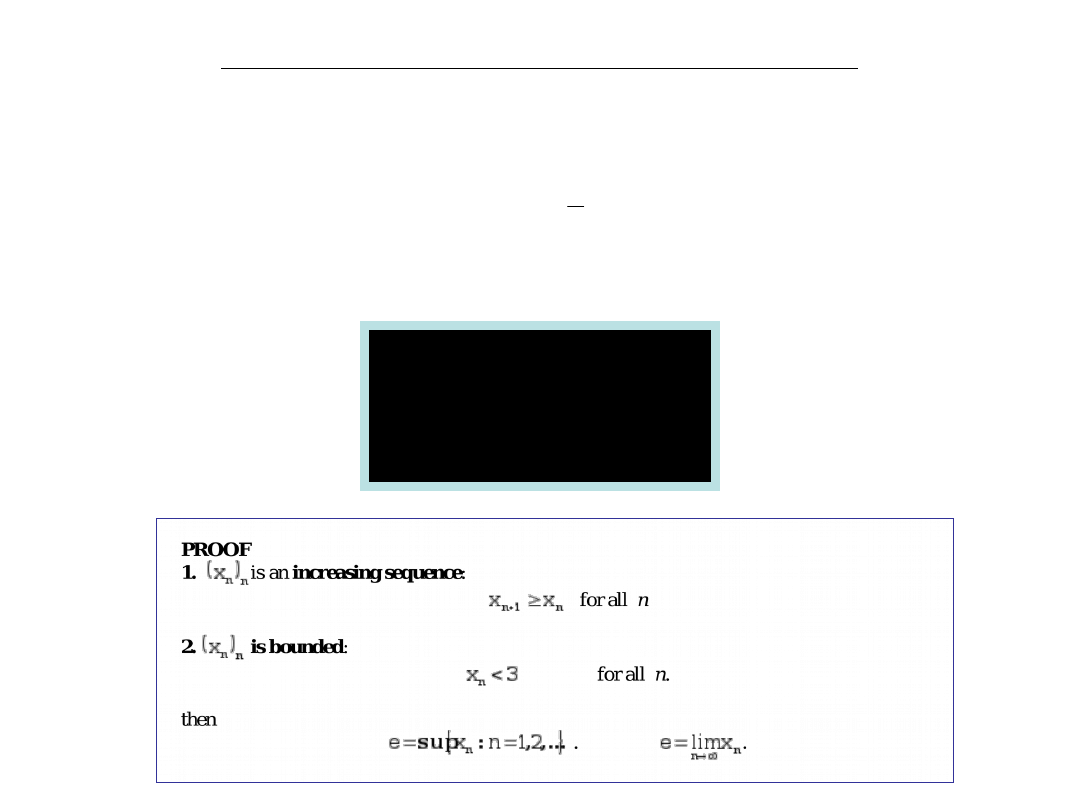
38

IMPORTANT EXAMPLE THE NUMBER e:

The definition (existence) of number e – the base of the
natural logarithm,
the Euler number

n

n

n

1

1

x











 



We will use the monotone bounded sequence theorem to prove the existence of

n

n

n

1

1

lim

e











 







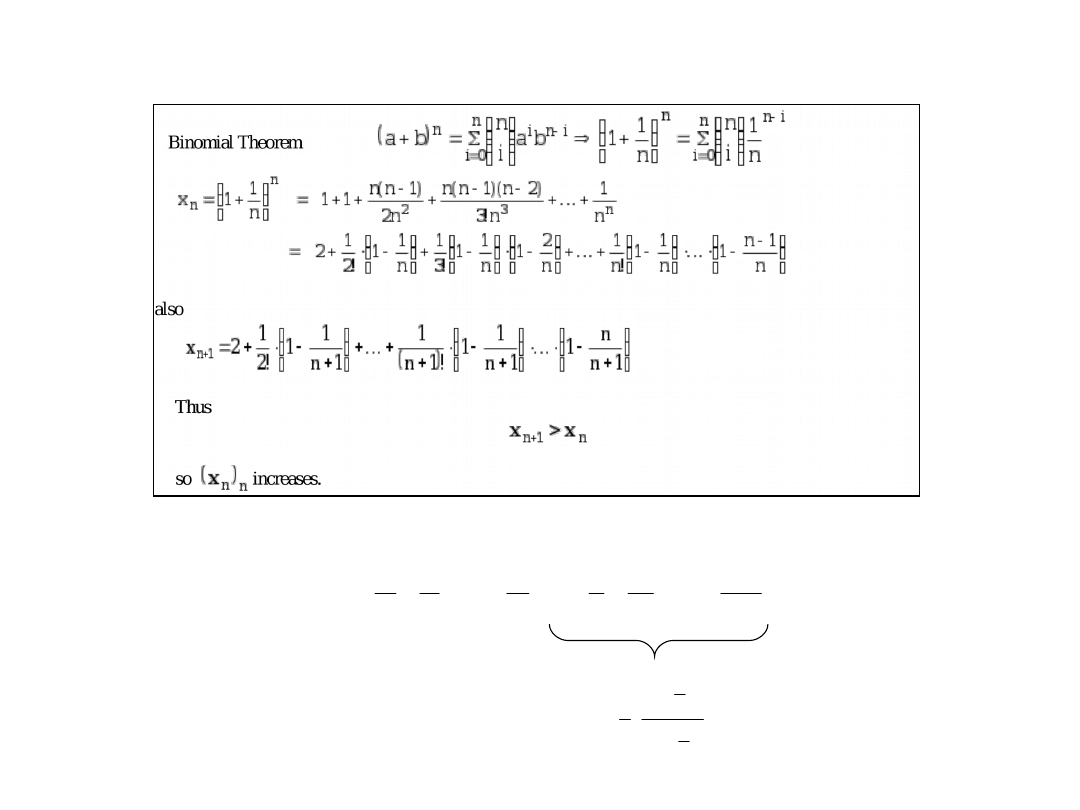
39

1.

We will show that

)

(

n

x

is an increasing sequence











































2

1

1

2

1

1

2

1

2

n

3

2

1

2

1

2

1

2

n

1

3

1

2

1

2

x

1

n

2

n





























!

!

!

2. And that (x

n

) is bounded

QED

40

e ≈ 2,718281828459045....

41

Definition
The sequence (a

n

) diverges to positive infinity iff

Example

The sequence is divergent to infinity





M

a

n

M

a

lim

n

n

n

























Definition
The sequence (a

n

) diverges to minus infinity iff





N

n

n

2



)

(

M

a

n

M

a

lim

n

n

n

























42

Definition
A subsequence of the sequence (x

n

) is a sequence of the form

( x

a(n)

), where the a(n) are natural numbers with a(n) < a(n+1)

for all n.

Intuitively, a subsequence omits (loses) some elements of the
original sequence.

Theorem
A sequence is convergent if and only if all of its
subsequences converge towards the same limit.

43

.

,

,

,

,

,

,

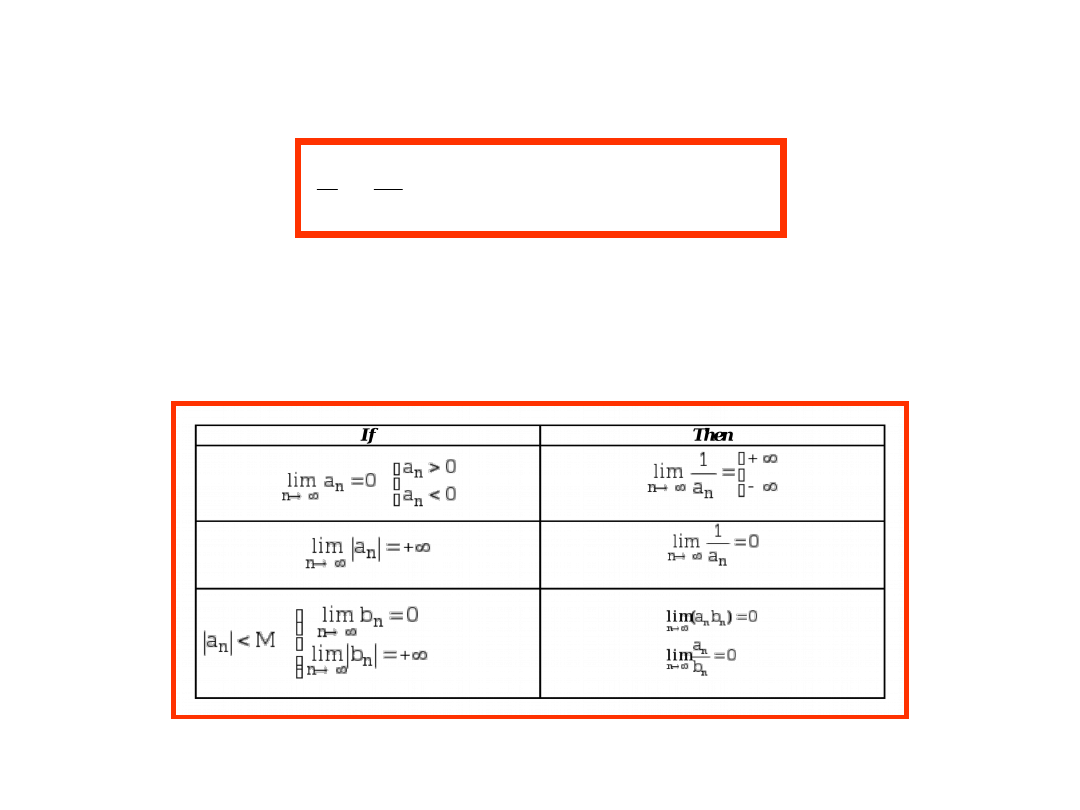
0

0

0

1

0

0

0



















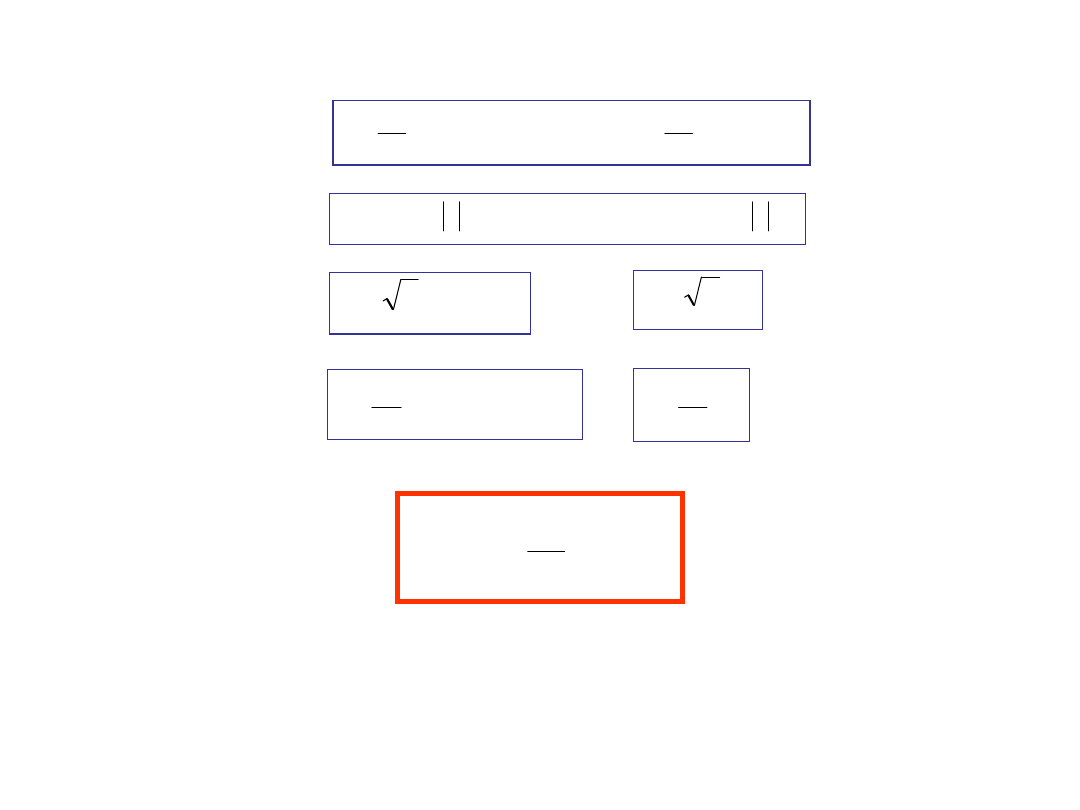
INDETERMINATE FORMS:

44

EXTENDED ARITHMETIC
For the sake of convenience in dealing with indeterminate forms, we
define the following arithmetic operations with real numbers, positive
infinity and negative infinity.
Let c be a real number and c > 0, then we define:





































































































































)

)(

(

)

)(

(

)

)(

(

.

6

0

)

(

)

(

.

5

0

c

0

c

0

c

,

0

c

.

4

,

.

3

)

(

)

c

(

,

)

(

)

c

(

,

)

(

c

,

)

(

c

.

2

,

c

.

1

c

c

45

0

p

,

n

1

lim

0

p

,

0

n

1

lim

p

n

p

n



















1

q

,

q

lim

1

q

,

0

q

lim

n

n

n

n



















1

a

,

1

a

lim

n

n









1

n

lim

n

n







0

k

,

1

a

,

n

a

lim

k

n

n













0

!

n

a

lim

n

n







A

a

n

a

e

a

A

1

lim

n

n





















;

;

,

;

;

;

.

IMPORTANT LIMITS

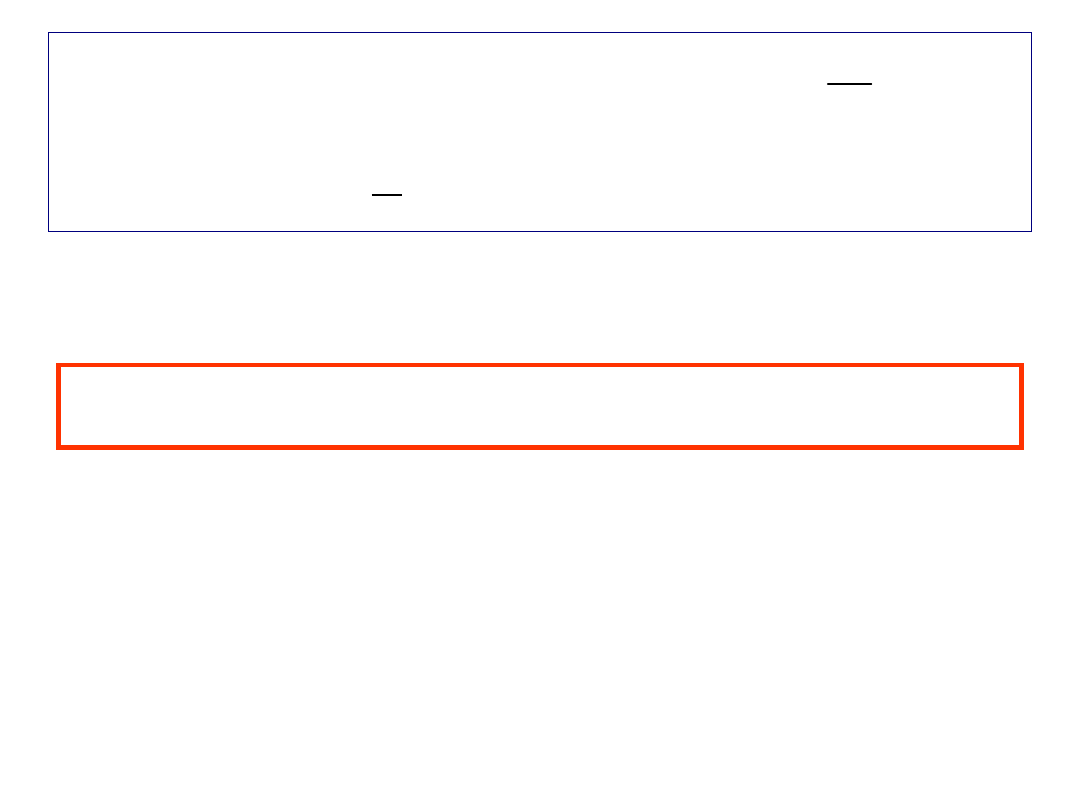
46

Let

n

n

b

a 

( a

n

is „slower” then b

n

) if

0

b

a

lim

n

n

n







then for some n > n

0

:





















n

n

n

n

n

k

3

2

a

n

n

!

n

a

2

n

n

n

n

n

log

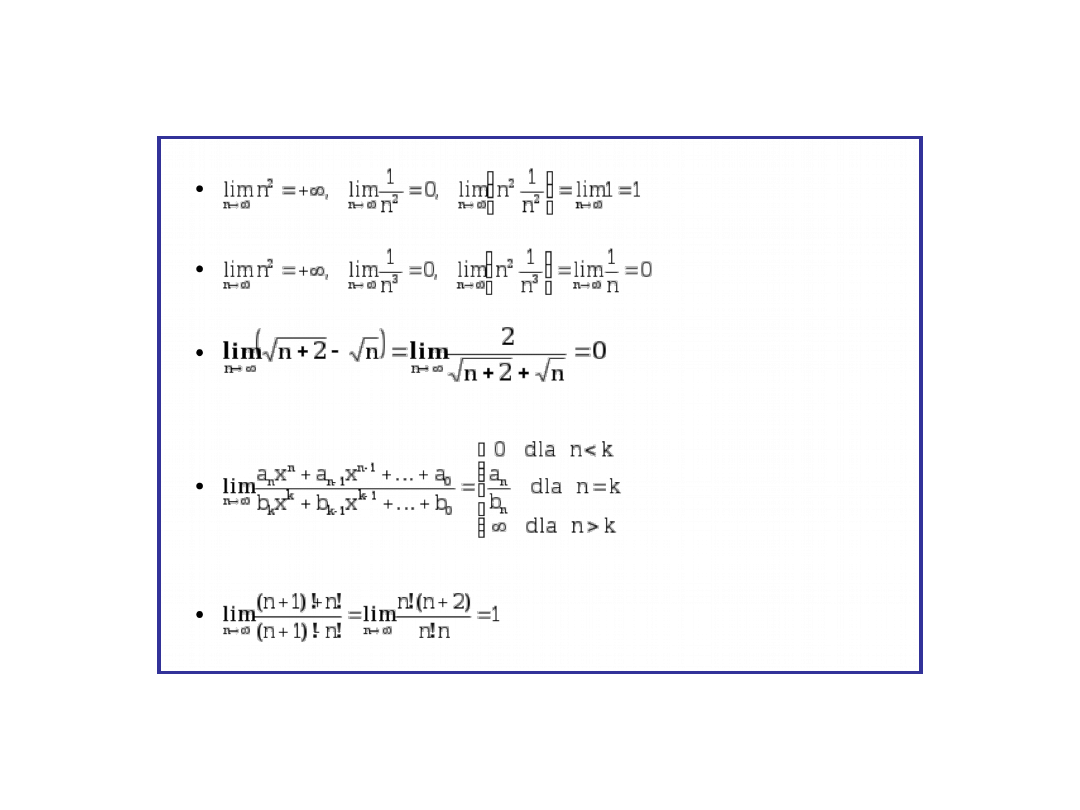
47

Examples