DERIVATIVES

Lecture 4

NOTATIONS - the ones you need to know how to read aloud

The definition

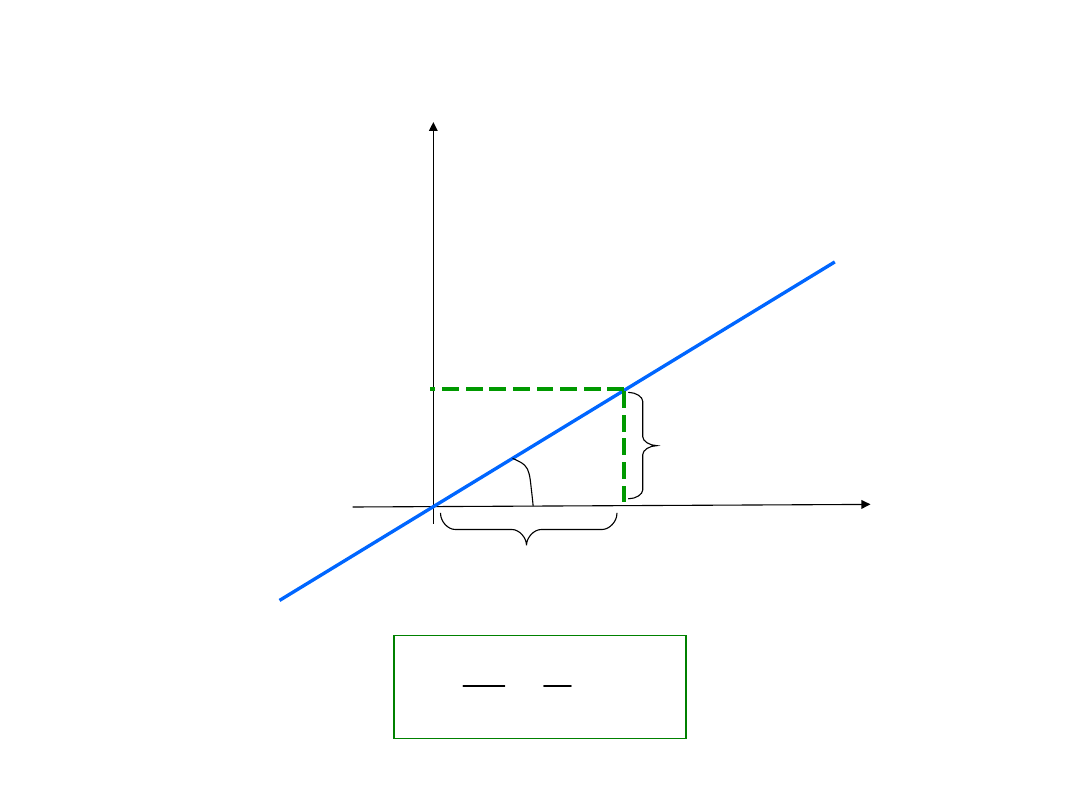
The

slope

of a line

y =

m

x +b



y = mx

A

B









tan

A

B

x

y

m

0

0

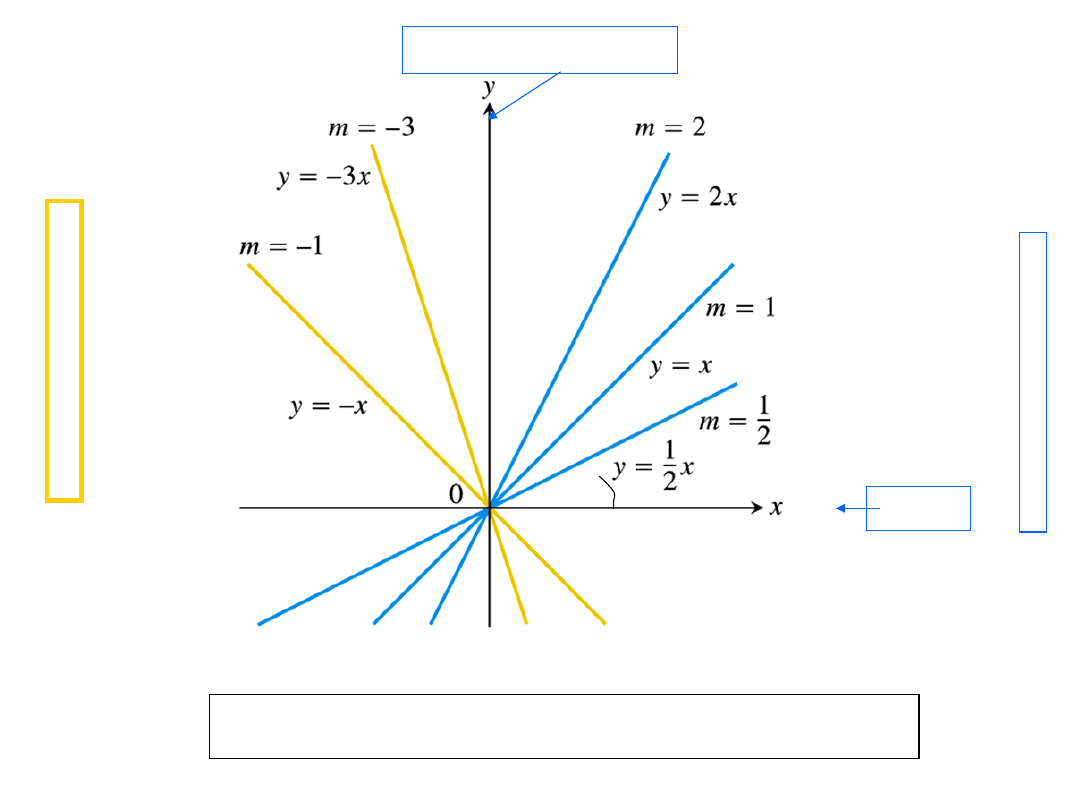
y = mx + b

m is the slope

m = 0

no slope ‘m = ∞’



tan

m



p
o
s
i
t
i
v
e

n
e
g
a
t
i
v
e

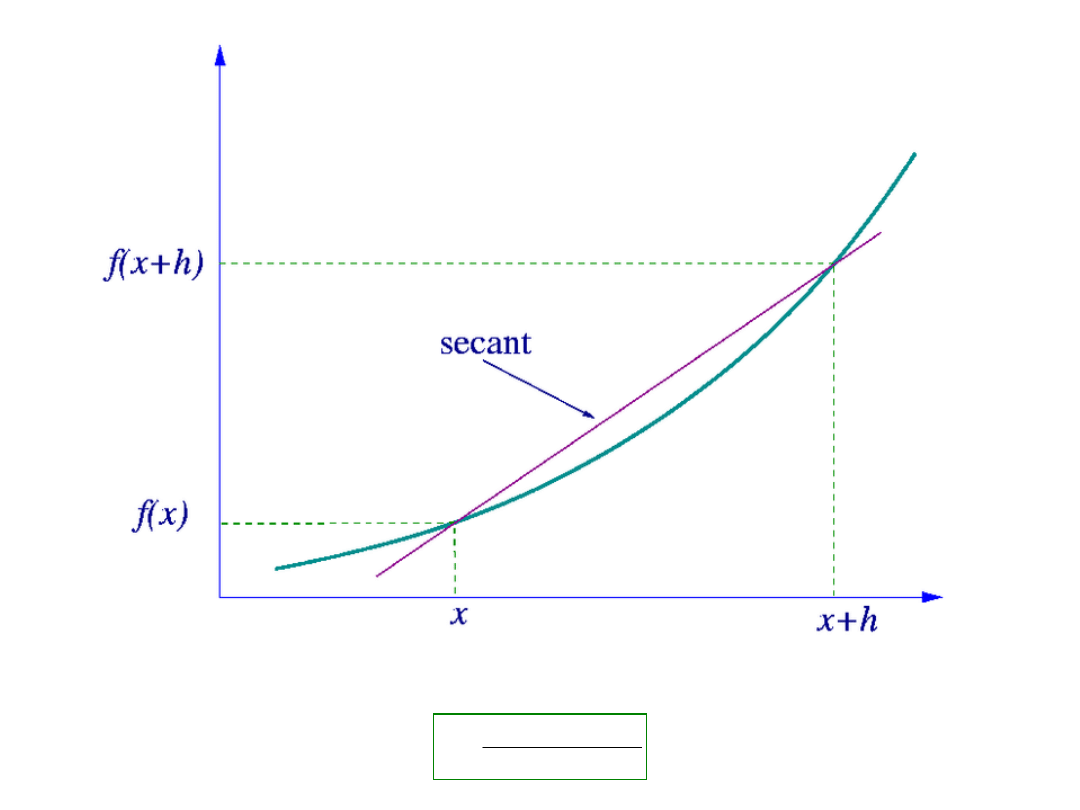
a function f

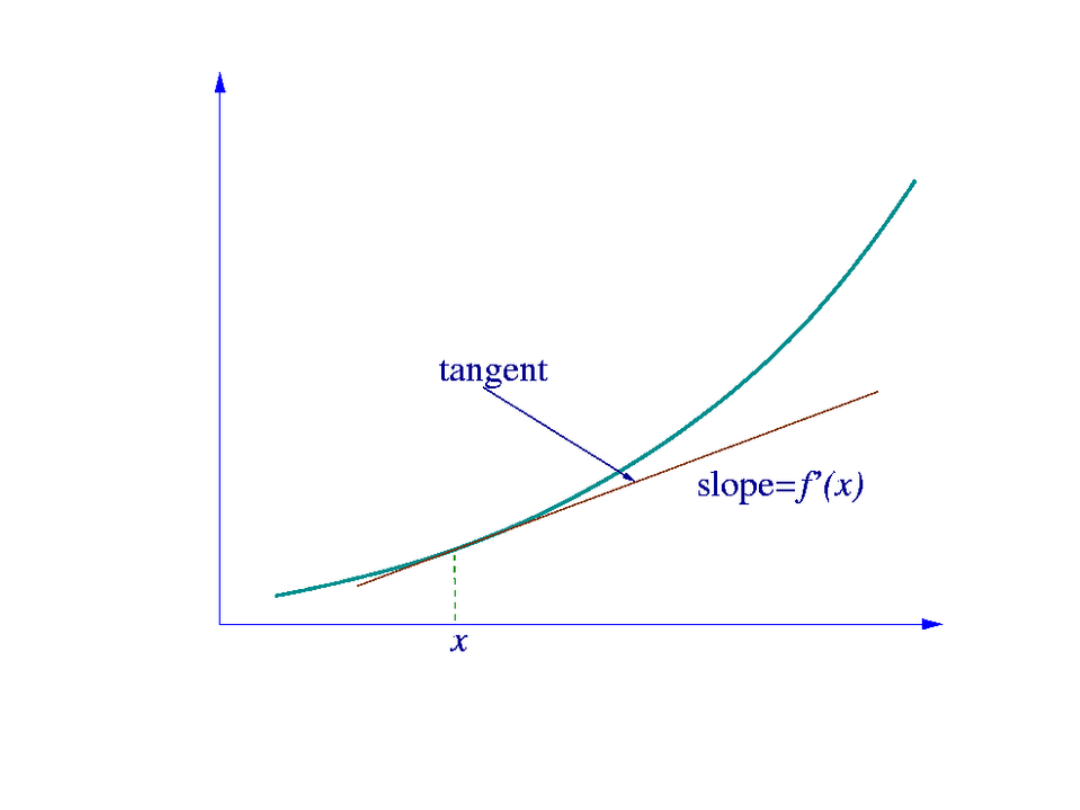
What is the solpe of the secant passing through x ?

h

h

x

f

x

f

m

)

(

)

(







)

h

x

(

f

0



)

x

(

f

0

h

x

0



0

x

h

)

x

(

f

)

h

x

(

f

x

)

h

x

(

y

y

x

y

tan

0

0

0

0

0























)

x

(

dx

df

)

x

(

'

f

h

)

x

(

f

)

h

x

(

f

lim

h

0

0

0

0

0











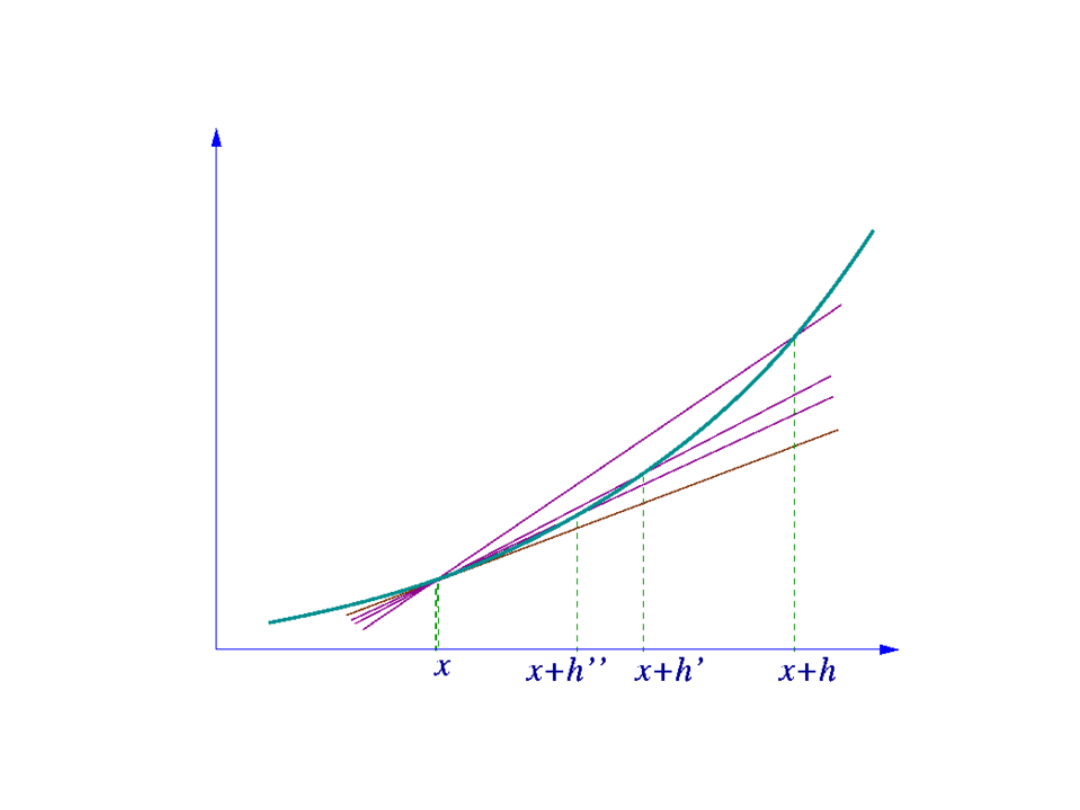
The derivative of f at point x

0

.

)

x

(

'

f

h

)

x

(

f

)

h

x

(

f

lim

h







 0

)

x

(

'

f

x

x

)

x

(

f

)

x

(

f

lim

x

x

0

0

0

0









)

x

(

'

f

x

)

x

(

f

)

x

x

(

f

lim

x

0

0

0

0















DIFFERENT NOTATIONS

0

0

0

x

x

dx

df

)

x

(

f

dx

d

)

x

(

dx

df







h

)

x

(

f

)

h

x

(

f

lim

)

x

(

'

f

h













0

h

)

x

(

f

)

h

x

(

f

lim

)

x

(

'

f

h













0

LEFT-HAND AND RIGHT HAND DERIVATIVES

Wikipedia

f ’(x) is a function which tells us the slope of the function at x.

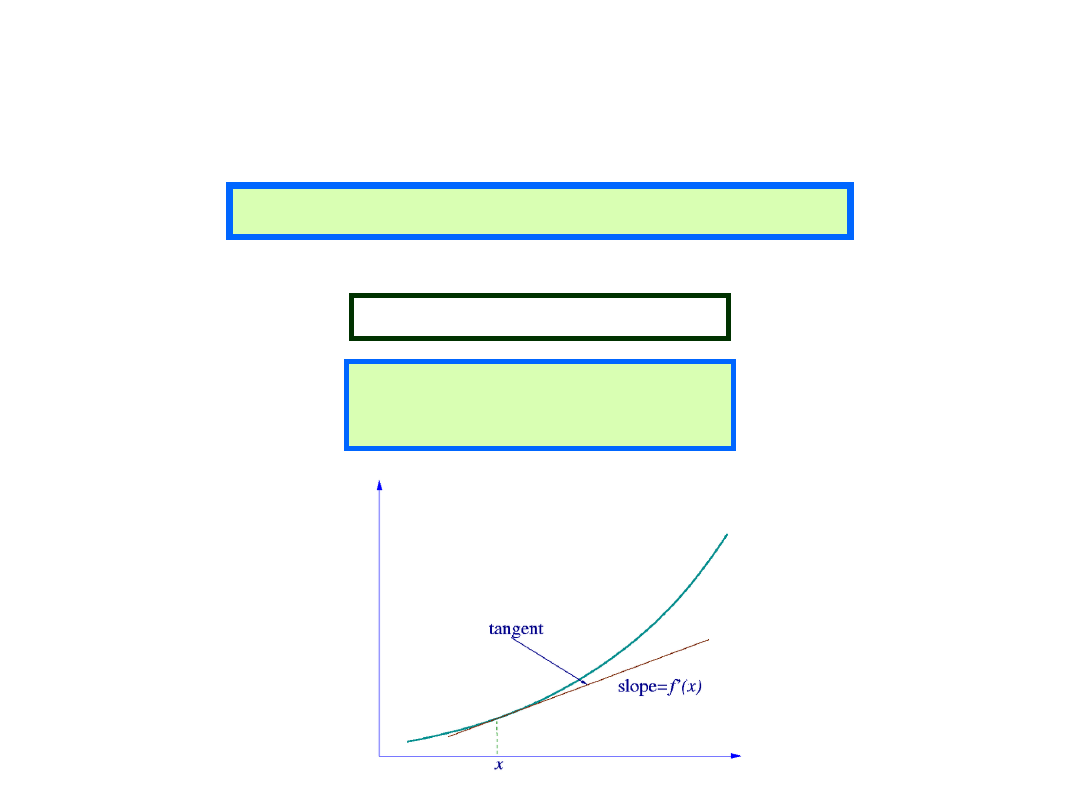
Tangent Line

The equation of the tangent line to the graph of
f at (c, f(c)) has slope f’(c) and equations.

)

c

x

)(

c

(

'

f

)

c

(

f

y

:

line

Tangent







)

c

)

c

(

'

f

)

c

(

f

(

x

)

x

(

'

f

y







c

)

c

(

'

f

)

c

(

f

b

),

x

(

'

f

m

,

b

x

m

y











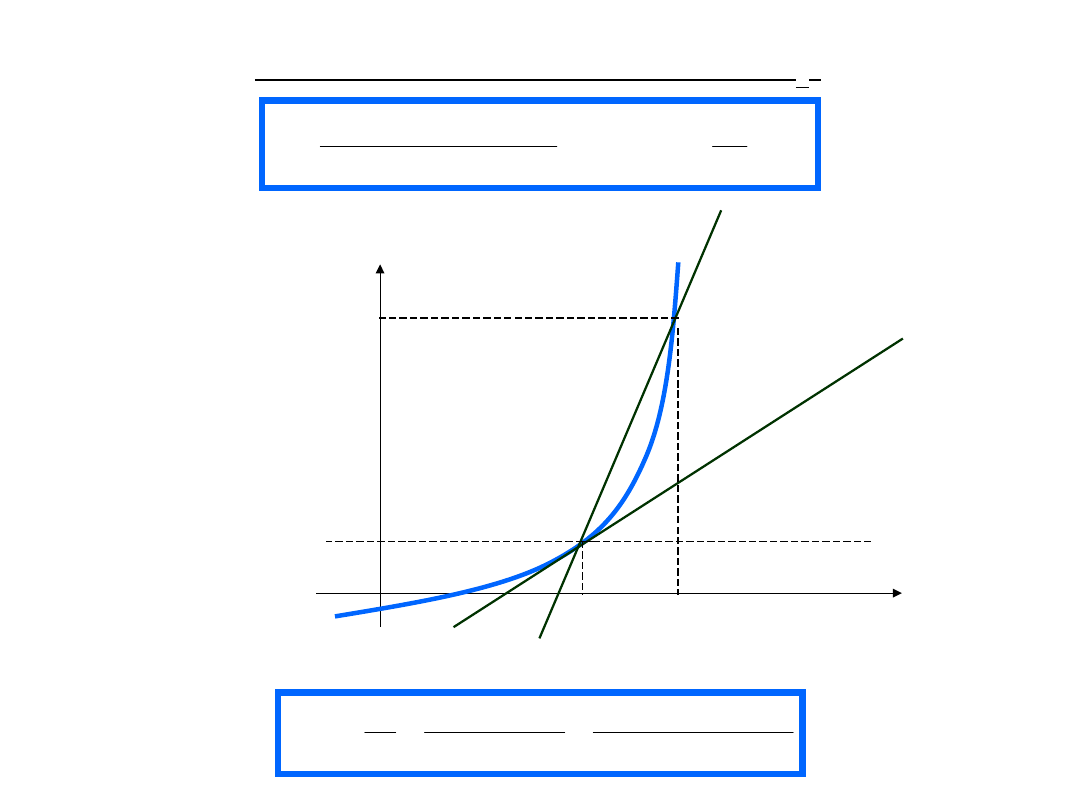
When a derivative does not exist...

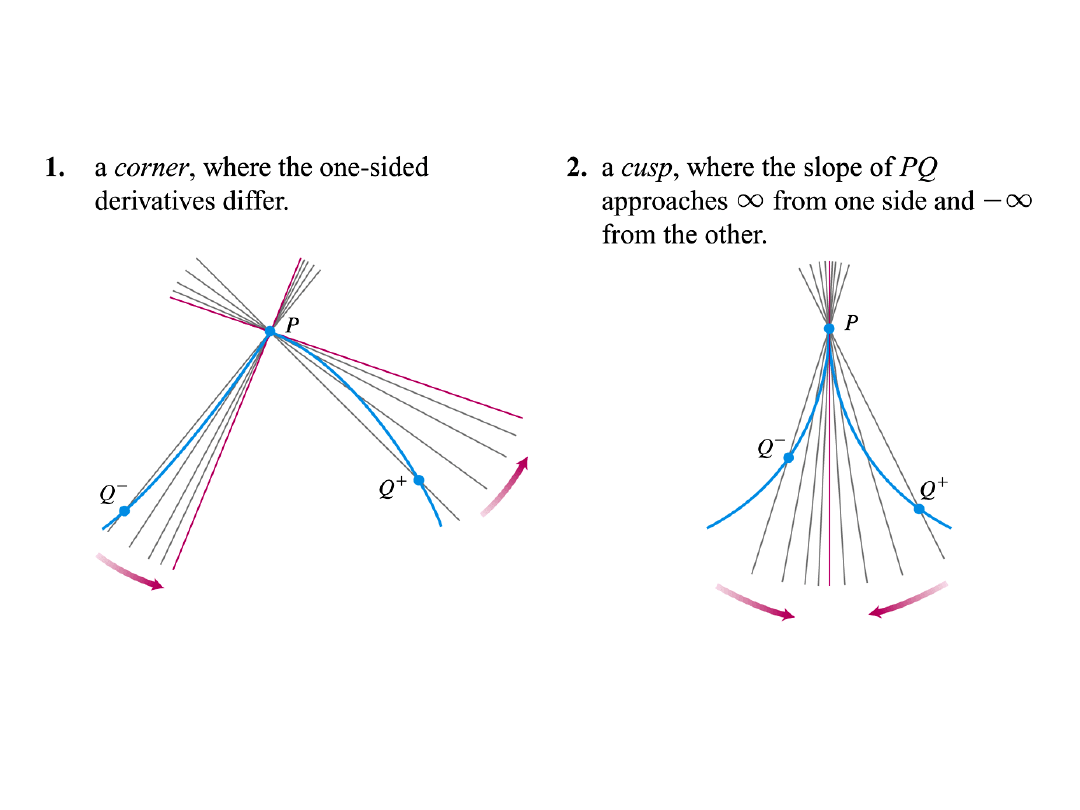
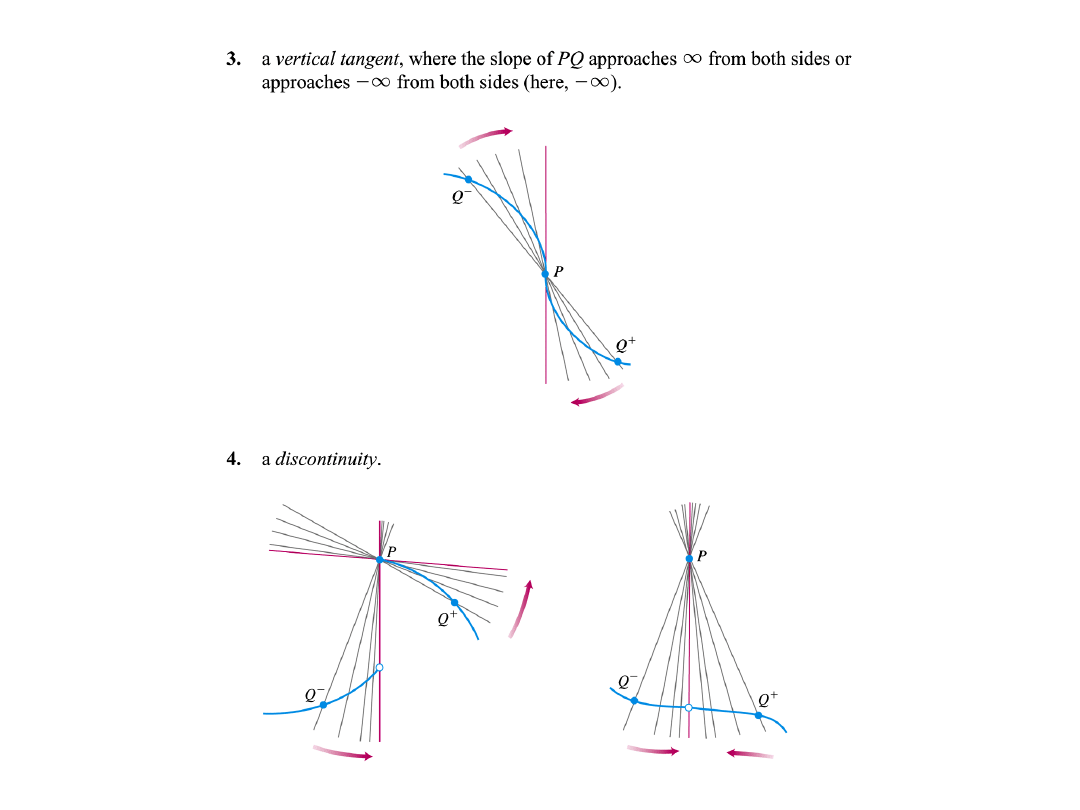
Differentiabiltity Implies Continuity

If f has a derivative at x = c, then f is continuous x = c

Examples of derivatives





h

x

h

x

h

x

h

)

x

(

f

)

h

x

(

f















0

2

0

2

0

0

0

2

 

2

x

x

f







0

0

0

0

0

0

2

2

x

h

x

lim

h

)

x

(

f

)

h

x

(

f

lim

h

h















Find the
derivative of

at point x

0

.

Example - how to find the derivative using the definition:

Find the derivative of at x

0

= –3, 0, 5.

 









2

0

2

0

2

0

0

3

0

3

0

0

0

3

3

3

x

h

h

x

x

lim

h

x

h

x

lim

x

'

f

h

h



















 

3

x

x

f



   

27

3

3

3

2









'

f

 

0

0

3

0

2







'

f

 

75

5

3

5

2







'

f

Example - how to find the derivative using the definition:

(C)’ = 0

The derivative of a constant is zero

c

y 

0





y

x

y 

1

'

y

x

y sin



x

y cos

'

x

y cos



x

y

sin

' 



x

a

y 

a

a

y

x

ln

'

x

e

y 

x

e

y 

'

For all

R

x

BASIC DERIVATIVES

n

x

y 

1

'





n

nx

y

,

1

'

1

,

2

1

)'

(

2

x

x

x

x



















The derivative of a

x

and the number „e

”

.

1

1

lim

0







h

e

h

h

e

a

a

a

x

x

log

1

)'

(



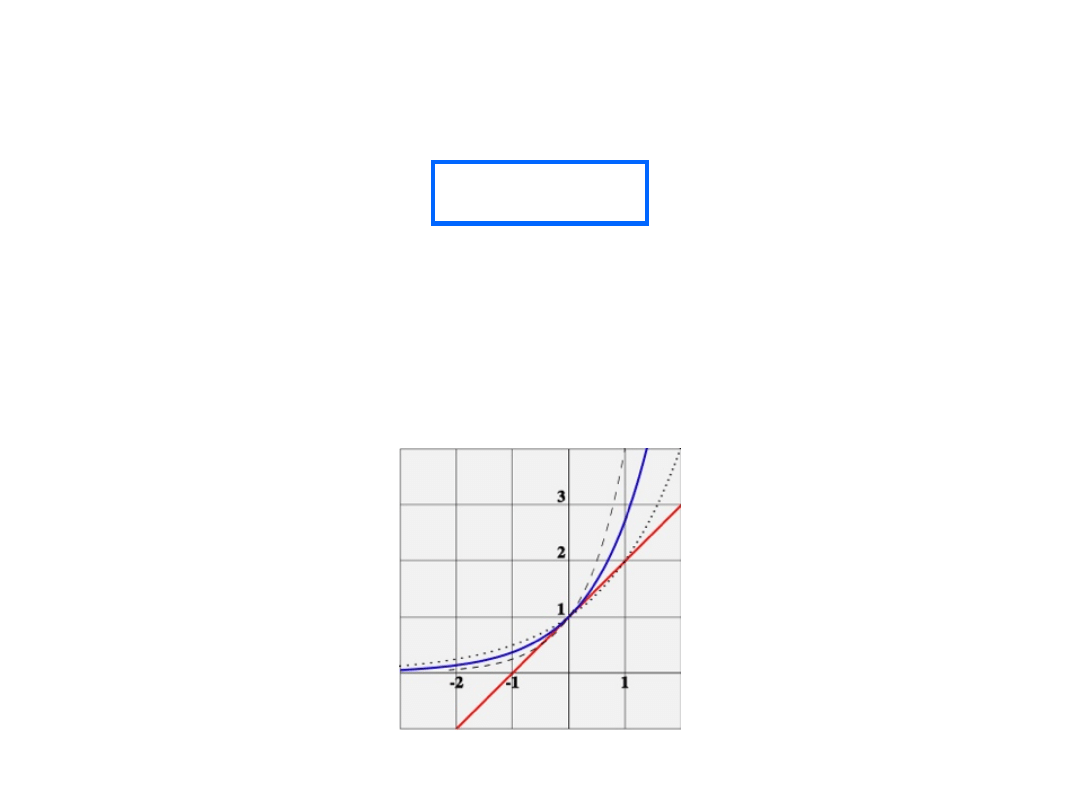
The number ‘e’ is the real number such that the
slope of the tangent line to the graph of the
exponential function y = e

x

at x = 0 is 1.

(e

x

)’ = e

x

If we take a = e
then

 

         

 

         

 

          

 

 

















Rule

Chain

)

x

(

'

g

)

x

(

g

'

f

)

x

(

g

(

f

.

Rule

Quotient

g

f

'

g

g

'

f

g

f

.

Rule

Product

'

g

f

g

'

f

g

f

.

'

g

'

f

g

f

.

'

f

c

cf

.

'

'

'

'

'



















































5

4

3

2

1

2

Basic Properties and formulas

If f (x) and g(x) are differentiable functions (the derivative exists),
c is any real number,

   







 



   

h

x

g

x

f

h

x

g

h

x

f

x

g

x

f

h















 0

lim

'



 



  



  



   

h

x

g

x

f

h

x

g

x

f

h

x

g

x

f

h

x

g

h

x

f

h

























 0

lim





   





   

x

f

h

x

g

h

x

g

h

x

g

h

x

f

h

x

f

h

h























0

0

lim

lim

   

   

x

f

x

g

x

g

x

f









'

'

Proof of the Product Rule

QED





g'

f

g

'

f

g

f

'











 

 









 

 

h

x

g

x

f

h

x

g

h

x

f

x

g

x

f

h





















 0

lim

'



  

  



  



h

h

x

g

x

g

h

x

g

x

f

x

g

h

x

f

h



















 0

lim



  

   

   

  



  



h

h

x

g

x

g

h

x

g

x

f

x

g

x

f

x

g

x

f

x

g

h

x

f

h



























 0

lim





   





 

 

  



h

h

x

g

x

g

x

f

x

g

h

x

g

x

g

x

f

h

x

f

h

























]

[

]

[

lim

0

   

   

 

2

)

(

'

'

x

g

x

g

x

f

x

g

x

f









Proof of the Quotient Rule

QED

2

'

g

f

g'

g

'

f

g

f





















dx

du

v

dx

dv

u

uv

dx

d





)

(

2

v

dx

dv

u

dx

du

v

v

u

dx

d

















OTHER NOTATIONS

For this problem, we must take the

derivative of the

outside

function

, then

multiply by the derivative of the inside

function

.

But the derivative of the inside function is also a chain rule
question.
So, we end up with the following:

f(x) =
sin(cos(x

2

))

f ‘(x) = cos(cos(x))·(- sin(x)) 2x

The Chain Rule - example

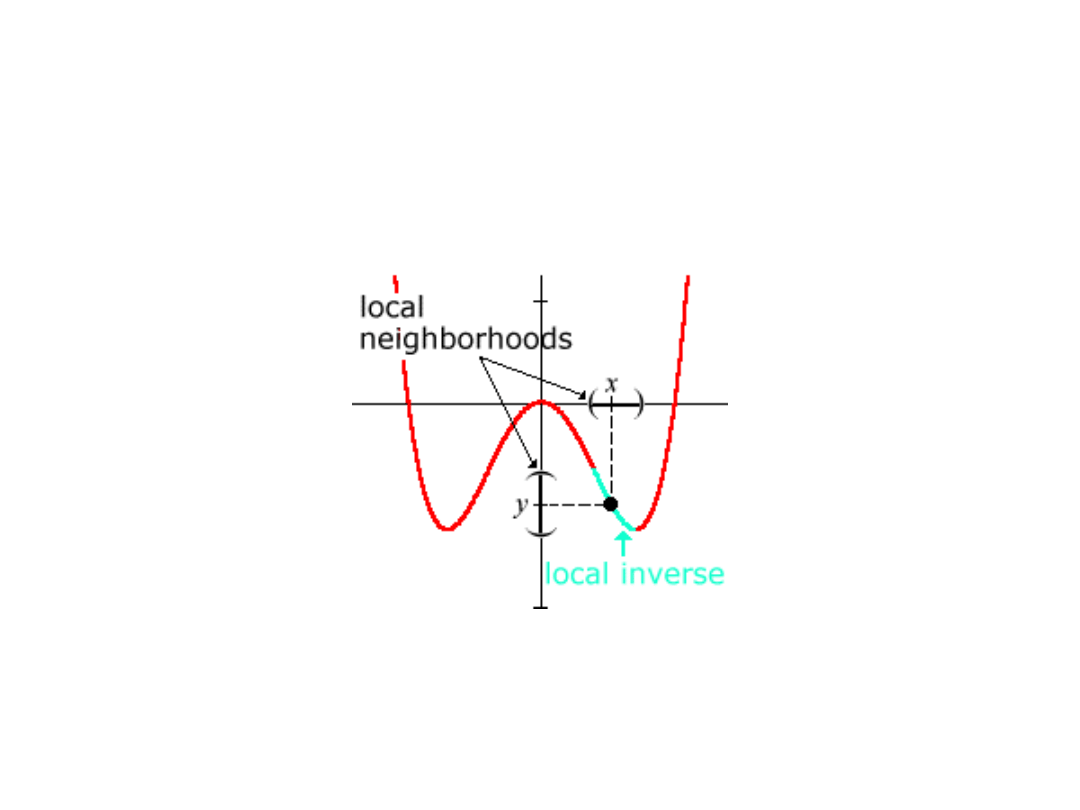
Suppose that a function f is continuous and strictly monotone
in the neighbourhood O(a) of point a.
If f ’(a) exists and is never zero, then f

-1

is differentiable at b = f (a)

(a = f

-1

(b)),





)

(

'

1

)

(

'

1

)

(

)'

(

1

1

a

f

b

f

f

b

f























du

dx

dx

du

1

or

DERIVATIVE OF INVERSE FUNCTION

)

b

(

f

x

b

x

df

dx

dx

f

d

1

1

1











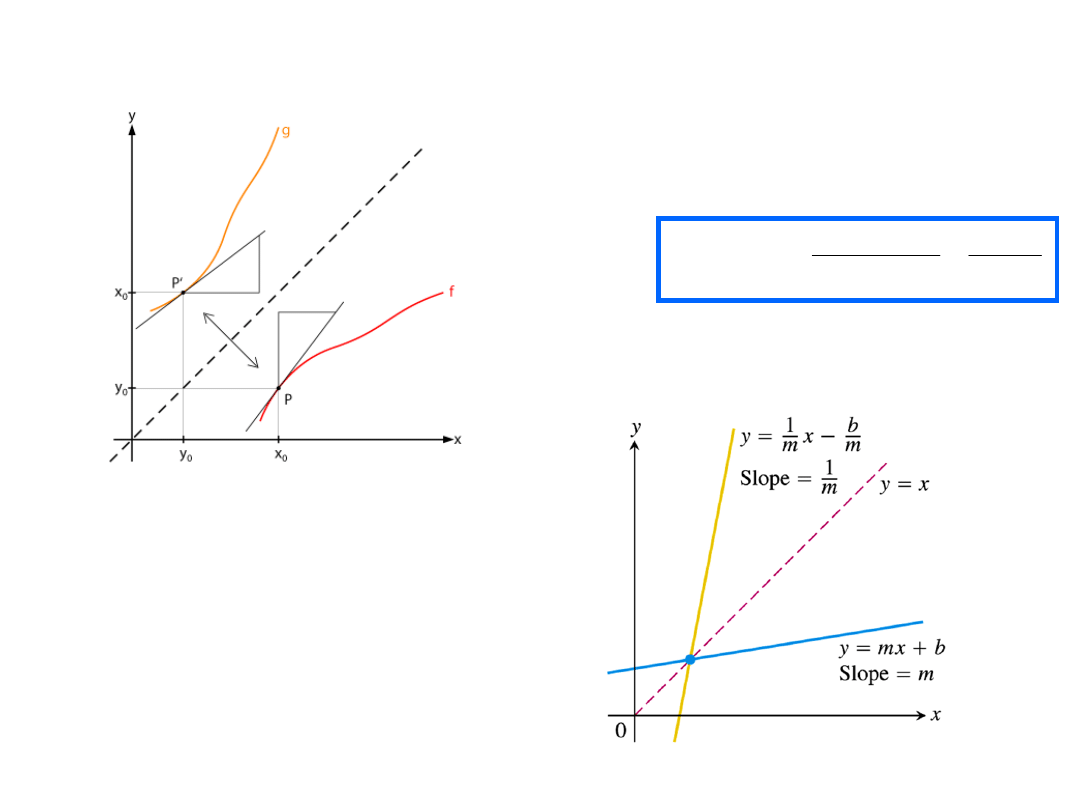
DERIVATIVE OF INVERSE FUNCTIONS





 

0

0

1

0

1

x

'

1

)

y

(

'

1

)

y

(

)'

(

f

f

f

f









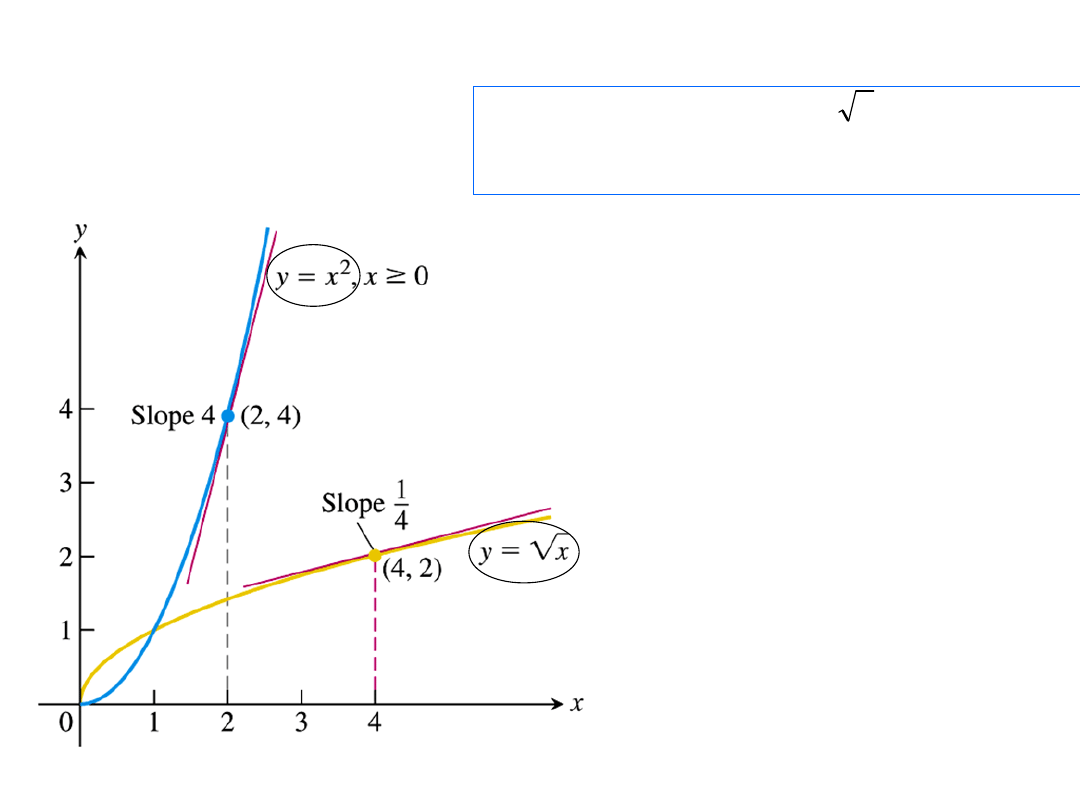
The derivative of f

-1

(x) = at point (4,2)

is the reciprocal of the derivative of f (x) = x

2

at (2,4).

x

x

arc

y

cos



x

y

a

log



x

y ln



x

arc

y

sin



x

arc

y

tg



x

arc

y

ctg



a

x

y

ln

1

'

x

y

1

'

2

1

1

'

x

y





2

1

1

'

x

y







2

1

1

'

x

y





2

1

1

'

x

y

















x

a

a

0

1

,

0

0



x

2

2

1

1





















y

x















y

x

0

1

1















2

2

y





 y

0

BASIC DERIVATIVES CD.





2

2

1

1

sin

1

1

cos

1

'

sin

1

)'

sin

(

x

y

y

y

x

arc













y

y

2

sin

1

cos







we choose

y

y

2

sin

1

cos

























2

;

2





y

0

cos 

y

Because

out of the possibilities

 

a

x

a

a

a

x

y

y

a

ln

1

ln

1

1

)'

(log

'







PROOFS





2

2

2

1

1

tg

1

1

cos

'

tg

1

)'

tg

(

x

y

y

y

x

arc

















2

2

2

2

2

2

1

1

1

1

cos

sin

sin

sin

'

1

)'

(

x

y

ctg

y

y

y

y

ctgy

arcctgx





























2

2

1

1

cos

1

1

sin

1

'

cos

1

)'

cos

(

x

y

y

y

x

arc



















PROOFS

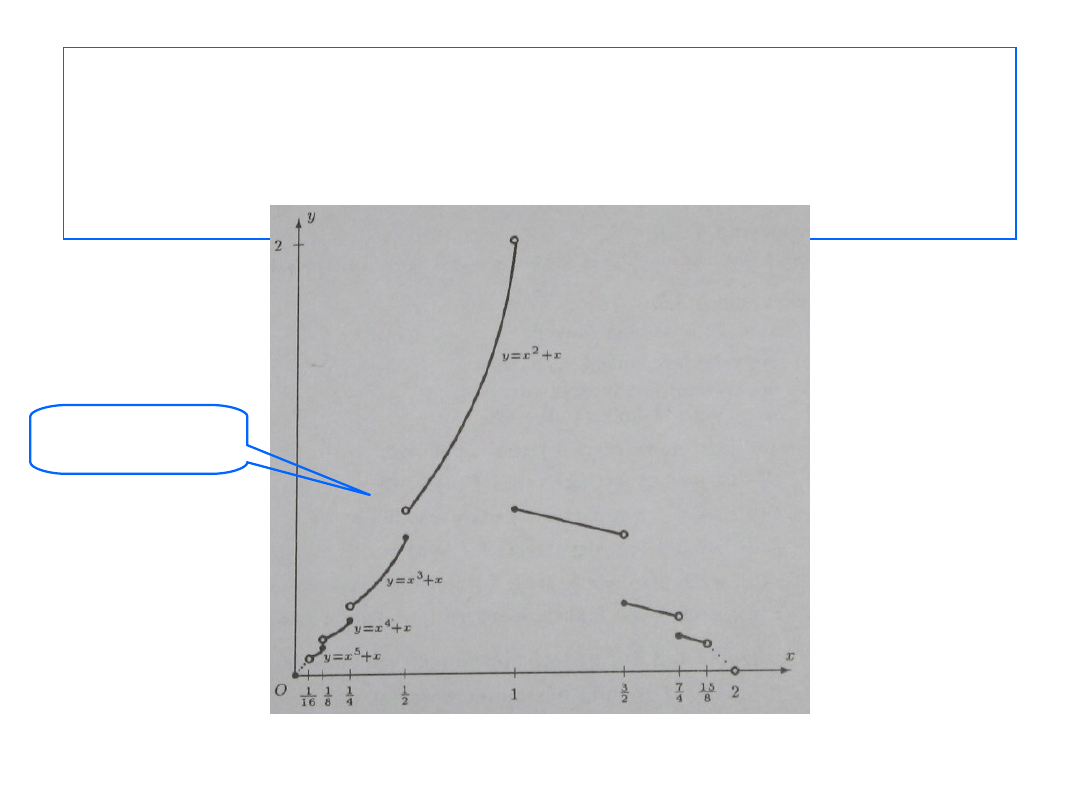
Example of a function not strictly monotone, continuous at
x = 0, f (0) = 0
with a nonzero derivative f’(0) = 1 and

f

-1

(x)

does not

have a derivative

at 0, because f

-1

(x) is not continuous

there.

M. Gewert, Z. Skoczylas, ‘Analiza matematyczna 1, Definicje, twierdzenia wzory’

The function is
defined as a
uniform limit

NOTE

NOTE
The derivative does not have to be continuous.

If the derivative of f (x) exists
then this implies that f (x) is
continuous.

Proof

A continuous function for which the

derivative

exists and the derivative is not continuous.

(a)

f(x) is differentiable and

(b)

f’(x) is not continuous

-0.1

-0.05

0.05

0.1

-0.004

-0.002

0.002

0.004

-0.1

-0.05

0.05

0.1

-0.075

-0.05

-0.025

0.025

0.05











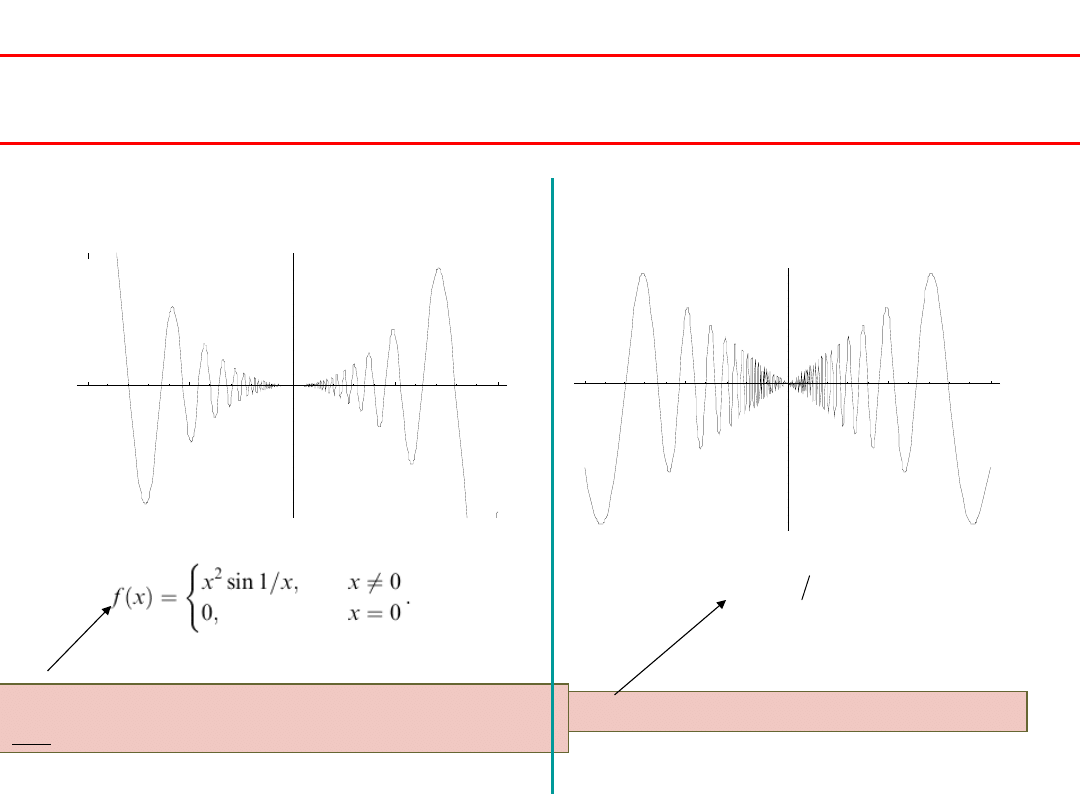
0

x

0

0

x

x

1

x sin

f is continuous, but has no derivative

f is continuous, has a derivative, but derivative
is not continuous

The derivative of a continuous function doesn't have to be continuous.
We cannot calculate f'(x

0

) by simply calculating f'(x) and putting x →x

0

.

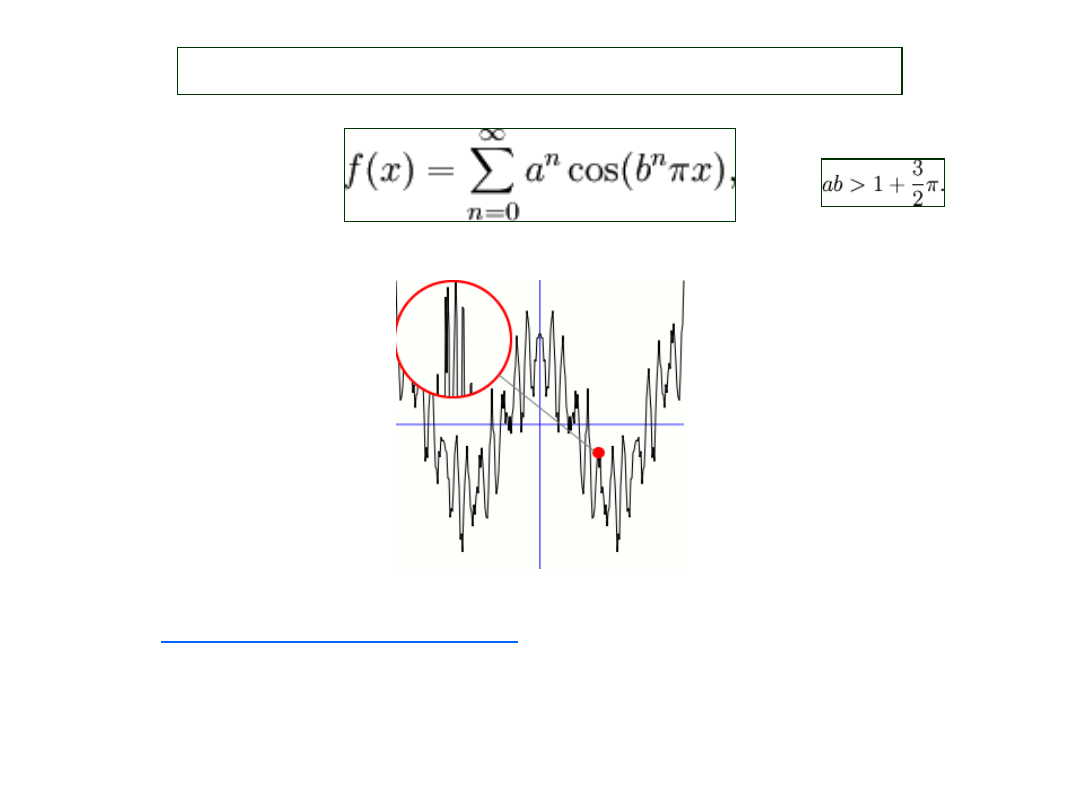
A continuous nowhere differentiable function.

Plot of

Weierstrass Function

over the interval [−2, 2]. The

function has a fractal behavior: every zoom (red circle) is
similar to the global plot.

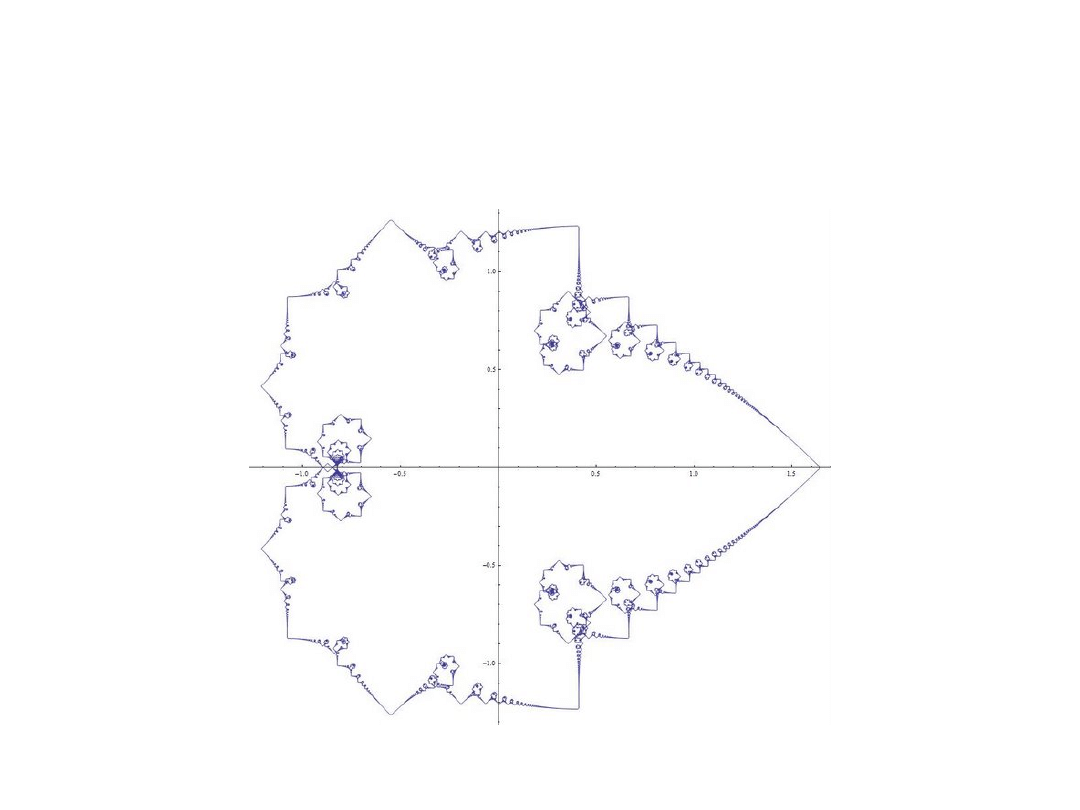
Weierstrass Function in the complex plane

THE MOST IMPORTANT DERIVATIVES (once more)

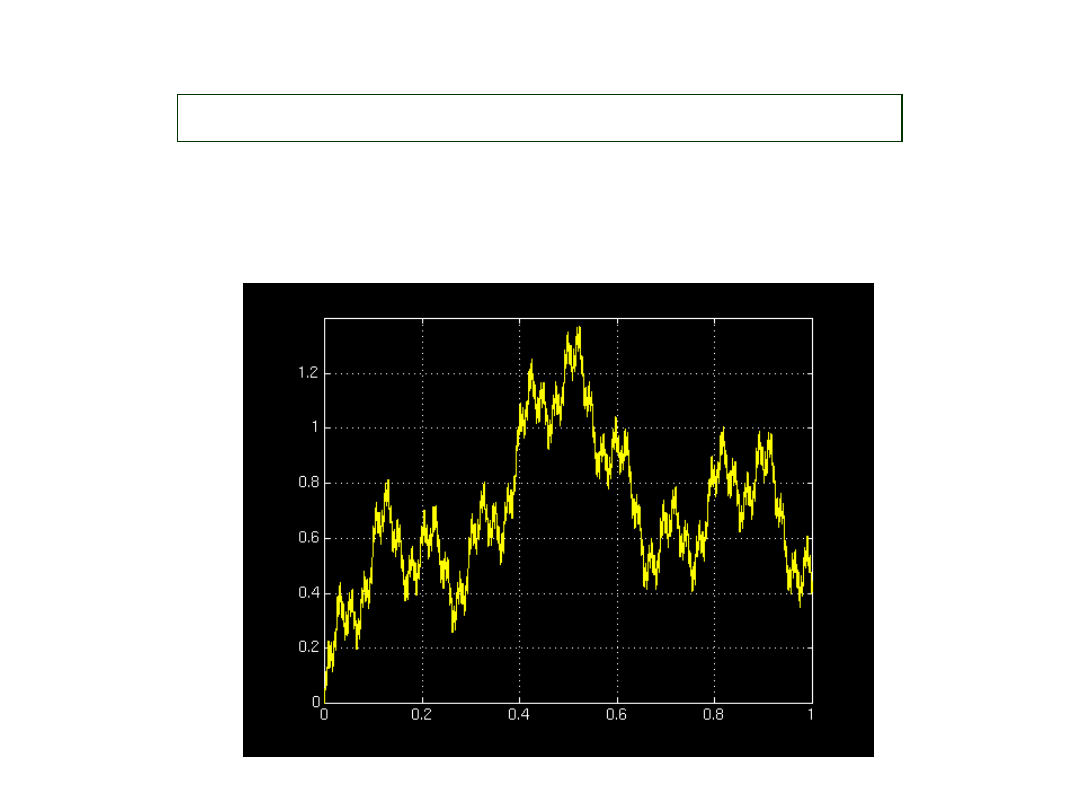
A continuous nowhere differentiable function.

A saw-like function with infinite teeth:

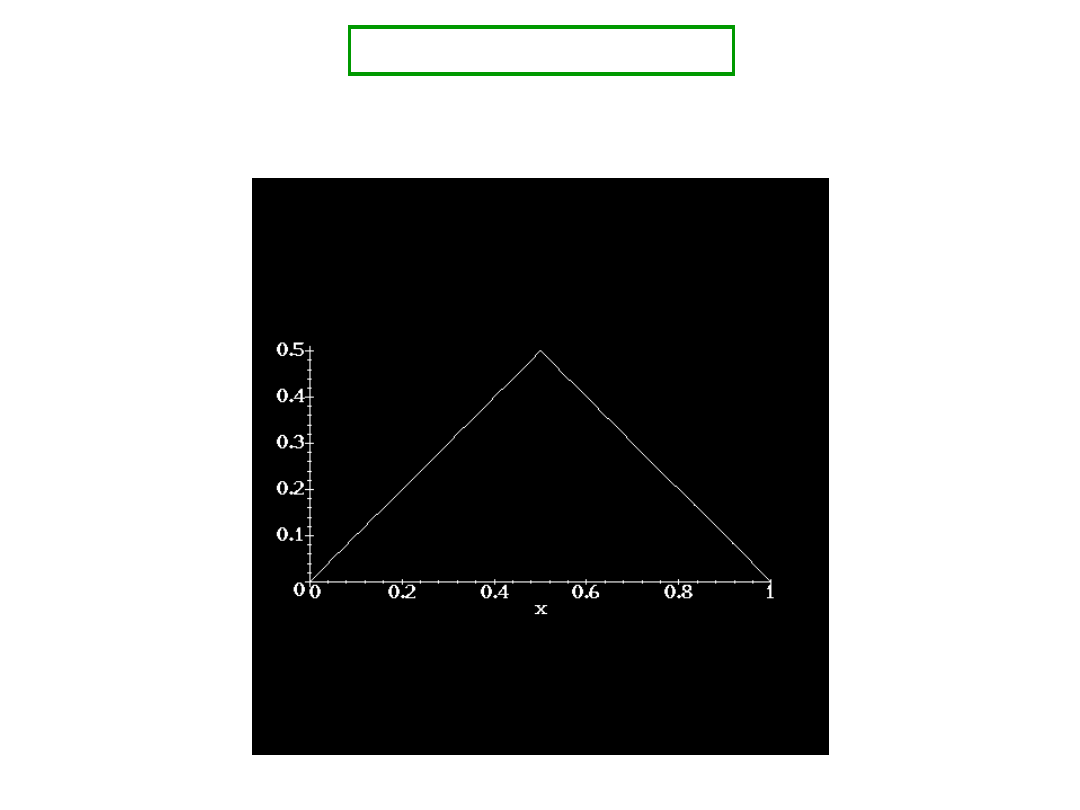
First define a saw-tooth function f(x) to be the distance from x
to the integer closest to x. Here's a plot of f:

SPECIFIC DEFINITION

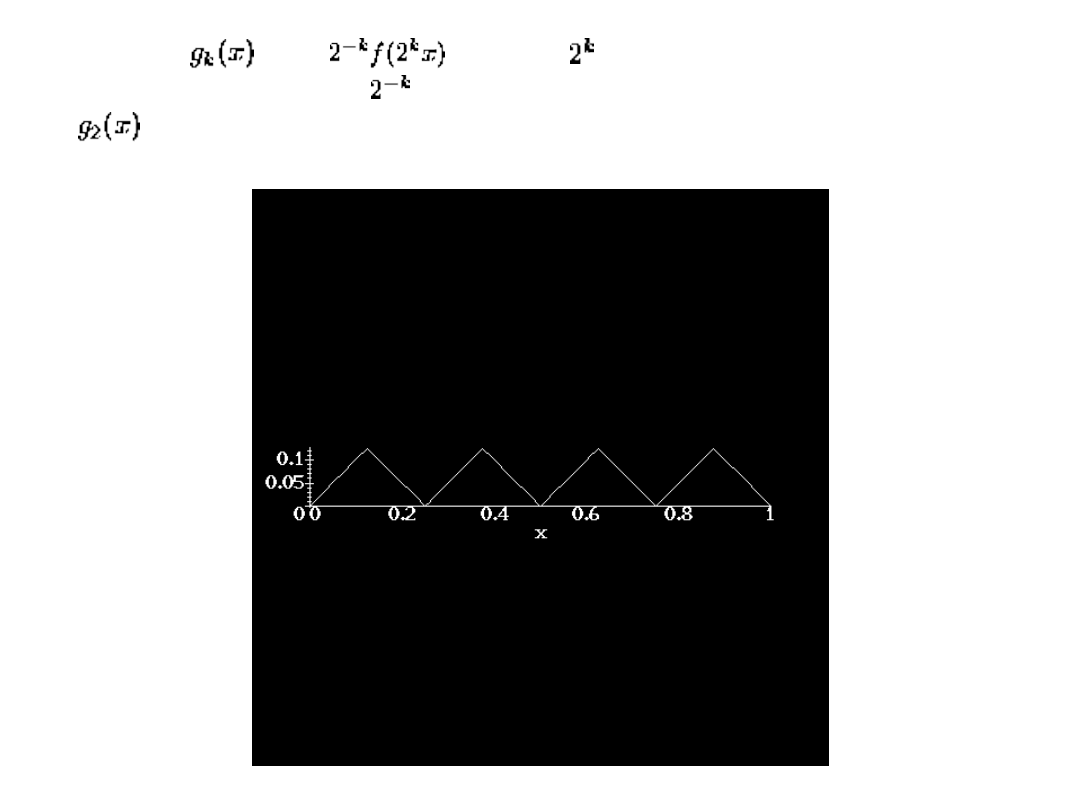
Now define       to be            . This has   as many ‘teeth’ as
f per unit interval, but their height is     times the height of the
teeth of f. Here's a plot of       , for example:
 

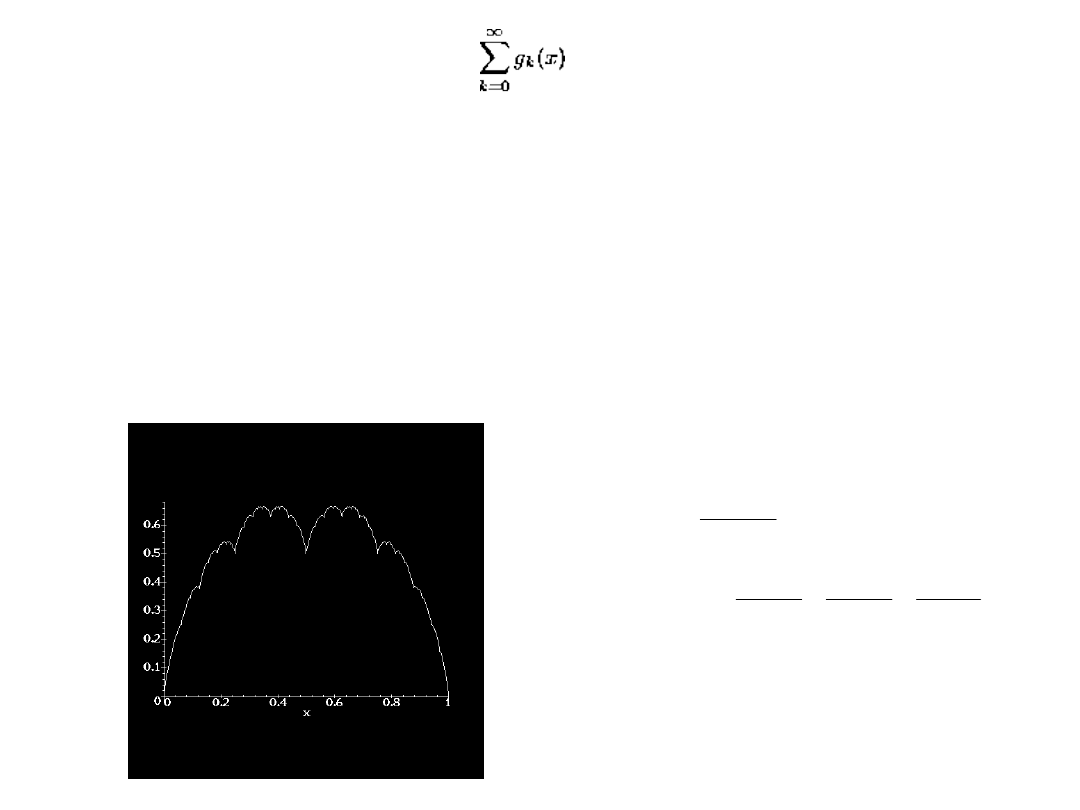
Finally, define h(x) to be the sum . For every x this
sum converges by

comparison with a geometric series.

It's already beyond elementary calculus to show that h(x) is
continuous (to advanced calculus students: h(x) is the sum of a
uniformly converging series of continuous functions, hence
continuous).
For the proof that h(x) is not differentiable:
the rough idea is that at every step we add more and more
corners. Here's a plot of h(x): (actually only a partial sum
rather than the infinite sum).

( )

( )

( )

( )

( )

( )

0

2

2

2

4

8

2

4

8

n

n

n

g

x

f x

g x

g x

g x

g x

�

=

=

=

+

+

+

+

�

L

Blancmange Function

It is an example of a fractal, in that it is infinitesimally
fractured, and self-similar. No matter how much you
zoom in on a point on the graph, the graph never
flattens out into an approximate non-vertical line
segment through the point.