STICKS & STONES NUMBER 5 

A leaflet for the left hand

 

Logical Twist 

Although Bob Walker independently developed this approach, credit 
must go to Daryl Martinez for the plot "twist" on Twisting the Aces. 
His version appears in Paul Harris Reveals Some Of His Most 
Intimate Secrets (1976) as "Twisted Aces". Walker worked out his 
approach as still another logical extension of what he calls the Crux 
Effect. He has developed these versions into an extensive 
monograph which may appear on the market in the near future, 
thus giving Twisters something to squirm about. Watch for it. 

EFFECT - The four Aces are shown and held face-down in the 
performer's left hand. Three of the Aces, each in turn, magically flip 
face-up. The last Ace vanishes and reappears face-up in the middle 
of the deck. 

WORKING 

1) Walker worked out two opening phases, each having features to 
recommend it. The purpose of the opening phase is to show the 
Aces and secretly get one of them reversed in the center of the 
deck. Martinez, by the way, uses a technique I call the Divided Bold 
Atfus. Students of his routine will understand the title. Instead of 
this questionable handling, Walker uses Marlo's Bold Atfus as one 
opening phase. This no-nonsense technique is very efficient and 
gets the job done. (See Kabbala Three or Marlo's Magazine for a 
description of this move.) The second possible opening phase uses 
another Marlo technique. To wit: 

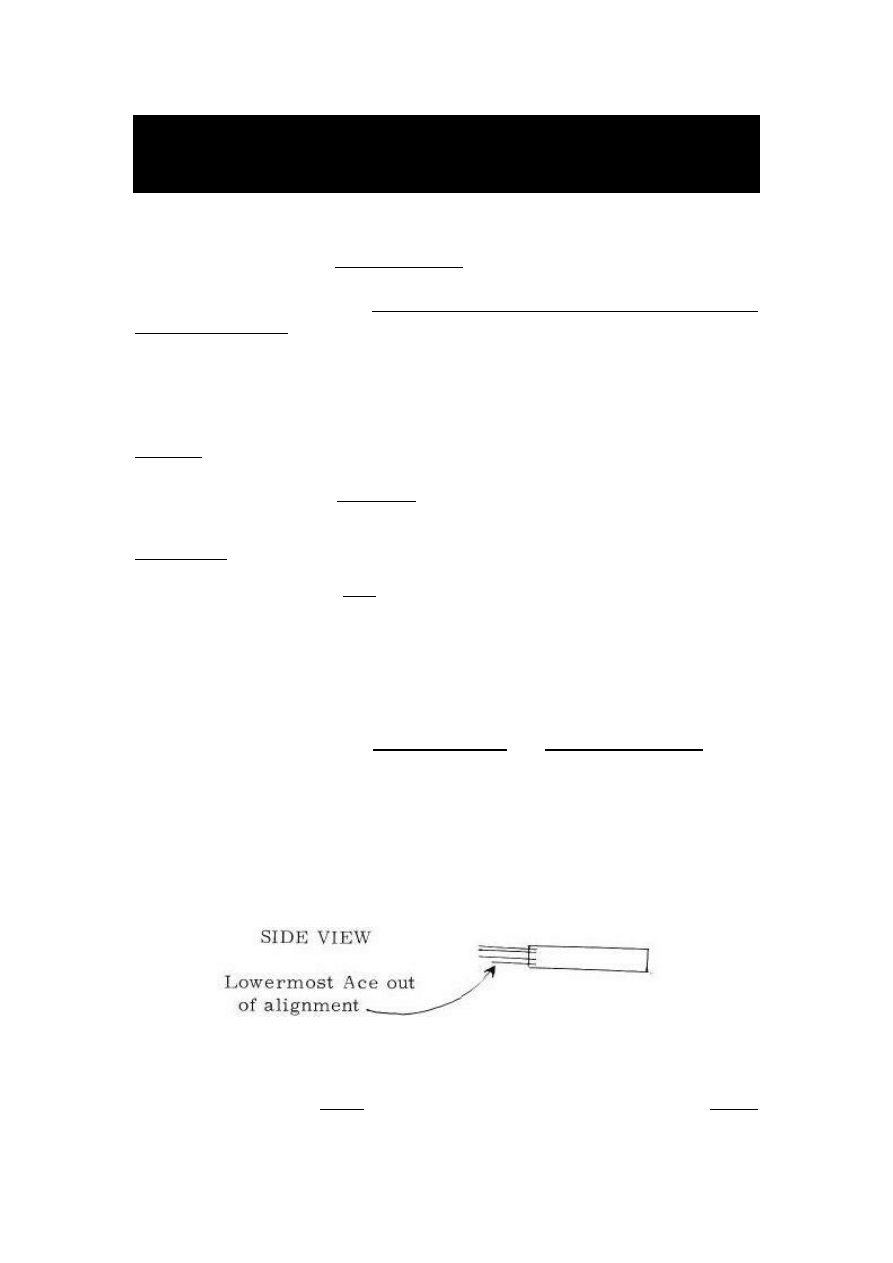
Upon getting a shuffled deck, spread the cards face-up between 
your hands. Up-jog each Ace when getting to it until all four Aces 
are culled. When you up-jog the final Ace, don't bring it up as far as 
the other three. Close the spread, leaving the Aces out-jogged as 
shown below-- 

 

 

Here your right hand comes over to pivot the out-jogged Aces 
clockwise to the right. As this is executed your left forefinger pushes 
the lowermost Ace flush with the deck. You now have only three 
Aces in your right hand, whereas the audience assumes you have 

all four. Table the deck and explain your premise: the magical 
flipping quality of the Aces. 

2) For purposes of this description let's assume that the AS is face-
up in the center of the tabled deck. The order of the remaining 
Aces--now face-up in your left hand—from the top is: AD - AC - AH. 

3) Flip the cards face-down as you say, "It would be impossible for 
me to hide the flipping of cards... like this... Execute a Double 
Turnover to disclose the AC as you add: "...whether the card is one 
or many..." 

4) Turn your left hand palm downwards (Glide position) as your 
right hand openly slides (actually glides) the AC out, turns it face-
up, and replaces it underneath. This action sequence is from the 
Vernon routine, only three cards are used instead of four. 

5) Turn your left hand palm up, revealing backs, and give the cards 
an Elmsley-type count. Explain that the AH has magically turned 
face-up. Retain the last card of the "count" in your right hand. Turn 
it face-up and show the AC. Under the misdirection of this display, 
your left hand once again turns palm downwards. This causes the 
left-hand pair of cards to turn over. Since they are face-to-face, a 
back still shows and the audience should be none the wiser. 

6) Replace the shown AC face-down below the left-hand cards, then 
adjust the packet for another Elmsley-type count. This time the AD 
will show during the "count". Place the last card on the bottom, 
retain a break, then execute Krenzel's Mechanical Reverse. As soon 
as the Reverse is completed, your left fingers slide out the 
lowermost card (AD) so that it's side-jogged to the left. Both red 
Aces will be disclosed. Say, "...that takes care of the red Aces... 
Now for the black Aces..." 

7) Flip the packet face-down and do an Elmsley-type count to show 
the face-up AC. Repeat the "count". This time, however, out-jog the 
AC and place the last card on top. Remove the face-up AC and turn 
it face-down on top. Repeat the "count" (3 cards as 4) with all the 
cards face-down as you say, "The Ace of Spades is the most difficult 
card... It doesn't turn face-up... It disappears!" Here you spread the 
three cards and toss them face-up onto the table. Ribbon spread 
the deck to conclude. 

- Robert Walker, (as told to Jon Racherbaumer) 

RACHERBAUMER NOTE: After Step 5 you can eliminate the 
Mechanical Reverse by doing the following: 

Replace the shown AC face-down below the left-hand cards as 
already explained, then adjust the packet for another Elmsley-type 

count. Do the "count" and place the last card on top. The AD will 
show up during the count and will end face-up on the bottom. 

Do the Through-the-Fist Flourish, thus secretly turning the cards 
over. Flex the. cards and openly remove the top card, revealing the 
face-up AC. Place the removed card on the bottom and immediately 
do a Double Turnover. Do an Elmsley-type count to apparently show 
four (?) face-down cards. 

Conclude as per "Logical Twist". While this version works, it has 
some obvious drawbacks. It is, however, angle-proof. 

"TOO PERFECT" Imperfect? 

My first reaction to Rick Johnsson's "The 'Too Perfect' Theory" is a 
question --to which I do not necessarily expect an answer: Does Mr. 
Johnsson perform magic regularly for laymen or is he one of the 
"for magicians only" specialists? I ask this because his supposed 
analysis of a spectator's reactions when watching a trick (p. 248, 
paragraph 3) is a perfect example of magician's thinking, yet there 
is no evidence to suggest that the lay spectator's thoughts follow 
such pattern. Indeed, on the basis of many years of performing 
exclusively for lay audiences, I would say that spectator reactions of 
the sort described by Mr. Johnsson will rarely be encountered 
outside of magic clubrooms or, perhaps, the Magic Castle, neither of 
which are very typical performing situations. 

RACHERBAUMER INTERJECTION: So begins Bill Zavis's rebuttal to 
Rick Johnsson's controversial article found in Hierophant 5-6 
(reprinted by Lou Tannen). This article stimulated several replies, all 
addressed to me. No other articles appeared, pro or con, in other 
magazines. Strange? 

In future issues of Sticks & Stones I plan to personally express my 
views on Johnsson's uncommon theory. Interested readers should 
find a copy of Johnsson's article. If anyone cares to comment 
further, please write me in care of the address included at the end 
of this issue. Now back to Zavis... 

Our theorist (Johnsson) opines that SOME TRICKS, BY VIRTUE OF 
THEIR PERFECTION, BECOME IMPERFECT (his caps, not mine). He 
then states, "As we have already tested the first half of the 
theory..." If this is logic, make mine superstition! His sole "test" is 
his wholly speculative "analysis," as unconvincing as it is 
unsupported, referred to above. It is true that most people seem to 
require some sort of explanation for the phenomenon they 
encounter, but it is equally true that few people require very 
detailed explanations to set their minds at rest. The lay spectator is 
rare who analyzes a trick in detail in order to discover the 

method...unless, of course, an ill-conceived or incompetently 
executed performance has partly exposed the method, giving the 
audience reason to speculate in detail; and heaven knows there are 
far too many ill-conceived and incompetently executed 
performances of magic these days, the average so-called magician 
being no more fitted to perform in public than any other untrained, 
amateurish actor. The lay spectator certainly realizes that the 
magician is playing "tricks" on him, but if these tricks are played 
with a modicum of skill, wit, grace, and charm, and if they are 
presented as entertainments rather than puzzles, the average 
audience seems quite content to let itself be entertained and 
puzzled, seldom worrying about detailed methods or whatever. 

In short, there is every reason to believe that a lay audience 
expects to be deceived by a magician and accepts that it will be. If 
not, why in heaven's name engage or go see a magician? 

Is there, then, such a thing as a "too-perfect" trick? I doubt it. The 
card trick Mr. Johnsson cites as an example certainly should not 
qualify as one. If anything, it is already sufficiently imperfect 
because its presentation is illogical, which might be part of the 
trouble Mr. Johnsson has with it. If all you are going to do is tell the 
spectator  what  card  he  looked  at,  there  is  no  reason  to  have  him 
look at the cards at all. If cards are used for the selection, they 
should be used for the revelation as well. (In fairness, it appears 
from his subsequent remarks that Mr. Johnsson realizes this, at 
least instinctively; it is his theory that I find nonsensical.) The 
perfect version of the trick would be to tell the spectator what card 
he merely thought of, with no cards being used. Here, of course, we 
enter the realm of the mentalist, whom Mr. Johnsson seems to have 
excluded from his general theory of deception. What good is such a 
theory if it cannot be universally applied? 

Perhaps the best answer to Rick Johnsson's illogic is to be found in 
his own words in the third paragraph from the end of his article. He 
acknowledges that many performers have been successfully 
presenting "too-perfect" effects for years, and explains this by 
assuming that "they were done at carefully chosen, psychologically 
correct times (with an abundant supply of acting ability thrown in 
for good measure)..." 

I'm sure they were. That is a good description of the correct 
approach for performing any trick, "perfect" or otherwise; though I 
don't know why acting ability should be relegated to a parenthesis. 
Perhaps it is the lack of such considerations, among others, that has 
set Mr. Johnsson to worrying about too-perfect tricks. Certainly 
such lacunae accounts for some of the poor performances I've seen 
over the years. Performers would do far better to concentrate on 

their imperfections rather than to worry about imagined 
hyperperfections in their tricks. 

- William Zavis 

 

 

Interpretation of a Dream 

July 18-20, 1976 

EFFECT - A card is selected and lost in the deck through shuffles 
and cuts. The cards are ribbon spread face-up. One card appears 
face-down. The spread is flipped over, but the reversed card is NOT 
the selection. The cards are scooped up and respread in a face-up 
condition. When the reversed card is removed and shown it proves 
to be the selection! 

Edward Marlo devised "a couple of methods to this conditional 
problem. I originally posed the problem in Talisman (Vol. 2-No. 19) 
in 1971. The conditions of the problem are crucial. Here are the 
exact words from Talisman: 

THE DREAM 

The Problem: A card is selected and apparently lost during 
subsequent shuffles and cuts. The deck is ribbon spread face-down. 
All the cards are apparently face-down. The ribbon spread is flipped 
over, using the conventional flip-over flourish. Once the ribbon 
spread is face-up, a single face-down card appears in the center of 
the spread. The spread is flipped over again to disclose the face-
down card. It is NOT the selection. It remains in place. The ribbon 
spread is scooped up and respread. The face-up selection has now 
changed into the selection. IMPORTANT CONDITIONS: The 
APPARENT handling should be very casual-looking with no delays, 
intermittent cuts, or illogical pauses. The deck should be borrowed 
and the performer, if using gaffs or other aids, should be clean at 
the end. It's permissable to scoop up the spread and respread 
rather than use the flip-over flourish. However, it would be more 
startling to have everything happen during the flip-over flourish. 

After this card problem appeared, no solutions immediately 
appeared. Finally some so-called solutions appeared in 1976 in a 
publication called Innovative Magic #1. The solutions, drafted by 
Jim Cozzens, Keith Best, and Roger Smith, deviate from the 
conditions set forth in Talisman. Cardmen commonly commit this 

error: they exceed the restrictions and stretch the boundaries and 
ignore detailed conditions of given problems. These elements are 
important; they characterize and specifically define the problem-
puzzle. Eliminate or alter these elements and you nullify the 
problem. 

Marlo's methods adhere to conditionals set forth in Talisman. As 
Freud interpreted real dreams, Marlo interprets cardmen's dreams... 

WORKING 

1) You need a double-faced card. Assume the gaff is AH/2H. If the 
cards are borrowed, secretly add the gaff to the bottom (face). Also 
slightly wax the gaff, i.e. put enough magician's wax to cause 
another card to adhere while simultaneously making it easy to 
separate such pairs. Experiment until you produce a formula that 
works for you. 

In any case, you begin the effect with a waxed double-facer on the 
face with its waxed side outwards. 

2) Have a card selected and contrive to get this selection reversed 
on the bottom. There are many ways to do this and experienced 
cardmen will have no trouble. Example: Have the spectator peek a 
card. Hold a break, then simply pull the card down and around the 
right side of the pack. Your right hand, holding the deck from above 
and by the ends, covers the action. The action itself automatically 
reverses the selection. 

3) Cut the cards and slightly squeeze, thus causing the selection to 
adhere to the double-faced card. Turn the deck face-up and ribbon 
spread the cards. A face-down card will show (back of selection). 

4) Flip the spread face-down and reveal the face (?) of the reversed 
card(s). The face of the double-faced card will show, proving to be 
the "wrong" card. Ask, "Is that your card?" When you receive a 
negative answer you have two options: 

(1) Scoop up the cards, turn them face-up, and 
respread. 

(2) Execute another flip-over flourish. 

Believers in the "Too Perfect Theory" will choose option 
(1). Believers in Marlo will choose option (2). 

5) After the above action the cards will be face-up with a single 
face-down card in the center. The audience will assume it's the 
same "wrong" card. 

6) Reach over with your right hand to slide the face-down card out 
of the spread. This card, of course, is the actual selection (still 
waxed to the double-faced card). You must separate the cards, then 
slide out and toss the face-down card onto the table. 

7) Ask, "What was the name of your selection?" When the card is 
named, add: "I thought that was the card I just showed you?" Here 
you disclose the tabled card as the selection. 

(Note: Separating the waxed cards can be a problem. Experiment 
and don't use too much wax. It's better to slide the cards apart 
instead of lifting or prying them apart. The latter technique "talks" 
and would tip off the situation to discerning eyes. 

Marlo also has a Second Version using the Cover-Up Cut, which 
more or less speaks for itself.) 

- Edward Marlo, July 18-20, 1976 

Tally-Hokum 

Audiences like anecdotal effects wherein the story is important and 
magical elements, if any, are secondary. 

"Jack the Bellhop" and the "Gentleman Soldier's Prayer Book" are 
not magical. They are stories and playing cards are used as visual 
aids. The deck is obviously set-up. 

Effects like "Wild Bill Hickok's Last Hand" and "Einstein and the 
Magician" are successful attempts at combining a story with 
elements of magic. One of the best combinations is found in "The 
Hotel or Motel Mystery". 

Here's the point: Audiences will remember the story long after 
they've forgotten the magic. 

"Tally-Ho!" is a puzzler. That is, spectators would have difficulty 
trying to reconstruct how each "spell" is determined. It's not, 
however, breath-taking magic. Henry Christ had another objective 
in mind: to entertain audiences with an exotic, plausible story. 
Harry Lorayne, publishing the routine in Reputation-Makers, 
conveys this objective by contributing a "presentation attitude". He 
also added a sleight-of-hand kicker. 

Gene Castillon worked out a version, including the Lorayne kicker, 
that's SELF-WORKING. 

SET-UP: Remove the Sixes and Nines from the deck. Place the Sixes 
on top of the talon. Cover them with an X card, then place the Nines 
on top. 

WORKING 

1) This version is a CONTINGENCY method, i.e. subsequent 
procedures are dependent on which word is chosen FIRST. There 
are only four words to choose; however, only two contingent 
procedures need to be memorized. 

The four words in the Christ routine are A. DOUGHERTY. TALLY-HO, 
PLAYING, and CARDS. The contingent procedure for CARDS and A. 
DOUGHERTY is the same. Likewise for TALLY-HO and PLAYING. 
Needless to say, the reader must be familiar with the original 
routine in Reputation-Makers (pp. 115-125). First study the original 
handling, then run through the Castillon version. 

2) If the spectator chooses to spell A. DOUGHERTY or CARDS, then 
proceed with contingent PROCEDURE #1: 

Perform the Christ routine exactly as outlined in Reputation-Makers. 
Once you've gone through the four spells and five packets are on 
the table, assemble them as Lorayne describes. 

When you re-spell the principal words, instead of dealing the cards 
into one pile, keep the cards used to spell each word in SEPARATE 
face-down piles. After disclosing the Nines, turn over the TOP cards 
of each packet to reveal the SIXES. 

ALTERNATE ENDING: Instead of the Separate Pile approach, deal all 
the "discards" of the spell into one pile. After disclosing the Nines, 
turn over the top card of the discards to reveal a Six. Toss it face-
up onto the table. Spell N-I-N-E and turn over the "E" card to reveal 
another Six. Toss it face-up onto the other Six. Spell L-I-N-O-I-D 
and turn over the "D" card to reveal a 3rd Six. Toss it aside. Spell F-
I-N-I-S-H, turn over the "H" card and reveal the last Six. 

3) If the spectator chooses to spell TALLY-HO or PLAYING, then 
proceed with contingent PROCEDURE #2: 

Perform the Christ routine until you've assembled the five packets; 
then deal all the discards into ONE PILE. 

After disclosing the Nines, pick up the Discard pile and spell L-I-N-
O-I-D. Turn over the "D" card, reveal a Six and toss it face-up onto 
the table. Drop the talon onto the dealt cards. Pick up the 
assembled cards. 

Spell F-I-N-I-S-H, turn over the "H" card to reveal another Six, and 
toss it aside. Drop the talon onto the dealt cards. Pick up the 
assembled cards. 

Spell T-H-E-U-S-P-L-A-Y-I-N-G-C-A-R-D-C-O (written on the side of 
the card case) and turn over the NEXT card to disclose another Six. 

Toss it aside and drop the talon onto the dealt cards. Pick up the 
assembled cards. The final Six is now 9th from the top. 

Using the "9" on the card case as an indicator count down to the 
9th card and reveal the last Six. (Racherbaumer Note: Instead of 
the "9" bit, spell F-A-N-T-A-S-T-I-C as you say, "Isn't this 
fantastic!") 

ALTERNATE ENDING: After revealing the Nines, pick up the Discard 
pile and Double-Cut the bottom two cards to the top. In this phase 
you spell and always turn over the NEXT card. 

Spell L-I-N-O-I-D and turn over the next card--a Six. Toss it face-up 
onto the table. Leaving the dealt cards on the table, spell F-I-N-I-S-
H and deal the cards onto those already on the table. Turn over the 
next card to show another Six. Toss it face-up onto the tabled Six. 
Drop the talon onto the dealt cards. Pick up the assembled cards. 

Spell R-E-G-U-S-P-A-T-O-F-F (written under A. Dougherty), then 
turn over the next card to show a Six. Toss the Six face-up onto the 
others. 

Spell N-I-N-E and turn over the next card to show the last Six. 

- Gene Castillon, November - 1971 

 

STICKS & STONES is a JON RACHERBAUMER production. All 
characters portrayed, including the contributors, are real. The 
effects will be as good as you make them. The reality of the rest will 
depend on your paranoia or practicality. Send your thoughts, pro 
and con, to: 

P.O Box 1142, Metairie, Louisiana 70004 

Send encouragement and financial aid to Lloyd!