The method of symmetrical

components

2 / 48

Symmetrical system

Voltages

Currents

a U

a I

a e

- p

=- +

=- -

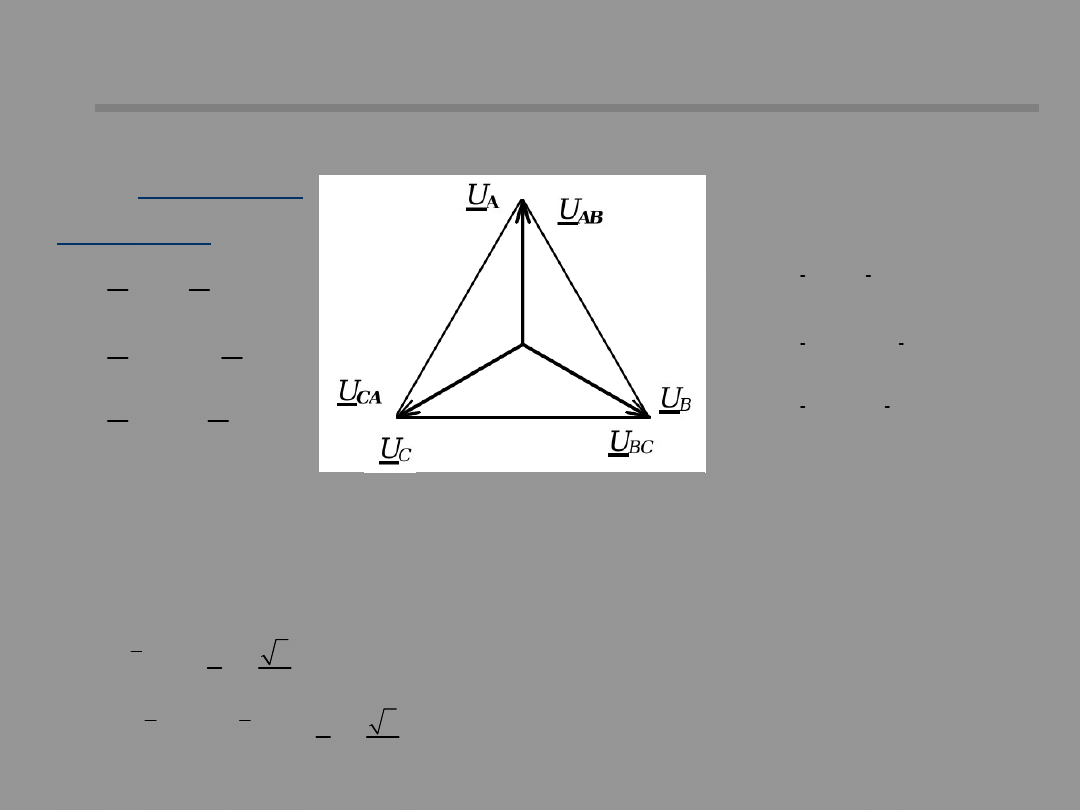
Phase notation: A, B, C lub R, S,

Vector diagram of voltages in three-

phase system

complex rotational

phasors:

3 / 48

Linear component

’



ELEMENT

TRÓJFAZOWY

OPISANY

MACIERZĄ Z



Equipment in power systems is represented by
equivalent circuits, which are designed for the
individual tasks of power system analysis. For the
calculation of short-circuits, the equivqalent
circuits iclude resistance and inductive reactance.
Shunt elements, i.e. capacitive reactance are
omitted.



The equivalent circuit has couplings in the three-
phase system which are of an inductive type.

4 / 48

The equations of linear component

RR R

RS S

RT T

Z I

= -

SR R

SS S

ST T

Z I

D = -

TR R

TS S

TT T

Z I

D =

U ZI

�

� �

�

� �

�

D = D

�

� �

�

� �

�

� �

�

� �

=��

� �

I
I

�

=�

�

The ralationship between voltages and currents of a
three-phase system can be described by the
following equations:

In a matrix notation

where

Matrix Z is the matrix of self-impedances and mutual
(coupling) impedances of the element. Self-
impedances Zii (i = R, S, T) are located on the main
diagonal, and mutual impedances outside it. For
symmetrical elements, the self-impedances of
individual phases are equal.

5 / 48

The equations of linear component

Mutual impedances between individual phases
depend on the type of element.



Static element (line, transformer, reactor) –
impedances are reciprocal:



Rotating element (electric motor, generator) –
impedances are circular:

Mutual impedances of a given phase in relation
to the other phases are not equal because
windings of these phases are differently
positioned in relation to the rotor.

Z Z

�

6 / 48

Impedance matrix

�

=�

�

= -

�

Thus the matrices of impedances can be written

if the form:

for a static component

for a

rotating component

Sign – (minus) indicates that currents in the other
phases induce in the given phase EMFs having the
opposite sense in relation to self-induction EMFs,
which results from the mutual location of stator
phase windings.

In the equations of 3-phase circuit the voltage loss in
each phase of the component is dependent on all
phase currents, and on the self-impedance of this
phase and the mutual impedances between this
phase and the other phases.

7 / 48

The equations of linear component

(

)

D =

s R

m R

Z I

Z a I

Z aI

Z Z I

(

)

D =

s S

m S

s S

m S

Z I

Z aI

Z I

Z a I

Z Z I

(

)

D =

s T

m T

s T

m T

Z I

Z a I

Z aI

Z I

Z Z I

For balanced load the set of equations
receives the form:

Voltage drop in each
phase depends only on
the current of this
phase – the equations
are
mutually independent
and Z impedance
matrix is diagonal.

�

Z Z

8 / 48

The method of symmetrical components

Let us consider the three-phase RL symmetrical circuit in

which currents and voltages may form unbalanced systems.

The multiplicity of couplings between phases complicates the

circuit analysis. Therefore, a mathematical transformation is

sought which transfers phase components to different

system. The following conditions should apply for the

transformation:



The transformed voltages should depend only on one

transformed current.



The transformation should be linear - the linear

relationship between current and voltage should be

retained.

The desired transformation (transformation 0, 1, 2) consists in

replacing the unbalanced systems of currents and phase

voltages by their symmetrical components, i.e. by the three

symmetrical vector systems – the zero-sequence (0), the

positive sequence (1) and the negative-sequence system (2).

9 / 48

The symmetrical component

transformation

The transformation diagonalising the matrix Z is introduced

D =

S U SZS SI

�

1 1 1

1 a a ;

1 a

1 a a

The matrix S is a third-order nonsingular
(determinant S ≠ 0) matrix, whose elements shall be
so selected that as a result of transformation S Z S

-1

the impedance matrix is diagonalised. Matrix S is
called

the

operator

matrix

symmetrical

components, and its element a – the complex
operator

- p

=- +

=- -

a e

10 / 48

Symmetrical components method

where I

– vector of sequence

currents,
ΔU

– vector of sequence

voltages:

D =

p p

U Z I

(0)

(1)

(2)

1 1 1

I I

1 a a

a I

1 a

a I

� �

+ +

�

��

�

� �

�

��

�

= =

� =

=� �

�

��

�

� �

�

��

�

��

�

� � �

I SI

(0)

(1)

(2)

1 1 1

1 a a

a U a U

1 a

a U a U

�

D +D +D

�

��

�

��

�

D = D =

�D

D + D + D

= D

�

��

�

��

�

D + D + D

�

��

�

� �

�

U S U

As a result of transformation the following
equation is obtained:

11 / 48

Transformation of impedances

(0)

(1)

(2)

1 1 1

1 a a

1 a

1 a a

Z 2Z

Z Z

0 Z

�

��

�

��

�

��

�

��

�

��

�

=�

�

� �

�

Z SZS



For a static component:

12 / 48

Transformation of impedances

(0)

(1)

(2)

1 1 1

1 a a

1 a

1 a a

Z Z

Z a Z

a Z

Z a Z

a Z

0 Z

�

�-

�

=�

�

Z SZS



For a rotating component

13 / 48

Symmetrical component method

Finally, the equations of 3-phase circuit may be
written in the form:

(0)

(1)

(2)

0 Z

�

� �

��

�

� �

��

�

� �

��

�

� �

��

�

��

�

(0)

(1)

(2)

(0)

(1)

(2)

0 Z

�

��

�

� �

��

�

��

�

� �

��

�

� �

��

�

The impedance matrix of symmetrical components is
diagonal.

Thus the coupled set of equations which described
the 3-phase circuit in phase variables becomes an
uncoupled set of equations in the symmetrical
components.

14 / 48

Interpretation of the symmetrical

components system

The reverse transformation of the 012-system to
the RST-system is achieved by the matrix S

-1

accordance with:

I S I U S U

(2)

(2)R

(2)

(2)S

(2)

(0)

(0)R

(0)

(0)S

(0)

(

(1)

(1)R

(1)

(1)S

(1)

(

(2)T

)

I
I

a I

= + + =

+ =

(2)

(2)R

(2)

(2)S

(2)

(0)

(0)R

(0)

(0)S

(0)

(

(1)

(1)R

(1)

(1)S

(1)

(

(2)T

)

a U

Reference

phase

The vectors of phase currents and voltages are

the linear combination of sequence

components.

15 / 48

The invariance of power in 012-system

S U I

U I

U I

* *

T T

U I

U S S I

U I

( )

T T

U S

( )

* *

S I

( ) ( )

(

)

S 3

3 U I

U I

U I

The transformation from RST-system to 012-

system is not invariant with respect to power.

In 012-system:

In RST-system:

because

Thus,

16 / 48

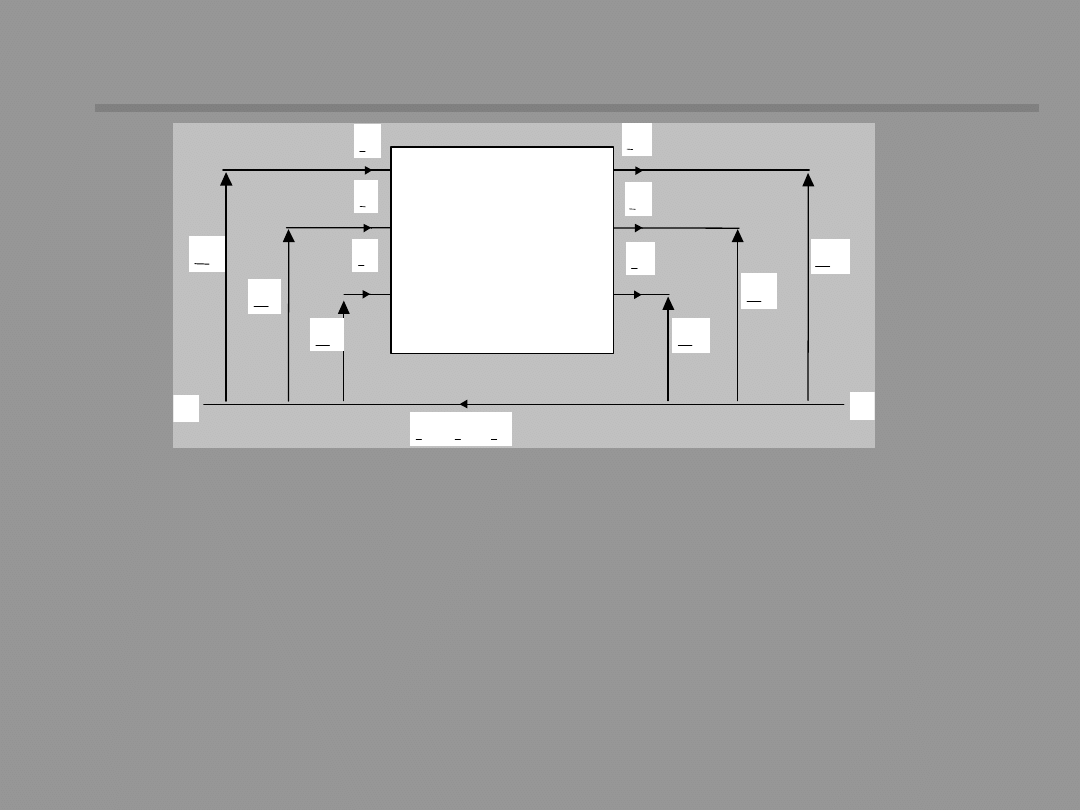
Equivalent circuits for symmetrical

components

’



ELEM

TRÓJFAZOW

OPISAN

ACIERZĄ



= +D +D

E U

Earthing impedance

The voltage equation of the

circuit:

17 / 48

Equivalent circuits for symmetrical

components

� �

=� �

� �

I
I

(

)

D =

+ +

u R

Z I

� �

=� �

� �

E
E

� �

=� �

� �

U
U

� � �

��

� � �

��

D = D

� � �

��

� � �

��

� �

�

� �

= + +

E U ZI Z I

SE SU SZS S I SZ S S I

= +

P P

uP P

Z I

012-transformation yields:

The voltage matrix equation
receive the form:

18 / 48

Equivalent circuits for symmetrical

components

The source EMF symmetrical components:

( )

� �

( )( )

�

0 0

1 1

2 2

SZS

�

=�

�

0 0

SZ S

The transformation of impedances:

for the

element

for the

earthing

19 / 48

Equivalent circuits for symmetrical

components

We assume that the source is

symmetrical:

a E

+ + =

(0)

a E

aE )

E (1 a

a) 0

(1)

aa E

a aE )

+ +

2 2

(2)

a a E

aaE )

E (1 a a ) 0

In such a case the symmetrical component of EMF are the following:

( )

� �

=� �

� �

Thus:

The EMF of sources form the

positive sequence system.

20 / 48

Equivalent circuits for symmetrical

components

( )

( )( ) ( )

(

)

( )

0 0 0

m 0

Z 2Z I

( )

( )( ) ( )

(

)

( )

1 1 1

m 1

Z Z I

( )

( )( ) ( )

(

)

( )

2 2 2

m 2

Z Z I

( )

u 0

3Z I

( )

u 1

( )

u 2

The equations of symmetrical components circuits:

( )

( )( ) ( )

( )

0 0 0

u 0

0 U

3Z I

( )

( )( ) ( )

1 1 1

( )

( )( ) ( )

2 2 2

0 U

( )

( )( )

(

)

( )

0 0

3Z I

( )

( )( ) ( )

1 1 1

( )

( )( ) ( )

2 2 2

Taking into account the Z

and Z

matrices yield the following equations for

voltage drop ΔU and ΔU

in symmetrical components:

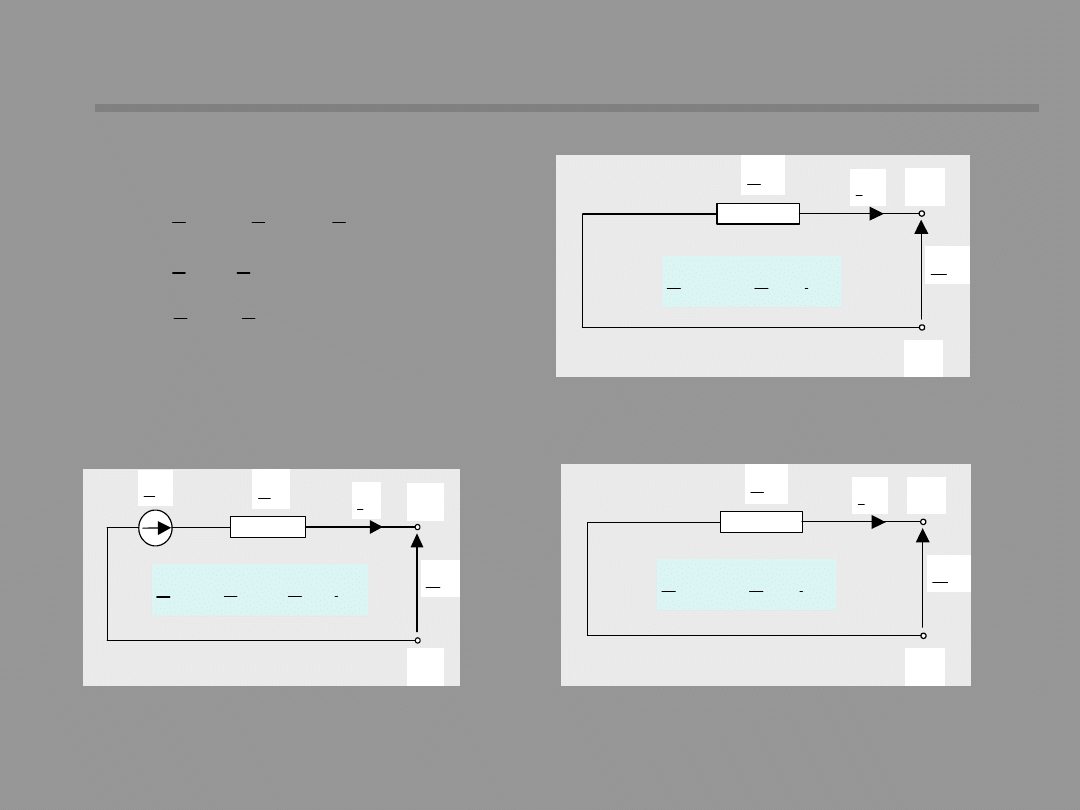
21 / 48

Single-phase sequence networks

 

(1)

 

(2)

 

(0)

( )

(

)

( )

( )( )

1 1

( )

( )( )

2 2

( )

( ) ( )

0 0

Z I

( )

( ) ( )

1 1

Z I

( )

( ) ( )

2 2

Z I

(0)

, Z

(1)

, Z

(2)

– equivalent impedances of network for zero, positive and

negative sequence components, respectively

Using the notation:

We can describe sub-

networks for each

symmetrical

component

22 / 48

Single line-to-earth short-circuit



Assumptions:

•

The network is unloaded before a fault

•

Phase R is short-circuited



Boundary conditions are:

( )

)

= =

( )

)

Using the inverse of

symmetrical

component

transformation

Using the

transformation

itself

These two expressions describe the sequence

network connection.

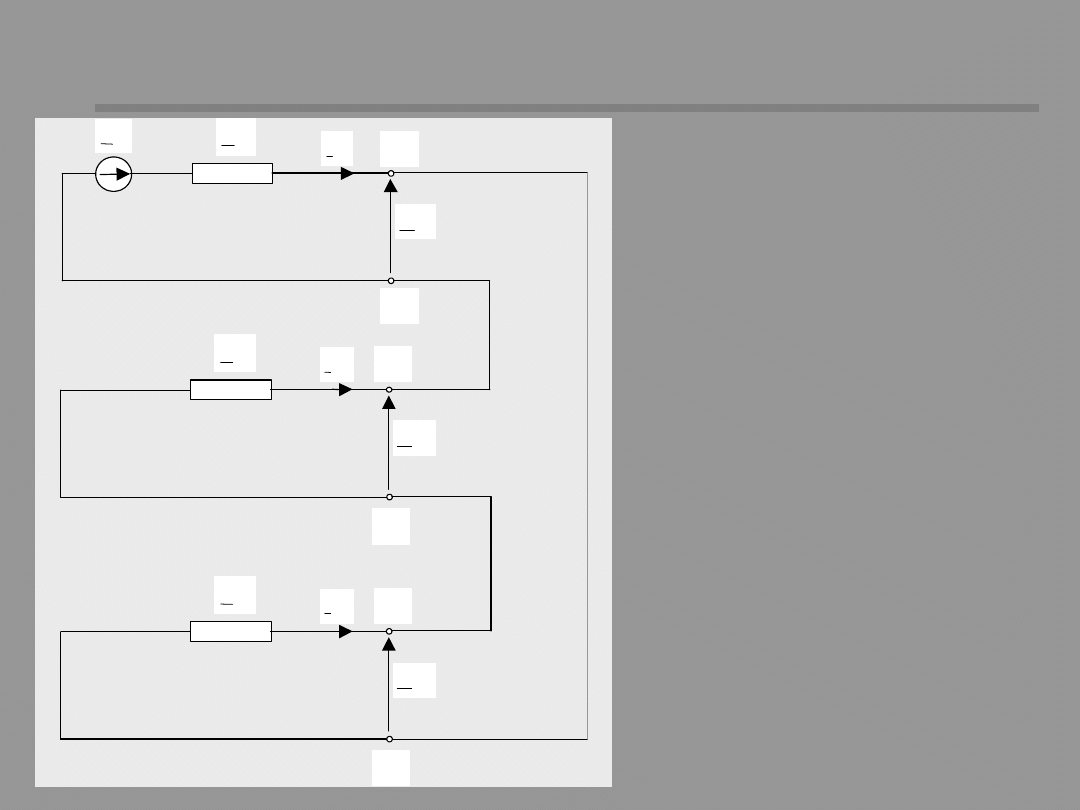
23 / 48

Single line-to-earth short-circuit

( )

( ) ( ) ( )

( )

= +

( )

= =

Using the sequence

network equations:

( )

( ) ( )

0 0

Z I

( )

( ) ( )

1 1

Z I

( )

( ) ( )

2 2

Z I

The condition 1) gives:

( )

( ) ( )

1 1

2 2

0 0

Z I

from

here:

Phase current can be

expressed by

Initial current

24 / 48



 



 

(1)

(2)

 

(0)

Single line-to-earth short-circuit

Sequence network
connection for single
phase short-circiut has
all three sequence
networks connected in
series.

25 / 48

Single line-to-earth short-circuit

( )

( ) ( )

( )

0 0

Z E

Z I

( )

( ) ( )

( )

1 1

Z I

( )

( ) ( )

( )

2 2

Z E

Z I

Voltage symmetrical

components:

Phase voltages:

( )

(

)

( )

a U

a a Z

a 1 Z

�

( )

(

)

( )

(

)

( )

a U

a a Z

a 1 Z

�

+ -

�

26 / 48

Single line-to-earth short-circuit

 

E 

 



 

a 

 

a

 

a

 

a 

 

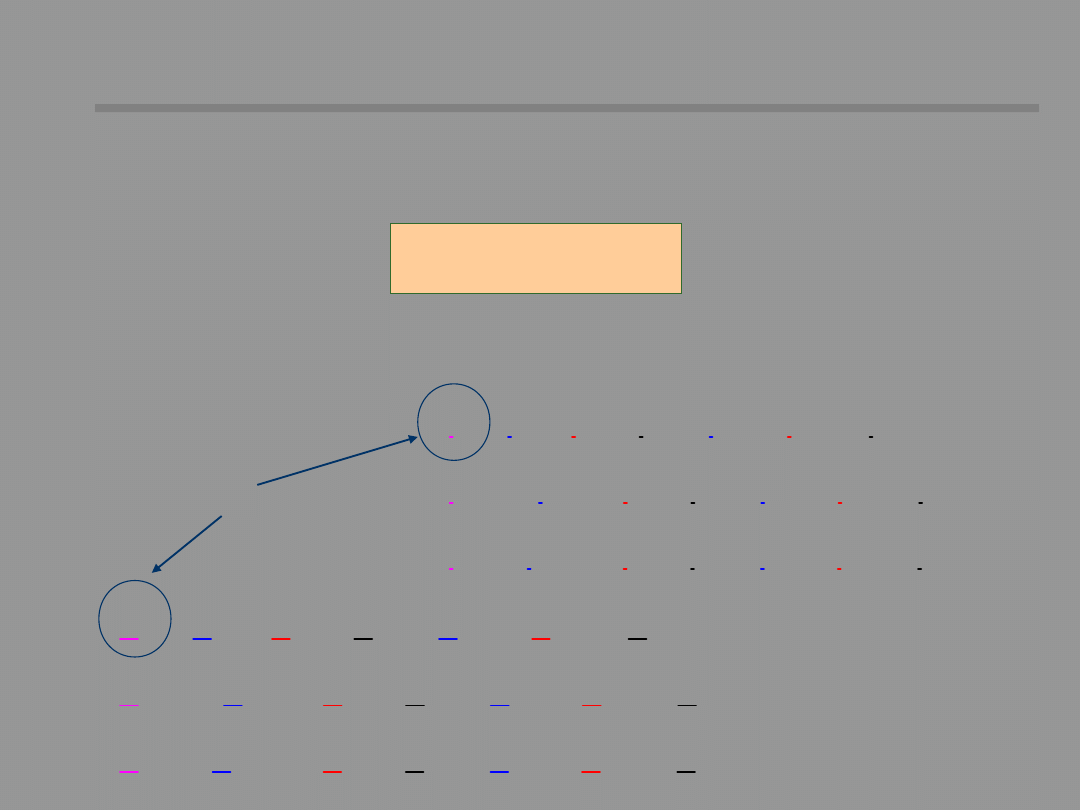
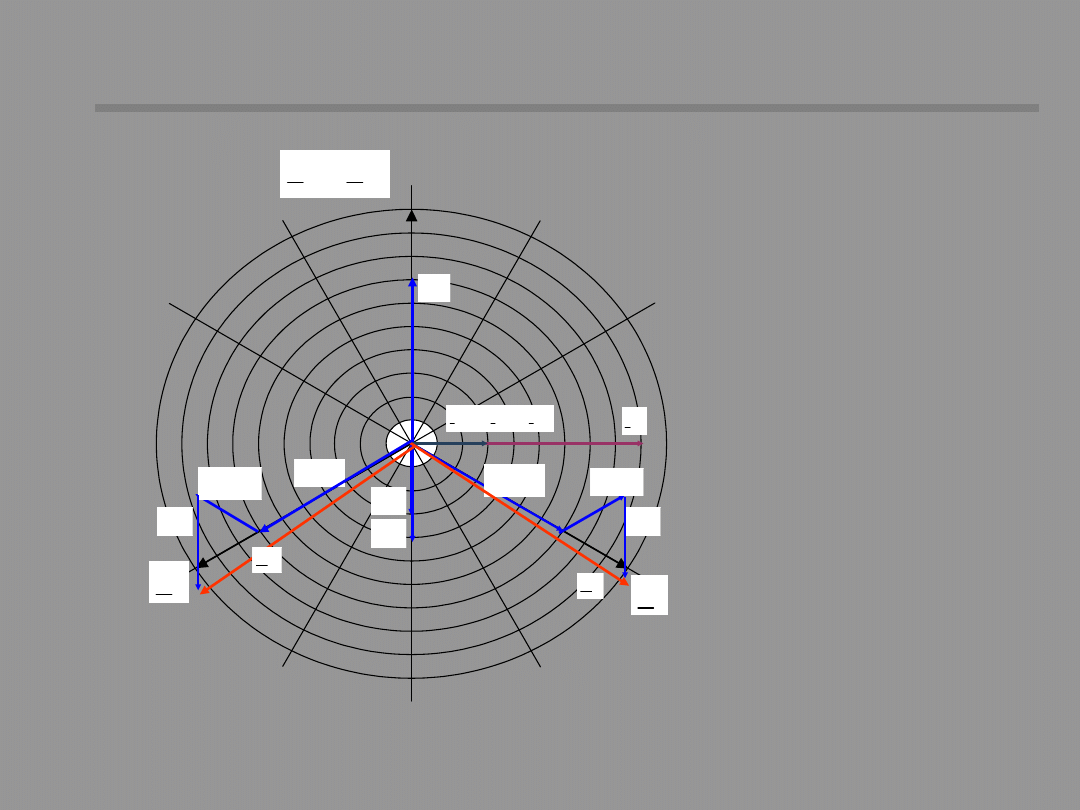
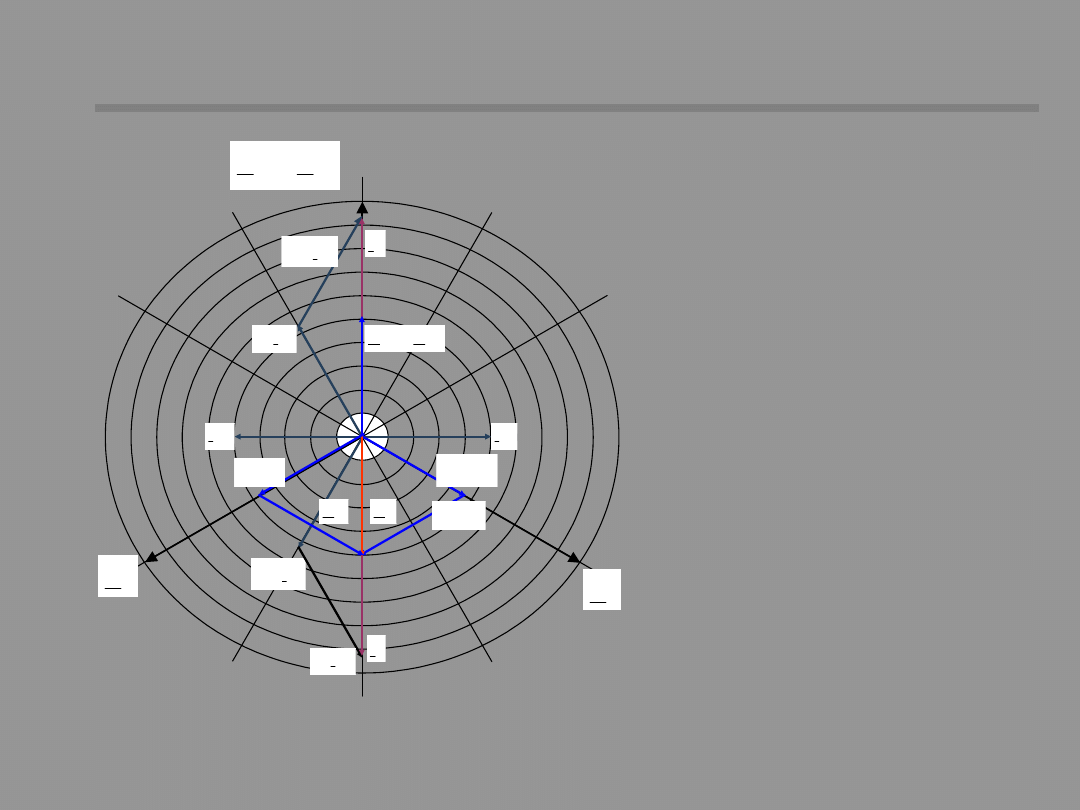
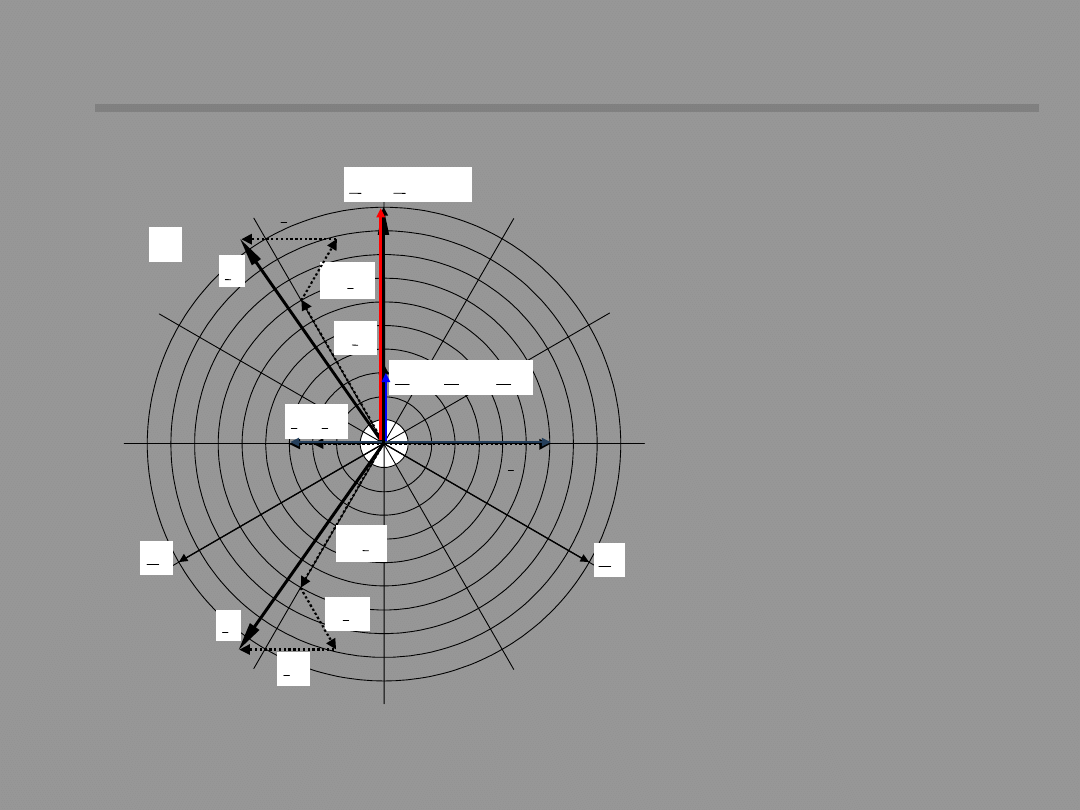
Vector diagram of currents
and voltages for single phase
short-circuit (network
resistance is omitted)

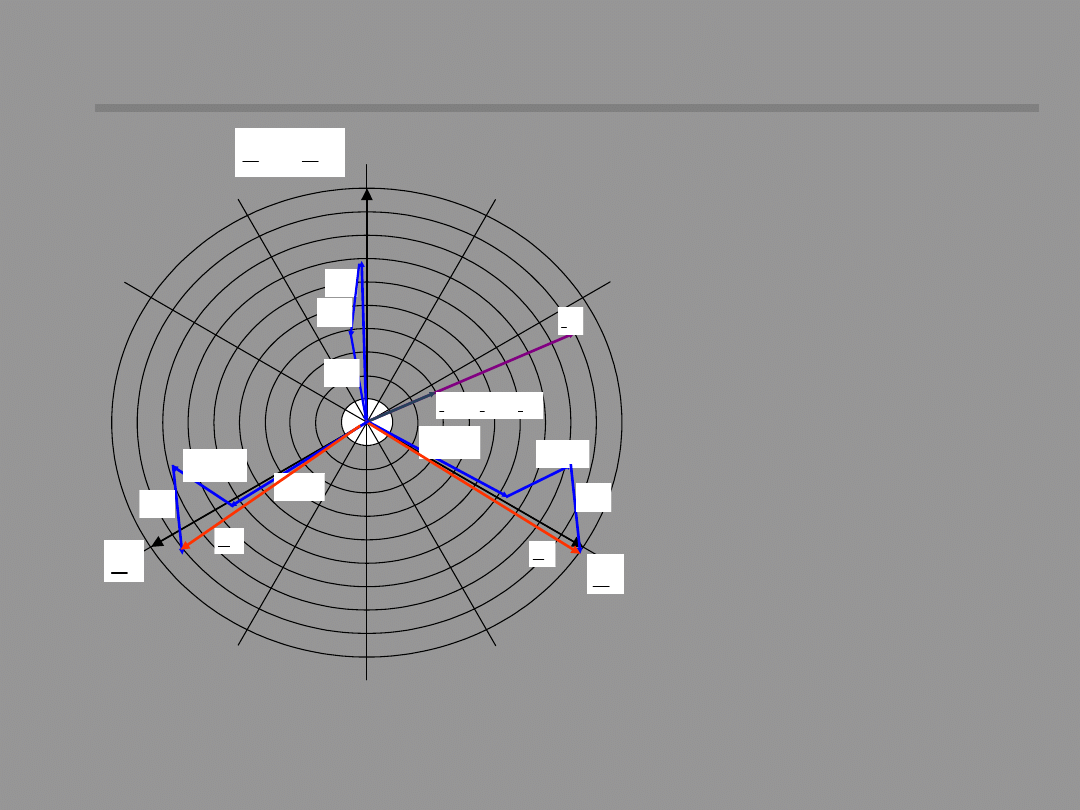
27 / 48

Single line-to-earth short-circuit

 

E 

 



 

a 

 

a

 

a

 

a 

 

Vector diagram of currents
and voltages for single phase
short-circuit (network
resistance is taken into
account).

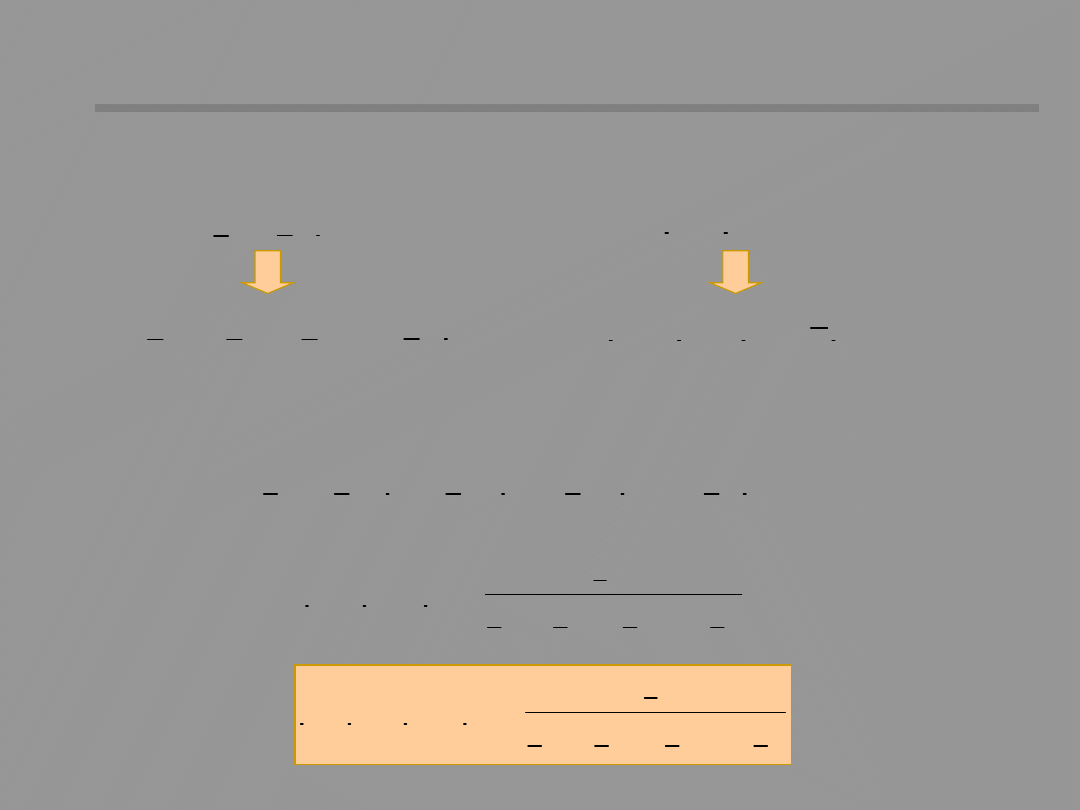
28 / 48

Single line-to-earth short-circuit

through

an impedance

Z R

Z I

= =

( )

Z 1

3Z I

( )

( ) ( )

( )

1 1

2 2

0 0

Z 1

Z I

3Z I

( )

( ) ( ) ( )

( )

= +

Boundary conditions:

)

The condition 1) gives:

From

here:

29 / 48

Single line-to-earth short-circuit

through

an impedance

( )

( ) ( )

( )

0 0

Z E

Z I

( )

( ) ( )

( )

1 1

Z I

( )

( ) ( )

( )

2 2

Z E

Z I

Voltage symmetrical components:

30 / 48

Single line-to-earth short-circuit

through

an impedance

( )

a U

( )

(

)

( )

a a Z

a 1 Z

3a Z

�

( )

a U

( )

(

)

( )

(

)

( )

a a Z

a 1 Z

3aZ

�

+ -

�

( )

u 0

3Z I

D =

Phase voltages:

31 / 48

Single line-to-earth short-circuit

through

an impedance

 

E 

 



 

a 

 

a

 

a

 

a 

 

Vector diagram of currents
and voltages for single phase
short-circuit through an
impedance (network
resistance is omitted)

32 / 48

Line-to-line short-circuit

( )

(

)

a a I

( )

(

)

a a I

( )

(

)

1 1 I

( )



Assumptions:

•

Load currents are omitted.

•

The fault involves the phases S and T



Boundary conditions:

)

Expressing these relationships in terms of the

symmetrical components:

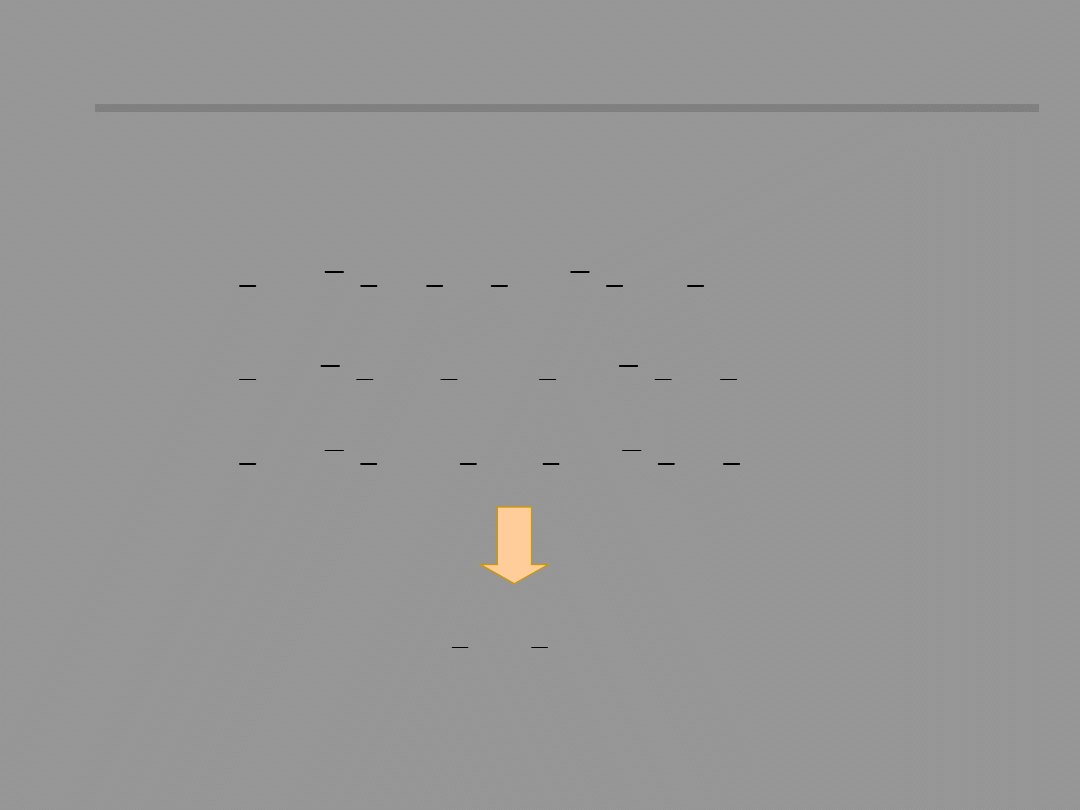
33 / 48

Line-to-line short-circuit

( )

(

)

(

)

+ +

( )

(

)

(

)

a U

( )

(

)

(

)

a U

( )

Voltage sequence components:

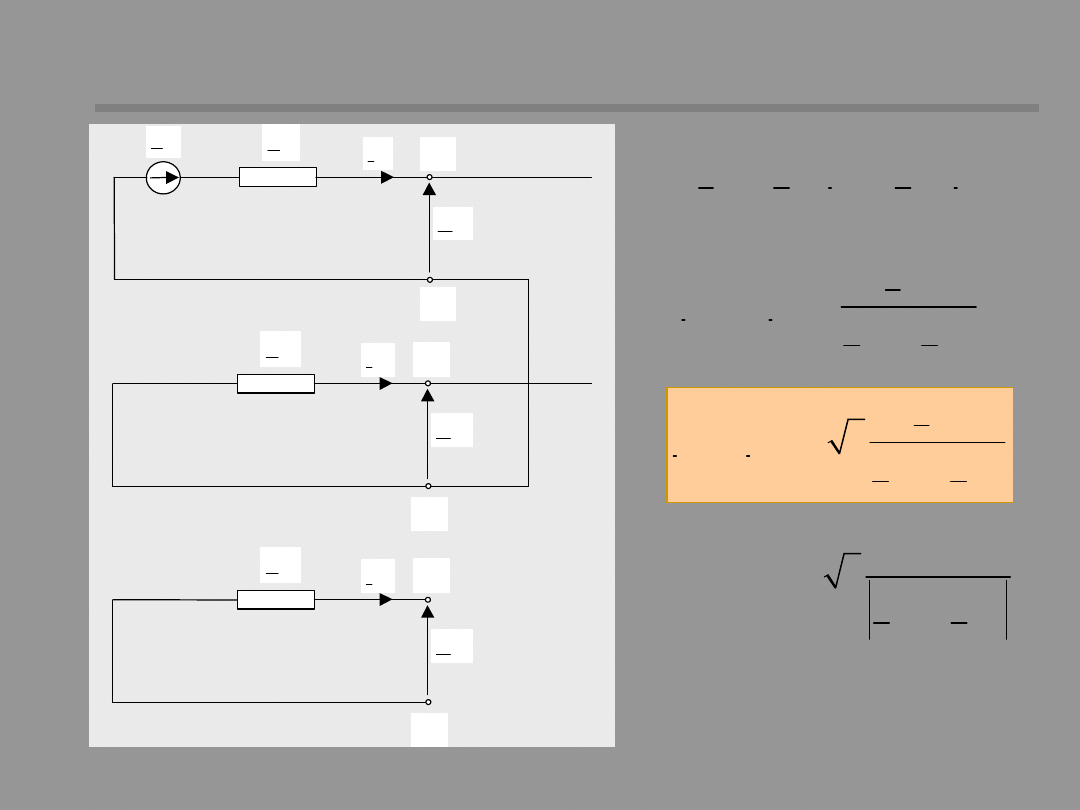
34 / 48

Line-to-line short-circuit

 

(1)

(2)

 

(0)

( )

( ) ( )

1 1

2 1

Z I

( )

j 3

=- =-

( )

= = =

Sequence network connection for
line-to-line short-circiut has two
sequence networks connected in
parallel.

35 / 48

Line-to-line short-circuit

( )

( ) ( )

( )

1 1

2 2

Z I

( )

( ) ( )

( )

Z E

a U

Phase voltages:

36 / 48

Line-to-line short-circuit

 

E 

 

a 

 

a

 

a

 

a 

 

U 

 

a 

 

a

 

a

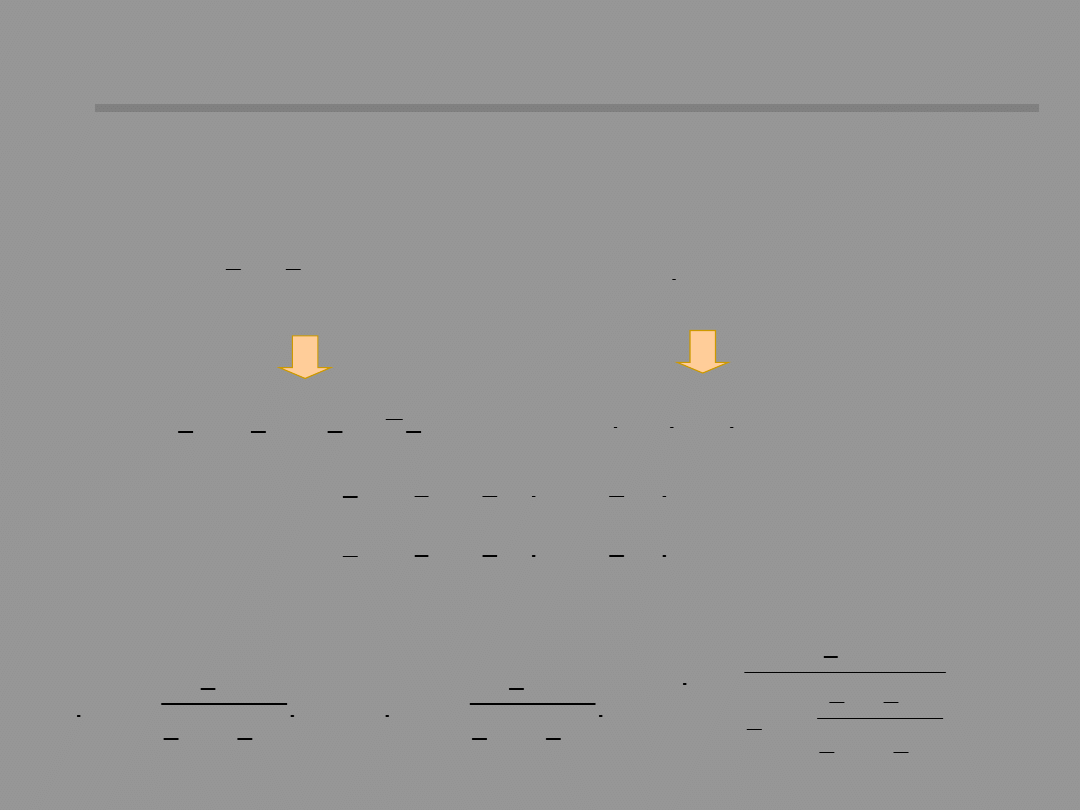
Vector diagram of
currents and voltages
for line-to-line short-
circuit (network
resistance is omitted)

37 / 48

Line-to-line short-circuit

through an

impedance

Z S

Z I

( )

(

) (

)

( )

(

)

( )

a U

a a U

( )

(

)

( )

Z S

Z 1

j 3 U

Z I

j 3Z I

( )

Z 1

Z I

( )

( ) ( )

( )

1 1

2 1

Z 1

Z I

( )

j 3

=- =-

Boundary conditions

)

38 / 48

Double line-to-ground short-circuit

( ) ( ) ( )

( )

= =

( )

( ) ( )

1 1

2 2

Z I

( )

( ) ( )

1 1

0 0

Z I

( )

( ) ( )

( )

Z Z

( )

The short-circuit involves phases S, T and ground.
Initial relationship at the point of the fault:

From solving the equations it follows that:

Using the sequence

transformation

39 / 48

Double line-to-ground short-circuit

 

(1)

(2)

 

(0)

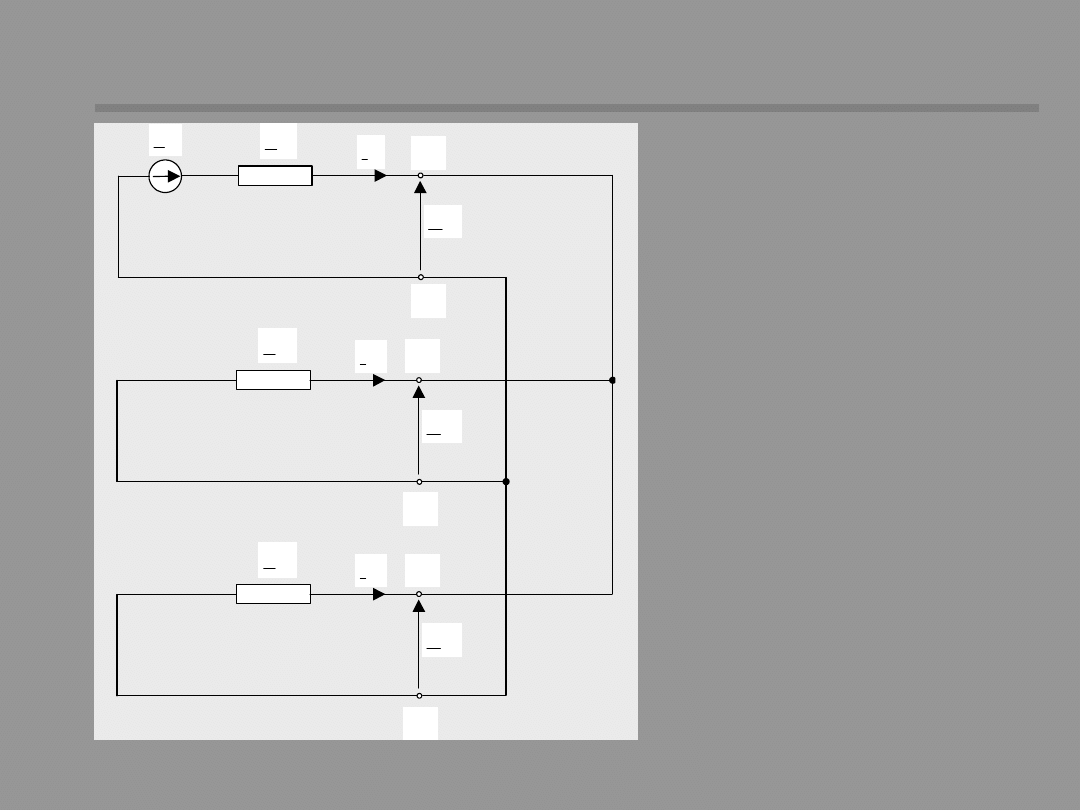
Sequence network connection
for double line-to-ground short-
circuit has three sequence
networks connected in parallel.

40 / 48

Double line-to-ground short-circuit

( )

a I

a a

�

( )

(

)

( )

(

)

j 3 X

2 X

�

( )

(

)

( )

(

)

j 3 X

2 X

�

Phase currents:

41 / 48

Double line-to-ground short-circuit

( )

(

)

( )

(

)

( )

( ) ( )

( )

(

)

X X

3 X

3 1

2 X

= = =

Phase current magnitudes:

Voltages:

( )

( ) ( )

( )

( ) ( )

( )

Z Z

( )

42 / 48

Double line-to-ground short-circuit

 



 



 

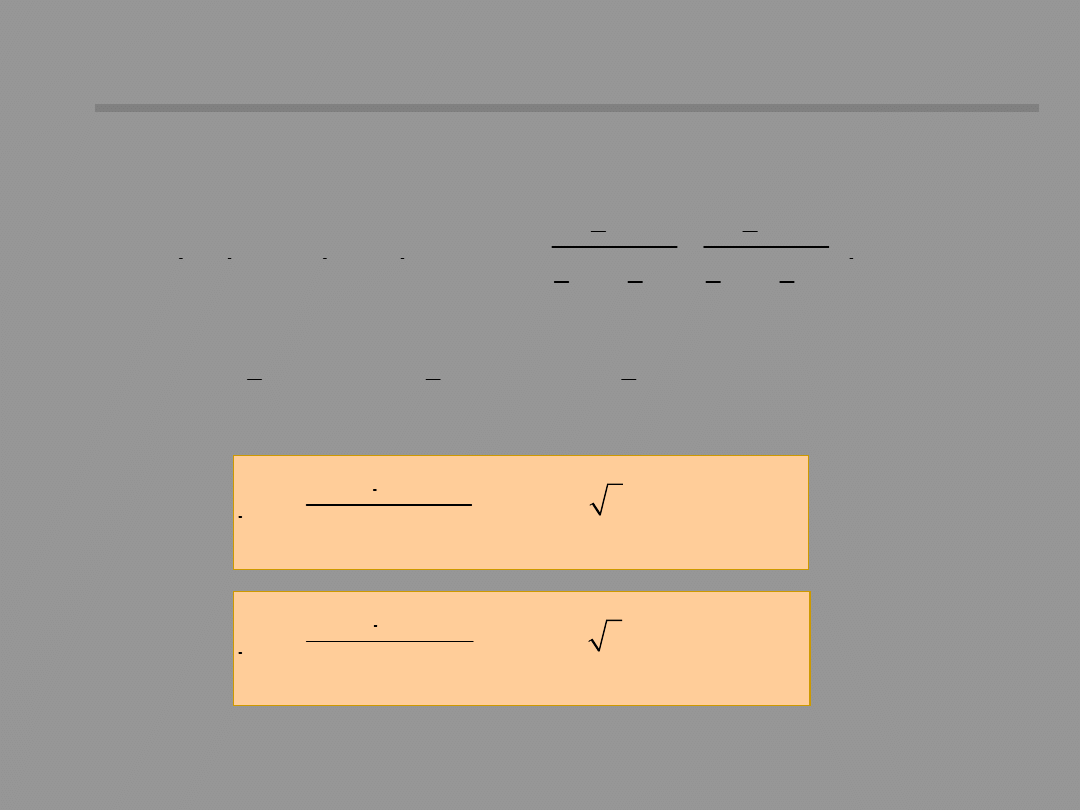
Vector diagram of
currents and voltages
for double line-to-
ground short-circuit
(network resistance is
omitted)

43 / 48

Double line-to-ground short-circuit

through an impedance

(

)

Z Z

Z S

Z I

( )

Z Z

Z 0

Z I

3Z I

( ) ( ) ( )

Boundary

conditions:

44 / 48

Three-phase, three-phase with ground

short-circuit

+ + =

( )

= =

( )

= =

Boundary conditions:

45 / 48

Initial current

Positive sequence initial current for different

types of short-circuits

 

 
 

I 

 





 





 

   

 





Three-phase short-circuit

Line-to-line

short-circuit

Single phase line-to-earth short-circuit

Double line-

to-earth short-circuit

46 / 48

Initial current

Z 



 

Z 



 







   

 







General

formula:

 







where

Three-phase short-

circuit

Line-to-line short-

circuit

Single phase line-to-earth

short-circuit

Double line-to-earth short-

circuit

47 / 48

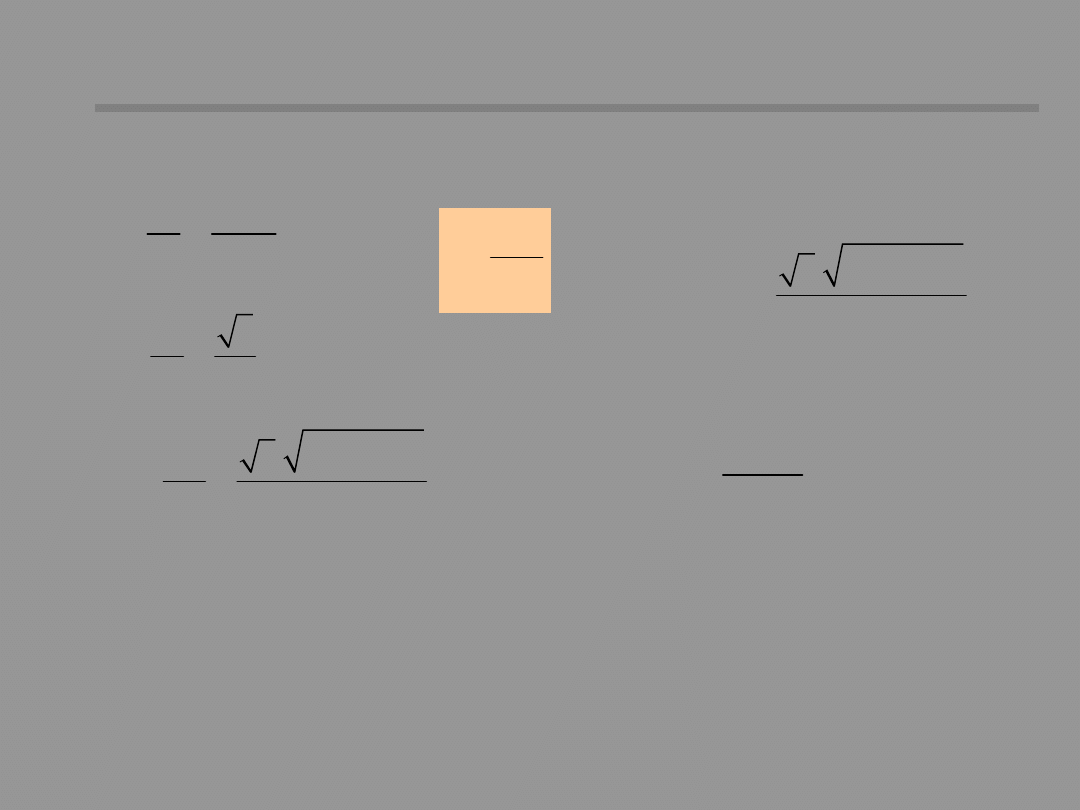
Initial current

 

I 

 

I 

 

I 

 





 







 

I 

Three-phase short-

circuit

Line-to-line short-

circuit

Single phase line-to-earth short-

circuit

Double line-to-earth

short-circuit

General

expression:

48 / 48

Currents and voltages in relation with

( )

2fz

3 1

1 2

+a +a

+ a

( )

a =

Assumptio

( )

3 1

+a +a

( )

2fz

1 2

+ a

If X

(0)

(1)

the 3-phase short-circuit current is the biggest one.

If X

(0)

(1)

the single-phase line –to-earth current is the biggest

one.

If X

(0)

(1)

voltages of unfaulted (healthy) phases for single-phase

and line-to-line short-circuits are > than EMF.

If X

(0)

(1)

voltages of unfaulted (healthy) phases for single-phase

and line-to-line short-circuits are < than EMF.

Document Outline

Slide 1
Slide 2
Slide 3
Slide 4
Slide 5
Slide 6
Slide 7
Slide 8
Slide 9
Slide 10
Slide 11
Slide 12
Slide 13
Slide 14
Slide 15
Slide 16
Slide 17
Slide 18
Slide 19
Slide 20
Slide 21
Slide 22
Slide 23
Slide 24
Slide 25
Slide 26
Slide 27
Slide 28
Slide 29
Slide 30
Slide 31
Slide 32
Slide 33
Slide 34
Slide 35
Slide 36
Slide 37
Slide 38
Slide 39
Slide 40
Slide 41
Slide 42
Slide 43
Slide 44
Slide 45
Slide 46
Slide 47
Slide 48

Wyszukiwarka

Podobne podstrony:
Symmetrical components method continued
Symmetrical components method continued
24 G23 H19 QUALITY ASSURANCE OF BLOOD COMPONENTS popr
Gunwitch Method
components microwave
Opponens Pollicis KT method
Sprawdzian symetria Iab
Definicja symetralnej
Fibularis (Peroneus) Longus KT method
ALAN42 MULTI component side
Flexor Hallucis Longus KT method
Popliteus KT method
Component Based Automation
Posterior Diaphragm KT method
Palmaris Longus KT method
Oblique Abdominis Externus KT method
Connexions 1 Methode de francais
Earned Value Method

więcej podobnych podstron

Symetrical components method

Document Outline