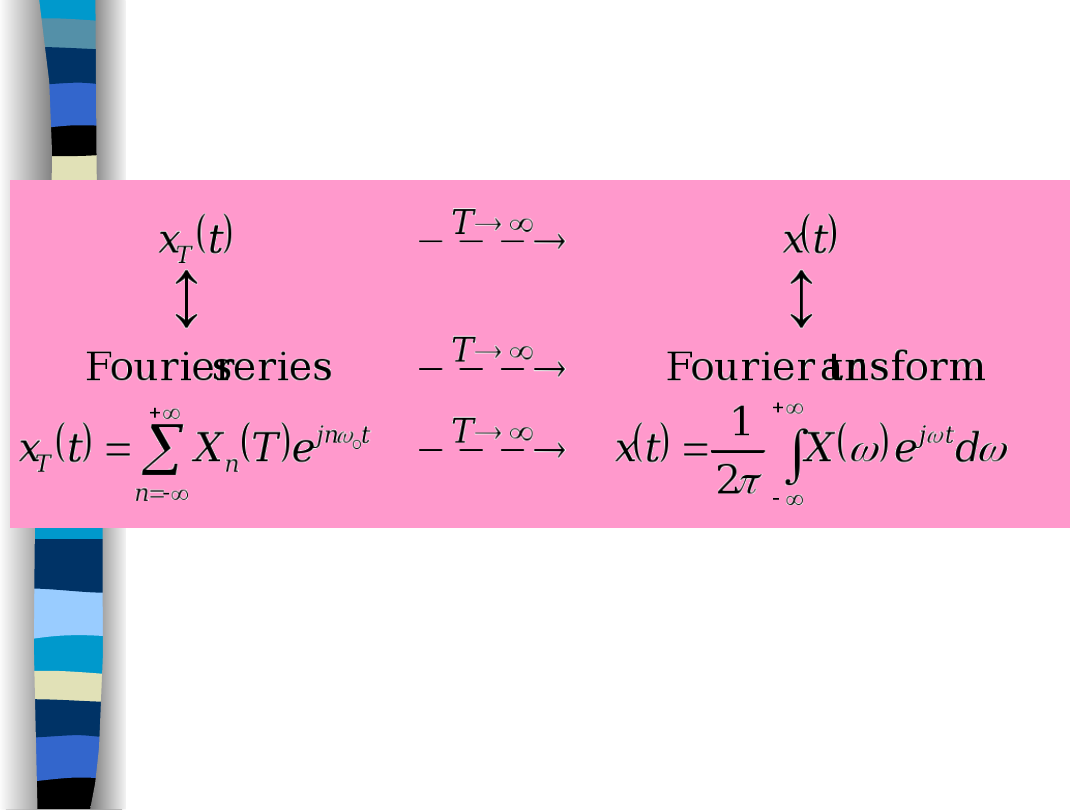
Fourier
Transform

„Signal Theory” Zdzisław Papir

•Periodicity of Fourier series

•Limiting behaviour of Fourier series

•Limiting form of Fourier series

•Fourier transform pairs
•Existence of Fourier transform

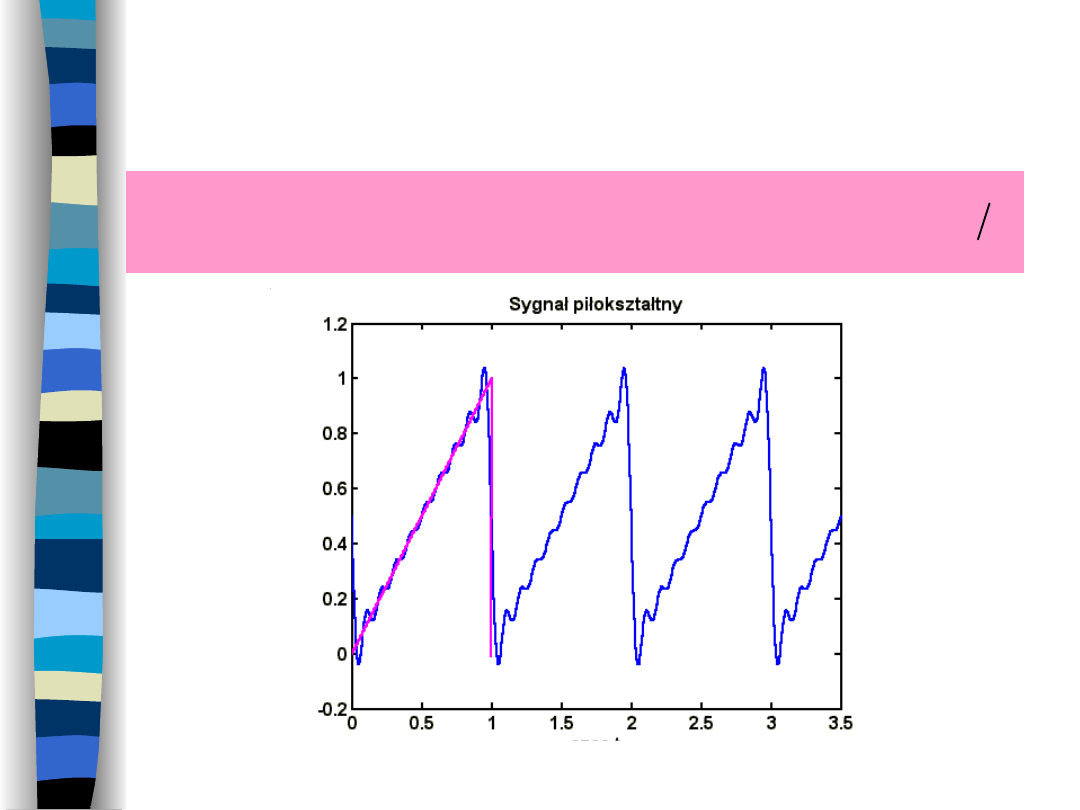
Periodicity of Fourier
series

„Signal Theory” Zdzisław Papir

 









T

e

X

T

t

x

e

X

t

x

n

T

t

jn

n

n

t

jn

n









2

,

o

o

o





























sawtooth signal

time t

period T = 1

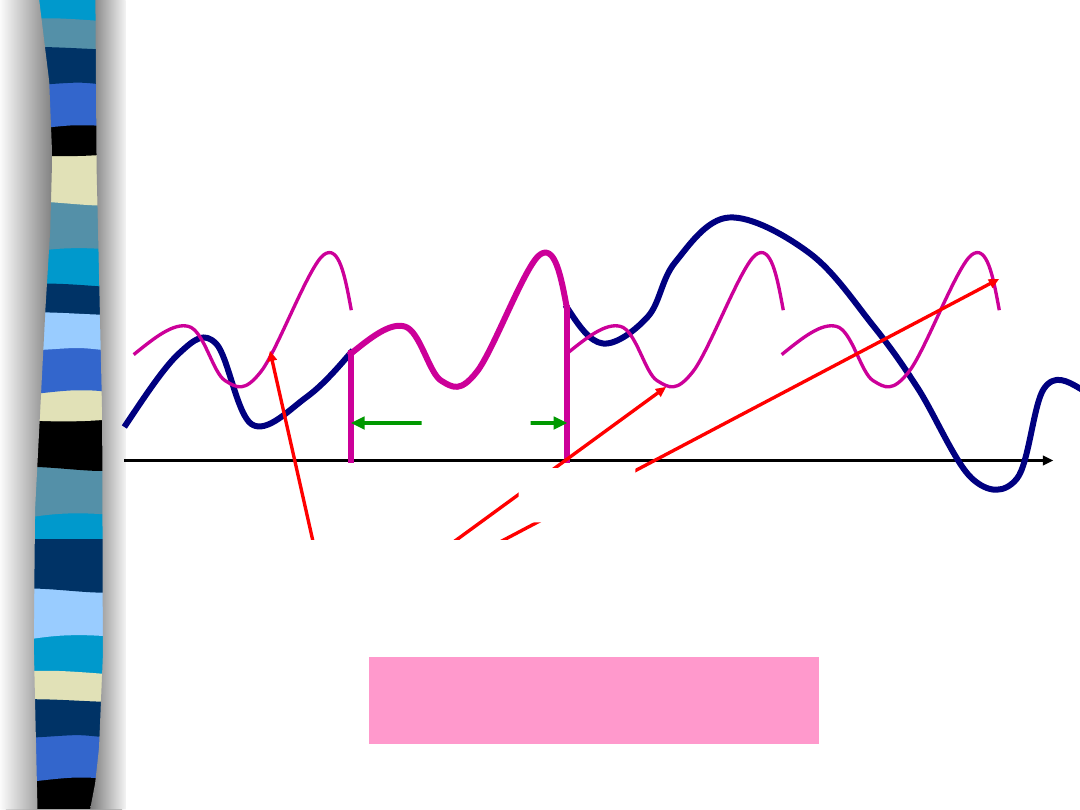
Limiting behaviour
of Fourier series

„Signal Theory”  Zdzisław

Papir

x(t)

time t

-T/2

x

T

(t)

Periodic extension of a signal window x

T

(t)

through Fourier series

+T/2

 

 

t

x

t

x

T

T



 







Limiting behaviour of Fourier
series

„Signal Theory” Zdzisław Papir

„Signal Theory” Zdzisław Papir

 





T

e

jn

T

T

X

T

n







2

,

1

1

1

1

o

o















   

t

e

t

t

x



1

Limiting behaviour of Fourier
series

„Signal Theory” Zdzisław Papir

 





T

e

jn

T

T

X

T

n







2

,

1

1

1

1

o

o















 

0

0

2

o



 





 













T

n

T

T

X

T





Limiting behaviour of Fourier
series

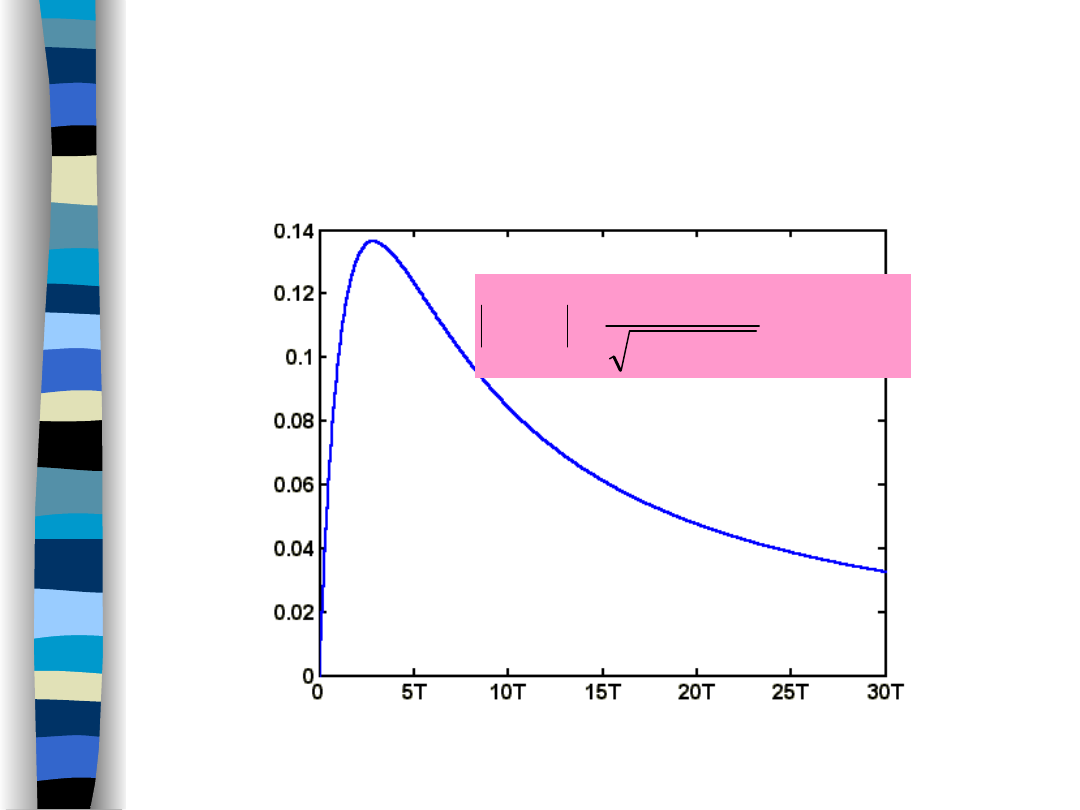
„Signal Theory” Zdzisław Papir

Limiting behaviour of Fourier
series

 

0

4

1

2

2

1



 















T

T

T

e

T

X



amplitude of the 1st spectrum line of an exponential puls

Fourier series window

 

0

4

1

2

2

1



 















T

T

T

e

T

X



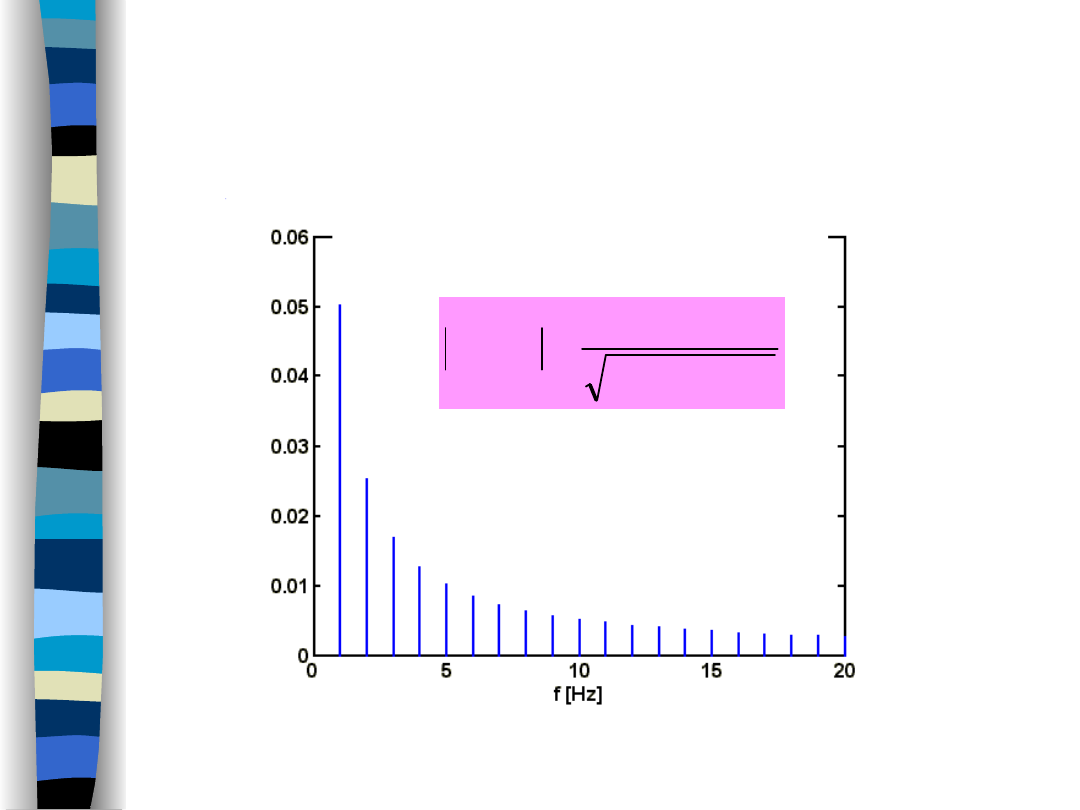
„Signal Theory” Zdzisław Papir

Limiting behaviour of Fourier
series

 

2

2

2

4

1

n

T

e

T

X

T

n











Fourier series
window T

amplitude spectrum –
exponential pulse

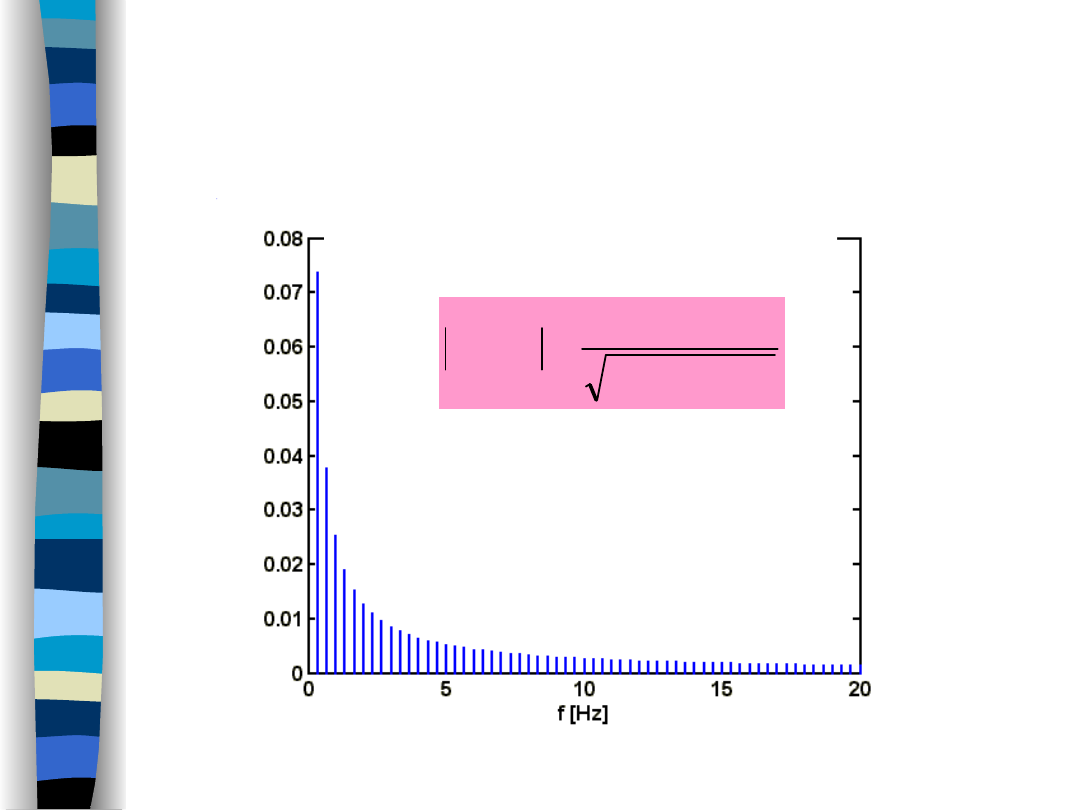
„Signal Theory” Zdzisław Papir

Limiting behaviour of Fourier
series

 

2

2

2

4

1

n

T

e

T

X

T

n











Fourier series
window 3T

amplitude spectrum –
exponential pulse

„Signal Theory” Zdzisław Papir

Limiting behaviour of Fourier
series

 

2

2

2

4

1

n

T

e

T

X

T

n











amplitude spectrum –
exponential pulse

Fourier series
window 10T

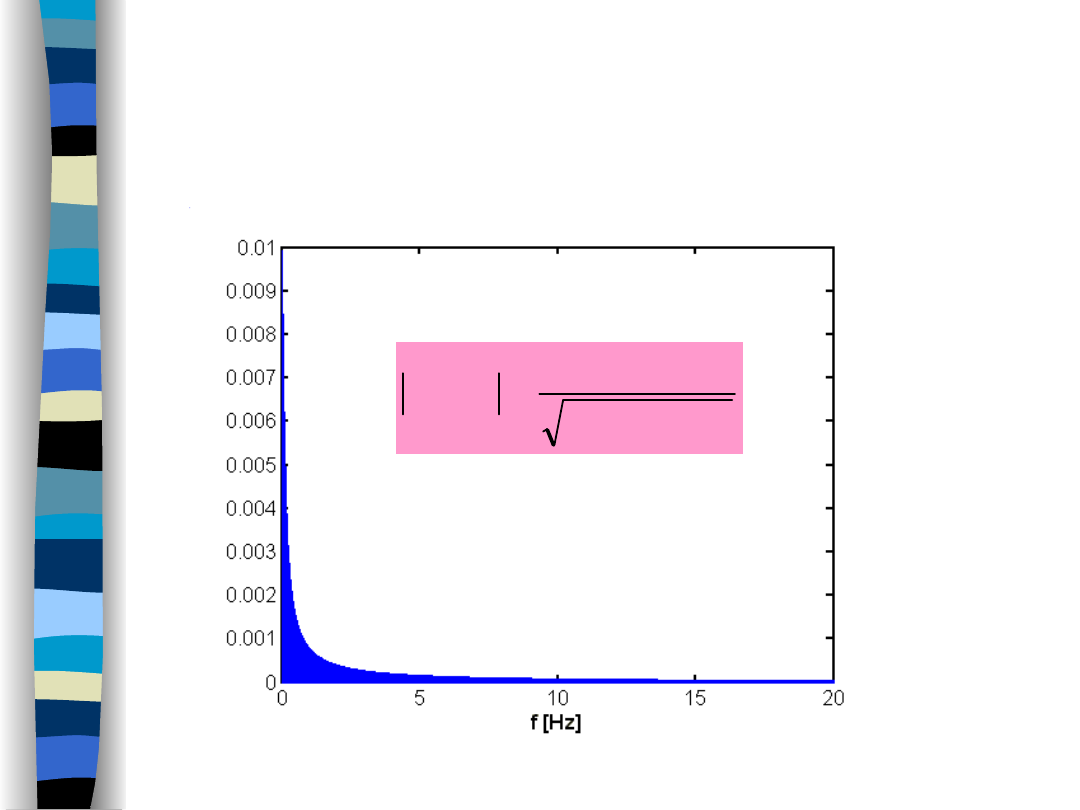
„Signal Theory” Zdzisław Papir

Limiting behaviour of Fourier
series

 

2

2

2

4

1

n

T

e

T

X

T

n











amplitude spectrum – exponential pulse

Fourier series window
100T

„Signal Theory” Zdzisław Papir

 

 









j

X

j

e

T

TX

T

n

T

n







 















1

1

1

1

Squeezing Fourier series coefficients in FREQUENCY:

 

 

n

T

n

T

n

j

e

T

TX

jn

e

T

TX

n

n







































1

1

1

1

o

o

Limiting behaviour of Fourier
series

Squeezing Fourier series coefficients in AMPLITUDE:

„Signal Theory” Zdzisław Papir

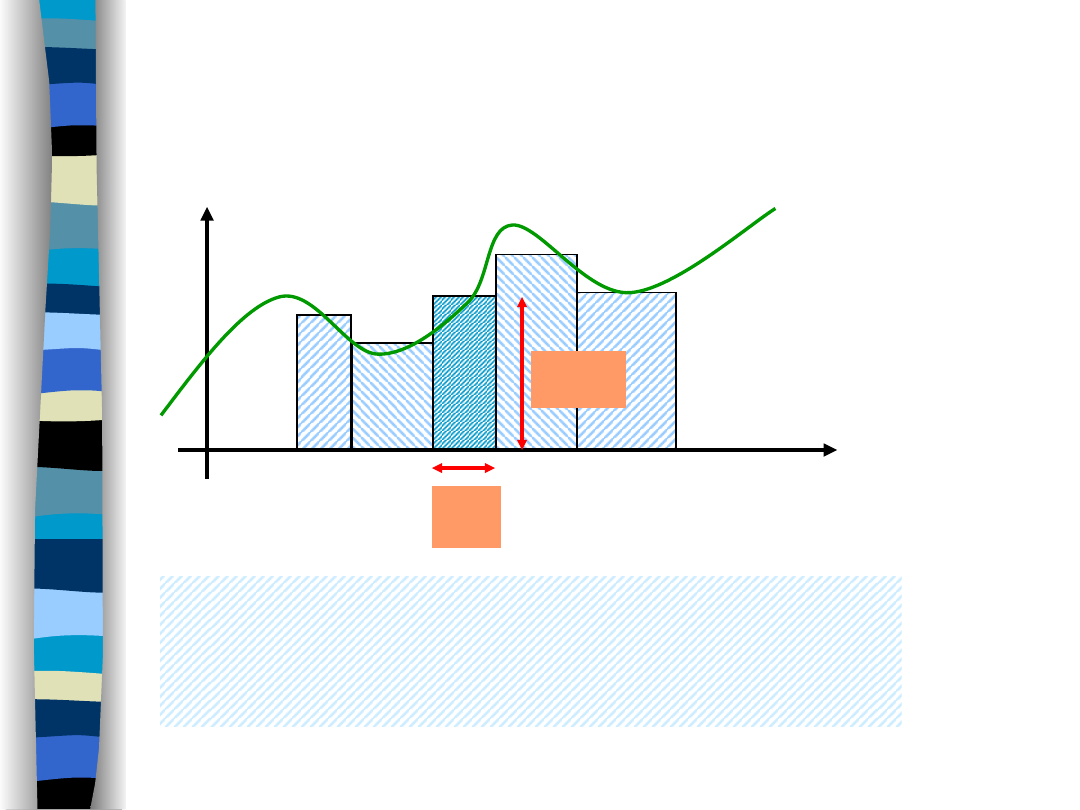
Riemann integral

a

b

x

f(x
)

n

x



 

n

x

f

 

 



























b

a

n

x

n

n

n

dx

x

f

x

x

f

S

n

0

max

Limiting form of Fourier
series

„Signal Theory” Zdzisław Papir

Fourier series coefficients:

 

 

 

 

















2

2

2

2

o

1

T

T

t

j

T

T

t

jn

n

dt

e

t

x

T

TX

dt

e

t

x

T

T

X

n

n







 

 

 



















dt

e

t

x

X

T

TX

t

j

T

n







lim

FORWARD FOURIER TRANSFORM:

Limiting form of Fourier series

„Signal Theory” Zdzisław Papir

Fourier series:

   

 



























d

e

X

t

x

t

x

t

j

T

T

1

lim

INVERSE FOURIER TRANSFORM:

 

 

 

 

 



























n

n

n

t

j

T

n

t

jn

n

n

t

jn

n

T

e

T

TX

t

x

e

T

TX

e

T

X

t

x



















2

1

2

1

o

o

o

„Signal Theory” Zdzisław Papir

 

 

 

 

 

 









































































 

 





































n

T

t

t

t

j

n

t

jn

T

t

t

jn

n

t

jn

T

t

t

jn

d

e

x

t

x

e

d

e

x

t

x

e

d

e

x

T

t

x

n

0

0

0

0

0

0

0

0

0

0

2

1

2

1

1

0











n

t

jn

n

e

X

t

x

0

)

(











T

t

t

t

jn

n

dt

e

t

X

T

X

0

0

0

)

(

1



Fourier Integral Theorem

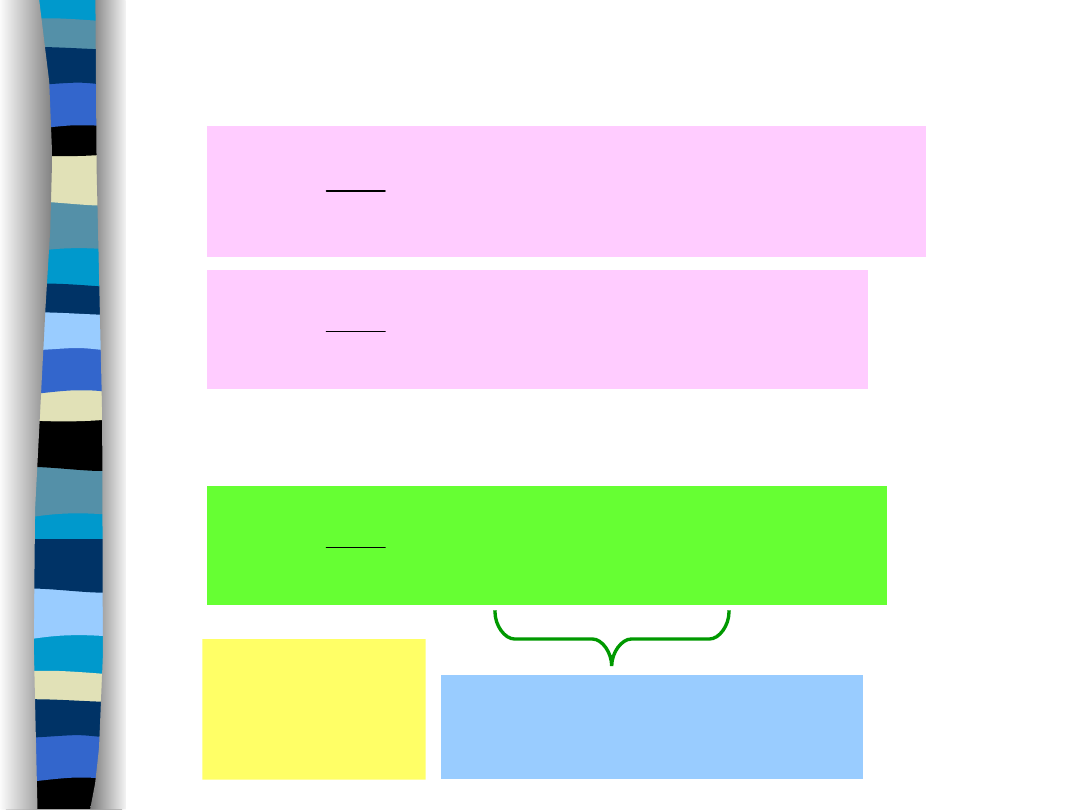
„Signal Theory” Zdzisław Papir

Fourier transform

 

 





























 













n

T

t

t

t

j

d

e

x

t

x

n

0

0

2

1

 

 

















d

d

e

x

t

x

t

j

 



























2

1

 

 













dt

e

t

x

X

t

j





 

 













d

e

d

e

x

t

x

t

j

j

 

























2

1

Fourier integral theorem

Forward
Fourier
transform

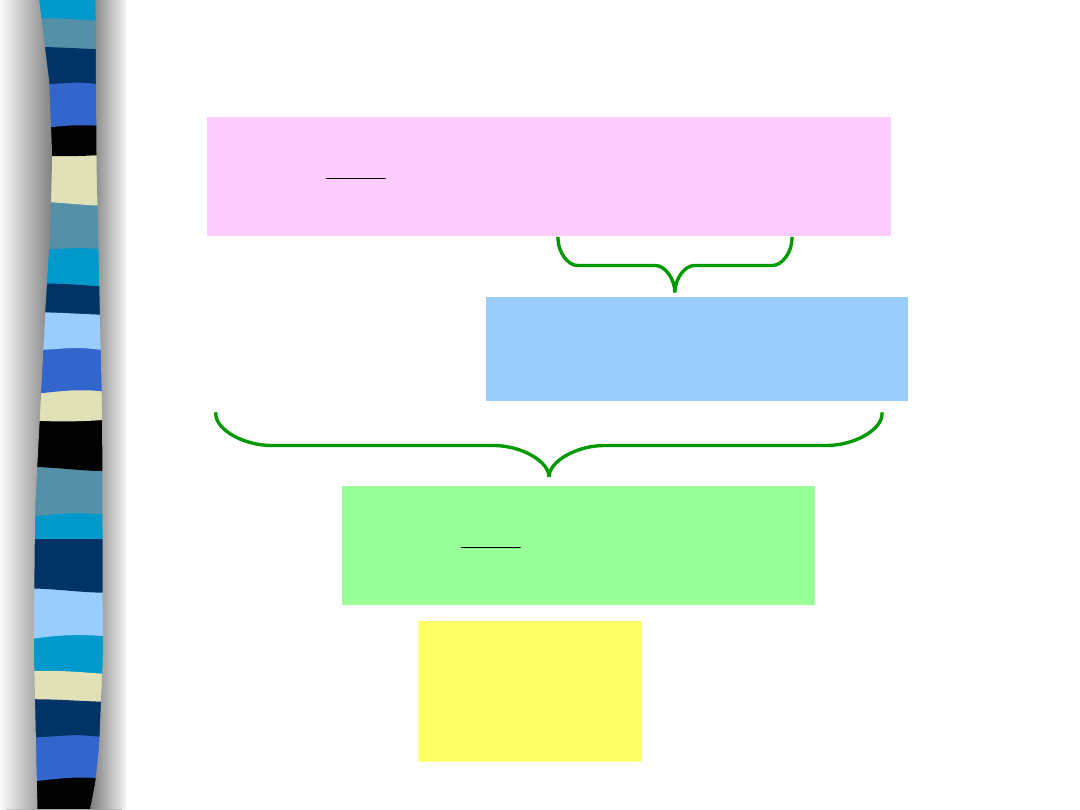
„Signal Theory” Zdzisław Papir

Inverse Fourier transform

 

 













d

d

e

x

e

t

x

j

t

j





























2

1

 

 

dt

e

t

x

X

t

j

















Inverse
Fourier
transform

 

 









d

e

X

t

x

t

j











2

1

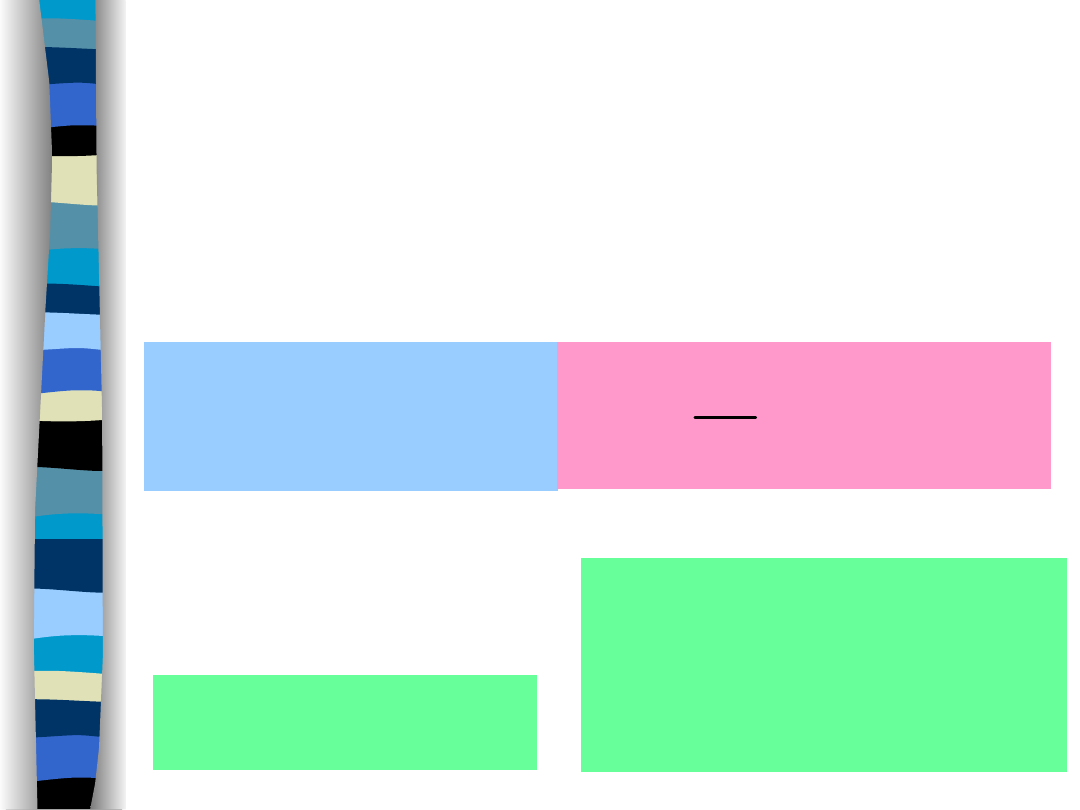
Fourier transform pairs

„Signal Theory” Zdzisław Papir

TRANSFORM

 

 













dt

e

t

x

X

t

j





 

 





















d

e

X

t

x

t

j

1

INVERSE

FORWARD

TRANSFORM
PAIRS

 

 



X

t

x 

 

 

 

 

 









X

t

x

t

x

X

1







F

F

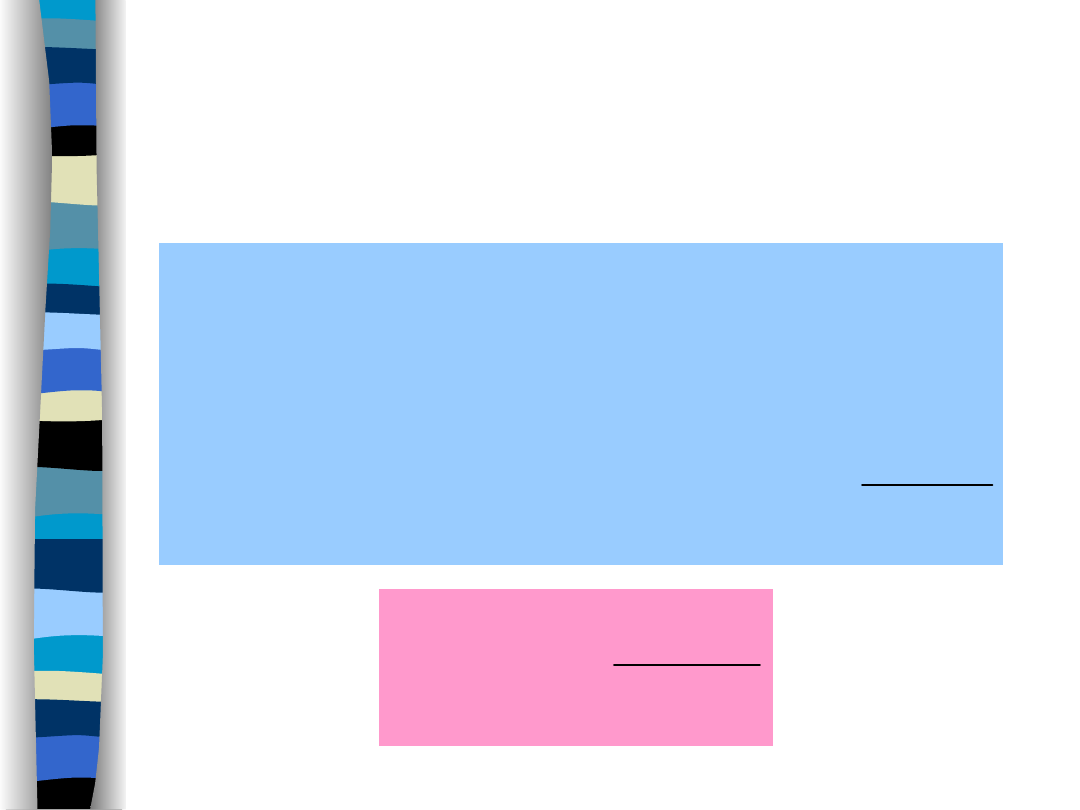
Fourier transform pairs

„Signal Theory” Zdzisław Papir

 

 

 













j

dt

e

dt

e

e

X

e

t

t

e

t

t

x

t

j

t

j

t

t

t











































1

1

0

,

0

,

0

o

1

o

1

FORWARD FOURIER TRANSFORM:

 



j

e

t

t







1

1

1

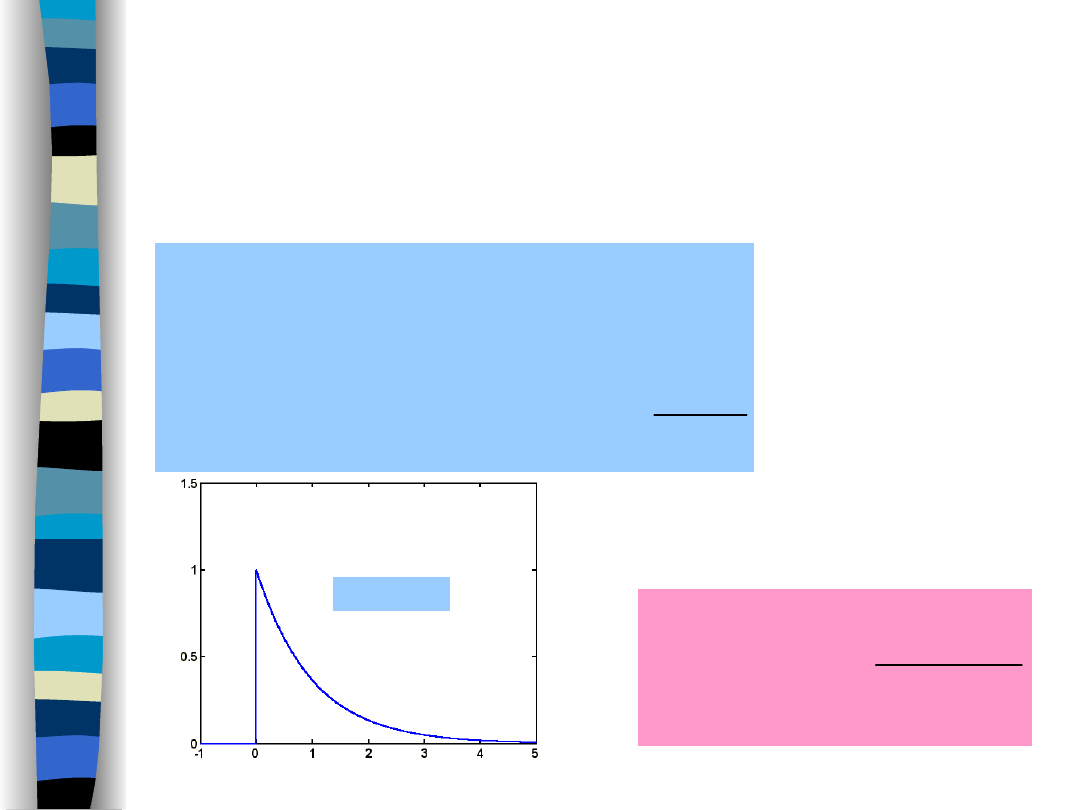
Fourier transform pairs

„Signal Theory” Zdzisław Papir

 



j

e

t

t







1

1

1

FOURIER TRANSFORM:

   

t

e

t

t

x



1

time t

 

 

 













j

dt

e

dt

e

e

X

e

t

t

e

t

t

x

t

j

t

j

t

t

t











































1

1

0

,

0

,

0

o

1

o

1

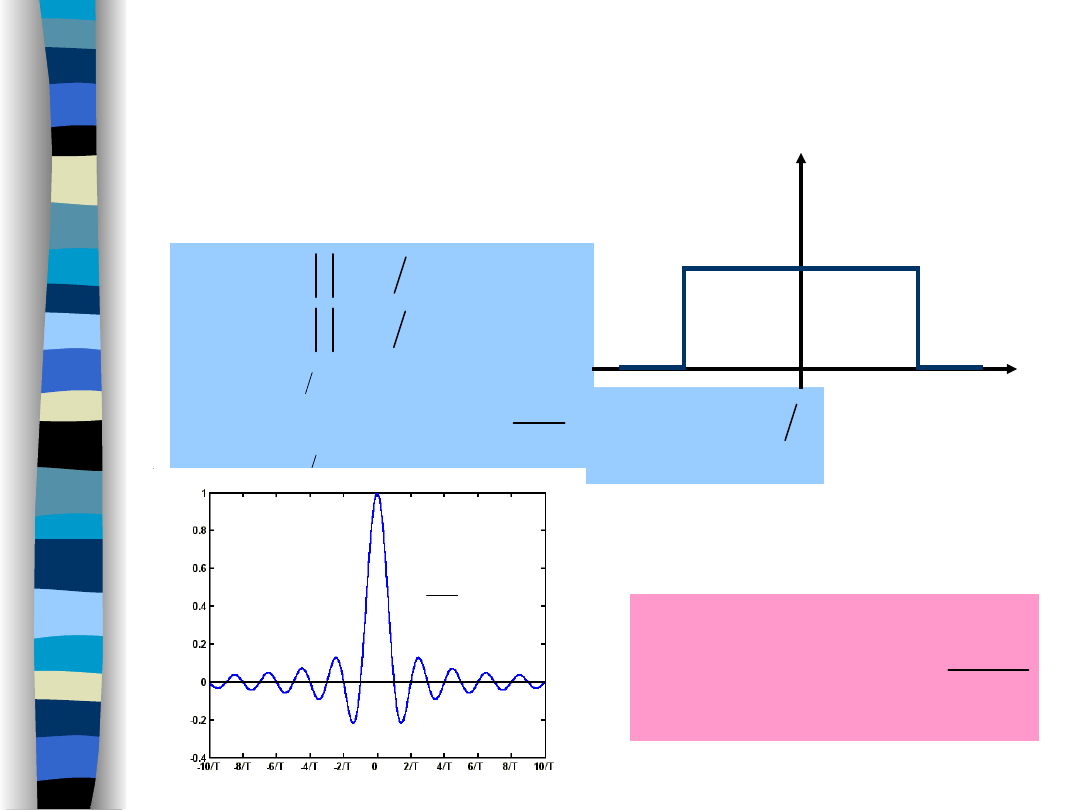
Fourier transform pairs

„Signal Theory” Zdzisław Papir

 

 

 

 

 

x

x

x

T

T

dt

e

X

t

T

t

T

t

t

x

T

T

t

j

T

sin

Sa

,

2

Sa

2

,

1

2

,

0

2

2

-

































 

2

Sa

T

T

t

T







FOURIER TRANSFORM:

T/2

-T/2

1

 

t

T



 

fT

T

t

x





Sa

2

Sa





frequency f

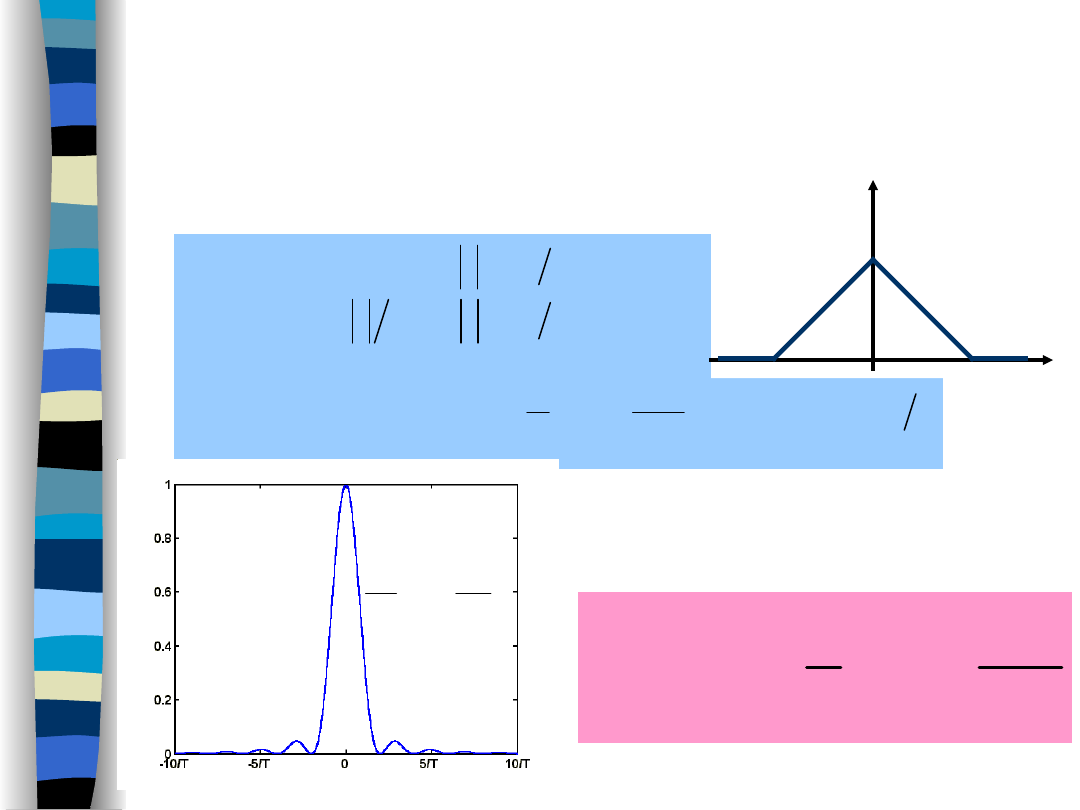
„Signal Theory” Zdzisław Papir

 

 

 

 

 

 

x

x

x

T

T

dt

e

t

X

t

T

t

T

t

T

t

t

x

t

j

T

T

sin

Sa

,

4

Sa

2

1

2

,

2

1

2

,

0

2

-









































 

4

Sa

2

1

2

T

T

t

T







FOURIER TRANSFORM:

T/2

-T/2

 

t

T



Fourier transform pairs

 

2

Sa

4

Sa

2

2

fT

T

t

x









frequency f

„Signal Theory” Zdzisław Papir

Existence of Fourier
transform

Dirichlet conditions are
necessary
for Fourier transform
existence.

• Signal x(t) must have only a finite number of maxima
and minima, as well as a finite number of discontinuities
over the entire range [–, + ].

• Signal x(t) is also allowed to be unbounded provided that
it is absolutely integrable:

 













dt

t

x

„Signal Theory”  Zdzisław Papir

Summary

•

Fourier series is a spectral decomposition of periodic signal or

produces a periodic extension of signal window.

•

Fourier transform is a tool for spectral decomposition of

nonperiodic signals.

•

Fourier transform is a limiting case of Fourier series with signal

window being extended up to infinity.

•

Dirichlet conditions are necessary for Fourier transform

existence.

•

In engineering applications it is commonly assumed that signals

of limited energy are Fourier transformable.