J. Micromech. Microeng. 9 (1999) 1–8. Printed in the UK

PII: S0960-1317(99)04605-7

Characteristic modes of electrostatic
comb-drive

X

–

Y

microactuators

Ty Harness and Richard R A Syms

†

† Optical and Semiconductor Devices Section, Department of Electrical and
Electronic Engineering, Imperial College, Exhibition Road, London, SW7 2BT, UK
JMM/104605/PAP17895ae26/11/9915.27

Received 21 May 1999

Abstract.

The design of dual axis microengineered comb-drive electrostatic actuators is

investigated. Experimental measurements of diode-isolated bulk micromachined x–y stages
with a folded, single-flexure suspension show the presence of unwanted two-dimensional
vibrational mode patterns. A combination of coupled-mode and finite-element analysis is
used to explain the existence of the spurious modes, and good agreement is obtained with the
experimental response provided that the intrinsic stress is taken into account. It is shown that
improved performance can be obtained simply by doubling the number of suspension
flexures.

1. Introduction

Laterally-resonant electrostatic comb-drive microactuators
are well established components. Single-axis devices have
been fabricated by surface micromachining of polysilicon
[1–5] and single-crystal silicon [6, 7], bulk micromachining
of doped silicon [8, 9],

combined surface and bulk

micromachining [10] and electroplating of metals [11, 12].
Applications have been found in lateral tunnelling units [11],
vibromotors [13, 14], microengines [15], external cavities for
laser diodes [16], scanning mirrors [14, 17], gyroscopes [18]
and pressure sensors [19]. Linearly-coupled devices have
been used as electromechanical filters [20] and orthogonally-
coupled devices have been used as x–y stages for atomic force
microscopy (AFM) applications [21–24]. Early devices were
formed from thin (2–10 µm) mechanical layers; more robust
components are now being developed [25, 26], based either
on bonded silicon-on-insulator [27, 28], deep dry etching
using an inductively coupled plasma [29, 30] or on metals
electroplated in deep molds [31].

The dynamics of single-axis devices are well understood

[1] and have been visualized in stroboscopic experiments
[32]. They consist of a single dominant mass—the moving
half of the electrode and its supporting shuttle—suspended
on elastic flexures and constrained to move along a single
axis. Due to the span of the electrode, its mass is distributed,
but the shuttle is designed to be sufficiently rigid so that the
assembly can be considered a pointlike mass for in-plane
motion. The suspension is often small enough, compared
with the shuttle, that it can be considered massless, although
the Rayleigh method can be used to estimate its effect on
resonant frequency [1, 2]. Although elastic nonlinearities
have been observed [33, 34], the effects may be neglected for
small deflections. Moreover, electromechanical linearization
is being developed [35, 36]. Single-axis devices are therefore
one-degree-of-freedom (1DOF) systems, and their dynamics

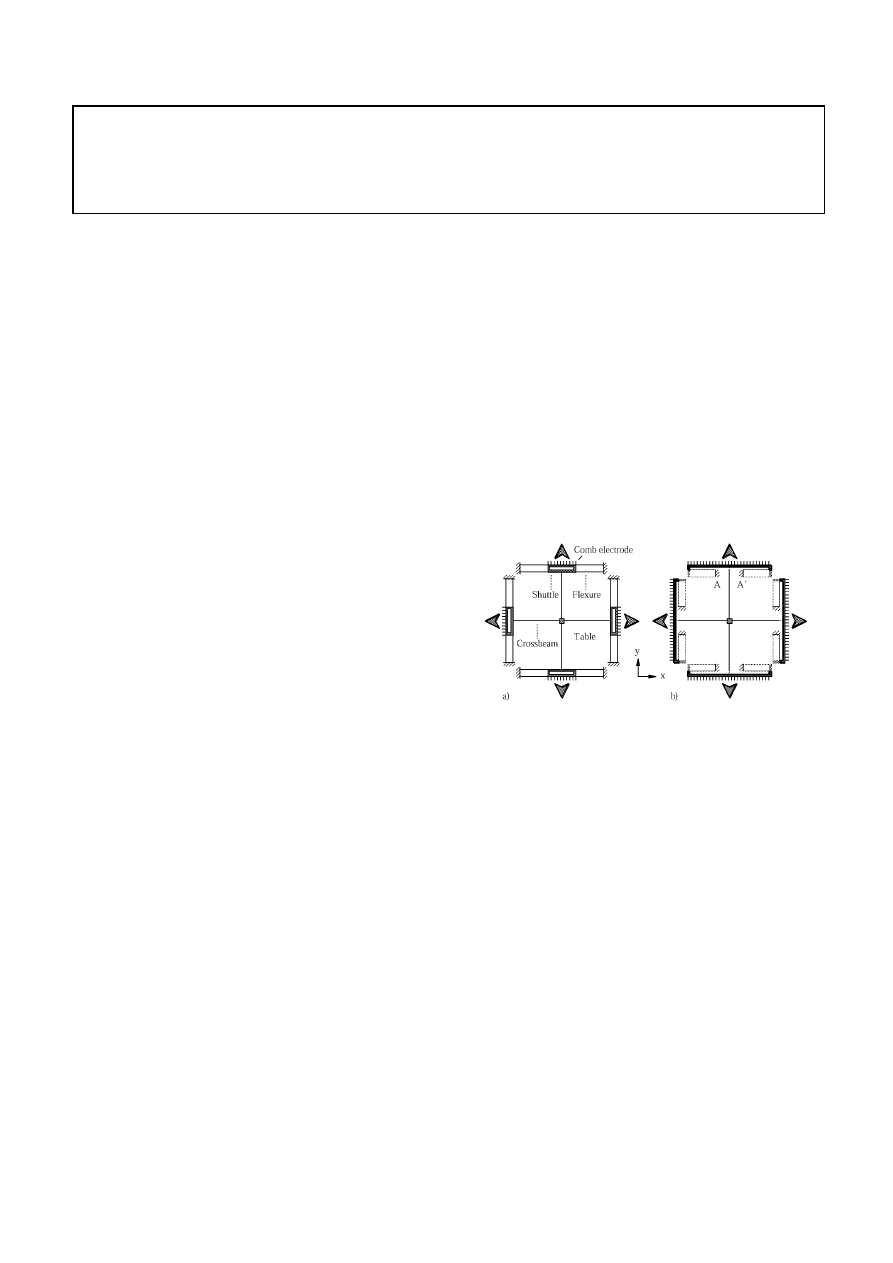
Figure 1.

Dual-axis electrostatic comb-drive actuators with

(a) hammock and (b) folded suspension.

can be described by linear, lumped-element and mass-spring-
damper models [1].

Refinements have generally concerned secondary

effects, such as the accurate modelling of damping [37–39],
electrostatic levitation caused by the substrate [40] or fringing
fields in the drive [41].

More complicated behaviour is expected in multi-

axis stages, since these contain several masses that are
independently suspended but linked by coupling beams.
Consequently they are multi-DOF systems, even for in-plane
motion. For example, figure 1(a) shows an x–y stage similar
to that used in [23, 24]. Here, a table is suspended between
four electrostatic drives by two flexible crossbeams. The
moving part of each drive consists of a shuttle carrying a comb
electrode which is suspended from a ‘hammock’ flexure
formed by four beams [22]. The fixed part of each drive
is omitted for simplicity. The majority of the system mass
lies in the shuttle assemblies. Each is constrained to translate
in one direction, and an additional constraint is provided by
the crossbeams, which force the masses to move in pairs.
Consequently, this is a 2DOF system.

With this design, the electrode can only occupy a small

part of the overall device span.

Figure 1(b) shows an

0960-1317/99/010001+08$19.50

© 1999 IOP Publishing Ltd

1

T Harness and R R A Syms

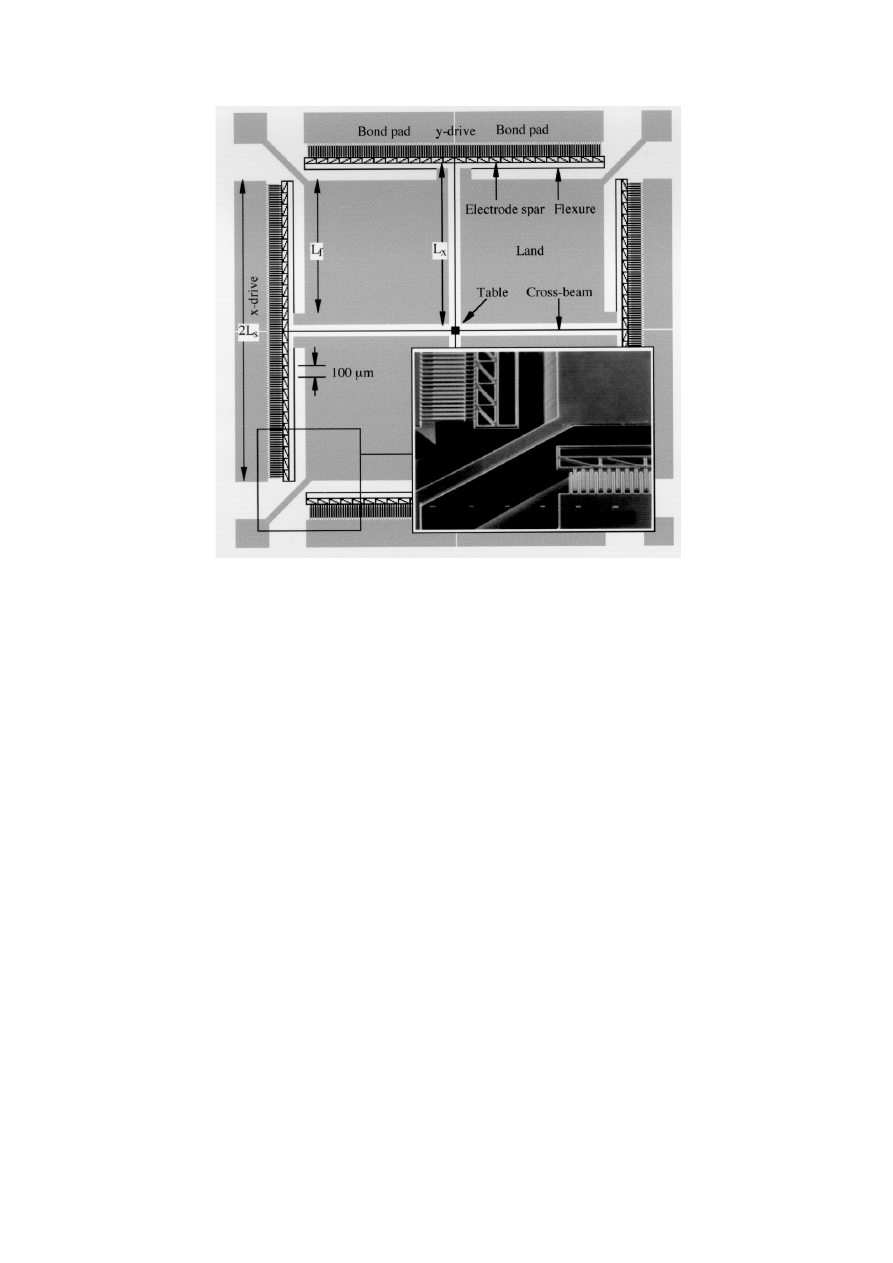
Figure 2.

Experimental layout of an x–y stage with a single-flexure suspension.

alternative, where the hammock is replaced by a folded
suspension.

Here, the electrode covers the entire span,

minimizing the drive voltage, and maximizing the current
obtained in the capacitative readout.

Either a single or

a double flexure may be used at each end of each comb.
However, care must be taken to design the suspension
to prevent rotation of the electrode spars, because linear
translation of the table will cause this rotation to be excited by
the crossbeam. The consequence will be unnecessary energy
dissipation, and the existence of four unwanted vibrational
modes, since the device is now a 6DOF system.

The dynamics of multi-DOF vibrating microsystems

have received very little attention, possibly because limited
motion often prevents the observation of spurious modes
in surface micromachined devices.

In this paper, we

examine the design of x–y stages with the layout of
figure 1(b), using a combination of analytic theory, finite-
element (FE) modelling and experiment [42]. The fabrication
and measurement of the bulk micromachined devices with
a single-flexure suspension are described in section 2. In
section 3, we use a coupled-mode model to demonstrate
the origin of spurious in-plane modes, and show that good
agreement may be obtained with experiment, provided that
the intrinsic stresses are taken into account.

The results

obtained from this model are compared with finite-element
analysis in section 5, and an improved design is presented.

2. Fabrication and measurement of dual-axis
electrostatic actuators

Experiments were performed using diode-isolated, laterally-
resonant, comb-drive electrostatic actuators, fabricated by

bulk micromachining of (100) Si [9]. By using a very deep
(

≈100 µm) undercut etch, this method allows very large

suspended structures without surface tension collapse [43].
Briefly, n-type substrates were doped with boron to form a p

+

etch stop of thickness τ

= 7 µm. This layer was patterned by

reactive ion etching, using a Cr mask, and undercut by etching
in ethylene diamene pyrocatechol. A thin (700 Å) Al contact
layer was then deposited, and the devices were packaged.
Voltages could then be applied between parts based on the
isolation provided by back-to-back p

+

–n junctions, up to

reverse breakdown at

≈15 V.

Figure 2 shows the first design, which had a single-

flexure suspension. The main movable parts are shown in
black, and the fixed parts in grey. The inset is a scanning
electron microscopy (SEM) view of a device near one corner,
showing the large undercut depth. Each electrode spar had
a span of 2L

s

= 2610 µm, and carried N = 127 electrode

fingers 7.6 µm wide by 95 µm long, on a truss formed by two
parallel 10 µm wide beams, separated by 50 µm and linked
by 7.6 µm wide cross-braces. The moving electrodes were
separated from the fixed electrodes by gaps g

= 2.4 µm.

The spars were supported on flexures L

f

= 1155 µm long

and 7.6 µm wide, and the central 75 µm

× 75 µm table was

supported on two crossbeams L

x

= 1407.5 µm long and

6.6 µm wide.

The design was skewed, so that its principle axes were

at 45

◦

to the intersections of the (111) planes with the

wafer surface, to allow rapid undercut of the suspended parts
[9]. During fabrication, the dimensions of most mechanical
parts were reduced by approximately 2 µm, mainly due to
lateral etching of the thick Cr mask during patterning by

2

Electrostatic comb-drive X–Y microactuators

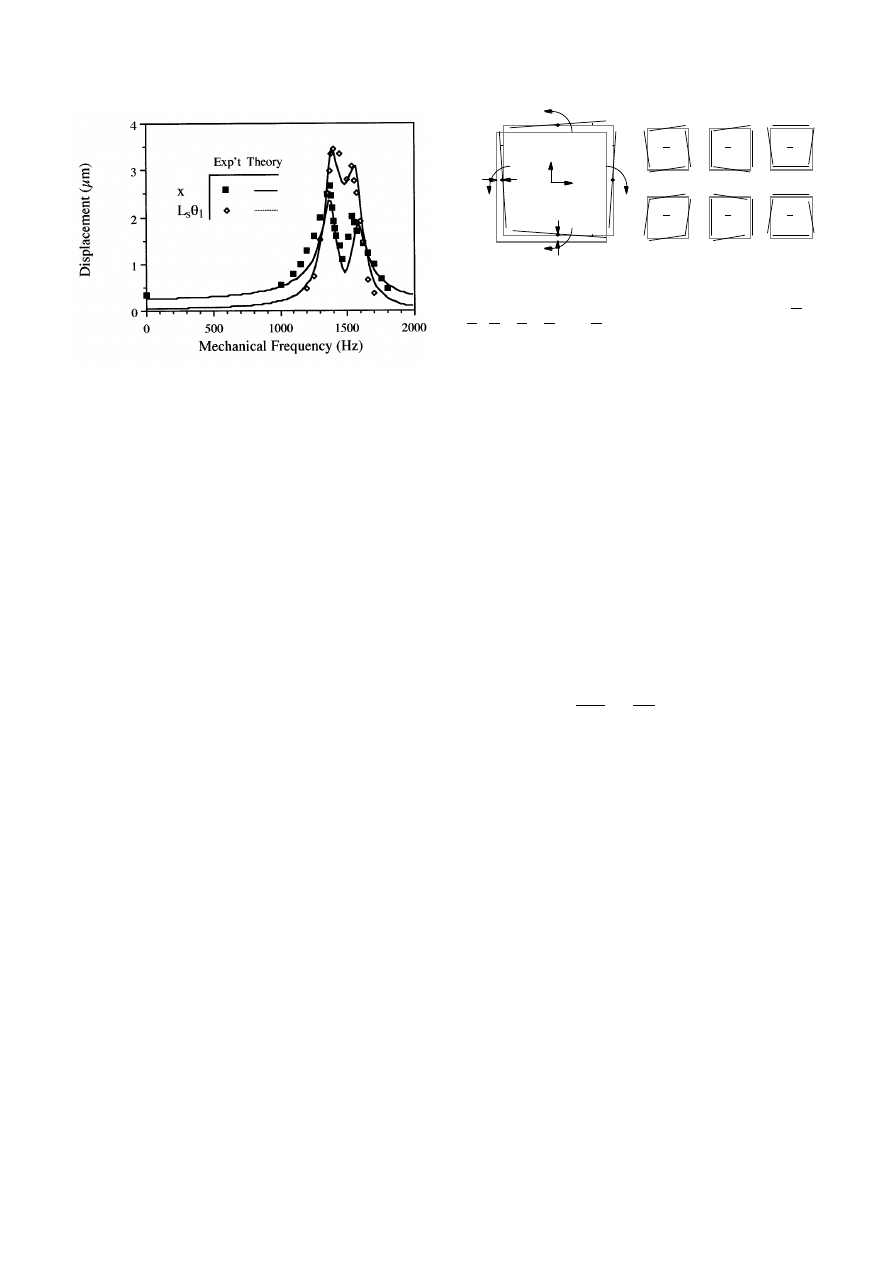
Figure 3.

Variation of linear and coupled angular deflections with

frequency, for an x–y stage with a single flexure suspension. The
points are experimental data and the lines are the best-fits to a
coupled-mode model, including the effects of axial stress on the
suspension.

isotropic wet etching. However, reduced lateral etching of the
electrode fingers was observed, presumably due to proximity.

Because of the large size of the device, the in-plane

motion was correspondingly large, and the deflection of
different parts of the structure could be determined using
an optical microscope. All measurements were performed
in air using sinusoidal drive voltages. When a single comb
electrode was driven, it was found that the central stage
translated linearly as expected.

However, movement of

other parts of the structure was also observed. For linear
motion in (say) the x-direction, the two undriven y-electrode
spars were seen to execute an angular vibration about their
midpoint. Furthermore, the magnitude of this vibration was
large enough to give rise to significant motion of the ends of
the rotating spars.

Figure 3 shows the displacements x (of the driven

x

-electrode) and L

s

θ

1

(of the end of one of the undriven

y

-electrodes) as a function of the mechanical frequency

(twice the electrical drive frequency) for a 10 V drive. The
response L

s

θ

3

of the other y-electrode was similar, but

not identical, implying slight asymmetry.

In each case,

the response is doubly peaked, suggesting the existence of
multiple mechanical resonances. Furthermore, the angular
resonance is sharper than the linear resonance, with a higher
peak, so the unwanted motion is very significant.

The observation of a doubly-peaked response, involving

both linear and angular motions, implies the excitation of
two characteristic modes from a larger set formed by the
coupling together of isolated linear and angular resonances.
As is well known, the relative amplitudes of the different
vibrations in a mode pattern of this type tend to be similar
when the isolated resonances have similar frequencies, i.e. in
a near-synchronous coupled-mode system [44].

A separate experiment was therefore performed to

determine the resonances of a structure consisting of a single
electrode spar, suspended at either end by a single flexure.
The fixed half of the comb drive was divided into two at its
midpoint, so that linear motion of the spar could be excited
by driving the two halves in-phase, and angular motion by

θ

1

θ

2

θ

3

θ

4

x

y

a)

X

3a

X

3b

X

4a

X

4b

X

1

X

2

b)

T

1

T

2

T

3

T

4

F

x

F

y

Figure 4.

(a) Coordinates of the simplified 6DOF model used in

the dynamical analysis and (b) the six allowed mode shapes X

1

,

X

2

, X

3a

, X

3b

, X

4a

and X

4b

.

driving them in anti-phase. As expected, almost identical
resonant frequencies were obtained.

3. Coupled-mode model

To explain the experimental results, we first construct a
simple coupled-mode model for all 6DOF systems with
similar symmetries, and then examine the behaviour implied
by the specific suspension used here. It is assumed that
the system dynamics are dominated by the translation of
two large, lumped-element masses (each comprising a pair
of electrode spars of combined mass 2m, moving together)
and the rotation of four lumped-element inertias (each a
single spar, of moment of inertia J , moving separately),
neglecting the masses of the suspension flexures, crossbeams
and central table. To describe these motions, the coordinate
set of figure 4(a) is assumed. Including viscous damping, the
equations of motion are then

M

d

2

X

dt

2

+

C

dX

dt

+

K

X

= F

(1)

where X

= [x, y, θ

1

, θ

2

, θ

3

, θ

4

]

T

is a 6-element coordi-

nate vector,

M

is a (6

× 6) diagonal matrix with elements

(2m, 2m, J, J, J, J ) describing the masses and moments
of inertia associated with each coordinate,

C

is a diag-

onal matrix containing elements (2c

L

,

2c

L

, c

A

, c

A

, c

A

, c

A

)

associated with damping of linear and angular motion,
F

= [F

x

, F

y

, T

1

, T

2

, T

3

, T

4

]

T

is a 6-element vector describ-

ing the external forces and torques acting in each coordinate
direction, and

K

is a (6

× 6) stiffness matrix. Assuming sym-

metry and reciprocity,

K

must have the form:

K

=














k

11

0

k

13

0

k

13

0

0

k

11

0

k

13

0

k

13

k

13

0

k

33

k

34

k

34

−k

34

0

k

13

k

34

k

33

−k

34

k

34

k

13

0

k

34

−k

34

k

33

k

34

0

k

13

−k

34

k

34

k

34

k

33














(2)

where k

11

, k

13

, k

33

and k

34

are constants that are determined

by the stiffnesses of the electrode spar suspension elements
and the crossbeams.

Rather than attempting to solve the complete 6DOF

problem directly, we note instead that if X and F are chosen
in the principal co-ordinate form X

1

= [0, 0, θ, −θ, −θ, θ]

T

and F

1

= [0, 0, T , −T , −T , T ]

T

, or in the similar form X

2

= [0, 0, θ, θ, −θ, −θ]

T

and F

2

= [0, 0, T , T , −T , −T ]

T

,

3

T Harness and R R A Syms

equations (1) are reduced to the completely uncoupled
equations:

J

d

2

θ

dt

2

+ c

A

dθ

dt

+ k

1

θ

= T

or

J

d

2

θ

dt

2

+ c

A

dθ

dt

+ k

2

θ

= T

(3)

where k

1

= k

33

− 3k

34

and k

2

= k

33

+ k

34

. Assuming the

solution θ

= θ exp (jωt), we may satisfy (3) for c

A

= 0,

T

= 0 (undamped free vibrations) with ω

1

= (k

1

/J )

1/2

(upper) and ω

2

= (k

2

/J )

1/2

(lower). The vectors X

1

=

[0, 0, 2,

−2, −2, 2]

T

and X

2

= [0, 0, 2, 2, −2, −2]

T

are therefore normal modes, with corresponding angular
resonant frequencies ω

1

and ω

2

. These modes are shown in

figure 4(b); they are patterns of pure rotation of the electrode
spars.

In a similar way, choosing X and F as X

3

= [x, 0, θ,

0, θ, 0]

T

and F

3

= [F

x

,

0, T , 0, T , 0]

T

, (1) may be reduced

to the pair of coupled equations given by

M

0

d

2

X

0

/

dt

2

+

C

0

dX

0

/

dt +

K

0

X

0

= F

0

(4)

where X

0

= [x, θ]

T

,

M

0

is a (2

× 2) diagonal matrix with

elements (2m, 2J ),

C

0

is a (2

× 2) diagonal matrix with

elements (2c

L

,

2c

A

), F

0

= [F

x

,

2T ]

T

and

K

0

is the (2

× 2)

stiffness matrix:

K

0

=

k

3

k

4

k

4

k

5

(5)

where k

3

= k

11

, k

4

= 2k

13

and k

5

= 2(k

33

+ k

34

)

= 2k

2

.

Assuming the solution X

0

= X exp(jωt), where X

= [X, 2]

T

, we obtain in the absence of damping, forces and

torques:

{−ω

2

M

0

+

K

0

}X = 0.

(6)

Equation (6) is a standard eigenmode problem [44], with two
solutions that are found from the determinantal equation

k

3

/

2m

− ω

2

k

4

/

2m

k

4

/

2J

k

5

/

2J

− ω

2

= 0.

(7)

Equation (7) predicts the existence of two further natural
frequencies ω

3a

and ω

3b

, given by

ω

2
3a,b

=

1
2

{(k

3

/

2m + k

5

/

2J )

± [(k

3

/

2m

− k

5

/

2J )

2

+ k

2

4

/mJ

]

1/2

}.

(8)

The two natural frequencies have associated eigenvectors X

a

and X

b

, in which the amplitudes 2

a,b

and X

a,b

are related by

2

a,b

= (2m/k

4

)

{ω

2
3a,b

− k

3

/

2m

}X

a,b

.

(9)

Using equation (9), the mode patterns X

3a

and X

3b

corresponding to the natural frequencies ω

3a

and ω

3b

may be found as X

3a

= [X

a

,

0, 2

a

,

0, 2

a

,

0]

T

and

X

3b

= [X

b

,

0, 2

b

,

0, 2

b

,

0]

T

. These patterns are also shown

in figure 4(b); in each case, they combine linear and
symmetric angular motions, but the two motions are in-phase
in X

3a

and in anti-phase in X

3b

.

It is trivial to show that a final choice of X

4

= [0, y, 0,

θ,

0, θ ]

T

and F

4

= [0, F

y

,

0, T , 0, T ]

T

results in a reduction

of (1) to a further pair of coupled equations similar to (4).
These predict two further eigenmodes X

4a

and X

4b

, that again

combine linear and angular motions as shown in figure 4(b),
with natural frequencies ω

4a

= ω

3a

and ω

4b

= ω

3b

.

Simple approximations for the coefficients in the reduced

equations, (3) and (4), namely the values of m, J , c

L

,

c

A

and the stiffness coefficients k

1

–k

4

, are now derived.

Once these are known, the constants in the full 6DOF model
(equation (1)) may be found if necessary, as

k

11

= k

3

k

13

= k

4

/

2

k

33

= (k

1

+ 3k

2

)/

4

k

34

= (k

2

− k

1

)/

4.

(10)

The mass m

= ρAτ, where ρ is the density of Si, A is the

area of the electrode spar pattern and τ is the thickness of the
mechanical layer. The moment of inertia J may be estimated
by considering the spar to be a thin rod of length 2L

s

and mass

m

, rotating about its midpoint, as J

≈ mL

2
s

/

3. Although this

approximation is crude, replacing the rod by a lamina or more
complex shape and assuming rotation about a displaced axis
makes surprisingly little difference.

The coefficient of linear damping c

L

is assumed to be

determined by the measurement of the resonance half-widths.
However, assuming that damping of angular motion has a
similar mechanism, a suitable estimate for c

A

may then be

obtained by integrating the effects of linear damping on a
long thin rod, as c

A

≈ c

L

L

2
s

/

3. Since c

A

/c

L

= J/m, the

system has proportional damping [44].

For the simple suspension used in the experiment, the

stiffness coefficients may be found from beam bending theory
(see e.g. [45, 46]) as follows.

Ignoring the crossbeams,

the stiffnesses k

L

and k

A

of each electrode spar’s flexure

suspension against linear and angular deflections is

k

L

= 2(12EI

f

/L

3
f

)

k

A

= 2[4EI

f

(

1 + 3α + 3α

2

)/L

f

]

(11)

where I

f

is the second moment of area of each flexure,

E

is Young’s modulus and the constant α is given

by α

= L

s

/L

f

− 1. Equation (11) implies that k

A

≈

k

L

L

2
f

/

3 when the flexures are roughly half the length

of the spar (α

≈ 0).

In this case, the resonant

frequencies for the linear and angular motion of an
isolated spar are approximately equal—as was found
experimentally—since (k

L

/m)

1/2

≈ (k

A

/J )

1/2

. This is an

inherent weakness in the design, since it automatically causes
near-synchronicity between the desired linear and undesired
angular resonances.

The terms k

1

and k

2

in (3) both depend on k

A

, but have

additional contributions k

x

1

and k

x

2

from the crossbeams. To

estimate these, we note that the table rotates in the pattern
X

1

of figure 4, but is stationary in X

2

. The extra terms may

thus be found by assuming that the crossbeams have no end
moment (for k

x

1

) and no end rotation (for k

x

2

) at the table, so

k

1

= k

A

+ k

x

1

and

k

2

= k

A

+ k

x

2

(12)

where k

x

1

= 3EI

x

/L

x

and k

x

2

= 4E

I

x/L

x

and I

x

is the

second moment of area of the crossbeams. The remaining
stiffness coefficients k

3

, k

4

and k

5

may then be found in a

similar way, as

k

3

= 2k

L

+ 2k

x

3

k

4

= 2k

x

4

and k

5

= 2k

2

(13)

4

Electrostatic comb-drive X–Y microactuators

Table 1.

Stiffness coefficients predicted by the coupled-mode and FE models.

k

11

k

13

k

33

k

34

Model

(N m

−1

)

(N)

(N m)

(N m)

Coupled mode

0.373

−1.857 × 10

−5

1.071

× 10

−7

1.089

× 10

−9

FE

0.373

−1.718 × 10

−5

1.065

× 10

−7

0.962

× 10

−9

k

1

k

2

k

3

k

4

(N m)

(N m)

(N m

−1

)

(N)

Coupled mode

1.039

× 10

−7

1.082

× 10

−7

0.373

−3.714 × 10

−5

FE

1.036

× 10

−7

1.075

× 10

−7

0.373

−3.435 × 10

−5

Figure 5.

The variation of the linear and coupled angular

deflections with frequency. The points are experimental data and
the lines are the best-fits to the coupled mode model, omitting the
effects of axial stress on the suspension.

where k

x

3

= 12EI

x

/L

3
x

and k

x

4

= −6EI

x

/L

2
x

.

To simulate the excitation by an electrostatic drive,

(4) may be solved by assuming that there is no external
torque (T

= 0), and that F

x

is the electrostatic force

F

x

=

1
2

dC/dx V

2

, where V

= V

0

cos(ωt ) is a harmonic

drive voltage and dC/dx

= 2Nε

0

τ/g

is the differential

of the electrode capacitance, so that F

x

= (Nε

0

τ V

2

0

/

2g)

[1 + cos(2ωt )] [1, 2].

Matching this model above to the data of figure 3

proved surprisingly difficult. The Si material constants of
ρ

= 2330 kg m

−3

and E

= 1.08 × 10

11

N m

−2

were taken

from the literature [47], leaving three parameters—the lateral
etch of the mechanical parts and the electrode fingers, and
the damping constant c

L

—to be adjusted for best agreement.

Figure 5 shows results obtained assuming a lateral etch of
2.0 µm, an electrode etch of 1.6 µm and a damping constant
of c

L

= 1.4 × 10

−6

N s m

−1

, which illustrate the problem:

although a double resonance is predicted, the positions and
heights of the peaks are incorrect.

While it was found

that further parameter adjustment could easily correct the
positions of the peaks, it could not rectify their heights.

An additional affect was therefore sought to explain

the discrepancy. It is well known that the level of boron
doping used in bulk machining causes shrinkage of the Si
lattice [46]. As a result, the suspension anchor points (e.g.
A and A

0

in figure 1(b)) are forced radially outward from

their relaxed positions by the substrate beneath. The most
significant consequence is that the flexures are placed under
compression.

Using standard beam bending theory (e.g.

Table 2.

Resonant frequencies predicted by the coupled mode and

finite element models.

f

3a

= f

4a

f

1

f

2

f

3b

= f

4b

Model

(Hz)

(Hz)

(Hz)

(Hz)

Coupled mode

1377

1455

1485

1571

FE

1361

1418

1444

1541

[45]), it can be shown that the effect of a compressive axial
load P is to modify the stiffness coefficients for linear and
angular deflections (equation (11)) to new values k

0

L

and k

0

A

,

given by

k

0

L

= k

L

[1

− a

L

(P L

2
f

/EI

f

)

]

with a

L

=

1

10

k

0

A

= k

A

[1

− a

A

(P L

2
f

/EI

f

)

]

with a

A

=

17
60

(

1

− 18α/17 + 21α

2

/

17).

(14)

For the dimensions here, a

A

≈

1
4

, so the linear and

angular stiffnesses have different sensitivities to axial force.
Axial loading can then adjust the synchronism between
the uncoupled resonances (k

0

L

/m)

1/2

and (k

0

A

/J )

1/2

. This

factor was found to be crucial in predicting the observed
response, and figure 3 actually shows the prediction for
P L

2
f

/EI

f

= 0.7 (corresponding to P = 5.8 µN, or an axial

stress σ

= 1.48 × 10

5

N m

−2

), with the other parameters

as in figure 5. The improvement in agreement is dramatic,
and the model now predicts all of the essential features of the
data.

The numerical values of the stiffnesses k

11

, k

13

, k

33

, k

34

and k

1

–k

4

are given in table 1, and the characteristic mode

resonant frequencies f

1

, f

2

, f

3a

and f

3b

corresponding to ω

1

,

ω

2

, ω

3a

and ω

3b

are shown in table 2, for comparison with

the results of the next section.

4. Finite-element analysis

The results obtained from the model of the previous
section were compared with numerical values obtained
from a FE model.

The shape of the structure was

first extracted from the computer assisted diagram (CAD)
pattern, allowing for lateral etching, and the electrode
spars were approximated as equivalent laminae.

The

inertial effect of the suspension flexures and the central
table were initially suppressed, but an axial load on the
flexures was included. FE simulations were performed using
ANSYS53 software, available commercially from ANSYS
Incorporated. The structure was meshed using PLANE42
elements, and calculations were performed by the wavefront
solution procedure, using the ANSYS prestress option.

5

T Harness and R R A Syms

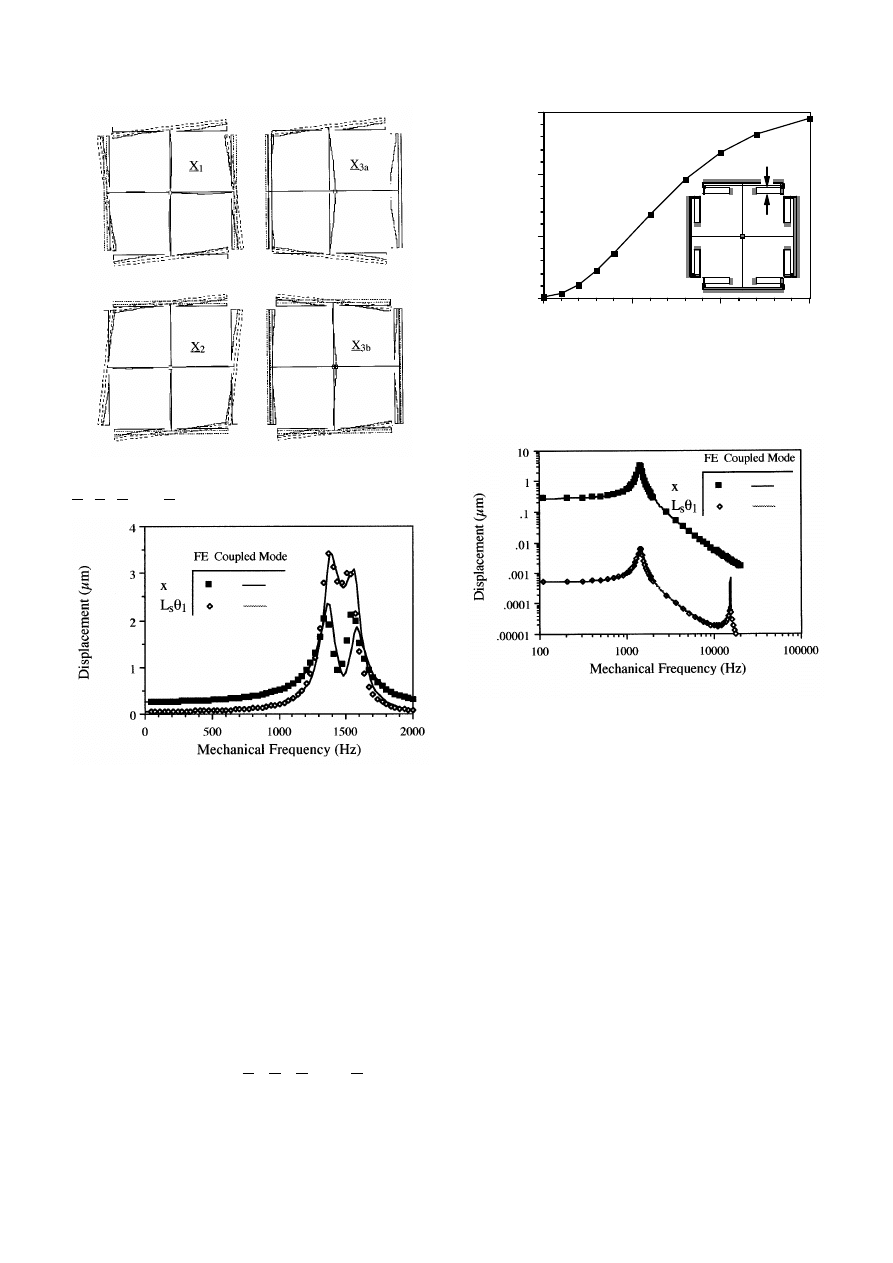
Figure 6.

The shapes predicted by the FE model for the modes

X

1

, X

2

, X

3a

and X

3b

.

Figure 7.

Comparison between the predictions of the

coupled-mode and FE models for the variation of linear and
coupled angular deflections with frequency.

The stiffness coefficients used in the analytic theory were

first compared with values extracted from the FE model.
To do so, an arbitrary force was applied to (say) the x-
electrode spar, while holding the y-electrode spars clamped.
From the deflection of the spar, the stiffness coefficient k

3

may be computed. By repeating the experiment, with the off-
driven spars free, the stiffness coefficient k

4

may be found,

and so on for the other stiffness coefficients. A comparison
of the results is given in table 1; generally, there is good
agreement, with maximum discrepancies of around 8%.

The normal mode shapes and resonant frequencies

predicted by the two models were then compared. Figure 6
shows the mode shapes for X

1

, X

2

, X

3a

and X

3b

obtained

from the FE model, which are clearly in agreement with
figure 4.

The corresponding resonant frequencies are

compared in table 2; again, there is reasonable agreement
between the two models.

The frequency responses predicted by the two methods

were then compared. To include damping in the FE model,

150

100

50

0

0

50

100

150

Separation (µm)

k30

Angular stiffness ratio k

AD

/k

AS

Flexure separation

δ

(

µ

m)

δ

Figure 8.

Variation of the stiffness ratio k

AD

/ k

AS

of a

double-flexure suspension with flexure separation δ, as predicted
by the FE model.

Figure 9.

Variation of the linear and coupled angular deflections

with frequency, for an x–y stage with a double-flexure suspension,
as predicted by the coupled-mode and FE models.

proportional damping of the form [C]

= α[M] was used,

where [C] and [M] are the FE damping and stiffness matrices,
respectively [44]. The value of the constant mass matrix
multiplier was taken as α

= c

L

/m

for consistency with

previous results. Figure 7 shows the variation of the linear
and angular deflections predicted by the FE model for
excitation of the x-drive electrode, together with results from
the coupled-mode model taken from figure 3.

Excellent

agreement is obtained between the two, suggesting that the
coupled-mode model is indeed a useful approximation.

Using ANSYS, the effects of a number of factors,

that are neglected in the coupled-mode model were
investigated.

Inclusion of the inertia of the suspension

flexures and crossbeams lowered the natural frequencies
by approximately 50 Hz, but this effect was difficult
to distinguish from similar changes caused by altering
the suspension lateral etch parameter by small amounts.
Inclusion of the inertia of the central table gave rise to a
seventh resonance, involving angular motion of the table, at
a much higher frequency. Consideration of the third (out-of-
plane dimension) again gave rise to higher-order modes, the
lowest being at around 3 kHz.

6

Electrostatic comb-drive X–Y microactuators

5. Discussion

We have shown that MEMS x–y stages are generally multi-
DOF mechanical systems, and that their in-plane dynamics
may be adequately explained by simple coupled-mode
models. Good agreement with experimental data from bulk
micromachined Si devices may be obtained, provided that
the effect of the intrinsic stress is included.

The strong mode coupling in the design investigated

here is due to the near-synchronicity of linear and angular
resonances of the isolated electrode spars on their individual
suspensions.

Improved performance will be obtained if

the excitation of the angular vibrations is suppressed as
far as possible.

This may be achieved by redesigning

the suspension to increase its angular stiffness k

A

, while

maintaining its linear stiffness k

L

.

The simplest modification is to replace the single-flexure

suspension by twin flexures, as shown, earlier, in figure 1(b),
reducing their widths to give the same combined linear
stiffness.

Figure 8 shows the variation of k

AD

/ k

AS

(the

ratio of the angular stiffnesses k

AD

and k

AS

for the double-

and single-flexure systems) predicted by the FE model, as
a function of the flexure separation δ, omitting the effect
of intrinsic stress for the sake of simplicity. For a modest
separation (δ

≈ 100 µm), k

AD

is approximately 115 times

larger than k

AS

.

The result is to increase the resonant

frequency for uncoupled angular motion approximately ten-
fold.

Figure 9 shows the frequency responses predicted

by both the coupled-mode and the FE model, plotted on
an expanded logarithmic scale.

Little angular motion is

excited, showing that this modification leads to performance
effectively similar to a 2DOF system, as required.

Acknowledgments

The authors are extremely grateful to Dr T J Tate for
performing the boron doping. The support of EPSRC through
grant GR/L28814 is also gratefully acknowledged.

References

[1] Tang W C, Nguyen T C H and Howe R T 1989 Laterally

driven polysilicon resonant microstructures Sensors
Actuators 20 25–32

[2] Tang W C, Nguyen T C H, Judy M W and Howe R T 1990

Electrostatic comb drive of lateral polysilicon resonators
Sensors Actuators A 21–23 328–31

[3] Furuhata T, Hirano T, Gabriel K J and Fujita H 1991

Sub-micron gaps without sub-micron etching Tech. Digest,
IEEE Micro Electro Mechanical Systems Workshop (Nara,
Japan, January 30–February 2, 1991) pp 57–62

[4] Hirano T, Furuhata T, Gabriel K J and Fujita H 1991

Operation of sub-micron electrostatic comb-drive
actuators Proc. 6th Int. Conf. on Solid State Sensors and
Actuators (San Francisco, CA, June 23–27, 1991) pp 1–4

[5] Jaecklin V P, Linder C, de Rooij N F and Moret J M 1992

Micromechanical comb actuators with low driving voltage
J. Micromech. Microeng. 2 250–5

[6] Zhang Z L and MacDonald N C 1992 A RIE process for

submicron silicon electromechanical structures
J. Micromech. Microeng. 2 31–8

[7] Shaw K A, Zhang Z L and MacDonald N C 1994

SCREAM I: a single-mask, single-crystal silicon, reactive

ion etching process for microelectromechanical structures
Sensors Actuators A 40 63–70

[8] Gianchandani Y B and Najafi K 1992 A bulk silicon

dissolved wafer process for micro-electromechanical
devices J. Microelectromech. Syst. 1 77–85

[9] Syms R R A, Hardcastle B M and Lawes R A 1997 Bulk

micromachined silicon comb-drive electrostatic actuators
with diode isolation Sensors Actuators A 63 61–7

[10] Selvakumar A and Najafi K 1994 High density vertical comb

array microactuators fabricated using a novel
bulk/poly-silicon trench refill technology Tech. Digest,
Solid State Sensors and Actuators Workshop
(Hilton Head, SC) pp 138–41

[11] Hirano T, Kobayashi D, Furuhata T and Fujita H 1994

Electroplated and dry-released metallic microstructures
for a lateral tunneling unit Japan. J. Appl. Phys. 33 1202–8

[12] Frazier A B, Chong H A and Allen M G 1994 Development

of micromachined devices using polyimide-based process
Sensors Actuators A 45 47–55

[13] Lee A P and Pisano A P 1992 Polysilicon angular

vibromotors J. Microelectromech. Syst. 1 70–6

[14] Daneman M J, Tien N C, Olgaard O, Pisano A P, Lau K Y

and Muller R S 1996 Linear vibromotor for positioning
optical components J. Microelectromech. Syst. 5 159–65

[15] Garcia E J and Sniegowski J J 1995 Surface micromachined

microengine Sensors Actuators A 48 203–14

[16] Uenishi Y, Honma K and Nagaoka S 1996 Tunable laser

diode using a nickel micromachined external mirror Elect.
Lett. 32 1207–8

[17] Kiang M-H, Solgaard O, Lau K Y and Muller R S 1998

Electrostatic combdrive-actuated micromirrors for
laser-beam scanning and positioning J. Microelectromech.
Syst. 7 27–37

[18] Tanaka K, Mochida Y, Sugimoto M, Moriya K, Hasegawa T,

Atsuchi K and Ohwada K 1995 A micromachined
vibrating gyroscope Sensors Actuators A 50 111–15

[19] Welham C J, Gardner J W and Greenwood J 1996 A laterally

driven micromachined resonant pressure sensor Sensors
Actuators A 52 86–91

[20] Lin L, Howe R T and Pisano A P 1998

Microelectromechanical filters for signal processing
J. Microelectromech. Syst. 7 286–94

[21] Yao J J, Arney S C and MacDonald N C 1992 Fabrication of

high frequency two-dimensional nanoactuators for
scanned probe devices Microelectromech. Syst. 1 14–22

[22] Jaecklin V P, Linder C, de Rooij N F, Moret J M, Bischof R

and Rudolf F 1992 Novel polysilicon comb actuators for
xy

-stages Micro Electro Mechanical Syst. (MEMS ’92)

(Travem¨unde, Germany, Feb. 4–7 1992) pp 147–9

[23] Inderm¨uhle P-F, Linder C, Brugger J, Jaecklin V P and

de Rooj N F 1994 Design and fabrication of an
overhanging xy-microactuator with integrated tip for
scanning surface profiling Sensors Actuators A 43 346–50

[24] Inderm¨uhle P-F, Jaecklin V P, Brugger J, Linder C,

de Rooij N F and Binggeli M 1995 AFM imaging with an
xy

-micropositioner with integrated tip Sensors Actuators

A 46–47 562–5

[25] McNie M E, King D O, Ward M C L, Blackstone S, Quinn C

and Burdess J 1997 Deep reactive ion etching of SOI for
silicon micromachined structures Proc. Digest, Intl. SOI
Conference (Yosemite, October 1997) pp 60–1

[26] Geiger W, Folkmer B, Sobe U, Sandmaier H and Lang W

1998 New design of micromachined vibrating rate
gyroscope with decoupled oscillation modes Sensors
Actuators A 66 118–24

[27] Benitez A, Esteve J and Bausells J 1995 Bulk silicon

microelectromechanical devices fabricated from
commercial bonded and etched-back silicon-on-insulator
substrates Sensors Actuators A 50 99–103

[28] Klaassen E H, Petersen K, Noworolski J M, Logan J,

Maluf N I, Brown J, Storment C, McCulley W and
Kovacs T A 1996 Silicon fusion bonding and deep

7

T Harness and R R A Syms

reactive ion etching: a new technology for microstructures
Sensors Actuators A 52 132–9

[29] Bhardwaj J and Ashraf H 1995 Innovative silicon trench

process proves a winner Surface Technology Systems
Newsletter July

[30] Kong S, Minami K and Esashi M 1997 Fabrication of

reactive ion etching systems for deep silicon machining
Trans. Inst. Electr. Eng. Japan. E 117 10–14

[31] Fan L S, Woodman S J and Crawforth L 1995 Integrated high

aspect ratio milliactuators Sensors Actuators A 48 221–7

[32] de Graaf G, Wolffenbuttel M R and Wolfenbuttel R F 1993

A measurement system for the visual analysis of
integrated micromechanical systems Sensors Actuators A
37–38 772–8

[33] Zook J D, Burns D W, Guckel H, Sniegowski J J,

Engelstad R L and Feng Z 1992 Characteristics of
polysilicon resonant microbeams Sensors Actuators A 35
51–9

[34] Pember A, Smith J and Kemhadjian H 1995 Long-term

stability of silicon bridge oscillators fabricated using the
boron etch stop Sensors Actuators A 46–47 51–7

[35] Adams S G, Bertsch F and MacDonald N C 1998

Independent tuning of linear and nonlinear stiffness
coefficients J. Microelectromech. Syst. 7 172–80

[36] Adams S G, Bertsch F, Shaw K A, Hartwell P G, Moon F C

and McDonald N C 1998 Capacitance based tunable
resonators J. Micromech. Microeng 8 15–23

[37] Hosaka H, Itao K and Kuroda S 1994 Evaluation of energy

dissipation mechanisms in vibrational microactuators
IEEE Micro Electro Mechanical Systems Workshop
(MEMS’94) (January 25–28, 1994) pp 193–8

[38] Zhang H and Tang W C 1994 Viscous air damping in

laterally driven microresonators IEEE Micro Electro
Mechanical Systems Workshop (MEMS’94) (January
25–28, 1994) pp 199–204

[39] Cho Y-H, Pisano A P and Howe R T 1994 Viscous damping

model for laterally oscillating microstructures
J. Microelectromech. Syst. 3 81–6

[40] Tang W C, Lim M G and Howe R T 1992 Electrostatic comb

drive levitation and control method J. Microelectromech.
Syst. 1 170–8

[41] Johnson W A and Warne L K 1995 Electrophysics of

micromechanical comb actuators J. Microelectromech.
Syst. 4 49–59

[42] Harness T and Syms R R A 1999 Spurious mode suppression

in electrostatic comb-drive X–Y microactuators IEE
Colloquium on ‘Microengineering, Modelling and
Design’ (Savoy Place, London, March 4, 1999)

[43] Mastrangelo C H and Hsu C H 1993 Mechanical stability

and adhesion of microstructures under capillary
forces—Part I J. Microelectromech. Syst. 2 33–43

Mastrangelo C H and Hsu C H 1993 Mechanical stability

and adhesion of microstructures under capillary
forces—Part II J. Microelectromech. Syst. 2 44–55

[44] Thomson W T 1993 Theory of Vibration with Applications

4th edn (London: Chapman and Hall)

[45] Young W C 1989 Roark’s Formulas for Stress and Strain

6th edn (New York: McGraw Hill International)

[46] Timoshenko S, Young D H and Weaver W 1974 Vibration

Problems in Engineering 4th edn (New York: Wiley)

[47] Zhang L M, Uttamchandani D and Culshaw B 1991

Measurement of the mechanical properties of silicon
microresonators Sensors Actuators A 29 79–84

8