Drying kinetics of prickly pear fruit (Opuntia ficus indica)

S. Lahsasni

a

, M. Kouhila

b,*

, M. Mahrouz

a

, J.T. Jaouhari

b

a

Unit

ee de Chimie Agroalimentaire (LCOA), Facult

ee des Sciences Semlalia, BP 2390, Marrakech, Morocco

b

Laboratoire d’Energie Solaire et Plantes Aromatiques et M

eedicinales, Ecole Normale Sup

eerieure, BP 2400, Marrakech, Morocco

Received 24 October 2002; accepted 12 March 2003

Abstract

The present work examines the effect of drying air conditions on drying kinetics of the prickly pear fruit in a convective solar

drier operating with an auxiliary heating system under air controlled conditions. Moreover, the prickly pear fruits are sufficiently
dried in the ranges between 32 and 36

°C of ambient air temperature, 50–60 °C of drying air temperature, 23–34% of relative

humidity, 0.0277–0.0833 m

3

/s of drying air flow rate and 200–950 W/m

2

of solar radiation. The results verified with good repro-

ducibility that drying air temperature is the main factor in controlling the drying rate and the experimental drying curves show only
a falling rate period. The expression of the drying rate equation is determined empirically from the characteristic drying curve. Eight
different thin layer drying models were compared according to their coefficients of determination to estimate solar drying curves.
The two-term model was found to satisfactorily describe the solar drying curves of prickly pear fruit with a correlation coefficient (r)
of 0.9999. The constants and coefficients of this model could be explained by the effect of drying air temperature with a correlation
coefficient (r) of 1.0000.
Ó 2003 Elsevier Ltd. All rights reserved.

Keywords: Characteristic drying curve; Drying curves; Modeling; Prickly pear fruit; Solar dryer

1. Introduction

The cactus pear (prickly pear) grows in all parts of the

American continent, from southern Canada to Pata-
gonia, and in the course of time has been cultivated in
different areas of Europe, particularly in the Mediter-
ranean countries, as well as in Africa and Australia.
Many different species of Opuntia are grown in Mexico
for fruit production whereas in Italy and the Mediter-
ranean region in general, Opuntia ficus indica is culti-
vated. Thanks to its ability to adapt to different
environmental conditions, the cactus pear grows in
plains, coastal regions, plateaus and among diverse
vegetation. A common feature of the areas where the
plant grows is a more or less marked degree of aridity to
which the plant has adapted thanks to its CAM photo-
synthetic metabolism (Feitosa-Teles, 1977).

Prickly pear fruit is a fleshy, and polyspermic unilo-

cular berry. The major components of the fruit pulp are
85% of water, 10–15% of carbohydrates, and substantial
amounts of vitamin C, 0.025–0.030% (Gurrieri et al.,

2000). Its nutritional value lies essentially in its glucose
and fructose content (6–8%) (Habibi, Mahrouz, &
Vignon, 2002). The level of ascorbic acid is moderate
(0.023%); and acidity is low (0.06%). The prickly pear can
be used in many ways in diverse sectors, utilizing different
parts of the plant. In the food sector, besides consump-
tion of the fresh fruit, jams, alcoholic, soft drinks, syrups,
candied fruit, and flour can be produced from the plant
and oil extracted from the seeds. The vegetable stems
(cladode) and fruits of prickly pear are useful to treat
diabetes, high blood cholesterol levels, inflammation and
obesity (Galati, Monforte, Tripodo, dÕAquino, & Mon-
dello, 2001; Park, Kahng, Lee, & Shin, 2001).

The objectives of the study were to determine the

effect of drying air temperature and air flow rate on
the drying kinetics of the prickly pear fruit, and to select
the best mathematical model for the drying curves.

2. Materials and methods

2.1. Materials

The prickly pear fruit used in the drying experiments

was grown in the region of Bengrir (near the town of

Journal of Food Engineering 61 (2004) 173–179

www.elsevier.com/locate/jfoodeng

*

Corresponding author. Tel.: [phone]; fax: [phone]-

22-87.

E-mail address:

[e-mail]

(M. Kouhila).

0260-8774/$ - see front matter

Ó 2003 Elsevier Ltd. All rights reserved.

doi:10.1016/S0260-8774(03)00084-0

Marrakech). Harvest period was between June and
October 2002. Marrakech is situated 140 km east of the
Atlantic, north of the High Atlas. Its geographic coor-
dinates are 8

° 2

0

west, 31

° 37

0

north and 463 m over the

sea level. Summer in Marrakech is hot and dry while
winter is temperate and humid with occasional rains
(Idlimam, Kaoua, Alem, & Daguenent, 1994).

The experimental apparatus consists of an indirect

forced convection solar dryer with a solar air collector,
an auxiliary heater, a circulation fan and a drying cab-
inet as shown in Fig. 1. The solar air collector had di-
mensions of 1 m by 2.5 m. A corrugated galvanised iron
sheet painted black was used as an absorber plate for
absorbing the incident solar radiation. It was oriented
southward under the collector angle of 31

°. This angle

was fixed by the control foot. A glass and plastic sheet
was used as a transparent cover for the air heater to
prevent the top heat losses. The frame was made of
wood. The drying cabinet was constructed with insulted
walls (dimensions, 1.40 m (length), 0.5 m (width), and
0.90 m (depth)) and has 10 shelves. A centrifugal ven-
tilator (0.0833 m

3

/s; 80 mm CE, 220 V) connected to the

north side of the drying cabinet provides a maximum air
velocity of 1.7 m/s and allowed to vary the drying air
flow rate from 0.0227 to 0.0833 m

3

/s. The circulation fan

to supply fresh air has a power of 0.1 kW. The auxiliary
heater has a power of 4 kW. It was connected to the inlet
of control box.

2.2. Experimental procedure

The drying materials were cut in the bits of 1

0.1 g

weight. The major diameter and length were 0.5

0.01

cm, and 2

0.03 cm, respectively. The loading density of

the drying trays was 3 kg/m

2

for prickly pear fruit. In the

experiments, the 2nd and 10th shelves were not selected
for the efficient utilisation of drying air. However, the
samples were uniformly spread evenly on a drying tray
that was then placed on the first shelf of the drying
cabinet. The heated air enters the drying cabinet below
the trays and flowed upwards trough the samples. The
amounts of solar radiation were measured with Kip-
Zonen solarmeter. Temperature measurements and re-
cordings at different points in the solar dryer were made
by Cr–alumel thermocouples (0.2 mm diameter) con-
nected to a data-logger enabling

0.1 °C accuracy and

the outlet temperatures were measured with thermo-
meter. The relative humidities were measured by ca-
pacitance sensors. These values were determined by
probes Humicolor

2%. A digital weighing apparatus

(

0.001 g) measures the mass loss of the product during

the drying process.

Nomenclature

CDC

characteristic drying curve

ðdM=dtÞ

0

initial drying rate (kg water/(kg dry

matter min))

dM=dt drying rate at any time of drying (kg water/

(kg dry matter min))

Dv

drying air flow rate (m

3

/s)

Exp

experiment

f

dimensionless drying rate

M

moisture content at any time of drying (kg
water/kg dry matter)

M

f

final moisture content (kg water/kg dry mat-
ter)

M

e

equilibrium moisture content (kg water/kg
dry matter)

MR

moisture ratio

M

0

initial moisture content (kg water/kg dry
matter)

N

number of observations

n

number of constants

r

correlation coefficient

Rh

relative humidity (%)

S

r

standard error

t

drying time (min)

T

drying air temperature (

°C)

v

reduced chi-square

Fig. 1. Schematic representation of the solar dryer. (1) Solar collector;
(2) direction of fan; (3) fan; (4) direction of aspiration; (5) control-box;
(6) auxiliary heating system; (7) shelves; (8) drying cabinet; (9) recy-
cling air; (10) control foot; (11) exit of air; (12) humidity probes;
(13) thermocouples.

174

S. Lahsasni et al. / Journal of Food Engineering 61 (2004) 173–179

2.3. Fitting of the solar drying curves

Nine experiments of prickly pear fruit were per-

formed by using the solar drying at T

¼ 50, 55, and 60

°C, and Dv ¼ 0:0227, 0.0556, and 0.0833 m

3

/s which

correspond to the average velocity of drying air in the
drying cabinet 0.5667, 1.1333 and 1.7 m/s, respectively.
The solar drying curves were fitted with eight different
moisture ratio equations (Table 1) (Basunia & Abe,
2001; Hassan & Hobani, 2000; Jayas, Cenkowski, Pabis,
& Muir, 1991; Mujumdar, 1987; Togrul & Pehlivan,
2002; Yaldiz & Ertekin, 2001; Yaldiz, Ertekin, & Uzun,
2001).

The moisture ratio MR can be calculated as:

MR

¼

M

M

e

M

0

M

e

ð1Þ

It is particularly emphasized that the correlation co-

efficient r is one of the primary criteria to select the best
equation to account for the variation in the solar drying
curves of the dried samples (Midilli & Kucuk, 2003). In
addition to r, the reduced chi-square (v

2

) was used to

determine the best of the fit (Togrul & Pehlivan, in
press).

Chi-square can be calculated as:

v

2

¼

P

N
i

¼1

ðMR

exp;i

MR

pre;i

Þ

2

N

n

ð2Þ

where MR

exp;i

is the ith experimental moisture ratio,

MR

pre;i

the ith predicted moisture ratio, N the number of

observations and, n the number of constants.

In this study, the relationship between the drying air

temperature and the coefficients of the best suitable
model was also determined. In order to determine the
most suitable model for prickly pear fruit, Marquardt-
Levenberg non-linear optimisation method, using the
computer program ‘‘curve Expert 3.1’’ was used.

3. Results and discussion

The solar drying experiments were carried out dur-

ing the period of June and July 2002 in Marrakech,
Morocco. Each experiment started at 8:30 a.m. and
continued until 6:00 p.m.

A total of nine drying experiments were run at dif-

ferent air conditions. During the experiments, solar ra-
diation changed between 200 and 950 W/m

2

, ambient

air temperature ranged from 32 to 36

1 °C, ambient

air relative humidity from 23 to 34

2%, inlet drying air

temperature from 50 to 60

0:1 °C, and drying air flow

rate from 0.0227 to 0:0833

0:002 m

3

/s. The initial

moisture content of the prickly pear fruit ranged from
5.9719 to 4.9336 kg water per kg dry matter and was
reduced to the final moisture content which varies from
0.0722 to 0.0297 kg water per kg dry matter (Table 2).

3.1. Determination of the drying curves

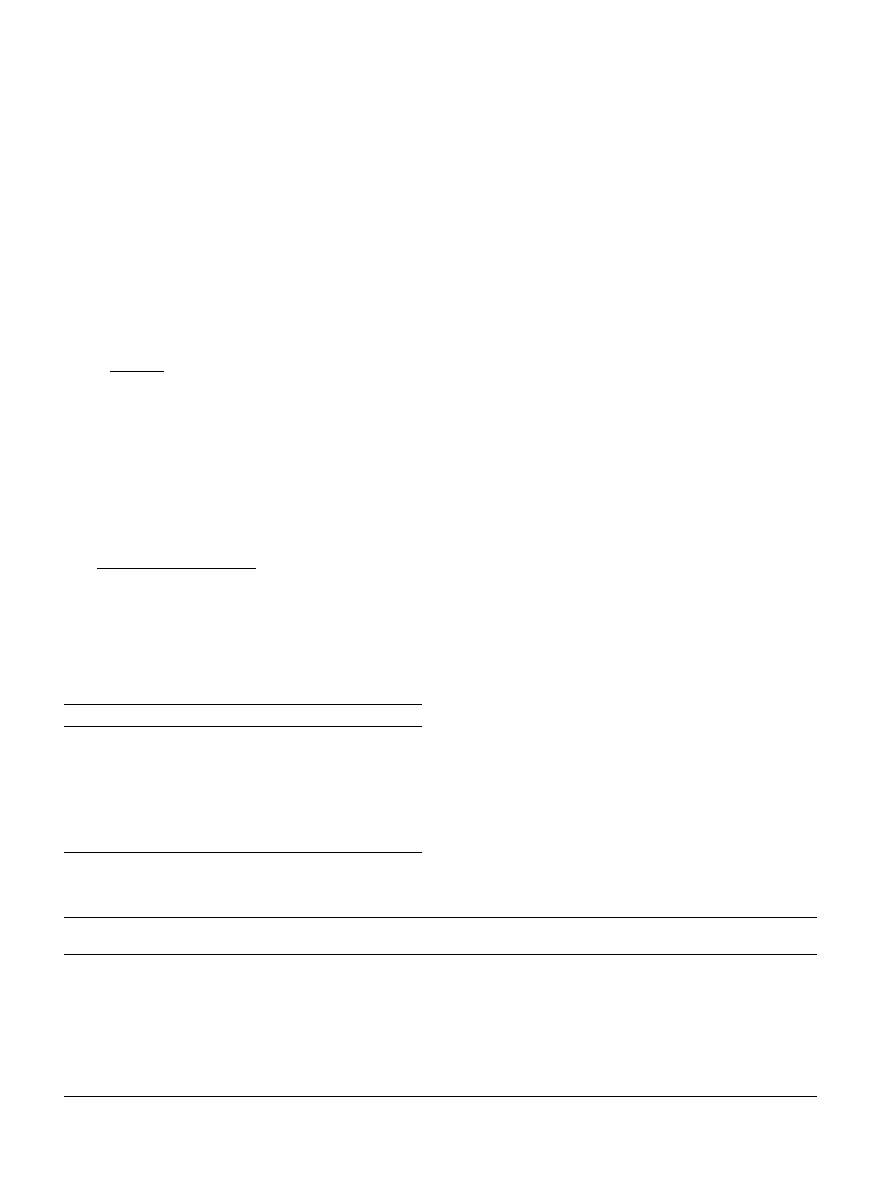
Fig. 2 shows the hourly variation of the measured

solar radiation of a typical summer day (23 June 2002).

The moisture content versus drying time and the

drying rate versus moisture ratio are shown in Figs. 3 and
4, respectively. The constant rate period is absent in the
solar drying of prickly pear fruit. The drying process
took place in the falling rate period. Drying rate de-
creases continuously with diminishing moisture ratio.
These results are in agreement with the observations
of earlier researchers (Bellegha, Amami, Farhat, &
Kechaou, 2002; Kouhila, 2001). Drying during the fall-
ing rate period is so governed by water diffusion in the
solid. This is a complex mechanism involving water in
both liquid and vapour states, which is very often

Table 1
Mathematical models applied to the drying curves

Model name

Model

Newton

MR

¼ expðktÞ

Page

MR

¼ expðkt

n

Þ

Modified Page

MR

¼ expððktÞ

n

Þ

Henderson and Pabis

MR

¼ a expðktÞ

Logarithmic

MR

¼ a expðktÞ þ c

Two-term

MR

¼ a expðk

0

t

Þ þ b expðk

1

t

Þ

Two-term exponential

MR

¼ a expðktÞ þ ð1 aÞ expðkatÞ

Wang and Singh

MR

¼ 1 þ at þ bt

2

Table 2
Drying conditions during experiments in the solar dryer

Experiment
number

Dv

0:002 (m

3

/s)

Inlet T

0:1 (°C)

Outlet Rh

2 (%)

M

0

(kg/kg dry

matter)

M

e

(kg/kg dry

matter)

M

f

(kg/kg dry

matter)

t

(min)

1

0.0227

50

42

5.4302

0.2536

0.0722

476

2

0.0227

55

46

5.9719

0.4026

0.0679

315

3

0.0227

60

38

5.5468

0.2317

0.0411

265

4

0.0556

50

38

5.2920

0.2375

0.0297

425

5

0.0556

55

31

4.9336

0.2067

0.0535

272

6

0.0556

60

36

4.9702

0.2221

0.0410

225

7

0.0833

50

32

5.4034

0.2106

0.0348

285

8

0.0833

55

35

5.4778

0.2239

0.0384

225

9

0.0833

60

30

5.4484

0.1948

0.0407

195

S. Lahsasni et al. / Journal of Food Engineering 61 (2004) 173–179

175

characterised by a so-called Ôeffective diffusivityÕ (Al
Hodali, 1997). These results indicated that diffusion is
the most likely physical mechanism governing moisture
movement in the prickly pear fruit.

3.2. Influence of temperature and air flow rate

In order to study the effect of air conditions, it was

observed that the main factor influencing drying kinetics
is the drying air temperature, as noted in other studies
(Belghit, Kouhila, & Boutaleb, 2000; Kechaou, Bagane,
Maalej, & Kapseu, 1996; Kouhila, Kechaou, Otmani,
Fliyou, & Lahsasni, 2002). Thus, a higher drying air
temperature produced a higher drying rate and conse-
quently the moisture ratio decreased (Fig. 5). This is due
to the increase of the air heat supply rate to the product
and the acceleration of water migration inside the
prickly pear fruit.

The drying rate does not vary a lot as a function of air

flow rate which seems to have a less important effect
than the drying air temperature (Fig. 6), as noted in
other studies (Kouhila, 2001; Kouhila et al., 2002).

6

8

10

12

14

16

18

20

0

200

400

600

800

1000

Global radiation (W/m

2

)

Time (h)

Fig. 2. Variation of solar radiation vs. time during a typical summer
day in Marrakech.

0

100

200

300

400

500

0

1

2

3

4

5

6

Exp. 1

Exp. 2

Exp. 3

Exp. 4

Exp. 5

Exp. 6

Exp. 7

Exp. 8

Exp. 9

Moisture content (kg water/kg dry matter)

Drying time (min)

Fig. 3. Variation of moisture content as a function of drying time.

0.0

0.2

0.4

0.6

0.8

1.0

0

1

2

3

4

5

6

7

Exp. 1

Exp. 2

Exp. 3

Exp. 4

Exp. 5

Exp. 6

Exp. 7

Exp. 8

Exp. 9

Drying rate (kg water/(kg dry matter.min))

Moisture ratio

Fig. 4. Variation of drying rate as a function of moisture ratio.

0.0

0.2

0.4

0.6

0.8

1.0

0

2

4

6

8

Exp. 7 (T=50˚C, Dv=0.0833 m

3

/s)

Exp. 8 (T=55˚C, Dv=0.0833 m

3

/s)

Exp. 9 (T=60˚C, Dv=0.0833 m

3

/s)

Drying rate (kg water/(kg dry matter.min))

Moisture ratio

Fig. 5. Variation of drying rate with temperature during drying of
prickly pear fruit.

0.0

0.2

0.4

0.6

0.8

1.0

0

2

4

6

Exp. 1 (T=50˚C. Dv=0.0227 m

3

/s)

Exp. 4 (T=50˚C. Dv=0.0556 m

3

/s)

Exp. 7 (T=50˚C. Dv=0.0833 m

3

/s)

Drying rate (kg water/ kg dry matter. min)

Moisture ratio

Fig. 6. Variation of drying rate with air flow rate during drying of
prickly pear fruit.

176

S. Lahsasni et al. / Journal of Food Engineering 61 (2004) 173–179

3.3. Determination of the characteristic drying curve

Models have been developed by which the analysis of

drying process of several products and air conditions
may be carried out based on only few laboratory drying
experiments. For example, using Van MeelÕs concept
(1958) of the characteristic drying curve, it is possible to
present the drying rate curves of a given product, ob-
tained under different air conditions by a single nor-
malized drying rate curve. This curve can be used to
generalize data for drying kinetics of prickly pear fruit in
a solar dryer with an auxiliary heating system.

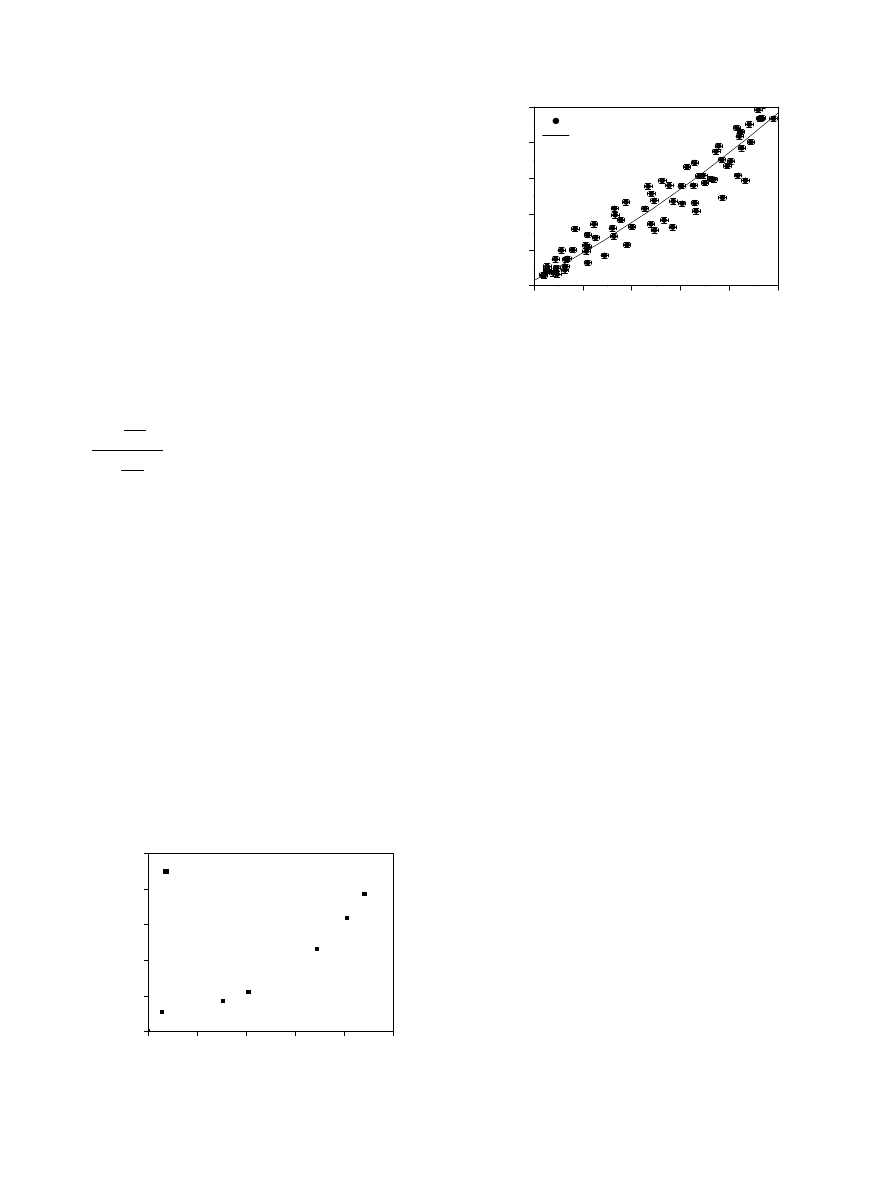
Kechaou (2000) and Kouhila (2001) used simply the

initial moisture content (M

0

) and the equilibrium mois-

ture content (M

e

) derived from desorption data (Fig. 7)

to obtain moisture ratio and initial drying rate
ðdM=dtÞ

0

to normalize the drying rate as follows:

f

¼

dM

dt

dM

dt

0

ð3Þ

where f is the dimensionless drying rate.

More details about the experiments and the instru-

ments used for determined M

e

may be found elsewhere

(Lahsasni, Kouhila, Mahrouz, & Kechaou, 2002;
Lahsasni, Kouhila, Mahrouz, & Fliyou, 2003).

The Van Meel transformation is applied for deter-

mining the characteristic drying curve of prickly pear
fruit. Experimental drying data are plotted in Fig. 8 to
represent f

¼ f ðMRÞ. This figure shows that all drying

curves obtained with the moisture ratio and dimen-
sionless drying rate, for the different tested conditions,
fall into a tight band, indicating that the effect of vari-
ation in different conditions is small over the range
tested.

The correlation coefficient (r) was one of the primary

criteria for selecting the best equation to define the
prickly pear fruit characteristic drying curve. In addition

to r, the statistical parameter standard error (S

r

) was

used to determine the goodness of fitting.

Marquardt-Levenberg non-linear optimization meth-

od, using the computer program ‘‘Curve Expert 3.1’’ was
used to find the best equation for the prickly pear fruit
characteristic drying curve:

f

¼ 0:03112 þ 0:7159 MR þ 0:2226 MR

2

ð4Þ

The criterion used to evaluate goodness of fit was the
standard error (S

r

¼ 0:076) and the correlation coeffi-

cient (r

¼ 0:969).

3.4. Modelling of the drying curves

Table 3 presents the drying constants and the values

of r and v-square of the eight models (see Table 1).
Generally r and v-square values were changed between
0.9963 and 0.9999 and 6.6800

10

4

and 1.0159

10

5

.

From Table 3, the two-term model gave the best results
in fitting the experimental data resulting from the con-
vective solar drying of prickly pear fruit with an r of
0.9999 and v

2

of 1.0159

10

5

. Consequently, it can be

said that the two-term model could sufficiently define the
convective solar drying of prickly pear fruit.

The coefficients of the accepted model (Eq. (5)) for

the convective solar drying of prickly pear fruit were
determined by Marquardt-Levenberg non-linear opti-
mization method. The degree 2 polynomial function
have shown the highest values of r and the lowest values
of S

r

. These coefficients are expressed as follows:

MR

¼ a expðk

0

t

Þ þ b expðk

1

t

Þ

ð5Þ

where

a

¼ 2:9205 þ 0:1117T 0:0011T

2

ð6Þ

k

0

¼ 1:1619 0:0439T þ 0:0004T

2

ð7Þ

b

¼ 2:3099 0:0547T þ 0:0005T

2

ð8Þ

k

1

¼ 0:0764 þ 0:0027T 2:1658 10

5

T

2

ð9Þ

0.0

0.2

0.4

0.6

0.8

1.0

0

20

40

60

80

100

Equilibrium moisture content

(kg water/kg dry matter)

Equilibrium relative humidity

Experimental data at T=50˚C

Fig. 7. Desorption isotherm of prickly pear fruit.

0.0

0.2

0.4

0.6

0.8

1.0

0.0

0.2

0.4

0.6

0.8

1.0

Exp. 1-9
CDC

Dimensionless drying rate

Moisture ratio

Fig. 8. Characteristic drying curve of prickly pear fruit.

S. Lahsasni et al. / Journal of Food Engineering 61 (2004) 173–179

177

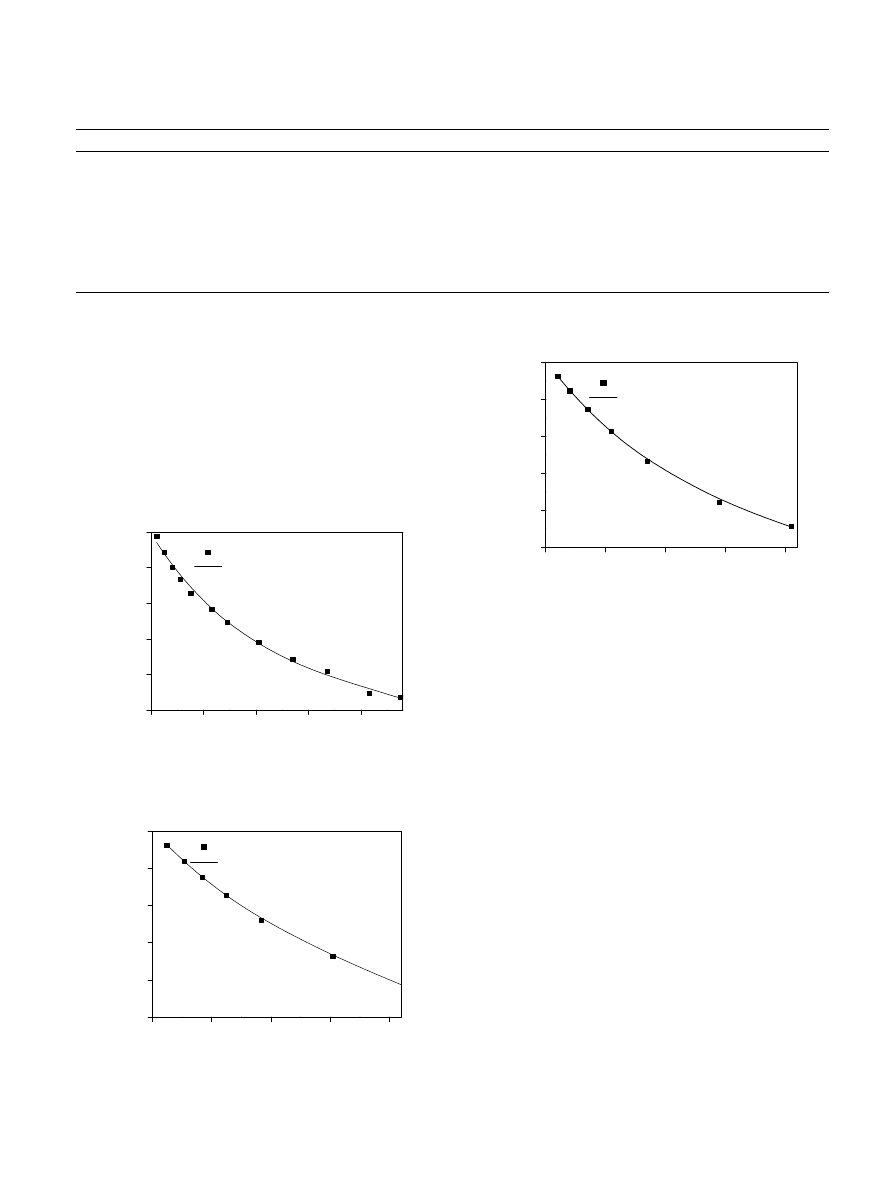
The four expressions (Eqs. (6)–(9)) predicted well the
moisture ratio (MR) at three drying temperatures 50, 55,
and 60

°C for the prickly pear fruit. The relationship

between coefficients of two-term model and drying air
temperatures was very significant, with an r of 1 and S

r

of 0 so that the moisture content of prickly pear fruit at
any time during the drying process could be estimated.
This result can be noted consequently from Figs. 9–11,

which compare experimental data with predicted values.
As these expressions are purely empirical, they would
hold good only for similar drying conditions of drying
materials (weight 1

0.1 g, diameter 0.5 0.01 cm,

length 2

0.03 cm), dryer capacity (3 kg/m

2

of prickly

pear fruit by tray), drying air temperatures (50–60

°C),

and drying air flow rates (0.0227–0.0833 m

3

/s) consi-

dered in this study.

4. Conclusions

From the drying kinetics study of prickly pear fruit, it

is observed that only the falling rate period exists. Also,
drying air temperature is the main factor influencing the
drying kinetics. The drying rate increases with a higher
drying air temperature and higher drying air flow rate.
The characteristic drying curve is obtained and the ex-
pression of the drying rate equation is determined.

According to these results, the two-term drying model

could adequately describe the thin layer drying behavior
of prickly pear fruit with an r of 0.9999 and v

2

of

1.0159

10

5

. When the effect of the drying air tem-

perature of the two-term model was examined, the re-
sulting model gave an r of 1 and S

r

of 0.

0

100

200

300

400

0.0

0.2

0.4

0.6

0.8

1.0

Exp. 1 (T=50

˚

C, Dv=0.0227 m

3

/s)

Two term model

Moisture ratio

Drying time (min)

Fig. 9. Experimental data of moisture ratio versus drying time fitted
with two-term model.

0

50

100

150

200

0.0

0.2

0.4

0.6

0.8

1.0

Exp. 2 (T=55

˚

C, Dv=0.0227 m

3

/s)

Two term model

Moisture ratio

Drying time (min)

Fig. 10. Experimental data of moisture ratio versus drying time fitted
with two-term model.

0

50

100

150

200

0.0

0.2

0.4

0.6

0.8

1.0

Exp. 3 (T=60

˚

C, Dv=0.0227 m

3

/s)

Two term model

Moisture ratio

Drying time (min)

Fig. 11. Experimental data of moisture ratio versus drying time fitted
with two-term model.

Table 3
Modelling of moisture ratio according to drying time for prickly pear fruit

Model

Coefficients

r

v

2

Newton

k

¼ 0:0070

0.9963

5.5666

10

4

Page

k

¼ 0:0043; n ¼ 1:1147

0.9991

1.5061

10

4

Modified Page

k

¼ 0:0060; n ¼ 1:1838

0.9963

6.6800

10

4

Henderson and Pabis

a

¼ 1:0329; k ¼ 0:0070

0.9978

3.9400

10

4

Logarithmic

a

¼ 1:2346; k ¼ 0:0052; c ¼ 0:2337

0.9998

2.3945

10

5

Two-term

a

a

¼ 0:0013; k

0

¼ 0:0182;

b

¼ 1:0125; k

1

¼ 0:0069

0.9999

1.0159

10

5

Two-term exponential

a

¼ 1:6283; k ¼ 0:0097

0.9993

1.1457

10

4

Wang and Singh

a

¼ 0:0061; b ¼ 1:0463 10

5

0.9995

8.2798

10

5

a

This model gives the best results for prickly pear fruit in a convective solar dryer.

178

S. Lahsasni et al. / Journal of Food Engineering 61 (2004) 173–179

Acknowledgements

This study was partially financed by the CNRST

(Morocco) for a project PROTARS III (Ref. D12/34) on
Solar Drying and Quality of Medicinal and Aromatic
plants.

References

Al Hodali, R. (1997). Numerical simulation of an agricultural

foodstuffs drying unit using solar energy and adsorption process.
Ph.D. Thesis, Universit

ee Libre de Bruxelles, Belgium, 309 p.

Basunia, M. A., & Abe, T. (2001). Thin layer solar drying character-

istics of rough rice under natural convection. Journal of Food
Engineering, 47, 295–301.

Belghit, A., Kouhila, M., & Boutaleb, B. C. (2000). Experimental

study of drying kinetics by forced convection of aromatic plants.
Energy Conversion and Management, 44(12), 1303–1321.

Bellegha, S., Amami, E., Farhat, A., & Kechaou, N. (2002). Drying

kinetics and characteristic drying curve of Lightly Salted Sardine
(Sardinella Aurita). Drying Technology, 20(7), 1523–1534.

Feitosa-Teles, F. F. (1977). Nutrient analysis of prickly pear. Ph.D.

Thesis, University of Arizona, Tucson, 157 p.

Galati, E. M., Monforte, M. T., Tripodo, M. M., dÕAquino, A., &

Mondello, M. R. (2001). Antiulcer activity of Opuntia ficus indica
(L.) Mill. (Cactaceae): ultrastructural study. Journal of Ethnophar-
macology, 76(1), 1–9.

Gurrieri, S., Miceli, L., Lanza, M. L., Tomaselli, F., Bonomo, P. R., &

Rizzarelli, E. (2000). Chemical characterization of Sicilian prickly
pear (Opuntia ficus indica) and perspectives for the storage of its
juice. Journal of Agricultural and Food Chemistry, 48, 5424–5431.

Habibi, Y., Mahrouz, M., & Vignon, M. (2002). Isolation and

structure of

D

D

-xylans from pericarp seeds of Opuntia ficus-indica

prickly pear fruits. Carbohydrate Research, 337, 1593–1598.

Hassan, B. H., & Hobani, A. I. (2000). Thin layer drying of dates.

Journal of Food Process Engineering, 23, 177–189.

Idlimam, A., Kaoua, M., Alem, L., & Daguenent, M. (1994). Etude

exp

eerimentale du rayonnement solaire

a

a Marrakech. In Comptes

techniques de dimensionnement des syst

eemes

eenerg

eetiques solaires,

Actes du colloque international, Perpignan, France.

Jayas, D. S., Cenkowski, S., Pabis, S., & Muir, W. E. (1991). Review of

thin layer drying and wetting equations. Drying Technology, 9(3),
551–588.

Kechaou, N., Bagane, M., Maalej, M., & Kapseu, C. (1996). Approche

empirique de la cin

eetique du s

eechage des dattes. Sciences des

Aliments, 16, 593–606.

Kechaou, N. (2000).

E

Etude th

eeorique et exp

eerimentale du processus de

s

eechage de produits agro-alimentaires, Th

eese de Doctorat dÕ

E

Etat,

Facult

ee des Sciences de Tunis, Tunisie, 192 p.

Kouhila, M. (2001). Etude exp

eerimentale et th

eeorique de cin

eetiques de

s

eechage convectif partiellement solaire des plantes m

eedicinales et

aromatiques (Menthe, Verveine, Sauge et Eucalyptus) de la r

eegion

de Marrakech, Th

eese de Doctorat dÕEtat, Universit

ee Cadi Ayyad

de Marrakech, Morocco, 170 p.

Kouhila, M., Kechaou, N., Otmani, M., Fliyou, M., & Lahsasni, S.

(2002). Experimental study of sorption isotherms and drying
kinetics of Moroccan Eucalyptus Globulus. Drying Technology,
20(10), 2027–2039.

Lahsasni, S., Kouhila, M., Mahrouz, M., & Kechaou, N. (2002).

Experimental study and modelling of adsorption and desorption
isotherms of prickly pear peel (Opuntia ficus indica). Journal of
Food Engineering, 55(3), 201–207.

Lahsasni, S., Kouhila, M., Mahrouz, M., & Fliyou, M. (2003).

Moisture adsorption–desorption isotherms of prickly pear cladode
(Opuntia ficus indica) at different temperatures. Energy Conversion
and Management, 44(6), 923–936.

Midilli, A., & Kucuk, H. (2003). Mathematical modeling of thin layer

drying of pistachio by using solar energy. Energy Conversion and
Management, 44(7), 1111–1122.

Mujumdar, A. S. (1987). Handbook of industrial drying. New York:

Marcel Dekker.

Park, E. H., Kahng, J. H., Lee, S. H., & Shin, K. H. (2001). An anti-

inflammatory principle from cactus. Fitoterapia, 72(3), 288–290.

Togrul, T. I., & Pehlivan, D. (2002). Mathematical modeling of solar

drying of apricot in thin layers. Journal of Food Engineering, 55,
209–216.

Togrul, T. I., & Pehlivan, D. (in press). Modeling of drying kinetics of

single apricot. Journal of Food Engineering.

Van Meel, D. A. (1958). Adiabatic convection batch drying with

recirculation of air. Chemical Engineering Science, 9, 36–44.

Yaldiz, O., & Ertekin, C. (2001). Thin layer solar drying of some

vegetables. Drying Technology, 19(3), 583–597.

Yaldiz, O., Ertekin, C., & Uzun, H. I. (2001). Mathematical modeling

of thin layer solar drying of sultana grapes. Energy, 26, 457–465.

S. Lahsasni et al. / Journal of Food Engineering 61 (2004) 173–179

179