
!
"#!$
%
&
'
(
)
*
)
¼
*
(
¼
+
,-
)
*
(
)
*
&
!
"#!$
.
#&
!
"#!$
/
'
(
0
)
¼
*
(
¼
)00*
"
&
!
"#!$
0

"
1
2
/
3&
!
"#!$
%
41
%
/
(
)
*
(
0
3&
41
+
+
'
!!"#
$
%
&
$'
(
)*
+
(
,*
'
-
-
.
)*
$
!!"#
/001
'
2201
"!(
3." #!#(.
4
%
&
$'
4
1
5
&
+
1
6
"
1
7

!
"#!$
1
+&
)
+
,8
92:
;
!:!
":2-
*
5
/
)00*
<
0=
)6
,-
!
"#!$
&
$
&
*
2
¼
(
<
>
¼
(
?@
)
*
3&
!
"#!$
'
55
6/
%781
55
921
55
68
:0;
; 1
55
55
;"!#<!=
";
55
,);
;
55
-);
);
"
!
"#!$
$
&
&
"
%
0
5
+
A
0
2
4
6
8
10
12
14
16
18
0
100
200
300
400
500
600
700
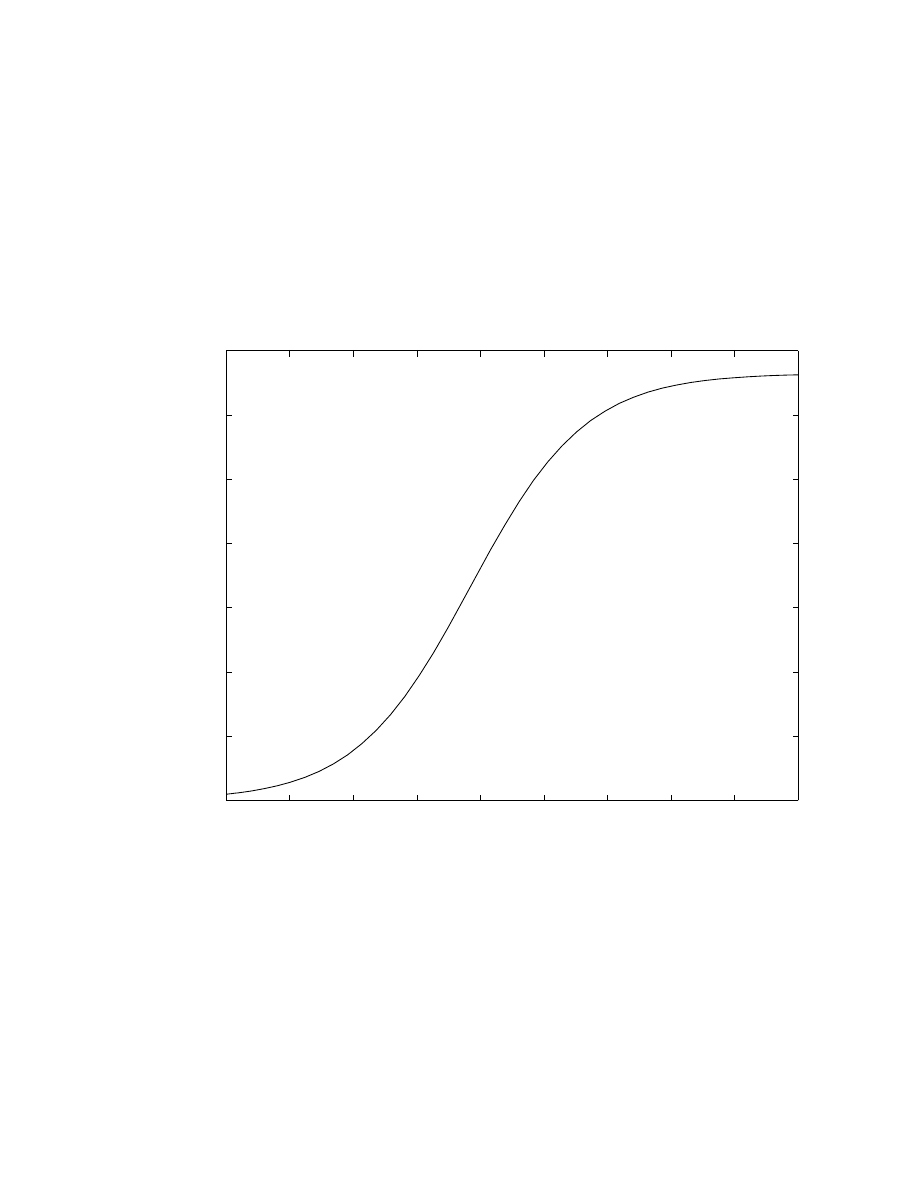
MATLAB Solution of Verhulst Model
t (hours)
N (biomass)
%
0'
2
/
!
"#!$
:0
;
'
(
<BB.
(
@@B
+
'
)<*
(
?@
C
+
'
(
)
*
)
¼
*
(
´¼µ
)77*
4
"
'
½
(
½
)
½
¾
*
¾
(
¾
)
½
¾
*
(
(
)
½
¾
*
'
½
)
¼
*
(
´¼µ
½
¾
)
¼
*
(
´¼µ
¾
(
)
¼
*
(
´¼µ
½
¾
!
)2
D
2
0<7
4D
2
0B*
"
1
"
'
(
)
*
(
)
*
)7A*
1
B

'
)
¼
*
(
¼
)
¼
*
(
¼
)7A*
&
4
$
!
"#!$
:0
E
&
$
"
!
"#!$
"
)7A*
!
"#!$
1
1
'
(
½
¾
½
(
¾
(
:4
)7A*
'
(
)
*
(
)
¾
*
½
)
½
*
¾
)
¼
*
(
¼
¼
)7C*
)77*
5
41
)
*
@

+
!!"#
,$
,
&
)
,
-
-$
-
&
,
-
(
)*
+
+%,
+>-
,
-
(
,*
)
.
)*
%
,$
>
-$
!!"#
?1
)0$>1
>1
%1
#!<!#
#!#
3#.
#.
".
3.(#
!(!=<
,+%1
-+>1
#
!
!
3.<"
3#.
@(#A
#@.
#(
+>%1
"!(
3." #!#(.
%4,&)4,4-1
>4-&4,4-1
5
4
<
7B
>
¼
(
<7
¼
(
<0=
)
>
*
"
!
"#!$
'
F

55
6/
>081
55
+6/>
/%781
55
6+8
:0;;+ 1
"
!
"#!$
>
"
+
"
1
½
¾
"
;
)
½
*
)
%
7*'
55
+*%
55
;
#;
55
,);;
55
-);,;
&
)
¾
*
)
%
A*'
55
+*>
55
;
#;
55
,);;
55
-);-;
!
)%
C*'
55
+
55
;
#;
55
,);;
55
-);
+;
%
)
*
)%
B*'
55
+*%+*>
55
;
;
55
,);,;
55
-);-;
=
0
0.5
1
1.5
2
2.5
0
5
10
15
20
25
30
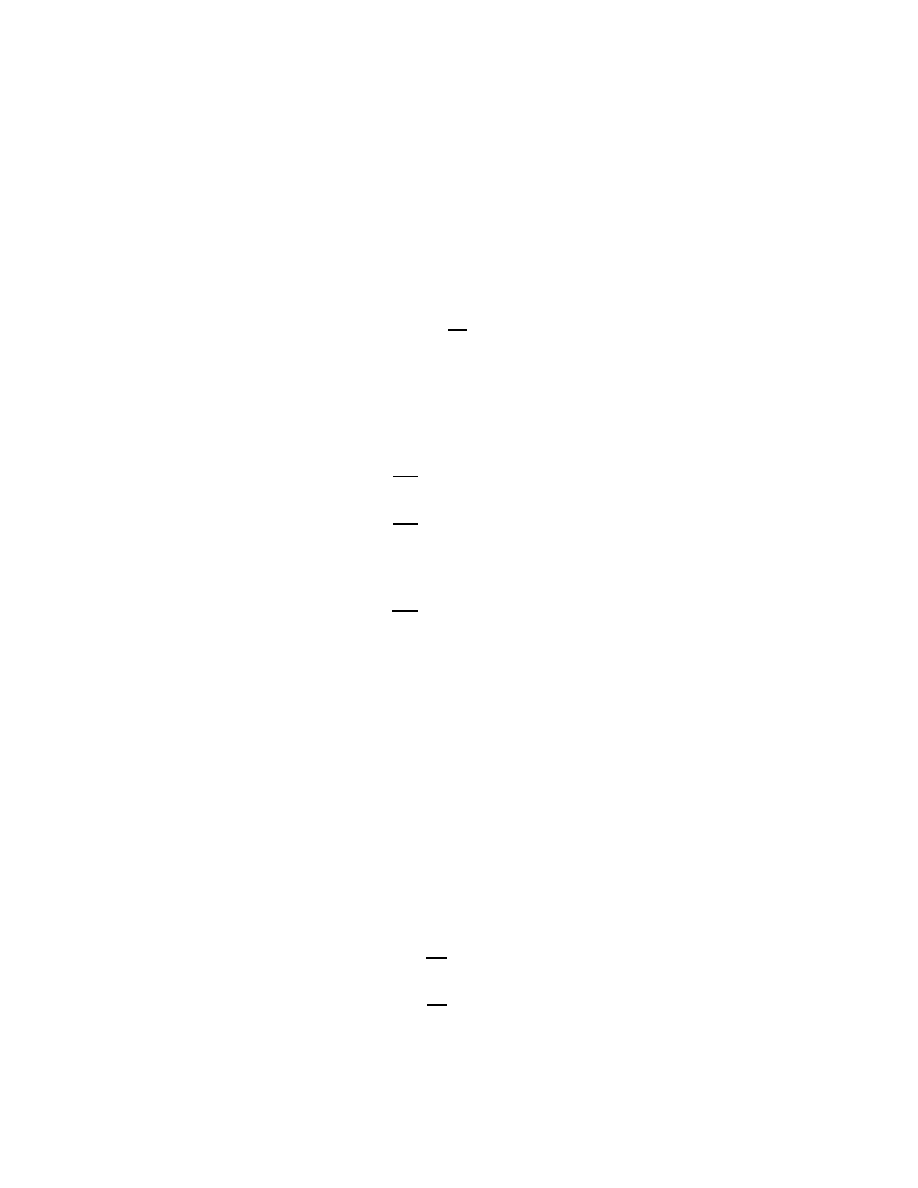
Population of First Species versus Time
t
x
%
7'
2
)7A*
;
'
(
A
(
B7
(
7
(
0
+
'
)<*
(
<7
)<*
(
<0=
?
0
0.5
1
1.5
2
2.5
0
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
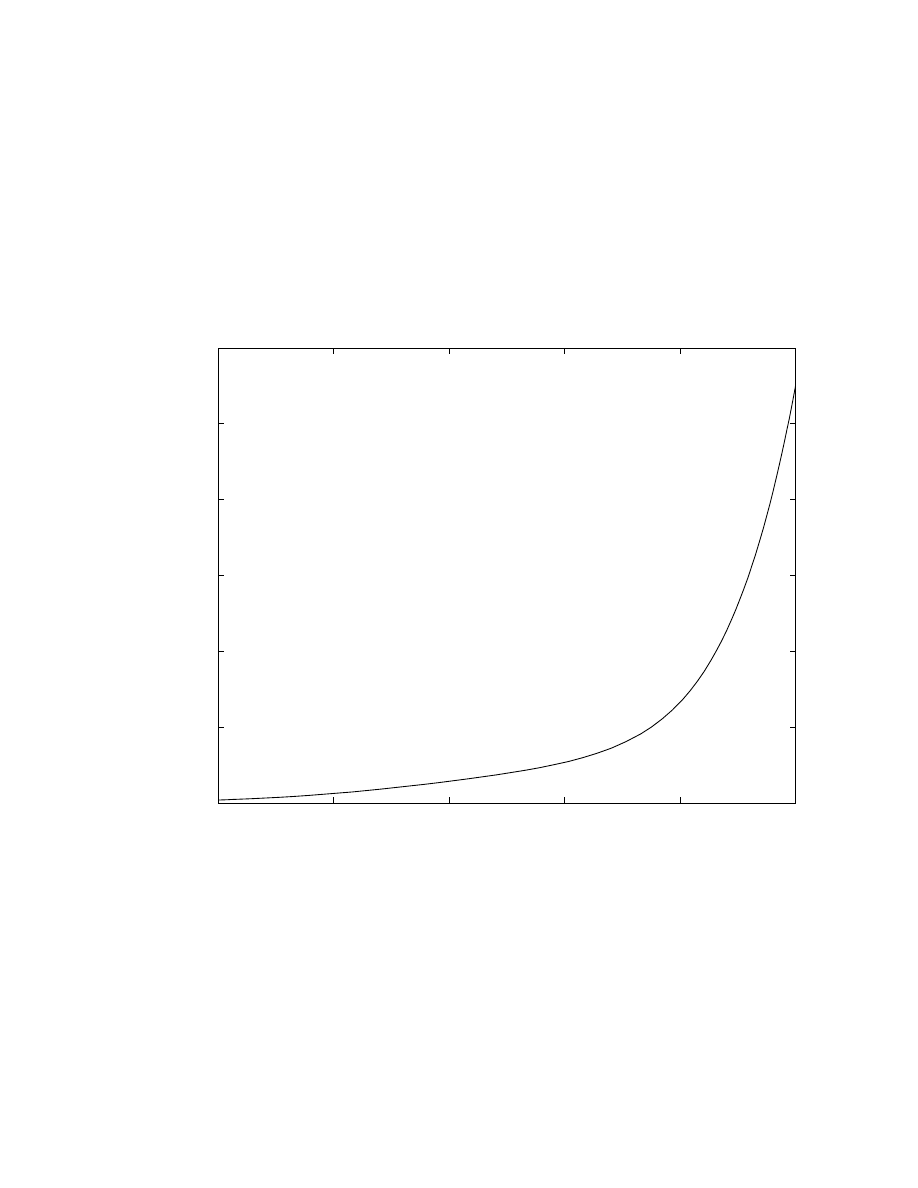
Population of Second Species versus Time
t
y
%
A'
2
)7A*
;
'
(
A
(
B7
(
7
(
0
+
'
)<*
(
<7
)<*
(
<0=
0<
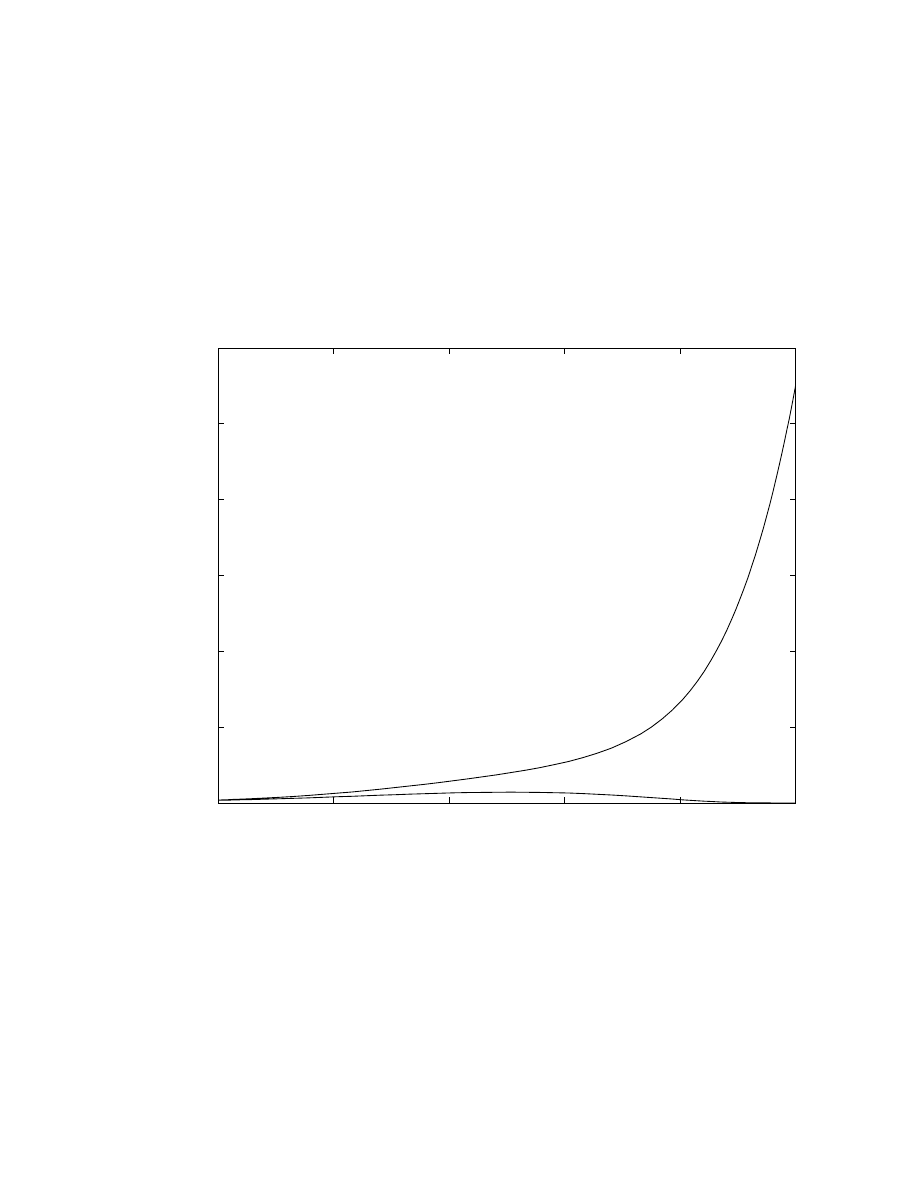
0
0.5
1
1.5
2
2.5
0
5
10
15
20
25
30
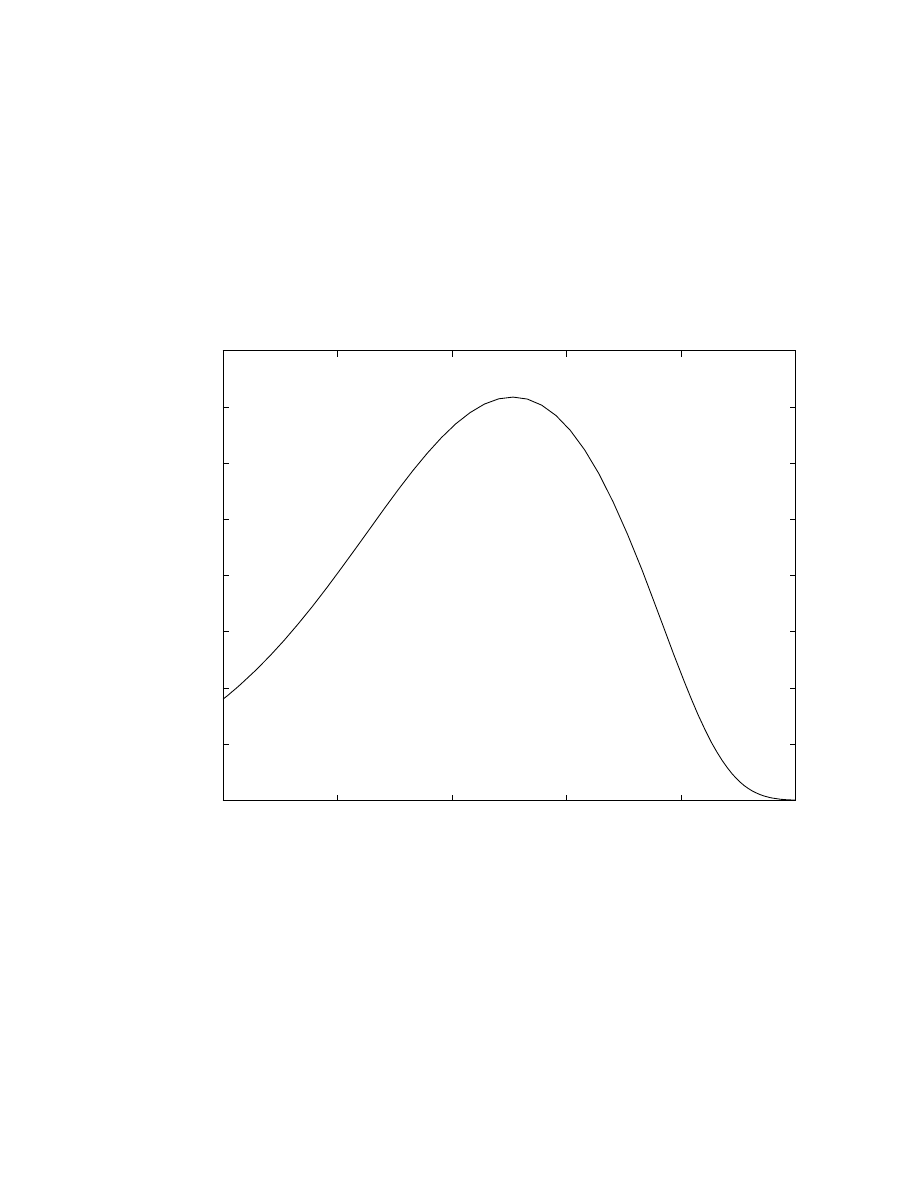
Populations versus Time
t
Population size
%
C'
2
)7A*
;
'
(
A
(
B7
(
7
(
0
+
'
)<*
(
<7
)<*
(
<0=
00
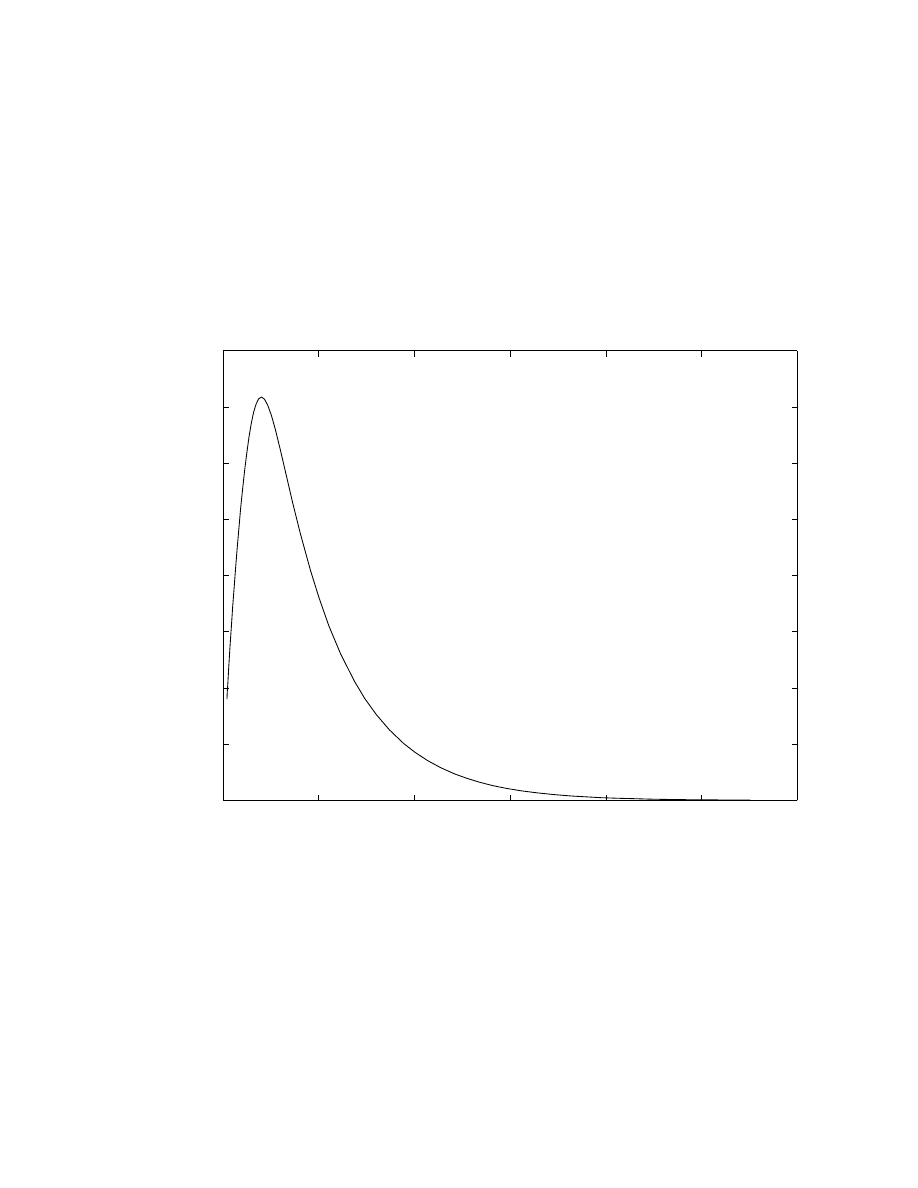
0
5
10
15
20
25
30
0
0.1
0.2
0.3
0.4
0.5
0.6
0.7
0.8
State Space Plot
x
y
%
B'
2
)7A*
;
'
(
A
(
B7
(
7
(
0
+
'
)<*
(
<7
)<*
(
<0=
07
Wyszukiwarka
Podobne podstrony:
The algorithm of solving differential equations in continuous model of tall buildings subjected to c
Mathematica package for anal and ctl of chaos in nonlin systems [jnl article] (1998) WW
Matlab Tutorial for Systems and Control Theory (MIT) (1999) WW
Generalized Power Series in a Non Archimedean Field [jnl article] (1991) WW
Minkowski Metrics [jnl article] (2002) WW
Wavelet Transforms that Map Integers to Integers [jnl article] (1996) WW
Elliptic Curves [jnl article] B Poonen WW
Connectionism Past, Present, and Future [jnl article] J Pollack WW
A Genetic Algorithm Tutorial [jnl article] D Whitley WW
The Birth of Model Theory [jnl article] C Badesa WW
Algorithm Collections for Digital Signal Processing Applications using Matlab E S Gopi
Algorithm Collections for Digital Signal Processing Applications using Matlab E S Gopi
Mathematical Basis for Physical Inference [jnl article] A Tarantola, K Mosegaard (2000) WW
Differential Equations for Reliability, Maintainability, and Availability
Pinchover Y , Rubinstein J An introduction to partial differential equations Extended solutions for
CALC1 L 11 12 Differenial Equations
G B Folland Lectures on Partial Differential Equations
więcej podobnych podstron