Wytrzymałość wykład 2 7WM

4. Pojęcie odkształcenia ciała sprężystego

y y P₁ P₂

D C D^' C^'

A B A^' B^'

P₃

0 x 0 x

D C P₄ D^' C^'

γ

dy dy+*(dy)

A dx B A^' dx+*(dx) B^'

Rys. 7 Wycinek ciała przed i po obciążeniu.

γ gamma, ε_x i ε_y noszą nazwę względnych wydłużeń

w kierunkach x i y i określone są wzorami:

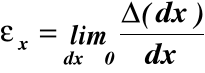
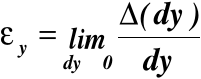
(b)

γ- nazywamy kątem odkształcenia postaciowego

i określamy z wzoru:

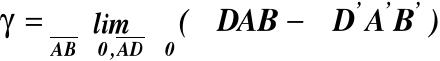
(c)

5. Zasada superpozycji 8WM

W przypadku działania na konstrukcję jednocześnie kilku

sił zewnętrznych można przy wyznaczaniu naprężeń i odkształceń stosować, w zakresie ważności prawa Hooke^'a,

zasadę superpozycji. Przykład podano na rysunku 8.

P₁ P₁

P₂ P₂

P₃ = + + P₃

Rys. 8 Ilustracja zasady superpozycji

6. Momenty gnące i siły tnące w belkach prostych

y y

M M T

x x

T

z z

M M T

dx T

Rys.9 Definicja znaków momentu gnącego M i siły tnącej T

Metoda postępowania przy określaniu sił wewnętrznych: 9WM

• określamy wartości reakcji więzów

• robimy myślowy przekrój w miejscu w którym określamy wartości sił wewnętrznych

• w miejscu myślowego przekroju przykładamy układ sił i momentów, który równoważy układ sił zewnętrznych działających na myślowo odciętą część konstrukcji

• wartości sił wewnętrznych określamy z warunków równowagi sił wewnętrznych i zewnętrznych działających na myślowo odciętą część konstrukcji

Uwaga: pod pojęciem sił wewnętrznych rozumiemy siły i

momenty działające w myślowym przekroju.

Analityczne określenie momentów gnących i sił tnących dla belki przedstawionej na rysunku 10

y

M₁ P₁ R_Ay M_i P_i R_B M_n P_n

α₁ R_Ax α_i α_B α_n

x

x₁ A B

a

x_i

b

x_n

l

Rys.10 Siły działające na belkę

Korzystając z równań równowagi, ciała znajdującego się w równowadze, możemy określić wartości reakcji podpór:

ΣP_x = P₁cosα₁ + ...+ P_icosα_i + ...+ P_ncosα_n + R_Ax +

+ R_Bcosα_B = 0 (a)

10WM

ΣP_y = P₁sinα₁ + ...+ P_isinα_i + ...+ P_nsinα_n + R_Ay +

+ R_Bsinα_B = 0 (b)

ΣM_A = (b-a)R_B sinα_B +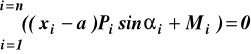
(c)

z równania (c) otrzymujemy:

(d)

Po określeniu reakcji R_B z równania (d), dwie pozostałe niewiadome R_Ax i R_Ay obliczamy z równań (a) i (b):

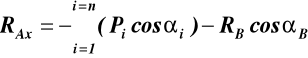
(e)

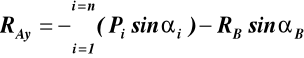
(f)

Oczywistym jest fakt że α_B 0. W przeciwnym przypadku

R_B ∞

Analiza sił wewnętrznych

y

M₁ P₁ R_Ay M_i P_i

α₁ R_Ax α_i N

0 x

x₁ A T M_x

a

x_i

x

Rys. 11 Siły wewnętrzne ( N, T, M_x ) w odciętej części beli

Zgodnie z rys.11otrzymujemy następujące 11WM

równania równowagi:

• w przedziale a₁ ≤ x ≤ a

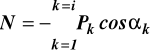
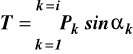
(g)

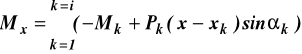
• w przedziale a≤x ≤ b

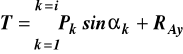
(h)

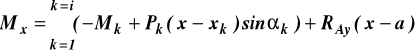
• w przedziale b ≤ x ≤ x_n

-R_B cosα_B

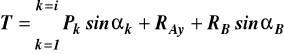
(i)

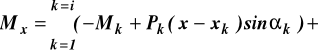
+R_Ay(x-a) +R_B(x-b)sinα_B

Zadanie 3 12WM

Sporządzić wykres momentów gnących i sił wewnętrznych dla belki przedstawionej na rysunku 12. Dane: a = 60cm, a₁ = 30cm, a₂ = 40cm, M = 60Nm, α = 30⁰.

y R_B

R_Ay R_Ax M α

C D x

a₁ a a a₂ Rys.12

Rozwiązanie

Obliczenie wartości reakcji podpór:

,

,

,

Obliczenie sił wewnętrznych i momentu gnącego

dla a₁ ≥ x ≥ 0 y M_x

N

C x

x 0

T Rys.12a

,

dla a₁ + a ≥ x ≥ a₁

y R_Ay M_x

R_Ax N

C x

a₁ x -a₁ 0

T Rys.12b

,

,

,

dla x = a₁ ,

dla x = a₁ + a

dla a₁ +2a ≥ x ≥ a₁ +a

y R_Ay M M_x

R_Ax N

C x

a₁ a 0

x

T Rys.12c

;

,

,
13WM

,

dla x = a₁ + a

dla x = a₁ +2a

dla a₁ + 2a + a₂ ≥ x ≥ a₁ + 2a

R_B

y R_Ay

M α M_x

R_Ax N

C x

a₁ a a 0

Rys.12d T

,

,

dla x = a₁ +2a

dla x = a₁ + 2a + a₂

Na rysunku 13 przedstawiono wykresy sił N,T oraz momentu gnącego M_x

C A E B D x

a₁ a a a₂

N = 0

- 86,6N

50N

T = 0

30Nm

M_x = 0

- 30Nm

Rys.13

90⁰-γ

---