Wytrzymałość Materiałów 3
Zadanie 4
Dla belki dwupodporowej z wysięgnikami podanej na
rys.14, obliczyć wartości reakcji RAx, RAy oraz RB, a także podaj
przebieg sił wewnętrznych. Obciążenie belki jest podane
w tabeli:
Tabela
i |
xi m |
Pi kN |
αi rad |
Mi kNm |
1 |
0 |
1 |
π/3 |
1 |
2 |
3 |
2 |
7π/6 |
-2 |
3 |
9 |
5 |
π/4 |
1 |
|
12 |
√2 |
π/4 |
1 |
Obliczenie wartości sił reakcji: dla αB = π /3
z równania (d) otrzymujemy
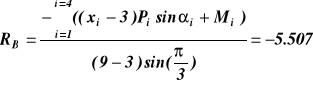
kN
równania (e) i (f) dają: RAx = −0.550 kN, RAy = 0.368 kN
RAy RB
P1 M2 αB P4
M1 α1 α2 RAx M3 P3
α3 4 α4
1 x
P2 2 3 M4
x2=a
x3=b
x4 = l
Rys. 14 Belka na dwóch podporach
Analiza sił wewnętrznych 15WM
- w przedziale 0≤ x≤ 3
P1
Mx
![]()
M1 α1 N
1 x
x T Rys.14a
∑Px = P1cosα1 + N = 0 N = - P1cosα1
∑Py = P1sinα1 - T = 0 T = P1sinα1
∑Mi = M1 - P1sinα1x + Mx = 0
- w przedziale 3≤ x≤ 9
RAy
P1 M1 M2
M1 α1 α2 RAx
N x
P2 A Mx
a T
x Rys.14b
∑Px = P1cosα1 + P2cosα2 + RAx + N = 0
∑Py = P1sinα1 + P2sinα2 + RAy - T = 0
∑Mi = M1 - M2 - P1sinα1x - P2sinα2 (x-a) + Mx = 0
- w przedziale x≥ 9
P4
Mx T 4 α4
N x
l - x M4 Rys.14c
∑Px = P4cosα4 - N = 0 ∑Py = P4sinα4 + T = 0
∑Mi = M4 + P4sinα4 (l-x) -Mx = 0
Wykresy sił wewnętrznych 16WM
T kN
0.866
0.230
0 x
1.78 -1.0
N kN
1.0
x
-0.50 5.0
Mx kNm 4.0
3.6
1.6
1.0
x
-1.0 Rys.14d
Naprężenia przy czystym zginaniu
P a A B a P
RA RB
Mg=Pa
T=P T = 0
T = P
Rys. 15 Realizacja czystego zginania
O 17WM
ρ - ro d* z
ρ ρ -z s
A B A B
s1 z z2
s x x
D C z1
D C
Rys. 13 Określenie odkształceń względnych
s = ρd*, s1 = (ρ - z)d*
![]()
(7)
Z prawa Hooke'a mamy: * = Eε = -Ez ρ (8)
x
z
M = My
Mz = 0
T = 0
z2 N = 0
z x
y
- z1
My y
Rys.17 y oś obojętna, * = 0
Rys.17a, obraz rzeczywistego stanu naprężeń Rys.17a
Określenie położenia osi obojętnej 18WM
1) z warunku N = 0
![]()

wniosek oś y (oś obojętna) jest osią przechodzącą przez środek ciężkości taka oś nazywa się
osią centralną takich osi jest nieskończona ilość
2) MZ = 0
![]()
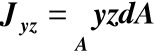
odśrodkowy moment bezwładności
Jeśli Jyz = 0 to osie y,z nazywamy osiami głównymi,
jeśli te osie przechodzą przez środek ciężkości przekroju to nazywamy je osiami głównymi centralnymi.
3) ![]()
My = Mg
![]()
(8a)
mnożymy i dzielimy (8a) przez z oraz przez (-1)
![]()
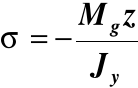
(9)
Przykład 5
Wyznaczyć średnicę d środkowej części osi wagonu (rys.18) aby ekstremalne naprężenia spełniały warunek *e ≤ 75 MPa.
1845 Z symetrii układu R1 = R2
P a P oznaczmy R1 = R2 = R
a-a
a z zmax
R1 1435 R2 Mg=Pb
P = 60kN b = (1845-1435) 2 = 205mm y
Rys.15 Do zadania 5
Rozwiązanie d
Aby określić z wzoru (9) wartość średnicy wału
należy znać wzór na Jy pola przekroju kołowego.
Wyprowadzenie wzoru.
z dF = dsdr = rd*dr
*
z=r sin*
x
dr Rys.16
![]()
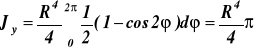
![]()
(10)
z wzoru (9) mamy : 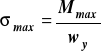
(10a)
gdzie 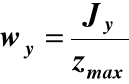
wskaźnik wytrzymałości
dla przekroju kołowego pełnego ![]()
Z wzoru (10a) i warunku że *max = *e = 75MPa mamy:
![]()
Linia ugięcia belki 20WM
Z wzoru (8a) mamy 1 ρ = Mg(x) EJy
w(x) ρ P
ϑ
A x
x wx
l
Rys.16 Określenie ugięć belki (w przekroju A belka
zamurowana)
Z geometrii różniczkowej wiemy, że krzywizna 1 ρ linii
w(x) wyraża się wzorem
1 ρ = w'' (1 + w'2)3/2 dla małych w' 1 ρ = w'' i wtedy:
![]()
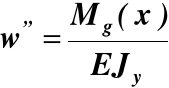
(11)
Przykład 6
Wyznaczyć linię ugięcia pryzmatycznej belki (rys.16) o przekroju kołowym. Określić ekstremalny kąt ugięcia ϑe i ekstremalne ugięcie
we = f, tzw. strzałkę ugięcia.
Przyjąć, że P = 10kN, l =1m, E = 2*105 MPa, a dopuszczalne naprężenie kr = kc = 100MPa
Rozwiązanie
Mg =P(l-x) dla 0* x * l
po scałkowaniu równania (11) otrzymujemy:
![]()
![]()
Całkując powyższe wyrażenie mamy:
![]()
Stałe całkowania wyznaczamy z warunków brzegowych:
wx=0 = 0; w'x=0 = 0 stąd C = 0; D = 0 w efekcie mamy:
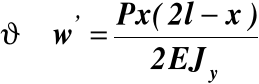
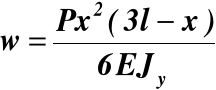
(a)
Dla x = l
![]()
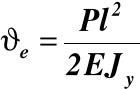
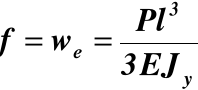
(12)
Po podstawieniu danych liczbowych do wzorów (12)
*e =0.290 f = 3.4mm
Jak widać (f /l) jest tu rzędu 1/300.
14WM
M z *
y
d*
r
R
19 WM
21WM
Wyszukiwarka
Podobne podstrony:
Laborki 2, Studia, Wytrzymałość materiałów II, Test z laborek wydymalka, lab
Laboratorium wytrzymałości materiałów
Wytrzymałość materiałów1 2 not
Wytrzymałość materiałów Ściąga 1
Mechanika i Wytrzymałość Materiałów zestaw2
A Siemieniec Wytrzymałość materiałów cz I (DZIAŁY PRZERABIANE NA PK WIITCH)
Mechanika i Wytrzymałość Materiałów W 1
test z wydymałki, Przodki IL PW Inżynieria Lądowa budownictwo Politechnika Warszawska, Semestr 4, Wy
POMIAR TWARDOŚCI SPOSOBEM BRINELLA, POLITECHNIKA POZNAŃSKA, LOGISTYKA, semestr I, mechanika i wytrzy
Labora~3, Rok I, semestr II, Rok II, Semestr I, Wytrzymałość materiałów I, laborki - materiały + spr
L4 - pytania, Studia, Wytrzymałość materiałów II, lab4 wm2 studek
OPIS UK ADU UK KO OWY, wytrzymałość materiałów
cw-9 p, NAUKA, Politechnika Bialostocka - budownictwo, Semestr III od Karola, Wytrzymałośc Materiałó
Spr. 1. Rozciąganie, Wytrzymałość materiałów
POLITECHNIKA LUBELSKA, Politechnika Lubelska, Studia, semestr 5, Sem V, Sprawozdania, MATERIAŁOZNAS
1 laborka -Układy liniowo sprężyste, Wytrzymałość materiałów(1)
A Siemieniec Wytrzymałość materiałów cz II
Wzor Naglowka, wytrzymałość materiałów laborki
więcej podobnych podstron