Prof. Sobczak[Author ID0: at Thu Nov 30 00:00:00 1899
]
Wykład: 21.09.2002[Author ID0: at Thu Nov 30 00:00:00 1899
]
Literatura:[Author ID0: at Thu Nov 30 00:00:00 1899
]
Maria Chromińska, Walentyna Ignatczyk, Statystyka,[Author ID0: at Thu Nov 30 00:00:00 1899
]
H. Kassyk-Rokicka, Statystyka nie jest trudna; Mierniki Statystyczne,[Author ID0: at Thu Nov 30 00:00:00 1899
]
Mieczysław Kędelski, Iwona Roeske-Słomka, Statystyka[Author ID1: at Wed Aug 20 16:25:00 2003
]
Pojęcie i przedmiot S[Author ID1: at Wed Aug 20 16:25:00 2003
]tatystyki[Author ID0: at Thu Nov 30 00:00:00 1899
]
Statystyka może być rozumiana dwojako, w sensie potocznym uważa się za nią niektóre zestawienia liczbowe charakteryzujące np. umieralność niemowląt, wydobycie kopalin, wypadki przy pracy, spożycie dobra na jednostkę itp. Tak pojmowana statystyka nie jest dyscypliną naukową. Przez statystykę bowiem rozumiemy naukę, która zajmuje się badaniem prawidłowości zachodzących w procesach masowych.
Procesy masowe rządzą się prawami wielkich liczb (na 1000 dzieci rodzi się 517 chłopców i 483 dziewczynki), nie mówimy o nich gdy mamy do czynienia z jednym i tylko jednym przypadkiem (w jednej rodzinie urodziły się cztery dziewczynki)
Prawidłowości statystyczne są wynikiem występowania tzw. przyczyn głównych, prawidłowości są odkształcane (zakłócane) poprzez występowanie przyczyn ubocznych, im większa jest liczba obserwacji tym mniejsze jest oddziaływanie przyczyn ubocznych, a gdy liczba obserwacji dąży do nieskończoności oddziaływanie przyczyn ubocznych wzajemnie się znosi (spada do zera).
Zadania statystyczne
Podstawowym zadaniem statystycznym jest dostarczanie wiarygodnych informacji w celu zarządzania wszystkimi dziedzinami życia.
Podział statystyki (wg różnych kryteriów)
Statystyka matematyczna
Zajmuje się weryfikacją hipotez statystycznych oraz estymacją (szacowaniem) punktową lub przedziałową parametrów.
Statystyka opisowa
Główne działy statystyki opisowej
Kompleksowa analiza struktury zbiorowości,
Analiza korelacji i regresji,
Analiza dynamiki zjawisk (badanie szeregów czasowych, tendencji rozwojowej).
Podstawowe pojęcia
ZNAKI UMOWNE |
|
- |
Zjawisko nie występuje |
0,0 |
Zjawisko występuje w ilościach mniejszych niż da się to wyrazić w przyjętej jednostce miary |
. |
Brak danych lub brak danych wiarygodnych |
X |
Wypełnienie rubryki nie dotyczy, nie ma sensy |
Zbiorowość statystyczna - nie definiujemy - stanowi odpowiednik zbioru w matematyce, podajemy jednak przykłady zbiorowości np. zbiorowość osób, przedmiotów, zjawisk (przyrodniczych, ekonomicznych, społecznych). Zbiorowość zwana inaczej populacją albo zbiorowością generalną składa się z jednostek statystycznych (odpowiedniki elementów w zbiorze). Każda jednostka zbiorowości ma pewne właściwości. Te właściwości nazywamy cechami statystycznymi - to one podlegają badaniu.
Ogólny podział cech statystycznych
Stałe,
Wspólne wszystkim jednostkom statystycznym i z uwagi na to nie są przedmiotem badania a tylko odpowiedniego grupowania zbiorowości na pewne podzbiorowości.
Zmienne,
Rozróżniają jednostki pomiędzy sobą. Dzielą się na:
cechy mierzalne inaczej ilościowe (takie, których wartość da się przedstawić za pomocą liczby).Wśród mierzalnych wyróżnia się cechy:
ciągłe, ich wartości przedstawia się w postaci dowolnej liczby rzeczywistej np. wiek, waga, wydajność pracy, cena, kurs, temperatura,
skokowe, ich wartość da się przedstawić wyłącznie za pomocą zera lub liczb naturalnych (np. liczba dzieci w rodzinie 0, 1, 2, 3...)
quasi ciągłe
cechy niemierzalne[Author ID3: at Tue Apr 15 18:21:00 2003 ]
jakościowe[Author ID3: at Tue Apr 15 18:21:00 2003 ] (niemierzalne[Author ID3: at Tue Apr 15 18:21:00 2003 ]jakościowe[Author ID3: at Tue Apr 15 18:21:00 2003 ]) to takie cechy, których wartości nie da się zmierzyć a jedynie opisać w sposób słowny (płeć, wykształcenie, kolor włosów).
Kompleksowa analiza struktury zbiorowości
W skład kompleksowej analizy struktury zbiorowości wchodzą:
Średnia (klasyczna i pozycyjna),
Miary rozproszenia (dyspersji),
Miary skośności (asymetrii),
Miary spłaszczenia (koncentracji).
Ad.1 Średnie klasyczne
Średnia arytmetyczna (średnia x -
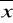
)
dla szeregów prostych gdy dane nie są uporządkowane wyraża się wzorem
![]()
xi - wartość badanej cechy i-tej jednostki statystycznej,
N - liczba badanych jednostek statystycznych.
PRZYKŁAD :
Średni wzrost mężczyzn (10 elementów)
x1 = 168 x2 = 178 x3 = 171 x4 = 185 x5 =180
x6 = 171 x7 = 179 x8 =183 x9 =180 x10 =175
![]()
= 177 cm
dla szeregu rozdzielczego - jeżeli w wyniku odpowiedniego grupowania danych nieuporządkowanych w szereg rozdzielczy w postaci:
średnia geometryczna
średnia harmoniczna
Dominanta (wartość typowa , modalna, dominująca) - to taka wartość badanej cechy, której odpowiada największa liczebność
uporządkować szereg rosnąco (czasami malejąco),
podsumować jednostki, które maja tę samą wartość.
Dominantą będzie wartość występująca najczęściej.
Mediana (wartość środkowa)
Kwartyl 1 - Q1 to taka wartość badanej cechy, która dzieli populację na dwie części w sposób następujący - 25% jednostek statystycznych jeszcze tej wartości nie osiągnęło a pozostałe 75% tę wartość przekroczyło.
Kwartyl 2 - Q2 - Me (mediana) to taka wartość badanej cechy, która dzieli populację na połowy, inaczej mówiąc jest to wartość środkowa. W medianie połowa populacji jeszcze nie osiągnęła wartości badanej cechy a druga połowa już tę wartość przekroczyła.
Kwartyl 3 - Q3 to taka wartość badanej cechy, której 75% liczebności jeszcze nie osiągnęło tej wielkości a 25% ją przekroczyło.
uporządkować dane w sposób rosnący,
zauważyć (przeliczyć) czy liczba obserwacji jest parzysta czy nieparzysta
Szereg prosty:[Author ID0: at Thu Nov 30 00:00:00 1899 ]cecha ciągła, (wiek posłów) 38, 45, 23, 58, 52, 41, 49, 63, 59, 62 n=10[Author ID0: at Thu Nov 30 00:00:00 1899 ]cecha skokowa (ilość mandatów karnych urzędników w ciągu roku)[Author ID0: at Thu Nov 30 00:00:00 1899 ]Szereg rozdzielczy jednopunktowy - cecha skokowa (ilu urzędników otrzymało określoną ilość mandatów w ciągu roku).[Author ID2: at Fri Feb 14 13:47:00 2003 ]Szereg rozdzielczy - cecha ciągła (staż pracy nauczycieli szkół średnich w miejscowości A)[Author ID2: at Fri Feb 14 13:47:00 2003 ]Najprostszą miarą dyspersji jest rozstęp oznaczający różnicę pomiędzy wartością minimalną a maksymalną badanej cechy
Odchylenie przeciętne
dla szeregu prostego ma postać:
dla szeregu rozdzielczego ma postać:
Odchylenie standardowe:
dla szeregu prostego ma postać:
dla szeregu rozdzielczego ma postać:
Odchylenie ćwiartkowe (stosujemy dla mediany)
W przedziale (-3σ,3σ) mieszczą się (zawierają się) prawie wszystkie badane jednostki statystyczne.
W przedziale (-2σ,2σ) znajduje się ponad 95% wszystkich badanych jednostek ststystycznych.
W przedziale (-σ,σ) znajduje się około 68% wszystkich badanych jednostek ststystycznych.
Średnia arytmetyczna:
obliczana na podstawie wszystkich danych szeregu,
na jej wartość duży wpływ mają wielkości skrajne,
nadaje się do przekształceń algebraicznych,
suma odchyleń od średniej równa się zeru (0).
Średnia harmoniczna:
obliczana na podstawie wszystkich danych szeregu,
nadaje się do przekształceń algebraicznych,
stosujemy głównie wtedy, gdy mamy do czynienia z odwrotnościami jakiejś wielkości.
Średnia geometryczna:
obliczana na podstawie wszystkich danych szeregu,
wartości skrajne mają na nią mniejszy wpływ niż na średnią arytmetyczną,
jest mniejsza od średniej arytmetycznej,
istnieje dla xi > 0,
jest pomocna przy obliczaniu średnich wskaźników.
Dominanta:
jest wartością najbardziej typową dla szeregu,
łatwo ją wyznaczyć z uporządkowanego szeregu prostego,
dla szeregu rozdzielczego można ją tylko oszacować,
przy małej liczebności może nie być dominanty, a przy dużej może wystąpić więcej niż jedna dominanta (przy dwóch dominantach szereg nazywamy bimodalnym).
Mediana:
nie mają na nią wpływu wartości skrajne,
stosuje się głównie dla szeregów skrajnie asymetrycznych.
Względna jednorodność zbiorowości ze względu na badaną cechę (analiza Vx ; najlepiej, gdy Vx ≤ 35%, gdy współczynnik jest nie większy niż 75%).
Gdy jest niewielka asymetria rozkładu (przy dużej asymetrii x arytmetyczna nie ma wartości poznawczej).
Nie stosujemy średniej gdy szereg nie jest domknięty dołem i górą.
Nie stosujemy mediany gdy szereg ma przedziały o różnej rozpiętości.
Dla rozkładów o dużej asymetrii stosujemy wyłącznie przeciętne pozycyjne ( dominanta i kwartyle [Me]}
Sporządzić wykres (histogram) - przez co uzyskamy odpowiedź na asymetrię i wzajemne położenie średniej, dominanty i mediany,
Obliczyć średnią arytmetyczną,
Sprawdzić jej „dobroć” za pomocą odchylenia standardowego i współczynnika zmienności,
Obliczyć rozstęp,
Wyznaczyć dominantę i medianę,
Zbadać asymetrię rozkładu, skośność,
Zbadać spłaszczenie rozkładu (koncentrację wokół średniej),
Wyznaczyć obszar typowy.
Średnia arytmetyczna i jej interpretacje (przekłamuje).
Mediana (interpretacja).
Miary dyspersji, odchylenie standardowe.
Czemu służy współczynnik zmienności, co wiemy dzięki niemu.
Interpretacja przedziału typowego i z czego to wynika.
Czemu służy histogram, jak w przybliżeniu wyznaczyć dominantę.
Odróżnić zbiorowość statystyczną, jednostkę statystyczną i badaną cechę (umieć opisać cechę: niemierzalna, mierzalna - ciągła i skokowa.
rx,y = ry,x korelacja pomiędzy (x) a (y) jest taka sama jak pomiędzy (y) a (x).
-1 ≤ rx,y ≤ 1
Zbadać czy pomiędzy wymienionymi cechami zachodzi liniowy związek korelacyjny.
Oszacować wielkość mieszkania (liczbę pokoi) dla rodziny liczącej 4 osoby.
Współczynniki natężenia - logiczne relacje dwóch różnych (niejednoimiennych wielkości) pozostających w pewnym związku logicznym. Współczynnikami natężenia są np. umieralność niemowląt w przeliczeniu na 1000 urodzeń żywych, zużycie paliwa na 100km (lub na 1 godz. lotu), plony z hektara, liczbę ludności na 100 km[Author ID2: at Fri Feb 14 13:51:00 2003 ]2[Author ID2: at Fri Feb 14 13:51:00 2003 ]. Współczynniki natężenia przelicza się zwykle: na jednostkę, na 100, 1.000, 10.000.[Author ID0: at Thu Nov 30 00:00:00 1899 ]Wskaźniki struktury - obrazują iloraz pewnej części do całości (części zbiorowości statystycznej do całości populacji). Można je także wyrażać w procentach lub promilach. Wskaźnik struktury możemy zapisać następująco:[Author ID2: at Fri Feb 14 13:51:00 2003 ]Wskaźniki dynamiki zjawisk (indeksy) - iloraz wartości zmiennej w okresie badanym i jej wartości z okresu stanowiącego podstawę porównań. Wyróżnia się indeksy:[Author ID0: at Thu Nov 30 00:00:00 1899 ]jednopodstawowe - o stałej podstawie, określają zmiany jakie nastąpiły w poziomie zjawiska, kolejnych okresach (momentach) w porównaniu z okresem (momentem) przyjętym za podstawowy (bazowy). Możemy je określić relacją:[Author ID2: at Fri Feb 14 13:51:00 2003 ]łańcuchowe - o zmiennej podstawie wyraża następująca relacja:[Author ID2: at Fri Feb 14 13:51:00 2003 ]Jeżeli[Author ID2: at Fri Feb 14 13:51:00 2003 ](i[Author ID2: at Fri Feb 14 13:51:00 2003 ]t/0[Author ID2: at Fri Feb 14 13:51:00 2003 ])[Author ID2: at Fri Feb 14 13:51:00 2003 ]lub[Author ID2: at Fri Feb 14 13:51:00 2003 ](i[Author ID2: at Fri Feb 14 13:51:00 2003 ]t/t-1[Author ID2: at Fri Feb 14 13:51:00 2003 ])[Author ID2: at Fri Feb 14 13:51:00 2003 ]są równe jeden (1) lub 100% to zjawisko pozostało na poziomie niezmienionym.[Author ID0: at Thu Nov 30 00:00:00 1899 ]Jeżeli[Author ID2: at Fri Feb 14 13:51:00 2003 ](i[Author ID2: at Fri Feb 14 13:51:00 2003 ]t/0[Author ID2: at Fri Feb 14 13:51:00 2003 ])[Author ID2: at Fri Feb 14 13:51:00 2003 ]lub[Author ID2: at Fri Feb 14 13:51:00 2003 ](i[Author ID2: at Fri Feb 14 13:51:00 2003 ]t/t-1[Author ID2: at Fri Feb 14 13:51:00 2003 ])[Author ID2: at Fri Feb 14 13:51:00 2003 ]są mniejsze niż jeden (1) lub 100% to mówimy, że poziom zjawiska spada.[Author ID0: at Thu Nov 30 00:00:00 1899 ]Jeżeli[Author ID2: at Fri Feb 14 13:51:00 2003 ](i[Author ID2: at Fri Feb 14 13:51:00 2003 ]t/0[Author ID2: at Fri Feb 14 13:51:00 2003 ])[Author ID2: at Fri Feb 14 13:51:00 2003 ]lub[Author ID2: at Fri Feb 14 13:51:00 2003 ](i[Author ID2: at Fri Feb 14 13:51:00 2003 ]t/t-1[Author ID2: at Fri Feb 14 13:51:00 2003 ])[Author ID2: at Fri Feb 14 13:51:00 2003 ]są większe niż jeden (1) lub 100% to oznacza wzrost poziomu zjawiska.[Author ID0: at Thu Nov 30 00:00:00 1899 ]Indeks fizycznych rozmiarów (ilość).[Author ID0: at Thu Nov 30 00:00:00 1899 ][Author ID0: at Thu Nov 30 00:00:00 1899 ]Metoda mechaniczna[Author ID5: at Wed Jan 29 11:20:00 2003 ]. [Author ID5: at Wed Jan 29 11:31:00 2003 ]Polega na zastosowaniu tzw. średnich ruchomych.[Author ID0: at Thu Nov 30 00:00:00 1899 ]
Metoda analityczna. Polega na opisie tendencji rozwojowej[Author ID5: at Wed Jan 29 11:21:00 2003 ] za pomocą funkcji matematycznej zwanej aproksymantą, dobranej tak aby spełniony był warunek [Author ID5: at Wed Jan 29 12:09:00 2003 ][Author ID5: at Wed Jan 29 12:10:00 2003 ][Author ID5: at Wed Jan 29 12:10:00 2003 ]
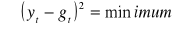
[Author ID5: at Wed Jan 29 12:10:00 2003 ][Author ID0: at Thu Nov 30 00:00:00 1899 ]Wyznaczyć na układzie współrzędnych wartości empiryczne[Author ID5: at Wed Jan 29 12:17:00 2003 ] zjawiska i na podstawie rozkładu punktów wybrać jedną z klas funkcji (kształt aproksymanty[Author ID5: at Wed Jan 29 12:18:00 2003 ]).[Author ID5: at Wed Jan 29 12:19:00 2003 ][Author ID0: at Thu Nov 30 00:00:00 1899 ]
Oszacować parametry aproksymanty[Author ID5: at Wed Jan 29 12:19:00 2003 ].[Author ID0: at Thu Nov 30 00:00:00 1899 ]
Oszacować „dobroć” aproksymanty.[Author ID0: at Thu Nov 30 00:00:00 1899 ]
oznacza średni poziom zjawiska w badanym okresie,[Author ID0: at Thu Nov 30 00:00:00 1899 ]
okresowe (roczne) przyrosty zjawiska (wzrost dla b>0, spadek dla b<0)[Author ID0: at Thu Nov 30 00:00:00 1899 ]
Określić zbiorowość, jednostkę statystyczną i badaną cechę.
Przeprowadzić kompleksową analizę cen ofertowych mieszkań.
|
ni |
x`i |
ni ⋅x`i |
700-800 |
11 |
750 |
8250 |
800-900 |
18 |
850 |
15300 |
900-1000 |
26 |
950 |
24700 |
1000-1800 |
36 |
1400 |
50400 |
1800-2400 |
32 |
2100 |
67200 |
2400-3000 |
16 |
2700 |
43200 |
suma |
N=139 |
|
209050 |
![]()
ni - liczebność i-tego przedziału klasowego (suma ni równa się N)
x`i - środek i-tego przedziału klasowego
stosujemy dla liczb względnych (procenty, promile np. roczne wykonanie planu).
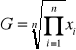
gdzie xi >0 (PI oznacza iloczyn)
jest odwrotnością średniej arytmetycznej - stosujemy gdy dane są podane jako odwrotność np. zużycie paliwa na jednostkę, wydajność na godzinę.
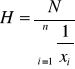
gdzie xi ≠0
Ad. 1 Średnie pozycyjne
Wynikają z pozycji w szeregu, wyznacza się na podstawie tzw. wzorów interpolacyjnych.
Sposób wyznaczania dominanty dla szeregu prostego
Sposób wyznaczania dominanty dla szeregu rozdzielczego
![]()
gdzie: xo - dolna granica przedziału w którym znajduje się dominanta,
co - rozpiętość przedziału dominanty,
nd - liczebność przedziału, w którym znajduje się dominanta,
nd-1 - liczebność przedziału poprzedzającego,
nd+1 - liczebność przedziału następnego po przedziale dominanty.
Dominantę z szeregu rozdzielczego można w przybliżeniu wyznaczyć także w sposób graficzny.
Sposób wyznaczania mediany dla szeregu prostego
Jeżeli szereg jest nieparzysty wartość mediany stanowi wartość cechy wyrazu środkowego
168, 178, 171, 185, 180, 171, 179, 183, 180, 175, 186
168, 171, 171, 175, 178, 179, 180, 180, 183, 185, 186
Me = 179
Jeżeli szereg jest parzysty są dwa wyrazy środkowe a medianę stanowi średnia arytmetyczna wartości badanej cechy wyznaczona z obu wyrazów środkowych
159, 168, 171, 171, 175, 178, 179, 180, 180, 183, 185, 186
Me = (178+179) ÷ 2 = 178,5 ≈ 179
Mediana dla szeregu rozdzielczego:
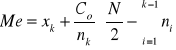
gdzie: xk = dolna granica przedziału, w którym znajduje się mediana (początek przedziału),
Co = rozpiętość przedziału, w którym znajduje się mediana (długość przedziału),
nk = liczebność przedziału, w którym znajduje się mediana (wielkość odpowiadająca przedziałowi),
k-1 = suma ni od początku do przedziału z medianą.
N/2 (a gdy liczba obserwacji jest nieparzysta (N+1)/2 - oznacza pozycję mediany w szeregu
Ćwiczenia 22-09-2002 dokończone 05.10.2002[Author ID0: at Thu Nov 30 00:00:00 1899
]
rozwiązanie:[Author ID0: at Thu Nov 30 00:00:00 1899
]
średnia = P/n = 490/10 = [Author ID2: at Fri Feb 14 13:47:00 2003
]49[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]
D (dominant[Author ID2: at Fri Feb 14 13:47:00 2003
]a) [Author ID2: at Fri Feb 14 13:47:00 2003
]nie występuje[Author ID2: at Fri Feb 14 13:47:00 2003
] ponieważ żadna z wartości nie występuje więcej razy niż inne[Author ID0: at Thu Nov 30 00:00:00 1899
]
Me (mediana) → uporządkowane - 23, 38, 41, 45, (49, 52), 58, 59, 62, 63,[Author ID0: at Thu Nov 30 00:00:00 1899
]
Me = (49+52)/2 = 50,5 [Author ID2: at Fri Feb 14 13:47:00 2003
]≈[Author ID2: at Fri Feb 14 13:47:00 2003
] [Author ID2: at Fri Feb 14 13:47:00 2003
]51[Author ID2: at Fri Feb 14 13:47:00 2003
] [Author ID0: at Thu Nov 30 00:00:00 1899
]
1, 3, 0, 0, [Author ID2: at Fri Feb 14 13:47:00 2003
]2, 4, 3, 2, 2, 0, 0, 1 n=12[Author ID0: at Thu Nov 30 00:00:00 1899
]
rozwiązanie:[Author ID0: at Thu Nov 30 00:00:00 1899
]
średnia = P/n = 18/12 = 1,5 [Author ID2: at Fri Feb 14 13:47:00 2003
]≈[Author ID2: at Fri Feb 14 13:47:00 2003
] [Author ID2: at Fri Feb 14 13:47:00 2003
]2[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
D (dominanta) = [Author ID2: at Fri Feb 14 13:47:00 2003
]0[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]
|
|
Najwięcej razy występuje [Author ID2: at Fri Feb 14 13:47:00 2003
]zero[Author ID2: at Fri Feb 14 13:47:00 2003
] (cztery razy) więc jest dominantą.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Me (mediana) → uporządkowanie - 0, 0[Author ID2: at Fri Feb 14 13:47:00 2003
], 0, 0, 1, (1, 2), 2, 2, 3, 3, 4[Author ID0: at Thu Nov 30 00:00:00 1899
]
Me = (1+2)/2 = 1,5 [Author ID2: at Fri Feb 14 13:47:00 2003
]≈[Author ID2: at Fri Feb 14 13:47:00 2003
] [Author ID2: at Fri Feb 14 13:47:00 2003
]2[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Średnia = 211/114 = 1,85 [Author ID2: at Fri Feb 14 13:47:00 2003
]≈[Author ID2: at Fri Feb 14 13:47:00 2003
] [Author ID2: at Fri Feb 14 13:47:00 2003
]2[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
D = [Author ID2: at Fri Feb 14 13:47:00 2003
]2[Author ID2: at Fri Feb 14 13:47:00 2003
] (dwójka występuje najwięcej razy)[Author ID0: at Thu Nov 30 00:00:00 1899
]
Me = [Author ID2: at Fri Feb 14 13:47:00 2003
]2[Author ID2: at Fri Feb 14 13:47:00 2003
] (która wartość(x) znajduje się w środku (114/2=57) ciągu uporządkowanego - zero występuje (23) razy więc nie przekracza połowy ciągu → dodajemy jedynki, które występują (15) razy - (23+15=48) jeszcze nie przekroczyliśmy połowy ciągu → dodajemy dwójki, które występują (43) razy - (48+43=91). W tym momencie przekroczyliśmy połowę ciągu (57) więc na pewno dwójka znajduje się w połowie ciągu. [Powinniśmy brać pod uwagę (57) i (58) pozycję ciągu ponieważ ciąg jest parzysty jednak w tym przypadku są to dwie dwójki (2+2)/2=[Author ID2: at Fri Feb 14 13:47:00 2003
]2[Author ID2: at Fri Feb 14 13:47:00 2003
]][Author ID0: at Thu Nov 30 00:00:00 1899
]
Średnia = 805/50 = [Author ID2: at Fri Feb 14 13:47:00 2003
]16,1 [Author ID2: at Fri Feb 14 13:47:00 2003
](nie zaokrąglamy ponieważ działamy na przedziałach, o których nie wiemy czy nie posiadają czasami wartości po przecinku).[Author ID2: at Fri Feb 14 13:47:00 2003
]
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]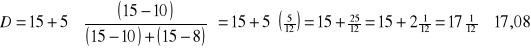
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]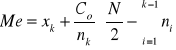
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID2: at Fri Feb 14 13:47:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:47:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Ćwiczenia 06-10-2002[Author ID0: at Thu Nov 30 00:00:00 1899
]
wykład 12-10-2002 r.[Author ID2: at Fri Feb 14 13:47:00 2003
]
Miary dyspersji (rozproszenia - zróżnicowania zjawiska)
R = xmax - xmin
Przykład:
Jeżeli w przedsiębiorstwie najwyższa płaca wynosi 4.800 zł. A najniższa 800 zł. To rozstęp wynosi 4.800 - 800 = 4.000 zł.
![]()
![]()


![]()
Współczynnik zmienności jest miarą „dobroci” średniej (arytmetycznej)
![]()
jeżeli: Vx ≤ 35% to średnia jest „bardzo dobra” (bardzo dobrze opisuje badaną rzeczywistość),
35% ≤ Vx ≤ 68% to średnia jest „dobra”,
68% ≤ Vx ≤ 75% to średnia jest „do przyjęcia”,
Vx > 75% to średnia traci swój sens poznawczy.
Miary skośności (asymetrii)
b - rozkład symetryczny (osią symetrii byłaby rzędna)
a, c - rozkłady asymetryczne; a - ma asymetrię lewostronną, c - asymetrię prawostronną
Najprostszą miarą asymetrii jest różnica pomiędzy średnią arytmetyczną a dominantą.
Rb =x - D = 0
Ra =x - D < 0 rozkład o asymetrii ujemnej
Rc =x - D > 0 rozkład o asymetrii dodatniej
Wzajemne położenie średniej, dominanty i Mediany w rozkładzie
Przy asymetrii ujemnej średnia arytmetyczna jest zaniżona, przy asymetrii dodatniej średnia arytmetyczna jest zawyżona.
Mierniki asymetrii
Nasilenie asymetrii możemy mierzyć dwojako:
Jako tzw. współczynnik asymetrii
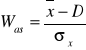
i zawiera się -1 ≤ Was ≤ 1
Za pomocą wyrażenia:
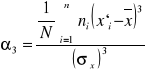
i zawiera się -2 ≤ α3 ≤ 2
Miary spłaszczenia (koncentracji wokół średniej)
Mierzymy ją wzorem:

Rozkład normalny - funkcja Gaussa.
Mamy z nią do czynienia gdy jednocześnie α3 = 0 i α4 = 3 i ma kształt symetryczny - dzwonowaty.
(wiadomo, że gdy α3=0 rozkład jest symetryczny).
Funkcja Gaussa ma postać:
( e ≈ 2,72 )
Okazuje się, że:
Regóła trzech “sigm”:
Przedział x - σx ≤ xtyp ≤ x + σx nazywamy “x - typowym”.
Charakterystyka średnich:
Warunki stosowania parametrów opisowych:
Ćwiczenia 26-10-2002[Author ID0: at Thu Nov 30 00:00:00 1899
]
WZORY WYŻEJ W TEKŚCIE[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:47:00 2003
]x = 29,8 Me = 30 D = 31, 875 [Author ID2: at Fri Feb 14 13:47:00 2003
]σ[Author ID2: at Fri Feb 14 13:47:00 2003
]x[Author ID2: at Fri Feb 14 13:47:00 2003
] = 10,44[Author ID0: at Thu Nov 30 00:00:00 1899
]
R = 50 Po = 8,8 v[Author ID2: at Fri Feb 14 13:47:00 2003
]x[Author ID2: at Fri Feb 14 13:47:00 2003
] = 35,03 dobra średnia W[Author ID2: at Fri Feb 14 13:47:00 2003
]as[Author ID2: at Fri Feb 14 13:47:00 2003
] = - 0,2 [Author ID0: at Thu Nov 30 00:00:00 1899
]
Q[Author ID2: at Fri Feb 14 13:47:00 2003
]1[Author ID2: at Fri Feb 14 13:47:00 2003
] [Author ID2: at Fri Feb 14 13:47:00 2003
]≈[Author ID2: at Fri Feb 14 13:47:00 2003
] 21,67 Q[Author ID2: at Fri Feb 14 13:47:00 2003
]2[Author ID2: at Fri Feb 14 13:47:00 2003
] [Author ID2: at Fri Feb 14 13:47:00 2003
]≈[Author ID2: at Fri Feb 14 13:47:00 2003
] 38,33 Q = 8,33 [Author ID0: at Thu Nov 30 00:00:00 1899
]
29,8 - 10,44 = 19,36 29,8 - 10,44 = 40,24 19,36 < x-typowe < 40,24[Author ID0: at Thu Nov 30 00:00:00 1899
]
Wykład 27-1[Author ID2: at Fri Feb 14 13:47:00 2003
]0-2002[Author ID2: at Fri Feb 14 13:47:00 2003
]
W październiku 1999 r. Na pewnym lokalnym rynku nieruchomości zanotowano następujące ceny ofertowe mieszkań:
Cena w tys. zł. |
Liczba mieszkań |
60 - 70 |
1 |
70 - 80 |
3 |
|
20 |
90 - 100 |
26 |
100 - 110 |
14 |
110 - 120 |
12 |
120 - 130 |
10 |
130 - 140 |
7 |
140 - 150 |
5 |
150 - 160 |
2 |
Razem |
100 |
Zbiorowość - liczba mieszkań.
Jednostka statystyczna - jedno mieszkanie.
Badana cecha - cena (x)
Kompleksowe badanie polega na:
x = 105,4 Me = 100 D = 93,3 σx = 19,85
R = 100 Po = 8,8 vx = 18,83% Was = - 0,61
105,4 - 19,85 = 85,55 105,4 + 19,85 = 125,25 85,55 < x-typowe < 125,25
Wykład 09-11-2002[Author ID0: at Thu Nov 30 00:00:00 1899
]
Najpotrzebniejsze informacje:
UWAGA : Zbiór wszystkich badanych cech statystycznych nazywamy zakresem badania.
===============================================================================================[Author ID2: at Fri Feb 14 13:48:00 2003
][Author ID1: at Wed Aug 20 16:25:00 2003
]
Analiza korelacji i regresji.
O korelacji mówimy, wtedy jeżeli średnim wartościom jednej cechy ściśle odpowiadają wartości drugiej cechy.
(funkcja - każdej wartości x odpowiada tylko jedna wartość y).
Wyróżnia się korelację liniową lub krzywoliniową - wśród której wyróżniamy korelację dwóch lub wielu cech statystycznych. Gdy objaśniamy jedną cechę (objaśnianą) całym zbiorem cech (objaśniających) to mamy do czynienia z korelacją wieloraką.
Jeżeli natomiast chcemy znać rzeczywistą współzależność pomiędzy dwoma cechami z wyeliminowaniem wpływu pozostałych cech to mamy do czynienia z tzw. korelacją cząstkową.
Dalsze rozważania ograniczamy do korelacji liniowej dwóch cech.
Prosty związek (współzależność) pomiędzy dwoma cechami statystycznymi można zbadać (zaobserwować) na podstawie tzw. diagramów korelacyjnych (wykresów korelacyjnych).
UWAGA : Badanie współzależności dwóch cech przeprowadzamy wtedy i tylko wtedy jeżeli pomiędzy tymi cechami zachodzi związek logiczny. (np. im wyższe wykształcenie tym wyższe kwalifikacje, ale nie koniecznie odwrotnie).
Równania regresji dwóch zmiennych służą do oszacowania średniej wielkości jednej zmiennej.
Diagram (C) wykazuje, że pomiędzy cechami (x) i (y) nie zachodzi żadna korelacja.
Diagram (D) mówi, że pomiędzy cechami (x) i (y) zachodzi korelacja krzywo-liniowa.
Diagramy (A) i (B) wskazują na istnienie korelacji liniowej. W przypadku diagramu (A) dodatniej a w diagramie (B) ujemnej.
Korelacja dodatnia oznacza związek wprost proporcjonalny, w którym wraz ze wzrostem wartości jednej cechy rośnie wartość cechy drugiej.
Korelacja ujemna oznacza związek odwrotnie proporcjonalny, w którym wraz ze wzrostem wartości jednej cechy maleje wartość cechy drugiej.
Korelację liniową mierzy się za pomocą współczynnika korelacji liniowej Pearsona, która ma postać:
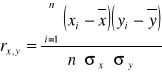
Właściwości współczynnika :
Jeżeli r = ± 1 to mamy do czynienia z zależnością funkcyjną, matematyczną.
Jeżeli 0 < r < 1 to mamy do czynienia ze związkiem wprost proporcjonalnym.
Jeżeli - 1 < r < 0 to mamy do czynienia ze związkiem odwrotnie proporcjonalnym.
Jeżeli r jest nie większa niż 0,3 to mówimy, że korelacja jest niewyraźna.
Jeżeli r jest większa niż 0,3 a mniejsza niż 0.5 to mówimy, że korelacja jest średnia.
Jeżeli r jest większa niż 0,5 to mówimy, że korelacja jest wyraźna.
Równanie regresji liniowej dwóch zmiennych ma zastosowanie wtedy gdy mając dane dla jednej cechy można w przybliżeniu określić średnie wielkości lub wartości drugiej cechy.
Zależność cechy (y) od cechy (x) można wyrazić za pomocą następujących równań regresji:
1. 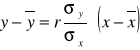
po przekształceniu otrzymamy
2. y = a + bx
Zależność cechy (x) od cechy (y) można wyrazić za pomocą następujących równań regresji:
3. 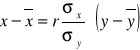
po przekształceniu otrzymamy
4. x = a + by
UWAGA : Kierunek regresji w obu równaniach (2 i 4) zależy wyłącznie od współczynnika (b). (a) nie ma związku ze współczynnikiem korelacji.
Wzajemne położenie linii regresji:
Jeżeli oba równania się pokrywają to korelacja przekształca się w związek funkcyjny (czyli r = -1 lub r = 1).
Jeżeli α = 90° to r = 0 więc nie ma korelacji
Szacując jedną cechę na podstawie drugiej popełnia się tzw. błędy standardowe szacunku:
Dla równania (2) ![]()
Dla równania (4) ![]()
Ćwiczenia 09-11-2002[Author ID0: at Thu Nov 30 00:00:00 1899
]
Szereg rozdzielczy otwarty[Author ID2: at Fri Feb 14 13:50:00 2003
]
|
||
Rozwiązanie:[Author ID0: at Thu Nov 30 00:00:00 1899
]
Mediana [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]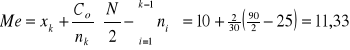
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Dominanta [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
I Kwartyl [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]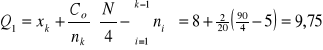
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
III Kwartyl [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]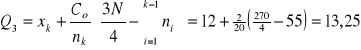
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Odchylenie ćwiartkowe [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]
x typowe Me - Q < x-typowe < Me + Q[Author ID2: at Fri Feb 14 13:50:00 2003
]
x-typowe ( 9,58 ; 13,08 )[Author ID2: at Fri Feb 14 13:50:00 2003
]
Kredytobiorcy wg wieku[Author ID0: at Thu Nov 30 00:00:00 1899
]
|
Średnia [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]x = 2514 / 74 = 33,97[Author ID0: at Thu Nov 30 00:00:00 1899
]
Odchylenie standardowe [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Dominanta [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Mediana [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]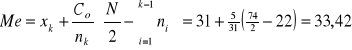
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Rozstęp 56 - 21 = 35 [Author ID2: at Fri Feb 14 13:50:00 2003
](choć nie jestem pewien czy można obliczać rozstęp skoro przedział był otwarty)[Author ID2: at Fri Feb 14 13:50:00 2003
] [Author ID0: at Thu Nov 30 00:00:00 1899
]
Odchylenie przeciętne [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Współczynnik zmienności [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
] - „bardzo dobra” średnia[Author ID0: at Thu Nov 30 00:00:00 1899
]
Współczynnik asymetrii [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]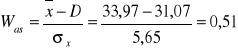
[Author ID2: at Fri Feb 14 13:50:00 2003
] - rozkład o asymetrii dodatniej (prawostronny)[Author ID0: at Thu Nov 30 00:00:00 1899
]
x- typowe (28,32 ; 39,62) 33,97 - 5,65 = 28,32 33,97 + 5,65 = 39,62[Author ID0: at Thu Nov 30 00:00:00 1899
]
czyli w przedziale pomiędzy 28,32 a 39,62 (lat) znajduje się około 68% wielkości całej badanej cechy (ilość kredytobiorców)[Author ID0: at Thu Nov 30 00:00:00 1899
]
Wykład 14-12-2002[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Korelacja - współzależność 2 cech
Przykład
W 20 losowo wybranych mieszkaniach zaobserwowano następujące relacje pomiędzy liczbą pokoi w mieszkaniu a liczbą zamieszkujących te pokoje osób.
Liczba pokoi (y) |
7 |
1 |
5 |
4 |
2 |
3 |
6 |
5 |
4 |
3 |
2 |
2 |
1 |
3 |
5 |
4 |
5 |
3 |
4 |
1 |
Liczba osób (x) |
6 |
1 |
6 |
4 |
2 |
2 |
5 |
4 |
3 |
3 |
3 |
1 |
3 |
4 |
4 |
4 |
5 |
4 |
5 |
1 |
Z wykresu korelacyjnego wynika, że mamy do czynienia z istotnym, liniowym, dodatnim związkiem korelacyjnym. Oznacza to, że korelacja ma charakter wprost proporcjonalny, gdzie wraz ze wzrostem liczby osób w rodzinie rośnie ilość zajmowanych przez tę rodzinę pokoi (z układu punktów wynika, że współczynnik korelacji musi mieć znak dodatni (+), a wartość współczynnika musi być powyżej 0,6.
Ponieważ korelacja jest widoczna można przystąpić do oszacowania wielkości mieszkania dla 4 osobowej rodziny (szacujemy „y”).
Korzystamy w tym celu z linii regresji szacującej na podstawie której oszacujemy średnią wielkość (y) na podstawie (x = 4).
x |
y |
x -x |
y -y |
(x -x)⋅(y -y) |
(x -x)2 |
(y -y)2 |
6 |
7 |
2,50 |
3,50 |
8,75 |
6,25 |
12,25 |
1 |
1 |
-2,50 |
-2,50 |
6,25 |
6,25 |
6,25 |
6 |
5 |
2,50 |
1,50 |
3,75 |
6,25 |
2,25 |
4 |
4 |
0,50 |
0,50 |
0,25 |
0,25 |
0,25 |
2 |
2 |
-1,50 |
-1,50 |
2,25 |
2,25 |
2,25 |
2 |
3 |
-1,50 |
-0,50 |
0,75 |
2,25 |
0,25 |
5 |
6 |
1,50 |
2,50 |
3,75 |
2,25 |
6,25 |
4 |
5 |
0,50 |
1,50 |
0,75 |
0,25 |
2,25 |
3 |
4 |
-0,50 |
0,50 |
-0,25 |
0,25 |
0,25 |
3 |
3 |
-0,50 |
-0,50 |
0,25 |
0,25 |
0,25 |
3 |
2 |
-0,50 |
-1,50 |
0,75 |
0,25 |
2,25 |
1 |
2 |
-2,50 |
-1,50 |
3,75 |
6,25 |
2,25 |
3 |
1 |
-0,50 |
-2,50 |
1,25 |
0,25 |
6,25 |
4 |
3 |
0,50 |
-0,50 |
-0,25 |
0,25 |
0,25 |
4 |
5 |
0,50 |
1,50 |
0,75 |
0,25 |
2,25 |
4 |
4 |
0,50 |
0,50 |
0,25 |
0,25 |
0,25 |
5 |
5 |
1,50 |
1,50 |
2,25 |
2,25 |
2,25 |
4 |
3 |
0,50 |
-0,50 |
-0,25 |
0,25 |
0,25 |
5 |
4 |
1,50 |
0,50 |
0,75 |
2,25 |
0,25 |
1 |
1 |
-2,50 |
-2,50 |
6,25 |
6,25 |
6,25 |
70 |
70 |
x |
x |
42,00 |
45,00 |
55,00 |
Średnia x x = 70 / 20 = 3,5
Średnia y y = 70 / 20 = 3,5
![]()
![]()
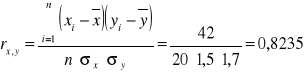
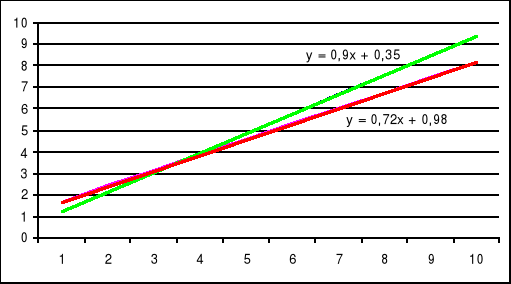
Obliczony współczynnik korelacji potwierdza istotną, liniową, dodatnia (wprost proporcjonalną) korelację pomiędzy wielkością rodziny i wielkością mieszkania. Logiczny związek pomiędzy tymi dwoma cechami oraz wysoki współczynnik korelacji sprawiają, że można przejść do oszacowania wielkości mieszkania dla 4 osobowej rodziny:
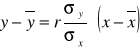
![]()
![]()
![]()
![]()
błąd oszacowania:
![]()
Wielkość mieszkania oszacowana przedziałowo z błędem (±1,02) pokoi wynosi (3,9 - 1,02 ; 3,9 + 1,02) czyli (3 ; 5)
Zadanie
Wyznaczyć linie regresji dla (x) i narysować wspólny wykres.
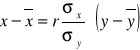
![]()
![]()
![]()
Ćwiczenia 14-12-2002[Author ID2: at Fri Feb 14 13:50:00 2003
]
Regresja - zależność[Author ID0: at Thu Nov 30 00:00:00 1899
]
Galton XIX w.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Regres w kierunku średniej[Author ID0: at Thu Nov 30 00:00:00 1899
]
R = korelacja - miara siły dobroci dopasowania liniowego.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Badamy zależność 2 cech[Author ID0: at Thu Nov 30 00:00:00 1899
]
Średnia x [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]x = 21 / 12 = 1,75 Średnia y [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]y = 174 / 12 = 14,5[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
] [Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]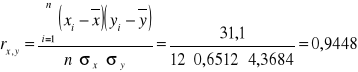
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]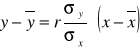
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID2: at Fri Feb 14 13:50:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Wykład 15-12-2002[Author ID2: at Fri Feb 14 13:50:00 2003
]
Wskaźniki statystyczne powstają w wyniku logicznego porównania wielkości absolutnych. Wszystkie wskaźniki statystyczne można sklasyfikować w trzy następujące grupy:[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:51:00 2003
] lub [Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:51:00 2003
] gdzie: C = 1;100,1000[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:51:00 2003
] lub [Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:51:00 2003
] (w procentach)[Author ID0: at Thu Nov 30 00:00:00 1899
]
gdzie: [Author ID2: at Fri Feb 14 13:51:00 2003
](Y[Author ID2: at Fri Feb 14 13:51:00 2003
]t[Author ID2: at Fri Feb 14 13:51:00 2003
])[Author ID2: at Fri Feb 14 13:51:00 2003
] - poziom zjawisk w okresie badanym,[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:51:00 2003
](Y[Author ID2: at Fri Feb 14 13:51:00 2003
]0[Author ID2: at Fri Feb 14 13:51:00 2003
])[Author ID2: at Fri Feb 14 13:51:00 2003
] - poziom zjawiska w okresie przyjętym jako podstawowy.[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:51:00 2003
] lub [Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:51:00 2003
] (w procentach)[Author ID0: at Thu Nov 30 00:00:00 1899
]
gdzie: [Author ID2: at Fri Feb 14 13:51:00 2003
](Y[Author ID2: at Fri Feb 14 13:51:00 2003
]t[Author ID2: at Fri Feb 14 13:51:00 2003
])[Author ID2: at Fri Feb 14 13:51:00 2003
] - określa wartość (poziom) zjawiska w okresie [Author ID2: at Fri Feb 14 13:51:00 2003
]bezpośrednio poprzedzającym[Author ID2: at Fri Feb 14 13:51:00 2003
] okres badany.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Interpretacja obu rodzajów zjawisk jest następująca:[Author ID0: at Thu Nov 30 00:00:00 1899
]
Przyrosty absolutne i względne - to najprostsze miary zmian zjawiska w czasie.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Przyrost absolutny jednopodstawowy to:[Author ID2: at Fri Feb 14 13:51:00 2003
]
P[Author ID2: at Fri Feb 14 13:51:00 2003
]ab/0[Author ID2: at Fri Feb 14 13:51:00 2003
] = Y[Author ID2: at Fri Feb 14 13:51:00 2003
]t[Author ID2: at Fri Feb 14 13:51:00 2003
] - Y[Author ID2: at Fri Feb 14 13:51:00 2003
]0[Author ID2: at Fri Feb 14 13:51:00 2003
] gdzie: [Author ID2: at Fri Feb 14 13:51:00 2003
]Y[Author ID2: at Fri Feb 14 13:51:00 2003
]t[Author ID2: at Fri Feb 14 13:51:00 2003
] [Author ID2: at Fri Feb 14 13:51:00 2003
]- poziom zjawiska w okresie t(t[Author ID2: at Fri Feb 14 13:51:00 2003
]1[Author ID2: at Fri Feb 14 13:51:00 2003
], t[Author ID2: at Fri Feb 14 13:51:00 2003
]2[Author ID2: at Fri Feb 14 13:51:00 2003
], t[Author ID2: at Fri Feb 14 13:51:00 2003
]3[Author ID2: at Fri Feb 14 13:51:00 2003
], ...)[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:51:00 2003
]Y[Author ID2: at Fri Feb 14 13:51:00 2003
]0[Author ID2: at Fri Feb 14 13:51:00 2003
] - poziom zjawiska w okresie przyjętym jako bazowy[Author ID0: at Thu Nov 30 00:00:00 1899
]
Przyrost absolutny łańcuchowy to:[Author ID2: at Fri Feb 14 13:51:00 2003
]
P[Author ID2: at Fri Feb 14 13:51:00 2003
]ab/t-1[Author ID2: at Fri Feb 14 13:51:00 2003
] = Y[Author ID2: at Fri Feb 14 13:51:00 2003
]t[Author ID2: at Fri Feb 14 13:51:00 2003
] - Y[Author ID2: at Fri Feb 14 13:51:00 2003
]t-1[Author ID2: at Fri Feb 14 13:51:00 2003
] [Author ID2: at Fri Feb 14 13:51:00 2003
]
P[Author ID2: at Fri Feb 14 13:51:00 2003
]ab/0[Author ID2: at Fri Feb 14 13:51:00 2003
] oraz [Author ID2: at Fri Feb 14 13:51:00 2003
]P[Author ID2: at Fri Feb 14 13:51:00 2003
]ab/t-1[Author ID2: at Fri Feb 14 13:51:00 2003
] może przyjmować wartość dodatnią (+), ujemną (-) lub zero (0)[Author ID2: at Fri Feb 14 13:51:00 2003
]
UWAGA: Przyrost absolutne są wielkościami mianowanymi wyrażanymi w takich samych jednostkach miary jak badane zjawisko, nie nadają się zatem do porównania dynamiki zjawisk wyrażonych w różnych jednostkach miary.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Liczba oddanych mieszkań w latach 1995 - 2001 przedstawia się następująco:[Author ID2: at Fri Feb 14 13:51:00 2003
]
Średnia chronologiczna ma zastosowanie do badania średniego poziomu zjawiska w szeregu czasowym zwykle dla jednostek czasowych krótszych niż jeden rok (kwartał, miesiąc, tydzień) i wyraża się następującym wzorem:[Author ID2: at Fri Feb 14 13:51:00 2003
]
[Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Stan zatrudnienia w przedsiębiorstwie w 2001 roku kształtował się następująco:[Author ID0: at Thu Nov 30 00:00:00 1899
]
Styczeń - 500 osób[Author ID0: at Thu Nov 30 00:00:00 1899
]
Luty - 600 osób[Author ID0: at Thu Nov 30 00:00:00 1899
]
Marzec - 620 osób[Author ID0: at Thu Nov 30 00:00:00 1899
]
Kwiecień - 660 osób[Author ID0: at Thu Nov 30 00:00:00 1899
]
Jakie było średnie miesięczne zatrudnienie w I kwartale 2001 roku[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:51:00 2003
]x[Author ID2: at Fri Feb 14 13:51:00 2003
]ch[Author ID2: at Fri Feb 14 13:51:00 2003
] = 250 + 600 + 620 + 330 = 600[Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID2: at Fri Feb 14 13:51:00 2003
]x[Author ID2: at Fri Feb 14 13:51:00 2003
]ar[Author ID2: at Fri Feb 14 13:51:00 2003
] = 500 + 600 + 620 = 573,3[Author ID0: at Thu Nov 30 00:00:00 1899
]
Indeksy zespołowe (agregatowe) umożliwiają badanie dynamiki takich zjawisk które nie mają wspólnego miana, czyli gdy są wyrażane w różnych jednostkach miary np. minimum socjalne ludności, badanie dynamiki produkcji zakładu wytwarzającego towar o różnej jednostce miary i różnym przeznaczeniu.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Wykład 04-01-2003[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
Badanie różnorodnych produktów i usług możliwe jest przy sprowadzeniu ich do jednego mianownika (pieniądz - wartość).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Wyróżnia się trzy rodzaje indeksów agregatowych:[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Stosunek (zakupionych, wyprodukowanych) ilości dóbr lub usług pomnożonych przez ich cenę w okresie badanym do wartości towarów lub usług w okresie bazowym (podstawowym).[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]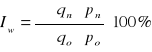
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
gdzie: q[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]n[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] - ilość dóbr lub usług w okresie badanym.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
q[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]o[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] - ilość dóbr lub usług w okresie bazowym.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
p[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]n[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] - ceny w okresie badanym.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
p[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]o[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] - ceny w okresie bazowym.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]UWAGA[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] - indeks ten mówi tylko o wartości spożycia dóbr lub usług. Na jego wpływ maja zarówno ilości (q) spożywanych dóbr lub usług jak i ceny (p) dóbr lub usług. [Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Dlatego też dla wyznaczenia wpływu na tę wartość cen stosuje się agregatowe indeksy cen a dla zbadania wpływu na tę wartość ilości zakupionych dóbr i usług stosuje się agregatowe indeksy fizycznych rozmiarów (ilość).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]Uwaga[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] - Wszystkie indeksy agregatowe można stosować jako indeksy jedno podstawowe lub o zmiennej podstawie.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
W agregatowych indeksach cen przyjmuje się stałość ilości (q - constans), zaś w agregatowych indeksach ilości jako stałe przyjmuje się cenę (p -constans).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Ogólna postać agregatowego indeksu cen przedstawia się następująco:[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]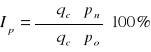
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Indeks ten można rozpatrywać wg dwóch formuł:[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
1. Laspeyresa 2. Paaschego[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
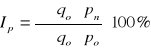
[Author ID5: at Mon Jan 27 14:41:00 2003
] [Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]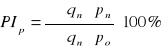
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Ogólna postać przedstawia się następująco:[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]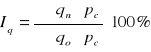
[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] gdzie - p[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]c[Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] - constans[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Podobnie jak indeksy cen tak i agregatowe indeksy ilości można rozpatrywać w dwóch formułach. [Author ID5: at Mon Jan 27 14:41:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:42:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
1. Laspeyresa 2. Paaschego[Author ID5: at Mon Jan 27 14:42:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
[Author ID5: at Mon Jan 27 14:43:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:43:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]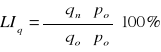
[Author ID5: at Mon Jan 27 14:43:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] [Author ID5: at Mon Jan 27 14:42:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:42:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Mon Jan 27 14:42:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]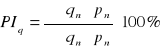
[Author ID5: at Mon Jan 27 14:42:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Indeksy obliczane wg obu wymienionych formuł mogą nawet znacznie się różnić. Różnice mogą być tym większe im bardziej różnią się wagi w okresie badanym i okresie przyjętym jako bazowy. Różnice te można w jakimś sensie zlikwidować stosując dwa następujące indeksy[Author ID5: at Mon Jan 27 14:47:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] Fishera. Oba indeksy są średnią geometryczną[Author ID5: at Mon Jan 27 14:49:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] indeksu cen wg formuł Laspeyresa i Paaschego.[Author ID5: at Mon Jan 27 14:50:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Wed Jan 29 11:13:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
[Author ID5: at Wed Jan 29 11:10:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Wed Jan 29 11:10:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID5: at Wed Jan 29 11:10:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] [Author ID5: at Wed Jan 29 11:10:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Wed Jan 29 11:13:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
mówi o ile średnio wzrosła (spadła) wartość dóbr lub usług w wyniku zmian cen.[Author ID5: at Wed Jan 29 11:10:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Wed Jan 29 11:13:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
[Author ID5: at Wed Jan 29 11:11:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Wed Jan 29 11:11:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]![]()
[Author ID5: at Wed Jan 29 11:11:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] [Author ID5: at Wed Jan 29 11:11:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
][Author ID5: at Wed Jan 29 11:13:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
daje możliwość zbadania [Author ID5: at Wed Jan 29 11:11:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]dynamiki wartości dóbr lub usług spowodowanej wzrostem (spadkiem fizycznych rozmiarów[Author ID5: at Wed Jan 29 11:12:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
] spożycia.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Nie można [Author ID5: at Wed Jan 29 11:13:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]odzwierciedlać wartości spożycia cenami ani fizycznym rozmiarem sp[Author ID5: at Wed Jan 29 11:14:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]ożycia.[Author ID5: at Wed Jan 29 11:15:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
Wykład 0[Author ID5: at Wed Jan 29 11:15:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]5-01-2003[Author ID5: at Wed Jan 29 11:16:00 2003
][Author ID2: at Fri Feb 14 13:51:00 2003
]
Badanie tendencji rozwojowej.[Author ID0: at Thu Nov 30 00:00:00 1899 ]
Tendencja rozwojowa [Author ID5: at Wed Jan 29 11:16:00 2003 ]-[Author ID5: at Wed Jan 29 11:17:00 2003 ]ogólna [Author ID5: at Wed Jan 29 11:16:00 2003 ]dążność zjawiska do wzrostu lub spadku. Bada się ją zwykle w okresach 10 i więcej lat. Warunkiem doboru okresu badania jest [Author ID5: at Wed Jan 29 11:17:00 2003 ]działanie[Author ID5: at Wed Jan 29 11:18:00 2003 ] tych samych przyczyn głównych[Author ID5: at Wed Jan 29 11:17:00 2003 ]. Ogólną tendencję ro[Author ID5: at Wed Jan 29 11:18:00 2003 ]zwojową zakłóca działanie tzw. przyczyn ubocznych, które można w jakimś sensie zneutralizować poprzez tzw. wygładzanie szeregów chronologicznych. Są dwie metody wygładzania szeregów chronologicznych.[Author ID5: at Wed Jan 29 11:19:00 2003 ][Author ID5: at Wed Jan 29 12:08:00 2003 ]
Jeżeli wartość zjawiska w kolejnych latach wynosi (y[Author ID5: at Wed Jan 29 11:21:00 2003 ]1[Author ID5: at Wed Jan 29 11:24:00 2003 ], y[Author ID5: at Wed Jan 29 11:24:00 2003 ]2[Author ID5: at Wed Jan 29 11:24:00 2003 ], y[Author ID5: at Wed Jan 29 11:24:00 2003 ]3[Author ID5: at Wed Jan 29 11:24:00 2003 ], y[Author ID5: at Wed Jan 29 11:24:00 2003 ]1[Author ID5: at Wed Jan 29 11:24:00 2003 ], ... y[Author ID5: at Wed Jan 29 11:24:00 2003 ]n-1[Author ID5: at Wed Jan 29 11:25:00 2003 ], y[Author ID5: at Wed Jan 29 11:24:00 2003 ]n[Author ID5: at Wed Jan 29 11:25:00 2003 ])[Author ID5: at Wed Jan 29 11:24:00 2003 ] to trzyletnie średnie ruchome mają postać[Author ID5: at Wed Jan 29 11:25:00 2003 ][Author ID5: at Wed Jan 29 11:28:00 2003 ]
[Author ID5: at Wed Jan 29 11:28:00 2003
][Author ID5: at Wed Jan 29 11:28:00 2003
]![]()
[Author ID5: at Wed Jan 29 11:28:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Następna średnia ruchoma[Author ID5: at Wed Jan 29 11:31:00 2003 ] powstanie z pierwszej przez opuszczenie wyrazu pierwszego i dodanie wyrazu czwartego[Author ID5: at Wed Jan 29 11:32:00 2003 ][Author ID5: at Wed Jan 29 11:33:00 2003 ]
[Author ID5: at Wed Jan 29 11:33:00 2003
][Author ID5: at Wed Jan 29 11:33:00 2003
]![]()
[Author ID5: at Wed Jan 29 11:33:00 2003
] itd.[Author ID0: at Thu Nov 30 00:00:00 1899
]
Ostatnia trzyelementowa [Author ID5: at Wed Jan 29 11:33:00 2003 ]średnia ruchoma mieć będzie postać:[Author ID5: at Wed Jan 29 11:48:00 2003 ]
[Author ID5: at Wed Jan 29 11:48:00 2003
][Author ID5: at Wed Jan 29 11:48:00 2003
]![]()
[Author ID5: at Wed Jan 29 11:48:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Pięcioelementowe średnie ruchome tworzymy w analogiczny sposób pamiętając, że[Author ID5: at Wed Jan 29 11:49:00 2003 ] uśredniamy element środkowy:[Author ID5: at Wed Jan 29 11:50:00 2003 ]
[Author ID5: at Wed Jan 29 11:50:00 2003
][Author ID5: at Wed Jan 29 11:50:00 2003
]![]()
[Author ID5: at Wed Jan 29 11:50:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Średnie ruchome umożliwiają eliminowanie z szeregu wahań[Author ID5: at Wed Jan 29 11:51:00 2003 ] przypadkowych. Im średnia ruchoma obejmuje więcej elementów tym bardziej są eliminowa[Author ID5: at Wed Jan 29 11:52:00 2003 ]ne przyczyny uboczne a szereg chronologiczny jest bardziej wygładzony.[Author ID5: at Wed Jan 29 11:53:00 2003 ]
Gdy liczba obserwacji jest parzysta średnia chronologiczna przybiera postać średniej ruchomej scentrowanej:[Author ID5: at Wed Jan 29 11:54:00 2003 ]
[Author ID5: at Wed Jan 29 11:54:00 2003
][Author ID5: at Wed Jan 29 11:54:00 2003
]![]()
[Author ID5: at Wed Jan 29 11:54:00 2003
][Author ID5: at Wed Jan 29 12:08:00 2003
]
Zaletą metody mechanicznej jest to, iż umożliwia ona uzyskanie obrazu uwolnione[Author ID5: at Wed Jan 29 12:04:00 2003 ]go od działania czynników ubocznych, wadą natomiast jest po pierwsze skracanie szeregu chronologicznego, po drugie niemożność zapisu tendencji rozwojowej w postaci matematycznej, po trzecie niemożność ekstrapolacji (przewidywania) zjawiska w przyszłości.[Author ID5: at Wed Jan 29 12:05:00 2003 ]
Suma kwadratów odchyleń wartości empirycznych (doświadczalnych) i uzyskanych na podstawie a[Author ID5: at Wed Jan 29 12:14:00 2003 ]proksymanty ma być jak najmniejsza (aproksymanta ma odpowiadać klasycznej metodzie najmniejszych kwadratów).[Author ID5: at Wed Jan 29 12:15:00 2003 ]
Sposób postępowania:[Author ID5: at Wed Jan 29 12:16:00 2003 ]
[Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
]![]()
[Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:20:00 2003
]
[Author ID5: at Wed Jan 29 12:55:00 2003 ] [Author ID5: at Wed Jan 29 13:00:00 2003 ]b [Author ID5: at Wed Jan 29 12:56:00 2003 ]>[Author ID5: at Wed Jan 29 12:55:00 2003 ] [Author ID5: at Wed Jan 29 12:56:00 2003 ]0[Author ID5: at Wed Jan 29 12:55:00 2003 ][Author ID0: at Thu Nov 30 00:00:00 1899 ]
[Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
]![]()
[Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:20:00 2003
]
[Author ID5: at Wed Jan 29 12:56:00 2003 ]b[Author ID5: at Wed Jan 29 12:57:00 2003 ] < 0[Author ID5: at Wed Jan 29 12:56:00 2003 ][Author ID0: at Thu Nov 30 00:00:00 1899 ]
[Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
]![]()
[Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:20:00 2003
]
[Author ID5: at Wed Jan 29 12:58:00 2003
][Author ID5: at Wed Jan 29 12:59:00 2003
][Author ID5: at Wed Jan 29 12:59:00 2003
]![]()
[Author ID5: at Wed Jan 29 12:59:00 2003
][Author ID5: at Wed Jan 29 12:20:00 2003
]
[Author ID5: at Wed Jan 29 12:57:00 2003 ]a > 1[Author ID5: at Wed Jan 29 12:59:00 2003 ][Author ID0: at Thu Nov 30 00:00:00 1899 ]
[Author ID5: at Wed Jan 29 13:00:00 2003
][Author ID5: at Wed Jan 29 13:00:00 2003
]![]()
[Author ID5: at Wed Jan 29 13:00:00 2003
][Author ID5: at Wed Jan 29 12:21:00 2003
]
[Author ID5: at Wed Jan 29 13:01:00 2003
][Author ID5: at Wed Jan 29 13:01:00 2003
]![]()
[Author ID5: at Wed Jan 29 13:01:00 2003
][Author ID5: at Wed Jan 29 12:23:00 2003
]
[Author ID5: at Wed Jan 29 13:01:00 2003
][Author ID5: at Wed Jan 29 13:01:00 2003
][Author ID5: at Wed Jan 29 13:01:00 2003
]![]()
[Author ID5: at Wed Jan 29 13:01:00 2003
][Author ID5: at Wed Jan 29 13:01:00 2003
][Author ID5: at Wed Jan 29 12:23:00 2003
]
Trend liniowy[Author ID0: at Thu Nov 30 00:00:00 1899 ]
W trendzie liniowym i w każdym innym trendzie jedyną determinantą rozwoju zjawiska jest [Author ID5: at Wed Jan 29 14:05:00 2003 ]czas[Author ID5: at Wed Jan 29 14:05:00 2003 ]. [Author ID0: at Thu Nov 30 00:00:00 1899 ]
W równaniu trendu liniowego w postaci[Author ID5: at Wed Jan 29 14:05:00 2003 ]
[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
]![]()
[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
] [Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
]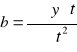
[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Równanie trendu linowego można wyznaczyć w sposób uproszczony postępując w następujący sposób:[Author ID0: at Thu Nov 30 00:00:00 1899 ]
1. Jeżeli szereg chronologiczny składa się z [Author ID5: at Wed Jan 29 14:05:00 2003 ]nieparzystej[Author ID5: at Wed Jan 29 14:05:00 2003 ] liczby danych to za wartość (t) dla wyrazu środkowego wpisujemy zero (0). Począwszy od zera w kierunku [Author ID5: at Wed Jan 29 14:05:00 2003 ]wcześniejszych[Author ID5: at Wed Jan 29 14:05:00 2003 ] wartości wpisujemy w miejsce (t) odpowiednio (-1, -2, -3, ...), dla wartości [Author ID5: at Wed Jan 29 14:05:00 2003 ]późniejszych[Author ID5: at Wed Jan 29 14:05:00 2003 ] począwszy od zera w miejsce (t) wpisujemy kolejno (1, 2, 3, ...).[Author ID0: at Thu Nov 30 00:00:00 1899 ]
Przykład (dane umowne)[Author ID0: at Thu Nov 30 00:00:00 1899 ]
Szereg nieparzysty[Author ID5: at Wed Jan 29 14:05:00 2003 ]
Szereg parzysty[Author ID5: at Wed Jan 29 14:05:00 2003 ]
Miarą dobroci oszacowań (np. przewidywanie produkcji) wartości uzyskanych na podstawie funkcji trendów jest wyrażenie:[Author ID5: at Wed Jan 29 14:05:00 2003 ]
[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
]![]()
[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID5: at Wed Jan 29 14:05:00 2003
] gdzie (K) jest liczbą parametrów funkcji[Author ID0: at Thu Nov 30 00:00:00 1899
]
dla funkcji (y=a+bt) dwa parametry (a,b), dla funkcji (y= a[Author ID5: at Wed Jan 29 14:05:00 2003 ]2[Author ID5: at Wed Jan 29 14:05:00 2003 ]+bt+c) trzy parametry (a,b,c)[Author ID0: at Thu Nov 30 00:00:00 1899 ]
Konie[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID1: at Wed Aug 20 16:25:00 2003
]c[Author ID2: at Mon Feb 17 12:38:00 2003
][Author ID1: at Wed Aug 20 16:25:00 2003
]C[Author ID2: at Mon Feb 17 12:38:00 2003
][Author ID1: at Wed Aug 20 16:25:00 2003
]c[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID1: at Wed Aug 20 16:25:00 2003
]Tematy ogólne na egzamin:[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Przedmiot i zadania statystyki[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Podział statystyki, podstawowe działy w statystyce opisowej.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Etapy badania statystycznego[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Badanie struktury zbiorowości.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Charakterystyka rozkładu normalnego (kształt, symetria, spłaszczenie, pole powierzchni pod krzywą, reguła trzech sigm).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Interpretacja zależności i współzależności cech statystycznych. Interpretacja współczynnika zależności Pearsona.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Linie regresji dwóch zmiennych (wzajemne położenie, punkty przecięcia, kąt rozwarcia, wykreślanie linii regresji).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Wykorzystanie linii regresji do ekstrapolacji zjawisk.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Rodzaje wskaźników statystycznych (współczynniki natężenia, wskaźniki struktury, indywidualne wskaźniki dynamiki).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Zastosowanie indywidualnych wskaźników dynamiki (jedno podstawowych, łańcuchowych).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Zastosowanie średniej chronologicznej.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Klasyfikacja indeksów zespołowych (agregatowych).[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Interpretacja zespołowych indeksów wartości, cen i fizycznych rozmiarów.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Na czym polega badanie dynamiki zjawisk.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Jakie przyczyny kształtują tendencję rozwojową a jakie zakłócają.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Sposoby „wygładzania” szeregów chronologicznych.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Na czym polega mechaniczna metoda wygładzania szeregów chronologicznych.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Na czym polega analityczna metoda wygładzania szeregów chronologicznych.[Author ID5: at Wed Jan 29 14:05:00 2003
][Author ID1: at Wed Aug 20 16:25:00 2003
]
Wady i zalety ogólnego wygładzania szeregów chronologicznych.[Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Badanie tendencji rozwojowej za pomocą trendu liniowego.[Author ID5: at Thu Jan 30 11:07:00 2003
][Author ID1: at Wed Aug 20 16:25:00 2003
][Author ID0: at Thu Nov 30 00:00:00 1899
][Author ID0: at Thu Nov 30 00:00:00 1899
]
Dodać wszystkie wartości danych (x) i podzielić przez ich liczbę
168+178+171+185+180+171+179+183+180+175 = 1770
1770 ÷10 = 177
W kolumnie (x) podano przedziały zarobków.
W kolumnie (ni) podano ilość osób które spełniają warunki kolumny (x).
W kolumnie (x`i) obliczono środek przedziału klasowego
Np. (700+800) ÷ 2 = 750
W kolumnie (ni⋅x`i) podano iloczyn dwóch wcześniejszych kolumn.
UWAGA: wartości graniczne przypisujemy do wyższej klasy (np. wartość 800 przypisujemy do drugiej klasy)
209.050 ÷ 139 = 1504
Liczebność (% lub liczby rzeczywiste
Wartość badanej cechy (cecha mierzalna
Dominanta rzeczywiste
wartość rzeczywiste
Dominanta
x
n
skumulowany szereg liczebności do przedziału poprzedzającego przedział z medianą
Na czerwono zaznaczono otrzymane dane
xi = średnia z przedziału
[np. (0+5)/2=2,5]
x` - średnia (16,1)
UWAGA - odchylenie standardowe podniesione do potęgi drugiej nosi nazwę wariancji
(σx)2 - wariancja
Środek przedziału klasowego
b
a
c
cecha
liczebność
b
a
c
x
ni
D
D
Me
średnia
średnia
Me
D
Me
średnia
a
b
c
Największa koncentracja
Im bardziej układ wysmukły, tym mniejsze rozproszenie
punkty przegięcia
- σx
σx
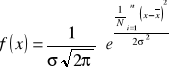
- σx
σx
- 2σx
- 3σx
3σx
2σx
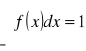
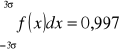
60
70
80
90
100
110
120
130
140
150
160
A
B
C
D
r > 0
r < 0
r = 0
Korelacja krzywo linowa
y
y
y
y
x
x
Korelacja ujemna
Korelacja dodatnia
x
x
y
y
x
x
y =y
x =x
Domknąć przedział.
Przeprowadzić kompleksową analizę
Przedział z Medianą
Przedział z Dominantą
Błąd oszacowania
± Sy
pokoje
osoby
1
2
3
4
5
6
7
1
2
3
4
5
6
Współczynnik korelacji podajemy z dokładnością do 4 miejsc po przecinku
Stałe ceny z okresu [Author ID5: at Mon Jan 27 14:44:00 2003 ]bazowego[Author ID5: at Mon Jan 27 14:45:00 2003 ]
Stałe ceny z okresu badanego[Author ID5: at Mon Jan 27 14:44:00 2003 ]
Wyszukiwarka
Podobne podstrony:
Podstawowe pojecia przyklady, Wielowymiarowa analiza statystyczna, Panek, wap
Przykladowe kolokwium- statystyka opisowai, Podstawy statystyki
Podstawy statystki z przykladami 15 stron , Podstawy statystyki
Podstawy Statystyki z przykładami [21 stron], Podstawy Statystyki z przykładami
Podstawy Statystyki z przykładami, Statystyka
Podstawy Statystyki z przykładami [21 stron], Statystyka opisowa
Metodologia SPSS Zastosowanie komputerów Brzezicka Rotkiewicz Podstawy statystyki
Strona 3, Podstawy Statystyki i Przedsiębiorczości
Podstawy statystyki
więcej podobnych podstron