Funkcje cyklometryczne zad1

28

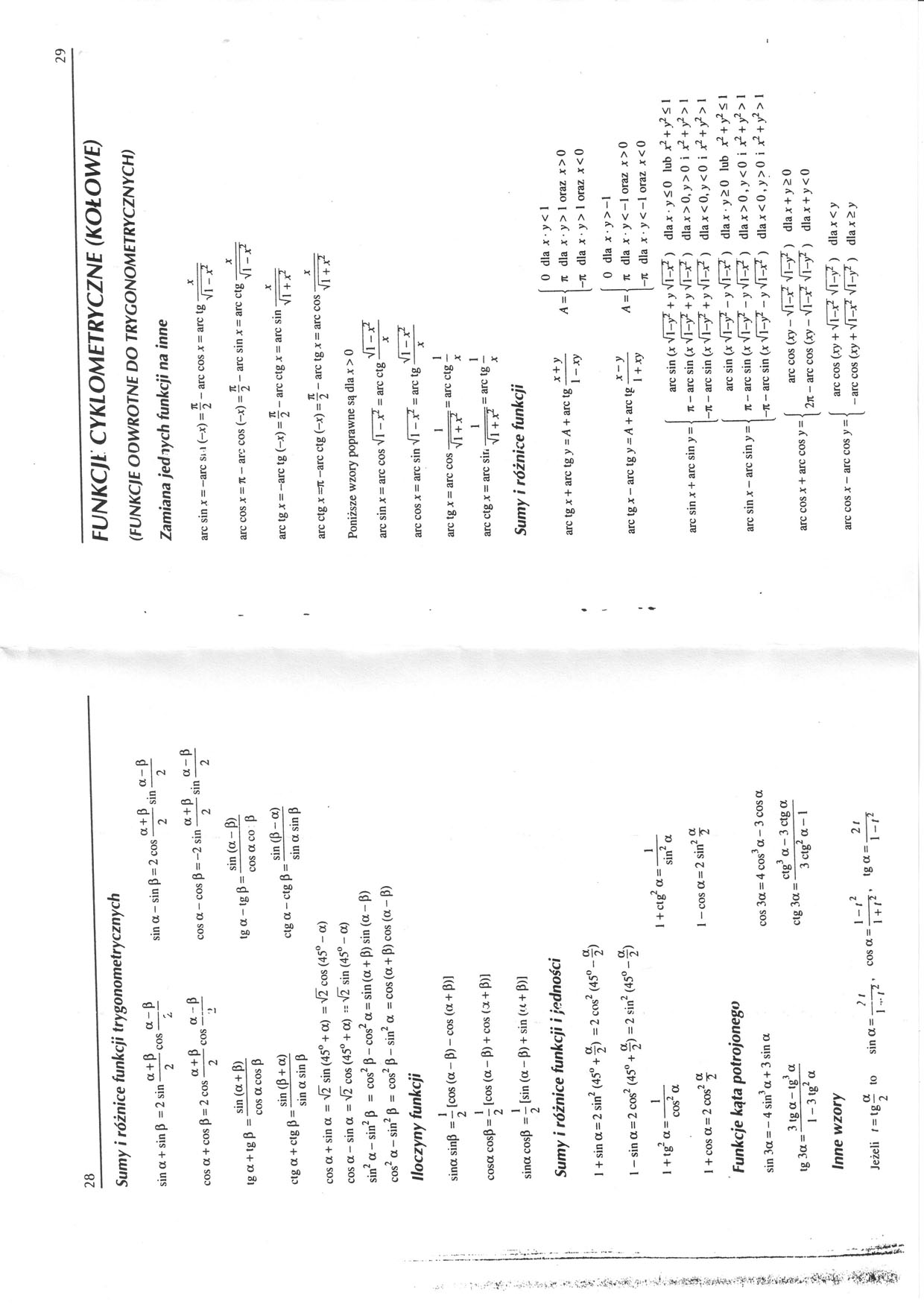
Sumy i różnice funkcji trygonometrycznych

28

„    a+P    a-p

sin a + sin P =2 sin-cos--

’    ”■>    <*

Ł    /.

a+P a-p sin a - sin p = 2 cos-sin-

cos a + cos 3 = 2 cos

a + p a-p

tg a + ig p =

2

sin (a + 3) cos a cos p

cos a - cos p = -2 sin

a+p . a-P

tg a - tg p =

sin (a - P) cos a co 3

„ sin (3 + a)

ctg a + ctg 3 = -;-—

sin a sin 3

„ sin (3 - a)

ctga-ct_gp = --—

sin a sin p

cos a + sin a = V2 sin (45° + a) = V2 cos (45° - a) cos a - sin a = Ś2 cos (45° + a) ■-'Ii sin (45° - a) sin² a - sin² p = cos² P - cos² a = sin (a + P) sin (a - P) cos² a - sin² p = cos² p - sin² a = cos (a + P) cos (a - P) Iloczyny funkcji

sina sinP = - [cos (a - 3) - cos (a + 3)]

cosa cosP = - [cos (a - 3) + cos (a + 3)]

2

sina cosP = — [sin (a - 3) + sin (a + 3)]

Sumy i różnice funkcji i jedności

I + sin a = 2 sin² (45° + f) = 2 cos² (45° -1) I - sin a = 2 cos² (45° + f) = 2 sin² (45° - f)

1 +tg-a = —— cos a

, 1

I + ctg a = —y

1 + cos a = 2 cos'

2 a

.    , .ja

I - cos a = 2 sin -j

Funkcje kąta potrojonego

sin 3a = - 4 sin³a + 3 sin a

3 tg a - tg³ a

tg 3 a =-5—

I - 3 tg² a

Inne wzory

cos 3a = 4 cos³ a - 3 cos a ctg³ a - 3 ctg a

ctg 3a = -

3 ctg² a - 1

a    2/    l-r    21

Jeżeli f = tg— to sina =--, cosa =-_y, tga = --*

⁶ 2    I-/²    1 +f²    l-/²

FUNKCJE CYKLOMETRYCZNE (KOŁOWE)

(FUNKCJE ODWROTNE DO TRYGONOMETRYCZNYCH) Zamiana jednych funkcji na inne

71    -»

arc sin * — -arc Si i (-*) =    - arc cos x = arc tg .    —

²    Vl -*²

K    X

arc cos x = Tl - arc cos (-*) = j - arc sin x = arc ctg ,, ²    Vl - jr

7t    X

arc tg x = -arc tg (~x) = -x - arc ctg x = arc sin .

²    Vl + X¹

arc ctg x =71 -arc ctg (-*) = -x - arc tg x = arc cos .    .

²    Vl + xr

Poniższe wzory poprawne są dla x > 0

fi—U    Vl — jr²

arc sin jt = arc cos v 1 - xr - arc ctg —-—

arc cos x = arc sin Vl -X¹ = arc tg

arc tg jr = arc cos

arc ctg x = arc sir.

,    - = arc ctg -

Vl + xr    x

I    I

-=== = arc tg — vl + xr    x

Sumy i różnice funkcji

arc tg x + arc tg y = A + arc tg

0 dla jc • y < I

A = i 7i dla x ■ y > 1 oraz jc > 0 |—7i dla x ■ y > I oraz Jt < 0

arc tg x - arc tg y = A + arc tg

arc sin x + arc sm y =

arc sin x - arc sin y =

arc cos x + arc cos y =

0 dla x ■ y >-l

A = Tl dla x ■ y<-l oraz x >0 —71 dla Jt • y < -1 oraz x < 0

arc sin (jr Vl-y* +y V1 —jr^ ) dlax y<0 lubj^ + y^SI Ti - arc sin (x Vl-y² + y VI-.*² ) dla * > 0, y > 0 i ot² + y² > 1 -Tl - arc sin (* V l-y² + y VT-7 ) dla * < 0, y < 0 i x* + y² > 1 arc sin (* VI —y² - y Vl-*² ) dla * • y 5 0 lub jt² + y² <, 1 Jt - arc sin (* Vl-y² - y VI-*² ) dla*>0,y<0i*²+y²>l —7t - arc sin (* VI —y² - y VI-*² ) dla*<0,y>0i*² + y²>I

arc cos (*y - VI-*³ VI —y² ) dla * + y 2 0 2n-arccos(*y-VI-*³ Vl-y² ) dla* + y<0

*-y

I +xy

arc cos (*y + Vl-*² VI—v² ) dla * < y arc cos * - arc cos y = i    .—_ .—1_

-arccos(*y + Vl-*² Vl-y² ) dla*>y