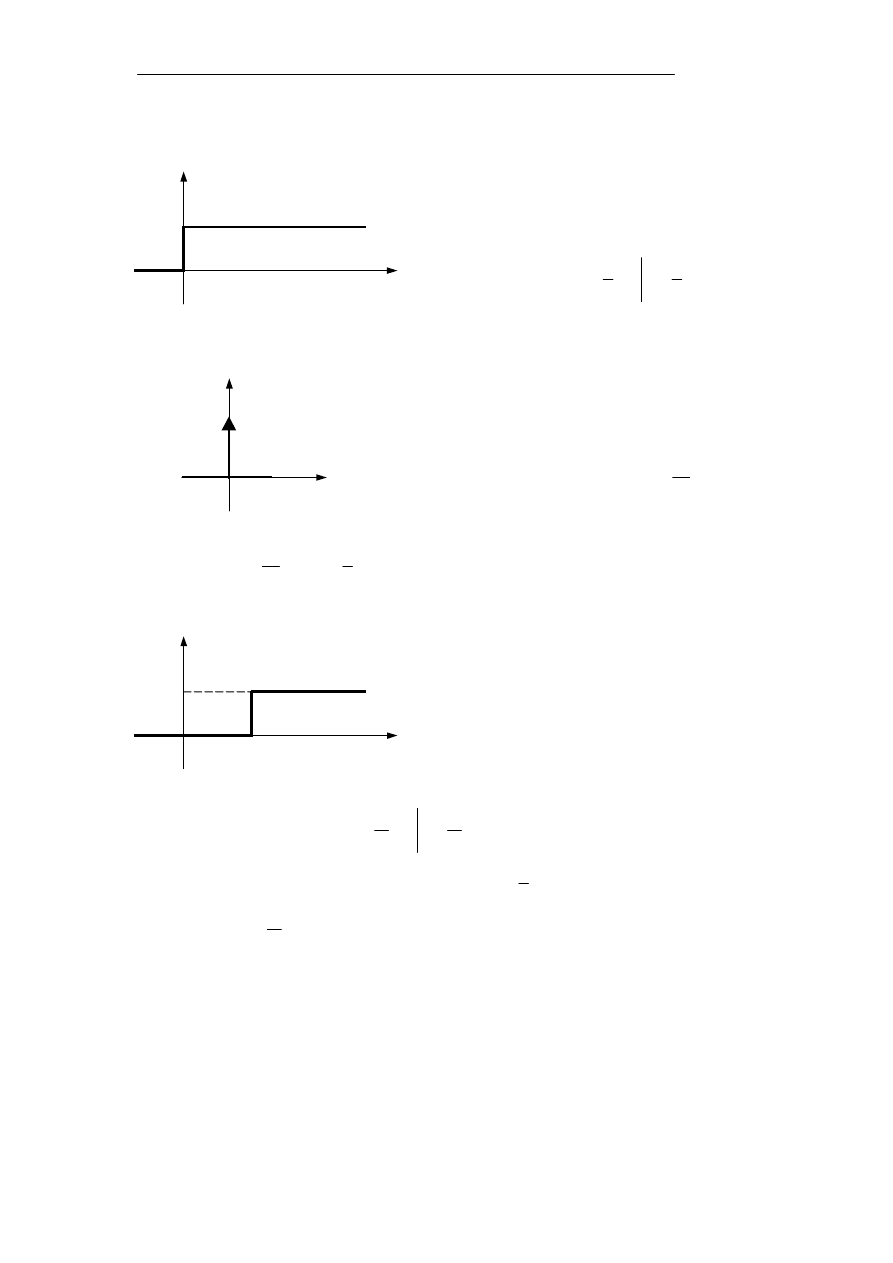
Materiały pomocnicze do ćwiczeń z Automatyki
Przykłady:
1. Znaleźć transformatę Laplace’a funkcji jednostkowej
f(t)
t
1
⎩
⎨
⎧
≥
<
=
=
0
1
0
0
)
(
1
)
(
t
t
t
t
f
Z definicji przekształcenia otrzymuje się:
( )
s
e
s
dt
e
s
F
st
st
1
1
1
0
0
=
−
=
⋅
=
∞
∞
−
−
∫
2. Znaleźć transformatę Laplace’a impulsu Diraca
)
(t
δ
f(t)
t
( )
( )
∫
∞
∞
−
=
⎩
⎨
⎧
=
∞
≠
=
1
0
0
0
dt
t
t
t
t
δ
δ
( )
( )
( ) ( )
t
t
dt
d
t
d
t
δ
τ
τ
δ
=
=
∫
∞
−
1
1
Wykorzystując twierdzenie o transformacie pochodnej można zapisać:
=
)
(s
F
L
{
}
=
)
(t
δ
L
1
1
)
(
1
=
=
⎭
⎬
⎫
⎩
⎨
⎧
s
s
t
dt
d
3. Znaleźć transformatę Laplace’a przesuniętej funkcji skokowej
f(t)
t
A
τ
⎩
⎨
⎧
<
≥
=
−
⋅
=
τ
τ
τ
t
t
A
t
A
t
f
0
)
(
1
)
(
Można wykorzystać definicję transformaty:
(
)
τ
τ
τ
τ
s
st
st
st
e
s
A
e
s
A
dt
e
A
dt
e
t
A
s
F
−
∞
∞
∞
−
−
−
=
−
=
=
−
⋅
=
∫
∫
0
1
)
(
ale także twierdzenie o opóźnieniu, wiadomo, że
L
{ }
s
t
1
)
(
1
=
, stąd
=
)
(s
F
L
{
}
τ
τ
s
e
s
A
t
A
−
=
−
⋅
)
(
1
1
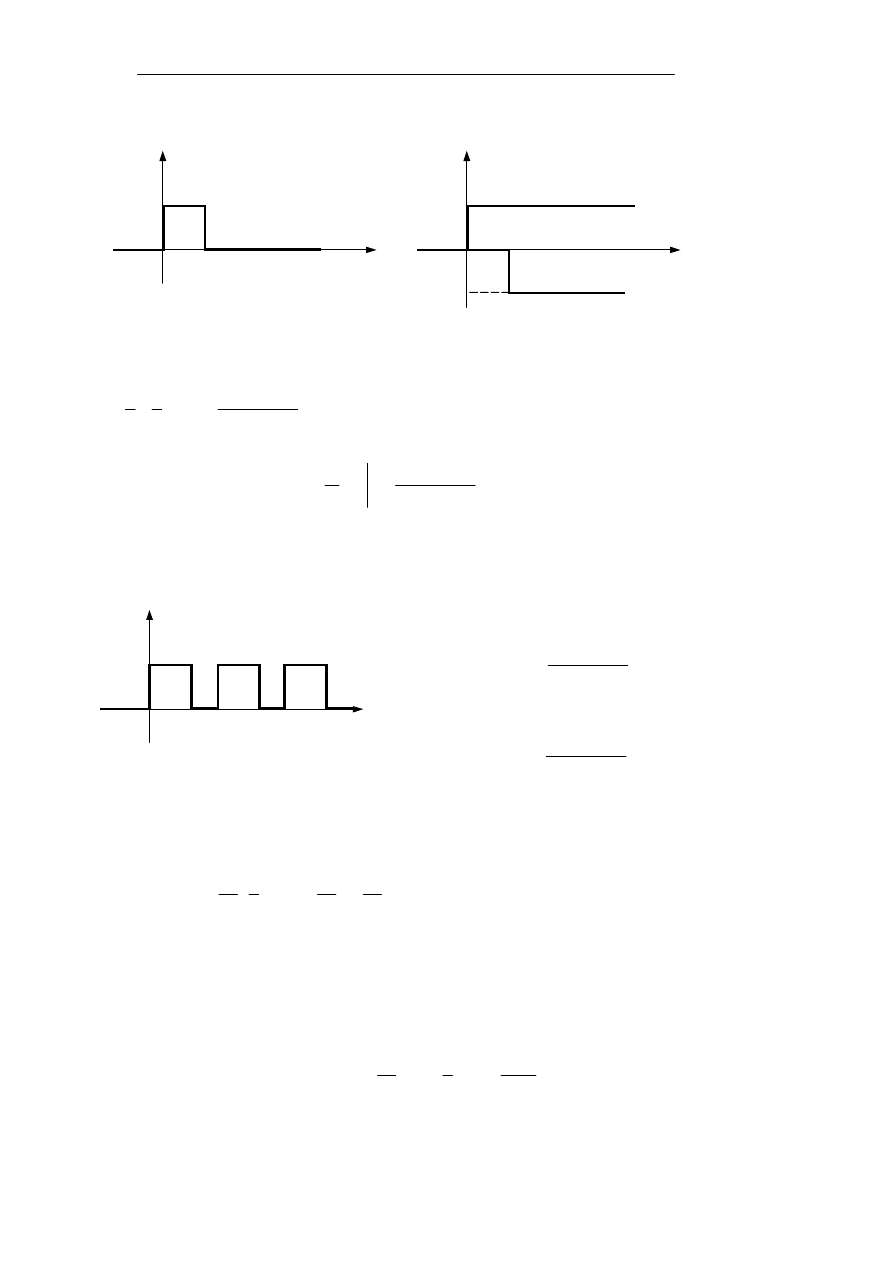
Materiały pomocnicze do ćwiczeń z Automatyki
4. Znaleźć transformatę Laplace’a impulsu prostokątnego
f(t)
t
A
τ
f(t)
t
A
τ
-A
)
(
1 t
A
⋅
)
(
1
τ
−
⋅
−
t
A
[
]
)
(
1
)
(
1
)
(
τ
−
−
=
t
t
A
t
f
=
)
(s
F
L
L
{
}
=
)
(t
f
[
]
{
}
[
)
(
1
)
(
1
A
t
t
A
=
−
−
τ
L
{
}
−
)
(
1 t
L
{
}
]
)
(
1
τ
−
t
=
s
e
A
e
s
s
A
s
s
)
1
(
1
1
τ
τ
−
−
−
=
⎥⎦
⎤
⎢⎣
⎡ −
=
Transformatę tę można także wyznaczyć korzystając z definicji przekształcenia:
s
e
A
e
s
A
dt
e
A
dt
e
t
f
s
F
s
st
st
st
)
1
(
)
(
)
(
0
0
0
τ
τ
τ
−
−
−
∞
−
−
=
−
=
=
=
∫
∫
5. Znaleźć transformatę Laplace’a ciągu impulsów prostokątnych
f(t)
t
A
τ
T
Transformata pojedynczego impulsu
prostokątnego została obliczona w przykładzie
poprzednim, wobec tego:
s
e
A
s
F
s
T
)
1
(
)
(
τ
−
−
=
;
Korzystając ze wzoru na transformatę funkcji
okresowej otrzymuje się:
( )
)
1
(
)
1
(
sT
s
e
s
e
A
s
f
−
−
−
−
=
τ
6. Obliczyć transformatę funkcji liniowej
)
(
1
)
(
t
t
t
f
⋅
=
W tym przypadku można skorzystać z twierdzenia o różniczkowaniu w dziedzinie zmiennej
zespolonej
L
{
} ( )
2
2
1
1
1
1
1
)
(
1
s
s
s
ds
d
t
t
=
⎟
⎠
⎞
⎜
⎝
⎛−
−
=
⎟
⎠
⎞
⎜
⎝
⎛
−
=
⋅
7. Znaleźć transformatę Laplace’a funkcji
)
2
(
1
)
3
2
(
)
(
−
⋅
−
=
t
t
t
f
.
Aby wyznaczyć transformatę podanej funkcji należy ją przekształcić
)
2
(
1
)
2
(
1
)
2
(
2
)
2
(
1
]
1
)
2
[(
2
)
2
(
1
)
3
2
(
)
(
−
+
−
⋅
−
=
−
⋅
+
−
=
−
⋅
−
=
t
t
t
t
t
t
t
t
f
Należy zwrócić uwagę na to, by argument funkcji, której transformatę się wyznacza był identyczny
jak argument skoku jednostkowego, stąd:
=
)
(s
F
L
{
}
s
s
s
e
s
s
e
s
e
s
t
t
t
2
2
2
2
2
2
1
2
)
2
(
1
)
2
(
1
)
2
(
2
−
−
−
+
=
+
=
−
+
−
⋅
−
2
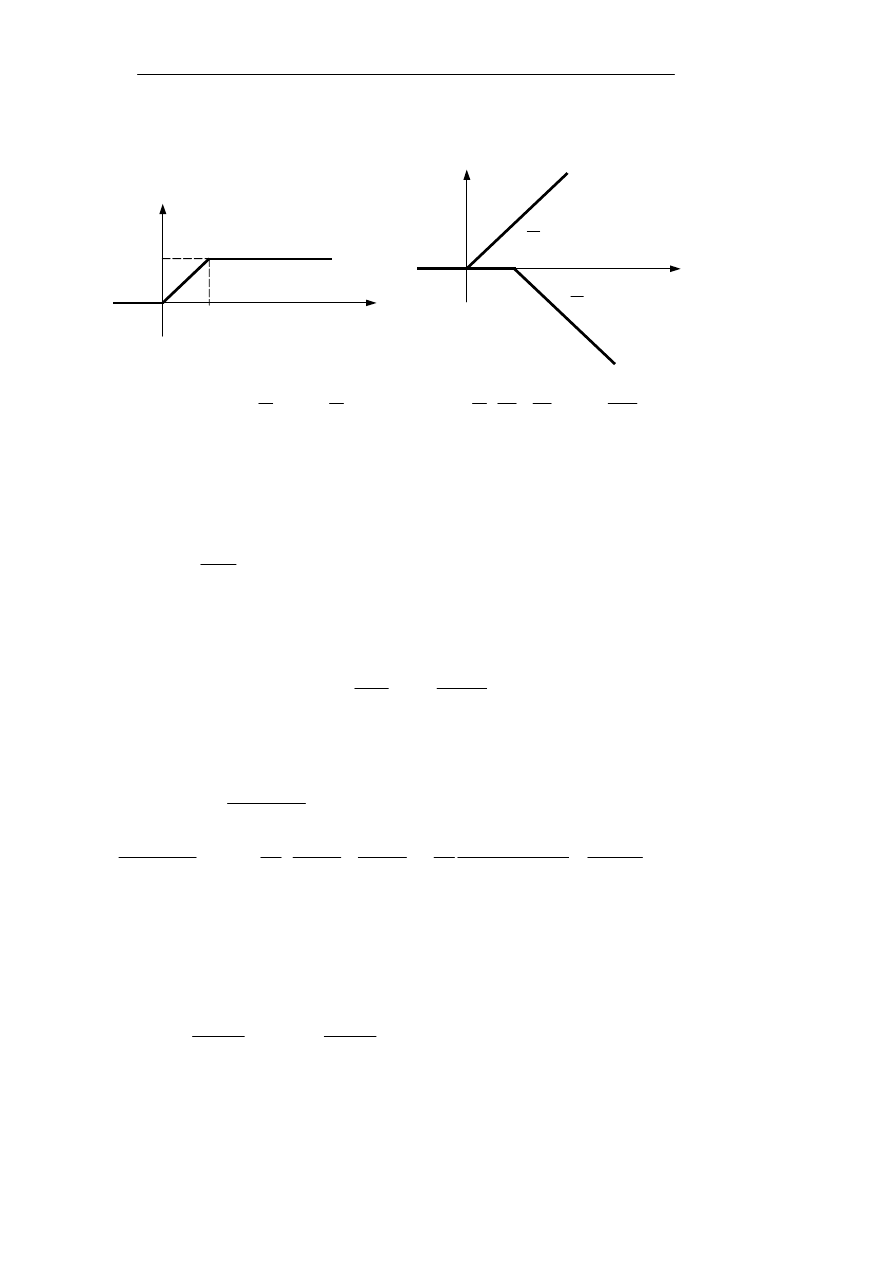
Materiały pomocnicze do ćwiczeń z Automatyki
8. Znaleźć transformatę Laplace’a funkcji, której przebieg pokazuje rysunek
Przedstawiony przebieg daje się rozłożyć na przebiegi opisane funkcjami, których transformaty
można w prosty sposób wyznaczyć:
f(t)
t
A
τ
)
(
1 t
t
A ⋅
τ
)
(
1
)
(
τ
τ
τ
−
⋅
−
−
t
t
A
f(t)
t
τ
=
)
(s
F
L
L
{
}
=
)
(t
f
)
1
(
1
1
)
(
1
)
(
)
(
1
2
2
2
τ
τ
τ
τ
τ
τ
τ
τ
s
s
e
s
A
e
s
s
A
t
t
A
t
t
A
−
−
−
=
⎟
⎠
⎞
⎜
⎝
⎛
−
=
⎭
⎬
⎫
⎩
⎨
⎧
−
⋅
−
−
⋅
;
W obliczeniach wykorzystano twierdzenie o opóźnieniu.
9. Obliczyć transformatę funkcji wykładniczej
)
(
1
)
(
t
e
t
f
at
⋅
=
−
Aby obliczyć transformatę tej funkcji możemy skorzystać z twierdzenia o przesunięciu w dziedzinie
zmiennej zespolonej
L
{
}
a
s
t
e
at
+
=
⋅
−
1
)
(
1
10. Znaleźć transformatę Laplace’a funkcji
.
)
2
(
1
)
(
)
2
3
(
−
⋅
=
−
−
t
e
t
f
t
)
2
(
1
)
2
(
1
)
2
(
1
)
(
)
2
(
3
4
4
)
2
(
3
)
2
3
(
−
⋅
⋅
=
−
⋅
=
−
⋅
=
−
−
−
−
−
−
−
−
t
e
e
t
e
t
e
t
f
t
t
t
=
)
(s
F
L
{
}
3
3
1
)
2
(
1
)
2
(
2
2
4
)
2
(
3
4
+
=
+
=
−
⋅
⋅
+
−
−
−
−
−
−
s
e
e
s
e
t
e
e
s
s
t
11. Obliczyć transformatę funkcji
)
(
1
sin
)
(
t
t
t
f
⋅
=
ω
Najprościej transformatę tę obliczyć, zapisując daną funkcję następująco:
)
(
1
2
)
(
1
sin
)
(
t
j
e
e
t
t
t
f
t
j
t
j
⋅
−
=
⋅
=
−
ω
ω
ω
L
( )
2
2
2
2
2
1
1
1
2
1
1
2
ω
ω
ω
ω
ω
ω
ω
ω
ω
+
=
+
+
−
+
=
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
+
−
−
=
⎭
⎬
⎫
⎩
⎨
⎧
⋅
−
−
s
s
j
s
j
s
j
j
s
j
s
j
t
j
e
e
t
j
t
j
12. Obliczyć transformatę Laplace’a funkcji
)
2
(
1
4
sin
)
(
−
⋅
=
t
t
t
f
.
)
2
(
1
)
2
(
4
cos
8
sin
)
2
(
1
)
2
(
4
sin
8
cos
)
2
(
1
]
8
sin
)
2
(
4
cos
8
cos
)
2
(
4
[sin
)
2
(
1
]
8
)
2
(
4
sin[
)
2
(
1
4
sin
)
(
−
⋅
−
⋅
+
−
⋅
−
⋅
=
=
−
⋅
⋅
−
+
⋅
−
=
−
⋅
+
−
=
−
⋅
=
t
t
t
t
t
t
t
t
t
t
t
t
f
=
)
(s
F
L
{
}
=
−
⋅
−
⋅
+
−
⋅
−
⋅
)
2
(
1
)
2
(
4
cos
8
sin
)
2
(
1
)
2
(
4
sin
8
cos
t
t
t
t
s
s
e
s
s
e
s
2
2
2
2
2
2
4
8
sin
4
4
8
cos
−
−
+
+
+
=
3
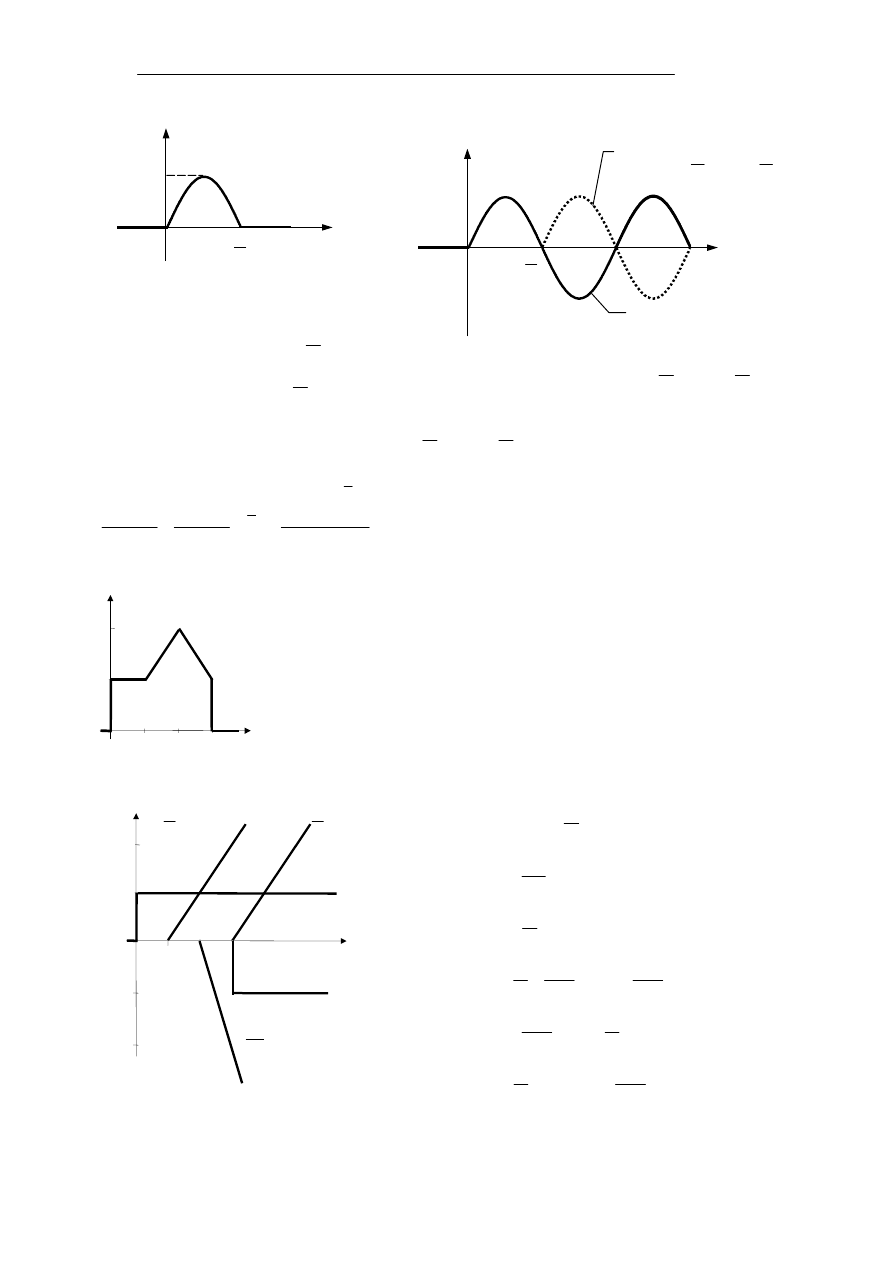
Materiały pomocnicze do ćwiczeń z Automatyki
13. Znaleźć transformatę funkcji, której przebieg przedstawia rysunek
f(t)
t
1
ω
π
⎪
⎪
⎪
⎩
⎪⎪
⎪
⎨
⎧
>
≤
≤
<
=
ω
π
ω
π
ω
t
t
t
t
t
f
0
0
sin
0
0
)
(
ω
π
⎟
⎠
⎞
⎜
⎝
⎛ −
⋅
⎥
⎦
⎤
⎢
⎣
⎡
⎟
⎠
⎞
⎜
⎝
⎛ −
ω
π
ω
π
ω
t
t
1
sin
f(t)
t
1
-1
)
(
1
sin
t
t
⋅
ω
⎟
⎠
⎞
⎜
⎝
⎛ −
⋅
⎥
⎦
⎤
⎢
⎣
⎡
⎟
⎠
⎞
⎜
⎝
⎛ −
+
⋅
=
ω
π
ω
π
ω
ω
t
t
t
t
t
f
1
sin
)
(
1
sin
)
(
=
)
(s
F
L
L
{
}
=
)
(t
f
=
⎭
⎬
⎫
⎩
⎨
⎧
⎟
⎠
⎞
⎜
⎝
⎛ −
⋅
⎥
⎦
⎤
⎢
⎣
⎡
⎟
⎠
⎞
⎜
⎝
⎛ −
+
⋅
ω
π
ω
π
ω
ω
t
t
t
t
1
sin
)
(
1
sin
2
2
2
2
2
2
1
ω
ω
ω
ω
ω
ω
ω
π
ω
π
+
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
+
=
+
+
+
=
−
−
s
e
e
s
s
s
s
;
14. Znaleźć transformatę Laplace’a funkcji, której przebieg pokazuje rysunek
2A
A
t
0
2t
0
3t
0
0
t
f(t)
Przedstawiony przebieg daje się rozłożyć na przebiegi opisane funkcjami, których transformaty
można w prosty sposób wyznaczyć:
t
0
-A
-2A
2A
A
2t
0
3t
0
0
f(t)
)
2
(
1
)
2
(
2
0
0
0
t
t
t
t
t
A
−
⋅
−
−
)
(
1
)
(
0
0
0
t
t
t
t
t
A
−
⋅
−
)
(
1 t
A
⋅
)
3
(
1
0
t
t
A
−
⋅
−
)
3
(
1
)
3
(
0
0
0
t
t
t
t
t
A
−
⋅
−
)
3
(
1
)
3
(
1
)
3
(
)
2
(
1
)
2
(
2
)
(
1
)
(
)
(
1
)
(
0
0
0
0
0
0
0
0
0
0
t
t
A
t
t
t
t
t
A
t
t
t
t
t
A
t
t
t
t
t
A
t
A
t
f
−
⋅
−
−
⋅
−
+
+
−
⋅
−
−
+
−
⋅
−
+
⋅
=
0
0
0
0
3
3
2
0
2
2
0
2
0
2
)
(
st
st
st
st
e
s
A
e
s
t
A
e
s
t
A
e
s
t
A
s
A
s
F
−
−
−
−
−
+
+
−
+
=
)
2
(
)
1
(
)
(
0
0
0
0
3
2
2
0
3
st
st
st
st
e
e
e
s
t
A
e
s
A
s
F
−
−
−
−
+
−
+
−
=
4
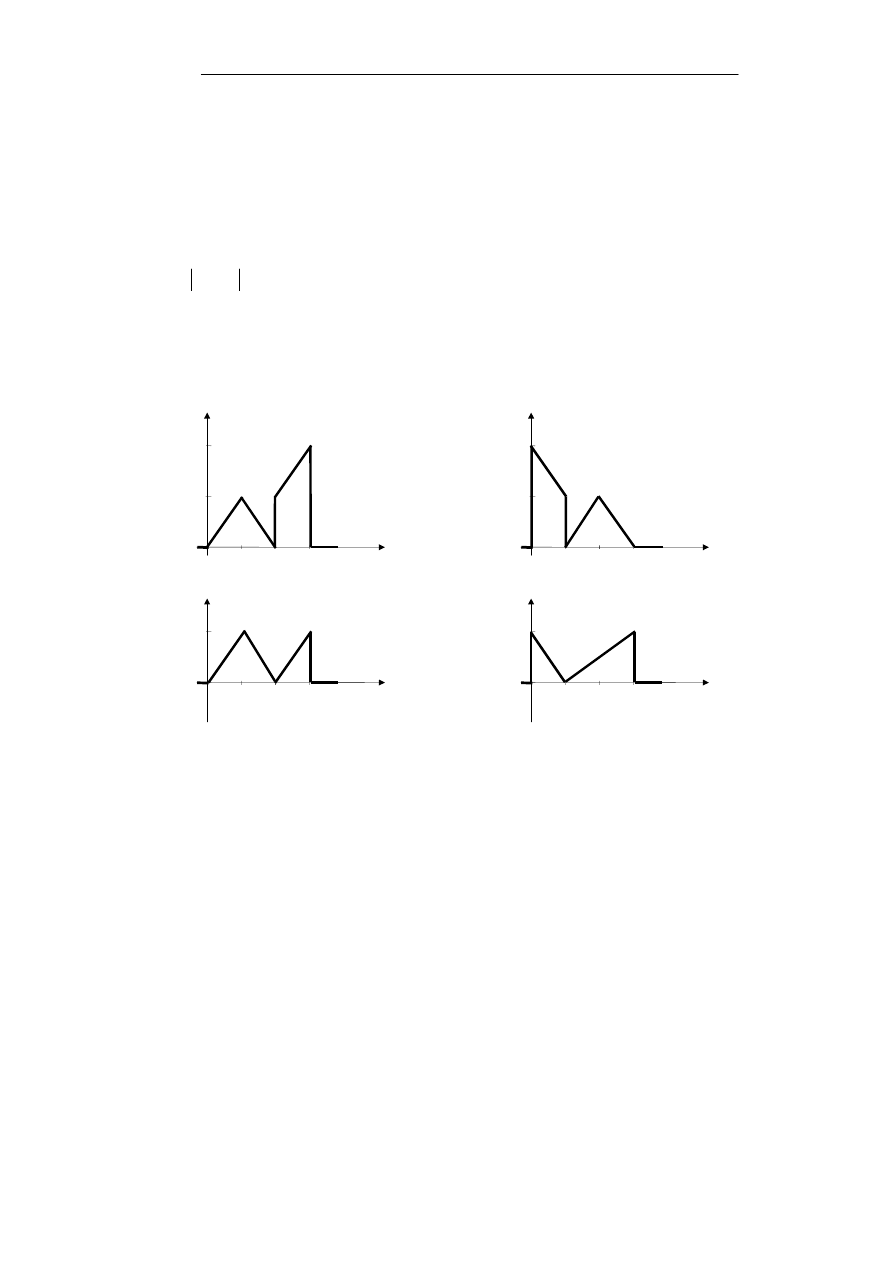
Materiały pomocnicze do ćwiczeń z Automatyki
Ćwiczenia do samodzielnego rozwiązania
1. Oblicz transformatę Laplace’a funkcji:
a.
)
(
1
)
3
2
(
)
(
t
t
t
f
⋅
−
=
b.
)
2
(
1
)
1
3
(
)
(
−
⋅
−
=
t
t
t
f
c.
)
2
(
1
)
(
)
1
(
4
−
⋅
=
−
−
t
e
t
f
t
d.
)
(
1
cos
)
(
t
t
t
f
⋅
=
ω
e.
)
(
1
sin
)
(
t
t
t
f
⋅
=
ω
f.
)
(
1
sinh
)
(
t
t
t
f
⋅
=
ω
g.
)
(
1
cosh
)
(
t
t
t
f
⋅
=
ω
h.
)
(
1
)
3
(
2
sin
)
(
t
t
t
f
⋅
−
=
2. Znaleźć transformatę Laplace’a funkcji, której przebieg pokazuje rysunek
a.
2A
A
t
0
2t
0
3t
0
0
t
f(t)
b.
2A
A
t
0
2t
0
3t
0
0
t
f(t)
c.
3
2
4
6
0
t
f(t)
d.
5
1
2
3
0
t
f(t)
5
Materiały pomocnicze do ćwiczeń z Automatyki
1. Znaleźć oryginał funkcji
a.
2
1
)
(
+
+
=
s
s
s
F
Należy zwrócić uwagę na fakt, że gdy transformata jest funkcją wymierną, to
musi być ściśle właściwą, stąd:
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
2
1
s
s
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
−
+
2
1
2
s
s
L
-1
)
(
1
)
(
2
1
1
2
t
e
t
s
t
⋅
−
=
⎭
⎬
⎫
⎩
⎨
⎧
+
−
+
−
δ
b.
2
4
1
3
)
(
+
+
=
s
s
s
F
L
=
)
(t
f
-1
4
3
2
4
1
3
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
s
s
L
-1
4
3
2
1
3
1
=
⎪
⎭
⎪
⎬
⎫
⎪
⎩
⎪
⎨
⎧
+
+
s
s
L
-1
)
(
1
8
1
)
(
4
3
2
1
6
1
1
2
t
e
t
s
t
⋅
−
=
⎪
⎭
⎪
⎬
⎫
⎪
⎩
⎪
⎨
⎧
+
−
+
−
δ
c.
4
4
1
)
(
2
+
+
=
s
s
s
F
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
4
4
1
2
s
s
L
-1
)
(
1
)
2
(
1
2
2
t
te
s
t
⋅
=
⎭
⎬
⎫
⎩
⎨
⎧
+
−
d.
13
4
1
)
(
2
+
+
=
s
s
s
F
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
13
4
1
2
s
s
L
-1
)
(
1
3
sin
3
1
3
)
2
(
3
3
1
2
2
2
t
e
t
s
t
⋅
⋅
=
⎪
⎭
⎪
⎬
⎫
⎪
⎩
⎪
⎨
⎧
+
+
⋅
−
e.
3
4
1
)
(
2
+
+
=
s
s
s
F
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
3
4
1
2
s
s
L
-1
)
(
1
sinh
1
)
2
(
1
2
2
2
t
e
t
s
t
⋅
⋅
=
⎭
⎬
⎫
⎩
⎨
⎧
−
+
−
lub
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
3
4
1
2
s
s
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
)
3
(
)
1
(
1
s
s
L
-1
=
⎪
⎭
⎪
⎬
⎫
⎪
⎩
⎪
⎨
⎧
+
−
+
+
3
2
1
1
2
1
s
s
)
(
1
2
1
2
1
3
t
e
e
t
t
⋅
⎟
⎠
⎞
⎜
⎝
⎛
−
=
−
−
6
Materiały pomocnicze do ćwiczeń z Automatyki
f.
5
2
1
3
)
(
2
2
+
+
+
+
=
s
s
s
s
s
F
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
+
+
5
2
1
3
2
2
s
s
s
s
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
−
+
5
2
4
1
2
s
s
s
L
-1
=
⎪
⎭
⎪
⎬
⎫
⎪
⎩
⎪
⎨
⎧
+
+
⋅
−
+
+
2
2
2
)
1
(
2
2
5
1
1
s
s
=
L
-1
)
(
1
2
sin
2
5
2
cos
)
(
2
)
1
(
2
2
5
2
)
1
(
1
1
2
2
2
2
t
e
t
t
t
s
s
s
t
⋅
⎟
⎠
⎞
⎜
⎝
⎛
−
+
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
−
+
+
+
+
−
δ
g.
1
2
1
3
)
(
2
2
+
+
+
+
=
s
s
s
s
s
F
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
+
+
1
2
1
3
2
2
s
s
s
s
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
2
)
1
(
1
s
s
=
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
−
+
+
2
)
1
(
1
1
1
s
s
L
-1
)
(
1
)
1
(
)
(
)
1
(
1
1
1
1
2
t
e
t
t
s
s
t
⋅
−
+
=
⎭
⎬
⎫
⎩
⎨
⎧
+
−
+
+
−
δ
h.
5
6
1
3
)
(
2
2
+
+
+
+
=
s
s
s
s
s
F
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
+
+
5
6
1
3
2
2
s
s
s
s
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
−
−
+
5
6
4
3
1
2
s
s
s
L
-1
=
⎪
⎭
⎪
⎬
⎫
⎪
⎩
⎪
⎨
⎧
−
+
⋅
+
+
−
+
2
2
2
)
3
(
2
2
5
)
3
(
3
1
s
s
=
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
−
+
+
−
+
+
−
2
2
2
2
2
)
3
(
2
2
5
2
)
3
(
3
3
1
s
s
s
)
(
1
2
sinh
2
5
2
cosh
3
)
(
3
t
e
t
t
t
t
⋅
⎟
⎠
⎞
⎜
⎝
⎛
+
−
+
=
−
δ
lub
L
=
)
(t
f
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
+
+
5
6
1
3
2
2
s
s
s
s
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
−
−
+
)
5
(
)
1
(
4
3
1
s
s
s
L
-1
=
⎭
⎬
⎫
⎩
⎨
⎧
+
+
+
+
5
1
1
2
1
s
A
s
A
Współczynniki rozkładu na ułamki proste:
4
11
1
4
3
4
1
5
4
3
5
2
1
1
−
=
+
−
−
=
−
=
+
−
−
=
−
=
−
=
s
s
s
s
A
s
s
A
=
L
-1
)
(
1
4
11
4
1
)
(
5
4
11
1
4
1
1
5
t
e
e
t
s
s
t
t
⋅
⎟
⎠
⎞
⎜
⎝
⎛
−
−
+
=
⎪
⎭
⎪
⎬
⎫
⎪
⎩
⎪
⎨
⎧
+
−
+
+
−
+
−
−
δ
7

Materiały pomocnicze do ćwiczeń z Automatyki
2. Rozwiązać równanie różniczkowe metodą przekształcenia Laplace’a.
a.
0
)
0
(
,
1
)
0
(
0
)
(
13
)
(
4
)
(
2
2
=
′
=
=
+
+
+
+
y
y
t
y
dt
t
dy
dt
t
y
d
Dokonuje
się przekształcenia Laplace’a z uwzględnieniem warunków
początkowych:
4
)
(
)
13
4
(
0
)
(
13
4
)
(
4
)
(
0
)
(
13
)
0
(
4
)
(
4
)
0
(
)
0
(
)
(
2
2
2
+
=
+
+
=
+
−
+
−
=
+
−
+
′
−
−
+
+
+
s
s
y
s
s
s
y
s
sy
s
s
y
s
s
y
y
s
sy
y
sy
s
y
s
Stąd transformata rozwiązania dana jest zależnością:
2
2
2
2
2
2
2
2
2
2
2
3
)
2
(
3
3
2
3
)
2
(
2
3
)
2
(
2
3
)
2
(
2
3
)
2
(
4
13
4
4
)
(
+
+
+
+
+
+
=
+
+
+
+
+
+
=
=
+
+
+
=
+
+
+
=
s
s
s
s
s
s
s
s
s
s
s
s
y
I ostatecznie rozwiązanie jest postaci:
)
(
1
3
sin
3
2
3
cos
)
(
2
t
t
t
e
t
y
t
⋅
⎭
⎬
⎫
⎩
⎨
⎧
⎟
⎠
⎞
⎜
⎝
⎛
+
=
−
b.
1
)
(
)
1
(
0
)
(
1
)
(
0
)
(
)
0
(
)
0
(
)
0
(
)
(
1
)
0
(
,
0
)
0
(
,
1
)
0
(
0
)
(
)
(
2
3
2
3
2
3
3
3
+
=
−
=
−
−
−
=
−
′′
−
′
−
−
=
′′
=
′
=
=
−
+
+
+
+
+
+
s
s
y
s
s
y
s
s
y
s
s
y
y
y
s
y
s
s
y
s
y
y
y
t
y
dt
t
y
d
Transformata
rozwiązania jest równa:
)
1
(
)
1
(
1
1
1
)
(
2
2
3
2
+
+
−
+
=
−
+
=
s
s
s
s
s
s
s
y
Transformatę tę można zapisać w postaci sumy dwóch ułamków:
)
1
(
)
1
(
1
)
1
(
)
1
(
)
1
(
)
(
)
1
(
1
1
)
(
2
2
2
3
2
2
1
2
3
2
1
+
+
−
+
=
+
+
−
−
+
+
+
+
=
+
+
+
+
−
=
s
s
s
s
s
s
s
s
A
s
A
s
s
A
s
s
A
s
A
s
A
s
y
Porównując współczynniki przy tych samych potęgach otrzymuje się układ
równań:
⎪
⎩
⎪
⎨
⎧
=
−
+
=
+
−
=
+
1
0
1
3
1
3
2
1
2
1
A
A
A
A
A
A
A
⎪
⎩
⎪
⎨
⎧
+
=
−
=
+
=
+
1
1
2
1
3
1
3
1
2
1
A
A
A
A
A
A
⎪
⎪
⎩
⎪⎪
⎨
⎧
=
−
=
=
+
1
3
2
1
3
1
1
2
1
A
A
A
A
A
8
Materiały pomocnicze do ćwiczeń z Automatyki
i
ostatecznie:
⎪
⎪
⎪
⎩
⎪
⎪
⎪
⎨
⎧
−
=
−
=
=
3
1
3
2
3
1
3
2
1
A
A
A
Stąd, transformata rozwiązania ma postać:
1
1
3
1
1
1
3
2
)
(
2
+
+
−
+
−
=
s
s
s
s
s
y
po
przekształceniach:
2
2
2
2
2
2
2
3
2
1
2
3
3
3
2
3
2
1
2
1
3
1
1
1
3
2
2
3
2
1
2
3
2
1
3
1
1
1
3
2
)
(
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
+
⎟
⎠
⎞
⎜
⎝
⎛ +
−
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
+
⎟
⎠
⎞
⎜
⎝
⎛ +
+
+
−
=
=
⎟⎟
⎠
⎞
⎜⎜
⎝
⎛
+
⎟
⎠
⎞
⎜
⎝
⎛ +
−
+
+
−
=
s
s
s
s
s
s
s
s
y
i ostatecznie rozwiązanie:
)
(
1
2
3
sin
3
3
2
3
cos
3
1
3
2
)
(
2
1
2
1
t
t
e
t
e
e
t
y
t
t
t
⋅
⎭
⎬
⎫
⎩
⎨
⎧
−
+
=
−
−
c.
1
)
0
(
,
1
)
0
(
)
(
1
2
)
(
4
)
(
4
)
(
2
2
−
=
′
=
⋅
=
+
+
+
+
y
y
t
t
y
dt
t
dy
dt
t
y
d
s
s
s
y
s
s
s
s
y
s
sy
s
s
y
s
s
s
y
y
s
sy
y
sy
s
y
s
2
3
)
(
)
4
4
(
2
)
(
4
4
)
(
4
1
)
(
2
)
(
4
)
0
(
4
)
(
4
)
0
(
)
0
(
)
(
2
2
2
+
+
=
+
+
=
+
−
+
+
−
=
+
−
+
′
−
−
+
+
+
Transformata
rozwiązania jest równa:
)
4
4
(
2
3
)
(
2
2
+
+
+
+
=
s
s
s
s
s
s
y
)
4
4
(
2
3
)
4
4
(
)
4
4
(
4
4
)
(
2
2
2
3
2
2
2
1
2
3
2
1
+
+
+
+
=
+
+
+
+
+
+
=
+
+
+
+
=
s
s
s
s
s
s
s
s
s
A
s
A
s
s
A
s
s
A
s
A
s
A
s
y
Porównując współczynniki przy tych samych potęgach otrzymuje się układ
równań:
stąd
⎪
⎩
⎪
⎨
⎧
=
=
+
=
+
2
4
3
4
1
1
3
1
2
1
A
A
A
A
A
1
2
1
2
1
3
2
1
=
=
=
A
A
A
9
Materiały pomocnicze do ćwiczeń z Automatyki
Stąd, transformata rozwiązania ma postać:
4
4
2
2
1
1
2
1
)
(
2
+
+
+
+
=
s
s
s
s
s
y
po
przekształceniach:
2
1
2
1
1
2
1
)
2
(
2
2
1
1
2
1
)
(
2
+
+
=
+
+
+
=
s
s
s
s
s
s
y
Rozwiązanie jest postaci:
)
(
1
)
1
(
2
1
)
(
2
t
e
t
y
t
⋅
+
=
−
Ćwiczenia do samodzielnego rozwiązania
1. Znaleźć oryginał funkcji:
a.
3
1
)
(
+
−
=
s
s
s
F
b.
1
4
1
2
)
(
+
−
=
s
s
s
F
c.
3
4
1
)
(
2
2
+
+
+
=
s
s
s
s
F
d.
9
6
1
3
)
(
2
2
+
+
+
+
=
s
s
s
s
s
F
e.
13
6
1
3
)
(
2
2
+
+
+
+
=
s
s
s
s
s
F
2. Rozwiąż równanie różniczkowe metodą odwrotnego przekształcenia Laplace’a:
a.
3
)
0
(
,
2
)
0
(
)
(
1
8
)
(
4
)
(
)
(
3
'
2
2
−
=
=
⋅
=
−
+
+
+
y
y
t
t
y
dt
t
dy
dt
t
y
d
b.
5
)
0
(
,
0
)
0
(
)
(
1
8
)
(
4
)
(
4
)
(
'
2
2
=
=
⋅
=
+
+
+
+
y
y
t
t
y
dt
t
dy
dt
t
y
d
c.
0
)
0
(
,
2
)
0
(
)
(
1
34
)
(
34
)
(
6
)
(
'
2
2
=
−
=
⋅
=
+
+
+
+
y
y
t
t
y
dt
t
dy
dt
t
y
d
10
Document Outline
Wyszukiwarka
Podobne podstrony:
Laplace 5 id 263390 Nieznany
5 Laplace id 40231 Nieznany (2)
Laplace 5 id 263390 Nieznany
Laplace theory id 263401 Nieznany
Laplace example id 263400 Nieznany
Abolicja podatkowa id 50334 Nieznany (2)
4 LIDER MENEDZER id 37733 Nieznany (2)
katechezy MB id 233498 Nieznany
metro sciaga id 296943 Nieznany
perf id 354744 Nieznany
interbase id 92028 Nieznany
Mbaku id 289860 Nieznany
Probiotyki antybiotyki id 66316 Nieznany
miedziowanie cz 2 id 113259 Nieznany
LTC1729 id 273494 Nieznany
D11B7AOver0400 id 130434 Nieznany
analiza ryzyka bio id 61320 Nieznany
więcej podobnych podstron